Skip to main content
PMC home page
PLOS ONE logoLink to PLOS ONE
. 2013 Sep 5;8(9):e73529. doi: 10.1371/journal.pone.0073529

Framingham Risk Score for Prediction of Cardiovascular Diseases: A Population-Based Study from Southern Europe

Luis M Artigao-Rodenas 1, Julio A Carbayo-Herencia 2,3, Juan A Divisón-Garrote 4, Vicente F Gil-Guillén 5,6,*, Javier Massó-Orozco 7, Marta Simarro-Rueda 8, Francisca Molina-Escribano 9, Carlos Sanchis 10, Lucinio Carrión-Valero 4, Enrique López de Coca 11, David Caldevilla 12, Juan López-Abril 1, Concepción Carratalá-Munuera 5, Adriana Lopez-Pineda 5; on behalf of the Grupo de Enfermedades Vasculares de Albacete (GEVA)
Editor: Yiqing Song13
PMCID: PMC3764050  PMID: 24039972

Abstract

Background

The question about what risk function should be used in primary prevention remains unanswered. The Framingham Study proposed a new algorithm based on three key ideas: use of the four risk factors with the most weight (cholesterol, blood pressure, diabetes and smoking), prediction of overall cardiovascular diseases and incorporating the concept of vascular age. The objective of this study was to apply this new function in a cohort of the general non Anglo-Saxon population, with a 10-year follow-up to determine its validity.

Methods

The cohort was studied in 1992-94 and again in 2004-06. The sample comprised 959 randomly-selected persons, aged 30-74 years, who were representative of the population of Albacete, Spain. At the first examination cycle, needed data for the new function were collected and at the second examination, data on all events were recorded during the follow-up period. Discrimination was studied with ROC curves. Comparisons of prediction models and reality in tertiles (Hosmer-Lemeshow) were performed, and the individual survival functions were calculated.

Results

The mean risks for women and men, respectively, were 11.3% and 19.7% and the areas under the ROC curve were 0.789 (95%CI, 0.716-0.863) and 0.780 (95%CI, 0.713-0.847) (P<0.001, both). Cardiovascular disease events occurred in the top risk tertiles. Of note were the negative predictive values in both sexes, and a good specificity in women (85.6%) and sensitivity in men (79.1%) when their risk for cardiovascular disease was high. This model overestimates the risk in older women and in middle-aged men. The cumulative probability of individual survival by tertiles was significant in both sexes (P<0.001).

Conclusions

The results support the proposal for “reclassification” of Framingham. This study, with a few exceptions, passed the test of discrimination and calibration in a random sample of the general population from southern Europe.

Introduction

Cardiovascular diseases (CVD) are widely accepted to be the most serious health care problem in both developed countries [1–3]. In addition, firm evidence supports the effectiveness of secondary prevention and there is almost unanimous agreement on its management [4–8]. However, the situation in primary care is not the same, particularly concerning the choice of methods for calculating or classifying cardiovascular risk (CVR) [9–16]. Guidelines for the prevention of CVD recommend the use of risk scores to identify adults at higher risk of CVD for whom preventive therapy has higher absolute benefits. Several scoring systems exist to help clinicians assess the 10-year CHD risk with the Framingham risk score the most widely used [17]. The Framingham-Study researchers [18] have therefore proposed a new model for use in the primary care setting, patently differentiated by gender. This “reclassification” of CVR is based on three basically sound ideas: first, use of just the four risk factors (RF) with the most weight as recognized in cardiovascular epidemiology: cholesterol, blood pressure (BP), diabetes mellitus and smoking; second, from the practical viewpoint, the physician is interested in determining the overall CVR, and the model therefore includes the prediction of all CVD events [coronary heart disease (CHD), cerebrovascular events (CVE), peripheral arterial disease (PAD) and heart failure (HF)], providing calibration factors for each entity that may be of interest to the physician; and third, inclusion of the concept of vascular age or heart age, and which can be calculated from the model.

There is reason to believe that the function, although practical, may not be applicable to the population of southern Europe or the Mediterranean, as is the population of Albacete (southeastern-Spain), where the prevalence of CHD (though not of other CVD) is lower than in northern, central and eastern Europe.

Marrugat et al. calibrated a Framingham-function, though in a population with a baseline coronary risk and one of the lowest incidences of CHD in the country. Their results suggest that this function underestimates the true risk in Spain [11]. The European alternative (SCORE) [9] is derived from a pool of various studies that are not strictly representative of the original population, and which excludes diabetic persons and morbidity, and which can only be used within the 40-65 year age group.

At the beginning of the 1990s (1992-1994), our research group studied a random stratified sample of the general population (N=1322) and calculated their risk for CHD using the classical Anderson-method [13] (as well as studying the prevalence of CVD and RF with their degrees of awareness, treatment and control) [19]. The aim of this present study was to “reclassify” this same cohort using the new proposal of D’Agostino [18] and determine their status after more than 10 years of follow-up. We would thus be able to assess, for the first time, to what extent this adapted function is valid in a strictly population-based study from southern-Europe with no Anglo-Saxon subjects.

Methods

Ethics Statement

The study was approved by the Healthcare Ethics Committee in the Albacete Health Region, in Spain. All study participants signed an informed consent document.

Setting

This cohort study involved two examinations: the first one took place between 1992 and 1994 and the second between 2004 and 2006. The participants were examined at their own primary care centers and were selected from the general population aged >18 years in the province of Albacete, Spain.

Selection and sample size

Our study sample comes from a cohort of the general population which was analyzed between 1992 and 1994 by our research team for a previous study [19–22]. This study comprised 1322 persons (69.5% response rate). The sample size of the current study is the same as the previous study, however, we selected the persons aged 30-74 years, as in the Framingham-study and other participants were excluded due to prior CVD or lack of laboratory results (N=872). The number of events of our study let us to estimate ROC curves of 0.80 or more with a standard error of 0.036 [23].

The sample was selected from the clinical records of primary care centers of Albacete by means of a two-stage sampling by conglomerates, stratified by size of population.

Measurements

At the first examination cycle, the prevalence of CVD and cardiovascular RF was evaluated. Measurements of weight, height and BP were performed by GEVA group, as well as an electrocardiogram (ECG) and a Doppler ultrasound examination in order to calculate the ankle-brachial index were done. In addition, venous blood was drawn for analysis [19,21,22,24].

The second examination cycle, 10-14 years after, included study for the presence of CVD, including CHD [clinically recognized myocardial infarction (MI), ECG evidence of previous silent MI, clinically documented angina and death due to any CVD], as well as any type of CVE and PAD in the lower limbs with effect from grade II according to the Fontaine classification. Data were collected by the two trained doctors and data sources were: interview, medical records from hospitals and primary care centers, death certificates, direct contact with doctors and, in some isolated cases, direct contact with patient’s family. When the cause of death was uncertain, the cause specified by the family physician was accepted (two cases). Data were also recorded on personal history, a family history of early CVD and major RF and personal history of these same factors. Questions were also asked about toxic habits, nutrition and physical activity. Finally, weight, height, waist circumference, sagittal abdominal diameter and BP were measured, an ECG was done and venous blood was drawn.

Measurement of the risk factors to calculate the CVR (first examination)

The BP was measured with a mercury sphygmomanometer in both arms, though we used the measurement of the left arm, like the Framingham-study, for comparative purposes. The subjects were seated and all the other conditions were as standardized. The use of antihypertensive medication was ascertained. The HDL-cholesterol was measured in the first examination by precipitation. All participants who smoked at least one cigarette per day were considered to be smokers. Diabetes was defined as fasting glucose >140 mg/dl and/or use of insulin or oral hypoglycemic medication.

Follow-up events (second examination)

All manifestations of CVD between the initial examination (1992-94) and the second examination (2004-2006) were considered as outcome events. These events were as follows:

  1. Any kind of clinically documented angina.

  2. MI with a clinical report including enzyme activity, an ECG and/or isotope and/or angiographic study, or definitive determination with baseline ECG.

  3. Stroke in the presence of permanent and objectified neurological deficit, or when neurological symptoms and signs were observed and resolved ad integrum and which the physician attributed to a transient ischemic attack.

  4. PAD in the lower limbs if participants had Fontaine grade II to IV (grade I was excluded because its symptoms may not be related to CVD).

  5. HF was only recorded if it was the cause of death.

  6. Death due to CVD was considered if the specific cause of death on the death certificate was a CVD event, except those for whom the main cause of death was discordant or could not be fully explained by a CVD event. In doubtful cases, clinical records in hospitals or primary health centers were checked, and the physicians who attended the participants at the time of death were consulted, as well as the family if necessary.

Statistical analysis

The data were analyzed using SPSS (SPSS for Windows, 15.0; SPSS Inc., Chicago,IL). The qualitative variables are expressed as the exact amount and percentage, the quantitative variables as the mean and standard deviation (SD). The association between qualitative variables was done with the chi-square test or Fisher’s test. Comparison between means was done with the Student t test for independent groups, or the Mann-Whitney U test if the conditions of normality using the Kolmogorov-Smirnoff or Shapiro Wilks tests were not met.

The individual risk was calculated with the D’Agostino-function, for men and women [18]. Its classification capacity in our population was confirmed with the measurement of the area under the ROC curve (discrimination). Calculations were then made of sensitivity, specificity, positive predictive value and negative predictive value for three different risk levels. The Hosmer–Lemeshow Χ2 statistic was used to evaluate the calibration or agreement between observed and predicted events and Mantel Haenszel test to evaluate the linear trend of the events in each of the three risk levels. All reported P values are two tailed.

Results

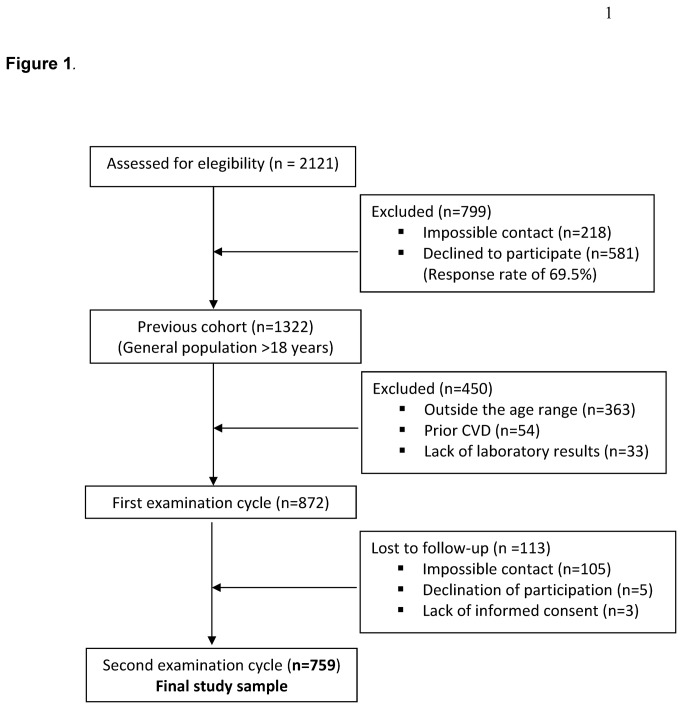
As we have mentioned, our study sample comes from the cohort of previous study, where, initially, 2121 eligible patients were identified. Of these, 1903 were able to be contacted giving a response rate of 69.5%, thus, the cohort of our previous study [19–22] (1992-1994) comprised 1322 patients. However, in the current study 363 were excluded because they were outside the age range (30–74) and 54 were excluded for prior CVD (Framingham criteria). A further 33 were excluded due to lack of laboratory results, and, of the remaining 872 subjects, 113 (12.9%) failed to attend the second examination (2004-2006) due to one of three reasons: impossible contact (93%), declination of participation (4%) or lack of informed consent (3%). Thus, the final study sample included 759 persons (55.3% women) (See Figure 1).

Figure 1. Flow of participants diagram.

Figure 1

Open in a new tab

Table 1 shows their baseline characteristics, also shows the corresponding baseline data for the participants in the Framingham-study which led to the new-model.

Table 1. Parameters used for the calculation of overall CVR and new events, according to sex, of the sample population versus the original population of the Framingham model.

Characteristics Women Albacete n = 420 Women Framingham n= 4522 (28% OFC) P Men Albacete n = 339 Men Framingham n= 3969 (22% OFC) P
Age, mean (SD) 51.6 (13.0) 49.1 (11.1) <0,001 50.6 (12.9) 48.5(10.8) <0,01
TC, mean (SD) mg/dL 203.4 (37.0) 215.1 (44.1) <0,001 210.4 (37.2) 212.5 (39.3) NS
HDLc, mean (SD) mg/dL 49.7 (12.1) 57.6 (15.3) <0,001 43.0 (11.0) 44.9 (12.2) <0,01
SBP, mean (SD) mm Hg 135.8 (23.3) 125.8 (20.0) <0,001 133.0 (19.1) 129.7 (17.6) <0,01
Treated hypertension n (%) 82 (19.5) 532 (11.8) <0,001 35 (10.3) 402 (10.1) NS
Smokers, n (%) 58 (13.8) 1548 (34.2) <0,001 155 (45.7) 1398 (35.2) <0,001
Diabetes, n (%) 47 (11.2) 170 (3.8) <0,001 26 (7.7) 258 (6.5) NS
New CV events, n (%) 31 (7.4) 456 (10.1) NS 43 (12.7) 718 (18.1) <0,05
Open in a new tab

OFC= Original Framingham cohort. SD= Standard deviation.

TC=Total cholesterol. HDLc= High-density-lipoprotein cholesterol.

SBP= Systolic blood pressure. CV= cardiovascular

New CV events: Coronary heart disease, cerebrovascular disease, peripheral arterial diseas, and heart failure. Albacete: coronary heart disease, cerebrovascular disease, peripheral arterial diseas, and lethal heart failure. Albacete excludes non-fatal heart failure

The mean follow-up of our cohort was 10.6 years (SD,2.3 years) and the mean overall risk for CVD, using the new function, was 19.7% (SD,15.7%) for men and 11.3% (SD,12.0%) for women.

Discrimination of the “reclassification-model” in the sample population

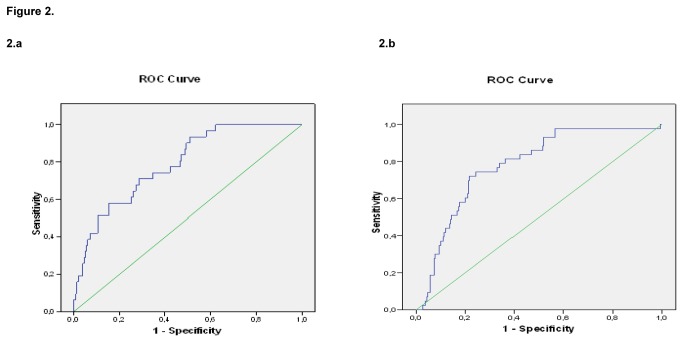
The discriminative power (correct classification of those who had or did not have events according to the predicted overall CVR) was quantified by calculating the area under the ROC curve. The area under the global ROC was 79.4% (95%CI, 74.3-84.4). Figure 2 shows the results of this calculation for women (2a) and for men (2b). As is known, this ROC curve has different cut points, depending on whether we desire more sensitivity or more specificity in the test. Thus, the cut points for overall CVR that consider 75% specificity were: for men, 26.6% (74% sensitivity) and for women, 14.2% (61.3% sensitivity). On the other hand, the cut points that consider 75% sensitivity were: 21.1% overall CVR for men (67.7% specificity) and 8.6% for women (57.4% specificity). Table 2 shows the results in more detail.

Figure 2. Area under the ROC curve for women (2.a) and men (2.b).

Figure 2

Open in a new tab

The calculated cardiovascular risk correctly classified overall 78.9% (95%CI, 71.6-86.3) of the women and 78.0% (95%CI, 71.3-84.7) of the men (P<0.001 both).

Table 2. Sensitivity, specificity and predictive values for different levels of cardiovascular risk in women and men.

CVR <10% (95% CI)
CVR 10 and <20% (95% CI)
CVR > 20% (95% CI)
Women Men Women Men Women Men
Se (%) 25.8 (10.4/41.2) 2.3 (-2.2/6.8) 22.6 (7.9/37.3) 18.6 (6.9/30.2) 51.61 (34.0/69.2) 79.1 (66.9/91.2)
Sp (%) 38.1 (33.2/42.9) 58.4 (52.8/64.0) 76.4 (72.1/80.6) 75.7 (70.8/80.6) 85.6 (82.1/89.1) 65.9 (60.5/71.3)
PPV (%) 3.2 (1.0/5.4) 0.8 (-0.8/2.4) 7.1 (2.0/12.1) 10.0 (3.4/16.6) 22.2 (12.6/31.8) 25.2 (17.9/32.5)
NPV (%) 86.5 (81.4/91.6) 80.5 (75.2/85.8) 92.5 (89.6/95.4) 86.5 (82.3/90.6) 95.7 (93.6/97.8) 95.6 (92.8/98.4)
Open in a new tab

CVR= cardiovascular risk. Se= Sensitivity. Sp= Specificity.

PPV= Positive predictive value. NPV= Negative predictive value.

Calibration. Agreement between predicted CVR and new events

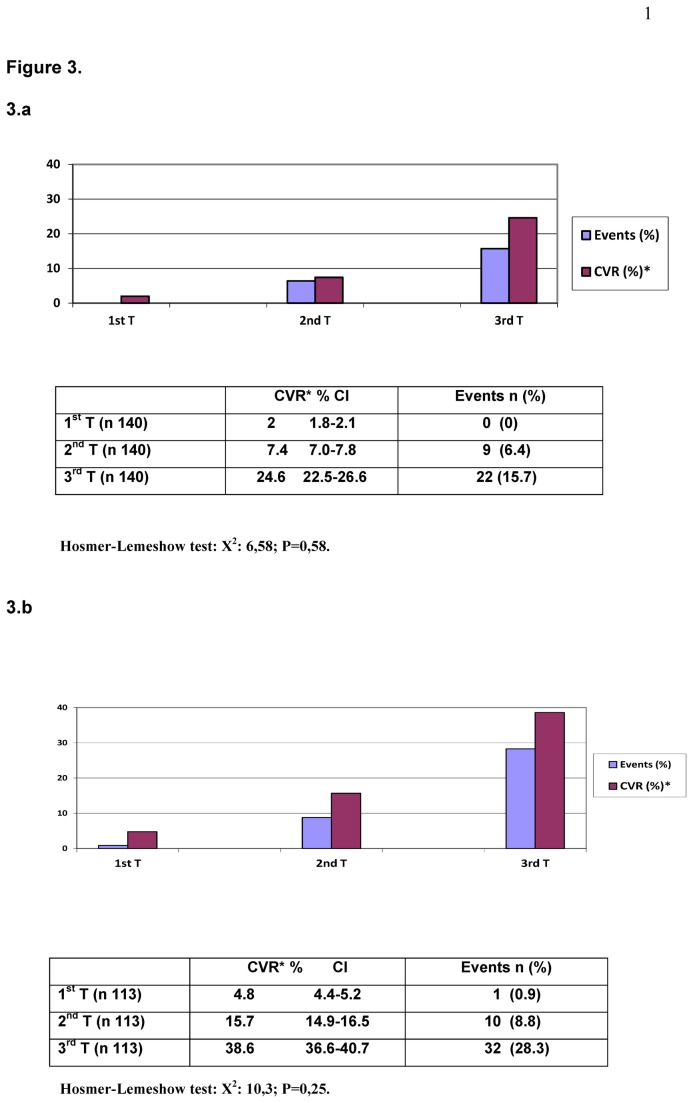
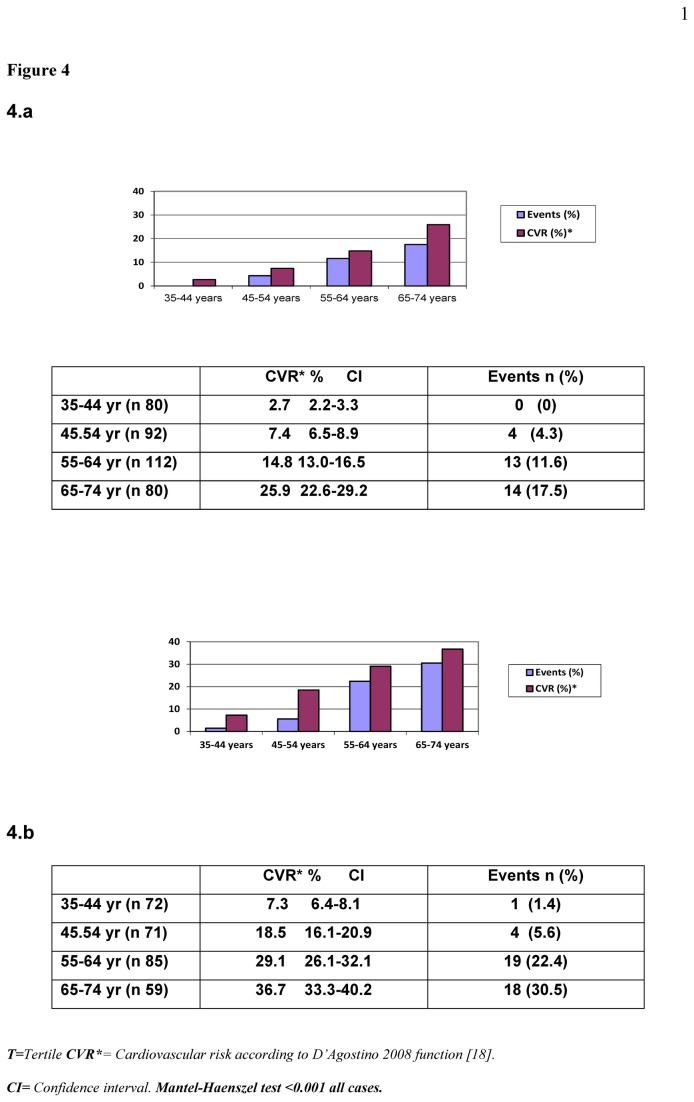
Figure 3 presents the CVR in percentage and the new events in each CVR tertile and Figure 4 shows the calculated CVR versus actual events grouped according to age and sex.

Figure 3. Calibration data in risk tertiles.

Figure 3

Open in a new tab

Mean predicted CVR (%) with the D’Agostino function versus actual events over 10.6 years of follow-up in risk tertiles (3.a women and 3.b men).

Figure 4. Calibration data by age-sex groups.

Figure 4

Open in a new tab

Mean predicted CVR (%) with the D’Agostino function versus actual events over 10.6 years of follow-up by age-sex groups (4.a women and 4.b men).

There were more events the higher the risk tertile, with a clear linear trend (Mantel-Haenszel test < 0.001), such that 71.0% of the events in women and 74.4% in men occurred in the highest risk tertile (Figure 3). The calibration between the two measurements, calculated with the Hosmer–Lemeshow test was 10.3 (P=0,25) in men and 6.58 (P=0,58) in women, indicating a significant relation.

According to age and sex, Figure 4 shows that the percentage of events was close to the lowest confidence intervals of the calculated CVR, with two exceptions: older women (65-74years) and middle-aged men (45-54years).

Discussion

Initial evaluation of the overall results suggests that applying the new “reclassification-function” cannot be recommended in our study population. The overall CVR was significantly greater in men than in women, P<0.001, whereas the events during follow-up, shown in Table 1, were 12.7% and 7.4%, respectively. In their model, D’Agostino found 18.1% and 10.1% incident events in men and women, respectively [18]. Three explanations may account for this and reduce the differences found: first, the mean follow-up periods were different, 12 years for the proposed reclassification and 10.6 years in our study, and the SD were very high in the Albacete cohort due to its small size (approximately 10% of the original-model); second, this study did not include cases of no-fatal HF as events (though they were included in the Framingham-model), which could explain an important part of the overall overestimation of the method, as there has been an increase in the incidence of HF in our area [25], and third, the levels of HDL-cholesterol were higher in the Framingham-cohort, which was not expected, though only 25% (28% in women and 22% in men–the original cohort-) of the measurements were done by precipitation, whereas 100% of our measurements were done by this method (the method available in the 1990s), and which underestimates its concentration; indeed, comparison between the old and current methods shows that the results with the new method are 10% higher, particularly when the HDL-cholesterol concentrations are low (<40 mg/dL) [26]. The CVR in our sample therefore tended to be higher.

Discrimination of the “reclassification-model” in the sample population

Despite the above, when we wish to determine the validity [27] of the risk-function and examine its discrimination we can see that the areas under the ROC curves (Figure 2) are acceptable since the area is between 0.75 and 0.90, for both men (78.0%) and women (78.9%). They are similar to those reported by Collins and Altman for an open cohort of over a million persons, with the same function (75.2% for men and 77.0% for women, and which are comparable to those of the classic-Framingham and QRISK) [28]. Equally acceptable are the cut points for taking decisions. For example, for 75% specificity, the cut point for overall CVR is 26.6% for men (74% sensitivity) and 14.2% for women, though here with a lower sensitivity (61.3%). We should recall that these apparently high figures for CVR relate to overall CVR (prediction of any CVD, including HF).

Of particular note were the negative predictive values (Table 2) which, for both sexes, were above 80% (in low risk) and 95% (in high risk). The reading of this is that those persons in our study who were “reclassified” as having a low probability of suffering an event really experienced few events, which is an added guarantee for the physicians entrusted with their care. Examination of this same table, however, does not permit us to conclude the same for the positive predictive values, which may be of concern to health care managers, as discussed below.

Calibration between predicted risk for CVD and new events

Examination of consistency is as important as that of discrimination when applying models derived from different populations to the study population [28]. The usual suggestion is to divide the study population into risk deciles, comparing predicted events with observed in each decile, or at least in each tertile. In our case we chose tertiles because of the sample size limitations. As expected, the events were found in the high-risk tertiles, in both men (32 of 43 events in the highest tertile, with 10 of the remaining 11 in the intermediate-risk tertile), and in women (22 of 31 events in the highest-risk tertile and the remaining 9 in the intermediate tertile).

In Figure 4, (4.a) highlights a relevant aspect when using any function to calculate CVR, i.e., the extraordinary weight of age. In our case, the theoretical population-based previsions (mean overall CVR calculated with the D’Agostino-function for each decade) slightly overestimated the risk, as occurred in the risk tertiles, but the percentage of actual events was still within the confidence intervals of the calculated CVR, assuming that the incidence of HF is similar in Albacete to the rest of Spain [25] (HF was not included at the second data collection but it was in the original model). This overestimate, therefore, is only relevant in older women (65-74 years) and middle-aged men (45-54 years), and may be partly explained by the greater documented intervention in the group of older women [19], and losses at the first study (significantly higher in active men, as usually occurs in cross-sectional studies). Nevertheless, Hosmer-Lemeshow statistic has shown a good calibration of Framingham function.

Limitations of the study

Our sample size does not permit us to determine whether the specific calibration factors proposed in the new model for CHD, CVE and PAD (and for HF, data for which are not available) are met in our population. Nor did we use data concerning vascular age, as it is designed for individual use.

The internal validity was hardly affected by the losses (12.9%). The external validity, on the other hand, did seem to be affected by the possible changes in the original sample from the 1990s and the losses from the first cut point [21,22].

The usual biases of historical cohorts also have to be considered, particularly those relating to determining the weight of the interventions, which can only be done in part, evaluating treatment and changes in risk level. It is reasonable to suppose that this may well have been relevant.

Future studies

Re-evaluation of this “reclassification” is needed, but replacing the analytical parameters of total cholesterol and HDL-cholesterol by the body mass index, as proposed and done by the Framingham-study researchers themselves [18]. Also necessary is the repetition of this study including HF in future cut points of the sample population. It would also be interesting to determine whether other models for evaluating CVR, particularly SCORE [9], REGICOR [11,29] and PROCAM [10], or the British models QRISK [30] and ASSIGN [31], improve the model evaluated herein. This can easily be attempted with PROCAM, which includes triglycerides and a family history of early CVD (with great weight on CVR) and which are all available for the sample. It is, however, less feasible with the other models mentioned, as the sample size in the 40-65 year age range and the fatal events are not sufficient in the case of SCORE, though it could be attempted with the British models, providing results for discussion about the controversy of whether, for low social and economic levels, the CVR is underestimated or overestimated, and vice versa in the more favored models (Matthew-effect) [32].

Finally, further information concerning the interventions and their results could be obtained by evaluating the changes in the CVR, in both older persons (with a high risk and different levels of RF) as well as in younger persons (with a low risk but certain levels of ‘treatable’ RF) [33–35].

Thus, we consider the new Framingham-proposal can be applied in our population since, in fact, the results exist despite the fact that the baseline statistics of the study cohort are completely different from those of the Framingham-cohort (Table 1). Pointing out these differences adds further value to the results that are acceptable (though not perfect), and in a practical way: cholesterol, BP, diabetes mellitus and smoking are the four most relevant RF and are sufficient for the classification of the CVR. Indeed, we believe this to be the case in populations and individuals of any origin.

In summary, although in general terms overestimation of the CVR seems to follow the usual norm for studies of populations in our area and may require calibration, as in other cases [29,30], particularly with the classic functions of Anderson (1991) [13] and Wilson (1998) [14], the statistics evaluating discrimination and consistency give more than acceptable results for the new model. Of particular note is the fact that the data for the persons reclassified as being of high risk indicate its applicability, as is the great ability of the model to detect those who will not suffer an event at all risk levels (see NPV in Table 2).

Conclusion

This study supports the idea of using the Framingham-function recommended for primary care [18]. Despite its imperfections, it surpassed the tests of discrimination and consistency in this sample from the general population of southern-Europe (southeastern-Spain).

Acknowledgments

We thank Professor Jose Ramon Banegas, School of Medicine, University Autonomous, Madrid, Spain, for his inestimable help and advice when preparing the manuscript.

The members of the Grupo de Enfermedades Vasculares de Albacete (GEVA) are: Francisco García-Gosálvez(13), Beatriz Rodríguez-Paños(12), Esperanza Martínez-Navarro(12), Rosalina Martínez-López(12), Isabel Ponce-García(9), Pilar Torres-Moreno(14), Josefina Monedero-Laorden(9), Beatriz González-Lozano(11), Lourdes Navarro-Sánchez(11), Miriam Martínez-Ramírez(11), Eva Argandoña-Palacios(12), Alfonso Campayo Serrano(15).

9. Community Medicine, Casas Ibáñez Primary Health Care Centre, Albacete, Spain.

10. Community Medicine, Primary Health Care Centre, Valencia, Spain.

11. Internal Medicine, Primary Health Care Centre, Albacete, Spain.

12. Universitary Hospitalary Complex, Albacete, Spain.

13. Emergency Department. Hospital of Hellin, Albacete, Spain.

14. Community Medicine. Primary Health Care Centre of Cuenca, Cuenca, Spain.

15. Primary Health Management Center. Albacete, Spain.

Funding Statement

This study was supported by two grants from Junta de Comunidades Castilla-La Mancha, Consejería de Sanidad, Spain (Orden 3 July 1992 del DOCM and Orden 14 September 1993 del DOCM), and funding from FISCAM Project 2003-2006 (03069-00) of Junta de Comunidades de Castilla-La Mancha, Spain (ALM, MD PhD, Principal Investigator). The funders had no role in study design, data collection and analysis, decision to publish, or preparation of the manuscript.

References

  • 1. Banegas JR, Rodríguez-Artalejo F, Graciani A, Villar F, Herruzo R (2003) Mortality attributable to cardiovascular risk factors in Spain. Eur J Clin Nutr 57 (Suppl. 1): S18-S21. doi:10.1038/sj.ejcn.1601804. PubMed: 12947446. [DOI] [PubMed] [Google Scholar]
  • 2. Menotti A, Lanti M, Puddu PE, Kromhout D (2000) Coronary heart disease incidence in northern and southern European populations: a reanalysis of the seven countries study for a European coronary risk chart. Heart. 84: 238-244. doi:10.1136/heart.84.3.238. PubMed: 10956281. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 3. Fuster V (2002) Applied Cardiological Research. Challenges for the New Millenium [Editorial] Rev Esp Cardiol 55: 327-332. doi:10.1016/S0300-8932(02)76609-8. PubMed: 11975897. [DOI] [PubMed]
  • 4. National Cholesterol Education Program (NECP) Expert Panel on Detection Evaluation and Treatment of High Blood Cholesterol in Adults (2002) Third Report of the National Cholesterol Education Program (NCEP) Expert Panel on Detection, Evaluation, and Treatment of High Blood Cholesterol in Adults (Adult Treatment Panel III) final report. Circulation. 106: 3143-3421. PubMed: 12485966. [PubMed] [Google Scholar]
  • 5. Stone NJ, Bilek S, Rosenbaum S (2005) Recent National Cholesterol Education Program Adult Treatment Panel III update: adjustments and options. Am J Cardiol 96 (Suppl.): 53E-59E. doi:10.1016/j.amjcard.2005.09.030. PubMed: 16098845. [DOI] [PubMed] [Google Scholar]
  • 6. Mancia G, De Backer G, Dominiczak A et al. (2007) Guidelines for the Management of Arterial Hypertension: The Task Force for the Management of Arterial Hypertension of the European Society of Hypertension (ESH) and of the European Society of Cardiology (ESC). J Hypertens 25: 1105–1187. doi:10.1097/HJH.0b013e3281fc975a. PubMed: 17563527. [DOI] [PubMed] [Google Scholar]
  • 7. Clark AM, Hartling L, Vandermeer B, McAlister FA (2005) Meta-analysis: secondary prevention programs for patients with coronary heart disease. Ann Intern Med 143: 659-672. doi:10.7326/0003-4819-143-9-200511010-00010. PubMed: 16263889. [DOI] [PubMed] [Google Scholar]
  • 8. McAlister FA, Lawson FM, Teo KK, Armstrong PW (2001) Randomised trials of secondary prevention programmes in coronary heart disease: systematic review. BMJ 323: 957-962. doi:10.1136/bmj.323.7319.957. PubMed: 11679383. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 9. Conroy RM, Pyörälä K, Fitzgerald AP et al. (2003) Estimation of ten-year risk of fatal cardiovascular disease in Europe: the SCORE project. Eur Heart J 24: 987-1003. doi:10.1016/S0195-668X(03)00114-3. PubMed: 12788299. [DOI] [PubMed] [Google Scholar]
  • 10. Assmann G, Cullen P, Schulte H (2002) Simple scoring scheme for calculating the risk of acute coronary events based on the 10-year follow-up of the prospective cardiovascular Münster (PROCAM) study. Circulation 105: 310-315. doi:10.1161/hc0302.102575. PubMed: 11804985. [DOI] [PubMed] [Google Scholar]
  • 11. Marrugat J, D’Agostino R, Sullivan L et al. (2003) An adaptation of the Framingham coronary heart disease risk function to European Mediterranean areas. J Epidemiol Community Health. 57: 634-638. doi:10.1136/jech.57.8.634. PubMed: 12883073. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 12. Aranceta J, Pérez Rodrigo C, Foz Sala M et al. (2004) Tables of coronary risk evaluation adapted to the Spanish population: the DORICA study. Med Clin (Barc) 123: 686-691. doi:10.1016/S0025-7753(04)75331-2. [DOI] [PubMed] [Google Scholar]
  • 13. Anderson KM, Wilson PW, Odell PM, Kannel WB (1991) An updated coronary risk profile. A statement for health professionals. Circulation 83: 356-362. doi:10.1161/01.CIR.83.1.356. PubMed: 1984895. [DOI] [PubMed] [Google Scholar]
  • 14. Wilson PW, D’Agostino RB, Levy D, Belanger AM, Silbershatz H et al. (1998) Prediction of coronary heart disease using risk factor categories. Circulation. 97: 1837-1847. doi:10.1161/01.CIR.97.18.1837. PubMed: 9603539. [DOI] [PubMed] [Google Scholar]
  • 15. Menotti A, Puddu PE, Lanti M (2000) Comparison of the Framingham risk function-based coronary chart with risk function from an Italian population study. Eur Heart J 21: 365-370. doi:10.1053/euhj.1999.1864. PubMed: 10666350. [DOI] [PubMed] [Google Scholar]
  • 16. Brindle P, Emberson J, Lampe F et al. (2003) Predictive accuracy of the Framingham coronary risk score in British men: prospective cohort study. BMJ. 327: 1267-1272. doi:10.1136/bmj.327.7426.1267. PubMed: 14644971. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 17. Rodondi N, Locatelli I, Aujesky D, Butler J, Vittinghoff E et al. (2012) Health ABC Study. Framingham risk score and alternatives for prediction of coronary heart disease in older adults. PLOS ONE. 7(3): e34287. doi:10.1371/journal.pone.0034287. PubMed: 22470551. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 18. D’Agostino RB Sr, Vasan RS, Pencina MJ et al. (2008) General cardiovascular risk profile for use in primary care: the Framingham Heart Study. Circulation. 117: 743–753. doi:10.1161/CIRCULATIONAHA.107.699579. PubMed: 18212285. [DOI] [PubMed] [Google Scholar]
  • 19. Puras A, Sanchis C, Artigao LM, Divison JA (1998) Prevalence, awareness, treatment, and control of hypertensión in a Spanish population. Eur J Epidemiol 14: 31-36. doi:10.1023/A:1007483916158. PubMed: 9517870. [DOI] [PubMed] [Google Scholar]
  • 20. Artigao M, López Abril J, Sanchis C (1991) Patología circulatoria y factores de riesgo vascular en la Zon Básica de Salud III de Albacete. In: Gómez Ruiz FT. Atención al paciente vascular en AP. Barcelona: Repro Disseny, S.L; pp. 53-65. [Google Scholar]
  • 21. Divisón JA, Sanchís C, Artigao LM et al. (1998). Prevalence of obesity and its relationship with cardiovascular risk in the population of Albacete. Aten Primaria 21: 205-12. [PubMed] [Google Scholar]
  • 22. Rodríguez Paños B, Sanchís C, García Gosálvez F et al. (2000) Prevalence of diabetes mellitus and its relationship with other risk factors in the population of Albacete. Aten Primaria 25:84-97. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 23. Hanley JA, McNeil BJ (1982) The meaning and use of the area under a receiver operating characteristic (ROC) curve. Radiology. 143(1): 29-36. PubMed: 7063747. [DOI] [PubMed] [Google Scholar]
  • 24. Carbayo JA, Divisón JA, Escribano J et al. (2007) Using ankle-brachial index to detect peripheral arterial disease: Prevalence and associated risk factors in a random population sample. Nutr Metab Cardiovasc Dis 17: 41-49. doi:10.1016/j.numecd.2005.08.009. PubMed: 17174225. [DOI] [PubMed] [Google Scholar]
  • 25. Banegas JR, Rodríguez-Artalejo F, Guallar-Castillón P (2006) Current Epidemiology of Heart Failure in Spain. Rev Esp Cardiol 6 (Suppl.): 4C-9C. doi:10.1157/13092028. [Google Scholar]
  • 26. Warnick GR, Nguyen T, Albers AA (1985) Comparison of improved precipitation methods for quantification of high-density lipoprotein cholesterol. Clin Chem 31: 217-222. PubMed: 2578337. [PubMed] [Google Scholar]
  • 27. O’Donnell CJ, Elosua R (2008) Cardiovascular Risk Factors. Insights From Framingham Heart Study. Rev Esp Cardiol 61: 299-310. doi:10.1157/13116658. PubMed: 18361904. [PubMed] [Google Scholar]
  • 28. Collins GS, Altman D (2009) An independent external validation of QRISK cardiovascular risk predictive: a prospective open cohort study. BMJ. 339: 2584-2593. doi:10.1136/bmj.b2584. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 29. Marrugat J, Subirana I, Comín E, Cabezas C, Vila J et al. (2007) Validity of an adaptation of the Framingham cardiovascular risk function: the VERIFICA Study. J Epidemiol Community Health. 61: 40-47. doi:10.1136/jech.2005.038505. PubMed: 17183014. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 30. Hippisley-Cox J, Coupland C, Vinogradova Y, Robson J, May M et al. (2007) Derivation and validation of QRISK, a new cardiovascular disease risk score for the United Kingdom: prospective open cohort study. BMJ 335: 136. doi:10.1136/bmj.39261.471806.55. PubMed: 17615182. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 31. Woodward M, Brindle P, Tunstall-Pedoe H, for the SIGN Group on Risk Estimation (2007) Adding social deprivation and family history to cardiovascular risk assessment: the ASSIGN score from the Scottish Heart Health Extended Cohort (SHHEC). Heart. 93: 172–176. PubMed: 17090561. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 32. Essink-Bot ML, Kruijshaar ME, Barendregt JJ, Bonneux LG (2007) Evidence-based guidelines, time-based health outcomes, and the Matthew effect. Eur J Public Health 17: 314–317. doi:10.1093/eurpub/ckl238. PubMed: 17060334. [DOI] [PubMed] [Google Scholar]
  • 33. Berry JD, Liu K, Folsom AR et al. (2009) Prevalence and progression of subclinical atherosclerosis in younger adults with low short-term but high lifetime estimated risk for cardiovascular disease: the Coronary Artery Risk Development in Young Adults Study and Multi-Ethnic Study of Atherosclerosis. Circulation 119: 382–389. doi:10.1161/CIRCULATIONAHA.108.800235. PubMed: 19139385. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 34. Marma AK, Lloyd-Jones DM (2009) Systematic examination of the updated Framingham Heart Study general cardiovascular risk profile. Circulation 120: 384–390. doi:10.1161/CIRCULATIONAHA.108.835470. PubMed: 19620502. [DOI] [PubMed] [Google Scholar]
  • 35. Pencina MJ, D’Agostino RB Sr, Larson MG, Massaro JM, Vasan RS (2009) Predicting the 30-year risk of cardiovascular disease: the Framingham Heart Study. Circulation 119: 3078–3084. doi:10.1161/CIRCULATIONAHA.108.816694. PubMed: 19506114. [DOI] [PMC free article] [PubMed] [Google Scholar]

Articles from PLoS ONE are provided here courtesy of PLOS

RESOURCES