Nematic phase of achiral dimers spontaneously bends and twists

Liquid crystals have been known since Reinitzer and Lehmann discovered that cholesterol melts in two steps, first becoming a cloudy liquid that becomes clear at higher temperature. By the middle of the 20th century it was found that many, mainly organic, molecules form a number of phases that are intermediate between a solid and ordinary isotropic liquid. These molecules fall into two classes. In the first class, now called “nematic” in a broader sense, the positions of the molecules are completely random, like in an ordinary liquid, but the molecules are locally all pointing in one average direction, called the “director.” This process gives rise to strong optical birefringence that, together with the ease of manipulating the director with electric fields, form the basis for the ubiquitous use of modern liquid-crystal displays. The second class of smectic and columnar phases exhibits, in addition to orientational order, partial positional ordering into planes or columns, with the molecules free to move in the planes and columns sliding past each other. Since the early 20th century just three nematic phases have been known. In PNAS, Chen et al. (1) report on experiments that confirm the existence of a fourth nematic phase with some very interesting properties.

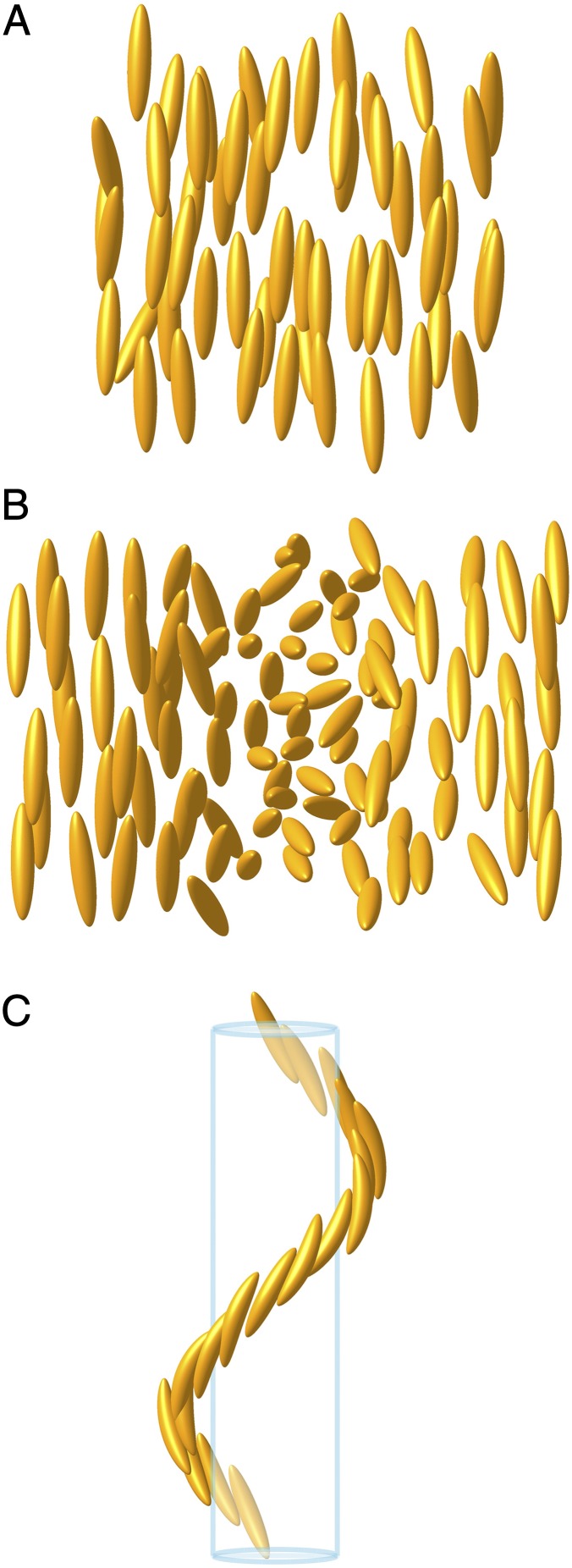
The simple nematic phase (N) is formed by the normally elongated molecules that are achiral; that is, they cannot be distinguished from their mirror image. In the lowest energy state, the director is uniform (Fig. 1A). Elongated molecules that do not poses chiral symmetry—that is, they are not equal to their mirror images—can form the chiral nematic phase (N*), also called the cholesteric phase. Locally, in regions of the order of tens of nanometers, the N* phase looks like the N phase. In the direction perpendicular to the local director, however, the director is slowly turning so that it makes a complete turn in a distance from a fraction of a micrometer to tens of micrometers. In such a twisted structure the tip of the director describes a helix with an axis perpendicular to the director. The period of the helix is called the “pitch” (Fig. 1B). Because of interference, chiral nematic liquid crystals reflect polarized light with a wavelength equal to the pitch. This aspect gives the crystals an iridescent look and is also used in a number of optical devices. Strongly chiral molecules may exhibit the so-called “blue” phase between the isotropic and N* phase. This phase is composed of cylinders in which the molecules twist in the radial direction. The cylinders form a lattice of cubic symmetry and are connected by a web of defects. The period of the lattice is such that it reflects blue or green light, so the blue phase is an example of a photonic crystal. Remarkably, in the blue phase the lattice is formed solely by the orientations of the molecules, although their positions are random and can diffuse around as in a simple fluid.

Fig. 1.

(A) Achiral nematic phase N. (B) Chiral nematic (cholesteric) phase N*. One-half pitch is shown. (C) Twist–bend nematic phase N_TB. The twist allows a homogenous spontaneous bend to fill the space.

For several decades the search has been on for new nematic phases, most notably for a biaxial nematic phase, but there is still no consensus regarding its existence. The phase confirmed by the experiments of Chen et al. (1) is quite different, but has been theoretically briefly conjectured by Meyer (2) and Dozov (3). This phase also appeared in a numerical simulation of a system of bent composites of two ellipsoids (4). In recent years there have been a number of experiments showing that dimers of nematogenic molecules connected by flexible aliphatic chains with an odd number of carbon atoms go first from the isotropic phase to the N phase, but at a lower temperature make a transition to a state with distinct new textures (5–9). Between two glass plates with surfaces treated with rubbed polyimide, giving usual nematics a homogeneously aligned director, a rope-like texture was observed with polarized microscopy. Sometimes the texture was reminiscent of smectic liquid crystals. However, X-ray scattering was consistent with nematic order, showing no Bragg reflections characteristic of smectic molecular layers (7). This texture also exhibited domains of opposite polarity (10, 11). It has been proposed (7) that the new phase is the phase conjectured by Meyer and Dozov (2, 3).

In a usual nematic phase, the state of lowest energy is obtained when the director is in the same direction everywhere. There are three possible types of deformation. In a small region around a point the director can: (i) diverge, which is splay deformation, (ii) it can turn in the direction perpendicular to itself, which is twist deformation, or (iii) it can curve in the direction parallel to itself, which is bend deformation. With the three deformations there are three corresponding elastic constants. Twist deformation can be either right- or left-handed and breaks the mirror symmetry of the N phase. The N* phase is thus a nematic phase with a spontaneous twist that is allowed by symmetry if the molecules are chiral: that is, if they do not possess a mirror symmetry.

The question posed in Meyer and Dozov (2, 3) was, what would be the result of a spontaneous bend? The tendency to spontaneously bend can be expected if the molecules themselves prefer a bent conformation, which is precisely the case for the dimers described above (12). In contrast to the twisted N* phase, however, it is not possible to fill space with homogeneously bent nematic. Meyer and Dozov (2, 3) realized that by inducing some twist, a state of constant bend is also possible. In such a twist–bend nematic phase (N_TB) the molecules form a helix (Fig. 1C). In contrast to the N* phase, the director is not perpendicular to the helix axis but forms an angle that depends on the magnitude of the spontaneous bend and the twist elastic constant. Therefore, the energy of the state is lowered by spontaneous bend but also somewhat increased by the induced twist. This frustration is likely the origin of the observed textures.

The transition to the N_TB phase has a very interesting property. The molecules are achiral, and the induced twist can be either right- or left-handed. At the transition a sample is expected to decompose into domains of opposite handedness (chirality). The N_TB phase is the only example of spontaneous chiral symmetry breaking in a fluid with no spatial order. The concept of symmetry breaking is fundamental in modern physics, the most famous example being the broken chiral symmetry of the Standard model of particle physics.

However suggestive the published evidence for the N_TB phase is, it falls short of a solid confirmation. In PNAS, Chen et al. (1) now present convincing evidence that flexible dimers indeed form the N_TB phase. The authors use two cyano-biphenyl units joined by a chain of seven carbon atoms (CB7CB). Chen et al.’s main method is freeze-fracture transmission electron microscopy (FFTEM). In this technique a thin sample between glass plates is first equilibrated at a temperature of interest and then rapidly transferred into liquid propane. The frozen structure is broken by taking apart the glass plates and made visible to electron microscopy by oblique evaporation of a 1.5-nm-thick film of platinum, creating shadows corresponding to the surface features of the broken sample. The

Chen et al. report on experiments that confirm the existence of a fourth nematic phase with some very interesting properties.

obtained images show domains with periodically spaced layers as is expected from a 3D structure of equidistant curved layers. The smallest period of around 8 nm in the image corresponds to fracture plane normal to the layers, and is equal to the layer spacing.

The observed periodic structure could also be the result of a smectic order. The FFTEM images make this unlikely, as the fracture plane tends to run perpendicularly to the layers, whereas smectic phases predominantly fracture parallel to the layers. Furthermore, in smectic phases the molecules preferentially sit in layers, giving rise to periodic electron density modulation, whereas in a nematic phase the spatial position of the molecules is completely random and the average electron density is uniform. To eliminate the possibility of smectic order, Chen et al. (1) carefully repeated X-ray scattering analysis of the phase. Ordinary X-ray scattering is sensitive only to the electron density; its periodic modulation gives rise to Bragg peaks in the scattered intensity that are a result of constructive interference of the reflections from adjacent layers. The smectic phase of the monomer octyl-cyano-biphenyl shows a very sharp and prominent Bragg peak, whereas in the N_TB phase of CB7CB nothing is observable above the noise level. This finding confirms that N_TB is indeed a nematic-type phase. By careful analysis of the FFTEM image, Chen et al. also concluded that the observed period corresponds to a full 2 π rotation of the molecules around the helix axis, so that the molecules cannot be perpendicular to the helix axis, as in the N* phase.

Further support for the structure and properties of the N_TB phase comes from the atomistic molecular dynamics simulations of CB7CB and the homolog with six carbon atoms in the linking chain, CB6CB. The system was first forced into an aligned N state and then allowed to equilibrate. CB7CB relaxed into N_TB phase with approximately the measured pitch, but CB6CB remained in the N phase. This result can be intuitively understood. The lowest energy conformation of the odd dimer is such that the biphenyl units are at an angle, favoring bend, whereas in the even dimer the biphenyl units are parallel. The constituent biphenyl units have a relatively large dipole moment along the axis, so a bent dimer has a net dipole moment perpendicular to the line joining the ends of the dimer. This dipole moment forms a helix, so the phase is also ferrielectric, like some chiral smectic phases, and analogous to helical magnetic phase. The possibility of a polar nematic phase has often been speculated upon, and the recently identified N_TB phase is probably as close as it can get. This finding means that this phase should also have interesting electrical properties.

Another interesting observation, both in experiment and in simulation, is that the pitch shows very little temperature dependence. This finding is at odds with the simple model of Dozov (3) or some recently proposed mechanisms for the formation of the N_TB phase. So questions remain. There is no satisfactory model for the N to N_TB transition, textures must be explained, and electrical properties investigated.