First-day iodine kinetics is useful for individualizing radiation safety precautions for thyroid carcinoma patients

Abstract

Objective

There is considerable variation in the national regulations of different countries for the release of patients from hospitals after radioiodine therapy. Individual variations make these practices, when based on the worst case scenarios, too restrictive for the majority of patients. However, there are cases in which strict rules are needed to comply with the dose limits to other individuals, especially children. We have developed a method to individualize radiation safety precautions.

Materials and methods

Twenty-three patients with differentiated thyroid carcinoma were included in the study. Four weeks after thyroidectomy, 1.1–3.7 GBq of radioiodine was administered and iodine kinetics were followed with external measurements until hospital discharge. The absorbed dose at the wrist holder was measured with thermoluminescence dosimetry (TLD) during hospital stay and after hospital discharge for up to 1 week. The TLD results were compared with the iodine kinetics. The dose to other individuals was estimated with extra TLDs located both on the patient’s bed and given to family members. The kinetics data were fitted in both monoexponential and biexponential models and both for the full measurement period (down to the residual activity level<400 MBq) and for the first 24 h after radioiodine administration.

Results

The biexponential model was capable of predicting the cumulated dose up to 1 week for both the longer and the shorter measured data set. The occupancy factors both for a person sleeping on the same bed and for a person living in the same apartment with the patient were in agreement with the recommended occupancy factor values of the American Thyroid Association. From these findings it is possible to individualize radiation safety precautions by taking into account the iodine pharmacokinetics and living conditions of a patient.

Conclusion

By measuring the activity content within the body for the first 24 h after radioiodine administration it is possible to individualize radiation safety precautions for thyroid carcinoma patients.

Introduction

After total or near-total thyroidectomy for papillary or follicular thyroid carcinoma, high activities of radioiodine (I-131) ranging from 1.0 to 5.5 GBq are administered to the patient. As the thyroid gland is mostly removed during thyroidectomy, the pharmacokinetics of radioiodine are in general much faster than those of radioiodine treatment for hyperthyroidism. The optimal absorbed dose (in Gy) to the thyroid tissue for ablation is not well known. In three randomized studies it was shown that 1.1 and 3.7 GBq gave similar outcomes 1–3, although there was significant variation in the calculated absorbed dose within the thyroid tissue 3. It is probable that the lack of an observed dose–response relationship is mainly due to difficulties in determining the radioiodine distribution with sufficient (even in submillimetre) resolution.

Radioiodine treatment is performed either on an outpatient basis or by hospitalization for a few days after administration of a therapeutic amount of radioiodine. The applied practice is dependent on the local regulations that are nowadays based on dose limits to other individuals. The dose limits can be converted to activity limits for release and duration of postrelease radiation precautions for a child and for adult family members and other people. This is done using either standard or individual kinetics of radioiodine. Standard kinetics generally include some conservative assumptions that are applicable both in worst case scenarios and in ‘normal’ cases. The determination of individual kinetics requires several activity or dose-rate measurements at different time points after radioiodine administration. The dose-rate measurements are usually taken only in the hospital, which means either longer hospitalization or several visits for measurements. One possibility is to perform a kinetics measurement before therapy with a smaller, diagnostic activity of radioiodine. However, according to the current knowledge this should be avoided as a stunning effect may reduce the effect of the following therapeutic dosage of radioiodine 4. To fulfil the dose limits in any circumstance, many centres apply stricter criteria based on the retained activity (400–800 MBq) as a hospital release limit because they are easy to apply in practice.

The absorbed dose to other individuals can be estimated as a product of the cumulative dose at 1-m distance, D_{1 m}, integrated from the measured or modelled dose-rate values at 1 m over the time period of interest, and an occupancy factor (OF), which combines the effect of the distance of another individual from the patient and that of the duration of contact. However, the radiation protection rules and guidelines use the concept of effective dose, which takes into account the variable absorbed dose distribution within different organs, different sensitivities of organs to long-term radiation effects, and radiation quality. In the European Community guidelines 5 the proposed dose constraints for other individuals are dependent both on the age of the person (for a child <1 mSv, for adults under 60 years <3 mSv, for adults over 60 years <15 mSv) and whether the person is pregnant (<1 mSv) or not. The accuracy of the dose estimate is dependent on both the accuracy of the dose-rate estimates as a function of time and the realistic estimation of OF 6.

In this study we have investigated the possibility of predicting radioiodine kinetics up to 1 week using the absorbed dose-rate measurements between 0 and 24 h after administration of the therapeutic dosage of radioiodine. As stated earlier, iodine kinetics for thyroid carcinoma patients are generally faster 7 than those for patients being treated for hyperthyroidism 8, as the thyroid gland of patients with cancer have been totally or almost totally removed surgically before radioiodine treatment. This makes postrelease radiation precautions moderately short in general. However, there are a few special circumstances that need to be recognized before releasing a patient from the hospital – for instance, high (>5%) iodine uptake in the thyroid bed and slow clearance of iodine from the body. The kinetics model should handle these situations with sufficient prediction accuracy. The prediction accuracy of the dose was estimated separately by the accuracy of dose-rate modelling and the distribution of OF.

Materials and methods

Calculation of absorbed dose from radioiodine therapy to other individuals

The absorbed dose, D, for other individuals was estimated using the standard method 9:

covering the time and distance relationship for the occupancy of a person in the vicinity of the patient. In principle, it is possible to calculate the cumulated dose at 1 m if there is an individual model for the kinetics of the radiopharmaceutical in order to integrate the activity content of the total body over the time of interest. Then, this cumulated activity is converted to the dose at a point of interest by multiplying it with the exposure or air-KERMA constant of the specific radionuclide. The patient self-absorption and scatter can be taken into account experimentally by normalizing the calculated dose-rate values according to the single external dose-rate measurement.

In the previous formulation OF=1 represents the case in which a person is at a 1 m distance from the patient for an infinitely long period of time. Using the general inverse square relationship of the dose rate as a function of distance from the point source, the actual OF can formally be calculated as a time average of the inverse square law

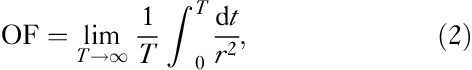where r=r(t) is the distance of a person from the patient at time t. The inverse square law is not valid at very close distances as the point source approximation cannot be applied 10. In addition, the accurate value for OF is practically impossible to calculate for normal life conditions after the patient is discharged from the hospital. Instead, some simple, preferably conservative, approaches must be used. In the recent recommendation of the ATA 9 the following OFs are proposed, which are based on the point source approximation:

1. For a person sleeping on the same bed with the patient – that is, staying at a distance of 0.3 m for 8 h (33% of the day): OF=0.33/0.3²=3.33.

2. For a person living in the same apartment with the patient but sleeping in another room – that is, staying at a minimum distance of 1 m for a maximal time of 8 h (33% of the day): OF=0.33/1²=0.33.

In this work we have studied a group of thyroid carcinoma patients treated with an ablative dose of radioiodine (described in detail in the Patients and radioiodine treatment section). We have measured the absorbed dose with two thermoluminescence dosimeters (TLDs) kept inside plastic holders strapped to the patients’ wrists for two successive measurement periods: from the time of iodine administration until the end of the patient’s stay in the hospital and after hospital discharge up to 1 week (see the TLD measurements section). During the patients’ hospital stay we measured the external dose rate with a calibrated dosimeter and modelled the dose rate as a function of time assuming the total body radioiodine content to decrease in either a monoexponential or a biexponential manner (see the Dose-rate measurements and pharmacokinetic modelling section). The capability of both exponential models to predict the total absorbed dose from a patient after hospital discharge was evaluated by comparing the modelled absorbed doses with wrist TLD measurements, normalizing the results according to the first measurement period. In addition, two or three TLDs were placed in the patient’s home to measure background dose, bed dose and dose to an adult family member during the first week after iodine administration. When these measurements were compared with calculated doses from patients, it was possible to estimate actual OFs (see the Estimation of occupancy factors section).

Patients and radioiodine treatment

Twenty-three patients with papillary or follicular thyroid carcinoma were treated 4 weeks after thyroidectomy with radioiodine activities ranging from 1.1 to 3.7 GBq. The patients were discharged from the hospital when the residual activity was less than 400 MBq. General instructions during the first week at home included sleeping in a separate bed or room and avoiding long-lasting (>3 h) close (<3 m) contact with children. The applied instructions were based on the recommendations from the Finnish Radiation and Nuclear Safety Authority, which were similar to the EU guidelines 5.

The study plan was accepted by the Surgical Ethics Committee of Helsinki and Uusimaa Hospital District. The participation of the patients in this dosimetric study did not have any effect on the radioiodine activity chosen, on the use of recombinant thyroid stimulating hormone (rhTSH), hospitalization, hospital discharge or radiation safety instructions for the patient after hospital discharge given according to the existing practice of the hospital.

TLD measurements

Four lithium fluoride (LiF) TLDs as pellets were located inside a plastic holder that was strapped to the patient’s wrist. Two such holders were used. Each patient used the first holder during the time interval between the iodine capsule administration and hospital discharge (measurement period 1=Δt₁). Thereafter, the second holder was used until the visit for total body scanning, 1 week from iodine administration (measurement period 2=Δt₂). Before the patient went home from the hospital two or three extra TLD detectors were delivered to the patient’s home. One of them was located on the patient’s bed; the second (background) detector was located elsewhere at home at a distance farther than 3 m from the patient. The third one was given to an adult family member. The bed detector had to be placed in the middle of the side not used by the patient. All the detectors were measured when returned to the hospital. The background signal was subtracted from the signals of the other detectors, and according to the appropriate calibration procedure the dose results were calculated.

Dose-rate measurements and pharmacokinetic modelling

Starting from the time of iodine administration until hospital discharge at 400 MBq residual activity, which was reached on the second or third day after iodine administration, the dose rate at 1 m from the patient was measured using the Rados RDS 110 dosimeter calibrated to Cs-137 (Rados Technology Oy, Turku, Finland). External measurements were taken from the anterior and posterior side of the patient and at the levels of the neck and stomach orthogonally from the skin surface while the patient was standing. The final dose-rate result (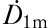) was calculated as a geometric average of the anterior and posterior measurements of each level and taking the arithmetic average from the neck and stomach level results. The measurements were taken four or five times when the patient was awake.

Two simple approaches were tested for modelling the total body content of radioiodine: a monoexponential model with a single effective decay constant (T₁) and a biexponential model with the fixed slower effective decay constant (T₂) set equal to the physical decay factor of I-131. The monoexponential model is represented by

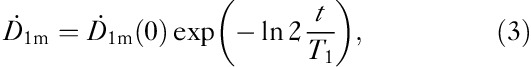where T₁ represents the effective half-life of iodine content and 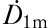the dose rate at a distance of 1 m from the patient. In the biexponential model we set the half-life in the thyroid tissue T₂ fixed:

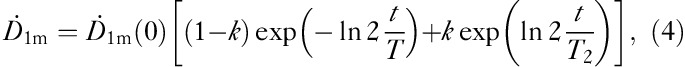where k represents the iodine proportion trapped within the thyroid tissue, 1−k is the iodine proportion for the rest of the body, T₁ is the effective half-life of iodine content in the body and T₂ is the effective half-life of iodine in the thyroid tissue. In this approach it was assumed that there is no biological clearance in the thyroid tissue and T₂ was given a fixed value – that is, the physical half-life of I-131=T_phys=8.1 days. With this approximation, the number of fitting parameters was reduced from three (k, T₁, T₂) to two (k, T_1biol) as the effective T₁ is replaced by the biological T_1biol. This is clearly seen when Eq. (4) is divided by the factor :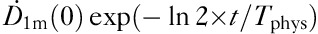

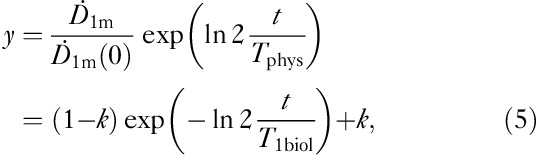which is used for fitting the observed (t,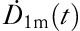) data to obtain k and T_1biol. Finally, T₁ is calculated from .

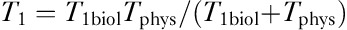Both models were tested against TLD measurements using the first wrist measurement (while the patient was in the hospital, Δt₁) for normalization and an attempt was made to forecast the values of (a) the sum of the first and second measurements – that is, wrist dose from the time of iodine administration up to 1 week, Δt₁+Δt₂ – and (b) the second measurement alone – that is, wrist dose from the time of hospital discharge up to 1 week from iodine administration, Δt₂. The values for the effective half-life T₁ and, for the biexponential model additionally, the proportion of the slower component k were calculated. The examples of data collection timeline for two patients are presented in Fig. 1.

Fig. 1.

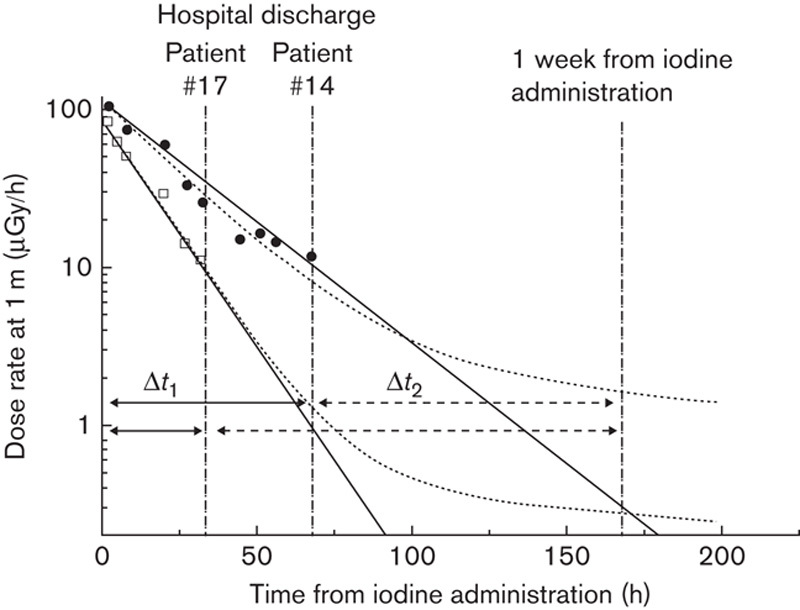
Examples of the measurement setup for patients 14 (slower kinetics) and 17 (faster kinetics) who stayed 68 and 33 h in the hospital after iodine administration. The measured dose rates at 1-m distance (in logarithmic scale) during the hospital stay are presented with solid circles (#14) and open squares (#17) with two different model fits, monoexponential (solid line) and biexponential (dotted curve). The first thermoluminescence dosimetry holder was used during the hospital stay (solid arrow, Δt₁) and the second holder was used after the hospital discharge up to 1 week from the administration of radioiodine (dashed arrow, Δt₂). The parameters of the biexponential model are #14: k=0.013, T₁=16.7 h (monoexponential: 17.1 h), and #17: k=0.006, T₁=10.4 h (monexponential: 10.5 h).

As an alternative approach to our current practice of keeping a patient in the hospital until residual activity of 400 MBq is reached, the better of the above-mentioned pharmacokinetic models was tested against the restricted data set of external dose-rate measurements during the first 24 h after iodine administration. If this approach gave a sufficiently accurate prediction of the cumulative dose for the rest of the week, there would be a possibility of evaluating the kinetics at 24 h and deciding which radiation safety instruction would be optimal for an individual patient according to the predicted pharmacokinetics and conditions at the patient’s home, in order to fulfil the absorbed dose limits for all family members.

Estimation of occupancy factors

The TLD measurements taken while the patient is in bed and the dose result of a family member living in the same apartment but not sleeping on the same bed were used to estimate the realistic OFs by substituting the TLD measurements in Eq. (1).

where D_TLD is the background-corrected measured dose in bed or of the adult family member and D_{1 m} is the calculated dose at 1 m from the kinetic model. The distribution of OF values was analysed statistically. From the analysis the average and maximum values (representing 95% of cases) of OF were calculated.

Results

The monoexponential kinetics model predicts the total wrist dose for the first week from iodine administration (measurement periods Δt₁+Δt₂; Fig. 2a and b), but the prediction accuracy of the monoexponential model is much worse than that of the biexponential model for the time period after hospital discharge up to 1 week – that is, for the measurement period Δt₂ only (Fig. 2c and d). Therefore, the preferred model is biexponential. The values of the modelled kinetics parameters are presented in Table 1. For the monoexponential model the mean effective half-life was T₁=13.0 h (range 8.1–19.7 h) and for the biexponential model the faster mean effective half-life was T₁=12.8 h (range 8.0–19.2 h) and the mean relative uptake in the thyroid tissue was k=0.010 (range 0.00–0.051). There was a slightly faster iodine clearance from the body of those patients who received rhTSH compared with patients with thyroxine withdrawal: mean T₁=12.2 h (rhTSH, n=13) versus 14.1 h (thyroxine withdrawal, n=10).

Fig. 2.

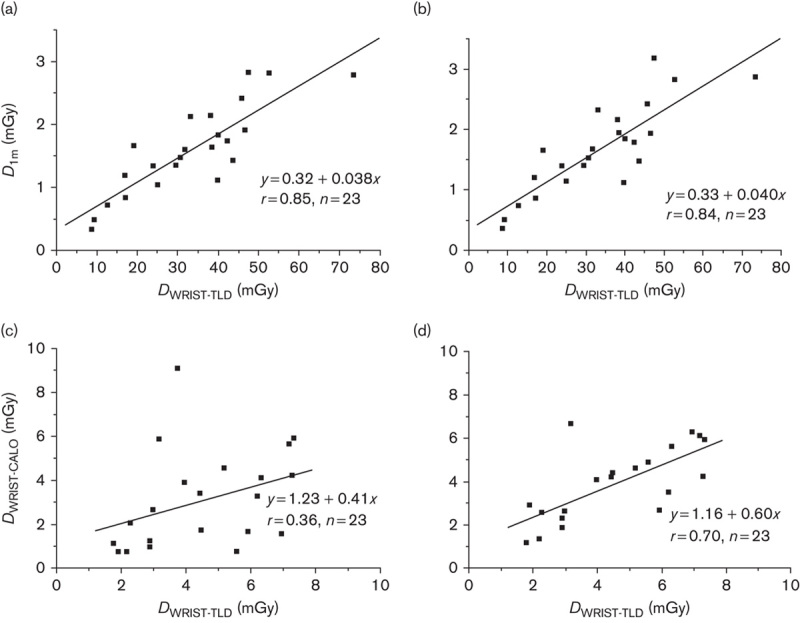
Calculated dose at 1 m as a function of the measured wrist dose (D_wrist,TLD) after iodine administration up to 1 week (a, b) and modelled wrist dose from hospital discharge up to 1 week (c, d) using either monoexponential (a, c) or biexponential (b, d) kinetics modelling.

Table 1.

Radioiodine treatments with monoexponential and biexponential modelling parameters

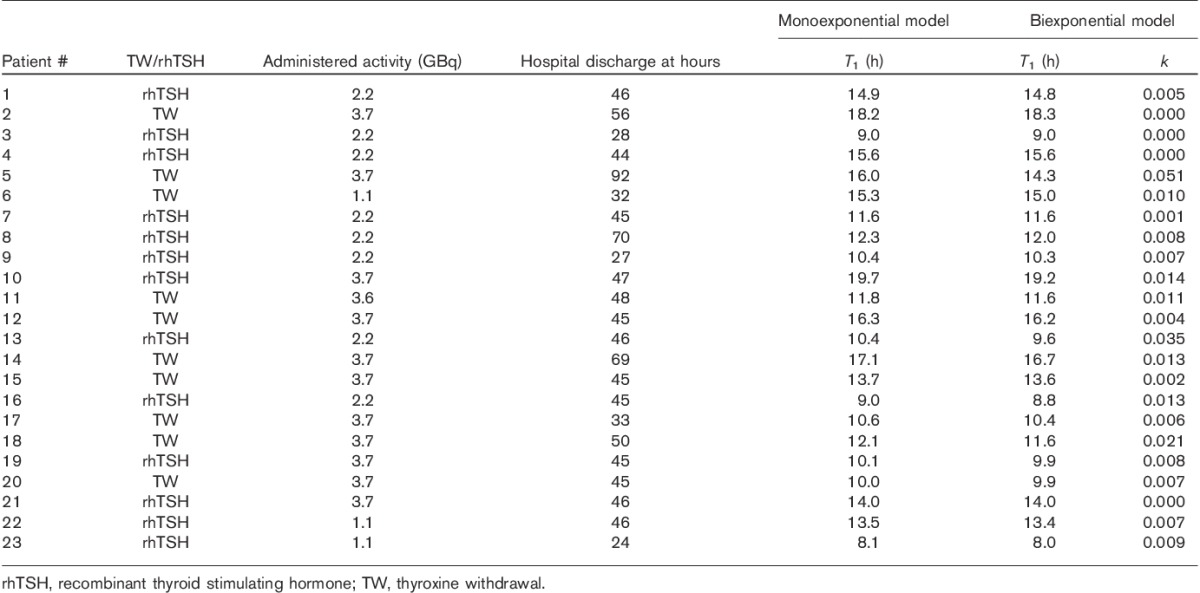
To demonstrate the validity of the biexponential model for a shorter measurement period, this comparison was repeated using the restricted kinetics data during the first 24 h after the iodine administration (Fig. 3). In this case a high prediction accuracy was also achieved. The ratio of the modelled dose at 1 m relative to the measured dose from 24 h to 1 week is 1.04±0.23 (mean±1 SD, range 0.75–1.73). On the basis of this result it seems possible that the restricted kinetics during the first 24 h would give acceptable accuracy for individual radiation safety precautions.

Fig. 3.

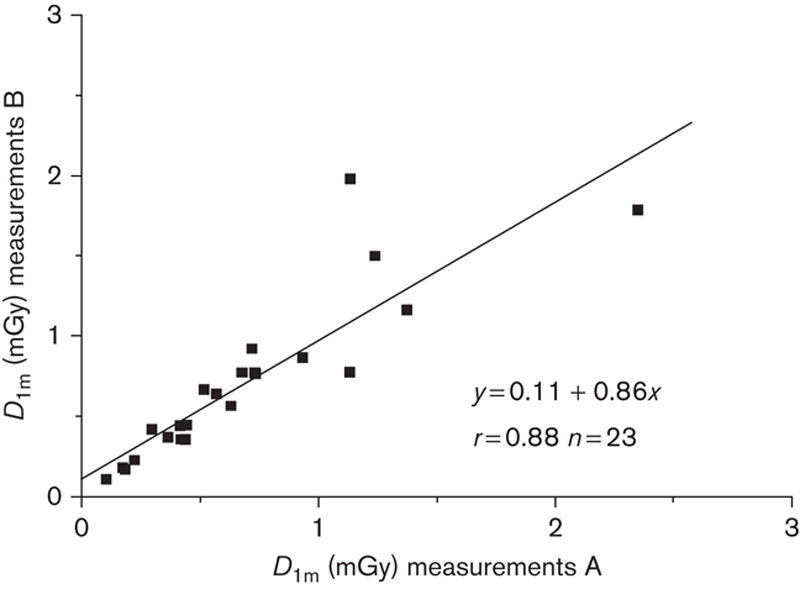
Accumulated dose after iodine administration up to 1 week at 1-m distance modelled as 0–24 h kinetics measurements B as a function of the accumulated dose from iodine administration up to 1 week at 1-m distance modelled with full series of kinetics measurements A.

Table 2 shows the data and essential statistics of TLD dosimetry and the calculated OFs at the patient’s home. In addition to the simple calculation of statistics in Table 2, we modelled the OF results with the exponential probability distribution (Fig. 4) showing the mean OF=0.83 for a person sleeping on the same bed with the patient (OF<2.5 in 95% of cases from the exponential fit and the maximum OF=2.4 from TLD measurements). The mean OF is 0.33 for a family member not sleeping on the same bed (OF<1.0 in 95 % of cases from the exponential fit and the maximum OF=0.55 from TLD measurements). The mean OFs directly calculated from the observations of Table 2 are lower than the values derived from the exponential fit. However, as the distribution of OF is not symmetric, as seen from Fig. 4, and the theoretical lower limit for OF is zero, the exponential distribution with its characteristic features obviously describes OF data better than the Gaussian distribution.

Table 2.

TLD results for different measurement periods Δt₁ (in hospital) and Δt₂ (home) with observed OFs for the bed and family member dose

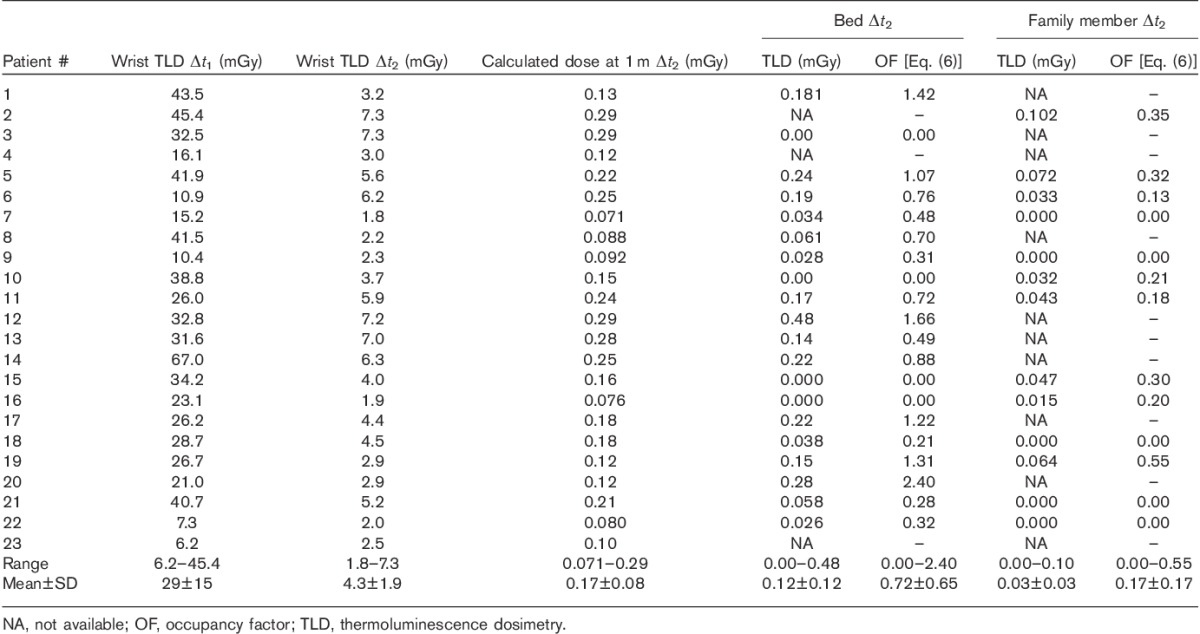
Fig. 4.

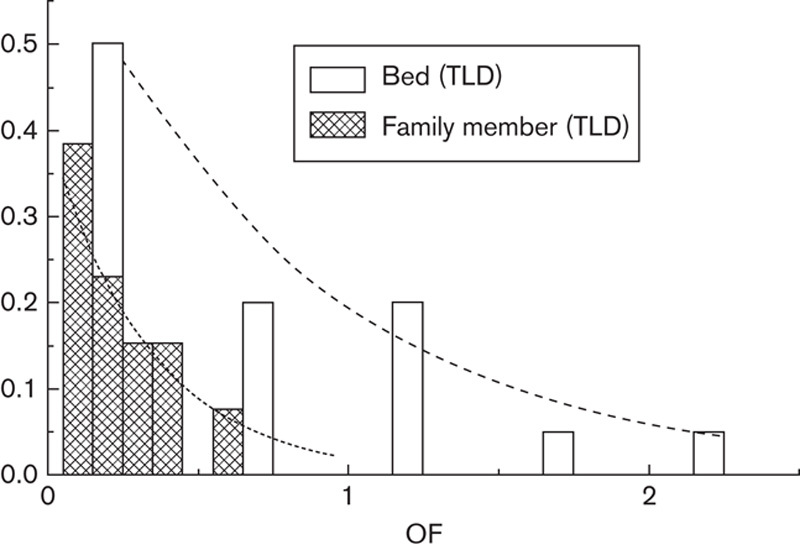
The distribution of occupancy factors (OFs) for bed thermoluminescence dosimeters (TLDs) (white columns) and for the measurements of family members (grey columns). Exponential probability density function is fitted for both bed measurements (dashed line, mean OF=0.83) and measurements of family members (dotted line, mean OF=0.33).

Discussion

The biexponential model proved to be a simple way of reaching sufficient accuracy in the prediction of dose to other individuals from a thyroid carcinoma patient discharged from hospital after radioiodine administration. We found that the dose-rate measurement within the first 24 h after iodine administration gave a sufficient basis for kinetics modelling and prediction of exposure for family members for the next 6 days. It is possible to discharge the patient soon after the administration of even high activities of radioiodine (<7.4 GBq) while adhering to ICRP dose limits 11. That practice is applied in many countries like the USA 12, Brazil 13 and India 14. However, we see some advantage in keeping the patient in the hospital for the first 24 h and letting him/her excrete the majority of radioiodine under controlled conditions. In addition, by following the kinetics of radioiodine during the first 24 h and taking the family conditions of the patient into account, it seems feasible to provide individual radiation safety precautions for the patient.

The calculated distribution of the OFs from our TLD measurements supported the general recommendations published by the ATA 9. There is a wide range of observed OF values because of the steep gradient of the dose rate around the patient, inaccurate and untraceable time–distance conditions of the TLDs from the patient and the uncertainty of the very low dose TLD measurements. Without any elaborate theoretical reasoning we modelled the OF distribution using the exponential probability model with a moderate visual agreement between the distribution model chosen and observed results. It is possible to calculate the maximum limit of OF for any percentage (e.g. 95%) of the cases, for instance, for modelling the exposure for the group of people. However, our OF results are based on a limited number of measurements and should be confirmed in another study.

Our model is based on a point source approximation and the measured or modelled absorbed dose concept is used as a surrogate for the effective dose. The point source approach is conservative because it overestimates the dose by not taking attenuation within the patient into account. In addition, the point source estimates the effective dose in the whole body assigning the single determined absorbed dose value on the person’s surface. Sparks et al. 15 have used the Monte Carlo method with an anthropomorphic geometry to model the effective dose more accurately. They found the point source approximation to overestimate the effective dose by a factor of 2.6 from the absorbed dose. As a practical approach for calculating the effective dose based on two external measurements from different sides of the body (higher dose value: Hi, lower dose value: Lo), NRC has presented a weighted formula for their concept ‘effective dose equivalent’=¾Hi+¼Lo 16.

In general, the measured absorbed doses received by the family members and recorded on the bed dosimeters were very low and far below the ICRP limits. This has been noticed in many other studies 17,18, even when poor compliance of patients and family members with radiation protection guidelines has been noticed 19. In some countries family members are even asked to take care of the patient soon after radioiodine administration, which can be done within acceptable observed exposure levels 13. From this point of view it may seem too complicated to create individual radiation safety precautions for each patient, and, indeed, the vast majority (80–90%) of the patients in our study could have been fitted to the average kinetics of k<0.02 (iodine uptake in thyroid tissue) and T_1/2<14 h. However, there were five out of 23 patients with k>0.03 or T_1/2>16 h without any risk factors of higher thyroid tissue uptake or slower kinetics. Our method can be valuable in identifying these patients from the majority of standard patients.

As a conclusion, the measurement of kinetics during the first 24 h after administration of a therapeutic amount of radioiodine is sufficient for predicting radiation exposure from the patient to other individuals up to 1 week from administration. The preferred kinetics model is biexponential with two fitting variables: effective half-life (T₁) for iodine not trapped within the thyroid tissue and the proportion of iodine trapped within the thyroid tissue (k). The model can be useful for individualizing radiation safety precautions for patients with different pharmacokinetics and living conditions.