RadRAT: A Radiation Risk Assessment Tool for Lifetime Cancer Risk Projection

Abstract

Risk projection methods allow for timely assessment of the potential magnitude of radiation-related cancer risks following low-dose radiation exposures. To estimate such risks directly through observational studies would generally require infeasibly large studies and long-term follow-up to achieve reasonable statistical power. We developed an online radiation risk assessment tool (RadRAT) which can be used to estimate the lifetime risk of radiation-related cancer with uncertainty intervals following a user-specified exposure history (https://irep.nci.nih.gov/radrat). The uncertainty intervals are a key component of the program because of the various assumptions that are involved in such calculations. The risk models used in RadRAT are broadly based on those developed by the BEIR VII committee for estimating lifetime risk following low-dose radiation exposure to the U.S. population for eleven site-specific cancers. We developed new risk models for seven additional cancer sites: oral, esophagus, gallbladder, pancreas, rectum, kidney and brain/central nervous system (CNS) cancers using data from the Japanese atomic bomb survivors. The lifetime risk estimates are slightly higher for RadRAT than for BEIR VII across all exposure ages mostly because the weighting of the excess relative risk and excess absolute risk models was conducted on an arithmetic rather than a logarithmic scale. The calculator can be used to estimate lifetime cancer risk from both uniform and non-uniform doses that are acute or chronic. It is most appropriate for low-LET radiation doses <1Gy, and for individuals with life-expectancy and cancer rates similar to the general population in the U.S.

Introduction

Risk projection methods allow for timely assessment of the potential magnitude of radiation-related cancer risks following low-dose radiation exposures. To estimate such risks directly through observational studies would generally require infeasibly large studies and long-term follow-up to achieve reasonable statistical power [1]. Risk projections methods have been used to assess the potential radiation-related cancer risk for a variety of exposures, including current levels of CT scan use in the U.S. [2], fallout from nuclear weapons testing [3] and residential radon exposure [4]. Nevertheless, these calculations can be complex when multiple organ exposures are considered. We developed an interactive online computer program (RadRAT available at https://irep.nci.nih.gov/radrat), which can be used to estimate the lifetime risk for radiation-related cancer and its uncertainty distribution following a user-specified exposure history. The uncertainty intervals are a key component of the program because of the various assumptions that are involved in such calculations.

The risk models used by the risk calculator are broadly based on those developed by the BEIR VII committee for estimating lifetime risk following low-dose radiation exposure to the U.S. population for eleven site-specific cancers: stomach, colon, liver, lung, breast, uterus, ovary, prostate, bladder, thyroid and leukemia [5]. We developed new risk models for seven additional cancer sites: oral, esophagus, gallbladder, pancreas, rectum, kidney and brain/central nervous system (CNS) cancers increasing the total number of site-specific cancer sites to eighteen.

In this paper we describe the models and methods employed in the risk calculator, focusing on the development of the new risk models and the modifications made to the BEIR VII methodology. We then present summary risk estimates for a variety of standard exposure scenarios.

Estimation of Lifetime Risk

After radiation exposure, the risk of radiation-related cancer has been shown to remain elevated for at least fifty years, effectively for the remainder of a person’s lifetime [6]. Therefore, a standard summary statistic for capturing the total potential detriment of an exposure is the cumulative excess lifetime risk; calculated as the sum of the age-specific risks adjusted for the probability of surviving to that age (i.e., survival function). A number of approaches have been developed for these type of calculations but in RadRAT, as in BEIR VII, the approach taken is the lifetime attributable risk [5,7]. The lifetime attributable risk is calculated using the survival function for a population unexposed to radiation, and is a close approximation to the more general risk of exposure-induced cancer (REIC) which is calculated using a survival function that accounts for deaths due to the same exposure to radiation for which risk is estimated [8]. Since the probability of death from developing more than one radiation-related cancer from a given exposure is extremely small at doses < 1 Gy, the lifetime attributable risk and REIC are virtually identical for most doses of interest considered in RadRAT.

The radiation risk calculator estimates lifetime attributable risk from the time of exposure until the end of expected lifetime. In addition, the calculator provides estimates of future risk defined as risk from present time until the end of expected lifetime. Future risks are given as risk attributable to radiation (i.e., the excess risk), the baseline risk (i.e., risk in the absence of exposure to radiation) and total risk (i.e., excess plus baseline).

The risk calculator can calculate risk from single or multiple exposures, and reports the risk from each individual exposure as well as the total risk summed across all exposures. Risks can be calculated for each cancer type. A total risk is obtained as a summation of risks across all cancer types. A complex exposure history may include multiple exposures, with each exposure including uniform, whole-body doses or non-uniform doses received by multiple organs or tissues. The number and type of organs or tissues exposed may vary from exposure to exposure. Multiple total and grand total risks are reported across exposures, across cancer types and by summing the risk from radiation with the baseline risk.

Radiation Risk Models

Our approach is based primarily on the methods used in BEIR VII [5], albeit with a number of small modifications. The differences are described below and summarized in Table A-1. The BEIR VII committee developed risk models for eleven cancer types: stomach, colon, liver, lung, breast, prostate, uterus, ovary, bladder, thyroid and leukemia. For most cancers the BEIR VII committee developed both Excess Relative Risk (ERR) models for the relative change in rates and Excess Absolute Risk (EAR) models for the absolute difference in rates for exposed compared to unexposed individuals. For solid cancers the risk was assumed to have a linear relationship with dose that depends on sex, attained age and age at exposure. The standard model for both the ERR and EAR, used for solid cancers other than breast and thyroid, has the form β_S D exp [γ e*] (a*)^η where β_S is the site-specific risk coefficient (for males or females), D is the dose in Gy, γ is the age at exposure parameter, e* is (e-30)/10 for e <30 and zero for e ≥ 30, where e is age at exposure in years, η is the attained age parameter and a*=(a/60) where a is attained age in years. The parameters were estimated using cancer incidence data from 1958-1998 from the Life Span study (LSS) of the Japanese atomic bomb survivors and DS02 dosimetry [6]. For most cancer sites this dataset offers many advantages including its large size, high quality cancer incidence data, wide range of doses and inclusion of all ages and both sexes. The only exceptions are breast and thyroid cancer; for which detailed pooled analyses are available based on data from the LSS and medically exposed populations. The risk of thyroid cancer is based on the ERR model derived from the pooled data of seven studies described by Ron et al [9], as analyzed by Land et al [10] and modified for gender-dependency by the BEIR VII committee [5]. The ERR thyroid model is similar in form to the standard model for solid cancers, but depends only on age at exposure and not on attained age (i.e., η is equal to zero). The preferred model for breast cancer is the EAR model from the pooled analysis of four cohorts by Preston et al [11]. That model has the same form as the standard model for solid cancers, but e* is (e-25)/10 for all ages e at exposures, and a* is (a/50). For leukemia, models are based on the LSS data for the period 1950-2000 and exclude chronic lymphocytic leukemia (CLL). The ERR or EAR BEIR VII models are linear-quadratic in dose and depend on sex, age at exposure (e) and time since exposure (t). In RadRAT, the linear-quadratic model is applied for acute exposures, but only the linear term is applied for chronic exposure (i.e., the quadratic term is dropped.) The parameter values for the BEIR VII risk models are provided in Table 1.

Table 1.

Preferred ERR and EAR models (per Sv) for estimating site-specific solid cancer incidence. Estimated parameters with 95% confidence intervals (CI)

Cancer site	ERR modela				EAR modela
	β males	β females	γ	η	β males	β females	γ	η
Oral Cavity	0.23 (<0-0.66)	0.53 (0.13-1.24)	−0.3	−1.4	0.44 (0.08-1.1)	0.29 (0.06-0.66)	−0.41	0.5
Esophagus	0.51 (<0-1.13)	0.82 (<0-3.1)	−0.3	−1.4	0.88 (0.11-2.1)	0.14 (<0-0.63)	−0.41	2.8
Stomach	0.21 (0.11-0.4)	0.48 (0.31-0.73)	−0.3	−1.4	4.9 (2.7-8.9)	4.9 (3.2-7.3)	−0.41	2.8
Colon	0.63 (0.37-1.1)	0.43 (0.19-0.96)	−0.3	−1.4	3.2 (1.8-5.6)	1.6 (0.80-3.2)	−0.41	2.8
Rectum	0.12 (<0-0.38)	0.12 (<0-0.38)	−0.3	−1.4	0.34 (0.09-1.1)	0.34 (0.09-1.1)	−0.41	2.8
Gallbladder	−0.018 (<0-0.29)	−0.018 (<0-0.29)	−0.3	−1.4	NA	NA	NA	NA
Pancreas	0.36 (<0-0.88)	0.36 (<0-0.88)	−0.3	−1.4	0.49 (0.09-1.1)	0.49 (0.09-1.1)	−0.41	2.8
Liver	0.32 (0.16-0.64)	0.32 (0.10-1.0)	−0.3	−1.4	2.2 (0.9-5.3)	1.0 (0.40-2.5)	−0.41	4.1
Lung	0.32 (0.15-0.70)	1.4 (0.94-2.1)	−0.3	−1.4	2.3 (1.1-5.0)	3.4 (2.3-4.9)	−0.41	5.2
Breast	NA	NA	NA	NA	NA	10 (7.0-14.2)	−0.50	3.5, 1.0a
Ovary	NA	0.38 (0.10-1.4)	−0.3	−1.4	NA	0.70 (0.2-2.1)	−0.41	2.8
Uterus	NA	0.055 (<0-0.22)	−0.3	−1.4	NA	1.2 (<0-2.6)	−0.41	2.8
Prostate	0.12 (<0 – 0.69)	NA	−0.3	−1.4	0.11 (<0-1.0)	NA	−0.41	2.8
Bladder	0.50 (0.18-1.4)	1.65 (0.69-4.0)	−0.3	−1.4	1.2 (0.4-3.7)	0.75 (0.3-1.7)	−0.41	6.0
Kidney	0.34 (<0-1.0)	0.34 (<0-1.0)	−0.3	−1.4	0.31 (0.08-0.68)	0.31 (0.08-0.68)	−0.41	2.8
Brain/CNS	0.71 (0.26-1.34)	0.24 (0.09-0.47)	−0.3	−1.4	NA	NA	NA	NA
Thyroid	0.53 (0.14-2)	1.05 (0.28-3.9)	−0.83	0	N.A	NA	NA	NA
Remainder	0.87 (0.45-1.69)	0.80 (0.33-1.93)	−0.3	−1.4	2.73 (1.55-4.82)	1.06 (0.49-2.28)	−0.41	2.8
Leukemiab	β males	β females	γ	δ	ϕ	θ
ERR model	1.1 (0.1-2.6)	1.2 (0.1-2.9)	−0.4 (−0.78-0)	−0.48 (−1.1-0.2)	0.42 (0-0.96)	0.87 (0.16-15)
EAR model	1.62(0.1-3.6)	0.93 (0.1-2.0)	0.29 (0-0.62)	0.0	0.56 (0.31-0.85)	0.88 (0.16-15)

^aThe form of model for solid cancers is ERR or EAR = β_s D exp (γ e*)(a*)^η, where D is dose in Gy, e is age at exposure (years), e* is (e-30)/10 for e<30, and zero for e≥ 30, a is attained age (years) and a* is (a/60). γ represents per-decade increase in age at exposure over the range 0-30 years. For breast cancer e* is (e-25)/10 for all e, and a* is (a/50), and η is 3.5 for a ≤ 50 and 1.0 elsewhere. The uncertainty in the β coefficient was represented by lognormal probability distributions for all original BEIR VII cancers other than prostate and uterus for which normal distributions were used. For the additional cancer types, other than central nervous system (CNS), the profiles of β coefficient have been directly used to define the cumulative distribution functions. For CNS, the profile was approximated by a lognormal distribution.

^bThe form of model for leukemia is ERR or EAR = β_S D (1+θ D) exp[γ e*+δ ln(t/25) + ϕ e* ln(t/25)], where D is dose in Gy, e is age at exposure (years), e* is (e-30)/10 for e<30, and zero for e≥ 30, and t is the time since exposure (years). The quadratic model is applied for acute exposures to low LET radiation (photons and electrons of any energy), while for chronic exposures the quadratic term is dropped (θ = 0) and only the linear term is kept. Propagation of uncertainties for leukemia models is performed using the “delta method” analytical approach (5); thus, no probability distribution functions for have been defined for the parameters of the leukemia model.

NA – not applicable.

For RadRAT we developed risk models for seven additional cancer sites using the 1958-1998 LSS dataset and the same basic solid cancer risk model formulation employed in BEIR VII, described above. We included cancers for which there were at least 100 incident cases available and that had been evaluated in detail in the LSS cancer incidence report [6]. Despite being the largest and longest study of the effects of low-dose radiation exposure the LSS is still limited in its ability to precisely estimate site-specific cancer risks taking account of key effect modifiers such as age at exposure and sex. Therefore, the approach used in the BEIR VII report and in RadRAT was to use the values of the parameters γ (age at exposure effect) and η (attained age effect) from a model based on all solid cancers (excluding thyroid and non-melanoma skin cancer). Only if these values were found to be incompatible with the data for an individual cancer site were site-specific values used. The results of this model fitting for the seven additional cancer sites, oral, esophagus, gallbladder, pancreas, rectum, kidney and brain/central nervous system, are shown in Table 1. Following BEIR VII, cancer sites are included in RadRAT even if the risk coefficients are not statistically significantly different from zero (see for example rectal cancer, Table 1). The uncertainty distributions for the risk coefficients for such cancer sites include a negative tail. This leads to estimates of lifetime risk that can have a negative lower bound, ensuring that the mean of the predicted lifetime risk is not biased.

For the original 11 sites evaluated in BEIR VII, the β_S coefficients were estimated separately for males and females; for the seven additional sites, sex-specific estimates were used only if the coefficients for the two sexes differed significantly. There was evidence of statistically significant differences (p<0.05) between men and women in the risk parameters for oral, esophageal, and brain/central nervous system cancers, but not for any of the other additional sites (Table 1). For tumors of the central nervous system, the LSS data indicated a highly significant dose-response for males but excess risk estimates that were close to zero for females. Based on the data from the LSS and several other studies of radiation-related malignant brain cancers [12-15], the ERR model used in RADRAT was estimated from the LSS data by fixing the male:female ratio at 3:1. No EAR model could be determined for the central nervous system because the LSS cases include benign tumors. For gallbladder we only included the ERR model because the central estimate for the EAR model was basically equal to zero. There was no evidence of significant departure from the standard parameterization for attained age and age at exposure for any of the seven additional sites.

The BEIR VII committee provided a risk model for the remainder category, which is a cancer grouping including all solid tumors other than the 10 solid tumors modeled explicitly, and excluding non-melanoma skin cancer. Since we developed risk models for an additional seven types of solid tumors, a new remainder was also developed for all solid cancers excluding these seventeen cancers. The ERR model for the new remainder category has a mathematical form similar to that applicable to most solid cancers and has a sex-specific risk coefficient (β). When the total cancer risk is estimated, the radiation risk calculator sums the risk across of all cancer types including the remainder cancer grouping, provided an appropriate dose estimate is available (see below for further details).

Dose and Dose Rate Effectiveness Factor

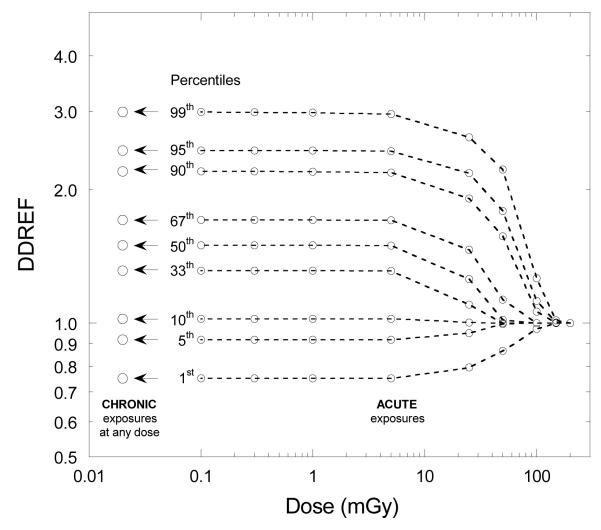
Because there is some evidence that the cancer risk per unit dose at low doses and dose rates may be somewhat lower than the risk at higher doses and dose rates many organizations have recommended applying a reduction factor when projecting risks derived from high-dose and dose-rate epidemiological data based on a linear, no-threshold (LNT) model. This adjustment factor is known as the dose and dose rate effectiveness factor (DDREF), and is applied as a divisor of the risk from exposures to high doses and dose rates. Different groups and organizations use different values and ranges for this factor. A point value of 2 for DDREF assigned by the ICRP (1991) [16] was subsequently adopted by other bodies for use in radiation protection. Consideration of uncertainty led to the development of probability distributions of DDREF for use in risk assessment. In addition to the probability distribution developed by the BEIR VII committee [5], probability distributions of DDREF were also assembled for use in IREP (Interactive Radio-Epidemiological Program) risk calculator [10,17], in the risk assessment methodologies of NCRP [18] and U.S. Environmental Protection Agency (EPA) [19], and in other risk assessments [20]. For the solid cancer risk models, the assumption in RadRAT follows that of BEIR VII by applying an uncertain DDREF [5] for all chronic exposures and for acute exposures below 100 mGy described by a lognormal distribution with a geometric mean (GM) of 1.5 and geometric standard deviation (GSD) of 1.35¹. That committee was only concerned with doses below 100 mGy of low-LET radiation and therefore did not make recommendations for acute exposures that exceeded 100 mGy (Table A-1). To allow risk estimates for acute exposures exceeding 100mGy in RadRAT, a DDREF is introduced (using a logistic function; Figure 1) for doses below a dose limit D_L [10,17]. The magnitude of the dose limit D_L is considered uncertain and is allowed to vary from 30 to 200 mGy using a log-uniform probability distribution. As a result, no DDREF is applied at acute doses greater than 200 mGy. On the other hand, the full distribution of DDREF applicable for chronic, low-dose, exposure rates is essentially applied if the acute dose is less than 3 mGy (Figure 1). This approach to the uncertainty is similar to that used for the IREP risk calculator [10,17]. For leukemia the uncertainty associated with the risks at low doses is accounted for by the uncertainty in the linear term in the linear-quadratic model.

Figure 1.

Application of DDREF for acute and chronic exposures

Adjustments for Minimum Latency Period

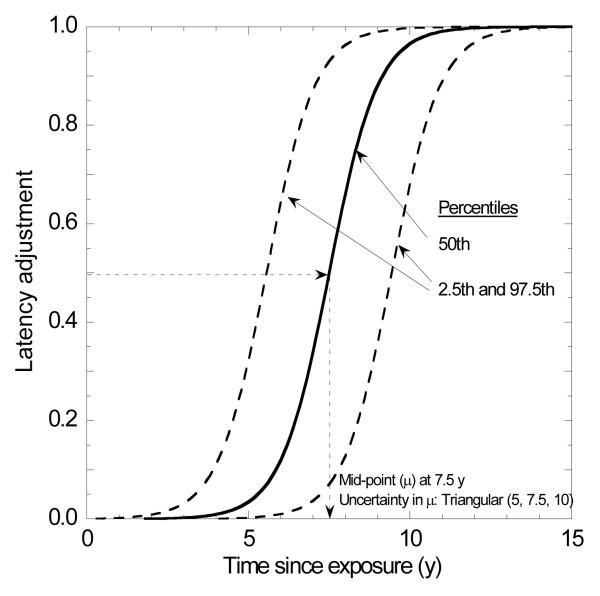
The latency period for radiation-related cancers is introduced as a step-function in BEIR VII at 2 years for leukemia and 5 years for solid cancers [5]. To avoid this abrupt change in risk in RADRAT we assumed an uncertain latency adjustment that is phased in between 4 and 11 years after exposure for solid cancers, 0.4 and 4.1 years for leukemia and 2.5 and 7.6 years for thyroid cancer [10]. The latency adjustment F_latency is represented by an S-shaped function (Figure 2):

$${\mathrm{F}}_{\text{latency}}\left(t\right)=\frac{1}{1+e^{-\frac{(t-\mu )}{S}}},$$ (Eq.Lat1)

where t is time since exposure (y), μ is the time since exposure (y) at the inflection point where F_latency = 0.5, and S is a shape parameter that defines the steepness of the function and ensures that F_latency reaches values of approximately 0.01 and 0.99 at the time limits listed above [10,17].

Figure 2.

Latency adjustment factor applied for most solid cancers

To represent uncertainty in the adjustments for minimum latency on risk estimates, the midpoint, μ, is described by the following triangular probability distributions: solid cancers other than thyroid, T(5, 7.5, 10); thyroid, T(3, 5, 7); and leukemia, T(2, 2.25, 2.5), where numbers represent time after exposure in years. The effect of uncertainty in μ on the adjustment for minimum latency for solid cancers except thyroid is indicated by the percentiles of the latency adjustment shown in Figure 2.

Transfer from the Japanese to the U.S. Population

One of the key issues in risk projection exercises is how to apply the risk estimates from the Japanese to the U.S. population. This is primarily an issue for cancer sites that have very different baseline rates in the two populations, such as stomach cancer (much higher in Japan) and lung cancer (lower in Japan). The BEIR VII committee addressed this problem by calculating a weighted mean of the estimates from the excess relative risk (ERR) and excess absolute risk (EAR) models [5]. The weights applied in BEIR VII to most organ sites, of 0.3 for the additive model estimate and 0.7 for the multiplicative model estimate, are subjective and reflect the BEIR VII committee’s assessment that risks were more likely to transfer multiplicatively than additively from the Japanese to the U.S. population. In RadRAT we followed the approach and used the weights developed by BEIR VII with one difference. BEIR VII used a weighted geometric mean because it simplified the calculation of their analytic uncertainty estimates. Since RadRAT uses Monte Carlo simulation to quantify the uncertainties (more details below) it was not necessary to weight on the geometric scale and therefore we weighted on the arithmetic scale. We made this choice because the arithmetic mean explicitly captures the subjective judgment about the likely appropriateness of each model, and insures additivity of site-specific risks and of risks from multiple exposures, properties that the geometric mean does not have. An arithmetic mean was also used previously in NCRP Report No. 126 [18], IREP [10], ICRP Publication 103 [21] and by the U.S. EPA [22]. For most organ sites this choice makes little difference, but we note that weighted arithmetic means of two different positive numbers are always greater than the correspondingly weighted geometric means.

For several sites, only an additive or multiplicative model estimate was used, as in BEIR VII. For breast cancer, the EAR model was used following the finding by Preston et al. [11] that the additive model breast cancer dose-response estimates from four study populations involving irradiation unrelated to existing breast disease were more compatible across populations than the corresponding multiplicative models. For thyroid cancer, the multiplicative model developed for IREP [10], based on the pooled analysis of Ron et al. [9] and adjusted for gender-dependency by BEIR VII committee [5], was used. For gallbladder cancer, only the multiplicative projection is applied to the U.S. population. For lung cancer, the additive and multiplicative models were given reversed weights of 0.7 and 0.3, respectively, to reflect A-bomb survivor evidence that radiation and smoking may act additively rather than multiplicatively with respect to the risk of lung cancer in the LSS [23]. For RadRAT we used only an ERR model for brain/CNS cancer because the LSS data were only available for benign and malignant tumors combined and this would result in over-estimation of the EAR for malignant tumors alone. An additive projection of risk of brain/CNS cancer to the U.S. population was simulated using the transfer between populations method described in IREP [17]:

$${\mathrm{ERR}}_{\mathrm{add}}={\mathrm{ERR}}_{\text{mult}}\mathrm{x}({\mathrm{B}}_{\text{Japan}}∕{\mathrm{B}}_{\mathrm{US}})$$

where B_Japan and B_US are age-adjusted baseline incidence rates in Japan and U.S. populations, standardized to the world population age distribution.

U.S. Population Data

In RadRAT the ERR calculations are based on U.S. baseline cancer incidence rates for the years 2000-2005 obtained from the 17 cancer registries database of Surveillance, Epidemiology and End Results (SEER) program [24]. Adjustments for competing causes of death were made using a survival function based on U.S. general population life tables for men and women [25]. BEIR VII used the SEER incidence rates for years 1995-1999 [5]. However, for most cancer sites the differences in age-specific rates between these two time periods will be small.

Uncertainty Calculations

A key component of RadRAT is that both statistical and subjective sources of uncertainty are accounted for in the calculations. Parameters are assigned probability distributions and Monte Carlo simulation is the primary method for uncertainty propagation, based on Latin-hypercube sampling performed by the software package Analytica® [26]. The result, an uncertainty distribution for estimated risk, provides information that can be used for a number of purposes. The full uncertainty distribution in the risk estimate can be used to calculate summary statistics such as the mean and various quantiles, but it can also be used for other purposes such as risk/benefit analyses

A particular advantage of the Monte-Carlo method is that it allows easy incorporation of multiple sources of uncertainties. The analytical approach used in BEIR VII included uncertainties in the risk model coefficients, in the transfer to the U.S. population, and in the DDREF [5]. RadRAT accounts for these three sources of uncertainty and, in addition, it accounts for uncertainty in radiation doses, and uncertainties in the adjustments related to the minimal latency period. The uncertainty distributions for each parameter are described below.

The uncertainty ranges for the parameters of the ERR and EAR risk models are presented in Table 1. For solid tumors, both the original BEIR VII models and the new models described in this paper carry uncertainty only in the sex-specific main effect parameters β, while the parameters γ and η are fixed with values estimated from all solid cancers combined. As in the BEIR VII report, the uncertainties in parameters γ and η were neglected, since the uncertainty in the estimated coefficient of dose (β) is quite large and is expected to dominate the uncertainty in the estimated lifetime risk [5]. For the original BEIR VII risk models for solid cancers the uncertainty in the parameter β was described by a lognormal probability distribution, with the exception of prostate and uterine cancers for which normal (Gaussian) probability distributions were used. The uncertainty in the parameter β for the seven new risk models was modeled using cumulative probability distribution functions obtained directly from the profiles produced by the regression analysis of the epidemiologic data.

For all risk models for solid tumors, Monte-Carlo methods were used to propagate uncertainty. The risk model for leukemia carries uncertainties in all its five parameters (Table 1), and these parameters are correlated. To properly account for the correlations among parameters, the risk calculator derives an approximate estimate of the variance of the lifetime risk for leukemia using the same “delta method” used by the BEIR VII committee [5]. The delta method is an analytical error propagation technique for linear models which relies on the first-order Taylor’s approximation, applied in logarithmic space, leading to estimates of the variance of the logarithm of the lifetime risk. This method implicitly uses the variance-covariance matrix of the parameters, thus accounting for the correlation among parameters.

In the BEIR VII report, the delta method for the leukemia model is applied to excess risk per unit dose. RadRAT is applied to a user-specified dose which may be uncertain, and the delta method is coupled with the Monte-Carlo method so that the dose uncertainty can be taken into account. That is, in any given Monte-Carlo iteration, a single dose value is generated and the associated lifetime risk and the variance of its logarithm are estimated using the delta method. A Monte-Carlo sample of the uncertain lifetime risk corresponding to the sampled dose value is obtained from a lognormal distribution with a geometric mean determined by the dose value, and a geometric standard deviation determined by the delta method. The procedure is repeated for each Monte-Carlo iteration of the dose, generating a collection of Monte-Carlo samples for lifetime risk of leukemia.

The transfer of risk from the Japanese to the U.S. population considers both a multiplicative and an additive projection of risk. The uncertainty in risk transfer arises from the lack of precise knowledge about which type of risk projection is correct. The true risk for the U.S. population is probably located within the range defined by the multiplicative and additive risk projections, although it can be shown that it is possible that the true risk exists outside this range [10]. In the risk calculator, as in BEIR VII report, discrete weights are assigned to the multiplicative and additive projections as a Bernoulli probability distribution. The Bernoulli distribution is applied in arithmetic space rather than in logarithmic space as was done by BEIR VII. For most solid tumors and for leukemia, the Bernoulli distribution for transport of risk from the LSS cohort to the U.S. population has a weight p = 0.7 assigned to the multiplicative projection, while the remaining 1-p = 0.3 weight is assigned to the additive projection. For lung cancer, the weights are reversed: 0.3 to multiplicative and 0.7 to additive. For breast cancer, only an additive projection is used. Multiplicative projections only are used for thyroid and gallbladder. That is, for the latter three cancers there is no uncertainty assigned to the model used for risk transport.

The total lifetime risk of cancer from exposures to multiple organs is obtained by summing the lifetime risks for each specific cancer type; the fact that many of the uncertainties are shared has to be accounted for in these calculations. When risks for each specific cancer type are calculated in a case of exposure to multiple organs, the correlations of various uncertainty sources among cancer types are handled in the Monte-Carlo uncertainty propagation procedure as described below:

1. The statistical uncertainties in model parameters are considered independent among cancer types. This includes the uncertainties in (a) the main effect parameters (β_s) for each specific cancer and (b) age- time-related parameters for leukemia. Parameters in the leukemia model are correlated among themselves, but they are independent from the parameters of the risk models for solid cancers.

2. The parameters describing the adjustment for the effect of minimum latency are assumed to be perfectly correlated among different cancer types, except those applied for thyroid and leukemia, which have different and independent parameters for the latency adjustment.

3. The uncertainties in the transfer weightings are treated as fully correlated among all cancer types having the same transfer model. Lung, breast, and thyroid have alternative models to transfer risk to the U.S. population. The transfer weightings for these cancers are assumed to be independent from the transfer weightings for any other cancer types. 4. The DDREF distributions are assumed to be fully correlated among all solid cancer sites.

BEIR VII did not present or estimate uncertainties for sums of site-specific risks. Its risk estimate (with uncertainties) for all solid cancer is based on a model in which all solid cancers were analyzed together, as a group, and on the assumption that all organs of the body receive the same dose. If the risk calculator is used to estimate risk for all solid cancers under this same assumption about organ doses, the uncertainties will be slightly larger than those reported by BEIR VII.

In addition to the correlations among risks for different cancer types, there are correlations among multiple exposures to the same organ site. This accounts for cases in which a person or a population receives several separate doses of radiation. We assumed full correlations in the calculation of total risk as a sum of risks across multiple exposures for each of the following:

1. Statistical uncertainties in model parameters

2. Uncertainties in parameters describing the adjustment for the effect of minimum latency

3. Uncertainties in transfer model parameters

4. DDREF

Input information required by RadRAT

RADRAT calculates the lifetime risk of radiation-related cancer for a user-defined exposure history, based on the following required inputs:

• Sex and year of birth

• Exposure history: number of exposures, year in which each exposure occurred, organ or organs that were exposed, dose to each exposed organ from each exposure, and exposure rate (chronic or acute) for each organ and each exposure.

• Run-specific parameters: the random seed and the sample size of the Monte-Carlo simulation used for propagation of uncertainties

The dose to each exposed organ is the absorbed dose, specified in mGy. The dose can be specified either with no uncertainty (i.e., constant, equal to the average dose to the organ) or with uncertainty, using one of several probability distribution functions: lognormal (GM, GSD), normal (mean, standard deviation), triangular or log-triangular (minimum, mode, maximum), and uniform or log-uniform (minimum, maximum). In cases of uniform, whole-body exposure, a single, whole-body dose (either uncertain or constant) is applied to all cancer sites including the remainder grouping of cancers. In cases of non-uniform, whole-body exposures the user needs to provide organ-specific doses, including a dose to the remainder grouping of cancers. The latter dose could be a weighted average of doses to affected organs, although there is no straightforward way to define these weights.

The calculator has flexible input tables that allow estimation of risk for complex exposure histories, and is able to handle cases which include multiple exposures, each exposure with a different list of organs and each organ with a different dose, accompanied by a different statement of uncertainty (i.e., different probability distributions). Past or future years of exposure can be specified in the exposure history, depending on the desired type risk assessment: that is, retrospective or prospective assessments.

Example risk calculations

Estimates of the excess number of cancers in a population of 1000 persons exposed to 0.1 Gy are provided in Table 2 according to age at exposure and sex for all eighteen cancer sites. Table 3 includes estimates of the excess cases of all solid cancers following chronic exposures to 0.1 Gy at age 10, 30 and 50, 1 mGy per year throughout life and 10 mGy per year from age 18-65 years for both RadRAT and BEIR VII. Table 4 provides risk estimates for leukemia, for acute and chronic exposure scenarios.

Table 2.

Lifetime risk of radiation-related cancer (per 1000 exposed individuals) according to age at exposure and cancer following a chronic exposure to 0.1 Gy

a) Females
	Age at exposure (yr)
Cancer	0	5	10	15	20	30	40	50	60	70	80
Oral Cavity	0.59	0.49	0.41	0.34	0.28	0.19	0.17	0.14	0.10	0.05	0.02
Esophagus	0.30	0.25	0.22	0.18	0.16	0.11	0.11	0.10	0.08	0.04	0.02
Stomach	2.30	1.90	1.50	1.30	1.00	0.68	0.63	0.56	0.44	0.29	0.13
Colon	2.50	2.20	1.80	1.60	1.30	0.93	0.89	0.80	0.65	0.41	0.16
Rectum	0.35	0.30	0.25	0.21	0.18	0.12	0.11	0.09	0.07	0.04	0.02
Liver	0.67	0.55	0.45	0.37	0.31	0.21	0.20	0.18	0.15	0.10	0.05
Gallbladder	−0.02	−0.01	−0.01	−0.01	−0.01	−0.01	−0.01	−0.01	−0.004	−0.003	−0.001
Pancreas	0.66	0.56	0.47	0.40	0.34	0.24	0.23	0.21	0.17	0.10	0.04
Lung	8.50	7.20	6.00	5.00	4.20	2.90	2.90	2.70	2.10	1.30	0.61
Breast	13.00	10.00	7.80	6.10	4.70	2.70	1.50	0.71	0.30	0.11	0.03
Ovary	1.10	0.95	0.79	0.65	0.54	0.37	0.33	0.27	0.19	0.10	0.04
Uterus	0.67	0.56	0.47	0.39	0.32	0.21	0.19	0.15	0.11	0.07	0.03
Bladder	2.60	2.30	1.90	1.60	1.40	1.00	0.98	0.91	0.74	0.47	0.20
Kidney	0.56	0.47	0.40	0.33	0.28	0.20	0.18	0.15	0.11	0.06	0.02
Brain/CNS	0.30	0.20	0.15	0.12	0.10	0.06	0.05	0.04	0.03	0.01	0.00
Thyroid	12.00	7.80	5.10	3.30	2.10	0.80	0.27	0.08	0.02	0.005	0.001
Remainder	4.30	3.60	3.00	2.40	2.00	1.30	1.10	0.83	0.58	0.32	0.12
Leukemia	2.40	1.20	0.92	0.80	0.74	0.66	0.66	0.67	0.68	0.63	0.45

b) Males
	Age at exposure (yr)
Cancer	0	5	10	15	20	30	40	50	60	70	80
Oral Cavity	0.60	0.51	0.42	0.35	0.29	0.20	0.18	0.13	0.08	0.04	0.01
Esophagus	0.72	0.61	0.51	0.43	0.36	0.26	0.24	0.21	0.15	0.08	0.03
Stomach	1.90	1.60	1.30	1.00	0.85	0.56	0.52	0.45	0.35	0.21	0.09
Colon	3.70	3.20	2.70	2.30	1.90	1.40	1.30	1.20	0.91	0.53	0.19
Rectum	0.38	0.32	0.27	0.23	0.20	0.14	0.13	0.11	0.08	0.04	0.01
Liver	1.20	0.99	0.82	0.67	0.56	0.38	0.36	0.31	0.24	0.15	0.07
Gallbladder	−0.01	−0.01	−0.01	−0.01	−0.01	−0.005	−0.004	−0.004	−0.003	−0.002	−0.001
Pancreas	0.64	0.55	0.47	0.40	0.34	0.24	0.23	0.21	0.15	0.09	0.03
Lung	3.60	3.00	2.50	2.10	1.70	1.20	1.20	1.10	0.92	0.59	0.27
Prostate	2.00	1.70	1.50	1.30	1.10	0.83	0.83	0.74	0.49	0.19	0.05
Bladder	2.70	2.30	2.00	1.70	1.40	1.00	1.00	0.97	0.80	0.49	0.19
Kidney	0.84	0.71	0.61	0.52	0.44	0.32	0.29	0.24	0.16	0.08	0.02
Brain/CNS	1.10	0.75	0.57	0.45	0.36	0.23	0.20	0.15	0.10	0.05	0.01
Thyroid	2.10	1.40	0.92	0.60	0.39	0.16	0.06	0.02	0.01	0.001	0.000
Remainder	7.10	6.00	4.90	4.00	3.20	2.10	1.80	1.50	1.10	0.57	0.19
Leukemia	2.80	1.50	1.20	1.00	0.95	0.85	0.85	0.87	0.89	0.83	0.56

Table 3.

Lifetime cancer risk estimates for all solid cancers (per 1000 exposed individuals) for various chronic exposure scenarios

a) Females
Exposure scenario	RadRAT 17 sitesa (95% subjective CI)	RadRAT 10 sitesb (95% subjective CI)	BEIR VII 10 sitesc (95% subjective CI)
0.1 Gy at age 10d	31 (15-56)	29 (14-53)	25 (13-49)
0.1 Gy at age 30d	12 (5.8-22)	11 (5.3-21)	10 (5-20)
0.1 Gy at age 50d	7.9 (3.7-15)	7.2 (3.3-14)	7 (4-13)
1 mGy per year throughout lifee	12 (5.9-23)	11 (5.5-22)	10 (5-18)
10 mGy per year from age 18-65e	50 (25-98)	46 (23-91)	40 (21-78)

b) Males
Exposure scenario	RadRAT 17 sitesa (95% subjective CI)	RadRAT 10 sitesb (95% subjective CI)	BEIR VII 10 sitesc (95% subjective CI)
0.1 Gy at age 10d	19 (7.6-41)	17 (5.7-36)	13 (7-27)
0.1 Gy at age 30d	9.1 (3.3-20)	7.7 (2.3-18)	6 (3-13)
0.1 Gy at age 50d	7.3 (2.5-17)	6.3 (1.6-15)	5 (2-11)
1 mGy per year throughout lifee	8.1 (3.6-18)	6.9 (2.8-16)	6 (3-11)
10 mGy per year from age 18-65e	40 (17-90)	34 (13-78)	26 (13-54)

^aSum of site-specific lifetime attributable risks for all solid cancers used in RadRAT, plus the remainder.

^bSum of site-specific lifetime attributable risks for the 10 solid cancers defined by BEIR VII committee, plus the remainder.

^cSum of site-specific lifetime attributable risks for the 10 solid cancers defined by BEIR VII committee, plus the BEIR VII remainder.

^dEstimates based on 2000 Latin Hypercube samples, for one exposure, at specified age.

^eEstimates based on 300 Latin Hypercube samples, for 120 and 48 years of exposures, respectively, at specified ages.

Table 4.

Lifetime cancer risk estimates for leukemia (per 1000 individuals) for various exposure scenarios

	Males		Females
Exposure scenario	RadRAT (95% subjective CI)	BEIR VII (95% subjective CI)	RadRAT (95% subjective CI)	BEIR VII (95% subjective CI)
0.1 Gy at age 10a	1.3 (0.37-3.3)	1.2 (0.4-3.6)	1.0 (0.27-2.8)	0.9 (0.3-3.0)
0.1 Gy at age 30a	0.91 (0.30-2.2)	0.8 (0.3-2.3)	0.7 (0.22-1.8)	0.6 (0.2-1.7)
0.1 Gy at age 50a	0.92 (0.21-2.3)	0.8 (0.2-2.9)	0.7 (0.17-2.0)	0.6 (1.6-2.3)
1 mGy per year throughout lifeb	0.9 (0.19-2.6)	0.7 (0.19-2.3)	0.68 (0.12-2.1)	0.5 (0.1-2.0)
10 mGy per year from age 18-65b	4.3 (1.1-11.5)	3.6 (1.1-11.4)	3.3 0.76-9.2	2.7 (0.8-9.2)

^aacute exposure

^bchronic exposure over 120 and 48 years of exposure, respectively (total doses 120 mGy and 480 mGy, respectively).

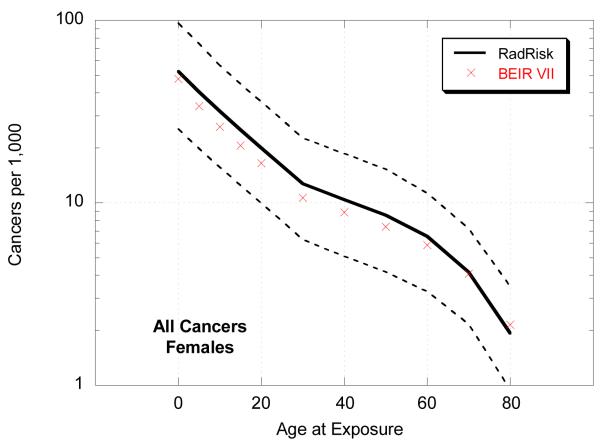
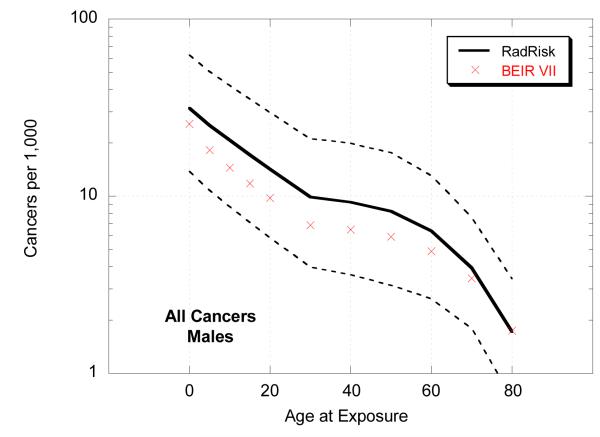
We also compared estimates of total risk for all cancers from RadRAT with those from BEIR VII following a single chronic dose of 0.1 Gy by age at exposure (Figure 3). The lifetime risk estimates were slightly higher for RadRAT than for BEIR VII across all exposure ages mostly because the weighting of the multiplicative and additive models was conducted on an arithmetic rather than a logarithmic scale. This was true for all site-specific cancers (data not shown). The differences were greater for younger ages at exposure and for males. The widths of the uncertainty intervals were, however, generally similar.

Figure 3.

Lifetime risk estimates (per 1000 exposed individuals) for all cancers, by sex and age, following 0.1 Gy chronic exposure

Discussion

We developed the first online radiation risk calculator that calculates the lifetime risk of radiation-related cancer for organ-specific doses. A key component of the calculator is the estimation of uncertainty distributions that take account of the major sources of uncertainty and assumptions involved in the calculations. The calculator also extends the BEIR VII report by including seven additional site specific cancer models.

As shown in Figure 3 the mean lifetime risk estimates from RadRAT are somewhat higher than those from BEIR VII, for the reasons discussed above. However, our estimates are generally at the lower end of the range of the cancer incidence results from UNSCEAR 2006 [27]. Although the UNSCEAR estimates were also based on the same LSS dataset the modeling formulations were different and rather than estimating a weighted average separate results were presented based on the additive and multiplicative models. For example, for a male exposed to 0.1 Gy at age 30 the lifetime risk estimates in UNSCEAR ranged from 8 cancers per 1000 under the additive model to 17 per 1000 under the multiplicative model [27,28], whereas in RadRAT the estimate was 9 cancers (95% UI: 3.3-20) per 1000 (Table 3).

A number of simpler calculators are available online that use effective dose and total cancer risk, see for example www.xrayrisk.com [29]. That approach has several limitations when applied to non-uniform doses because tissue weighting factors are not age-specific. Furthermore, most of these calculators do not provide uncertainty estimates.

RadRAT can be used to estimate risks from acute or chronic doses lower than about 1 Gy, childhood exposures, or about 2 Gy for adult exposures. The risk from higher doses may be overestimated or may even exceed one for children, when using the lifetime attributable risk methodology. Furthermore, there is limited information about the shape of the dose-response curve above about 2 Gy [5]. For the low-dose exposures (<100 mGy) these estimates depend on the linear no-threshold assumption [30] as it is difficult to quantify risks at these dose levels directly in most studies due to lack of statistical power [1]. We assumed that the risk per unit dose is lower for low-doses and low dose rates as determined by a specified, uncertain DDREF. Most international radiation protection organizations support the use of this assumption [27,31].

Although we expanded the number of site-specific risk models there are still a number of sites that could not be included explicitly because we were not able to estimate stable risk models with the small number of cases in the Japanese atomic bomb survivors study (n<100) and also because about one quarter of the cancers in the remainder group were cancers for which the primary site could not be determined. However, the eighteen specific cancer sites that were included together account for about 80% of annual cancers in the US [32]. We also provided a risk model for the remainder cancer sites, which can be used for estimating the total cancer risk from uniform whole body exposures. There is no straightforward way in which to include these remainder cancer sites if the whole-body exposure is non-uniform because of the difficulty in assigning an appropriate dose. Ignoring the risk from the remainder cancer sites will likely result in some under-estimation of total cancer risk for non-uniform exposures.

The life-expectancy of the exposed individuals is another key assumption in lifetime risk estimation. In RadRAT, the estimates are calculated using the U.S. general population survival probabilities [25]. For individuals who have shorter life-expectancy, for example current smokers or people who are very sick, the lifetime cancer risk from a radiation exposure will be lower due to short life-expectancy from higher competing risks.

RadRAT is most appropriate for risk estimation for exposures from gamma rays and high-energy photons since it does not include adjustments for the additional uncertainty associated with the risk from exposure to other types of radiation, such as lower energy photons and electrons (i.e., x-rays) and high-LET radiation types (i.e., various energies of neutrons and alpha particles). In agreement with BEIR VII we do not include adjustments for lower energy photons because of the conflicting epidemiological and biological data on this issue (5]. Whilst some laboratory studies suggest that the relative biological effectiveness of X-rays may be higher by a factor of 2 or 3, studies in persons exposed to X-rays for medical reasons generally find lower risk estimates than in the atomic bomb survivors [33,34]. However, there are other differences between the study populations that might contribute to the lower risks in the medically exposed and it is not possible to disentangle the various effects. Given the very large uncertainties regarding the relative biological effectiveness of high-LET data we recommend against the use of the calculator for exposures of these types at this time [5].

Summary

In conclusion, RadRAT is an online radiation risk assessment tool that calculates lifetime cancer risk with uncertainty distributions for complex exposure histories from both uniform and non-uniform doses. It is most appropriate for low-LET radiation doses <1Gy, and for individuals with life-expectancy and cancer rates similar to the general population in the U.S. RadRAT will be available online at https://irep.nci.nih.gov/radrat). .

Table A.1.

Summary of differences between the NCI RadRAT and BEIR VII methodologies

BEIR VII	NCI RadRAT
Risk models
11 cancers: stomach, colon, liver, lung, breast, prostate, uterus, ovary, bladder, thyroid and leukemia plus 1 remainder cancer grouping (solid cancers other than the ones modeled individually in BEIR VII)	11 cancers: stomach, colon, liver, lung, breast, prostate, uterus, ovary, bladder, thyroid and leukemia from BEIR VII plus 7 new cancers: oral cavity and pharynx, esophagus, rectum, gallbladder, pancreas, kidney, central nervous system plus 1 remainder cancer grouping (solid cancers other than the ones modeled individually in NCI RadRAT)
Formulation of breast cancer EAR model adapted from but not identical to the model by Preston et al. (2002).	Formulation of breast cancer EAR model exactly as in Preston et al. (2002).
Linear-quadratic model for leukemia applied for both acute and chronic exposures to low doses.	Linear-quadratic model for leukemia applied for acute exposures; for chronic exposures only the linear term of the model is applied.
Adjustments for the Effects of the Minimum Latency Period
Threshold function of time since exposure causing risk to abruptly change from zero to maximum risk at times since exposure equal to 2 years for leukemia and 5 years for other solid cancers.	S-shaped function of time since exposure with a smooth transition from zero to maximum risk. The mid point of the S-shaped function is 2.25 years for leukemia, 5 years for thyroid and 7.5 years for other solid tumors, with non-zero risk value at times since exposure lower than the mid-point.
No uncertainty associated with assumed latency adjustment.	Uncertainty in latency adjustment described by probability distributions for the mid-point of the S-shaped function.
Transfer from the Japanese to the U.S. Population
Additive and multiplicative projections of the lifetime risk are weighted in logarithmic space. The assigned weights are 0.7 to the multiplicative projection and 0.3 to the additive projection, for most cancer types other than lung for which the two weights are reversed (0.3 to multiplicative and 0.7 to additive projections, respectively). No transfer is applied for thyroid cancer for which only the multiplicative projection is used, or for breast cancer for which the additive projection is preferred.	Additive and multiplicative projections of annual risk at each attained age are weighted in linear space. The assigned weights are 0.7 to the multiplicative projection and 0.3 to the additive projection, for most cancer types other than lung for which the two weights are reversed (0.3 to multiplicative and 0.7 to additive projections). No transfer is applied for thyroid cancer for which only the multiplicative projection is used, or for breast cancer for which the additive projection is preferred.
	No transfer is applied for gallbladder cancer for which only the multiplicative projection is used.
	No EAR model could be derived for central nervous system from the LSS data. An additive projection is determined using the method applied in the IREP code (Land et al. (2003; Kocher et al. 2008) based on the ratio of the baseline rates in the US and Japan.
Dose and Dose-Rate Effectiveness Factor (DDREF)
The DDREF is described by a lognormal probability distribution with a geometric mean equal to 1.5 and a geometric standard deviation equal to 1.35 and is applied for all cancer sites except leukemia for which the model is linear quadratic. The DDREF is applied for chronic exposures (i.e., exposures to low dose rates), but also for acute exposures at dose less tha 100mGy, which in the BEIR VII report are assumed to consist only of low or very low doses of radiation.	The DDREF is described by a lognormal probability distribution with a geometric mean equal to 1.5 and a geometric standard deviation equal to 1.35 and is applied for all cancer sites except leukemia for which the model is linear quadratic. The DDREF is applied by for chronic exposures (i.e., exposures to low dose rates). For acute exposure, a DDREF is applied only when a given equivalent dose is less than an uncertain reference dose, DL. At doses > DL, DDREF is assumed to be unity. The uncertain dose, DL, below which a DDREF is applied, is described by a loguniform probability distribution between 0.03 and 0.2 Sv.
Propagation of Uncertainties
Uncertainties are propagated using analytical methods	Uncertainties are propagated using Monte-Carlo techniques (Latin Hypercube sampling) for all cancer types except leukemia, for which a combination of analytical and Monte- Carlo methods is used.
Estimated lifetime risks incorporate uncertainties in:    - Risk models    - Transfer between populations    - DDREF	Estimated lifetime risks incorporate uncertainties in:    - Radiation doses    - Risk models    - Adjustment for minimal latency period    - Transfer between populations    - DDREF    - Baseline incidence rates.
The uncertainty in the total risk obtained by summation across cancer types is based on the BEIR VII risk model for “all solid cancers”.	The uncertainty in the total risk obtained by summation across cancer types is based on Monte-Carlo calculations which specifically account for correlations among different components in the summation.