Changing hydration level in an internal cavity modulates the proton affinity of a key glutamate in cytochrome c oxidase

Significance

Cytochrome c oxidase is an important proton pump that utilizes the chemical energy released by oxygen reduction to generate the transmembrane proton concentration gradient. A conserved glutamate residue has been proposed to play a key role in proton pumping, although factors that control the timing and destination of proton transfers by this residue remain poorly understood. By integrating results from multiple computational methodologies, we propose a mechanism in which changes in local hydration and electrostatic interactions regulate the proton affinity of this key residue and, as a result, proton transfer activities. The results highlight the functional significance of local protein motions and hydration state of internal cavities.

Abstract

Cytochrome c oxidase contributes to the transmembrane proton gradient by removing two protons from the high-pH side of the membrane each time the binuclear center active site is reduced. One proton goes to the binuclear center, whereas the other is pumped to the low-pH periplasmic space. Glutamate 286 (Glu286) has been proposed to serve as a transiently deprotonated proton donor. Using unrestrained atomistic molecular dynamics simulations, we show that the size of and water distribution in the hydrophobic cavity that holds Glu286 is controlled by the protonation state of the propionic acid of heme a₃, a group on the proton outlet pathway. Protonation of the propionate disrupts hydrogen bonding to two side chains, allowing a loop to swing open. Continuum electrostatics and atomistic free-energy perturbation calculations show that the resultant changes in hydration and electrostatic interactions lower the Glu proton affinity by at least 5 kcal/mol. These changes in the internal hydration level occur in the absence of major conformational transitions and serve to stabilize needed transient intermediates in proton transport. The trigger is not the protonation of the Glu of interest, but rather the protonation of a residue ∼10 Å away. Thus, unlike local water penetration to stabilize a new charge, this finding represents a specific role for water molecules in the protein interior, mediating proton transfers and facilitating ion transport.

Water is essential to the structure, dynamics, and function of biomolecules (1, 2), and its role in protein folding, association (3), and dynamics (4, 5) has been well documented. The highly polar and polarizable water molecules play diverse roles in protein interiors. Water can aid catalysis in enzyme active sites (6–8). Water or water chains are often observed in proteins that are (9, 10) proton or ion transporters or pumps (11–14). Internal cavities holding functional water molecules are believed to have a fairly constant level of hydration throughout the protein reaction cycle, unless significant conformational changes occur (15). Water penetration in response to the ionization or reduction of internal groups has been extensively discussed (16, 17), although it is usually described as part of protein's local dielectric response.

Cytochrome c oxidase (CcO) adds to the transmembrane proton gradient through proton transport coupled to electron transfer reactions (12, 18, 19). In the overall reaction, electrons from four cytochromes c are transferred to oxygen to make two water molecules at the binuclear center (BNC). The four protons needed for chemistry are bound only from the high-pH, N side of the membrane. Coupled to the process, four more protons are transferred across the membrane from the high- to low-pH (P) side of the membrane. Thus, eight charges are transferred across the membrane as each O₂ is reduced.

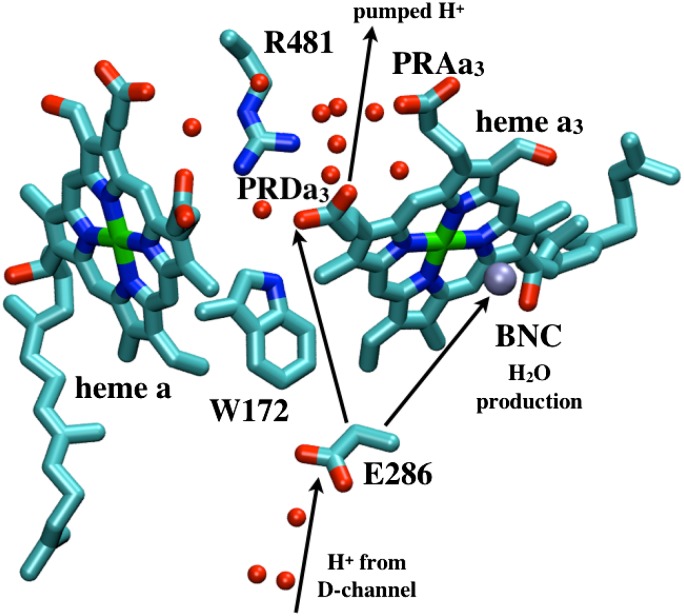
Glu286 is a required, conserved residue that is expected to transfer protons from the D channel either to the BNC or the proton-loading site (PLS) each time CcO is reduced (Fig. 1). Experiments assign a functional pK_a to Glu286 near 9.4 (20). Thus, at higher pH, proton binding to the Glu becomes rate-limiting for steady-state turnover. The current understanding of the reaction cycle shows that protons are pumped in each of the four distinct BNC redox states (12, 18, 19). The reaction mechanism needs Glu286 to be deprotonated twice to pass a proton to the PLS and to the BNC in each CcO reduction step. Previous continuum electrostatics (21–24) and semimacroscopic (25, 26) calculations obtained pK_a values for Glu286 near 9–10. However, recent microscopic calculations have found significantly higher pK_a values of more than 12 (17, 27), making it unclear how a proton could be lost from this site, whereas others do not address the proton affinity of the essential Glu (28, 29). The discrepancy between experiment and simulations may result from technical issues such as the use of static protein structures and limited sampling of protonation states of titratable groups, or it may arise from changes in the protein that have been missed. Thus, a key question remaining is how the proton affinity of this essential Glu is modulated so it can donate a proton to the PLS and the BNC through the reaction cycle.

Fig. 1.

Illustration of key residues near the hydrophobic cavity in CcO and general proton pathways to and from Glu286.

In this work, computational studies show the hydration level of an internal cavity near Glu286 changes substantially without needing global conformational changes. Rather, the structure of an internal loop is controlled or anchored by the protonation state of the D-propionic acid of heme a₃. This potentially important motion has not been noted in previous computational studies in which part of the protein structure was constrained (21, 27, 28). Both continuum electrostatics and quantum mechanical/classical mechanical (QM/MM) free-energy simulations show that the resultant changes in Glu286 hydration level and electrostatic interactions significantly affect its pK_a (proton affinity). These findings point to a molecular mechanism to modulate the timing of proton transfers in the CcO proton pumping cycle by modifying the proton affinity of this key acid. More generally, the results show that changes in protein internal hydration may occur with only small, distal conformational changes, and these can serve as an important regulatory mechanism in ion transport, thus going beyond being part of generic dielectric response of proteins.

Results and Discussion

Hydration Level of the Hydrophobic Cavity Near Glu286 Depends on the Protonation State of the Heme a₃ Propionate D.

The hydrophobic cavity that bridges Glu286 and propionate D of heme a₃ (PRDa₃), ∼10 Å away, is a functionally important region in CcO (Fig. 1). It is surrounded by the key cofactors: heme a, heme a₃, and Cu_B. The latter two form the BNC, which catalyzes the reduction of molecular oxygen to water, providing the overall thermodynamic driving force for proton pumping. No water molecules are observed in the cavity around the Glu in the various crystal structures of CcO from different organisms (SI Appendix, Table S2), although it is assumed that they will be needed to mediate proton transfers through this region. Disordered and dynamic water molecules are hard to see in crystal structures (30, 31). Molecular dynamics (MD) simulations typically find four to five molecules in the region (27, 29, 32–34), especially following Glu ionization (17). However, most previous simulations sampled relatively short times, often did not include a detailed membrane or solvent environment, and most importantly constrain a significant number of atoms to their crystallographic positions.

Here we have carried out a comparison of the hydrophobic cavity near Glu286 in different CcO chemical states in fairly long timescale (multiple 15–50 ns) unconstrained atomistic MD simulations in an explicit membrane environment. We focus on four key substates in the P_R → F transition, which has been extensively characterized by experiments (12, 18, 19). The four states are denoted: P_R, 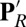, , 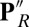and ′F. Analyses considering different force field parameters (27, 35), including the effect of electronic polarization (16), and conditions for the MD simulations test the robustness of the results (SI Appendix).

The P_R state has the Glu protonated and the PRDa₃ ionized. In 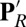the proton has transferred from Glu, which is now ionized, to the now neutral, protonated PRDa₃. In 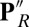, reprotonation of the Glu leaves both acids protonated. In ′F the acids maintain the 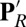protonation states, but a proton is added to the hydroxyl on Cu_B, representing proton transfer into the BNC for oxygen reduction chemistry. Thus, P_R explores the initial CcO protonation state, 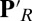, the one after a proton has moved to the pumping site, and ′F, the one where the proton has transferred to the BNC, before proton release from the PLS. The identity of the proton-loading or pumping site is unknown. Likely candidates are the heme a₃ propionates (19, 25) or a His ligand of Cu_B (36). Here we take PRDa₃ as the PLS because it is spatially closer to Glu286 and the D channel, which mediates the transfer of protons taken up from the N side of the membrane (Fig. 1). The BNC is fixed in the specific redox states here (Table 1). However, this should not be critical, as proton pumping is posited to occur via the same mechanism in all CcO redox transitions (18, 19, 37).

Table 1.

Computed Glu286 proton affinities (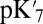 and pK_a) using continuum electrostatics (SCCE, MCCE) and microscopic QM/MM thermodynamic integration

Cavity	Input structures*	State†	QM/MM	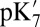SCCE 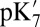‡	MCCE 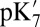‡	MCCE titration pKa‡
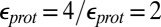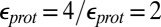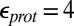Small	1M56	XDD–RO	18.5 ± 0.6	10.2 ± 0.7/15.1 ± 1.6	11.4 ± 1.0/18.0	14.1 ± 1.5
Small	1M56	XPD–RO	–	−/−	9.7 ± 0.9 /15.9	12.4 ± 1.8
Small	1M56 + 9w	XDD–RO	14.0 ± 0.6	−/−	−/−
Small	1M56 + 9w	XPD–RO	11.2 ± 0.9	−/−	−/−
Large	PBC′F	XDD–RO	14.3 ± 0.8	8.7 ± 0.8/11.8 ± 1.6	9.3 ± 0.7/12.8	11.1 ± 1.4
Large	PBC′F	XPD-RO	10.6 ± 0.7	6.5 ± 1.2/8.3 ± 2.7	7.7 ± 0.8/10.7	7.5 ± 1.0
pK Changes§
Dependence on PRDa3 protonation
1M56			–	−/−	1.7/2.1	1.7
1M56+9w			2.8	−/−	−/−	–
PBC′F			3.7	2.2/3.5	1.6/2.1	3.6
Dependence on cavity hydration
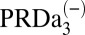
			4.2	1.5/3.3	2.1/5.2	3.0
PRDa3H			–	−/−	2.0/5.2	4.9
Combined effect			7.6	3.7/6.8	3.7/7.3	6.6

QM/MM and SCCE 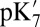values are calculated with the protonation states for all other titratable groups fixed at their equilibrated protonation states found in MCCE calculations (23) at pH 7 (SI Appendix, Table S4); the MCCE 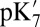and pK_a calculations allow the protonation states for all titratable groups other than those specified in SI Appendix, Table S1, to equilibrate at each pH. SI Appendix provides additional computational details and analyses. , , 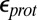dielectric constants for the cavity and protein; GSBP, generalized solvent boundary potential; MCCE, multiple-conformer continuum electrostatics; PBC, periodic boundary condition; PRDa₃, propionate D of heme a₃; SCCE, single-conformer continuum electrostatics; w, water molecules.

^*Local GSBP simulations start with different initial coordinates. 1M56: the crystal structure; 1M56 + 9w: nine additional water molecules are included near the cavity; PBC′F: an equilibrated snapshot from PBC simulation for the ′F state.

^†The states are labeled with a five-character notation. The first three letters indicate the protonation state (protonated or deprotonated) of Glu286, propionate D of heme a₃ (PRDa₃), the ligand of Cu_B [hydroxide (D) or water (P)]. The last two letters indicate the reduction state (reduced or oxidized) of heme a and Cu_B, respectively. “X” indicates 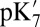simulations in which the protonation state of Glu286 is varied.

^‡The 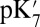values before and after the slashes are computed with 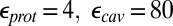and 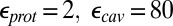, respectively. Results with other values for the dielectric constants are in SI Appendix, Table S12–S13. For the MCCE pK_a calculations, 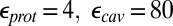is always used.

^§The effects of cavity size and protonation of PRDa₃ are calculated based on the computed 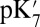and pK_a values. The combined effect is obtained by taking the difference between pK values computed with a small cavity (low hydration) with 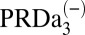and a large cavity (high hydration) with PRDa₃H.

Hydration of the Cavity Near Glu286.

The hydration levels of the cavity in different chemical states of CcO are compared in several ways. It is very small in the unconstrained MD trajectories for the P_R state, with Glu286 protonated and PRDa₃ deprotonated, similar to that found in CcO crystal structures (SI Appendix, Fig. S3 and Table S2). The free volume found by a probe sphere of 1.4-Å radius is near zero. The continuum solvation energy penalty for Glu ionization in MD snapshots is 6.0 ± 0.5 kcal/mol. Although the MD structure starts with five water molecules in the cavity, they diffuse away in nanoseconds. In independent, 15–50-ns trajectories, there are typically only two water molecules left in the cavity by the end of the simulation. By contrast, the cavity is full of water molecules in both the 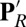and ′F simulations, each of which have moved a proton off Glu286 onto PRDa₃ (Fig. 2). The hydration level is slightly higher in the ′F state where the BNC has an extra proton. The MD trajectories now keep 8–10 water molecules in the cavity, whose volume has expanded to ∼155 ± 21ų (SI Appendix, Fig. S3, for an illustration). The Glu continuum solvation penalty for ionization is now only 3.9 ± 0.5 kcal/mol. Thus, the protonation of the Glu and PRDa₃ changes the hydration level (Fig. 3) with a small, dry cavity in the P_R state and a large, hydrated cavity in the 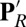and ′F states. These are referred to as the small- and large-cavity structures in the following.

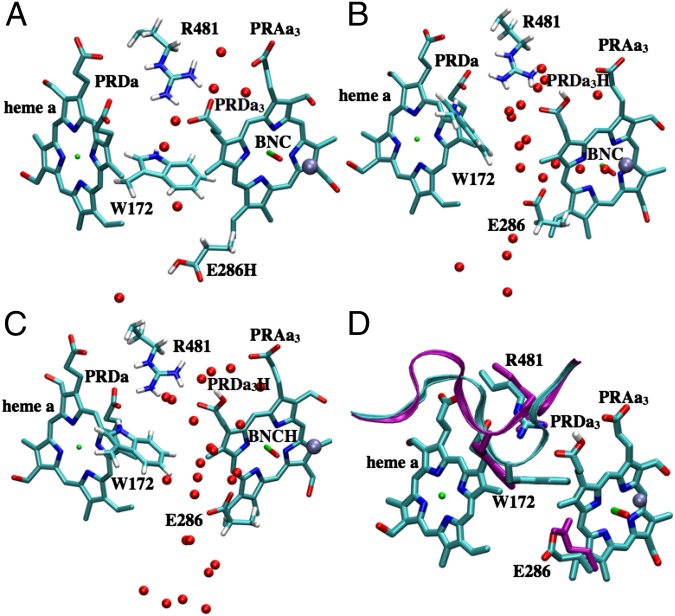
Fig. 2.

Snapshots from unconstrained PBC–MD simulations illustrating the hydration level and local conformational changes of the hydrophobic cavity near Glu286 in different chemical states. (A) P_R; (B) 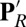; (C) ′F; (D) Superposition of snapshots from P_R (loop165–177 in cyan with Glu286 protonated, PRDa₃ deprotonated) and 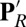(purple loop with Glu286 deprotonated, PRDa₃ protonated) showing that the overall structure does not undergo any major changes, whereas the loop that bears Trp172 moves significantly in response to the protonation of PRDa₃. SI Appendix, Fig. S5, provides data from additional CcO states and SI Appendix, Table S1, for the protonation or oxidation states of key groups in the various enzyme states.

Fig. 3.

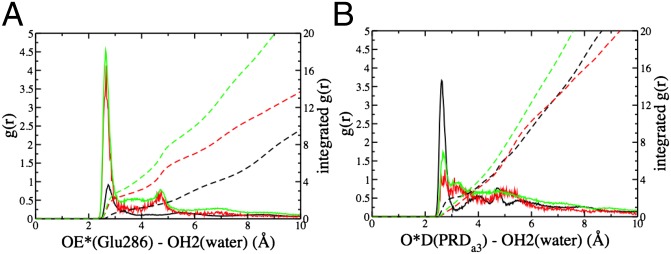
Radial distribution (solid lines) and integrated radial distribution (dashed) of water oxygens in PBC simulations for different CcO states (black: P_R; red: 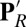; green: ′F): (A) around carboxylate oxygens of E286; (B) around carboxylate oxygens of PRDa₃. SI Appendix, Fig.S5, provides data from additional CcO states.

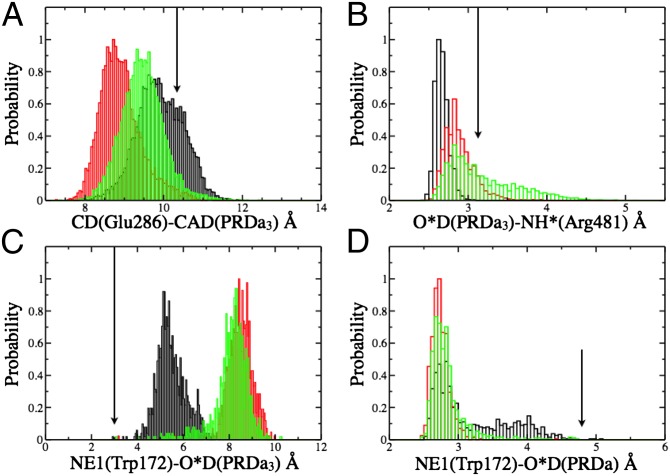
The cavity contacts explain how its size and hydration level are changed. As in the crystal structures, in the P_R state, the charged PRDa₃ engages in stable hydrogen-bonding interactions with the side chains of Arg481 and Trp172 (Figs. 2A and 4). Moreover, Glu286 is charge-neutral and so does not stabilize the accumulation of a significant number of water molecules. These features lead to a dehydrated compact cavity. In contrast, in 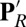and ′F, PRDa₃ is protonated and overall neutral, with weaker interactions with Arg481 and Trp172. This Trp is in a loop with a highly conserved sequence motif GxGxGWxxYxPL (SI Appendix, Fig. S2). The PLS region is constantly more mobile in MD trajectories with a protonated PRDa₃ as monitored by the distribution of the distance between PRDa₃–Arg481 and PRDa₃–Trp172 (Fig. 4). Previous MD simulations where Arg481 has been mutated to a Lys show similar changes in this loop (32). Simulation of a 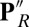state where both Glu286 and PRDa₃ are protonated also leads to an expanded, solvated cavity (SI Appendix, Table S1 and Fig. S5), supporting a model where the protonation of PRDa₃, rather than deprotonation of Glu286, triggers changes in the cavity.

Fig. 4.

Comparison of key distance distributions for residues near the hydrophobic cavity calculated for different chemical states of CcO with PBC simulations (black: P_R; red: 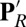; green: ′F). (A) E286–PRDa₃; (B) R481–PRDa₃; (C) W172 side chain-PRDa₃; (D) W172 side chain-PRDa. The arrows indicate the distances in the crystal structure (PDB code 1M56). SI Appendix, Fig.S5, provides data from additional CcO states.

A comparison of structures from these unconstrained simulations with explicit membrane indicates that the significant changes in the hydration of the hydrophobic cavity do not need global conformational transitions. The RMSD of subunit 1 that contains the active centers is less than 1.4 Å. Local structural flexibility, however, is important. In particular, rearrangement of the loop that bears Trp172 (32) is essential for water penetration into the cavity (Fig. 2D). For example, in local, generalized solvent boundary potential (GSBP) MD simulations where part of this loop is constrained to the crystallographic position, no water molecules penetrate or leave the cavity on the nanosecond timescale in either 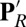or ′F simulations (SI Appendix, Figs. S6–S8).

pK_a and 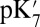of Glu286.

To understand the functional implication of the hydration level differences in the large and small-cavity structures, the proton affinity of Glu286 is computed with protonation state of PRDa₃ and the BNC fixed to define the states of interest. The free energy of ionization of an acid (A) when the ionization states of all other titratable groups are equilibrated at pH 7 is

Thus, 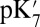, reported here, represents a transient energy for deprotonating the Glu, as the other groups remain out of equilibrium with the change in Glu charge. The Glu286 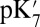is estimated with multiple computational approaches (Table 1) that include both microscopic [QM/MM–thermodynamic integration (TI) (27, 38)] and continuum electrostatic methods [single-conformer continuum electrostatics (SCCE) (39, 40) and multiple-conformer continuum electrostatics (MCCE) (41)]. The range of 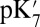determined with the different methods highlights the difficulty of computing the absolute proton affinity of a deeply buried group in large transmembrane proteins like CcO (17). Therefore, our approach is to compare the results of the very different computational methodologies and identify consistent trends (SI Appendix provides additional details and analyses). Finally, the true pK_a is also determined with MCCE titration, a process that keeps the protonation states of all residues at equilibrium with the imposed solution pH.

Dependence of the Glu286 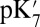on the Cavity Size.

Calculations will first be described in the P_R-like XDD–ROg state (defined in Table 1 and SI Appendix, Table S1), with a deprotonated PRDa₃, which has a small cavity in both local (GSBP) and unconstrained [periodic boundary condition (PBC)] simulations. These structures remain close to the crystal structure 1M56 (42), and so the results can be more readily compared with previous calculations (17, 27). Regardless of the simulation technique used, ionization of Glu286 is very unfavorable at pH 7. The 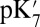ranges from 18.5 with the QM/MM–TI technique to 10.2 using SCCE with a protein dielectric constant 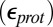of 4, whereas MCCE calculates a value of 11.4. MCCE calculates a true pK_a of 14.1. This value is substantially higher than the MCCE 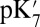because pK_a is calculated with all residues remaining in equilibrium with the pH, so the protein is much more negative overall. Using an 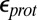of 2, which has been recommended when multiple conformations from MD simulations are used (40), gives a 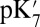of 15–18 in MCCE or SCCE calculations, closer to that found with the microscopic QM/MM–TI technique.

The 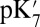is also calculated imposing the same XDD–ROg charge in structures generated by unconstrained simulations in the ′F state, which result in large cavities. The calculated 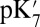is lowered significantly with all methods (Table 1). The drop is 3.3 pH units (4.5 kcal/mol) using SCCE calculations with 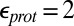and ∼2 pH units (2.7 kcal/mol) with MCCE, 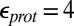. The MCCE titration pK_a drops by ∼3 pH units to 11.1. Thus, opening the cavity moves the free energy required to deprotonate the Glu to near the functional, experimental value (20). The high pK_a indicates the Glu will be neutral at physiological pH.

Microscopic, QM/MM–TI 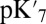Calculations and the Effect of Cavity Hydration.

In local MD simulations of the crystal structure (GSBP–1M56), the cavity remains occupied with approximately five water molecules throughout the thermodynamic integration simulations, giving a high 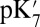of 18.5. Glu286 becomes better solvated as it becomes increasingly negative (as the titration coordinate λ approaches 1), drawing in water molecules from both the cavity and top of the D channel (SI Appendix, Fig. S11). The TI 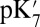drops by 4.2 pH units to ∼14 in large-cavity structures, a shift that is similar to that found in the SCCE or MCCE continuum electrostatic calculations (Table 1). The importance of cavity water molecules is seen when nine extra water molecules (9w) are added to the small-cavity structures (1M56 + 9w). In the short (∼1–3 ns) local MD simulations, the water molecules cannot escape but relax as best they can in the small cavity. The QM/MM–TI 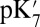in the overly hydrated small cavity are now close to that found in the equilibrated, well-hydrated large cavity, showing the cavity decreases the 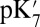primarily by solvating the ionized Glu286. The 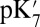value of ∼14 is similar to that obtained by Chakrabarty and Warshel using a novel approach that adds more water molecules to the cavity as Glu286 titrates (17).

Dependence of the Glu286 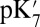on the PRDa₃ Ionization State.

The Glu286 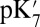and pK_a are calculated with different methods in structures with large and small cavities with the protonation state of PRDa₃ (and the BNC) fixed. In all structures, removing the −1 charge from this acid, ∼10 Å from Glu286, reduces its 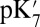by 1.6–3.7 pH units, indicating the proton affinity has dropped by at least 2 kcal/mol. The nature of the shift is independent of the type of calculation or the size of the cavity near Glu286. The cost of deprotonating the Glu is thus seen to be affected independently and by a similar, significant amount by the opening of the cavity and by the protonation of PRDa₃ (Table 1).

The results found here support a model where a large cavity will be found when PRDa₃ is protonated and a small one when it is ionized. The Glu286 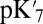shifts by 3.7–7.6 pH units when the PRDa₃ is protonated and the cavity expanded (Table 1), indicating the small change in CcO structure decreases the Glu proton affinity by at least 5 kcal/mol. The MCCE titration pK_a shifts to 7.5 in the large-cavity PRDa₃ neutral state, indicating that the Glu would be ∼half-ionized at equilibrium at pH 7 under these transiently existing conditions. Now the proton affinity of the BNC does not need to be very high to receive a proton from Glu286 (43).

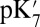Values Calculated with Different Methods and Input Parameters.

The different methods for calculating 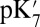and pK_a yield a consistent picture that the hydration and electrostatic properties of the hydrophobic cavity control the proton affinity of Glu286. The ground state structure increases the proton affinity by ∼3 kcal/mol due to the small cavity and by another ∼3 kcal/mol because PRDa₃ is ionized. The Glu proton affinity decreases significantly when PRDa₃ is protonated, and the cavity expands, as expected when the PLS is ready for pumping.

The absolute 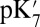values predicted by different methods differ substantially. For example, given a small cavity and ionized PRDa₃, the 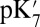varies by 8.3 pH units, a 11.4 kcal/mol difference in the calculated proton affinity (Table 1). The found with SCCE and MCCE methods depends on 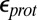and 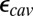, the dielectric constants for the protein and cavity. As discussed in previous work (40, 44), a lower 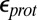(e.g., 2) may be appropriate for 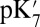calculations when the protein structure is equilibrated with different protonation states for the titratable group in the linear response framework. The precise value for 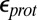would depend on the degree of sampling (45, 46). In addition, water molecules in protein cavities may be more constrained than in bulk (47), so that 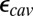may be less than 80. Using PBC trajectories and the Kirkwood–Fröhlich formalism (48), the local dielectric constants of the hydrophobic cavity and D channel range from 4 to 9 in the chemical states studied here (SI Appendix, Table S3). Although one should be cautious about using such computed local dielectric constants in 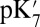calculations (46), these values suggest the cavity may be surprisingly rigid even in the large, hydrated conformation. As detailed in Table 1 and SI Appendix, Tables S12–S13, as 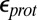and 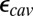are varied, the Glu286 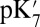estimated by both SCCE and MCCE change significantly and by similar amounts. Lowering 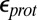while maintaining a high (80) 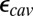, the estimated 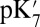of Glu286 increases by a few pH units, and the impact of the cavity size and protonation of PRDa₃ become closer to that predicted by QM/MM–TI. When 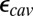is reduced to 4–9, the impact of the cavity size on the Glu286 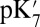becomes smaller as expected, whereas the effect of PRDa₃ protonation remains ∼3–4 pK_a units. Thus, whereas it is difficult to establish the absolute 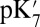, SCCE/MCCE calculations with 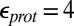and 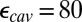likely lead to the lower limit for this crucial value.

Implication for the CcO Proton-Pumping Mechanism.

Despite decades of experimental and theoretical analyses, it remains unclear how CcO couples the redox chemistry of O₂ reduction to the transport of eight charges across the protein to add to the transmembrane proton gradient. Oxygen chemistry occurs in the R to P transition, with four electrons accumulated by CcO in previous intermediates now transferred to O₂, without generating other reactive oxygen intermediates. The oxidized protein is then rereduced back to the R state through donation of four electrons from cytochrome c. Concomitant with each redox reaction, one proton is transferred to the pumping site, here assumed to be PRDa₃, to be pumped into the P side of the membrane. Another proton is delivered to the BNC.

The mechanism that controls the branching competition between proton transfer to the pumping site or the BNC is not understood (43). It must reflect changes in the proton affinity of the donor and acceptor sites, particularly Glu286, the pumping site, and the BNC (19, 25), as well as control of the proton transfer pathways (18, 37). Using a kinetic network model for CcO, Hummer and coworkers (49, 50) analyzed trends in the proton transfer rate constants that would lead to efficient pumping. Their analysis supports proton transfer to the BNC being gated by protonation of the pumping site. This is consistent with the results found here where the protonation of PRDa₃ increases the thermodynamic driving force for proton transfer to the BNC by reducing the Glu286 .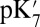

The proton affinity of the BNC and pumping site will be affected by the chemical states of the enzyme. For example, each CcO reduction step goes through a stage when heme a is reduced and BNC oxidized. Heme a reduction increases the pumping site 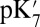, favoring proton transfer there rather than to the BNC, whereas electron transfer from heme a to the BNC increases the BNC proton affinity, attracting the proton from Glu286. This may be important in some BNC reduction steps such as from ferric to ferrous heme which do not have a strong thermodynamic driving force for coupled proton uptake (43).

Despite very different approximations and limitations, our continuum electrostatic and microscopic calculations show that the proton affinity as monitored by the 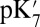of Glu286 is unusually high when the presumed pumping site (PRDa₃), 10 Å away, is deprotonated (e.g., in P_R). The high pK_a and 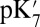is due to the Glu being in a dehydrated, hydrophobic cavity. In contrast, when PRDa₃ is protonated (as in 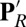, , 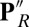and ′F states here), its hydrogen-bonding interactions with Arg481 and Trp172 weaken, leading to the displacement of the loop-bearing Trp172 and an expanded, more solvated cavity. Both continuum electrostatics and microscopic calculations indicate that these changes in hydration and local electrostatics lead to substantial depression of Glu286 pK_a to the experimentally measured range of 9–10, which was estimated based on a specific kinetic model (20, 26). Thus, it is the proton affinity of Glu286, not the BNC, that is modulated by the loading of the pumping site. This is an attractive model (SI Appendix, Fig. S12) as it requires that proton transfer to the pumping site precede that to the BNC, a feature that would minimize the amount of “slippage,” where chemistry is done without pumping. The model also provides a microscopic framework for the kinetic gating phenomena identified in the kinetic network analysis (50) and discussed previously (51, 52). Although our calculations focus on states implicated in the P_R to ′F transition, because the key driving force for the Glu286 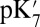modulation is protonation of the presumed pumping site (PRDa₃), it does not depend on the specific chemical state of the BNC. Thus, this mechanism for raising and lowering the Glu286 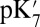can be repeated each time CcO is reduced.

A role for changing hydration in determining proton or electron transfer activities has been considered as one general mechanism to modulate the proton affinity of buried charges (16, 44). A specific role of water penetration has been proposed to influence the Glu286 pK_a (17). The current study is distinct in that it captures a specific local loop motion coupled to the protonation of a remote (10 Å from Glu286) group that triggers the change of cavity hydration level. This in turn modulates the proton affinity of Glu286, thus potentially establishing the molecular mechanism that controls the hydration level and proton affinity of this key residue.

Conclusion

In the course of the reaction cycle, Glu286 is presumed to donate a proton to the proton-loading site and the BNC. This requires modulation of the acid’s proton affinity twice. The work here presents a hypothesis that provides a solution to half the problem, given that PRDa₃ is the PLS. The results of unrestrained MD simulations show that when the loading site is protonated, the 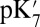of Glu286 is significantly depressed, making it more favorable to transfer a proton to the BNC. The mechanism provides both a thermodynamic push for the proton transfer and controls the sequence of events so that the BNC will not receive its proton until the pumping site is loaded. The hypothesis provides a challenge to experiment, to detect a cavity of ∼155 ų that might be expected to live for the millisecond timescale associated with each pumping step (19). In addition, the importance of this loop suggests a region for mutations near Trp172 (53) that may have a significant impact on the pumping efficiency and/or the rate of proton transfer to the BNC.

However, the mechanism for decreasing the proton affinity of Glu286 does not provide an answer to how the relative proton affinities are modified so that PRDa₃ becomes protonated initially. Indeed, QM/MM calculations found that the direct proton transfer from Glu286 to a deprotonated PRDa₃ is energetically very unfavorable in a P_R-like state. The energetics for an alternative “concerted transfer” mechanism that involves the participation of an additional proton in the D channel (54) may be more consistent with kinetic data for the P → F transition. Previous empirical valence bond calculations also thoroughly discussed direct vs. concerted proton transfers (55) and emphasized the importance of including Glu286 flexibility (25, 26). We hope the impact of the surprising cavity opening reported here stimulates additional experiments and simulations to dissect robust elements that modulate the proton transfers.

Materials and Methods

The MD and QM/MM–TI simulations use the same protocol as in our previous studies (27, 56). The MCCE calculations use parameters and methods fully described in Refs. 23 and 57. SI Appendix provides more complete descriptions and additional simulation results.