Biomechanical comparison of laminectomy, hemilaminectomy and a new minimally invasive approach in the surgical treatment of multilevel cervical intradural tumour: a finite element analysis

Abstract

Purpose

The objective of this study was to investigate the impact of the less invasive procedures of hemilaminectomy and unilateral multilevel interlaminar fenestration (UMIF) on the cervical spinal biomechanics.

Methods

A validated nonlinear finite element model of the intact cervical spine (C2–C7) was modified to study the biomechanical changes as a result of surgical alteration for treatment of intradural tumours at C3–6 using multilevel laminectomy (ML), multilevel hemilaminectomy (MHL) and UMIF with or without unilateral graded facetectomy.

Results

Under the load-controlled method, the greatest biomechanical changes occurred at the surgical segments. The largest increases occurred in flexion motions following ML approach with 70, 62 and 60 % increase at C3–4, C4–5 and C5–6, respectively. The increases were significantly reduced to no more than 14 % under MHL and UMIF. When combined with graded facetectomy, the changes in flexion under ML approach have a significantly further increase, up to 110 % at C3–4. The further increase was not significantly following MHL and UMIF, with no more than 31 % increase at C3–4, C4–5 and C5–6. The motion following UMIF was only slightly smaller in axial rotation than MHL. The maximum stresses in the annulus occurred during flexion in ML model, with 39, 34 and 38 % more stress than the intact at C3–4, C4–5 and C5–6, respectively. The increases of stress were significantly reduced to 5–7 % under MHL and UMIF.

Conclusions

The less invasive approaches of UMIF and MHL greatly preserved the flexion motion (more than 48 %) of the cervical spine compared with laminectomy, and the preserved motion mean the low-risk of postoperative spinal instability. UMIF and MHL also reduced the increased stress of annulus caused by ML, and the lesser stress will lower the risk of postoperative disc degeneration. The posterior bone elements play a slight role in spinal stability after removal of the attached ligaments.

Introduction

Laminectomy is the traditional approach for intradural tumours, and involves dissection of bilateral paraspinal muscle and removal of the posterior spinal elements, including the vertebral laminae, the spinous processes, the ligamentum flavum, and the supraspinous and interspinous ligaments. However, the removed structures are very important for the stability of the spine, and clinical investigations have constantly reported that laminectomy leads to spinal instability and deformities in patients with intradural tumours [1–3]. In high-risk populations, the reported rates of post-laminectomy deformity varied from 27 % [2] to as high as 100 % [1].

Since 1980s, hemilaminectomy has been described in the microsurgery of spinal intradural tumours, and it is generally considered to have great advantages in preserving spinal stability [4–7]. The biomechanical superiority of hemilaminectomy had been investigated in lumbar finite element (FE) model [8]. However, no biomechanical data exist in evaluating the benefit of hemilaminectomy in cervical spine, where the risk of post-laminectomy deformity is generally considered greater than in thoracic and lumbar spine [8, 9]. Unilateral multilevel interlaminar fenestration (UMIF) is a new minimally invasive approach for spinal lesions. It was first reported to be used for spinal tumours by Koch-wiewrodt, and has been proven to be safe and effective in acquiring both sufficient exposure of the tumours and long-term spinal stability [10]. In addition, UMIF preserves more structures than hemilaminectomy, and is assumed to be more stable. However, the biomechanical benefits from its considerable preservation of posterior column also remain unstudied.

Facetectomy is necessary to get a good exposure for many spinal intradural tumours, while it is also recognized as a risk of post-laminectomy deformities in cervical spine. The previous studies have investigated the biomechanical effects of facetectomy on the intact spine [11, 12]; however, its effects on the spine after surgical procedures need to be studied as well.

Acquiring the biomechanical effects of these surgical procedures allows surgeons to better prevent spinal instability. The objective of this study was to evaluate the biomechanical impact of the three surgical procedures with or without facetectomy in surgical treatment of cervical intradural tumours using FE method. Multilevel laminectomy means a higher risk of post-laminectomy deformities [2, 3, 13], and it is hypothesized that MHL and UMIF would also show advantages in multilevel spinal surgeries. So, we simulated these surgical procedures involving multi-segment in this study.

Materials and methods

The development and validation of the FE model

A 3-dimensional FE model of C2–C7 segment was developed and used in this study (Figs. 1a, 2). The model was developed from the computed tomographic (CT) scan of a 25-year-old healthy man (height 175 cm, weight 70 kg). The images of the CT were used to construct the geometrical surface model of each vertebra in software Mimics 10.01 (Materialise Inc., Leuven, Belgium). The geometrical surface of the vertebra was smoothed by a reverse tool of Geomagic Studio 12.0 (Geomagic, Inc., Research Triangle Park, NC, USA), and then the model was meshed as solid model in Hyperworks v10.0 (Altair engineering, Inc., Executive Park, CA, USA).

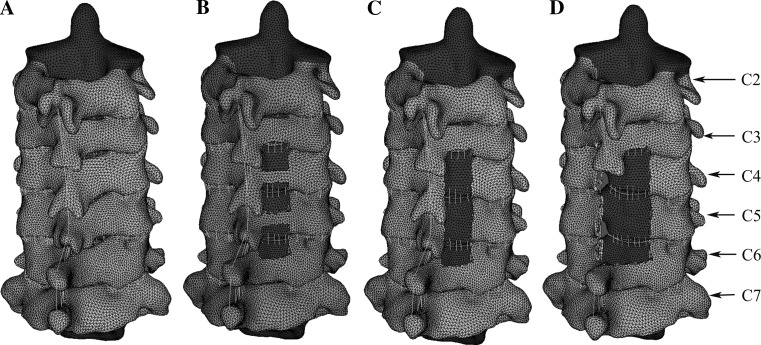
Fig. 1.

Posterolateral view of the intact model (a), UMIF model (b), MHL model (c) and ML model (d) (UMIF unilateral multilevel interlaminar fenestration, MHL multilevel hemilaminectomy, ML multilevel laminectomy.)

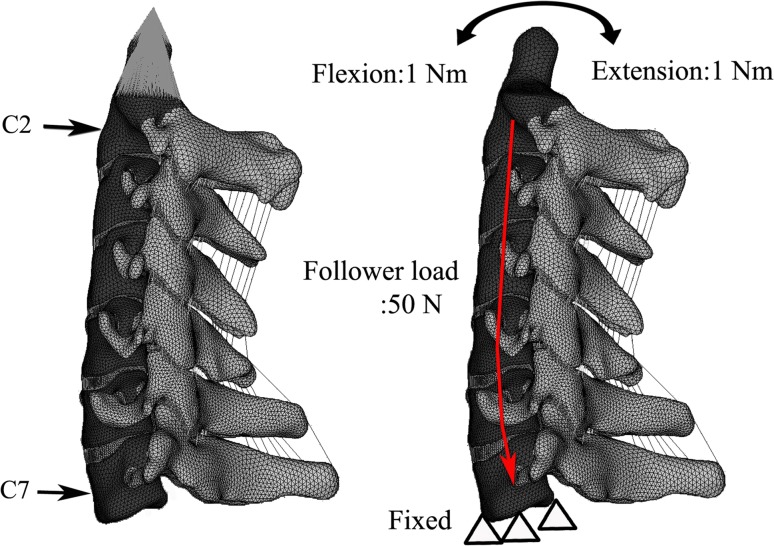
Fig. 2.

The lateral view of the intact finite element model of C2–7 segment. The flexion–extension moments were applied to the superior surfaces of C2. The inferior surface of the C7 vertebra was fully constrained

The vertebral bodies of the model consisted of the cortical bone, the cancellous bone, and the posterior bony structure. For modelling the cancellous bone and posterior elements of the vertebrae, 209735 4-noded solid tetrahedral elements C3D4 were used. The cortical bone and endplates vary in thickness between 0.35 and 0.89 mm in the previous literatures [14–16]. In this study, an average value of 0.5 mm was used for the thickness of endplate and cortical bone [17]. Solid elements were also used for simulating the cortical bone and the endplates [18], and they were composed of 74750 6-noded solid elements C3D6. The intervertebral discs were modelled between the vertebras based on qualitative anatomical data, and each was composed of disc annulus, disc nucleus and endplates. The disc annulus was modelled as fibers embedded in the ground substance. The fibers were modelled using 13009 tension-only linear contact elements. The fiber content in the annulus fibrosus was assumed to be approximately 20 % of the matrix volume and was positioned a mean of 25° against the horizontal plane [19]. The annulus matrix and nucleus were simulated using 54535 advanced 8-noded solid elements with reduced-integration method, because these type of elements are very accurate in calculating the displacement.

Five major ligaments mimicking the ligamentous structures in the cervical spine were incorporated into the model, including the anterior longitudinal, posterior longitudinal, capsular ligaments, ligamentum flavum, and interspinous ligament. To simulate these ligaments, groups of 35, 35, 100, 35 and 35 tension-only nonlinear spring elements, respectively, were introduced between the anatomical insertion areas. Spring elements can use a force–deflection curves material property input [20], so it can well-simulate the nonlinear response of the ligaments. The facet joints were modelled as 24401 frictionless surface-to-surface contact elements. The final FE model can be seen in Fig. 2. The types of elements that were used in the present model are similar to the previous FE models [17, 19, 21]. In a certain range, increasing the number of mesh elements can improve the accuracy of the model and realize the smooth surface of the vertebrae. Thus, a relatively large number of elements were used in our model. Accuracy analysis was also performed to assure the accuracy of the model, and the number of elements was proved to be optimal by accuracy analysis.

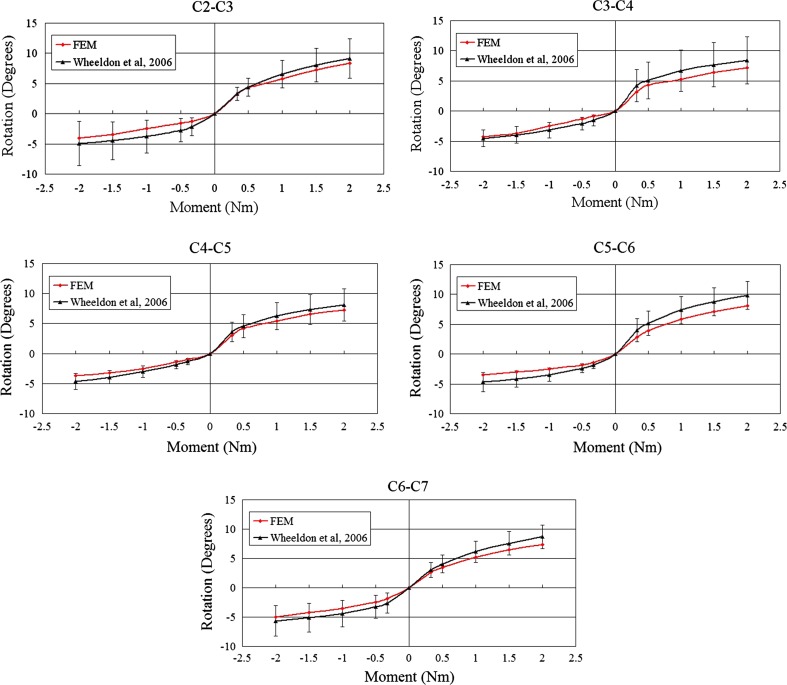
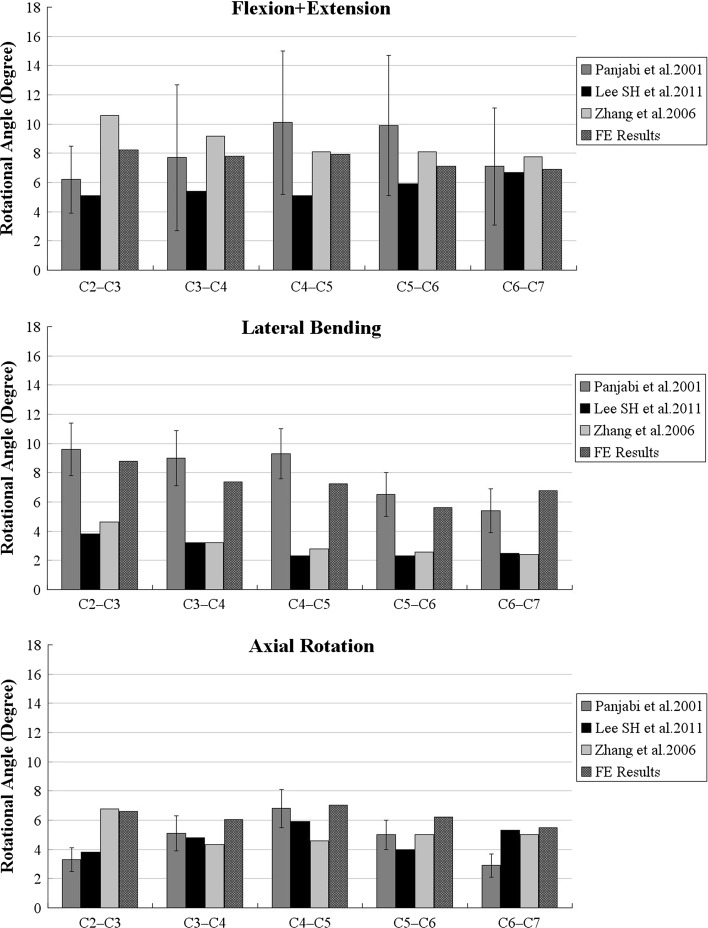
The detailed values for various materials are tabulated in Table 1, which are the most commonly used values obtained from the literature [19–23]. The analysis was performed using the FE software ABAQUS v6.9.1. (SIMULIA Inc, Providence, RI, USA). For validation of the FE model of C2–C7, the model was loaded in two different quasi-static loads. First, pure flexion–extension moments of 0.33, 0.5, 1.0, 1.5 and 2 Nm were applied to the superior surfaces of C2 with the C7 firmly fixed, and the results were compared with Wheeldon et al. [24]. By comparing with their results, the nonlinear response of the cervical spine to pure sagittal load could be proved (Fig. 3). Second, pure moments (sagittal, transverse, and frontal planes) of 1.0 Nm and a 50 N of compressive follower load were applied to the superior surfaces of C2 with the C7 firmly fixed (Fig. 2). The three plane motion was compared against the results of Panjabi et al. [25], Lee et al. [19], Zhang et al. [26] to assess the validity of the FE model (Fig. 4).

Table 1.

Material Property for the Human Cervical Spine Finite Element Model [14–17]

Description	Element type	Young’s modulus (MPa)	Poisson’s ratio
Cortical bone	3-D solid (6 node)	10,000	0.29
Cancellous bone	3-D solid (4 node)	100	0.29
Posterior elements	3-D solid (4 node)	3,500	0.4
Endplate	3-D solid (6 node)	500	0.29
Annulus ground substance	3-D solid (8 node)	3.4	0.4
Annulus fibrosus	Tension-only linear contact elements (2 node)	450	0.3
Disc–nucleus	3-D solid (8 node)	1	0.499
Ligaments	Nonlinear tension-only spring (2 node)

Anterior longitudinal		Posterior longitudinal		Spinous		Ligamentum flavum		Capsular
Deflection (mm)	Force (N)	Deflection (mm)	Force (N)	Deflection (mm)	Force (N)	Deflection (mm)	Force (N)	Deflection (mm)	Force (N)
C2–C5
−10	0	−10	0	−10	0	−10	0	−10	0
−1	0	−1	0	−1	0	−1	0	−1	0
0	0	0	0	0	0	0	0	0	0
1	28	1	25	1	7	2	38	2	55
2	52	2	44	2	12.5	4	60	4	130
3	72	3	62	3	18	6	80	6	180
4	89	4	78	4	22.5	8	108	8	210
5	102	5	89	5	26			10	230
6	115			6	30
				7	32.5
C5–C7
−10	0	−10	0	−10	0	−10	0	−10	0
−1	0	−1	0	−1	0	−1	0	−1	0
0	0	0	0	0	0	0	0	0	0
1	20	1	20	1	8	2	30	2	75
2	40	2	40	2	14	4	68	4	145
3	58	3	60	3	20	6	102	6	204
4	78	4	78	4	25	8	130	8	250
5	98	5	92	5	29	10	145	10	265
6	112			6	32.5
7	120			7	35

Fig. 3.

Flexion-extension results for C2–C7 and comparison with Wheeldon et al. (2004)

Fig. 4.

Comparison of the intersegmental rotation angle between the present simulations and the literature (Panjabi et al. 2001; Lee et al. 2011; Zhang et al. 2006) under the flexion–extension, lateral bending and axial rotation moments

FE surgical models simulation

The validated intact model was then modified to simulate the anatomical changes resulting from three different approaches to intradural pathology at C4–C5 with part of C3 and C6 involved: the UMIF approach (Fig. 1b), hemilaminectomy (Fig. 1c) and laminectomy (Fig. 1d). The three models were simulated as the reported clinical surgical procedures [7, 10, 27].

1. Unilateral multilevel interlaminar fenestration approach (UMIF): The upper and lower arches of the unilateral laminae (right side) from C4 to C5, the ipsilateral inferior part of the C3 laminae and the superior of the C6 laminae, the base of the spinous process and 4/7 of the ligamentum flavum at the surgical sites were removed.

2. Multilevel hemilaminectomy approach (MHL): From the UMIF, the bone bridges between the outer parts of the laminae and the spinous process were removed.

3. Multilevel laminectomy approach (ML): From the MHL, the spinous process, interspinous ligaments, all the ligamentum flavum and the contralateral lamina were removed.

Each of the three above model was also used to simulate the medialis removal of one-fourth and one-half of the right facet at C3–C4, C4–C5 and C5–C6 levels. So another six models were also formed: UMIF, MHL and ML with 25 % unilateral facetectomy; UMIF, MHL and ML with 50 % unilateral facetectomy.

Both the load-controlled method and “hybrid approach” were used for analysis of the surgical models. For the load-controlled method, pure moments (sagittal, transverse, and frontal planes) of 1.0 Nm and a 50 N of compressive follower load were applied to the nine models with the C7 firmly fixed. The intersegmental motions of the models were calculated in the three plane motion (sagittal, transverse, and frontal planes). The von Mises stress in the annulus at C3–4, C4–5 and C5–6 was also considered to be significant measurements of biomechanical changes between the surgical groups and are presented here. The “hybrid approach”, in which 50 N of follower load and pure moments were applied until the rotations across the C2–C7 segment became equal to the intact case, was also applied to the surgical models. Because of the enormous amount of data, the “hybrid approach” is limited to flexion results being clinically relevant. The moments for the surgical models that produced C2–C7 rotations almost equal to the intact model are tabulated in Table 2. The intersegmental motions were also calculated to compare with the intact model.

Table 2.

The flexion moments applied to the different surgical models during hybrid approach

The surgical model	Moment (Nm)
ML	0.691
MHL	0.927
UMIF	0.928
ML with 25 % unilateral facetectomy	0.632
MHL with 25 % unilateral facetectomy	0.882
UMIF with 25 % unilateral facetectomy	0.883
ML with 50 % unilateral facetectomy	0.580
MHL with 50 % unilateral facetectomy	0.844
UMIF with 50 % unilateral facetectomy	0.845

Results

FE modelling and validation results

The final intact FE model (Fig. 1a) included 376670 elements and 132240 nodes and all the critical components of the cervical spine. Figs. 3 and 4 show the comparison of the motions of intact model with the previously published data. The FE model’s predicted segmental motions were in the range of results observed in previous experiments studies.

Intersegmental motion of the surgical models

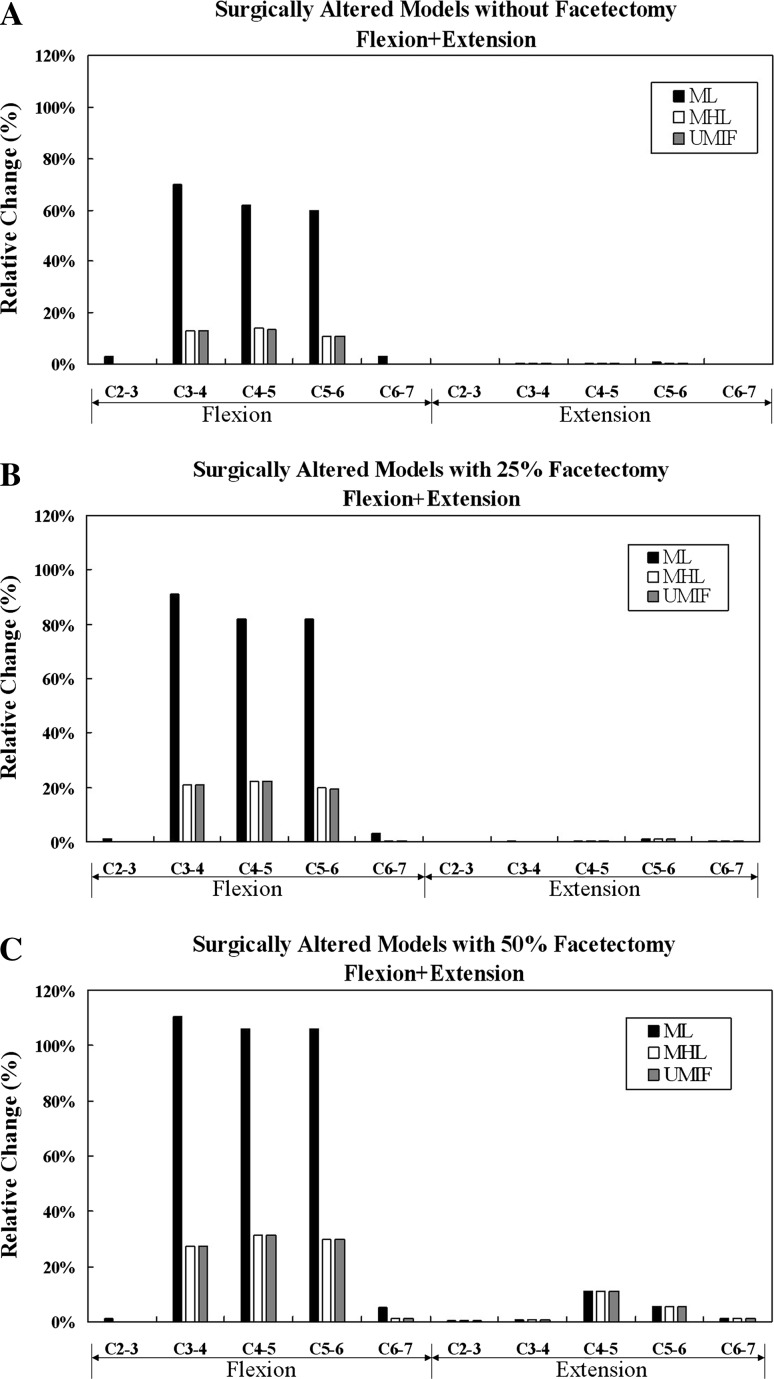
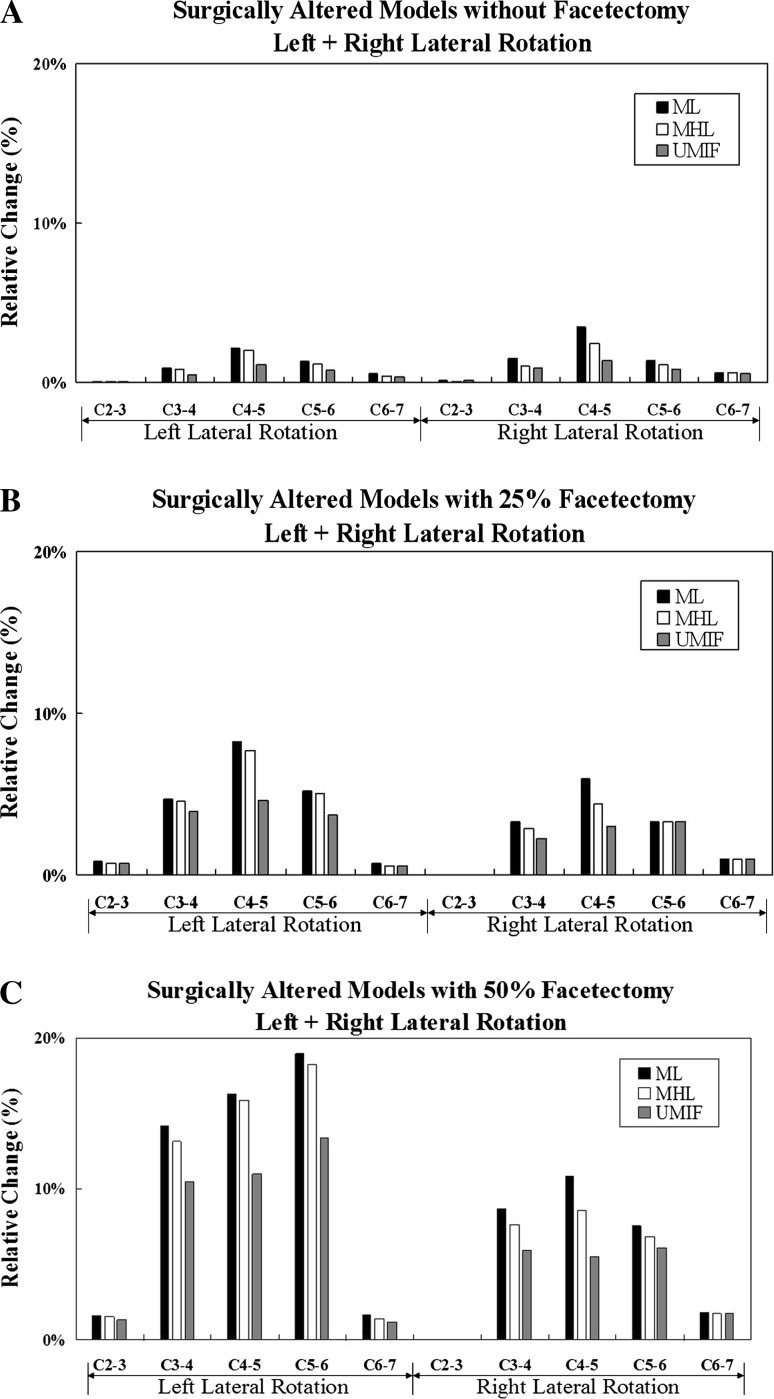
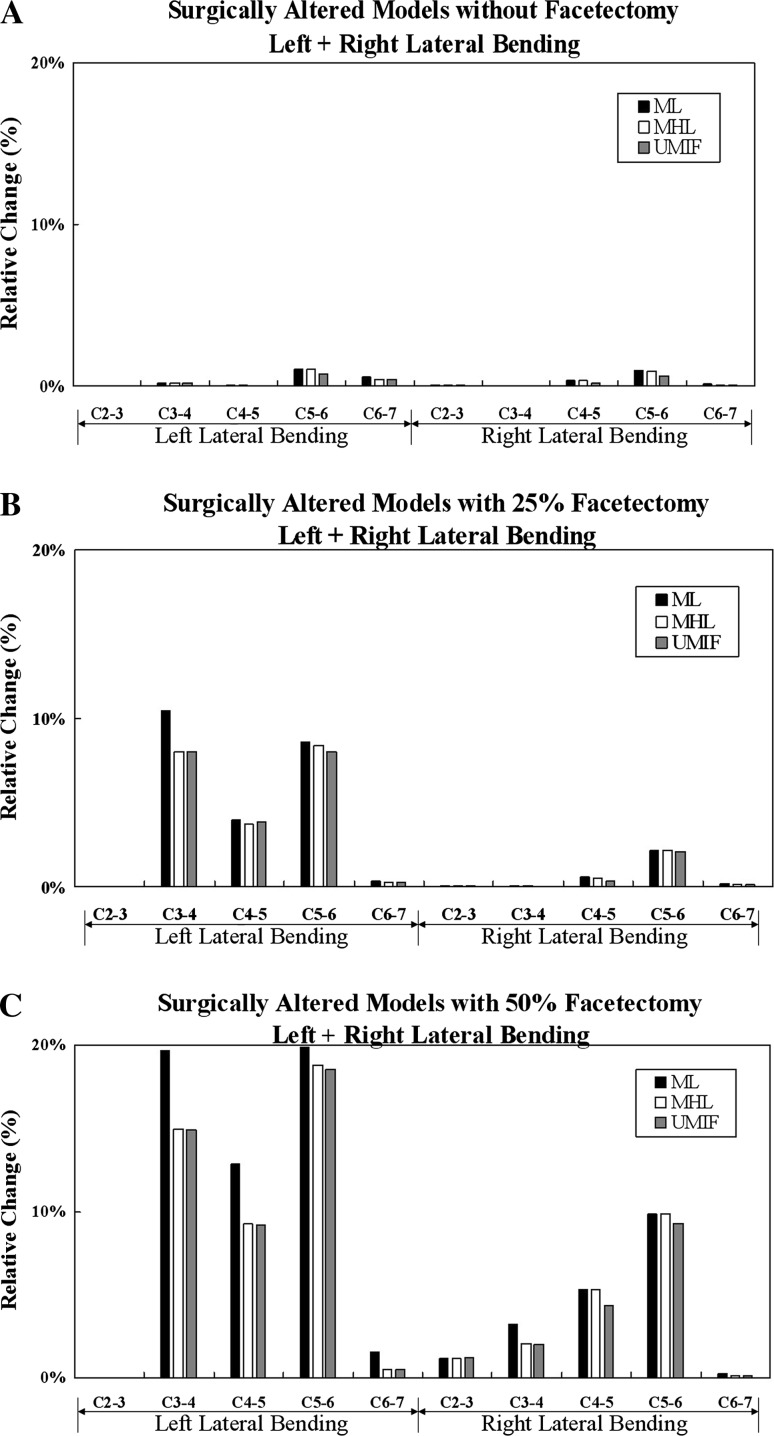
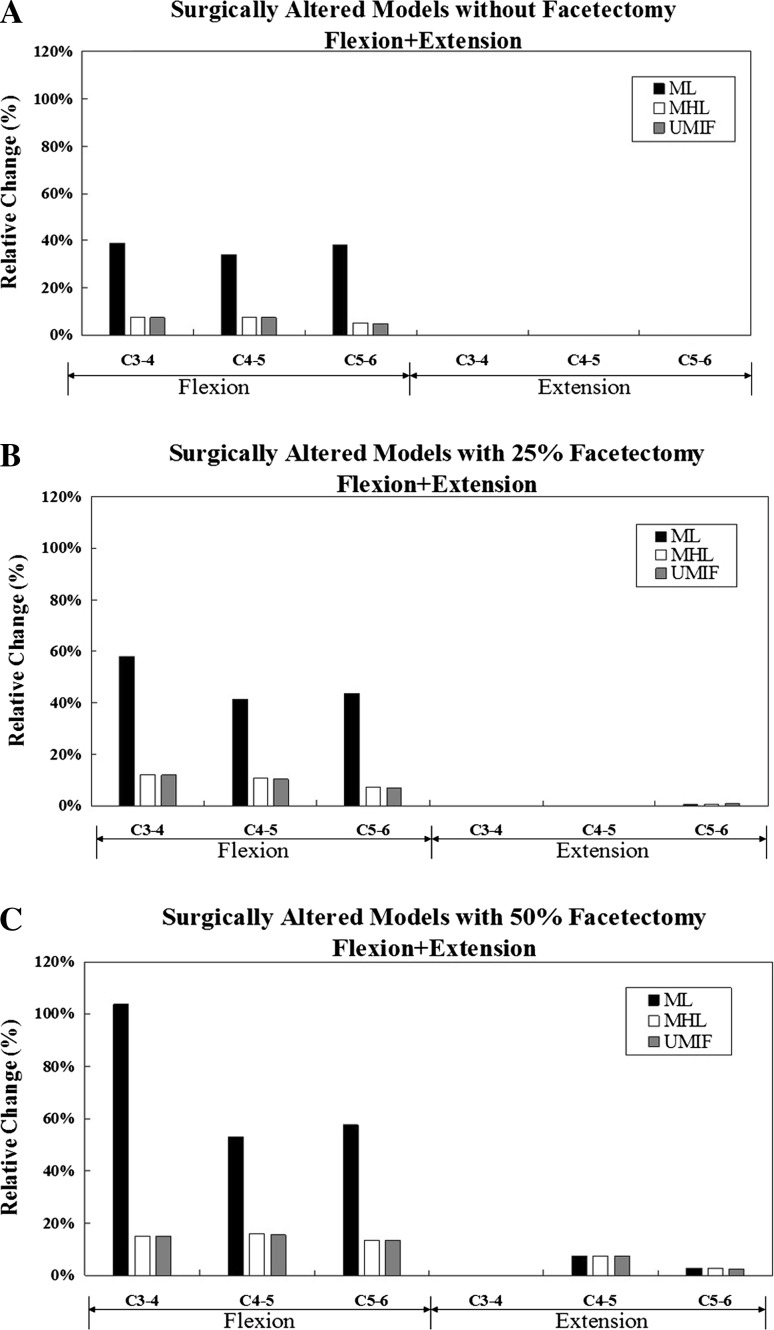
Figures 5, 6, and 7 show the percentage increases in intersegmental rotational motions of the surgical models under the load-controlled method. Under each loading type, the greatest biomechanical changes occurred at the surgical segments. Slight intersegmental increases of up to 5 % could be observed at the adjacent levels of C2–3 and C6–7 under all the surgical models. The greatest increase in segmental motion occurred in flexion under all surgical models.

Fig. 5.

Comparison of percentage increase in intersegmental motions under flexion–extension due to UMIF approach, MHL approach and ML approach without facetectomy (a), with 25 % facetectomy (b) and with 50 % facetectomy (c)

Fig. 6.

Comparison of percentage increase in intersegmental motions under axial rotation due to UMIF approach, MHL approach and ML approach without facetectomy (a), with 25 % facetectomy (b) and with 50 % facetectomy (c)

Fig. 7.

Comparison of percentage increase in intersegmental motions under lateral bending due to UMIF approach, MHL approach and ML approach without facetectomy (a), with 25 % facetectomy (b) and with 50 % facetectomy (c)

During flexion loading (Fig. 5), the greatest changes in motion occurred at C3–4 segment under ML without facetectomy with 70 % more motion than the intact, followed by a 62 % increase at C4–5 and a 60 % increase at C5–6. The increases were significantly reduced under MHL and UMIF approach, with the highest increase of 14 % at C4–5. When combined with graded facetectomy, the changes in motion under ML approach have a significantly further increase, even up to 110 % at C3–4. However, the further increase was not significantly following MHL and UMIF approach, with the highest further increase of 17 % at C4–5. The three surgery approaches made no significant difference in extension (Fig. 5).

As for torsion (Fig. 6), slight intersegmental responses were observed in all the models without facetectomy. Further increase in axial rotation can be seen during progressive facetectomy, and notably in left axial rotation. The segmental motions of MHL were similar to ML in axial rotation, with the greatest increase of 18 % when combined with 50 % facetectomy at C5–6 segment, whereas the UMIF with or without facetectomy had the smallest segmental motion. However, the preserved axial motion was not so significant, with no more than 5 % at C4–5.

The three surgical simulations had no significant difference in the changes of lateral bending motions, and further increase in motions due to graded facetectomy was similar to the axial rotation motions (Fig. 7).

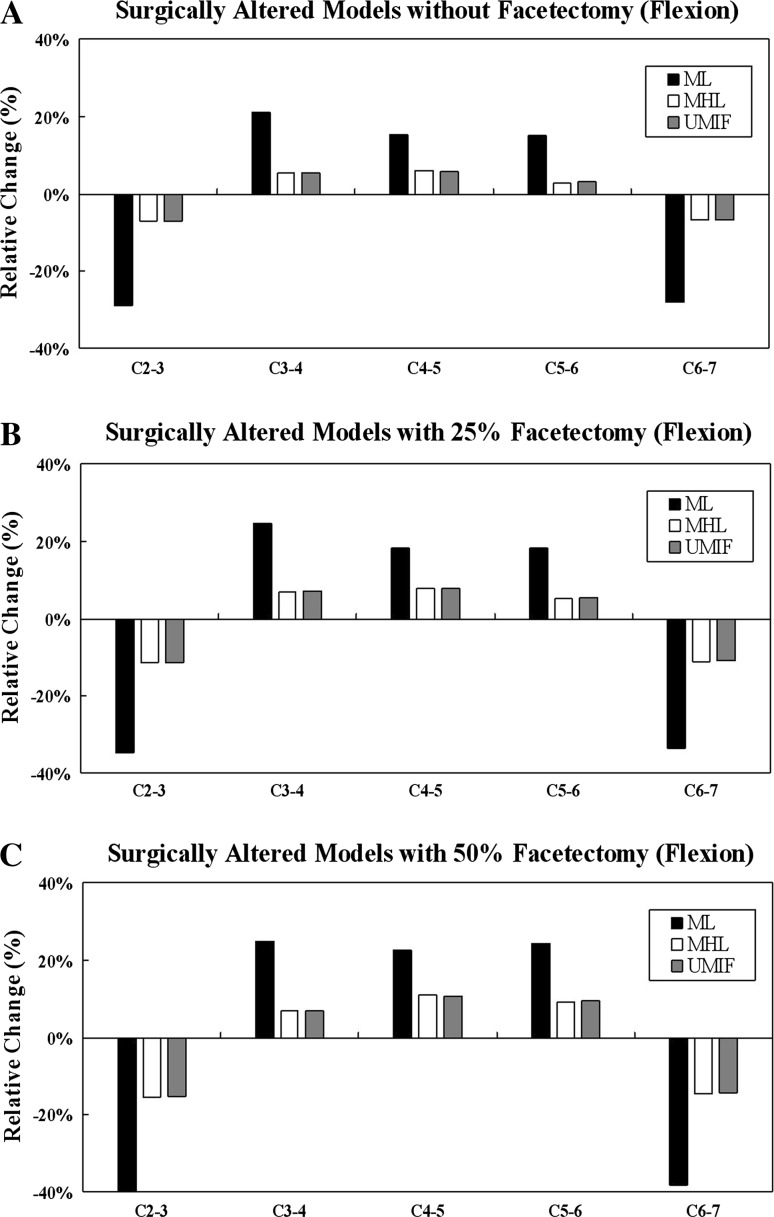
For the hybrid approach, the ML, MHL, UMIF model without facetectomy required 0.691, 0.927 and 0.928 Nm of moment, respectively with 50 N of follower load in flexion to achieve the intact model rotation (Table 2). The greatest increases in motion occurred at C3–4 segment under ML without facetectomy with 21 % more motion than the intact, followed by a 15 % increase at C4–5. The increases were also significantly reduced under MHL and UMIF, about 5–6 % at C3–4 and C4–5. Unlike the load-controlled method, significant decrease of motions could be observed at the adjacent levels. At C2–3, the corresponding decreases in motion were 30, 7 and 6.9 %, respectively for the ML, MHL and UMIF approach without facetectomy. When combined with graded facetectomy, the motions under each approach did not have a significant further change (Fig. 8).

Fig. 8.

Comparison of percentage increase in intersegmental flexion motions of the surgical models under hybrid approach. Compared to the load-controlled method shown in Fig. 5, the motions across the C2–C7 segment increased by smaller amounts at the surgical levels, and decreased at the adjacent levels

Stress in the annulus

The von Mises stresses in the annulus of the disc were compared for motions that generated large changes. The maximum stresses in the annulus occurred during flexion in ML model with 39, 35 and 38 % more stress than the intact at C3–4, C4–5 and C5–6, respectively. The stresses increase was significantly reduced under MHL and UMIF approaches, about 5–7 % increase. When combined with 50 % facetectomy, the maximum stresses increase more than 100 % under ML model at C3–4 (Fig. 9).

Fig. 9.

Comparison of percentage increase in maximum von Mises stresses in the annulus of the disc under flexion–extension due to UMIF approach, MHL approach and ML approach without facetectomy (a), with 25 % facetectomy (b) and with 50 % facetectomy (c)

Discussion

Spinal instability has always been defined as the range of motion of the spine developing abnormally large augmentation under physiologic load [12]. Therefore, the segment motion following each surgical model, which was mainly studied by the present study, will help surgeons to understand the stability of the spine after various surgical approaches.

Biomechanical analysis of cervical laminectomy, MHL and UMIF

Cervical spine instability after laminectomy has been proved in various in vitro and FE studies and the biomechanical alteration mainly occurred in sagittal plane. Cusick et al. [27] tested the stability of C2–T1 specimens with three-level laminectomy on C4–C6, and found that the multilevel cervical laminectomy induced a significant increase of 117 % in sagittal rotation angle. Hong-Wan et al. [18] simulated C5 laminectomy on a FE model of C2–C7, and the results indicated an increase in multisegmental responses of 59.5 % in flexion–extension, 1.8 % in lateral bending, and 4 % in axial rotation. According to our models, the significant alterations after laminectomy occurred in flexion at the surgical sites, with the highest increase of 70 % at C3–4, and there was no significant increase in lateral bending (up to 2 %) and axial rotation (up to 3.5 %). Our results corresponded well with the previous reported data, and can explain the clinical observations that post-laminectomy deformities occur mainly in the sagittal plane, such as swan-neck deformities and cervical kyphosis.

In our study, the changes in flexion after laminectomy were greatly reduced under UMIF and MHL approaches. The reduction can be explained by the preservation of the posterior element, including interspinous ligaments, contralateral lamina and 3/7 of ligamentum flavum, which are the critical structures in maintaining the spinal stability in sagittal plane.

The increased stress on the annulus correlated to the increased motion. The laminectomy model created much more stress at the surgical site than the intact, MHL and UMIF models. The results meant that increased flexibility caused by posterior element removal leads to an increase in stresses of the annulus, and the increased stresses may increase the risk of disc degeneration.

Panjabi et al. [28] have proposed hybrid approach, which applies different moments so that the same overall ranges of motions (ROMs) are achieved for both intact and implant models. And it is advocated to be more clinically relevant in evaluating the adjacent levels’ effect of the fusion cases.

However, it will be a different situation for non-fusion cases, for example, laminectomy. In real life, patients may sustain the same external moments during lifting activities after laminectomy [29], and the same moment is precisely a reason of excess ROMs of the spine and spinal instability for the patients after laminectomy. Thus, the load-controlled method can better explain how spinal instability occurs after laminectomy. And it is more suitable for evaluating the patient’s normal life work-loading condition after laminectomy. Zhong et al. [29] also indicated that the load-controlled method will emphasize the effects of the non-fusion implant. The ML, MHL and UMIF are all non-fusion cases, so the results of load-controlled method were mainly discussed in our study.

Biomechanical analysis of facetectomy

Facetectomy is imperative to acquire adequate exposure of many kinds of intradural tumours, but it would aggravate the spinal instability after laminectomy [13]. Our results suggested that the changes in flexion under laminectomy with graded facetectomy had a significant further increase, while it was inconspicuous following MHL and UMIF approaches. The difference can be interpreted as the synergistic effect of the capsular ligaments and the posterior ligaments on the stability of sagittal plane, and the posterior ligaments play the leading role. So when most of the posterior ligaments were preserved by UMIF and MHL, significant further increase in flexion cannot be seen even with 50 % facetectomy. The surgical models made no significant further increase in axial rotation and lateral bending motions even after 50 % facetectomy.

Clinical investigations on laminectomy, MHL and UMIF

Our biomechanical results suggested that UMIF and MHL largely preserved the stability of the spine, even combined with facetectomy, and the results are consistent with the clinical investigations. Post-laminectomy deformities in cervical spine have been recognized by many surgeons, and children appear to have the highest risk [1–3]. de Jonge reviewed 76 pediatric patients who had spinal malignant tumours treated with laminectomy or laminoplasty and/or radiation therapy, and all the 64 patients who were treated with laminectomy and/or radiation developed deformity [1]. Adults are supposed to experience a lower rate of post-laminectomy deformity than children, but some reported rates are still significant [13, 30]. Kaptain, et al. [30] reported a 21 % incidence of postoperative kyphosis in adult patients who have undergone laminectomy for cervical spondylotic myelopathy.

In contrast, hemilaminectomy has always been reported to have advantages in preserving spinal stability [4, 6]. In a series of 51 cervical spinal cord tumours, Asazuma et al. [4] reported that worsening of cervical curvature occurred in 1 of 15 patients with hemilaminectomy, 5 of 14 patients with laminectomy and 7 of 22 patients with laminoplasty. UMIF approach has been most commonly used in the surgical treatment of degenerative lumbar stenosis, and the rate of postoperative instability is relatively low [31]. Koch-wiewrodt first reported its use in 78 patients with intraspinal space-occupying lesions, and also found no spinal deformities in up to 8 years’ follow-up [10].

Now, laminectomy and fusion are frequently used to prevent spinal instability in the surgery of intradural tumours. However, laminectomy and fusion for multilevel mean the reduction of range of motions in multilevel and patients’ poor quality of life. Furthermore, fusion for multilevel is an expensive procedure for patients [32]. Therefore, according to the above clinical literature and our biomechanical results, UMIF and MHL approaches without fusion, which preserve spinal stability well, may be a beneficial choice for selective tumours.

The feasibility and limits of unilateral approaches

Some surgeons may criticize the potential risk of injury to the spinal cord when unilateral approaches are used, due to the relatively narrower exposure than laminectomy. In fact, many intradural tumours are hemilateral and unilateral approach is enough to get adequate exposure. And with removing the base of the spinous process, an adequate visualization to the whole axial spinal canal can be achieved (Fig. 10). The safety of unilateral approaches for removal of many kinds of spinal tumours has been proven by Yaşargil, Chiou and many other surgeons [5–7]. Besides, the UMIF creates multiple spinal keyholes, and the entire longitudinal intraspinal space can get an adequate view through the side-by-side keyholes [10], too.

Fig. 10.

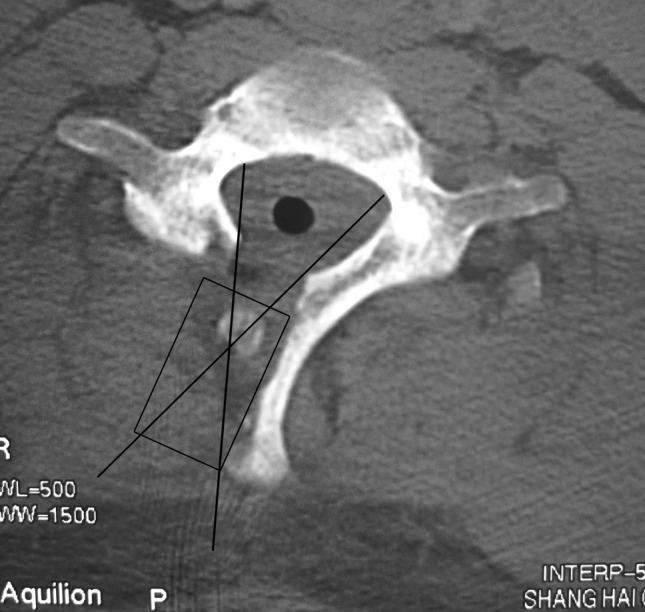
One cross-section of the UMIF approach. The base of the spinous processes was undercut to gain access to the contralateral side lesion. The rectangle represents the path of the surgery, and the two lines represent the region what we can see through the microscope

However, surgeons should keep in mind that the most important thing for intradural tumours is to resect the tumour completely without any injury to the spinal cord, and the unilateral approaches is just suitable for the selected cases, not all the spinal tumours. For example, the huge tumours with scalloping of vertebrae, easy bleeding tumours spreading to both sides, meningiomas with broad dural attachment will be difficult to manage by unilateral approaches [6].

Biomechanical comparison between UMIF and MHL

The UMIF approach only showed slight advantage over MHL in axial motion according to our results. The advantage can be interpreted as that the axial force can be exerted along the normal trajectory as the intact spine by preserving the posterior vertebral arches. However, the advantage was not significant (up to 5 %). It is unknown whether this slight advantage can predict conspicuous clinical significance. Therefore, further clinical investigations are needed to identify whether UMIF shows superiority in preserving spinal stability than MHL. In our study, UMIF model only preserved more bone element than MHL, so this also indicate that the posterior bone elements play a slight role in spinal stability after removal of the attached ligaments.

The musculature’s effect

The limitation of the present study is that the musculature’s effect to the stability of the cervical spinal was not considered. Nolan and Sherk have found that the extensor muscle may act as significant stabilizers of the cervical spine [33]. Laminectomy need to dissect the bilateral extensor muscle from the lamina and spinous, while UMIF and MHL preserve the contralateral muscle very well, so UMIF and MHL probably have more advantages than the study has demonstrated.

Conclusion

ML with or without facetectomy demonstrated significant increases in sagittal plane motion compared with the intact model, which were greatly reduced under UMIF and MHL. UMIF and MHL also reduce the increased stress of annulus caused by ML. The results suggest that the less invasive approaches of UMIF and MHL greatly preserve the normal motion of the cervical spine compared with laminectomy and minimize the risk of postoperative spinal instability and disc degeneration. Besides, according to the current clinical literature, UMIF and MHL approaches without fusion are safe and effective in resection of many selective tumours, so it is useful for clinical application. The UMIF only showed slight advantage over MHL in the biomechanical study and indicated that the posterior bone elements play a slight role in spinal stability after removal of the attached ligaments.