Dynamics of the Late Na⁺ current during cardiac action potential and its contribution to afterdepolarizations

Abstract

The objective of this work is to examine the contribution of late Na⁺ current (I_Na,L) to the cardiac action potential (AP) and arrhythmogenic activities. In spite of the rapidly growing interest toward this current, there is no publication available on experimental recording of the dynamic I_Na,L current as it flows during AP with Ca²⁺ cycling. Also unknown is how the current profile changes when the Ca²⁺-calmodulin dependent protein kinase II (CaMKII) signaling is altered, and how the current contributes to the development of arrhythmias. In this study we use an innovative AP-clamp Sequential Dissection technique to directly record the I_Na,L current during the AP with Ca²⁺ cycling in the guinea pig ventricular myocytes. First, we found that the magnitude of I_Na,L measured under AP-clamp is substantially larger than earlier studies indicated. CaMKII inhibition using KN-93 significantly reduced the current. Second, we recorded I_Na,L together with I_Ks, I_Kr, and I_K1 in the same cell to understand how these currents counterbalance to shape the AP morphology. We found that the amplitude and the total charge carried by I_Na,L exceed that of I_Ks. Third, facilitation of I_Na,L by Anemone toxin II prolonged APD and induced Ca²⁺ oscillations that led to early and delayed afterdepolarizations and triggered APs; these arrhythmogenic activities were eliminated by buffering Ca²⁺ with BAPTA. In conclusion, I_Na,L contributes a significantly large inward current that prolongs APD and unbalances the Ca²⁺ homeostasis to cause arrhythmogenic APs.

1. Introduction

The late Na⁺ current (I_Na,L) influences the cardiac action potential (AP) repolarization [1–5], participates in Na⁺-Ca²⁺ homeostasis of cardiac cells [6–13], and can promote arrhythmogenic activities [13–17]. This tiny, persistent Na⁺ current has received increasing attention since its selective inhibition eliminated arrhythmogenic APs [5, 15–17], but electrophysiological recording of the I_Na,L current has been technically challenging. Our current knowledge on I_Na,L is mostly based on the voltage-clamp data obtained under simplified conditions (rectangular voltage pulse, ion substitutions, exogenous Ca²⁺ buffer etc.). The data was then used in model simulations to predict the dynamic profile of the current during AP in order to understand its role in shaping the AP. Although this approach has contributed valuable knowledge, using standard voltage-clamp technique to study I_Na,L suffers a great limitation due to a unique feature of the Na⁺ channel called non-equilibrium gating. Clancy et al. [13] first discovered an enhancement of I_Na,L under a repolarizing voltage ramp (instead of a rectangular voltage step), and this can be explained by a fast recovery of the Na⁺ channel from inactivated state and reactivation during repolarization ramp (non-equilibrium condition). The non-equilibrium gating gives rise to a large portion of I_Na,L and, importantly, this portion of the current cannot be activated by rectangular pulse voltage-clamp protocol [13, 15]. The divergent I_Na,L profiles reported in previous literature highlight the complexity in Na⁺ channel gating and the necessity to directly record I_Na,L under AP-clamp, because dynamic changes of the membrane potential during AP would significantly influence I_Na. In this study we use a special version of AP-clamp technique called self AP-clamp (sAP-clamp) [18–20] to directly record the I_Na,L during AP in the guinea pig ventricular myocytes under a triad of conditions: use the cell’s own steady state AP as the voltage command; keep physiological ionic compositions in the internal and external solutions; and preserve the intracellular Ca²⁺ cycling. The goal is to visualize and analyze I_Na,L as it flows during cardiac AP under physiological conditions in order to understand its contribution to shaping cardiac APs.

Voltage gated Na⁺ channels are known to be regulated by Ca²⁺, calmodulin (CaM), and Ca²⁺-CaM dependent protein kinases II (CaMKII); each of these molecules also individually and collectively modulates I_Na,L [21–23]. Previous studies exploring the individual elements of this complex regulation reported conflicting results. For example, Wingo et al. proposed that Ca²⁺ modulates Na⁺ current by directly binding to the EF-hand motif in the C-terminus [24], but different studies provided evidence against this notion [25, 26]. Tan et al. showed that CaM enhances slow inactivation of I_Na [27], and Kim et al suggested that CaM binding minimizes the sustained channel activity [25]. The current consensus is that Ca²⁺-CaM-CaMKII signaling facilitates cardiac Na⁺ current [23]. However, the simplified conditions used in traditional voltage-clamp experiments make it difficult to determine the integrated effects of Ca²⁺-CaM-CaMKII signaling pathway on modulating the I_Na during AP. Furthermore, many previous studies used rectangular pulse voltage-clamp protocol and the non-equilibrium gating of Na⁺ channel was absent from those data [28–30]. In this study we use the sAP-clamp technique to directly record the profile of I_Na,L during the AP with Ca²⁺ cycling, after Ca²⁺ overloading or chelation, and following CaMKII inhibition. The results provide the first experimental measure of CaMKII modulation of the dynamic I_Na,L during cardiac AP. We also further investigated changes in the AP profile when I_Na,L is facilitated by Anemone toxin II (ATX-II) to explore the role of I_Na,L in arrhythmogenesis.

2. Methods

All animal handling and laboratory procedures conform to the Guide for the Care and Use of Laboratory Animals published by the US National Institutes of Health, and to our Institutional Animal Care and Use Committee approved protocols. Chemicals and reagents were purchased from Sigma-Aldrich if not specified otherwise. Tetrodotoxin (TTX) and ATX-II were from EMD Chemicals. E4031 and Chromanal-293B were from TOCRIS. All experiments were conducted at 36±0.2 °C.

2.1. Cell isolation

Hartley guinea pig (male, 3–5 months old, purchased from Charles River Laboratories USA) were first injected with heparin (800u, I.P.) and then anesthetized with nembutal (100 mg/kg, I.P.). After achieving deep anesthesia a standard enzymatic technique was used to isolate ventricular myocytes at 37 °C. Hearts were mounted on a Langendorff system, and retrogradely perfused for 3–5 minutes with oxygenated solution containing (in mmol/l): NaCl 135, KCl 5.4, CaCl₂ 1, MgCl₂ 1, NaH₂PO₄ 0.3, HEPES 10, glucose 10; pH=7.2. Then a Ca²⁺-free solution containing (in mmol/l): NaCl 135, KCl 5.4, MgCl₂ 1, NaHPO₄ 0.3, HEPES 10, glucose 10, EGTA 0.05 (pH=7.2) was perfused for 3 minutes to stop the beating of the heart. Next, a solution containing (in mmol/l): NaCl 135, KCl 5.4, MgCl2 1, NaHPO4 0.3, HEPES 10, glucose 10), and supplemented with 0.6 mg/ml type II collagenase (298 U/mg; Worthington, USA) and 0.05 mg/ml protease type XIV (Sigma, USA) was perfused to enzymatically dissociate cells. After perfusion, the left ventricle was minced and further incubated in the above solution for 40, 60, 80 minutes at 37 °C. Cells were then harvested and stored in a modified Tyrode solution (BTY) containing (in mmol/l): NaCl 120, KCl 5, CaCl₂ 2, MgCl₂ 1, HEPES 10, NaHCO₃ 25, Glucose 10, pH=7.3 (adjusted using NaOH) and osmolarity=295–300 mmol/kg.

2.2. Electrophysiology

Cells were placed in a temperature controlled plexiglass chamber (Cell Microsystems, USA) and superfused with BTY solution (see above). Electrodes were fabricated from borosilicate glass (World Precision Instruments, USA) with tip resistances of 2–2.5 MΩ when filled with internal solution. To preserve the normal Ca²⁺ cycling during AP, we used the internal solution containing (in mmol/l): K-Aspartate 100, KCl 45, Mg-ATP 3, HEPES 5, cAMP 0.1, phosphocreatine dipotassium salt 10, Fura-2 K⁺ salt 0.02 (pipette loading), with pH=7.3 (adjusted using KOH) and osmolarity = 284–288 mmol/kg. To buffer intracellular Ca²⁺ to 100 nM (MaxChalator calculation) we used the internal solution containing (in mmol/l): K-Aspartate 74, KCl 43, KOH 25, Mg-ATP 3, HEPES 5, cAMP 0.1, phosphocreatine dipotassium salt 10, BAPTA 10, CaCl₂ 2.48, Fura-2 K⁺ salt 0.02, with pH=7.3.

sAP-clamp Sequential Dissection experiments were conducted as described in our previous publication [19]. Basic steps include: (1) Record the cell’s steady state AP under I-clamp (I=0) at 1 Hz pacing frequency. (2) Apply this AP onto the same cell as the voltage command under V-clamp at 1 Hz. The net current output, I_BG should be zero. (3) Isolate the current of interest by using its specific blocker to remove it from the net current output, I_drug. (4) The current of interest is then obtained by subtraction: I = I_BG - I_drug. (5) Next, isolate the 2^nd current of interest by applying the 2^nd channel blocker, and then obtained the 2^nd current by subtraction: I₂ = I_drug1 - I_drug2. Repeat (5) to isolate the 3^rd, the 4^th, and more currents by sequentially adding the specific blocker for each channel. The currents were recorded after they had reached steady-state. The following blockers used for dissecting out the currents: 10 µM chromonal-293B for I_Ks, 1µM E4031 for I_Kr, and 50 µM Ba²⁺ for I_K1.

Current-clamp experiments were conducted to record action potentials (APs). Cells were stimulated with supra-threshold depolarizing pulses (2 ms duration) delivered via the patch pipette. APs were recorded at various pacing frequencies. After reaching steady state at each frequency, at least 50 consecutive APs were recorded to examine the average behavior. The frequency series were first recorded under control condition (in BTY), and then repeated after treatment with 10, 30 and 100 nM of ATX-II.

2.3. Measurement of intracellular Ca²⁺ concentration, [Ca²⁺]_i

Cells were loaded with 20 µM Fura-2 K⁺ salt (Invitrogen, USA) through the patch pipette for simultaneous recording of the electrical sig_Na,L and the Ca²⁺ sig_Na,L in the same cell. [Ca²⁺]_i was measured using IonOptix system (Ionoptix, USA) with dual excitation at 340 nm and 380 nm, and emission at >510 nm (long pass filter). The background fluorescence was measured after tight seal formation. After subtracting the background signal, the fluorescence ratio (F340/F380) was then used to measure [Ca²⁺]_i as described previously [31].

2.4. Ca²⁺ loading of ventricular myocytes

The myocytes were stimulated at 1 Hz under current-clamp mode during the entire Ca²⁺ loading and washing procedure below. To increase the cytosolic Ca²⁺ concentration the cells were superfused with a modified Tyrode’s solution with lower Na⁺ concentration (110 mM instead of 145 mM, osmolarity was compensated with NMDG) for 10 minutes (Ca²⁺ loading), and then returned to BTy solution for 5 minutes (washing). This protocol resulted in significant increases in the diastolic Ca²⁺ concentration as measured by Fura-2 at the end of the washing procedure. Then the sAP-clamp experiments were immediately carried out to measure the I_Na,L under high Ca²⁺ load condition.

2.5. Statistical Analysis

The numerical values are presented as Mean ± Standard Error of Mean (SEM) in figures if not noted otherwise. Paired or unpaired Student’s t-test with equal variance (after using F-test to confirm equal variance) and 2 tails is used to evaluate the difference in the mean values, and the difference between two groups is deemed significant if p<0.05.

3. Results

3.1. Profile of I_Na,L under sAP-clamp with Ca²⁺ cycling

In order to study the magnitude and time course of I_Na,L during AP in the ventricular myocyte, we used sAP-clamp technique to directly record I_Na,L under a triad of conditions that mimic in situ environment: during the cell’s steady state AP, in a physiological ionic milieu (no ion substitution), and with intracellular Ca²⁺ cycling (no exogenous Ca²⁺ buffer). Fig.1A shows a set of Na⁺ currents obtained as TTX-sensitive currents under sAP-clamp. Notice that while the increasing TTX concentrations (between 0.3–30 µM,) inhibit larger and larger fraction of the plateau current the profile of the Na⁺ current keeps the same shape, indicating that TTX is a highly specific blocker in this concentration range. The TTX-sensitive current displayed a fast component that declined within 20 ms after the AP upstroke and a slow component that turned around to increase during the AP plateau and persisted throughout the AP; these two components demonstrate that TTX blocked both the fast and the late Na⁺ current. The slow current seen from the turning point to the end of AP is late Na⁺ current, I_Na,L.

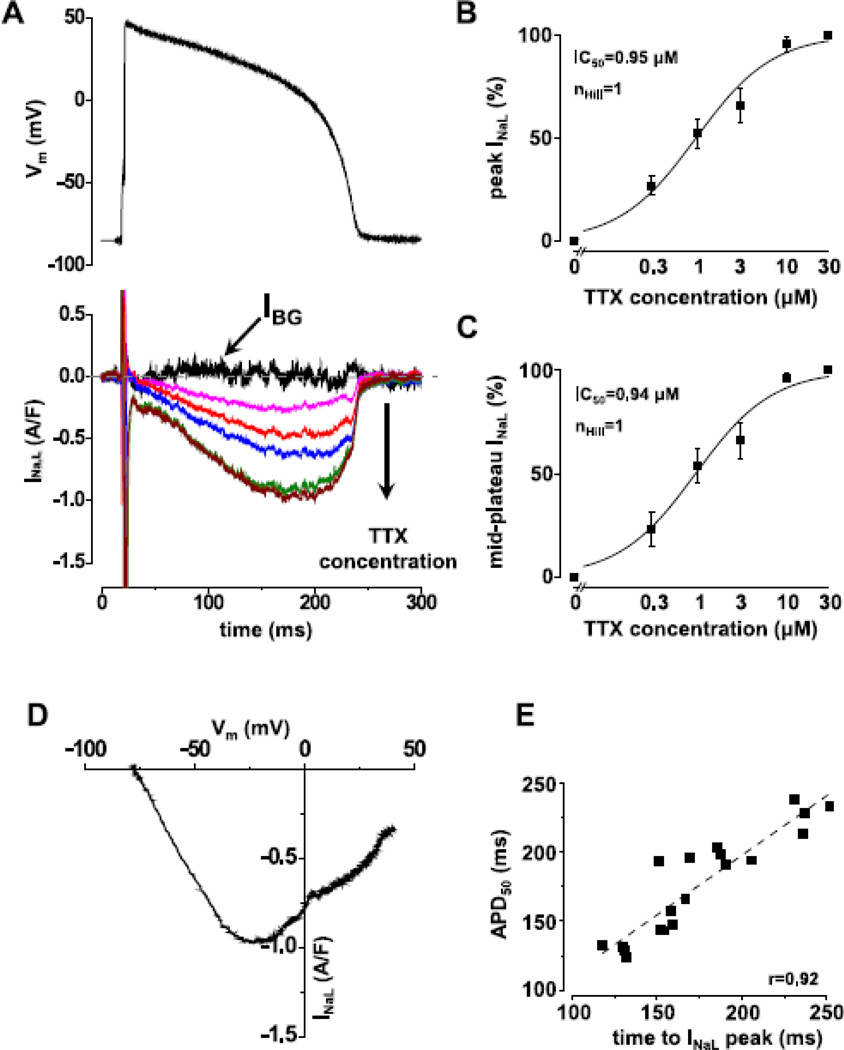
Fig.1. sAP-clamp recording of I_Na,L as TTX-sensitive current in guinea pig ventricular myocyte.

Panel A shows a representative set of I_Na,L current recorded under sAP-clamp by using TTX. As the TTX concentration increases (0, 0.3, 1, 3, 10, 30 nM), larger fraction of I_Na,L is visualized. B & C show the TTX concentration dependence of peak and mid-plateau current magnitudes (Mean±SEM, n=7cells/3animals). The Hill coefficient and IC₅₀ values are the same at the peak and mid-plateau point, indicating the high specificity of TTX in blocking I_Na,L within the concentration range examined. Panel D shows the dynamic I-V relationship of the full amount of I_Na,L dissected out by 30 nM TTX plotted against the membrane potential. Panel E shows a tight correlation between APD₅₀ and the time to peak I_Na,L (n=19cells/11animals). Similar correlations were observed for APD₇₅ and APD₉₀.

I_Na,L recorded under sAP-clamp displays a strikingly different profile from the small and monotonically decaying current seen under rectangular pulse voltage-clamp; the late Na⁺ current is now clearly separated from the fast Na⁺ current. As shown in Fig.1A, I_Na,L was a small current at AP phase-1, increased gradually during phase-2 and -3, reached a peak at APD50, and then declined rapidly. Fig.1B&C demonstrate TTX dose-response of the peak (Fig.1B) and the mid plateau (Fig.1C) values of I_Na,L; the data were fitted to Hill equation with Hill coefficient of 1 and IC50 of 0.94 µM and 0.95 µM, respectively. Hence, using 10–30 µM TTX was sufficient for dissecting out the full measure of I_Na,L. The peak current density of I_Na,L under sAP-clamp reached 0.78±0.07 A/F, demonstrating a surprisingly larger current. The instant I-V relationship of I_Na,L shows a bell-shaped curve with the peak current occurring at −15.3±4.6 mV (Fig.1D). A strong correlation also exists between the time to peak I_Na,L and APD50 (Fig.1E).

3.2. Modulation of I_Na,L during AP by Ca²⁺ and CaMKII

We further investigate how the I_Na,L profile during AP changes by increasing or decreasing the intracellular Ca²⁺, and by CaMKII inhibition. When the cytosolic Ca²⁺ concentration was clamped to 100 nM by using BAPTA, the AP was significantly lengthened and the I_Na,L recorded under sAP-clamp was significantly enlarged (Fig.2A). At the beginning, the I_Na,L in the Ca²⁺ clamped cell progressed at the same rate as in the control, but it continues to rise as the AP is lengthened and thus reached a higher peak. The instant I-V relationship for the control and the Ca²⁺ buffered cell showed difference in magnitude but no shift in the voltage dependency. The I_Na,L current density was significantly increased during AP (Fig.2C) and the total amount of Na inflow carried by I_Na,L was tripled in Ca²⁺ buffered cells (Fig.2D). Therefore, the lengthening of AP and elevation of plateau voltage under the low Ca²⁺ condition facilitates the I_Na,L during AP.

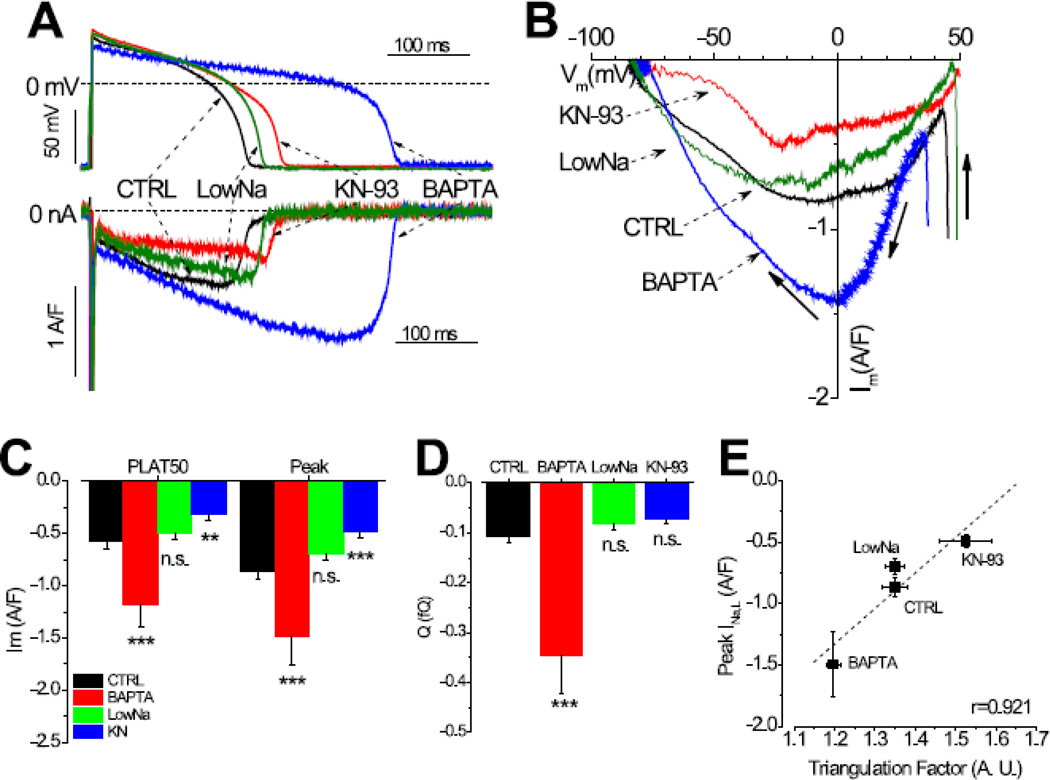
Fig.2. Modulation of I_NaL by Ca²⁺ and CaMKII.

Panel A shows representative traces of I_Na,L recorded in control cells (n=19cells/11animals), in BAPTA treated cells (n=11cells/5animals), in Low Na treated cells (n=7cells/3animals), and in 1 µM KN93 treated cells (n=10cells/5animals). Panel B shows the dynamic I-V relationship of I_Na,L when the Ca²⁺ and CaMKII signaling were normal and altered by the above different interventions. Panel C & D show the total charge carried by I_Na,L (Q_Na) and the peak current magnitude, respectively. Panel E shows a tight correlation between the amplitude of I_Na,L and the triangulation factor of the AP shape, demonstrating the presence of non-equilibrium gating.

Since I_Na,L was increased by chelating Ca²⁺, next we investigate whether increasing cytosolic Ca²⁺ would have the opposite effect. We used a Ca²⁺ loading procedure (see Method #2.4) to increase the amplitude and the baseline of the Ca²⁺ transient during AP, and recorded I_Na,L under sAP-clamp. Contrary to the expectation, the profile of I_Na,L during AP and the I-V relationship did not decrease but is similar to that of the control (Fig.2A & 2B); the current density and the total charge carried by the current are also similar to the control (Fig.2C & 2D). These data suggest two possibilities: one is that the I_Na,L sensitivity to Ca²⁺ might be already saturated at the control condition so there is no further change with Ca²⁺ overload; another is that the I_Na,L might not be sensitive to Ca²⁺ and the facilitation of I_Na,L in Ca²⁺ buffered cells solely results from the altered voltage profile of AP.

Furthermore, we investigated the effect of CaMKII inhibition on the I_Na,L profile during AP. The cells were treated with 1 µM KN-93 in bath while being paced at 1 Hz at current-clamp mode for 10 minutes. KN-93 treatment reduced the magnitude of I_Na,L during AP (Fig.2A) without significantly altering the I-V relationship (Fig.2B). The mid-plateau current magnitude and the peak amplitude were significantly decreased (Fig.2C). Nevertheless, the total amount of charge carried by the current during the AP was not significantly different from the control (Fig.2D), due to a lengthening of the APD₉₀ by KN93 treatment (244.2±18.2ms vs. 204.7±9.2ms, p<0.05) that compensated for the decreased current amplitude.

To investigate the non-equilibrium gating, we use a Triangulation Factor (defined as TF=APD₉₀/APD₂₀) to characterized the membrane voltage change during AP. As shown in Fig.2E, BAPTA decreased TF as the AP lengthened and hence increased the peak I_Na,L amplitude. Low Na treated cells had similar TF and the peak I_Na,L as the control. KN93 treatment increased TF and reduced the peak I_Na,L. In fact, the peak I_Na,L current amplitude displays a tight positive correlation with TF (Pearson’s r value, r=0.921) under all experimental conditions tested, demonstrating a prominent influence of the non-equilibrium gating on the I_Na,L current amplitude during AP.

3.3. I_Na,L contributes significantly to the AP plateau

To understand the role of I_Na,L in shaping AP we investigated how the inward charge movement through I_Na,L is counterbalanced by the outward charge movement through K⁺ currents. We recorded the I_Ks, I_Kr I_K1, and I_Na,L from the same cell using the sAP-clamp Sequential Dissection method (Fig.3A). Under control condition, the peak current density of I_Na,L is higher than that of I_Ks but lower than that of I_Kr or I_K1. The I_Na,L peaks at the end of phase-2 of AP, about the same time when I_kr and I_k1 increase rapidly, but the magnitudes of the K⁺ currents become much larger and thus resulting in an acceleration of repolarization in the phase-3 of AP(Fig 3A). Surprisingly, the amount of inward charges carried by I_Na,L during the AP is comparable to the outward charges carried by each individual K⁺ current.

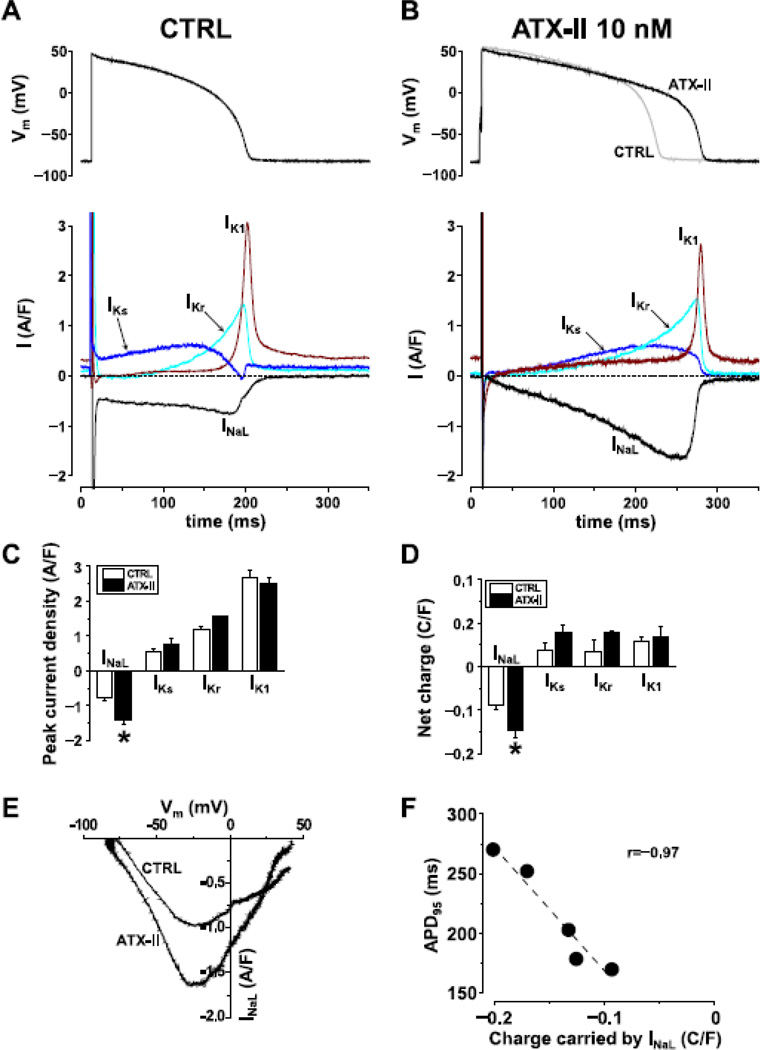
Fig.3. sAP-clamp Sequential Dissection of I_NaL and three major K⁺ currents from the same cell.

Representative traces of I_NaL, I_Ks, I_Kr and I_K1 recorded from control cells (Panel A, n=6cells/5animals) and following facilitation by 10 nM ATX-II treatment (Panel B, n=5cells/3animals). ATX increased the peak current density (Panel C) and net charge (Panel D) without altering the K⁺ currents. Facilitation with ATX-II did not alter the dynamic I-V relationship (Panel E). When AP was lengthened the net charge carried by the current was increased as is shown by the good correlation with APD₉₀ (Panel F).

Facilitation of I_Na,L with 10 nM ATX-II resulted in a robust increase in I_Na,L magnitude without altering the K⁺ currents (Fig.3B & 3C). Moreover, ATX-II treatment further increased the total charge carried by I_Na,L which significantly exceeds the outward charges carried by any individual K⁺ current (Fig.3D). The voltage dependence of I_Na,L shown in the instant I-V relationship was not altered (Fig.3E). Facilitation of I_Na,L by ATX-II resulted in significant lengthening of AP (APD₉₀ = 212.0±19.9ms ATX-II vs. 192.5±15.6ms control; Fig.3B) without altering the voltage dependence of I_Na,L. A tight positive correlation is found between the net charge carried by I_Na,L and the AP duration (Fig.3F). These data reveals a significant contribution of the I_Na,L to shaping the AP morphology, and upregulation of I_Na,L can give rise to long APD.

3.4. Upregulation of I_Na,L leads to arrhythmogenic APs

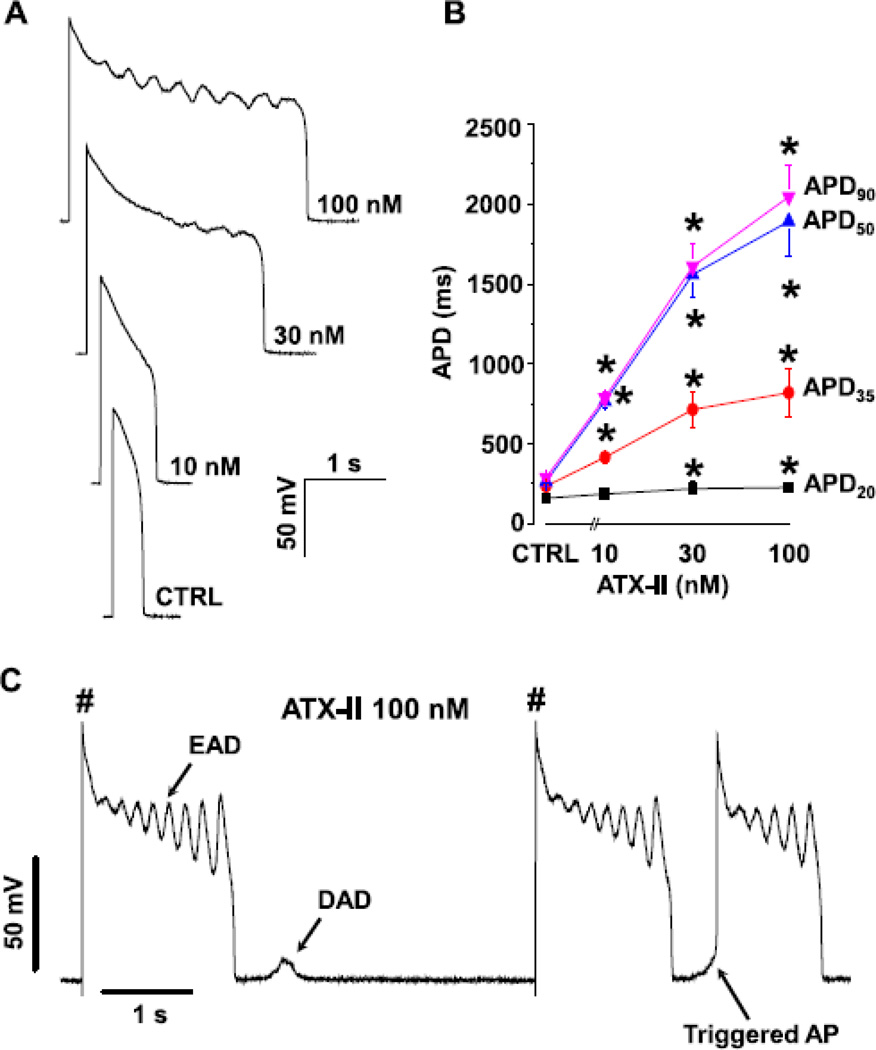
To further investigate the role of I_Na,L in arrhythmogenesis, we recorded the action potentials under current-clamp mode and studied the effect of upregulating I_Na,L using ATX-II. Our data show that ATX prolonged APD and induced arrhythmogenic APs in a dose-dependent manner (Fig.4A). While APD₂₀ was not significantly altered by ATX-II in the range of 10–100 nM, APD₃₅, APD₅₀ and APD₉₀ were greatly lengthen by ATX-II at 10 nM and above (Fig.4B). Early afterdepolarizations (EADs) emerged with 30–100 nM ATX-II treatment; EADs often occurred during early plateau phase, within 400–500 ms after the upstroke of AP. In addition to EADs, delayed afterdepolarizations (DADs) and triggered APs (TAPs) also emerged in 100 nM ATX-II in the same cell (Fig.4C), suggesting a common origin for these different forms of arrhythmogenic APs.

Fig.4. Effect of I_Na,L on AP morphology.

Panel A shows representative APs recorded in the presence of different ATX-II concentrations. Using ATX-II to upregulate I_NaL resulted in AP lengthening and generation of EAD. Panel B shows the concentration dependent AP lengthening effect of ATX-II at 0.2 Hz stimulation rate (n=6–11cells/6 animals). Panel C show the induction of various forms of arrhythmogenic APs (EAD, DAD, TAP) with ATX-II 100 nM in the same cell.

3.5. Spontaneous Ca²⁺ release from SR mediates I_Na,L induced arrhythmogenic APs

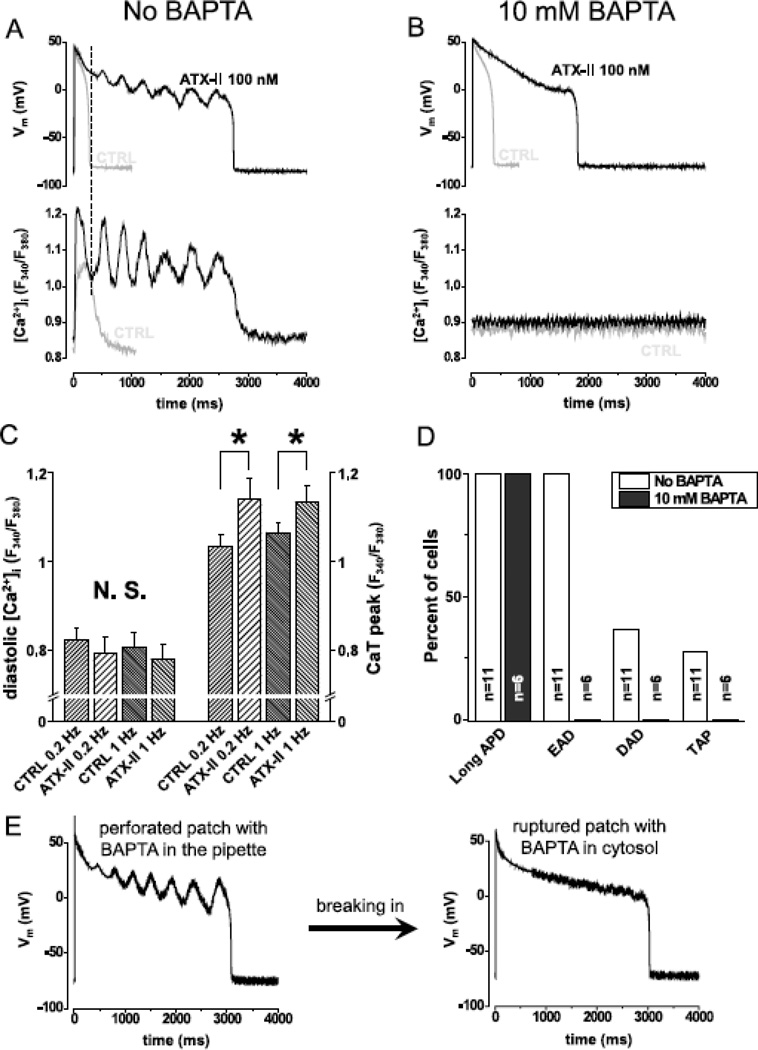
We hypothesized that ATX-II induced EADs are caused by Ca²⁺ release from SR (which also causes DADs and TAPs), rather than by reactivation of the L-type Ca²⁺ current during prolonged AP. To test this hypothesis we simultaneously recorded AP and the intracellular Ca²⁺ concentration ([Ca²⁺]_i) using Fura-2 under the current-clamp mode. Fig.5A demonstrates that EADs occurred in concomitant with intracellular Ca²⁺ oscillations. As a tell-tale sign, the first spontaneous Ca²⁺ wave after the paced systolic Ca²⁺ transient always rises before the membrane voltage turns upward to form EAD. These data suggest that the Ca²⁺ wave drives the inward Na⁺/Ca²⁺ exchange current (forward mode) to give rise to EAD. In support of this notion, eliminating Ca²⁺ oscillations using BAPTA also abolished EADs (Fig.5B). Recall that BAPTA treatment also prolonged APD (Fig.2B) which should increase the reactivation of L-type Ca²⁺ current. Hence the cause of ATX-II induced EADs is not reactivation of L-type Ca²⁺ current but spontaneous Ca²⁺ release from SR. In further support of this notion, the systolic Ca²⁺ transient was higher in ATX-II treated cells than in the control (Fig.5C), demonstrating a higher SR load that would facilitate spontaneous Ca²⁺ release from SR. In relating to arrhythmogenic APs, I_Na,L upregulation by 100 nM ATX-II induced long APD and EADs in all cells examined, and also DADs and TAPs in some cells (Fig.5D). In contrast, Ca²⁺ buffering by BAPTA prolonged APD, but eliminated EAD, DAD and TAP. As a final confirmation for the role of Ca²⁺ oscillation in generating EAD, we conducted a self-controlled experiment in the same cell. As shown in Fig.5E, we first used perforated patch technique with the pipette solution containing 10 mM BAPTA and recorded EADs evoked by 100 nM ATX-II treatment; the Ca²⁺ oscillations were behind the EAD generation since the BAPTA could not get into the cell in the perforated patch configuration (Amphotericin was used to form small perforations in the cell membrane patch). Then we ruptured the membrane patch to form a ruptured whole-cell configuration to allow BAPTA diffusion into the cytosol; this eliminated Ca²⁺ oscillation and EAD but did not shorten APD. Therefore, I_Na,L directly contribute to lengthening APD, but the effect of I_Na,L on evoking EAD, DAD, and TAP is Ca²⁺ dependent through altered Na⁺ – Ca²⁺ homeostasis.

Fig.5. Spontaneous Ca²⁺ oscillation gives rise to EAD.

Panel A shows simultaneous recording of AP (upper trace) and Ca²⁺ concentration (lower trace) under sAP-clamp (n=9cells/5animals). Grey and black lines show traces recorded in the absence and the presence of 100 nM ATX-II, respectively. ATX-II lengthened APD but EADs were seen only when Ca²⁺ was not buffered. Dashed line in panel A shows that the rise in Ca²⁺ signal precedes the turning point of membrane potential indicating that Ca²⁺ drives EAD. Panel B shows that when Ca²⁺ was clamped to 100 nM using BAPTA (n=6cells/3animals), ATX-II lengthened AP but no EAD was observed (grey line control, black line ATX-II). Statistical analysis of the Ca²⁺ transients (Panel C,) reveal that systolic, but not diastolic Ca²⁺ concentration was increased by ATX-II at 0.2 Hz (n=9cells/5animals) and 1.0 Hz (n=8cells/5animals) pacing rate. Panel D shows the percent of cells developing various forms of arrhythmogenic APs in the presence of 100 nM ATX-II. When Ca²⁺ cycling was not buffered (n=11cells/6animals), all cells developed long APD and EADs and some developed DADs and TAPs. When Ca²⁺ was buffered with BAPTA (n=6cells/3animals), the cells developed long APD, but no EAD, DAD or TAP was seen.

4. DISCUSSION

In order to understand the contribution of I_Na,L to the normal and arrhythmogenic APs, we have recorded I_Na,L during the cell’s steady state AP in a physiological ionic milieu with Ca²⁺ cycling, while the cell was undergoing excitation-contraction at body temperature. To our best knowledge, this is the first time I_Na,L has been recorded under these conditions designed to closely mimic the physiological environment. Several pioneering studies used different variance of AP-clamp technique to measure I_Na,L [32–35]. However, all of them used AP waveforms recorded from other cells or reconstructed from model and therefore caused the measured current to deviate from the original current naturally flowing under the cell’s own AP. Moreover, the earlier AP-clamp experiments also used ion substitution (replacing Na⁺ with Cs⁺ or K⁺ with TEA) and exogenous Ca²⁺ buffer which, albeit useful for dissecting the biophysical properties of the channel, also deviate from physiological conditions and introduce some artifacts. The present sAP-clamp study was designed to directly record the I_Na,L following under the cell’s own AP during excitation-contraction coupling under physiological conditions. The data so obtained revealed novel findings on the dynamics of I_Na,L during AP and its role in arrhythmogenesis as discussed below.

4.1. Non-equilibrium gating is a chief factor determining the profile of I_Na,L during AP

Our data show that I_Na,L has a saddle-like profile during the AP in guinea pig ventricular myocyte (Fig.1A): after the fast Na⁺ current declines during phase-1, the late Na⁺ current (I_Na,L) takes a turn to increase during phase-2, reaches a peak at about APD₅₀, and then rapidly declines during phase-3, and returns to baseline at phase-4. First of all, note that the profile of I_Na,L recorded with rectangular voltage-clamp protocol differs significantly from that I_Na,L current seen during AP. The former is invariably triangular in all species due to proliferating inactivation of sodium channels under a constant voltage. The latter, however, was traditionally visualized by computer simulation based on rectangular voltage-clamp data and such simulations often involve simplifications in the model hence still need to be verified experimentally. Our recordings consistently show saddle-like I_Na,L profile during AP under control condition and also under ATX-II treatment in guinea pig myocytes (Fig.3A&B). This does not preclude that I_Na,L profile could be somewhat different in different species. Saddle-like profile is consistent with the simulation results from some models (canine [36], human [37], and guinea pig ventricular myocyte [38], and recombinant Na⁺ channel expressed in cell line 293 [13]), but differ from some other model simulations predicting triangle-like profile during AP (canine [33]; guinea pig [32]). Thus, our experimental data confirm those models, where I_Na,L accumulates during the AP plateau giving a peak before terminal repolarization.

The profile of I_Na,L is shaped by several mechanisms including ‘window current’ and ‘non-equilibrium gating’ [16]. A ‘window current’ occurs when ion channels reactivate in a voltage range where the steady-state activation and inactivation curves overlap. The window current voltage range for the Na⁺ channel is quite negative (e.g. −80 mV to −50 mV) [39]. The AP plateau, however, falls in the positive voltage range before repolarization. Hence the window current should be negligible during plateau. On the other hand, repolarizing voltage ramp was found to facilitate I_Na,L [13], and this phenomenon was termed non-equilibrium gating. Therefore, a steep repolarization in AP is expected to boost I_Na,L. Moreover, non-equilibrium gating is not detectable by the rectangular pulse voltage-clamp experiments that measure steady-state currents [13]. Therefore, earlier models based on steady-state currents would miss the non-equilibrium gating and produce a triangle-like I_Na,L profile without the saddle-like profile obtained in our sAP-clamp experiment. Our data is consistent with non-equilibrium gating, and provide a realistic measure of the I_Na,L during dynamic changes of the membrane potential during AP for fine-tuning future models.

4.2. Ca²⁺ dependent modulation of I_Na,L during AP

Ca²⁺ was proposed to modulate I_Na,L by direct and indirect mechanisms. Direct Ca²⁺ binding to hH1 domain of sodium channel was shown to induce a rightward shift in the steady state inactivation increasing the availability of channels at more positive potentials [24], therefore buffering cytosolic Ca²⁺ should reduce Na⁺ current. Inhibition of CaM-CaMKII pathway showed divergent effects on I_Na,L in different previous reports. In our experiments when BAPTA was used to buffer cytosolic Ca²⁺, The APD and plateau height were significantly increased, which give rise to substantially increased I_Na,L during the AP (Fig 2). The positive correlation between the triangulation factor of AP and the peak amplitude of I_Na,L further supports the conclusion that I_Na,L is more strongly influenced by the voltage profile during the AP rather than by Ca²⁺.

CaMKII is known to facilitate Na⁺ current [23]. In agreement with this notion, in our experiments CaMKII inhibition using KN-93 reduced I_Na,L magnitude throughout the time course of AP and also in the I-V relationship. Meanwhile, increasing Ca²⁺ load did not further increase I_Na,L, indicating that the Ca²⁺-CaMKII modulation of I_Na,L is already saturated at the control condition. The voltage dependence of the I_Na,L recorded under sAP-clamp did not change under all the conditions tested, seen as no horizontal shift in the instant I-V relationship albeit changes in the amplitude of the current density (vertical shift in I-V),. Our data provide, for the first time, a visualization of the impact of Ca²⁺ chelation and CaMKII inhibition on the dynamic profile of I_Na,L during the AP.

4.3. The magnitude of I_Na,L during AP in comparing to K⁺ currents

We found that I_Na,L presents a substantial inward current with peak current density of 0.78±0.32 A/F (Mean±SD) and total charge movement of 88.3±43.2 mC/F during the AP in guinea pig ventricular myocytes (Fig.3C&D). For long time it was thought that I_Na,L was a tiny sustained current during AP, especially in comparing to the fast Na⁺ current with much larger amplitude. Now we found that the I_Na,L during the AP is surprisingly large, even in comparing to the major K⁺ currents. The inward charge movement through I_Na,L should depolarize the membrane potential, which is counterbalanced by the outward charge movement through K⁺ channels. To investigate the interplay of I_Na,L and K⁺ currents in shaping the AP morphology, we recorded I_Na,L, I_Ks, I_Kr and I_K1 from the same cell. Previously, the relationship between I_Na,L and K⁺ channels was studied only in model simulations [36], and the ratio between I_Na,L and K⁺ channels was shown to affect arrhythmogenesis. Now our experimental data show, interestingly, the I_Na,L magnitude and time course of seems to mirror that of I_Ks (Fig.3A). The peak current density of I_Na,L is comparable to I_Ks, about 50% of I_Kr and 30% of I_K1 (Fig.3C). The inward charge carried by I_Na,L is more than the outward charge carried by any individual K⁺ current during the AP cycle, although less than the total charge carried by all three K⁺ currents (Fig.3D). Therefore our data reveal that I_Na,L is a major determinant of the AP morphology, and significantly contributes to arrhythmogenic AP activities

4.4. Spontaneous Ca²⁺ release from SR mediates I_Na,L induced EAD, DAD and TAP

Upregulation of I_Na,L is linked to development of arrhythmias in heart failure and other acquired heart diseases [15, 40]. However, the exact role of I_Na,L in arrhythmogenesis remained unclear, especially in the genesis of EADs. At least two possible mechanisms have been proposed [16]: one involves reactivation of the L-type Ca²⁺ current; the other involves SR Ca²⁺ overload. In the first mechanism, upregulation of I_Na,L prolongs the AP plateau which allows recovery and reactivation of the L-type Ca²⁺ current; then the Ca²⁺ influx disrupts the membrane repolarization to cause EADs [14, 16]. This mechanism emphasizes the window current of the L-type Ca²⁺ channel as the depolarization drive for EADs. Nevertheless, our data show that the first EAD occurred at positive voltages (>10 mV, see Fig.3A) above the Ca²⁺ ‘window’ current voltage range (−30 mV to 0 mV) [41]; hence, the window current is not responsible for generating the EAD.

In the second mechanism, upregulation of I_Na,L increases intracellular Na⁺ concentration which causes SR Ca²⁺ overload; SR overload then causes spontaneous Ca²⁺ release from SR which drives forward mode Na⁺/Ca²⁺ exchange to depolarize the membrane, resulting in EADs [6, 42, 43]. Our data provide several evidences to support this mechanism. First, the ATX-II treated cells showed elevated systolic Ca²⁺ transient indicating elevated SR load (Fig.5C). Second, the spontaneous Ca²⁺ wave precedes the first EAD (Fig.5A, indicated by dash line); this timing is consistent with increased cytosolic Ca²⁺ drives inward (forward mode) Na⁺/Ca²⁺ exchanger to cause EAD. Third, using BAPTA to buffer Ca²⁺ abolished EADs (Fig.5B), although caused a prolonged phase-2; this long plateau feature is clearly different from the EADs driven by Ca²⁺ oscillations (Fig.5A). Therefore, spontaneous Ca²⁺ release from SR is a prerequisite for the generation of EADs. Taken together, our data suggest that spontaneous Ca²⁺ release from SR is a common mechanism underlying I_Na,L induced EADs, DADs and TAPs. The common mechanism of EAD and DAD was already proposed in Cs⁺ and isoproterenol induced early afterdepolarizations [44, 45] and Udrovinas et al. showed that augmented I_Na,L contributes to diastolic Ca²⁺ accumulation [6]. Our data provide experimental evidence to support the common mechanisms of afterdepolarizations proposed in these earlier works.

Highlights.

• First recording of I_Na-L during action potential with Ca²⁺ cycling

• Comparing I_Na-L with three major K⁺ currents in the same cell

• Novel finding of large I_Na-L comparable to I_Ks and I_Kr

• Reveal significant role of I_Na-L in action potential repolarization

• Interplay between I_Na-L and Ca²⁺ dynamics causes EAD, DAD, TAP