Radiobiological comparison of two radiotherapy treatment techniques for high-risk prostate cancer

Abstract

Background

To make a radiobiological comparison, for high risk prostate cancer (T3a, PSA > 20 ng/ml or Gleason > 7) of two radiotherapy treatment techniques. One technique consists of a treatment in three phases of the pelvic nodes, vesicles and prostate using a conventional fractionation scheme of 2 Gy/fraction (SIMRT). The other technique consists of a treatment in two phases that gives simultaneously different dose levels in each phase, 2 Gy/fraction, 2.25 Gy/fraction and 2.5 Gy/fraction to the pelvic nodes, vesicles and prostate, respectively (SIBIMRT).

Materials and methods

The equivalent dose at fractionation of 2 Gy (EQD₂), calculated using the linear quadratic model with α/β_prostate = 1.5 Gy, was the same for both treatment strategies. For comparison the parameters employed were D95, mean dose and Tumour Control Probabilities for prostate PTV and D15, D25, D35, D50, mean dose and Normal Tissue Complication Probabilities for the rectum and bladder, with physical doses converted to EQD₂. Parameters were obtained for α/β_prostate = 1.5, 3 and 10 Gy and for α/β_oar = 1, 2, 3, 4, 6 and 8.

Results

For prostate PTV, both treatment strategies are equivalent for α/β_prostate = 1.5 Gy but for higher α/β_prostate, EQD₂ and TCP, decrease for the SIBIMRT technique. For the rectum and bladder when α/β_oar ≤ 2 Gy, EQD₂ and NTCP are lower for the SIMRT technique or equal in both techniques. For α/β_oar ≥ 2–3 Gy, EQD₂ and NTCP increase for the SIMRT treatment.

Conclusions

A comparison between two radiotherapy techniques is presented. The SIBIMRT technique reduces EQD₂ and NTCP for α/β_oar from 2 to 8 Gy.

1. Background

Advances in radiotherapy delivery and a modern understanding of prostate cancer radiobiology suggest new approaches in dose fractionation to improve prostate cancer control while decreasing treatment-related toxicity.¹ Radiobiologically, slowly proliferating prostate cancer cells are thought to have a low α/β ratio; the α/β ratio of prostate carcinoma is still being discussed but it is well known to be lower than the typical value of 10 Gy of most other solid tumours.^2–4 This low α/β value probably situated between 1.5 Gy and 3 Gy, suggests that prostate cancer has high sensitivity to dose per fraction. Therefore, a hypofractionated radiation delivery regimen, i.e. a large radiation dose in a smaller number of fractions, should be able to increase the therapeutic ratio.^4–6 Furthermore, dose escalation via hypofractionation may be biologically advantageous in the event that surrounding critical organs such as the bladder and rectum have lower sensitivity to fractionation changes, with α/β ratio thought to be between 3 and 6 Gy.^7,8

Usually, high risk prostate tumours are treated in sequential two-phase treatment with an initial irradiation of the pelvic nodes, seminal vesicles and prostate followed by a prostate boost, with doses ranging from 1.8 to 2 Gy per fraction. Mohan et al.⁹ suggested a single-phase approach called simultaneous integrated boost IMRT (SIBIMRT) for head and neck cancers which consists in delivering simultaneously different dose levels to different tissues in a single treatment session. This technique increases simultaneously the target dose conformality and the critical structure sparing.

1.1. Aim

The aim of this study is to make a radiobiological comparison of an IMRT treatment of the pelvic nodes, vesicles and prostate in three phases (SIMRT), using a conventional fractionation scheme of 2 Gy/fraction versus a treatment in two phases that delivers different dose levels simultaneously (SIBIMRT) in each phase. The SIBIMRT technique delivers a higher dose per fraction to the prostate, 2.5 Gy/fraction, and vesicles, 2.25 Gy/fraction, and the same dose as in the three phases treatment to the pelvic nodes, 2 Gy/fraction.

2. Materials and methods

2.1. Patient data

A non-randomised cohort of 20 patients with high-risk prostate adenocarcinoma was selected. The eligible patients had at least one of the following features: clinical Stage T3a disease, prostate-specific antigen level >20 ng/ml, or Gleason score >7. Two treatment strategies were evaluated for these 20 patients. The first treatment modality (SIMRT) consisted of three sequential phases: in the initial phase the pelvic nodes, vesicles and prostate were irradiated to 46 Gy (23 fractions, 2 Gy/fraction), the second phase treated the prostate and vesicles to 24 Gy (12 fractions, 2 Gy/fraction) and the last phase treated the prostate to 12 Gy (6 fractions, 2 Gy/fraction). The total physical doses were 46 Gy for the pelvic nodes, 70 Gy for the vesicles and 82 Gy for the prostate.¹⁰

A different fractionation scheme was introduced^11,12 for the prostate and seminal vesicles hypofractionation with the objective of improving a therapeutic ratio⁵ and reducing treatment time while achieving economical and patient comfort benefits. This second treatment strategy (SIBIMRT) consisted of two phases. The first phase treated simultaneously the pelvic nodes, seminal vesicles and prostate in 23 factions with doses of 46 Gy, 51.8 Gy and 57.5 Gy, respectively. The corresponding doses per fraction were 2 Gy/fraction, 2.25 Gy/fraction and 2.5 Gy/fraction, respectively. The second phase treated simultaneously the vesicles and prostate in 6 fractions with doses of 13.5 Gy, 2.25 Gy/fraction, and 15 Gy, 2.5 Gy/fraction, respectively. The total physical doses for the combination of the two phases were 46 Gy for pelvic nodes, 65.25 Gy for vesicles and 72.5 Gy for the prostate. Total doses were calculated to obtain the same equivalent dose at fractionation of 2 Gy (EQD2) for both treatment strategies, using the linear quadratic model (LQ) with α/β = 1.5.

2.2. Pre-treatment

Patients were instructed to have an empty bladder and rectum for their CT-simulation and for each treatment appointment. Bowel and rectum instructions involved the use of a suppository or enema prior to CT-simulation and before each treatment session. Also a dietary advice was given to patients to prevent changes in the rectum size as much as possible.

2.3. Contouring

The patients were scanned in a supine position in a Somatom Sensation Open CT scanner (Siemens AG., Germany, Munich). A knee rest and ankle support were used. The patients were scanned from L3–L4 down to the top third of the femur in 3-mm slices. The prostate gland (P-CTV), proximal seminal vesicles (SV-CTV), pelvic nodes (PN-CTV), bladder, rectum, and femoral heads were contoured on the CT images with the radiation therapy simulation system Advantage SIM™ MD 6.0.102 (GE Healthcare, Chalfont, and St. Giles, United Kingdom). The prostate base was defined on CT using bladder contrast. P-CTV and SV-CTV were defined as anatomical structures observed in the CT images with no margins.

Prostate, vesicles and pelvic nodes planning target volumes were generated (P-PTV, SV-PTV and PN-PTV, respectively). For P-PTV and SV-PTV, a 6-mm margin was added to P-CTV and SV-CTV respectively, in all directions. A margin of 1 cm in all directions was added to PN-CTV to obtain PN-PTV. The margins are based on our experience in prostate movement using IGRT.¹³

Rectum was defined from the anus to the sigmoid flexure and bladder was contoured entirely.

2.4. Planning

Forward planning IMRT was performed applying an in-house developed prostate class solution.¹⁴ Plans were generated for step and shoot delivery. The planning system employed was Phillips Pinnacle 8.0 h (Phillips, Best, The Netherlands). Table 1 shows the treatment planning details.

Table 1.

Prostate class solution for each treatment phase.

	First phase	Second phase	Third phase
Number and energy of fields	10 18 MV fields	7 18 MV fields
Gantry angles	0° 30° 55° 95° 145° 180° 215° 265° 305° 330°	0° 52° 95° 155° 205° 265° 308°
Total segments	SIMRT: 32 + 5 on average to tailor the plan to the particular patient. SIBIMRT: 32 + 5 previous segments + 6 to boost prostate.	SIMRT: 19 + 3 on average to tailor the plan to the particular patient. SIBIMRT: 19 + 3 previous segments + 3 to boost prostate.	SIMRT: 19 + 3 on average to tailor the plan to the particular patient.
Types of segments	Irradiate the whole planning target volume and improve homogeneity. Block the intersection between PTV and organs at risk to achieve dose constraints. Increase fluency near the OAR to compensate for fluency lost due to previous segments. Compensate for the shape of the contour of the patient and for the heterogeneities. ONLY FOR SIBIMRT: Segments to boost prostate.
Segments weights	Based on the work of Arrans et al. [15]

The arrangement described in Table 1 provides a fast and efficient solution to the prostate case from the perspective of planning and treatment delivery.¹⁴ Fig. 1 shows the total summed dose distribution obtained in a transverse and coronal plane for the SIMRT and for the SIBIMRT techniques.

Fig. 1.

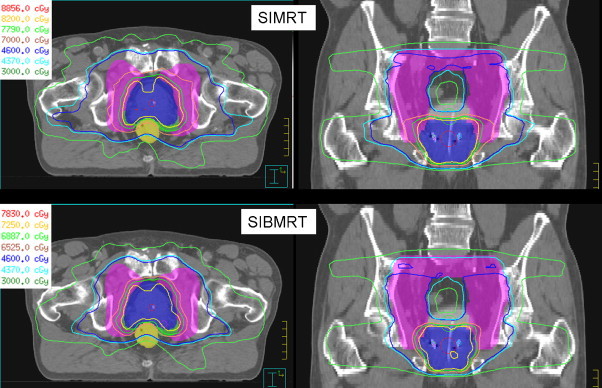
Isodose curves in a transverse and coronal plane for the SIMRT and the SIBIMRT techniques.

2.5. Treatment

Patients were implanted with four gold seeds (3 mm long, 1 mm in diameter) in the prostate gland with the aid of ultrasound medical equipment 1 week before the planning CT scan (two at the base, one at the apex, and one at the centre, as asymmetrically positioned as possible). The seeds were used to position the prostate daily before treatment using the kV image-guided radiotherapy (IGRT) system (ExacTrac X-Ray6D).

2.6. Equivalent dose calculations

To design the fractionation scheme of both treatment strategies, the prescription dose to the prostate and vesicles using the SIBIMRT technique was calculated to obtain the same EQD₂ as for the SIMRT modality. Calculations of EQD₂ were done using the Linear Quadratic Model:

$${\text{EQD}}_2=D\times \frac{d+(\alpha /\beta )}{2+(\alpha /\beta )}$$

where d is the dose per fraction, and D is the total physical dose.

In this study we used the value α/β = 1.5 to calculate prescription doses for the SIBIMRT technique based on predictions of previous studies^2–4 that indicate a low value for the prostate α/β.

To compare dose distributions given at different dose per fraction, EQD₂ was calculated for each voxel separately in all patients and for both treatment modalities. The cumulative dose volume histograms (DVH) were obtained from these EQD₂ dose distributions.

2.7. Parameters

To compare both treatment modalities the parameters analyzed for the prostate PTV were D95 and mean dose with physical doses converted to EQD₂, i.e. EQD₂ received by 95% of volume (EQD₂ 95) and mean EQD₂. These parameters were calculated for α/β_prostate values of 1.5, 3 and 10 Gy.

To compare both treatment modalities the parameters selected for the rectum and bladder were those from RTOG 0415 (D15, D25, D35, D50 and mean dose) with physical doses converted to EQD₂, i.e. EQD₂ received by 15%, 25%, 35% and 50% of the organ at risk volume (EQD₂ 15, EQD₂ 25, EQD₂ 35 and EQD₂ 50, respectively) and mean EQD₂. These parameters were calculated for α/β_oar = 1, 2, 3, 4, 6 and 8.

Tumour Control Probabilities were calculated using a Poisson-based model with the values of D₅₀ and γ₅₀ estimated in the work of Okunieff et al.¹⁶ and for α/β_prostate = 1.5, 3 and 10 Gy.

Normal Tissue Complication Probabilities for the rectum and bladder were calculated using the model of Lyman–Kutcher–Burman (LKB).^17–19 Parameters used in the LKB model for bladder complications were n = 0.12, m = 0.15 and TD50 = 80 Gy from Lyman et al.¹⁷ and for the incidence of late rectal bleeding complications were n = 0.084, m = 0.108 and TD50 = 78.4 Gy from Söhn et al.²⁰ NTCP values were obtained for α/β_oar = 1, 2, 3, 4, 6 and 8.

2.8. Statistical analysis

A two-tailed paired t test was used two compare the treatment strategies. The results were considered significant at the 5% level (p < 0.05).

3. Results

Table 2 shows that for α/β_prostate = 1.5 there is no significant difference for PTV EQD₂ 95 and mean EQD₂ between SIBIMRT and SIMRT modalities. However, for α/β_prostate = 3 and 10 Gy EQD₂ 95 and mean EQD₂ are higher for the SIMRT case, the difference being higher for α/β_prostate = 10 Gy. Table 2 also shows that there is no difference in TCP between both techniques for α/β_prostate = 1.5, whereas for α/β_prostate = 3 and 10 Gy, TCP is higher in the 3 phases technique.

Table 2.

Mean value and standard deviation for EQD₂ 95, mean EQD₂ and TCP for prostate PTV, α/β = 1.5 Gy, 3 Gy and 10 Gy for SIBIMRT and SIMRT modalities.

	α/β = 1.5 Gy			α/β = 3 Gy			α/β = 10 Gy
	SIBIMRT	SIMRT	p	SIBIMRT	SIMRT	p	SIBIMRT	SIMRT	p
EQD295 (Gy)	79 ± 2	79 ± 2	0.6	77 ± 1	79 ± 2	<0.05	73 ± 1	80 ± 1	<0.05
Mean EQD2 (Gy)	87 ± 1	86 ± 1	0.1	83 ± 1	85 ± 1	<0.05	78 ± 1	85 ± 1	<0.05
TCP (%)	93.4 ± 0.5	93.0 ± 0.4	0.3	92.0 ± 0.6	93.0 ± 0.4	<0.05	89.6 ± 0.6	92.8 ± 0.3	<0.05

Fig. 2 shows that for α/β_oar = 1 Gy there is no significant difference between both modalities for the rectum EQD₂ 15, EQD₂ 25, EQD₂ 35, EQD₂ 50 and mean EQD₂. However, for α/β_oar between 2 and 8 Gy those values increase in the SIMRT treatment, the difference being higher when the α/β_oar value rises.

Fig. 2.

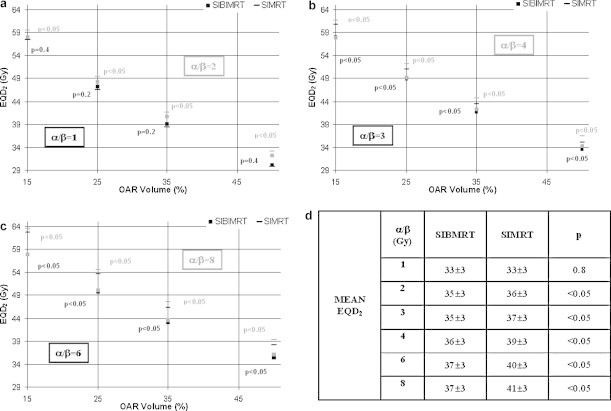
Mean rectum EQD₂ 15, EQD₂ 25, EQD₂ 35 and EQD₂ 50 for SIMRT and SIBIMRT techniques and for α/β_oar values of 1, 2 (a) 3, 4 (b) and 6, 8 Gy (c). The black and grey colours correspond to the lower and higher α/β_oar values in each subfigure. For each EQD₂ the p value is indicated in the graph. (d) Mean rectum EQD₂ for SIMRT and SIBIMRT techniques for α/β_oar = 1, 2, 3,4, 6 and 8 Gy.

A similar behaviour is found for the bladder. Fig. 3 shows that for α/β_oar = 1 Gy, bladder EQD₂ 15 is higher in the SIBIMRT technique, whereas there is no significant difference between both modalities for bladder EQD₂ 25, EQD₂ 35, EQD₂ 50 and mean EQD₂. However, for α/β_oar between 2 and 8 Gy these values increase in the SIMRT treatment, the difference being higher with rising α/β_oar values.

Fig. 3.

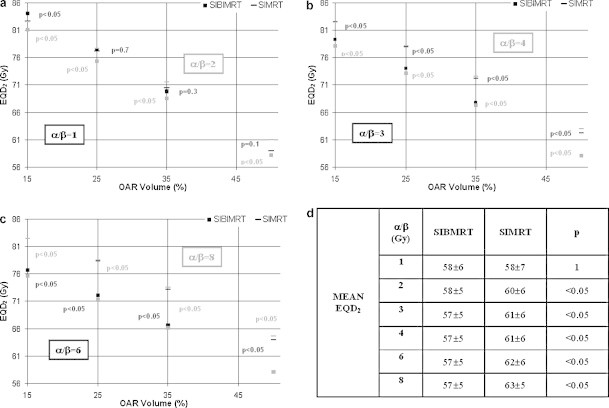
Mean bladder EQD₂ 15, EQD₂ 25, EQD₂ 35, EQD₂ 50 for SIMRT and SIBIMRT techniques and for α/β_oar values of 1, 2 (a) 3, 4 (b) and 6, 8 (c). The black and grey colours correspond to the lower and higher α/β_oar values in each subfigure. For each EQD₂ the p value is indicated in the graph. Mean bladder EQD₂ for SIMRT and SIBIMRT techniques for α/β_oar = 1, 2, 3, 4, 6 and 8 Gy.

Fig. 4 shows that for α/β_oar = 1 Gy rectum NTCP is lower for the SIMRT than for the SIBIMRT treatment, while for α/β_oar = 2 Gy no significant difference was found between both techniques. In contrast, for α/β_oar ≥ 3 rectum NTCP increases for the SIMRT technique. For bladder NTCP and α/β_oar = 1 Gy, no significant difference was found between both techniques, however, for α/β_oar ≥ 2 Gy, NTCP increases for the SIMRT technique. For both organs at risk the difference between both treatments increases when α/β_oar rises.

Fig. 4.

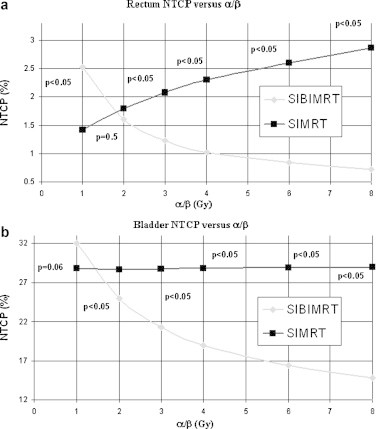
Rectum (a) and bladder (b) NTCP versus α/β_oar with α/β_oar = 1, 2, 3, 4, 6 and 8 Gy. For each NTCP the p value is indicated in the graph.

4. Discussion

The EQD₂ calculations were done using the LQ model that can be used if the following assumptions are made:

There is no repair of sublethal damage during irradiation. Wang et al.² reported a repair half time value of the prostate of 16 min. For the bladder and rectum, the repair half time found in the literature is between 0.2 and 2 h.²¹ The prostate treatment duration using external beam radiotherapy in our centre is around 5 min, therefore, it is a reasonable assumption to neglect repair during irradiation. A longer treatment time or a shorter repair half time would result in an EQD₂ reduction.^22,23 This would be advantageous for OARs in an hypofractionated scheme but could decrease tumour control, which is undesirable.

Sufficient time is allowed for complete repair of sublethal damage between fractions. In our centre the radiotherapy treatment is fractionated with an interfraction interval of 1 day. In this case, this assumption is fully accomplished.^2,21

There is no repopulation during the overall fractionated radiotherapy treatment. This is a reasonable assumption for prostate carcinoma which can be considered as a late-reacting tissue in which little accelerated repopulation of clonogenic tumour cells occurs during the RT course.^24,25

There is a high uncertainty in the available data for radiobiological parameters: sublethal repair half time, time after the start of treatment when proliferation starts, etc. Also, many assumptions are made in the LQ model. Therefore, EQD₂ calculations cannot be used as predictor outcomes for individual patients. However, if a consistent set of parameters is managed, as is the case of this work, EQD₂ can be used for two purposes: to design treatment strategies that should be tested clinically in the future and to compare different modality strategies with different fractionation.⁹

For NTCP and TCP calculations, there is no consensus on the values used for the parameters n, m and TD50 for the LKB model and D₅₀ and γ₅₀ for the Poisson-based TCP model. Due to the uncertainty in the model parameters, the NTCP and TCP cannot be used as a confident predictor of organ at risk toxicity or tumour control, however, they can be used for comparing treatment modalities in a relative sense.¹⁸

The results obtained for prostate PTV indicate that if α/β_prostate = 1.5 Gy both treatment techniques are equivalent in terms of target coverage and TCP. This was expected because α/β_prostate = 1.5 Gy is the value hypothesized in this work to design the SIBIMRT treatment strategy. For higher α/β_prostate values, the dose per fraction has less effect over the resulting EQD₂, and it becomes more similar to the physical dose. Consequently, for α/β_prostate higher than 1.5, SIMRT and SIBIMRT are not equivalent and the last one results in less EQD₂ to the tumour. It has to be noted that the reduction in EQD₂95, mean EQD₂ and TCP is 2 Gy, 1 Gy and 1% for α/β_prostate = 3 and 7 Gy, 7 Gy and 3.2% for α/β_prostate = 10, therefore, for α/β_prostate = 3 Gy, although a significant difference between both techniques exist, this difference is very low.

It has been observed that for α/β_oar = 1 Gy, EQD₂ values for the rectum and bladder are equivalent for both treatment techniques or, in the case of EQD₂15, superior for the SIMRT. However when α/β_oar ≥ 2 Gy, EQD₂ values for the SIMRT technique are higher.

The EQD₂ reduction for OARs for the SIBIMRT technique is due to the combination of two facts. On one hand, when the α/β_oar value rises the resulting EQD₂ is more similar to the physical dose which is lower for the SIBIMRT technique. Therefore, it is natural to have a reduction in EQD₂ given to OARs when the α/β_oar value rises. On the other hand, for α/β_oar values as low as 2 Gy, EQD₂ has also been observed to be lower. The reason for this reduction could be the most conformal dose distribution when designed to be delivered as a SIBIMRT. When a treatment is planned to be delivered sequentially, tissues irradiated during the large field phase receive undesired additional dose during the boost phase. Dose distributions of SIBIMRT are significantly superior in terms of conformality because they are designed to simultaneously deliver different dose levels to different tissues in a single treatment session and the extra dose given to the tissues surrounding the boost area is optimized to be as low as possible (Fig. 1).⁹

The results for α/β_oar = 1 can be explained by the higher dose per fraction to organs at risk of the SIBIMRT technique, which results in EQD₂ increasing for very low α/β_oar values. Despite of the improved dose conformality of this treatment modality EQD₂ given to OARs, when treating the prostate and pelvic nodes simultaneously, is equivalent or in some cases higher for the SIMRT technique. However, the α/β_oar values proposed in the literature for the rectum and bladder are thought to be between 3 and 6 Gy.^7,8 For these values, the SIBIMRT technique is clearly superior to the SIMRT in terms of dose to organs at risk.

The NTCPs show a similar tendency to the dosimetric parameters. For α/β_oar ≥ 3 Gy for the rectum and α/β_oar ≥ 2 Gy for the bladder, the EQD₂ reduction that shows the SIBIMRT technique results in a lower NTCP value. For α/β_oar ≤ 2 Gy for the rectum and α/β_oar ≤ 1 Gy for the bladder, the higher dose per fraction of the SIBIMRT affects the NTCP obtaining equivalent values for both techniques or even a lower NTCP value with the SIMRT technique. For the α/β_oar values recommended in the literature, i.e. between 3 and 6 Gy, there is an important reduction in the NTCP values and, therefore, it is clearly more advantageous to treat the patients with the SIBIMRT. Although α/β_oar values are delimited in the literature, this work analyses changes in EQD₂ and NTCP for a wide range of α/β_oar's to predict what could happen if the values referenced were not exact or if they were different for a particular patient.

The results obtained for NTCP show a very high value for the bladder. Although this value is improved with the SIBIMRT technique it is still very high. Probably these high NTCP values are due to high doses received in the intersection of the bladder and the PTV. A more realistic value may be obtained if the organ considered is the bladder without the intersection with PTV. It is important to note that patients are treated with an empty bladder.

A more advantageous solution than the one used in this work would be to treat the patients with the SIBIMRT technique in just one phase because it reduces treatment duration, which benefits the patient while reducing logistic and financial requirements. However, a new study should be done in terms of radiobiological parameter calculations to check if the SIBIMRT is still superior to the SIMRT. Rising dose per fraction to prostate also increases dose per fraction to organs at risk, which could increase EQD₂ and NTCP values for low and medium α/β_oar values. Also, it would be difficult to optimize the dose to the pelvic nodes nearest to the prostate PTV and they would receive higher doses than the prescribed dose.Conclusion

The current trend in prostate radiotherapy is hypofractionation based on the low α/β_prostate estimation of many studies.⁵ In this work, a technique was introduced to hypofractionate prostate in high risk prostate cancer, which reduces or achieves equal EQD₂ and NTCP even for very low α/β_oar values. Also, for the α/β_oar values proposed in the literature for the rectum and bladder, there is a significant reduction in EQD₂ and NTCP and this reduction is more important for higher α/β_oar values. Only in the improbable case of α/β_oar = 1, rectum NTCP and EQD₂15 for the bladder are higher for the SIBIMRT technique.

Conflict of interest

None.

Financial disclosure

Not applicable.