Beyond the diffusion limit: Water flow through the empty bacterial potassium channel

Abstract

Water molecules are constrained to move with K⁺ ions through the narrow part of the Streptomyces lividans K⁺ channel because of the single-file nature of transport. In the presence of an osmotic gradient, a water molecule requires <10 ps to cross the purified protein reconstituted into planar bilayers. Rinsing K⁺ out of the channel, water may be 1,000 times faster than the fastest experimentally observed K⁺ ion and 20 times faster than the one-dimensional bulk diffusion of water. Both the anomalously high water mobility and its inhibition observed at high K⁺ concentrations are consistent with the view that liquid–vapor oscillations occur because of geometrical confinements of water in the selectivity filter. These oscillations, where the chain of molecules imbedded in the channel (the “liquid”) cooperatively exits the channel, leaving behind a near vacuum (the “vapor”), thus far have only been discovered in hydrophobic nanopores by molecular dynamics simulations [Hummer, G., Rasaiah, J. C. & Noworyta, J. P. (2001) Nature 414, 188–190; and Beckstein, O. & Sansom, M. S. P. (2003) Proc. Natl. Acad. Sci. USA 100, 7063–7068].

Water molecules and K⁺ ions move through the narrow part of the Streptomyces lividans K⁺ (KcsA) channel by a single-file process, that is, they cannot pass each other within the channel. Movement of dehydrated H₂O through the channel's selectivity filter that is designed to handle dehydrated K⁺ ions (1) may be rate-limiting for both ion and water transport. Within the channel, water molecules are unable to form the usual number of interwater hydrogen bonds. This change of hydration environment may be the major transport barrier, similar to what has been observed in other single-file processes (2). Mainly because of differences in the hydrogen bond-mediated enthalpic barriers, the velocity of water movement varies as much as 100-fold between different narrow pores (3).

Two of the four ion-binding sites of the KcsA selectivity filter are found to be occupied almost all of the time by dehydrated K⁺. A third K⁺ resides at the center of a water-filled cavity located halfway across the membrane (4). Structural data suggest that, even at low millimolar K⁺ concentrations, there would have to be at least one ion in the filter (5) to compensate for the partial charges from carbonyl groups and, thus, to stabilize the tetrameric channel assembly. The empty KcsA channel has not been observed in x-ray crystallographic measurements (5). Already the loss of one of the two dehydrated K⁺ ions is accompanied by compensatory structural changes that are believed to render the channel nonconductive (1), which is exactly what is expected if K⁺ ions in the pore are rinsed out by osmotic water flow (i.e., volume flux through the channel shifts the equilibrium from its conducting to its nonconducting conformation). Thus, the selectivity filter is predicted to close in the presence of an osmotic gradient. A paradoxical picture emerges: Although water-permeable, the KcsA channel should not allow osmotic water flow. Being pushed by osmotic pressure, extremely fast water molecules crossing the channel in only 10 ns along with accompanying ions (1, 5, 6) are anticipated to stop suddenly. In contrast to electroosmosis (water is dragged by ions), solvent drag (ions are dragged by water) should be forbidden, because in its low K⁺ coordination structure, the selectivity filter is impermeable.

To test this hypothesis, we measured osmotic water flow through purified KcsA channels (7, 8) by imposing an osmotic gradient across reconstituted planar bilayers and detecting the resulting small changes in ionic concentration close to the membrane surface. This approach has been used for water flux measurements through peptide channels (9, 10), water channels (11, 12), lipid bilayers (13, 14), and epithelial cells (15). Now we report that osmotic volume flow depletes K⁺ out of the KcsA protein. The one-dimensional file of water molecules interacting with the pore moves 1,000 times faster than ions through the channel, indicating that ion transport is rate-limiting. The high water-transport rate is consistent with the view that liquid–vapor oscillations occur in the channel. They represent bursts of collective water motion that are followed by a transition to the loosely packed (vapor) state of the channel as visualized for water conduction through hydrophobic nanotubes by recent molecular dynamics simulations (16, 17) and random-walk studies (18).

Materials and Methods

Water concentrates the solution it leaves and dilutes the solution it enters (19). To monitor these concentration changes, which are restricted to stagnant water layers in the immediate membrane vicinity, Mg²⁺- and K⁺-sensitive microelectrodes made of glass capillaries were used. Their tips (1–2 μm in diameter) were filled with mixture A of magnesium ionophore II or mixture B of potassium ionophore I (both Fluka), respectively. Stepwise movement of the electrodes relative to the membrane was realized by a hydraulic step drive (Narishige, Tokyo). The dependence of solute concentration at the interface, C_s, from distance, x, to the membrane was fitted by the equation C(x) = C_sexp(–vx/D + bx³/3D) to reveal the linear drift velocity of the osmotic volume flow –ν and the stirring parameter b (13). D is the solute-diffusion coefficient. ν is related to the osmotic water permeability P_f by P_f = ν/(C_osmV_w), where C_osm and V_w are the transmembrane osmotic gradient and the molecular volume of water (20), respectively. C_osm was obtained to correct the bulk urea concentration for dilution in the immediate membrane vicinity (21). At its final concentration of 1 M, urea augments the kinematic buffer viscosity by only 2% (22), which results in a negligible error in the determination of ν (21).

Functional KcsA reconstitution into a bilayer (11, 23) made from Escherichia coli lipids (Avanti Polar Lipids) was confirmed by simultaneous measurements of membrane conductance. Two pairs of electrodes were exploited. The first pair of Ag/AgCl pellets was used to monitor the current step. A 1-kHz square wave-input voltage (source: model 33120A, Hewlett–Packard) was applied to the membrane. The output signal was amplified by a current amplifier (AD549, Analog Devices, Norwood, MA) and visualized by an oscilloscope (model TDS 220, Tektronix). Through the second pair of pellets, the resulting potential difference was recorded by an impedance converter (AD549) and displayed on the second channel of the oscilloscope.

Results

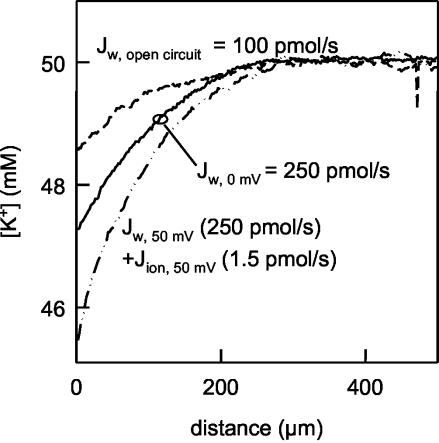
By using two pairs of Ag/AgCl pellets and a 1-kHz square wave-input wave, a conductance of 3 μS was determined, corresponding to 50,000 open channels with a unitary conductance, g, of 40 pS (6). Determination of water flux, J_w,c, across the channel requires the water flux across the lipid bilayer, J_w,l = ν/V_w = 100 pmol/s, to be taken into account. It was derived from the K⁺ concentration profile recorded in an open-circuited configuration (9) (Fig. 1). An open-circuited membrane containing only cation-selective channels allows for short-term voltage fluctuations, but in the long term, the number of K⁺ ions exiting and entering on one side of the channel must be equal (24). Because of the lack of a net cation flux and the single-file nature of transport, both K⁺ ion and water fluxes across the KcsA channel are inhibited. J_w,l corresponds well to the lipid water permeability, P_f,l = 20 μm/s, known for model membranes from E. coli lipids (11, 12). Thus, there are no defects in membrane integrity at the lipid–protein interface. Under short-circuited conditions, both J_w,l and J_w,c contribute to the K⁺ profiles, adding to the total flux J_w. From concentration profiles of impermeable solutes, J_w is usually determined with an error well below 10% (13). J_w,c (equal to 150 pmol/s), derived from the concentration profile of the permeable K⁺ ions, represents the lower limit of the actual water flow, because K⁺ ions that move along the transmembrane electrochemical concentration gradient (pseudo solvent drag) and K⁺ ions that are dragged through the channel by water (true solvent drag) lead to an underestimation of J_w (9). Still, J_w,c is much larger than J_ion (J_ion = I/zF = 1.5 pmol/s at 50 mV). It is concluded that at a potential of 0 mV, KcsA channels transport at least 100 water molecules per ion. Streaming potential measurements indicate that every ion is accompanied by a minimum of two and a maximum of four water molecules moving together in a single file (25). There is no contradiction in the stoichiometries obtained by flux (Fig. 1) and streaming potential measurements (25) if most of the channels most of the time do not contain an ion. This situation is well known from gramicidin channels, which may transport thousands of water molecules before an ion passes through (9, 26), but once an ion has entered the channel, it is accompanied by five water molecules in a single file (9, 27). However, a KcsA channel empty of ions is in sharp contrast to the common belief that any K⁺ channel contains several ions at any time, which cannot be passed by water molecules (28).

Fig. 1.

Water and ion fluxes through planar bilayers reconstituted with purified KcsA. The K⁺ flux was equal to 1.5 pmol/s at a stationary potential of 50 mV, as revealed by current measurements. It was accompanied by a voltage-insensitive water flux of at least 250 pmol/s, as derived from the K⁺ dilution in the immediate vicinity of a membrane clamped to 0 mV. Cation polarization was measured by scanning microelectrodes at the indicated distances from planar lipid bilayers. In an open circuit, there is no ion transport across a membrane containing only cation-selective channels. Because of the single-file nature of transport, water flow is inhibited also. It is conducted only by the lipid bilayer, which enables a flux of 100 pmol/s. The buffer contained 50 mM KCl, 100 mM choline chloride, 100 μM MgCl₂, and 10 mM Hepes. Osmotic water flux was induced by 1 M urea.

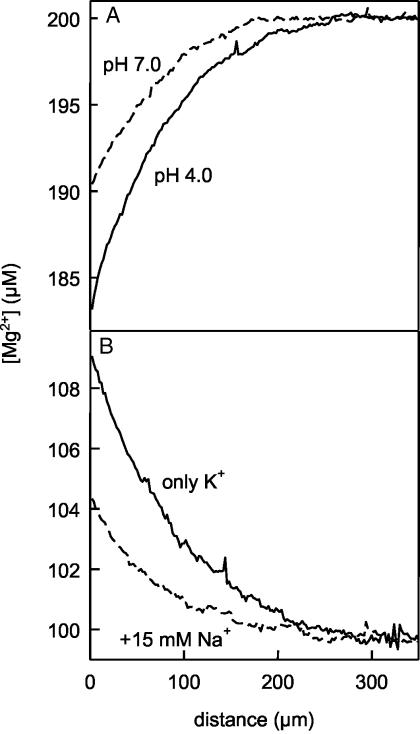
Although being hydrophobic, the closed gate is still wide enough to accommodate a water molecule. To test experimentally whether the closed conformation is water-permeable, the pH dependence of the gate conformation is exploited. It opens at pH 4 and closes at pH 7 (29). In these experiments, J_w is determined from the dilution of the impermeable Mg²⁺ ions within the hypotonic compartment immediately adjacent to the membrane (Fig. 2). At pH 7, J_w is close to J_w,l, indicating that the dilution of Mg²⁺ ions (Fig. 2 A) is caused by water flow across the lipid matrix. At pH 4, a much higher-concentration polarization of the impermeable ion is measured in the vicinity of the same bilayer. The incremental P_f,c (P_f,c = P_f – P_f,l) is caused by water flow across the KcsA channels, which now have opened, as revealed by the increase of electrical conductance (Fig. 2 A). Thus, acidic pH is required to open the channel for both K⁺ and water. Closing the channel by pH and adding Na⁺ both inhibit water flow, suggesting that the block of electrical current by sodium (7) also blocks water movement (Fig. 2B). It is concluded that the extraordinarily large water conductivity is mediated by KcsA and that there is no water flow in the region of the protein–lipid interface.

Fig. 2.

Gating (A) and block (B) of water transport. (A) The osmotic water permeability (46 μm/s) was determined (13) from spatially resolved measurements of Mg²⁺ dilution in response to a 1 M urea gradient at pH 4. Augmenting pH to 7 closed the KcsA channels, as indicated by the increase of the electrical membrane resistance (measured at a frequency of 1 kHz), R, from 8 × 10⁵ to 10⁸ Ω. Simultaneously, channel closing decreased P_f to 25 μm/s. (B) Channel block by 15 mM Na⁺ changed R and P_f from 5 × 10⁶ to 5 × 10⁸ Ω and from 35 to 24 μm/s, respectively.

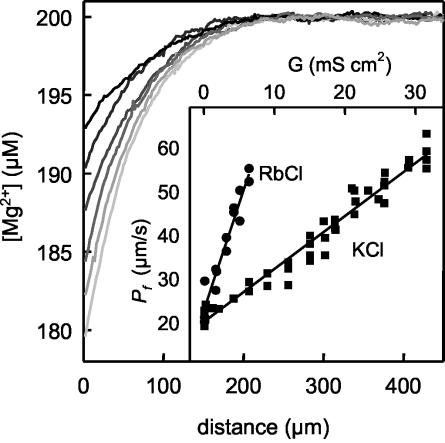
The single channel water permeability, p_f is obtained according to p_f = P_f,cAg/G, where the ratio of membrane, G, and single-channel, g, conductivities reflects the number of activated KcsA channels in the membrane occupying the area A (9, 20). Reconstitution of increasing amounts of KcsA (Fig. 3) allowed construction of a plot of P_f,c against G. The slope revealed a p_f of 4.8 × 10^–12 cm³/s. With only 6–11 × 10^–14 cm³/s (30, 31), p_f of a channel specialized in water transport, aquaporin-1, is much smaller. The KcsA channel seems to have the highest water permeability ever reported for single-file transport. p_f is 20-fold larger than predicted by simple diffusion across a cylindrical pore (10, 20) of length, L, = 1.2 nm, p_f,theory = V_wD_wN/N_AL² = 2 × 10^–13 cm³·s^–1, where V_w, D_w, N_A, and N are the molecular volume of water, the bulk water-diffusion coefficient, Avogadro's number, and the number of water molecules in the selectivity filter, respectively. Inadequacies of this model have been recognized before, because also the water permeabilities of desformylgramicidin (p_f = 1.1 × 10^–12 cm³/s) (10) or aquaporin-4 (p_f = 2.4 × 10^–13 cm³/s) (31) are higher than predicted. p_f allows calculation of the water-turnover number of the channel (32): M_w = N_Ap_f/V_w = 1.6 × 10¹¹ molecules per s. The maximum possible unidirectional ion flux M_ion occurring when the filter always contains at least one ion (32) is then M_ion = M_w/R = 10¹¹ ions per s, where R = 2 denotes the number of water molecules transported along with one ion (25). From the maximum single-channel conductance of 300 pS (6), the maximally observed unidirectional flux of potassium is 10⁸ ions per s. Thus, it is 3 orders of magnitude smaller than M_ion, suggesting that the K⁺ flux through the pore is limiting.

Fig. 3.

Single-channel water permeability. Membrane conductance, G, was measured in a four-electrode configuration. The black line denotes Mg²⁺ dilution near the unmodified bilayer by osmotic flow. Increase in the number of reconstituted KcsA channels (8,300, 17,000, 25,000, 36,000, and 45,000 channels with a unitary conductance of 40 pS) resulted in increasing concentration polarizations (from dark gray to light gray), which allowed calculation of the corresponding P_f values (27, 34, 43, 51, and 59μm/s, respectively). (Inset) Four additional runs of the experiment and three similar experiments, in which 50 mM K⁺ were substituted for 25 mM Rb⁺, were analyzed. From the slope of the plot P_f versus G and the known single-channel conductances of 40 and 10 pS for K⁺ and Rb⁺, respectively, p_f was calculated (9) to be 4.8 × 10^–12 cm³·s^–1.

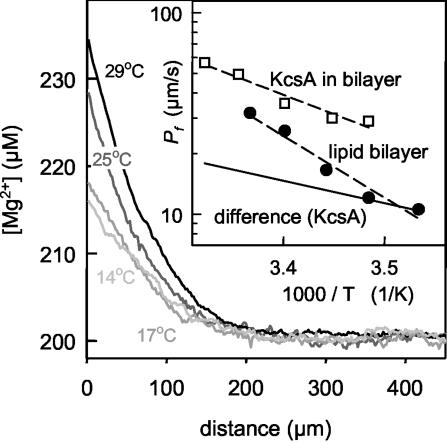
Exploiting its temperature dependence, we measured the energy barrier for fast water transport. With 5.1 ± 0.3 kcal/mol (Fig. 4), it is above the energy barrier for K⁺ ions (2.7 kcal/mol) found for the multiple occupied channel (33) but still in the range usually observed for transport across water-filled pores (11, 30, 34–36). It is the mutual destabilization resulting from the high ionic occupancy that leads to the unusually small activation energy for K⁺ ions. For lower occupancies, the energy barrier for K⁺ conduction is ≈7 kcal/mol higher, because the resident ions bind too tightly to the pore (37).

Fig. 4.

Activation energy for water flux across KcsA channels. Osmotic volume flux-induced Mg²⁺ dilution was measured as a function of temperature. Reconstitution of KcsA channels increased G from 3 nS·cm^–2 (bare bilayer) to 20 mS·cm^–2. (Inset) Arrhenius plot for water flow across protein-free lipid bilayers and KcsA containing bilayers. From the slope, an activation energy of ≈14 kcal/mol was obtained for protein-free lipid bilayers (filled circles). Protein reconstitution decreased the activation energy (open squares). The incremental water permeability caused by KcsA corresponds to an activation energy of 5.1 kcal/mol (solid line).

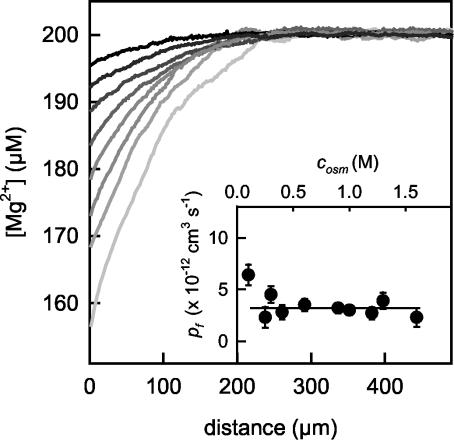
Ion removal by osmotic water flow suggests that p_f decreases as the osmotic gradient itself decreases. In contrast, the experiment revealed an invariant p_f over the whole osmotic pressure interval (Fig. 5), indicating an all-or-none mechanism of the “fast” water-transport mode. The higher p_f estimate obtained at the lowest osmotic driving force does not compromise this result, because it reflects methodological limitations arising when the noise of the microelectrode becomes comparable to the voltage (concentration) changes that are detected. The hypothesis about the all-or-none mechanism implies that the fast turnover of 1.6 × 10¹¹ molecules per s is inhibited every time an ion enters the pore, because subsequent dehydration and rehydration reactions of K⁺ allow only turnover rates <10⁹ molecules per s (“slow” transport mode). Consequently, under the condition in which most of the channels contain at least one ion, the transport rate of water should drop down to the rate of K⁺. In agreement with this prediction, a steep change in P_f,c with K⁺ concentration was observed. It dropped to a value close to zero in a single step (Fig. 6). The underlying drastic decrease of p_f rules out that KcsA transports both water and K⁺ in the fast mode. Otherwise, the decrease in p_f had to occur in four steps, mirroring subsequent binding events of K⁺ to the four binding sites (24): p_f,occupied/p_f = (1 + a/K₁ + a²/K₁K₂ +...)^–1, where K₁, K₂,... are the dissociation constants for the first, second,... K⁺ ions and a is their activity. Because the osmotic force sweeps ions out of the channel, the inhibition does not occur in the range of K₁ = 1 μM (37). It is observed at 0.2 M K⁺, i.e., at a concentration ensuring partition of a second ion into the selectivity filter before the first is squeezed out. In the absence of an osmotic pressure, this K⁺ concentration would correspond to the occupancy of three of four binding sites (38).

Fig. 5.

p_f does not depend on the driving force. An increase of the osmotic gradient from 0.1 to 1.5 M urea (from dark to light gray) resulted in an increased Mg²⁺ dilution close to the membrane, allowing determination of P_f. (Inset) From G measured simultaneously, the number of open channels and thus the single-channel hydraulic conductivity were calculated. p_f was constant over the interval of applied osmotic pressure.

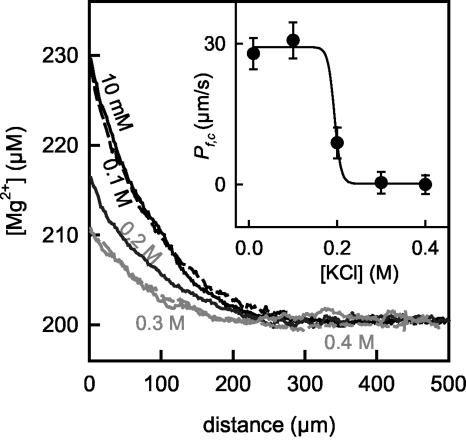
Fig. 6.

All-or-none mechanism of fast water conductance: inhibition of fast water transport by K⁺. G was equivalent to ≈20,000 open channels. At 10 and 100 mM KCl, the channels mediated a water permeability P_f,c of ≈30 μm/s (black solid and dashed lines). At 0.3 and 0.4 M K⁺, P_f,c was immeasurably small (Inset), indicating inhibition of the fast transport mode (gray solid and dashed lines). At the intermediate 0.2 M K⁺ concentration (dark gray), part of the channels showed high water permeability.

Discussion

Confinement to a single file and interaction with molecules forming the channel wall is expected to affect water diffusion. The resulting 20-fold increase of the water diffusion coefficient, however, is unparallel. Among the transported species, water molecules heavily outnumber K⁺ ions. This observation indicates that the channel most of the time does not contain an ion. The ion concentration in the filter is unlikely to be increased by fluctuations of occupancy, because the ion cannot exit and reenter the filter without regaining and loosing its waters of hydration. The rate constant is ≈10⁹ s^–1 for these substitutions of single-hydration water molecules in the inner shell of K⁺ (39), i.e., it is 2 orders of magnitude slower than the turnover number found for water molecules. In contrast, M_w agrees well with the known value of 10¹¹ s^–1 for H₂O exchange around another H₂O molecule (39). Although resulting in K⁺ depletion, osmotic water flow does not cause (i) the channel to enter the closed state or (ii) the tetrameric channel assembly to fall apart. Conceivably, the channel is stabilized in its conducting conformation by short-term visits of K⁺, which take place every 0.1 μs according to the ion water-flux ratio. Even after total removal of permeant ions, it may require minutes before an irreversible loss of channel activity occurs (40).

A channel empty of ions contrasts with electrophysiological measurements in which movement of a tracer ion was found to sweep other ions with it (28). A dissociation constant in the submillimolar range (37) buttresses the general picture that K⁺ inside the selectivity filter is an essential part of the structure of the protein. Most costly, from an energetic point of view, is the removal of the last ion. The calculated energy of the protein with three K⁺ in the cavity is 8.5 kcal/mol (41). The difference between one and three ions is at maximum 10 kcal/mol (41). Thus, E = 18.5 kcal/mol is the upper limit for the binding energy of the first K⁺. The resulting force required to drag one ion through the filter is F_b = E/LN_A = 100 pN. To empty the channel, the osmotic force, F_o, acting on the particle has to be larger than F_b. Because the actual osmotic gradient across the narrow part is difficult to assess, the frictional force that is equal in size is used for calculations (20), F_o =Nγν = NkTM_wL/D = 1600 pN, where γ is the frictional coefficient and ν is the velocity. Because F_o » F_b, it is likely that the osmotic gradient sweeps ions out of the channel. Consequently, the activation energy of water permeation across the channel should result from a superposition of ion removal and water transport. Given the ratio of ion and water fluxes, the impact of the former should be small. As expected, the energy barrier of 5.1 kcal/mol (Fig. 4) is close to that of a typical water channel (11) and matches the energy enough to functionally close a channel (42).

In contrast to other single-file transport processes (32), ion movement was found to be not limited by water transport. A possible explanation for the incredible high rate of water movement in an osmotic gradient may be provided by capillary evaporation. The extreme H₂O diffusion rate across KcsA is still >2 orders of magnitude smaller than in bulk vapor. Recently, liquid–vapor oscillations have been observed by molecular dynamics simulations in narrow channels (17) or nanopores (16). Confinement to narrow pores, however, may result in freezing of water molecules (43) as well. Crucial for freezing or vaporization to occur is the exact geometry of the channel.

The vapor theory is consistent with the view that the change from the fast to the slow transport mode occurs in a single step. Occupancy of the selectivity filter by two ions does not leave enough room to accommodate a vaporized water molecule, because the distance between two such water molecules exceeds the filter length (17).

Our results clearly show that in single-file transport, water molecules are more than just spacer molecules between the ions. They are not only required for electrostatic reasons but add important features to the overall transport process. Whether the fast transport mode occurs in Na⁺, Ca²⁺, other K⁺ channels, or even in members of the aquaporin family, which all realize single-file transport (44, 45), as well as the physiological importance of this phenomenon remain to be elucidated. With respect to the high density of K⁺ channels in the nodes of Ranvier (44), for example, a contribution to water homeostasis in neurons is likely.