A bias-free measure of retinotopic tilt adaptation

Abstract

The traditional method of single stimuli for measuring perceptual illusions and context effects confounds perceptual effects with changes in the observer's decision criterion. By deciding consciously or unconsciously to select one of the two response alternatives more than the other when unsure of the correct response, the observer can shift his or her psychometric function in a manner indistinguishable from a genuine perceptual shift. Here, a spatial two-alternative forced-choice method is described to measure a perceptual aftereffect by its influence on the shape of the psychometric function rather than the mean. The method was tested by measuring the effect of motion adaptation on the apparent Vernier offset of stationary Gabor patterns. The shift due to adaptation was found to be comparable in size to the internal noise, estimated from the slope of the psychometric function. By moving the eyes between adaptation and test, it was determined that adaptation is retinotopic rather than spatiotopic.

Introduction

Previous investigations of the tilt aftereffect and other “illusions” have typically used the method of single stimuli (MSS) to measure the shift of the mean (50%) point of the psychometric function (Knapen, Rolfs, & Cavanagh, 2009; McGraw, Whitaker, Skillen, & Chung, 2002; Zimmermann, Morrone, Fink, & Burr, 2013). The problem with this method (MSS) is that it confounds any perceptual effect with a change in the observer's decisional criterion. For example, merely by responding “leftward” when uncertain in a Vernier alignment task, observers can shift the mean point of their psychometric functions with no change in slope (Morgan, Dillenburger, Raphael, & Solomon, 2012). It is thus unclear whether small shifts in the psychometric function can be taken as evidence for a genuine perceptual bias, for example, the motion aftereffect caused by imagining the adapting stimulus (Winawer, Huk, & Boroditsky, 2010). Even in the case of incontrovertible perceptual effects that can be proven by demonstration, we cannot be certain that parametric measurements of their magnitude by MSS are accurate (Harris & Morgan, 1993; Harris, Morgan, & Still, 1981). The confounding of decisional and perceptual biases in MSS may explain conflicting results concerning the retinotopy versus spatiotopy of the tilt aftereffect (Knapen et al., 2009; Turi & Burr, 2012; Zimmermann et al., 2013) and the effects of attentional load on motion adaptation (Morgan, 2011; Taya, Adams, Graf, & Lavie, 2009).

Garcia-Perez and Alcala-Quintana (2012) have pointed to the advantages of using two-alternative forced choice (2AFC) to measure perceptual biases separately from response biases. The 2AFC (Blackwell, 1952) or 4AFC (Jakel & Wichmann, 2006) procedure has been mainly advocated as a measure of sensitivity rather than bias. In a 2AFC procedure, the test stimulus can occur in either the first or second interval or position, so a response bias cannot be confused with a bias toward the test. However, as soon as the intervals or positions are distinguished by the presence of adapting stimuli or some other context, a perceptual bias will be indistinguishable from an interval or position bias. For example, Gheorghiu, Kingdom, Bell, and Gurnsey (2011) used spatial 2AFC with two different adapting stimuli in different locations and two different tests in the same location as the adaptors. The difference between the tests was adjusted, keeping their geometric mean constant, in order to find the point at which the two tests seemed the same. Suppose that the observer selects the upper test when unsure of the direction of the difference. This bias will be indistinguishable from a perceptual bias induced by the adaptors. Garcia-Perez and Alcala-Quintana suggest overcoming this problem by introducing a third “unsure” response alternative. This method is certainly capable of revealing illusions (Garcia-Perez & Alcala-Quintana, 2012) and the effects of adaptation (Morgan, 2012), but it incorporates the assumption that the observer uses a symmetrical criterion for certainty. An asymmetrical criterion would once again be indistinguishable from a perceptual bias due to adaptation. Nor is there anything in the procedure to define for the subject when the “unsure” button is to be used. In effect, the three-button procedure is a partial replacement of forced choice with a measure based on confidence rating.

Another solution to the problem of measuring adaptation and illusions without criterion bias uses multiple interleaved test conditions, analogous to the use of a roving pedestal in discrimination studies (Macmillan & Creelman, 2009). Morgan (2013) adapted observers to two oppositely moving (leftward and rightward) grating patches placed above and below a fixation point. Test stimuli were then briefly flashed in the position of the adaptors, and observers had to decide whether the top or bottom test appeared to move more quickly. Four test conditions were randomly interleaved: (a) both tests moved leftward, (b) both tests moved rightward, (c) the top test moved left and the bottom moved right, (d) the top test moved right and the bottom moved left. If an adapting stimulus reduces perceived velocity in a test moving in the same direction at the same location, we expect conditions a and b to produce response biases in opposite directions and conditions c and d to produce no response biases. These expectations were fulfilled, and the combined results of all four staircases could be modeled with two parameters: one representing the level of internal noise and the other a bias toward reduction in velocity caused by adaptation. The point is that the bias parameter is not the same as a response bias due to the interleaving of conditions. To obtain the same pattern of results as that produced by adaptation, the observer would have to guess when unsure in different directions, depending on the test condition.

Here, this methodology is extended to the case of the motion-induced tilt aftereffect. Motion within a stationary aperture causes an apparent shift in position of the aperture opposite to the direction of motion (De Valois & De Valois, 1991; Hayes, 2000; Ramachandran & Anstis, 1990). Thus, two vertically aligned Gabor patches with stationary envelopes and carriers moving in opposite directions appear to have a Vernier misalignment. Adaptation to such a stimulus causes a tilt aftereffect in the opposite direction. The purpose of the experiments reported here was to determine whether this kind of adaptation is retinotopic or spatiotopic and to confirm a previous report that the strength of adaptation is independent of the relative orientation of adapting and test carrier gratings (McGraw et al., 2002).

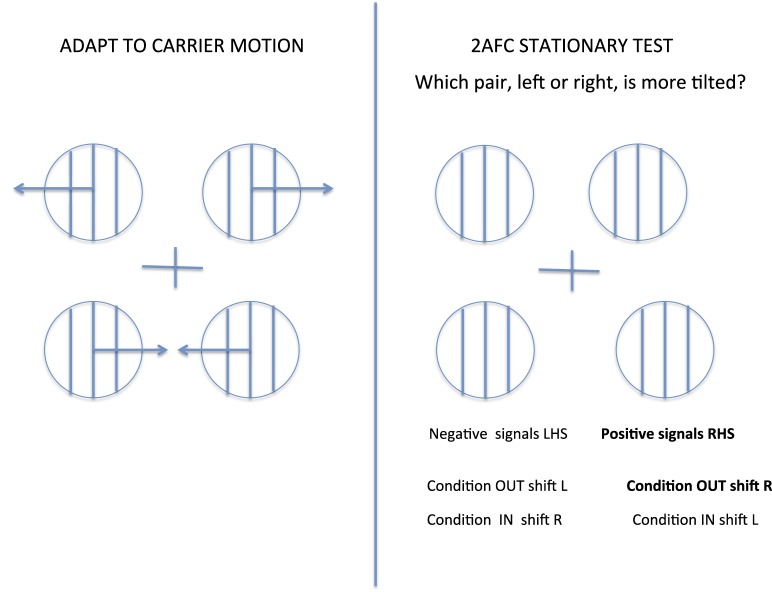
The adapting stimulus consisted of a 2 × 2 square array of drifting Gabor patches with a central fixation point (see Figure 1). The top pair of patches moved outward, and the bottom pair moved inward. The test consisted of a 2 × 2 array of stationary Gabor patches in the same position as the adapting array. The actually square adapting array appears trapezoidal because of the moving carrier gratings. A subsequently viewed square test array will appear trapezoidal in the opposite direction. The normal approach to measuring this apparent distortion would be to determine the physical shape that appears square by the MSS. We reject this approach because it fails to distinguish a genuine perceptual bias from a response bias. Instead, we move the position of one of the lower patches and ask the subject to report which of the two patch pairs, left or right, appears more tilted from the vertical. The displacement is added to the left or right lower patch randomly on different trials, making this a 2AFC procedure. In the absence of a sensory bias due to adaptation, the psychometric function for reporting “right more tilted” would increase from 0% for large displacements added to the left-hand side up to 100% for large displacements added to the right-hand side, going through a 50% point for a zero shift.

Figure 1.

Schematic representation of the stimuli used for adaptation and test. The subject adapts (left) to four Gabor patches with their carriers moving in the directions shown by the arrows. They are then tested with four patches in approximately the same position as the adapting patches (right). In each trial, a signed spatial displacement is selected. This displacement shifts one of the two bottom patches horizontally. Negative stimuli shift the left-hand patch, and positive stimuli shift the right-hand patch. The direction of the shift (leftward or rightward) depends on the condition. In condition “Out,” the patch is shifted outward in the same direction expected from the aftereffect. In condition “In,” the patch is shifted inward, opposite to the expected direction of adaptation. The case shown is a positive signal in condition “Out” (bold type). Conditions “Out” and “In” were randomly interleaved.

Now consider the effect of adaptation. This will apparently move both lower patches outward relative to the upper patches. There is no effect on the 50% point of the psychometric function because, with zero displacement, the two pairs of patches will appear equally misaligned from the vertical. However, if one of the two lower patches is moved inward by a small amount, it will appear more aligned than the patch on the other side, not less. As the displacement increases, the test patch will appear perfectly aligned when the displacement is equal and opposite to the illusory bias. Larger displacement will cause it to appear increasingly misaligned until it is indistinguishable from the reference patch. There will thus be two 50% points in the psychometric function: one when the displacement is zero and the other when it is twice the illusory bias. A response bias would not produce this effect.

Next, consider the effect of moving the test patch outward. This will always make it appear less aligned than the reference patch. The psychometric function will be monotone with a single 50% point when the displacement is zero. Outward and inward displacements were run in separate, randomly interleaved conditions, each controlled by its own independent adaptive probit estimation (APE) procedure.

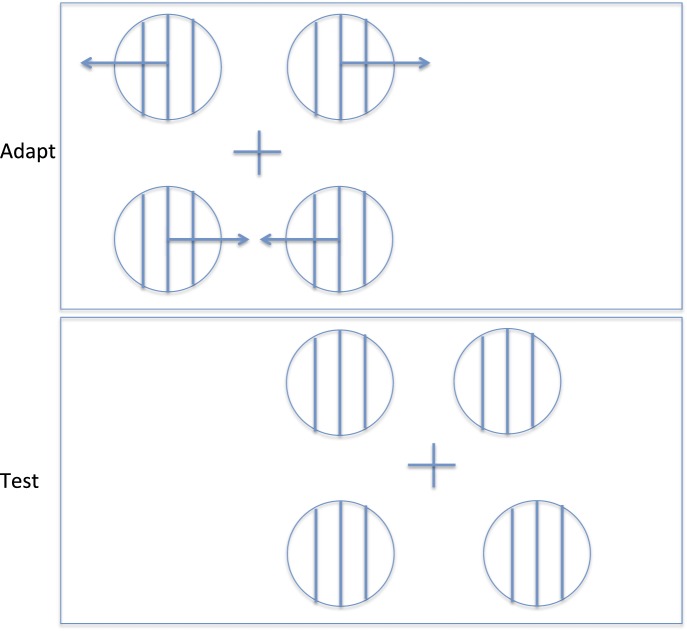
In the spatiotopic version of the experiment, the adapting and test stimuli occupied the same position of the screen, and the subjects maintained their gaze on the fixation cross. Eye position was monitored by an EYELINK 1000 infrared reflection system. In the retinotopic condition (Figure 2), the fixation cross moved rightward between the adapting period and the test, and the subject attempted to refixate it as quickly as possible. The test was presented as soon as the eye position recorder detected the movement to the new fixation cross. The new test stimulus had the same spatial relationship to the fixation cross as before, such that the left-hand test pair fell on the same spatial location as the right-hand adapting pair. If adaptation occurs in a spatiotopic rather than a retinotopic reference frame, this would be expected to reverse the relation between the “Out” and “In” conditions. In other words, the condition in which the stimulus was in the opposite direction to the aftereffect in the spatiotopic condition would be in the same direction to the aftereffect in the retinotopic condition.

Figure 2.

Stimulus configuration used in the retinotopic version of the experiment. The rectangles represent the outline of the display screen, not to scale. The test stimulus jumps horizontally as soon as the adapting period ends. The vertical position of the patches is unchanged. For further explanation see the text.

General methods

Apparatus

Stimuli were presented on a Sony Trinitron monitor with resolution 1400 × 1050 pixels viewed at 75 cm so that 1 pixel subtended 1.3 arcmin. The background screen luminance was 25 cd/m². The monitor frame rate was 60 Hz. Stimulus presentation was controlled by MATLAB and the PTB3 version of the Psychtoolbox (Brainard, 1997). Stimuli were viewed binocularly through natural pupils with appropriate corrective lenses for each subject if normally worn. Testing took place in a dimly lit room.

Subjects

The subjects were the author (MM) and a psychophysically experienced colleague at City University (DM), who was unaware of the purpose of the experiment or the detailed methods.

Stimuli

The adapting stimuli consisted of vertical sinusoidal gratings (spatial frequency 2.4 c/°) windowed with a stationary Gaussian envelope (standard deviation 0.625°). The test gratings were either horizontal or vertical in different sessions. In the control condition, the gratings were stationary; in the adapt condition, they were drifting at a rate of 45° of phase angle (π) every frame—equivalent to a temporal frequency of 7.48 Hz. Mean luminance of the grating was 25 cd/m², and the Michelson contrasts of adaptor and test were 52%. A large, white fixation cross was present in the center of the screen (0, 0) with the four stimuli placed symmetrically at distances of ±3.125° from the fixation cross (see Figure 1).

Procedure

Each trial began with a presentation of the adaptor with the fixation cross. Subjects attempted to maintain gaze on the center of the fixation cross at all times. After 4 s of adaptation, the screen went briefly (<100 ms) blank gray, and then the test configuration was presented for 0.5 s. The screen then went blank gray until the subject pressed either the left or right arrow key on the keyboard to indicate whether the left- or right-hand pair of patches was tilted more from the vertical. As soon as the response was made, the next adapting period began.

Psychophysics

In each trial, a horizontal shift was applied to one or the other of the two lower patches in the test display. For a description of how this was done for the two independent “Out” and “In” conditions, see the legend to Figure 1. Each condition was controlled by an APE procedure (Watt & Andrews, 1981), which estimated the standard deviation of the psychometric function (σ) and selected testing levels of the cue at ±σ around the mean of zero. Note that the adaptive procedure did not attempt to track the mean (μ) of the function. The use of the standard APE procedure was not ideal because, in some conditions, the expected psychometric function was not monotonic. For this reason, the APE procedure was slightly varied by adding a jitter randomly chosen from a list (−0.76°, 0°, 0.76°) to the cue value in order to sample the whole psychometric function more broadly than is done by the normal APE procedure. Even if the resulting procedure was less than ideal, this affected only the efficiency of the data collection, not the subsequent analysis of the data, which did not assume a monotone function. In practice, the data collected were well placed for estimating the parameters of the psychometric function, so the use of APE does not seem to have been a problem (see the functions in Figure 3). Each testing session comprised 128 trials (64 for each condition). At least three sessions were run for each condition, and the different conditions were randomly interleaved. Using the symbols H and V to denote the orientation of the test grating and R and S to denote retinotopic and spatiotopic conditions, respectively, the conditions were RV, RH, SV, and SH. In addition, subject MM experienced conditions RHm and RHs in which the test stimulus had an envelope with space constants (σ) one half (0.5) and one quarter (0.25) of the adaptor, respectively (Experiment 2).

Figure 3.

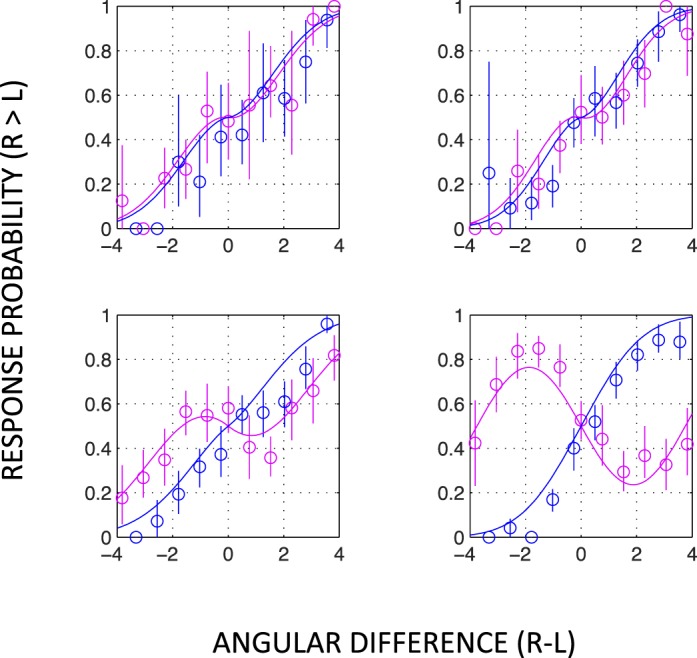
Psychometric functions for two observers (MM, left; DM, right) in the control condition in which the adapting rating was stationary (top) and in the combined moving-adaptor conditions for conditions RV, RH, SV, and SH in Experiment 1 (bottom). The y-axis shows the probability of choosing the right-hand stimulus pair as being more tilted; the x-axis shows the actual displacement (in degree of tilt) with positive values presented to the right-hand pair of patches and negative values to the right. In the outward condition (blue), the absolute stimulus level was in the same direction as the bias expected from adaptation; in the inward case (magenta), it was in the opposite direction. The continuous lines are a two-parameter (μ, σ) fit to the combined data in the top and bottom panels. For further details see the text.

Eye movement recording

Eye position was monitored with an EYELINK 1000 system. Each testing session began with a calibration procedure. In the retinotopic condition, the fixation cross moved as soon as the adapting stimulus had finished. As soon as gaze moved into a small area around the new fixation cross, the test stimulus was presented.

Results

Experiment 1

Psychometric functions are shown in Figure 3. It is important to be clear that these functions plot the probability of a “right-hand side” response against the difference (right-left) between the displacement on the two sides. Thus, negative values represent cases in which the displacement was added to the left-hand side and positive values when it was added to the right-hand side. The direction of displacement (outward or inward) is not influenced by the sign and is different between the two conditions (blue and magenta).

The psychometric functions were fit with cumulative Gaussian distributions with parameters σ and μ represented the bias arising from adaptation. The observer is assumed to monitor vertically oriented filters in the two positions and to choose the position having the maximum absolute deviation from the vertical. The model is described fully in the appendix to Raphael, Dillenburger, and Morgan (2013). The internal noise σ is assumed to be the same in the two filters. The data from the two conditions were fit together with identical parameter values by the MATLAB “fminsearch” procedure, minimizing negative log likelihood.

Best-fitting values of σ for the control condition (stationary adaptor) were 1.36° (MM) and 1.2° (DM) similar to previous hyperacuity thresholds for orientation in the literature (Morgan, 1990; Westheimer, 1981). Values of μ were not significantly different from zero. In all other cases (SV, SH, RV, RH), the values of μ were in the direction expected from adaptation. To see if values of μ were significantly greater than zero, a chi-squared test based on likelihood ratios was used. The log likelihood of fits to the combined psychometric functions were calculated using either a two-parameter fit (μ, σ) or a one-parameter fit (sigma with μ assumed = 0). Twice the difference in log likelihoods between the two fits was assumed to be distributed as chi-squared with 1° of freedom (e.g., Hoel, Port, & Stone, 1971). The rightmost column of Table 1 shows chi-squared values for the comparisons between two-parameter and one-parameter (zero-mean) fits. The two-parameter fits are significantly better in all conditions except the control. The data are consistent with the predicted shape of the psychometric function, which shows a small “flat” spot in the center due to the 2AFC design (Solomon, Lavie, & Morgan, 1997) as opposed to the reported functions for MSS, which have their maximum slope in the center.

Table 1.

Best-fitting parameter values (σ, μ) and negative log likelihoods of fits (Λ) in Experiment 1 for spatiotopic vertical test (SV), spatiotopic horizontal test (SH), retinotopic vertical test (RV), and retinotopic horizontal test (RH) conditions.

	σ	μ	Λ	Λ zero μ	χ2
MM
SV	1.58	0.76	284.48	293.12	17.28
SH	1.53	0.71	277.42	285.76	16.67
RV	1.76	1.22	140.34	147.97	15.26
RH	1.40	0.93	120.57	128.19	15.23
Control	1.36	0.11	207.49	207.69	0.41
DM
SV	1.29	2.10	269.26	336.33	134.13
SH	1.22	1.93	496.48	629.11	265.27
RV	1.07	1.78	120.99	161.00	80.03
RH	1.14	1.39	114.05	137.22	46.35
Control	1.20	0.16	418.57	419.76	2.40

In all the moving-adaptor conditions, values of μ were higher than in the control condition, and in every condition, the difference from the control was significant (Table 1). There was no significant differences in σ.

Pairwise comparisons between the four moving-adaptor conditions (RV, RH, SV, and SH) were carried out by comparing the likelihood of four- versus two-parameter fits. (That is, different values of μ, σ for the two cases vs. the same values). These (Table 2) showed no significant (p < 0.05) differences for MM and only two out of a possible six for DM, arising because μ for condition HS was smaller than in the other three conditions. All four pairwise comparisons with the control were significant. Therefore, for an overall summary of the data, all four noncontrol conditions are combined in Figure 3 (bottom two panels). It will be seen that both the observed and the theoretical psychometric functions for the two conditions are very different. When the stimulus is reinforced by the bias (blue), the flattening of the function in the middle is reduced. When the stimulus is countered by the bias (magenta), the slope of the function is reversed in the center. In other words, the subjects classified small stimuli in the wrong direction because the test with the stimulus (tilt = |bias-stimulus|) would seem less tilted than the test without the stimulus (tilt = |bias|). Only when the stimulus is more than two times the bias is the direction of the function reversed. It should be mentioned that the fits in Figure 3 are not polynomial fits to the data, but the fits of the two-parameter model. No attempt has been made to account for the left-right bias evident in DM's data by the addition of a third parameter, although this could easily be done, at the cost of simplicity.

Table 2.

Chi-squared values for paired comparisons between spatiotopic vertical test (SV), spatiotopic horizontal test (SH), retinotopic vertical test (RV), and retinotopic horizontal test (RH) and the control (stationary adaptor).

	SH	RV	RH	Control
MM
SV	0.15	3.81	0.95	10.56
SH	0.00	5.31	0.89	9.07
RV	0.00	0.00	3.97	20.10
RH	0.00	0.00	0.00	10.12
DM
SV	1.33	3.21	13.51	133.91
SH	0.00	1.44	10.66	163.38
RV	0.00	0.00	3.85	77.79
RH	0.00	0.00	0.00	41.65

Figure 4 shows the data from the retinotopic conditions (combining RH and RV) with predictions of the spatiotopic model, which asserts that the effect of an adapting stimulus on a test depends on the relative screen positions of the two stimuli—not on their retinal positions. Thus, the value of μ for the left-hand stimulus should be reversed from that found in the spatiotopic condition, and that for the right-hand test should be zero. It is clear from Figure 4 that this is a poor prediction. The data are much better fit, as in Figure 3, with the model asserting that the parameter values for the left and right tests are μ and –μ.

Figure 4.

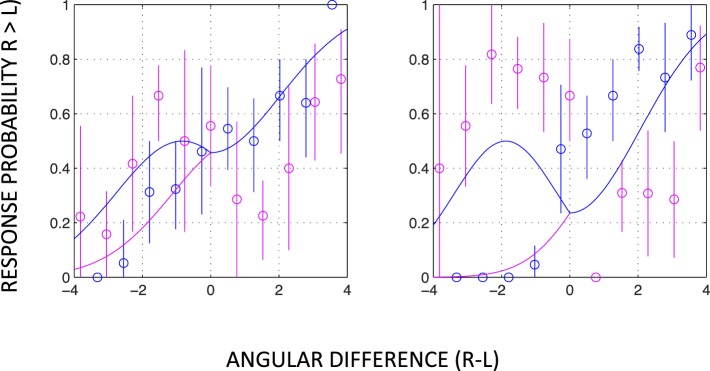
Data from the retinotopic condition and the predictions of the model asserting that adaption takes place in a spatiotopic reference frame. The coloring of the curves representing the two independent conditions is the same as in Figure 3. Left-hand panel: subject MM. Right-hand panel: subject DM. Note that the curves are not fits to the data, but predictions based on the assumption that adaptation is purely spatiotopic, using the parameter values μ, σ from the spatiotopic condition.

Table 3.

Best-fitting parameter values and negative log likelihoods (Λ) from conditions SV (spatiotopic vertical test) and SH in Experiment 1 and conditions SHs (spatiotopic, small vertical test) and SHs (spatiotopic, medium vertical test) in Experiment 2.

	σ	μ	Λ
MM
SV	1.58	0.76	284.48
SH	1.53	0.71	277.42
SHs	1.45	1.42	262.77
SHm	1.26	1.06	230.35
Control	1.36	0.11	207.49
All	1.48	1.00	1065.24

Table 4.

Pairwise chi-squared comparisons between the conditions in Table 3. Notes: Significant (p < 0.05) values are highlighted.

	SHl	SHs	SHm	Control
SV	0.15	10.04	4.98	10.56
SH	<0.1	12.26	4.75	9.07
RHs	<0.1	<0.1	7.43	36.89
RHm	<0.1	<0.1	<0.1	19.24

Experiment 2

In addition, subject MM experienced conditions HRm and HRs in which the test stimulus had an envelope with space constants (σ) one half (0.5) and one quarter (0.25) of the adaptor, respectively. The extent of adaptation measured by the values for μ were all higher with the smaller tests than with the tests used in Experiment 1, which had the same size as the adapting stimuli. The difference between the largest test (in Experiment 1) and the smallest test was significant; that between the largest size test and the medium was marginally significant (p < 0.1), and the difference between the smallest and the medium was significant (p < 0.01). There were no obvious effects on σ. An interesting observation, which may have some bearing on the effect of test size, is that the small and medium tests, unlike the largest, were seen to move in the test exposure in the direction that would be expected from a motion aftereffect. If so, the motion aftereffect is second order because the envelope seemed to move, not the horizontal carrier.

Discussion

This paper establishes the feasibility of measuring the perceptual effect of adaptation with a relatively bias-free 2AFC procedure as an alternative to the MSS. In this new procedure, the subject could not mimic the effects of perceptual adaptation by consciously or unconsciously selecting one of the two response alternatives when uncertain or by selecting clockwise rather than anticlockwise tilts. To produce the observed pattern of results, they would have to use different reference tilts for the vertical on the left- and right-hand sides of the pattern. This would be indeed difficult to distinguish logically from a perceptual shift.

The mean size of the aftereffect over all moving-adaptor conditions in Experiment 1 was 0.91° of tilt (subject MM) and 1.88° (DM). McGraw et al. (2002), using the MSS, reported shifts in the region of 2°. This similarity suggests that the MSS can be a reliable method of parametric measurement in the right circumstances. However, previous conflicting results between experiments using MSS suggest that this may not always be the case. The retinotopy versus spatiotopy of the tilt aftereffect is controversial (Knapen et al., 2009; Turi & Burr, 2012; Zimmermann et al., 2013) as are the effects of attentional load on motion adaptation (Morgan, 2011; Taya et al., 2009).

We suggest that, in these cases, the conflict should be resolved by the use of less-biased procedures than MSS. In the present experiment, we found evidence against spatiotopy. A spatiotopic aftereffect would have produced a reversed pattern of results in the retinotopic condition to the one we observed (Figure 4). This finding does not bear directly on the controversy regarding spatiotopy of the static tilt aftereffect because previous experiments have used single grating patches and tilts of the carrier rather than shifts of the envelope. Also, we pitted the retinotopic and putative spatiotopic effects against one another, which may not be the most sensitive way of revealing the latter. However, the 2AFC method could be used in the tilted-carrier case with the same stimulus arrangement as in the present experiment but asking the observer to decide which pair of gratings (left or right) are more different in their orientation.