Role of intermediate phase for stable cycling of Na₇V₄(P₂O₇)₄PO₄ in sodium ion battery

Significance

Utilizing low-cost materials, sodium ion batteries (SIBs) are beginning to attract considerable attention, particularly for large-scale utility grid applications. However, electrochemical performance of most SIB active materials is still insufficient for various practical applications. In the current study, we discovered a vanadium-based ortho-diphosphate, Na₇V₄(P₂O₇)₄PO₄, or VODP, that holds exceptional electrochemical properties represented by well-defined high voltage profiles at 3.88 V (vs. Na/Na⁺) and substantial capacity retention over 1,000 cycles. A theoretical analysis suggests that an intermediate phase encountered during phase transformation of VODP is crucial for better kinetics during battery operations, which can be expanded as a general principle in understanding diverse battery materials.

Abstract

Sodium ion batteries offer promising opportunities in emerging utility grid applications because of the low cost of raw materials, yet low energy density and limited cycle life remain critical drawbacks in their electrochemical operations. Herein, we report a vanadium-based ortho-diphosphate, Na₇V₄(P₂O₇)₄PO₄, or VODP, that significantly reduces all these drawbacks. Indeed, VODP exhibits single-valued voltage plateaus at 3.88 V vs. Na/Na⁺ while retaining substantial capacity (>78%) over 1,000 cycles. Electronic structure calculations reveal that the remarkable single plateau and cycle life originate from an intermediate phase (a very shallow voltage step) that is similar both in the energy level and lattice parameters to those of fully intercalated and deintercalated states. We propose a theoretical scheme in which the reaction barrier that arises from lattice mismatches can be evaluated by using a simple energetic consideration, suggesting that the presence of intermediate phases is beneficial for cell kinetics by buffering the differences in lattice parameters between initial and final phases. We expect these insights into the role of intermediate phases found for VODP hold in general and thus provide a helpful guideline in the further understanding and design of battery materials.

Sodium ion batteries (SIBs) provide advantages of unlimited resource, low material cost, and easy worldwide accessibility that could make them the material of choice for grid-scale energy storage systems (1, 2). Unfortunately, the electrochemical performance of current SIBs remains inferior to that of lithium ion batteries (LIBs). In particular, we require SIB cathode materials that give a long cycle life with large capacities and high voltages under fast operating conditions, comparable to the Li counterparts.

Numerous layered Na_xMO₂ [M = Co, Mn, Fe, Ni, Cr, and multicomponent transition metals (TMs)] have been intensively studied as candidate SIB cathodes because such layer-structured materials typically exhibit higher capacities than other classes of materials (3–10). However, they suffer from a substantial capacity decay with cycling, owing to crystal structure collapse and/or unstable electrode–electrolyte interfaces (10). For more stable host frameworks, various polyanion structures have also been studied as SIB cathodes as a natural extension of the success shown in the LIB materials of the same classes, including TM fluorophosphates (11–15), pyrophosphates (16–18) and phosphates (19–22). However, most of these materials still exhibited insufficient cycle lifetimes and large voltage steps that limit their practical capacity. The Prussian blue family has also been extensively investigated for both organic (23, 24) and aqueous electrolytes (25) owing to its unique advantages related to low material cost, low temperature synthesis, and decent electrochemical performance from well-developed ionic channel structures. However, the as-synthesized Prussian blue family is usually in the potassium form and thus requires ion exchange with Na ions.

Herein, we report a vanadium-based ortho-diphosphate, Na₇V₄(P₂O₇)₄PO₄, or VODP, that addresses these limitations of the present SIB cathodes. By engaging the V³⁺/V⁴⁺ redox couple, VODP exhibits single well-defined voltage plateaus at 3.88 V vs. Na/Na⁺, which is optimal for stable operations within a usable electrolyte window. Combined with the robust host frameworks, VODP exhibits unprecedented cycle life, maintaining substantial capacity retention over 1,000 cycles. In particular, we find that the observed single plateau and long cycle life is due to the existence of a very shallow intermediate phase along the battery reaction, a concept that can be used to understand existing electrochemical properties or for future developments. To this end, we also propose a simple theoretical method to estimate the reaction barrier for two-phase reactions in general.

Results and Discussion

Synthesis and Crystal Structure of Na₇V₄(P₂O₇)₄PO₄.

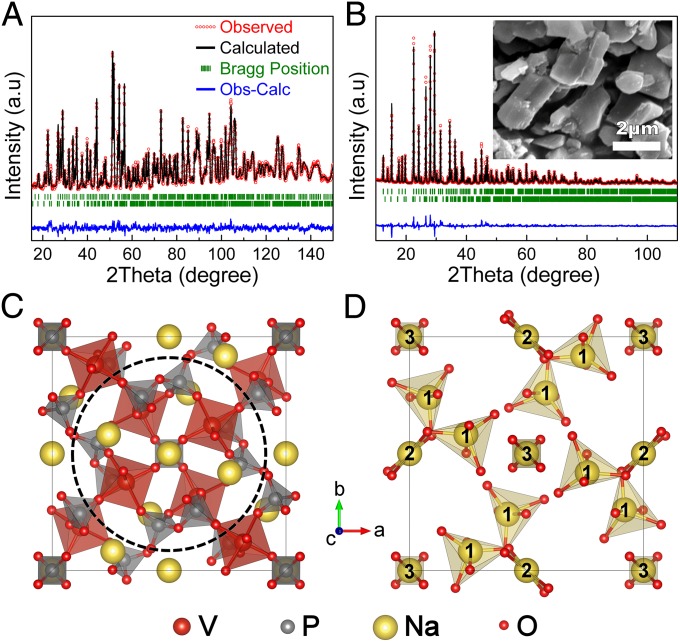
Na₇V₄(P₂O₇)₄PO₄, or VODP, was synthesized by simple solid state reactions. For this, stoichiometric amounts of precursors, sodium carbonate (Na₂CO₃), vanadium pentoxide (V₂O₅), and ammonium phosphate (NH₄H₂PO₄), were first mixed thoroughly and then reacted at 800 °C under argon atmosphere. Detailed synthetic procedures are described in Materials and Methods. The crystal structure was resolved by analyzing the complementary X-ray diffraction (XRD) and neutron diffraction (ND) patterns (Fig. 1 A and B) together. VODP exhibits the tetragonal P-42₁c space group with lattice parameters a = 14.225(3) Å and c = 6.364 (17) Å (Tables S1 and S2). Although the diffraction patterns include peaks from a small amount (<4%) of Na₃V₂(PO₄) as an impurity (26, 27), these impurity peaks are well separated from those of the VODP phase, at least in the low and medium angle regions. An SEM image shown in Fig. 1B, Inset indicates that the solid state reaction produced particles with dimensions in the range of 1–3 μm.

Fig. 1.

(A) Neutron and (B) X-ray diffraction patterns of Na₇V₄(P₂O₇)₄PO₄ and their Rietveld refinement profiles: observed (red open circles), calculated (black line), and difference (blue line). Bragg positions for both Na₇V₄(P₂O₇)₄PO₄ (upper green) and Na₃V₂(PO₄)₃ (minor phase, lower green) are also presented. The inset in B shows an SEM image of as-synthesized sample. (C) A projected crystal structure along the c axis. Gray tetrahedrons, PO₄; red octahedrons, VO₆; yellow spheres, Na atoms. The (VP₂O₇)₄PO₄ unit is denoted as a dashed circle. (D) Schematic illustration of the various Na sites (Na1∼Na3) projected along the same c-axis.

As shown in Fig. 1C, the structure of VODP can be described by the repetition of a basic unit, (VP₂O₇)₄PO₄, using fourfold symmetry (dotted line in Fig. 1C). In the (VP₂O₇)₄PO₄ unit, a central tetrahedron [PO₄] shares corners with four [VO₆] octahedra, and each diphosphate group [P₂O₇] bridges the two adjacent [VO₆] octahedra by sharing the corners. The interconnected (VP₂O₇)₄PO₄ units form a 3D framework with well-defined ionic channels for Na (de)insertion. Na ions (Fig. 1D) occupy three different crystallographic positions, Na1, Na2, and Na3, adopting bipyramidal, square planar, and tetrahedral coordinations, respectively. A transmission electron microscopy image and its fast Fourier transformation pattern confirm the particle dimensions and the given crystal structure (Fig. S1). Although the isostructures, Na₇M₄(P₂O₇)₄PO₄ (M = Al, Cr, and Fe), have been investigated (28), the vanadium version of this framework has been neither reported as a battery electrode material nor even documented in the materials database.

Electrochemical Properties of Na₇V₄(P₂O₇)₄PO₄.

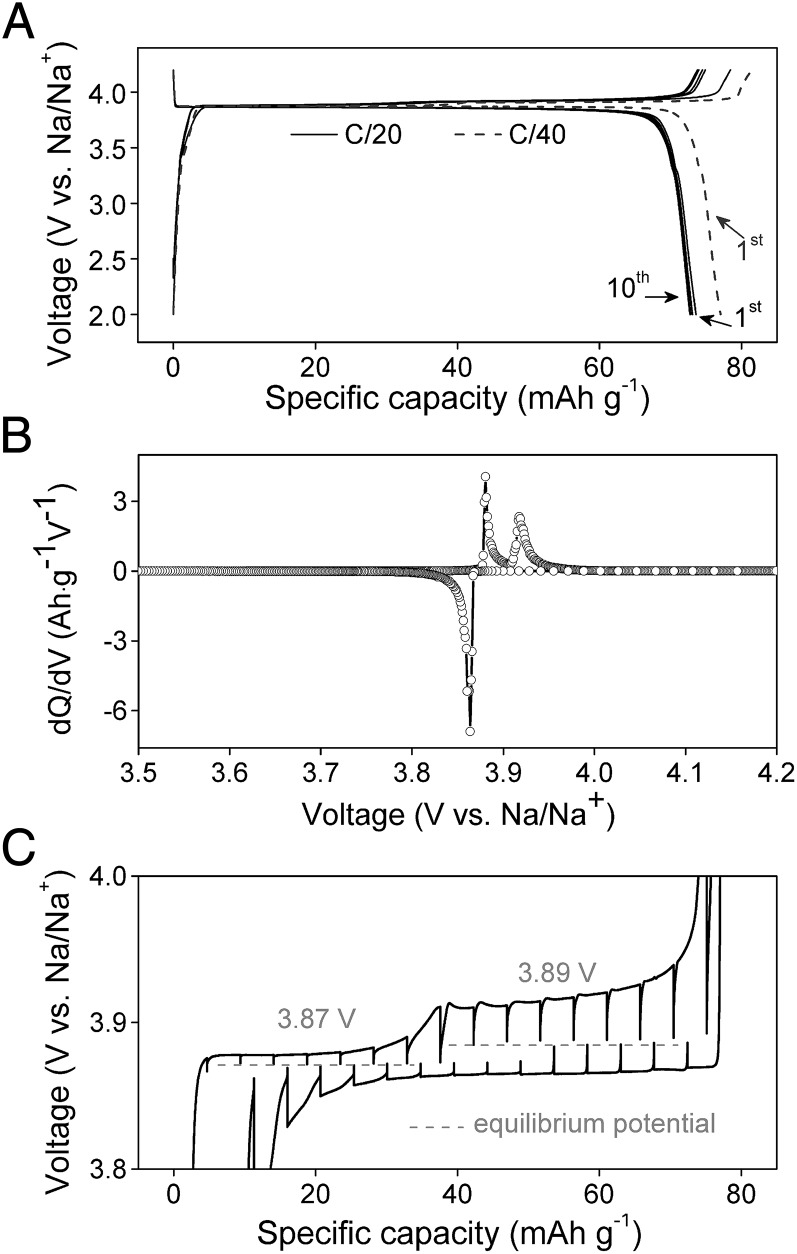
To evaluate VODP as an SIB cathode, coin-type half cells were prepared in which sodium thin disks were used as both the reference and counter electrodes. The galvanostatic profiles measured at C/40 and C/20 rates (Fig. 2A) exhibit single well-defined plateaus at 3.88 V throughout both charging and discharging processes. The potential of 3.88 V is quite noticeable considering that this material does not contain fluorine to increase the inductive effect (29), and the cell voltages of SIBs are generally lower than those of LIBs by 0.2–0.6 V (30).

Fig. 2.

(A) Galvanostatic profiles of Na₇V₄(P₂O₇)₄PO₄ measured at C/20 and C/40 in the first cycles. The profiles at C/20 in the cycle range of 2∼10 are also presented. (B) The differential capacity curve (dQ/dV) obtained from the galvanostatic profile. (C) A QOCP profile measured at C/20.

The galvanostatic data (Fig. 2A) measured at C/40 indicate that capacities are 81.5 and 77.1 mAh⋅g⁻¹ for charge and discharge, respectively, which correspond to 3.5 and 3.3 Na ions per each formula unit of Na₇V₄(P₂O₇)₄PO₄. At C/20, the charge and discharge capacities are 78.5 and 73.6 mAh⋅g⁻¹, respectively. These values imply that four Na ions per each formula unit are theoretically available (92.8 mAh⋅g⁻¹) in this material within the given potential window (2.0–4.2 V vs. Na/Na⁺).

The differential capacity (dQ/dV) data (Fig. 2B) exhibit a discrepancy between charging and discharging peaks. When measured at C/20, the charging profile shows two separate peaks at 3.88 and 3.92 V, whereas the discharging profile shows only a single peak at 3.86 V. Such distinctive behavior between the charge and discharge is similar to the case of NaFePO₄ (19, 20), where the origin is speculated to be the different reaction pathways during charge and discharge (19). However, in VODP the distinctive behavior is likely to be attributed to different kinetics, rather than different reaction pathways, from the quasi-open circuit potential (QOCP) result (Fig. 2C). Whereas the QOCP profile confirms the equilibrium potentials at 3.87 V and 3.89 V, the profile indicates two separate regions, the first half with small polarizations (7 mV) and the other half with relatively larger polarizations (30 mV and 20 mV for charge and discharge, respectively). From this, it is anticipated that the split peaks during the charge in the dQ/dV data are related to these two separate regions with their distinctive kinetics. However, the two peaks in the dQ/dV data become merged during the discharge scanned in the reverse direction owing to the larger polarizations of the second half region. The split peaks in the dQ/dV data also indicate the existence of one stable intermediate phase around x = 5 in Na_xV₄(P₂O₇)₄PO₄. It is anticipated that the large polarizations observed at the end of charge and discharge originate from the smaller electronic conductivities of the fully charged and discharged phases where all vanadium atoms are oxidized to V⁴⁺ and reduced to V³⁺, respectively. As evidence of this scenario, we find that the large polarizations disappeared upon integration with reduced graphene oxide (rGO), which decreases electrical impedance on the electrode film scale by reducing electronic transport length (Fig. S2A).

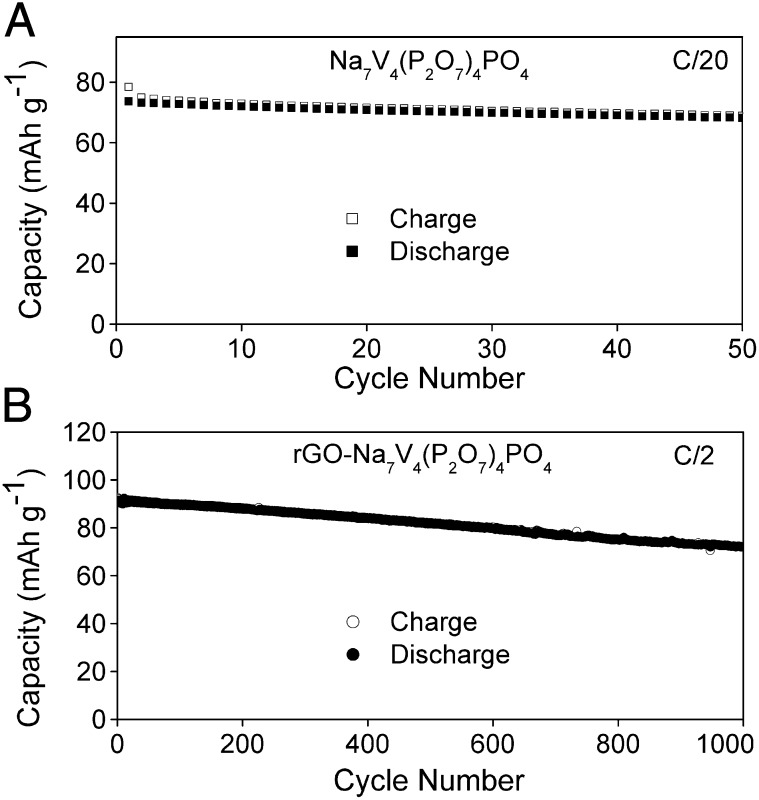
The bare VODP without carbon coating exhibits stable cycling (Fig. 3A), such as 92.6% retention after 50 cycles, using the intrinsic characteristics of this material such as the corner sharing of polyhedrons that accommodates structural changes and the spectator portions (Na₃ and PO₄) that do not participate in the electrochemical reaction but contribute to stability of the framework. Furthermore, we integrated VODP with rGO (Fig. S2) because rGO integration has been known to diminish the resistances between particles and improve electrochemical performance (31–34). Upon rGO integration, VODP retains 89.7% and 78.3% of the initial capacity (91.0 mAh⋅g⁻¹) even after 500 and 1,000 cycles (Fig. 3B), respectively. The small volume change (2.4%) for VODP may play a role in this robust electrochemical operation. The rate and cycling performances of the rGO-integrated and bare samples measured under different conditions are presented in Fig. S3. It should be noted that the bare VODP without rGO integration shows reasonably good performance even in the micrometer dimension (Fig. S3E): 78.9% capacity retention after 100 cycles when measured at C/2 and 75.9% capacity retention (78.4 → 59.5 mAh⋅g⁻¹) upon the C-rate increase from C/40 to C/2.

Fig. 3.

The capacity retentions of (A) bare and (B) rGO integrated samples. In both A and B, the rates for charge and discharge are the same in each cycle. In B, the specific capacities were calculated based on the mass of Na₇V₄(P₂O₇)₄PO₄ only because the exclusive contribution from rGO is difficult to be isolated. From a control experiment based on rGO only, we found that rGO has its own capacity of ∼38 mAh⋅g⁻¹ in the given potential range.

Intermediate Phase and Single-Valued Plateaus.

To obtain deeper insights into the charging/discharging processes, we performed ex situ XRD measurements (Fig. 4A) and density functional theory (DFT) calculations.

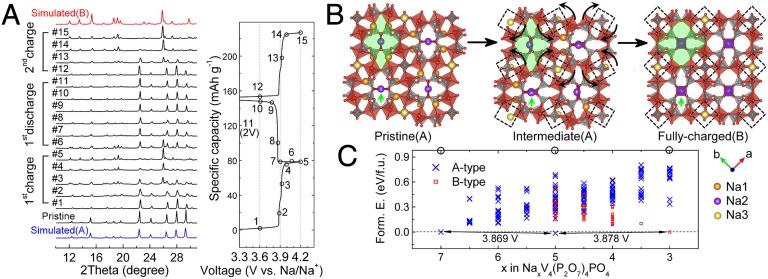
Fig. 4.

(A) Ex situ XRD spectra obtained at the points denoted in the galvanostatic curve on the right-hand side. The XRD spectra of the A-type and B-type phases simulated from the DFT calculations are also presented (blue and red lines). (B) The structure rearrangement during phase transformation between pristine and charged phases. Crystallographically different sodium sites are colored with different colors (Na1, orange; Na2, violet; Na3, yellow). (C) Formation energies of the A- and B-type phases and the estimated voltages obtained from calculations.

In the first charge, consistent with the QOCP data, the ex situ XRD analyses show distinctive spectra for the first and second halves of the curve. On the first plateau (2 in Fig. 4A), namely, the two-phase region between the pristine and intermediate phases, the split peaks were observed at the 2θ range of 22–30°, indicating that the intermediate phase is structurally very close to the pristine phase. During the second half of the charge (3→5), the two-phase region between the intermediate and fully charged phases, new peaks gradually grow in corresponding to the fully charged phase, eventually reaching a new spectrum at the end of the charging (5). The smaller polarization in the first half compared with the second half (7 mV vs. 20–30 mV) may be associated with the smaller volume change (0.4% vs. 2.0% from Fig. S4). This point will be discussed below. The spectra during the discharge in the same cycle show largely reversed peak changes, indicative of reversible phase transformation of Na₇V₄(P₂O₇)₄PO₄ during Na insertion and deinsertion. The same peak changes were consistently observed for the second charge as well.

The calculated and experimental diffraction spectra for both pristine and fully charged phases are in excellent agreement as shown in Fig. 4A (blue and red lines). Fig. 4B illustrates the phase transformation of the crystal structure during charging. We denote the structurally similar pristine and intermediate phases as A-type and the fully-charged phase as B-type. When the structure transforms from the A-type to the B-type phases, each unit of (VP₂O₇)₄PO₄ (dashed squares in Fig. 4B) rotates clockwise and counterclockwise symmetrically by 11.7°. As a result, in the B type, the repeated units of (VP₂O₇)₄PO₄ are aligned in a more orderly fashion with the neighboring units. One can compare the green shaded regions of both structures in Fig. 4B. The activation barrier for the rotation of the (VP₂O₇)₄PO₄ unit is calculated to be ∼60 meV (Fig. S5), implying that the latter structural change is energetically almost free and hence does not compromise the reversibility of this material upon cycles. This nearly barrierless rotation can be understood as follows. Because all of the four active Na ions are extracted from the Na1 sites (orange spheres in Fig. 4B), the original structural distortion that was needed to stabilize the Na1 ions in the bipyramidal units is now released in the charged state. During this relaxation, the overall crystal structure rearranges to stabilize the remaining Na2 sites (violet spheres in Fig. 4B) via the transformation from the square planar to the tetrahedral units (green arrows in Fig. 4B). Therefore, the relative stability of Na1 and Na2 sites and their occupation govern the phase transformation between A- and B-type phases as shown in Fig. 4C (red being more stable after x = 5).

The calculated convex hull connecting the minimum formation energies at different x values (Fig. 4C) confirms the experiments that the A-type intermediate phase indeed exists at x = 5, albeit extremely shallow, yielding almost single-valued plateaus. The formation of such an intermediate phase is attributed to the exceptional Na and V³⁺/V⁴⁺ orderings at x = 5 (Fig. S6), where relatively stronger electrostatic repulsions between Na⁺ and V⁴⁺ ions allow the close proximity of Na⁺ and V³⁺ ions.

The present material shows a desirable single-valued voltage plateau as shown in Fig. 2. Although all of the Na ions involved in the reaction are from the equivalent crystallographic sites (Na1) throughout the entire charging process, the single-valued plateaus are indeed peculiar because the current material experiences the phase transformations that would not any more leave all of the Na sites in the same environments. That is, structurally, the host material undergoes significant geometric rotations during the second half of the charge as discussed above, but energetically the material maintains the energy loss (gain) for the Na extraction (insertion) almost constant throughout the entire charge (discharge). In other words, the amount of stabilization for the charge ordering in the intermediate phase is similar to that for the framework rotation in the fully charged phase.

Presence of Intermediate Phases for Better Kinetics.

The “interfacial energy penalty” between adjacent phases is an important factor in two-phase reactions, especially when kinetics is limited by the secondary phase nucleation and growth that should be overcome for charging and discharging. It typically arises from atomic reorganizations such as rotation, elongation, breaking, and forming of bonds. Therefore, to incorporate the latter effects into the theoretical framework, the following issues need to be addressed: (i) the reorganization depends on a large number of degrees of freedom; (ii) although the initial and final states (or reactant and product) of the reaction are clearly known, the transition state (TS) that determines the kinetics is not, and thus consequently (iii) the reorganization energy associated with phase boundary migration is difficult to quantify.

To resolve the second and third problems, we can draw relative energy penalties compared with the equilibrium (bulk) state as a function of a “proper” predefined reaction coordinate (Fig. 5A). Here, the reactant and product are phases A and B, respectively, and the reaction is the phase transformation from A to B. We define the coordinate R_m that minimizes a sum of energy penalties to be the TS, and the total energy penalty at R_m, E(R_m) = E_A(R_m) + E_B(R_m), to be the activation energy of the reaction. We note that the solution R_m satisfies two conditions at TS: the same “lattice coordinates” and “forces” (slopes) with opposite sign for the two phases. For example, for a one-dimensional lattice model, the former and latter conditions would ensure the lattice match and force equilibrium, respectively, at TS.

Fig. 5.

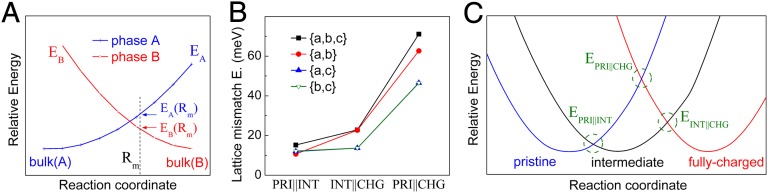
(A) Relative energies of phases A and B as a function of geometry changes. The minimum value of the total energy penalties, E_A(R_m) + E_B(R_m), is the activation energy. (B) The lattice mismatch energies between the pristine and intermediate phases (PRI||INT), the intermediate and fully charged phases (INT||CHG), and the pristine and fully charged phases (PRI||CHG). (C) The presence of intermediate phase reduces the lattice mismatch energy that should be overcome for the phase boundary migration.

The first problem, a large number of degrees of freedom, can be addressed by choosing a collective variable that can represent the reaction progress properly. One good example of a collective variable for reorganizations in solids might be the lattice parameters that have also been often used to explain kinetics of cathode materials in the battery field (35). Because the cathode materials have a coherent or semicoherent interface between charged and discharged phases (36), the lattice parameters evolve gradually between those of the two phases, implying that the reaction pathway can be well defined along the changing lattice parameters. These lattice parameters can also represent detailed atomic reorganizations reasonably in most cases because they are the results of atomic species and their bond structures.

We then define the energy penalty associates with the lattice parameter mismatch as the lattice mismatch energy (LME). Note that this LME does not contain detailed atomic interactions owing to the adjacent phase that do not cause any lattice changes. Fig. 5B shows the calculated LME of VODP between the pristine and intermediate phases (E_PRI||INT), the intermediate and fully charged phases (E_INT||CHG), and the pristine and fully charged phases (E_PRI||CHG), where the latter is a hypothetical case. For the reaction coordinate, sets of lattice parameters, {a,b}, {a,c}, {b,c}, and {a,b,c}, were used under the assumption that they evolve linearly from one phase to another phase. For example, the {a,b} indicates that the lattice constants a and b are altered gradually along the constrained reaction path between two phases, whereas the c parameter is freely relaxed. That is, {a,b} indicates the LME perpendicular to the ab plane (or the LME along the c direction). In the same manner, {a,c} and {b,c} are the LME along the b and a directions, respectively. The most constrained, {a,b,c}, can be considered as the averaged upper bound of the LME. With these definitions, the following points are noteworthy in Fig. 5B.

First, along all of the directions, the LME between the pristine and intermediate phases (E_PRI||INT = 11–12 meV) is lower than that between the intermediate and fully charged phases (E_INT||CHG = 14–23 meV). We expect that this smaller LME for the first plateau is the origin of the smaller polarization observed in Fig. 2C.

Second, the E_PRI||INT is almost identical in all of the directions; therefore, the (de)insertion process between pristine and intermediate phases is isotropic in terms of LME. However, the E_INT||CHG along the c direction (red line in Fig. 5B) is higher than that along the other directions by ∼10 meV, implying the (de)insertion process could have directionality in the second half of the charge as in the LiFePO₄. Note that the anisotropy of LME in LiFePO₄ is 12–35 meV with the lowest value along the a direction, consistent with experiments (37).

Third, more importantly, the LME for the hypothetical two-phase reaction between pristine and fully charged phases in the absence of an intermediate phase (E_PRI||CHG) is notably higher than the E_PRI||INT and E_INT||CHG, as well as the sum of them, suggesting that the presence of intermediate phases can enhance the cell kinetics by reducing LME. Fig. 5C shows this interpretation schematically in the relative energy vs. reaction coordinate picture. When the lattice parameters or volume is set as the reaction coordinate, the energy curves of each phase are generally quadratic as a function of reaction coordinate (38, 39). In such a case, the TSs of each biphasic reaction locate around the cross point (dashed circles in Fig. 5C) owing to the similar elastic moduli between two phases, implying that the smaller difference in reaction coordinate would yield the lower activation barrier for the reaction. In this manner, the presence of intermediate phases can improve the cell kinetics by buffering the differences in the lattice parameters.

As another example, the vanadium-based NASICON structure Na₃V₂(PO₄)₃ exhibits a single plateau without intermediate phases at low current rates; however, it shows a peak split at high current rates, as in the VODP (40). In the fast charge/discharge conditions, thermodynamically inaccessible intermediate phases in slow rates can be viable owing to the increased polarizations. In such cases, kinetics determines the reaction pathway whether through the intermediate phase or not. The split peak at high rate indicates that the reaction pathway through the intermediate phase is favorable in kinetics when both pathways are thermodynamically allowed. In other words, even for the unstable intermediate phases, if their energy level is shallow enough the presence of intermediate phases is beneficial for cell kinetics in fast rate conditions. Likewise, we expect that the small polarization and high rate capability of Na₄Co₃(PO₄)₂P₂O₇ are also attributed to the multiple intermediate phases with small lattice differences (41).

However, because the intermediate phases always involve corresponding voltage steps, the single-valued plateau observed in our material is rather unusual. Similarly, the single-phase reaction, which can be considered as an infinite sequence of two-phase reactions via countless “intermediate phases” that are infinitesimally different, provides better kinetics for the reasons described above but typically shows a sloping voltage behavior. In this point of view, VODP retains remarkably the advantages of both two-phase reaction (well-defined voltage) and a single-phase reaction (improved kinetics) owing to the intermediate phase with a small energy difference.

Conclusions

We have discovered an SIB cathode material, Na₇V₄(P₂O₇)₄PO₄, or VODP, that exhibits single well-defined plateaus at 3.88 V vs. Na/Na⁺ throughout the entire capacity range. A theoretical analysis reveals that the very shallow energy level of an intermediate phase owing to the iso-energetic structural rearrangements (charge ordering and framework rotation) is the origin of this single-valued voltage behavior. We suggested a simple way to quantify activation barriers for two-phase (de)insertion reactions, yielding an intuitive conclusion that the smaller changes in lattice parameters lead to the lower reaction barriers. By extension, as a rule of thumb, we show that the presence of intermediate phases can reduce reaction barriers by buffering the differences in lattice parameters between two end members, giving a better kinetics.

Although the presence of such stable intermediate phases is an intrinsic property of materials that is not easy to tune, using intermediate phases is a useful concept to improve kinetic properties of battery materials. This approach may be achievable, for example, by controlling the size of particles, which can alter the reaction free energy curves (42). Also, the well-behaved high voltages and impressive cycling and rate capability of VODP would be a significant step forward to make functional SIB systems in the near future.

Materials and Methods

Synthesis.

Na₇V₄(P₂O₇)₄PO₄ (VODP) was synthesized by conventional solid-state reaction. Stoichiometric amounts of Na₂CO₃ (≥99.5%; Aldrich), V₂O₅ (≥98%; Aldrich), and NH₄H₂PO₄ (≥99.9%; Aldrich) were mixed by ball-milling in the presence of acetone for 2 d, and acetone was then evaporated at 80 °C. Next, the mixture was dried in an oven at 70 °C overnight and was then pelletized. The pellets were heat-treated at 250 °C for 3 h under argon flow. The pellets were cooled down to room temperature and were ground once again in a mortar to ensure uniform mixing between the precursors. Finally, the mixture was reacted at 800 °C for 6 h under argon flow, completing the synthesis of VODP. rGO was obtained by first preparing graphene oxide (GO) suspension from commercial graphite (Alfa Aesar) based on a modified Hummer’s method. In this method, 1 g of graphite was oxidized in an acid solution where 0.5 g of sodium nitrate (NaNO₃; Aldrich) and 3.5 g of potassium permanganate (KMnO₄; Aldrich) were dissolved in 23 mL of sulphuric acid (Aldrich). Then, the graphite oxide suspension was sonicated for exfoliation. Finally, the 200-mL GO suspension (1 mg⋅mL⁻¹) was reduced by introducing 400 μL of hydrazine monohydrate (N₂H₄∙H₂O; Sigma Aldrich), and the solution was then refluxed at 98 °C for 5 h. The final suspension was filtered and washed with deionized water and ethanol several times to remove any impurities. For rGO Na₇V₄(P₂O₇)₄PO₄, VODP and rGO were mixed using low-energy ball milling for 12 h. Then, the mixture was heat-treated in a furnace at 200 °C for 1 h under argon atmosphere to facilitate electrically better contacts between both components.

Crystal Structure Characterization.

Powder X-ray diffraction measurements were carried out with a Rigaku DMAX-2200PC X-ray diffractometer equipped with a graphite monochromator (λ = 1.5418 Å). A step scan mode was used in the 2θ range of 10.10–110° with a step size of 0.02° and counting time of 5 s for each step. Neutron powder diffraction data were obtained with a high resolution powder diffractometer at Hanaro Center of Korea Atomic Energy Research Institute. A vanadium canister was used as a sample holder. The data were collected in the 2θ range of 0–159.95° with a step size of 0.05°. The refinements of XRD and ND data were carried out by the Rietveld method with the Fullprof program (43). For ex situ XRD characterization of the battery cycled electrodes, a multipurpose attachment X-ray diffractometer (D/Max-2500; Rigaku) with Cu Kα was used in the 2θ range of 10–31° at a scan rate of 1°⋅min⁻¹.

Electrochemical Tests.

The battery electrodes were fabricated by first making slurries. For the pure VODP, the active material, super P, and poly(vinylidene fluoride) (PVDF) were dispersed in N-methyl-2-pyrrolidone (99.5%; Aldrich) in a mass ratio of 6: 3: 1. In the case of rGO Na₇V₄(P₂O₇)₄PO₄, the slurry was made in a mass ratio of VODP: rGO: super P: PVDF of 6: 1.5: 1.5: 1. The slurries were cast onto the aluminum foils and were then dried inside a vacuum oven at 70 °C for 12 h. The mass loading of the active material was 2.0 mg⋅cm⁻² for all of the samples. For the actual electrochemical tests, 2,032 coin-type half cells were assembled by using sodium thin disks as both the reference and counter electrodes. One molar sodium perchlorate (98.0%; Aldrich) (NaClO₄) in cosolvent of ethylene carbonate (99.9%, anhydrous; Aldrich) and propylene carbonate (99.7%, anhydrous; Aldrich) with a 1:1 volume ratio was used as electrolyte. The final cells were assembled by sandwiching polyethylene separators (Celgard 2400) between the active electrodes and the sodium thin disks in a glove box. The electrochemical properties were assessed by galvanostatic measurements in the voltage range of 2.0–4.2V (vs. Na/Na⁺) with a battery cycler (WBCS 3000; Wonatech Co.). The QOCP measurements were done at C/20 with a 2-h relaxation time before potential acquisition at each data point. Detailed C-rate conditions are presented in figure legends. All of the C rates addressed in this paper are with respect to 1 C (92.8 mA⋅g⁻¹), not actual charge/discharge durations.

DFT Calculations.

For the voltage and formation energy calculations, up to the 70 lowest electrostatic energy configurations at each composition were sampled and fully optimized with the Vienna Ab initio Simulation Package (44, 45). We adopted the projector-augmented wave method (46) and Perdew–Burke–Ernzerhof exchange-correlation functional (47) with Hubbard parameters (U_eff = 4.5 eV for vanadium) to address the self-interaction energy (48). We used a plane wave basis set with an energy cutoff of 520 eV and sampling the 2 × 2 × 4 Monkhorst–Pack k-point mesh (49). The 1 × 1 × 2 supercells were additionally explored to determine the intermediate phase at the x = 5 composition. Rotation barriers were calculated with the nudged elastic band method (50). All crystal structures were visualized with VESTA software (51).