Ionic Size Effects: Generalized Boltzmann Distributions, Counterion Stratification, and Modified Debye Length

Abstract

Near a charged surface, counterions of different valences and sizes cluster; and their concentration profiles stratify. At a distance from such a surface larger than the Debye length, the electric field is screened by counterions. Recent studies by a variational mean-field approach that includes ionic size effects and by Monte Carlo simulations both suggest that the counterion stratification is determined by the ionic valence-to-volume ratios. Central in the mean-field approach is a free-energy functional of ionic concentrations in which the ionic size effects are included through the entropic effect of solvent molecules. The corresponding equilibrium conditions define the generalized Boltzmann distributions relating the ionic concentrations to the electrostatic potential. This paper presents a detailed analysis and numerical calculations of such a free-energy functional to understand the dependence of the ionic charge density on the electrostatic potential through the generalized Boltzmann distributions, the role of ionic valence-to-volume ratios in the counterion stratification, and the modification of Debye length due to the effect of ionic sizes.

1 Introduction

Electrostatic interactions between macromolecules and mobile ions in an aqueous solvent generate strong, long-ranged forces that play a key role in biological processes. In such interactions, ionic sizes or excluded volumes can affect many of the detailed chemical and physical properties of an underlying biological system. For instance, differences in ionic sizes can affect how mobile ions bind to nucleic acids [3,4,32]. The size of monovalent cations can influence the stability of RNA tertiary structures [21]. The ionic size effect is more profound in the ion channel selectivity, see, e.g., [16, 24]. Detailed Monte Carlo simulations and integral equations calculations also confirm some of these experimentally observed properties due to the non-uniformity of ionic sizes [17, 28, 34, and 36].

The classical Poisson–Boltzmann (PB) equation (PBE) is perhaps the most widely used and efficient model of the electrostatics in ionic solutions, particularly in biomolecular systems [2, 10, 12, 14, 27, 30]. Despite of its many successful applications, this mean-field theory is known to fail in capturing the ionic size effects and ion-ion correlations [15, 17, 28, 35, and 36]. Numerical calculations based on the classical PBE often predict unphysically high concentrations of counterions near a charged surface [5, 38].

Recently, there has been a growing interest in studying the ionic size e ffects within the PB-like mean-field framework [5–7,9,11,18–20,22,23,25,26,31,33,38]. One of the main approaches is to include the additional entropic effect of solvent molecules in the free-energy functional of all local ionic concentrations, c₁= c₁(x),…, c_M= c_M(x) (M being the total number of ionic species), similar to that in the classical PB model. Given the volume υ_i of an ion of the ith species (1 ≤ i ≤ M) and the volume υ₀ of a solvent molecule, one can define the local solvent concentration c₀ = c₀(x) by

$$c_0(x)={\upsilon }_0^{-1}\left[1-\sum _{i=1}^M{\upsilon }_i{c}_i(x)\right].$$ (1.1)

The size-modified electrostatic free-energy functional is then given by [5, 20, 22, and 26]

$$F\left[c_1,\dots ,c_M\right]=\int \left[\frac{1}{2}\rho \psi +{\beta }^{-1}\sum _{i=0}^M{c}_i\left[ln\left({\upsilon }_i{c}_i\right)-1\right]-\sum _{i=1}^M{\mu }_i{c}_i\right]\mathit{\text{dV}}.$$ (1.2)

Here, $\rho ={\displaystyle {\sum }_{i=1}^M{q}_i{c}_i}$ is the ionic charge density, where q_i= z_ie, and z_i is the valence of an ion of the ithe species and e is the elementary charge, β =(k_BT)⁻¹ with k_B the Boltzmann constant and T the temperature, and μ_i is the chemical potential of an ion of the ith species. The electrostatic potential ψ is determined by Poisson's equation −∇ · ɛ∇ψ = ρ, together with some boundary conditions, where ɛ is the dielectric coefficient.

One easily verifies that the equilibrium conditions δ_CiF = 0 (i = 1,…, M) are given by

$$\frac{{\upsilon }_i}{{\upsilon }_0}ln({\upsilon }_0{c}_0)-ln({\upsilon }_i{c}_i)=\beta (q_i\psi -{\mu }_i),i=1,\dots ,M,$$ (1.3)

where ψ is the electrostatic potential corresponding to the equilibrium ionic concentrations c₁,…, c_M. It is shown in [22] that this system of nonlinear algebraic equations has a unique solution c_i= B_i(ψ)(i= 1,…,M), defining the generalized Boltzmann distributions. If υ₀= υ₁= ··· = υ_M, then these distributions are [22,23]

$$c_i=\frac{c_i^{\infty }{e}^{-\beta q_i\psi }}{1+{\sum }_{j=1}^M{\upsilon }_j{c}_j^{\infty }(e^{-\beta q_j\psi }-1)},i=1,\dots ,M,$$

where

$$c_i^{\infty }=\frac{{\upsilon }_i^{-1}{e}^{\beta {\mu }_i}}{1+{\sum }_{j=1}^M{e}^{\beta {\mu }_j}},i=1,\dots ,M.$$

Note that, for each i, $c_i^{\infty }$ is the value of c_i when ψ = 0. In general, if υ₀, υ₁,…, υ_M are not all the same, then explicit formulas for c_i= B_i(ψ)(i=1,…,M) seem unavailable.

In studying the ionic size effects in biomolecular reactions, Lu and Zhou [26] minimized the free-energy functional (1.2)) by solving for a steady-state solution of a size-modified Poisson–Nernst–Planck system of partial differential equations. In [38], Zhou et al. developed a constrained optimization method for numerically minimizing the functional (1.2) with Poisson's equation as a constraint. They found the remarkable stratification of ionic concentrations near a highly charged surface. Moreover, they found that the ionic valence-to-volume ratio is an important parameter that determines the stratification. Specifically, the counterion with the largest valence-to-volume ratio has the highest ionic concentration near the surface, the counterion with the second largest such ratio has also the second highest ionic concentration, and so on. Subsequently, Wen et al.[37] performed Monte Carlo simulations that confirm such a phenomenon.

In this work, we further study the size-modified, mean-field free-energy functional (1.2). We begin with a description of two different forms of the electrostatic free-energy functional, one with and the other without the constraint of total number of ions for each ionic species. We show the equivalence of these two forms; cf. Theorem 2.2. We also derive the conditions for the equilibrium concentrations; cf. (1.3).

We then focus on the equilibrium conditions (1.3) to analyze the generalized Boltzmann distributions. In particular, we characterize the behavior of the equilibrium ionic charge density ${\rho }_i={\sum }_{i=1}^M{q}_i{c}_i$ as a function of ψ. Our main results of analysis are as follows:

1. The concentration of ions with the largest and that with smallest valence-to-volume ratios monotonically decreases and monotonically increases, respectively, with respect to the electrostatic potential ψ. As ψ → –∞ (or ∞), the concentration of ions with the largest (or smallest) valence-to-volume ratio approaches to the inverse of its corresponding volume, while all other concentrations approach zero.

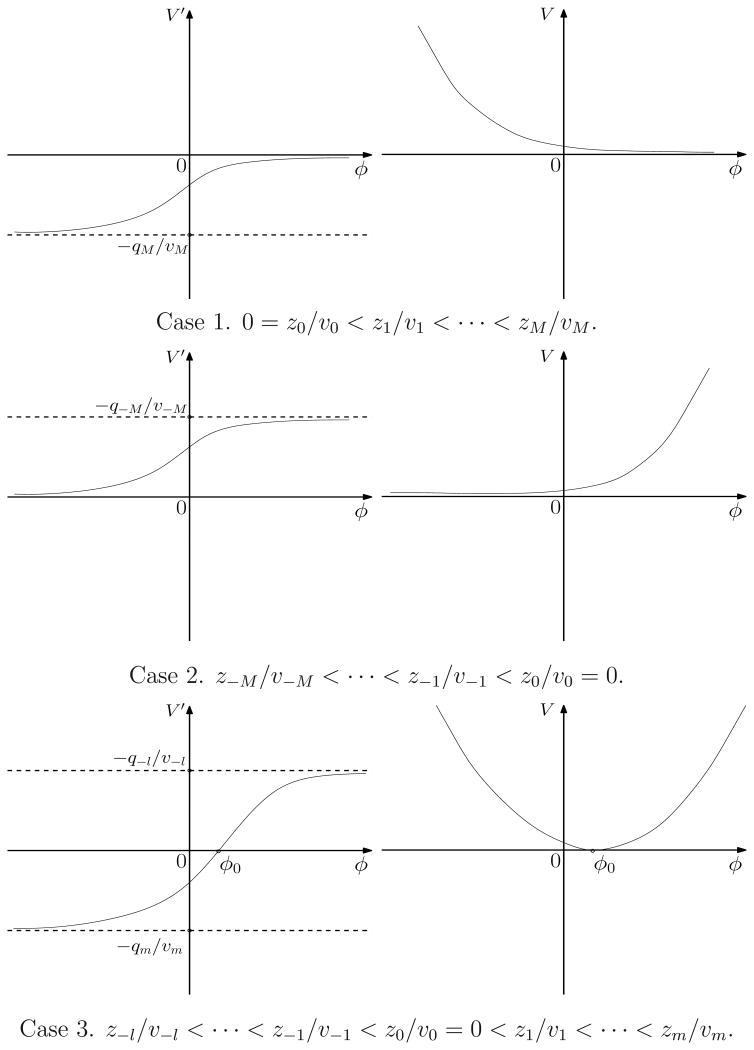
2. The total ionic charge density ρ_i= ρ_i(ψ) is a monotonic function of the potential ψ. If all ions are cations (i.e., all z_j> 0), then this density is always positive, ρ_i(∞) = 0, and ρ_i(–∞) exists and is finite. If all ions are anions (i.e., all z_j< 0), then this density is always negative, ρ_i(–∞) = 0, and ρ_i(∞) exists and is finite. If both cations and anions exist, then both ρ_i(–∞) and ρ_i(∞) exist and are finite, and the density reaches zero at a unique value of the potential ψ. All these are summarized in Figure 2 in Subsection 3.2.

3. A size-modified Debye length, λ̂_D, is derived with a weak potential limit. A formula of of λ̂ _D is given in (3.23) in Subsection 3.3.

Figure 2.

Graphs of V′ = V′(ϕ) (left) and V = V(ϕ) (right) for the three different cases. The value −V′(ϕ) is the ionic charge density at a (shifted) electrostatic potential ϕ.

Note that ionizable groups of biomolecules can dissociate in aqueous solution to produce anions or cations. So, studying an ionic system with only cations or only anions is of interest. In general, such a system can serve a model approximation system, as near a charged surface the concentration of coions is usually very low.

We finally consider a charged spherical molecule of radius R₀ in an ionic solution that occupies a region defined by {R₀< |x| < R_∞} for some R_∞ > R₀, and study the PBE, both classical and size-modified. In the case R_∞ is finite, the detailed properties of the equilibrium electrostatic potential are described through two parameters: one is the prescribed surface charge density σ at the boundary |x| = R₀ and the other is an effective surface charge density σ_∞ at the boundary |x| = R_∞. This effective density is set by the total charge neutrality. When σσ_∞< 0 the potential ψ is monotonic. Otherwise, it decreases then increases, or it increases then decreases. These are summarized in Theorem 4.2 and illustrated in Figure 3 in Section 4. With the same setting of a charged spherical molecule, we numerically minimize the functional (1.2) and confirm our analysis; cf. Figure 4 in Section 5. If R_∞= ∞, then only in the case that both cations and anions exist and they neutralize in the bulk does the PBE (classical or size-modified) permit a unique solution, the electrostatic potential, that is radially symmetric and monotonic, and vanishes at infinity. We remark that considering a finite R_∞ is of certain interest as it is known that the effect of finite size of charged system can be significant, cf. e.g., [29], and as it is practically useful in numerical computations.

Figure 3.

The graph of electrostatic potential ψ.

Figure 4.

The electrostatic potential ψ (left) and the equilibrium ionic concentrations (right). Top. Case I: (N₁, N₂, N₃,N₄) = (60,30,20,50), σ < 0, and σ_∞ > 0. Bottom. Case II: (N₁, N₂, N₃,N₄) = (50,50,50,50), σ < 0, and σ_∞ < 0. The concentrations profiles are marked by the corresponding ionic valences.

The rest of this paper is organized as follows: In Section 2, we describe the electrostatic free-energy functionals and derive the conditions of equilibrium concentrations. In Section 3, we present an analysis on the equilibrium conditions and on the stratification of counterions of multiple valences and sizes near a charged surface. In Section 4, we study a charged spherical molecule immersed in an ionic solution. In Section 5, we report numerical calculations to confirm our analysis. Finally, in Section 6, we draw conclusions.

2 The Free-Energy Functional and Equilibrium Conditions

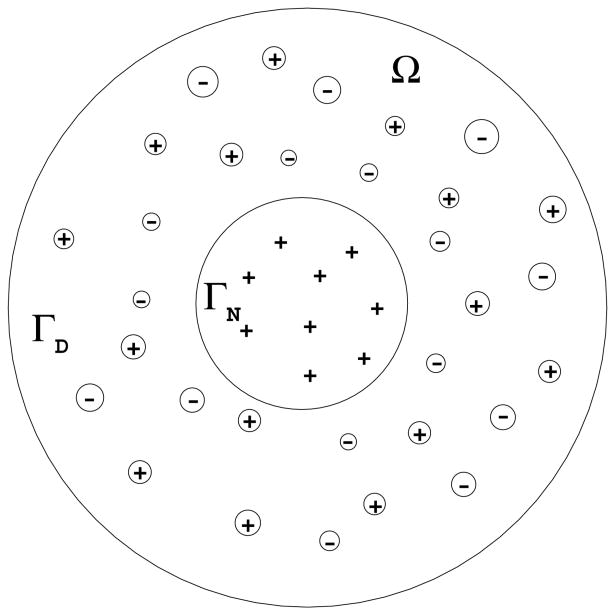
We consider an ionic solution with M (M ≥ 1) ionic species, occupying a region Ω that is a bounded, open, and connected subset of ℝ³ with a smooth boundary Γ ≠ ∅. The boundary Γ is divided into two disjoint and smooth parts Γ_D and Γ_N (with D for Dirichlet and N for Neumann). We assume that a surface charge density is given on Γ_N and the electrostatic potential is specified on Γ_D. Our set up covers the case that Γ_N = ∅ and Γ_D = Γ and that Γ_D = ∅ and Γ_N = Γ. It also covers the important case of biomolecular solvation with an implicit solvent: The region Ω is the solvent region and the part of the boundary Γ_N is the solute-solvent interface; cf. Figure 1. The surface charge density on Γ_N effectively replaces the density of partial charges carried by solute atoms.

Figure 1.

A schematic view of a charged molecule immersed in an ionic solution. The molecule is represented by the inner sphere filled with symbols +.

For each i (1 ≤ i ≤ M), we denote by c_i= c_i(x) the local ionic concentration of the ith ionic species at position x∈Ω. As before, $\rho ={\sum }_{i=1}^M{q}_i{c}_i$ is the ionic charge density. We also denote by υ_i (1 ≤ i ≤ M) the volume of an ion of the ith species and by υ₀ the volume of a solvent molecule. The local concentration of the solvent, c₀ = c₀(x), is then defined by (1.1). For convenience we denote c =(c₁,…, c_M). We consider minimizing the mean-field electrostatic free-energy functional

$$F\left[c\right]={\int }_{\mathrm{\Omega }}\frac{1}{2}\rho \psi \mathit{\text{dV}}+{\int }_{{\mathrm{\Gamma }}_{\mathrm{N}}}\frac{1}{2}\sigma \psi \mathit{\text{dS}}+{\beta }^{-1}\sum _{i=0}^M{\int }_{\mathrm{\Omega }}{c}_i\left[ln({\upsilon }_i{c}_i)-1\right]\mathit{\text{dV}}-\sum _{i=1}^M{\int }_{\mathrm{\Omega }}{\mu }_i{c}_i\mathit{\text{dV}},$$ (2.1)

$$\text{with}\{\begin{array}{ll}\nabla \cdot \varepsilon \nabla \psi =-\rho \hfill & \text{in}\mathrm{\Omega },\hfill \\ \psi ={\psi }_{\infty }\hfill & \text{on}{\mathrm{\Gamma }}_{\mathrm{D}},\hfill \\ \varepsilon \frac{\partial \psi }{\partial n}=\sigma \hfill & \text{on}{\mathrm{\Gamma }}_{\mathrm{N}}.\hfill \end{array}$$ (2.2)

Here, the first two terms in (2.1) describe the electrostatic potential energy. The electrostatic potential ψ is the unique solution of the boundary-value problem of Poisson's equation (2.2), where ɛ = ɛ(x)(x ∈ Ω) is the dielectric coefficient assumed to be Lebesgue measurable and bounded above and below by positive constants, and n denotes the unit exterior normal at the boundary Γ. Both ψ_∞ : Γ_D → ℝ and σ : Γ_N → ℝ are given bounded and smooth functions, representing the electrostatic potential at Γ_D and the surface charge density at Γ_N, respectively. The third term in (2.1) has the form –TS with S being the entropy. The given parameters μ_i(i = 1,…, M) in the last term in (2.1) are the chemical potentials of the ions.

We define ψ_D : Ω → ℝ and ψ_N : Ω → ℝ by

$$\{\begin{array}{ll}\nabla \cdot \varepsilon \nabla {\psi }_{\mathrm{D}}=0\hfill & \text{in}\mathrm{\Omega },\hfill \\ {\psi }_{\mathrm{D}}={\psi }_{\infty }\hfill & \text{on}{\mathrm{\Gamma }}_{\mathrm{D}},\hfill \\ \varepsilon \frac{\partial {\psi }_{\mathrm{D}}}{\partial n}=0\hfill & \text{on}{\mathrm{\Gamma }}_{\mathrm{N}},\hfill \end{array}\begin{array}{c}\text{and}\end{array}\{\begin{array}{ll}\nabla \cdot \varepsilon \nabla {\psi }_{\mathrm{N}}=0\hfill & \text{in}\mathrm{\Omega },\hfill \\ {\psi }_{\mathrm{N}}=0\hfill & \text{on}{\mathrm{\Gamma }}_{\mathrm{D}},\hfill \\ \varepsilon \frac{\partial {\psi }_{\mathrm{N}}}{\partial n}=\sigma \hfill & \text{on}{\mathrm{\Gamma }}_{\mathrm{N}},\hfill \end{array}$$

respectively. For a given function f : Ω → ℝ that is in the Sobolev space H⁻¹(Ω)[1,13], let us denote by Lf :Ω → ℝ the unique weak solution to the following boundary-value problem of Poisson's equation

$$\{\begin{array}{ll}\nabla \cdot \varepsilon \nabla (Lf)=-f\hfill & \text{in}\mathrm{\Omega },\hfill \\ Lf=0\hfill & \text{on}{\mathrm{\Gamma }}_{\mathrm{D}},\hfill \\ \varepsilon \frac{\partial (Lf)}{\partial n}=0\hfill & \text{on}{\mathrm{\Gamma }}_{\mathrm{N}}.\hfill \end{array}$$

(The uniqueness is guaranteed if Γ_D has a nonzero surface measure.) Notice that L is a linear and self-adjoint operator from the space H⁻¹(Ω) to that consisting of H¹(Ω)-functions vanishing on Γ_D. With these the solution ψ to the boundary-value problem (2.2) can be decomposed as

$$\psi =\widehat{\psi }+{\psi }_{\mathrm{D}}+{\psi }_{\mathrm{N}},$$ (2.3)

where $\widehat{\psi }=L\left({\sum }_{i=1}^M{q}_i{c}_i\right).$ By integration by parts, we have

$$\begin{array}{lll}{\int }_{{\mathrm{\Gamma }}_{\mathrm{N}}}\sigma \widehat{\psi }\mathit{\text{dS}}\hfill & =\hfill & {\int }_{{\mathrm{\Gamma }}_{\mathrm{N}}}\varepsilon \frac{\partial {\psi }_{\mathrm{N}}}{\partial n}\widehat{\psi }\mathit{\text{dS}}={\int }_{\mathrm{\Gamma }}\varepsilon \frac{\partial {\psi }_{\mathrm{N}}}{\partial n}\widehat{\psi }\mathit{\text{dS}}\hfill \\ \hfill & =\hfill & {\int }_{\mathrm{\Omega }}\widehat{\psi }\nabla \cdot \varepsilon \nabla {\psi }_{\mathrm{N}}\mathit{\text{dV}}+{\int }_{\mathrm{\Omega }}\varepsilon \nabla \widehat{\psi }\cdot \nabla {\psi }_{\mathrm{N}}\mathit{\text{dV}}={\int }_{\mathrm{\Omega }}\varepsilon \nabla \widehat{\psi }\cdot \nabla {\psi }_{\mathrm{N}}\mathit{\text{dV}}\hfill \\ \hfill & =\hfill & {\int }_{\mathrm{\Omega }}(-\nabla \cdot \varepsilon \nabla \widehat{\psi }){\psi }_{\mathrm{N}}\mathit{\text{dV}}={\int }_{\mathrm{\Omega }}\left({\displaystyle \sum _{i=1}^M{q}_i{c}_i}\right){\psi }_{\mathrm{N}}\mathit{\text{dV}}.\hfill \end{array}$$

Therefore the free-energy functional F[c] can now be rewritten as

$$F\left[c\right]={\int }_{\mathrm{\Omega }}\frac{1}{2}\left(\sum _{i=1}^M{q}_i{c}_i\right)L\left(\sum _{j=1}^M{q}_j{c}_j\right)\mathit{\text{dV}}+\sum _{i=1}^M{\int }_{\mathrm{\Omega }}\left[q_i\left(\frac{1}{2}{\psi }_{\mathrm{D}}+{\psi }_{\mathrm{N}}\right)-{\mu }_i\right]c_i\mathit{\text{dV}}+{\beta }^{-1}\sum _{i=0}^M{\int }_{\mathrm{\Omega }}{c}_i[ln({\upsilon }_i{c}_i)-1]\mathit{\text{dV}}+{\int }_{{\mathrm{\Gamma }}_{\mathrm{N}}}\frac{1}{2}\sigma ({\psi }_{\mathrm{N}}+{\psi }_{\mathrm{D}})\mathit{\text{dS}}.$$

By formal and routine calculations using the decomposition (2.3) and the fact that L is self-adjoint, we obtain the first variations [8,22,23,and 26]

$${\delta }_{c_i}F[c]=q_i\left(\psi -\frac{1}{2}{\psi }_{\mathrm{D}}\right)-{\mu }_i+{\beta }^{-1}\left[ln({\upsilon }_i{c}_i)-\frac{{\upsilon }_i}{{\upsilon }_0}ln({\upsilon }_0{c}_0)\right],i=1,\dots ,M,$$ (2.4)

where ψ is determined by (2.2) with $\rho ={\sum }_{i=1}^M{q}_i{c}_i$. The equilibrium conditions δ_ciF[c] = 0 (i=1,…,M) are then given by

$$\frac{{\upsilon }_i}{{\upsilon }_0}ln({\upsilon }_0{c}_0)-ln({\upsilon }_i{c}_i)=\beta \left[q_i\left(\psi -\frac{1}{2}{\psi }_{\mathrm{D}}\right)-{\mu }_i\right],i=1,\dots ,M.$$ (2.5)

The following result can be proved by the argument used in [22]:

Theorem 2.1

1. The functional F[c] is convex. Moreover, it has a unique minimizer in the set of all concentrations c₁,…,c_M such that c_i ≥ 0 (i = 1,…, M) and ${\sum }_{i=1}^M{\upsilon }_i{c}_i\le 1$ in Ω.

2. There exist θ₁, θ₂ ∈ ℝ with 0 < θ₁ < θ₂ < 1 that bound uniformly from below and above, respectively, the free-energy minimizing ionic concentrations and the corresponding concentration of solvent molecules.

3. The minimizer is characterized by the equilibrium conditions (2.5).

We now consider a different formulation: minimize the electrostatic free-energy functional

$$\widehat{F}\left[c\right]={\int }_{\mathrm{\Omega }}\frac{1}{2}\rho \psi \mathit{\text{dV}}+{\int }_{{\mathrm{\Gamma }}_{\mathrm{N}}}\frac{1}{2}\sigma \psi \mathit{\text{dS}}+{\beta }^{-1}\sum _{i=0}^M{\int }_{\mathrm{\Omega }}{c}_i[ln({\upsilon }_i{c}_i)-1]\mathit{\text{dV}},$$ (2.6)

$$\text{with}\{\begin{array}{ll}\nabla \cdot \varepsilon \nabla \psi =-\rho \hfill & \text{in}\mathrm{\Omega },\hfill \\ \psi ={\psi }_{\infty }\hfill & \text{on}{\mathrm{\Gamma }}_{\mathrm{D}},\hfill \\ \varepsilon \frac{\partial \psi }{\partial n}=\sigma \hfill & \text{on}{\mathrm{\Gamma }}_{\mathrm{N}},\hfill \end{array}$$ (2.7)

$$\text{subject to}{\int }_{\mathrm{\Omega }}{c}_i\mathit{\text{dV}}=N_i,i=1,\dots ,M,$$ (2.8)

where again $\rho ={\sum }_{i=1}^M{q}_i{c}_i.$ Note that the functional F[c] defined in (2.1) has the term of the chemical potentials μ_i. Here the functional F̂ [c] does not have that term. On the other hand, the minimization of F̂ [c] is subject to the conservation of total number of ions in each of the M ionic species, defined in (2.8) in which N_i(i = 1,…, M) are given positive numbers. The system (2.7) is the same as (2.2).

It again follows from standard arguments that the functional F̂[c] with (2.7) has a unique minimizer ĉ =(ĉ₁, …, ĉ_M) among all c = (c₁,…, c_M) subject to (2.8). By a similar argument used in deriving (2.4), we have for any c =(c₁,…, c_M) that

$${\delta }_{c_i}\widehat{F}\left[c\right]=q_i\left(\psi -\frac{1}{2}{\psi }_{\mathrm{D}}\right)+{\beta }^{-1}\left[ln({\upsilon }_i{c}_i)-\frac{{\upsilon }_i}{{\upsilon }_0}ln({\upsilon }_0{c}_0)\right],i=1,\dots ,M.$$ (2.9)

For the minimizer ĉ, we have

$${\int }_{\mathrm{\Omega }}\left({\delta }_{c_i}\widehat{F}\left[\widehat{c}\right]\right)d_i\mathit{\text{dV}}=0\forall d_i:{\int }_{\mathrm{\Omega }}{d}_i\mathit{\text{dV}}=0,i=1,\dots ,M.$$

By Lemma 2.1 below, these lead to the existence of constants, still denoted by μ_i (i = 1,…, M), such that δ_ci F̂[ĉ]= μ_i(i = 1,…, M). By (2.9), this is exactly (2.5).

We have in fact proved the following:

Theorem 2.2

The variational problem of minimizing the functional (2.1) with (2.2) is equivalent to that of minimizing the functional F̂ [c] with (2.7) and (2.8).

We remark that, if one knows the chemical potentials μ_i (i = 1,…, M), then the first formulation, i.e., minimizing F[c], can be mathematically and computationally simpler than the second one that has the constraint of total numbers of ions. The question is how the chemical potentials can be estimated by experiment or other models. A similar practical issue for the second formulation with the constraint (2.8) is that one needs to have a good estimate of each N_i, the total number of ions of the ith species in the system.

Lemma 2.1

Let u ∈ L²(Ω) be such that

$${\int }_{\mathrm{\Omega }}u\upsilon \mathit{\text{dV}}=0\forall \upsilon \in L^2(\mathrm{\Omega }):{\int }_{\mathrm{\Omega }}\upsilon \mathit{\text{dV}}=0.$$

Then there exists a constant μ ∈ ℝ such that u = μ almost everywhere in Ω.

Proof

Denote for any w ∈ L²(Ω)

$$\overline{w}={\int }_{\mathrm{\Omega }}-w\mathit{\text{dV}}=\frac{1}{\left|\mathrm{\Omega }\right|}{\int }_{\mathrm{\Omega }}w\mathit{\text{dV}},$$

where |Ω| is the volume (i.e., the Lebesgue measure) of Ω. Note that the integral of w – w̄ over Ω vanishes. For any υ ∈ L²(Ω), we have by the assumption of the lemma that

$${\int }_{\mathrm{\Omega }}(u-\overline{u})\upsilon \mathit{\text{dV}}={\int }_{\mathrm{\Omega }}(u-\overline{u})(\upsilon -\overline{\upsilon })\mathit{\text{dV}}={\int }_{\mathrm{\Omega }}u(\upsilon -\overline{\upsilon })\mathit{\text{dV}}=0.$$

Therefore u = ū almost everywhere in Ω. Set μ = ū. The Lemma is proved. Q.E.D.

3 Equilibrium Ionic Concentrations and Modified De-bye Length

We consider the equilibrium conditions (2.5) that determine the equilibrium ionic concentrations. Introducing a shifted potential ϕ = ψ – ψ_D/2, we can rewrite (2.5) as

$$\frac{{\upsilon }_i}{{\upsilon }_0}ln({\upsilon }_0{c}_0)-ln({\upsilon }_i{c}_i)=\beta (q_i\varphi -{\mu }_i),i=1,\dots ,M.$$ (3.1)

As c₀ is given in (1.1), this is a system of nonlinear algebraic equations for the unknowns c₁,…, c_M. In [22], Li proved the following:

Theorem 3.1

1. For each ϕ ∈ ℝ the system (3.1) has a unique solution c_i = B_i(ϕ) ∈ $(0,{\upsilon }_i^{-1})$ (i = 1,…,M). Moreover each B_i:ℝ→ $(0,{\upsilon }_i^{-1})$ is a smooth function.

2. Define V : ℝ → ℝ by

   $$V(\varphi )=-\sum _{i=1}^M{\int }_0^{\varphi }{q}_i{B}_i(\xi )d\xi +V_0,$$ (3.2)

   where V₀ is a constant. Then the function V : ℝ → ℝ satisfies V″ > 0 on ℝ and is thus strictly convex.

The relations c_i = B_i(ϕ) (i = 1,…, M) define the generalized Boltzmann distributions for the equilibrium concentrations c₁, …, c_M. In general, explicit formulas of such distributions seem to be unavailable. Notice that $-V^{\prime }\left(\varphi \right)={\sum }_{i=1}^M{q}_i{B}_i(\varphi )$ with ϕ = ψ – ψ_D/2 is the ionic charge density. The proof of V″ > 0 in ℝ given in [22] (cf. the proof of Lemma 5.2 in [22]) applies to our present case; cf. the remark in Subsection 3.1. The electrostatic potential ψ is now the unique solution to the boundary-value problem of the generalized and implicit PBE

$$\nabla \cdot \varepsilon \nabla \psi -V^{\prime }\left(\psi -\frac{{\psi }_D}{2}\right)=0.$$ (3.3)

The boundary conditions are the same as in (2.2). The existence and uniqueness of the equilibrium concentrations c_i (i = 1,…, M) lead to that of the solution to this boundary-value problem.

3.1 Equilibrium ionic concentrations

For convenience, we denote z₀= 0, q₀= 0, and μ₀= 0. We assume that all the M ionic species have different ionic valence-to-volume ratios. We assume there are l ≥ 0 species of anions and m ≥ 0 species of cations. So M = l+m. If l = 0 then all ions are cations. If m = 0 then all ions are anions. We label all the M ionic species and the solvent concentration by −l,…, −1, 0,1,…,m, and assume that

$$\frac{z_{-l}}{{\upsilon }_{-l}}<\cdots <\frac{z_{-1}}{{\upsilon }_{-1}}<\frac{z_0}{{\upsilon }_0}=0<\frac{z_1}{{\upsilon }_1}<\cdots <\frac{z_m}{{\upsilon }_m}.$$ (3.4)

For convenience, we denote J = {−l,…, −1,0,1,…, m}. With our notation, the equilibrium conditions (2.5) then become

$$\frac{{\upsilon }_i}{{\upsilon }_0}ln({\upsilon }_0{c}_0)-ln({\upsilon }_i{c}_i)=\beta (q_i\varphi -{\mu }_i)\forall i\in J.$$ (3.5)

Note that by Theorem 3.1 c_i= B_i (ϕ) for any i ∈ J with i ≠ 0. We thus have by (1.1) that

$$c_0=B_0(\varphi )={\upsilon }_0^{-1}\left[1-\sum _{i\in J,i\ne 0}{\upsilon }_i{B}_i(\varphi )\right].$$ (3.6)

Theorem 3.2

Let c_i = B_i(ϕ) (i ∈ J) be the equilibrium concentrations defined by (3.5) and (3.6). Assume (3.4) holds true.

1. Monotonicity of concentrations with the largest and smallest valence-to-volume ratios. We have ${c^{\prime }}_{-l}(\varphi )>0$ and ${c^{\prime }}_m(\varphi )<0$ for all ϕ ∈ ℝ.

2. Stratification of concentration profiles. If i ∈ {−l,…, m − 1} then

   $${({\upsilon }_i{c}_i)}^{1/{\upsilon }_i}<{({\upsilon }_{i+1}{c}_{i+1})}^{1/{\upsilon }_{i+1}}\mathit{\text{if and only if}}\varphi <\frac{{\mu }_{i+1}/{\upsilon }_{i+1}-{\mu }_i/{\upsilon }_i}{q_{i+1}/{\upsilon }_{i+1}-q_i/{\upsilon }_i}.$$

   If i ∈ { −l+1,…,m} then

   $${({\upsilon }_i{c}_i)}^{1/{\upsilon }_i}>{({\upsilon }_{i-1}{c}_{i-1})}^{1/{\upsilon }_{i-1}}\mathit{\text{if and only if}}\varphi >\frac{{\mu }_{i-1}/{\upsilon }_{i-1}-{\mu }_i/{\upsilon }_i}{q_{i-1}/{\upsilon }_{i-1}-q_i/{\upsilon }_i}.$$

3. Asymptotic behavior of concentrations. We have

   $${\upsilon }_{-l}{c}_{-l}\to 1\mathit{\text{and}}{c}_i\to 0(i=-l+1,\mathrm{\dots },m)\mathit{\text{as}}\varphi \to \infty ,$$ (3.7)
   $${\upsilon }_m{c}_m\to 1\mathit{\text{and}}{c}_i\to 0(i=-l,\dots ,m-1)\mathit{\text{as}}\varphi \to -\infty .$$ (3.8)

Proof

We first prove that

$${({\upsilon }_i{c}_i)}^{1/{\upsilon }_i}=e^{-\beta ({\mu }_j/{\upsilon }_j-{\mu }_i/{\upsilon }_i)}{e}^{\beta (q_j/{\upsilon }_j-q_i/{\upsilon }_i)\varphi }{({\upsilon }_j{c}_j)}^{1/{\upsilon }_j}\forall i,j\in J.$$ (3.9)

In fact, it follows immediately from (3.5) that

$${({\upsilon }_0{c}_0)}^{1/{\upsilon }_0}=e^{-\beta {\mu }_i/{\upsilon }_i}{e}^{\beta (q_i/{\upsilon }_i)\varphi }{({\upsilon }_i{c}_i)}^{1/{\upsilon }_i}\forall i\in J.$$ (3.10)

This also implies

$${({\upsilon }_i{c}_i)}^{1/{\upsilon }_i}=e^{\beta {\mu }_i/{\upsilon }_i}{e}^{-\beta (q_i/{\upsilon }_i)\varphi }{({\upsilon }_0{c}_0)}^{1/{\upsilon }_0}\forall i\in J.$$ (3.11)

It follows from (3.10) and (3.11) that (3.9) holds true if one of i and j is 0. Now let i,j∈J with i ≠ j,i≠0, and j ≠ 0. We have by (3.5) that

$$\frac{1}{{\upsilon }_i}ln({\upsilon }_i{c}_i)+\frac{\beta q_i}{{\upsilon }_i}\varphi -\frac{\beta {\mu }_i}{{\upsilon }_i}=\frac{1}{{\upsilon }_j}ln({\upsilon }_j{c}_j)+\frac{\beta q_j}{{\upsilon }_j}\varphi -\frac{\beta {\mu }_j}{{\upsilon }_j}.$$

Consequently,

$${({\upsilon }_i{c}_i)}^{1/{\upsilon }_i}=e^{-\beta ({\mu }_j/{\upsilon }_j-{\mu }_i/{\upsilon }_i)}{e}^{\beta (q_j/{\upsilon }_j-q_i/{\upsilon }_i)\varphi }{({\upsilon }_j{c}_j)}^{1/{\upsilon }_j}.$$

This, together with (3.10) and (3.11), implies (3.9).

We now prove parts (1)–(3).

1. Denote γ = υ₀c₀ and η_i = e^βμi for each i ∈ J. It follows from the equilibrium conditions (3.5) that

   $$c_i=\frac{{\eta }_i}{{\upsilon }_i}{\gamma }^{{\upsilon }_i/\upsilon 0}{e}^{-\beta q_i\varphi }\forall i\in J.$$ (3.12)

   This and the definition of c₀ (cf. (1.1)) imply

   $$\sum _{i\in J}{\eta }_i{\gamma }^{{\upsilon }_i/\upsilon 0}{e}^{-\beta q_i\varphi }=1.$$ (3.13)

   Taking the derivative of both sides of (3.13) with respective to ϕ, and using our notation z₀= 0 and μ₀= 0, we obtain

   $${\gamma }^{\prime }(\varphi )=\frac{\beta {\upsilon }_0\gamma {\sum }_{i\in J}{\eta }_i{q}_i{\gamma }^{{\upsilon }_i/{\upsilon }_0}{e}^{-\beta q_i\varphi }}{{\sum }_{i\in J}{\eta }_i{\upsilon }_i{\gamma }^{{\upsilon }_i/{\upsilon }_0}e-\beta q_i\varphi }.$$

   Fix k ∈ J. Taking the derivative of both sides of (3.12) with i = k with respect to ϕ and using the above expression of γ′ (ϕ), we get

   $${c^{\prime }}_k(\varphi )=\beta {\eta }_k{\gamma }^{{\upsilon }_k/{\upsilon }_0}e-\beta q_k\varphi \frac{{\sum }_{i\in J}{\eta }_i{\upsilon }_i(q_i/{\upsilon }_i-q_k/{\upsilon }_k){\gamma }^{{\upsilon }_i/{\upsilon }_0}{e}^{-\beta q_i\varphi }}{{\sum }_{i\in J}{\eta }_i{\upsilon }_i{\gamma }^{{\upsilon }_i/{\upsilon }_0}{e}^{-\beta q_i\varphi }}.$$ (3.14)

   This, the assumption (3.4), and the definition q_i= z_ie (i ∈ J) imply that ${c^{\prime }}_m(\varphi )<0$ and ${c^{\prime }}_{-l}(\varphi )>0$.

2. This follows directly from (3.9).

3. Since 0 ≤ υ_−lc_−l ≤ 1, we have again by (3.9) with j = −l and (3.4) that for any i ∈ J with i > −l

   $${({\upsilon }_i{c}_i)}^{1/{\upsilon }_i}\le e^{-\beta ({\mu }_{-l}/{\upsilon }_{-l}-{\mu }_i/{\upsilon }_i)}{e}^{\beta (q_{-l}/{\upsilon }_{-l}-q_i/{\upsilon }_i)\varphi }\to 0\text{as}\varphi \to \infty .$$

   Since ${\sum }_{i\in J}{\upsilon }_i{c}_i=1$, we thus also have υ_−lc_−l → 1 as ϕ → ∞. This proves (3.7). The proof of (3.8) is similar. Q.E.D.

Remark

By (3.14) and the Cauchy–Schwarz inequality, we obtain through a series of calculations that

$$\sum _{k\in J}{q}_k{c^{\prime }}_k(\varphi )=\frac{\beta \left[{\left({\sum }_{i\in J}{q}_i{\upsilon }_i{c}_i\right)}^2-\left({\sum }_{i\in J}{q}_i^2{c}_i\right)\left({\sum }_{i\in J}{\upsilon }_i^2{c}_i\right)\right]}{{\sum }_{i\in J}{\upsilon }_i^2{c}_i}\le 0.$$

Since q_i/υi(i ∈ J) are not all the same, this is a strict inequality. Thus the function V : ℝ → ℝ, defined in (3.2) with −V′(ϕ) being the total ionic charge density, is a strictly convex function of ϕ; cf. Theorem 3.1.

3.2 Equilibrium charge density

We now study the properties of the ionic charge density $V^{\prime }(\varphi )=-{\sum }_{i\in J}{q}_i{B}_i(\varphi )$. By (3.2), the function V is given now by

$$V(\varphi )=-\sum _{i\in J}{\int }_0^{\varphi }{q}_i{B}_i(\xi )d\xi +V_0.$$ (3.15)

We distinguish three cases:

1. l = 0, m = M, and J = {0,1,…, M};

2. l = M, m = 0, and J = {−M,…,−1,0};

3. l ≥ 1, m ≥ 1, l + m = M, and J = {−l,…, −1,0,1,…, m}.

We denote f(−∞) = lim_{s→ − ∞}f(s) and f(∞) = lim_{s→ ∞}f(s) if the limits exist.

Theorem 3.3

Assume (3.4) holds true.

1. If l = 0 and m = M, and J = {0,1,…, M}, then V′(−∞) = −q_M/υ_M< 0, V′ (∞) = 0, and V′(ϕ) < 0 for all ϕ ∈ ℝ. Moreover, V(−∞) = ∞, and V(∞) = 0 with a suitably chosen constant V ₀in (3.2).

2. If l = M, m = 0, and J = {−M, …,−1,0}, then V′(−∞) = 0, V′(∞) = −q_−M/υ_−M > 0, and V′(ϕ)> 0 for all ϕ ∈ ℝ.Moreover, V(−∞) = 0 with a suitably chosen constant V₀in (3.2), and V(∞) = ∞.

3. If l ≥ 1, m ≥ 1, l + m = M, and J = {−l,…, −1,0,1,…,m}, then V′(−∞) = −q_m/υ_m < 0, V′(∞) = −q_−l/υ_−l> 0, and −q_m/υ_m < V′(ϕ)< −q_−l/υ_−lfor all ϕ∈ ℝ. Moreover, min_ϕ∈ℝV(ϕ)= 0 with a suitably chosen constant V₀in (3.2), V(−∞) = ∞, and V(∞) = ∞.

In Figure 2, we show the schematic behavior of the convex function V for the three different cases.

Proof of Theorem 3.3

1. In this case, we have V′(−∞) = −q_M/υ_M < 0 by (3.8) and V′(∞) = 0 by (3.7). Since V″ > 0 in ℝ by Theorem 3.1, V′ is a strictly increasing function. Therefore, V′(−∞) < 0 and V′(∞) = 0 imply that V′(ϕ) < 0 for all ϕ ∈ ℝ. Fix ϕ₀∈ ℝ so that V′(ϕ₀) < 0. Then for any ϕ < ϕ₀ we have V(ϕ)= V(ϕ₀) + V′(ξ)(ϕ − ϕ₀), where ξ ∈ (ϕ, ϕ₀). Notice that V′(ξ) < V′(ϕ₀) < 0. So, V(ϕ) → ∞ as ϕ → − ∞, i.e., V(−∞) = ∞. Finally, setting j = 0 in (3.9), we obtain that

   $${({\upsilon }_i{B}_i(\varphi ))}^{1/{\upsilon }_i}={({\upsilon }_i{c}_i)}^{1/{\upsilon }_i}\le \left(\underset{1\le i\le M}{max}{e}^{\beta {\mu }_i/{\upsilon }_i}\right)e^{-\beta (q_i/{\upsilon }_i)\varphi }.$$

   Therefore, the integral of ${\sum }_{i=1}^M{q}_i{B}_i(\varphi )$ from 0 to ϕ > 0 is a bounded function of ϕ. Thus, by the definition of V (cf. (3.2)), V(ϕ) is bounded below. Since V′ < 0 on ℝ, V decreases. Therefore, V(∞) exists. Choosing the constant

   $$V_0=\sum _{i\in J}{\int }_0^{\infty }{q}_i{B}_i(\varphi )d\varphi$$

   in (3.15), we then have V(∞) = 0.

2. This part can be proved similarly.

3. The fact that V′(−∞) = −q_m/υ_m< 0 and V′(∞) = −q_−l/υ_−l > 0 can be proved by the same argument used for proving part (1). Since V″ > 0 in ℝ, V′(ϕ) is a strictly increasing function. Therefore, −q_m/υ_m< V′(ϕ)< −q_l/υ_l for all ϕ ∈ ℝ. Again by the same argument used in part (1), we have V(−∞) = ∞ and V(∞) = ∞. It is clear now that there exists a unique ϕ₀ ∈ ℝ such that V′(ϕ₀) = 0 and V(ϕ₀) = min_ϕ∈ℝV(ϕ). Setting in (3.15),

   $$V_0=\sum _{i\in J}{\int }_0^{\varphi 0}{q}_i{B}_i(\varphi )d\varphi ,$$

   we have min_ϕ∈ℝV(ϕ) = 0. Q.E.D.

3.3 Modified Debye length

We now linearize V′(ϕ) at ϕ = 0 to obtain a modified Debye length. We first define $c_i^{\infty }=B_i(0)$ for any i ∈ J. Note that

$${\upsilon }_0{c}_0^{\infty }=1-\sum _{i\in J,i\ne 0}{\upsilon }_i{c}_i^{\infty }.$$ (3.16)

By (3.10) with ϕ = 0 and η_i= e^βμi, we also have that

$${\left({\upsilon }_0{c}_0^{\infty }\right)}^{{\upsilon }_i/{\upsilon }_0}={\eta }_i^{-1}{\upsilon }_i{c}_i^{\infty },i\in J.$$ (3.17)

We denote again γ = υ₀c₀ which depends on ϕ. We have for |ϕ| ≪ 1 that

$$\gamma ={\tau }_0+{\tau }_1\varphi +O\left({\varphi }^2\right),$$ (3.18)

where τ₀ and τ₁ are two constants to be determined. Using our notation, (1.1) becomes

$${\upsilon }_0{c}_0=1-\sum _{i\in J,i\ne 0}{\upsilon }_i{c}_i.$$ (3.19)

This and (3.16) imply that

$${\tau }_0={\upsilon }_0{c}_0(0)=1-\sum _{i\in J,i\ne 0}{\upsilon }_i{c}_i^{\infty }={\upsilon }_0{c}_0^{\infty }.$$ (3.20)

Substituting (3.18) into (3.12) for a fixed i ∈ J with i ≠ 0, we obtain by (3.20) and (3.17) that

$$\begin{array}{lll}{c}_i\hfill & =\hfill & \frac{{\eta }_i}{{\upsilon }_i}{({\tau }_0+{\tau }_1\varphi +O({\varphi }^2))}^{{\upsilon }_i/{\upsilon }_0}(1-\beta q_i\varphi +O({\varphi }^2))\hfill \\ \hfill & =\hfill & \frac{{\eta }_i}{{\upsilon }_i}{\tau }_0^{{\upsilon }_i/{\upsilon }_0}{\left(1+\frac{{\tau }_1}{{\tau }_0}\varphi +O({\varphi }^2)\right)}^{{\upsilon }_i/{\upsilon }_0}(1-\beta q_i\varphi +O({\varphi }^2))\hfill \\ \hfill & =\hfill & c_i^{\infty }\left(1+\frac{{\upsilon }_i{\tau }_1}{{\upsilon }_0{\tau }_0}\varphi +O({\varphi }^2)\right)(1-\beta q_i\varphi +O({\varphi }^2))\hfill \\ \hfill & =\hfill & c_i^{\infty }+c_i^{\infty }\left(\frac{{\upsilon }_i{\tau }_1}{{\upsilon }_0{\tau }_0}-\beta q_i\right)\varphi +O({\varphi }^2).\hfill \end{array}$$ (3.21)

It now follows from (3.18), (3.19), (3.21), and (3.20) that

$${\tau }_0+{\tau }_1\varphi +O({\varphi }^2)=1-\sum _{i\in J,i\ne 0}{\upsilon }_i{c}_i^{\infty }-\sum _{i\in J,i\ne 0}{\upsilon }_i{c}_i^{\infty }\left(\frac{{\upsilon }_i{\tau }_1}{{\upsilon }_0^2{c}_0^{\infty }}-\beta q_i\right)\varphi +O({\varphi }^2).$$

Comparing the leading-order terms, we obtain exactly (3.20). Comparing the O (ϕ)-terms and noting that z₀= 0, we obtain

$${\tau }_1=\frac{\beta {\upsilon }_0^2{c}_0^{\infty }{\sum }_{i\in J}{q}_i{\upsilon }_i{c}_i^{\infty }}{{\sum }_{i\in J}{\upsilon }_i^2{c}_i^{\infty }}.$$

This and (3.21) then lead to

$$c_i=c_i^{\infty }+\beta c_i^{\infty }\left(\frac{{\upsilon }_i{\sum }_{j\in J}{q}_j{\upsilon }_j{c}_j^{\infty }}{{\sum }_{j\in J}{\upsilon }_j^2{c}_j^{\infty }}-q_i\right)\varphi +O({\varphi }^2)\forall i\in J\text{with}i\ne 0.$$

Consequently,

$$V^{\prime }(\varphi )=-\sum _{i\in J}{q}_i{c}_i=-\sum _{i\in J}{q}_i{c}_i^{\infty }-\beta \left[\frac{{\left({\sum }_{i\in J}{q}_i{\upsilon }_i{c}_i^{\infty }\right)}^2}{{\sum }_{i\in J}{\upsilon }_i^2{c}_i^{\infty }}-\sum _{i\in J}{q}_i^2{c}_i^{\infty }\right]\varphi +O({\varphi }^2).$$

In the small |ϕ| approximation, we then obtain by (3.3) and the shift ϕ = ψ − ψ_D/2 the linearized PBE with ionic size effects

$$\nabla \cdot \varepsilon \nabla \psi -\varepsilon {\widehat{\lambda }}_D^{-2}\psi =-\sum _{i\in J}{q}_i{c}_i^{\infty }-\frac{1}{2}\varepsilon {\widehat{\lambda }}_D^{-2}{\psi }_D,$$ (3.22)

where λ̂_D > 0 is defined by

$${\widehat{\lambda }}_D^{-2}=\frac{\beta }{\varepsilon }\left[\sum _{i\in J}{q}_i^2{c}_i^{\infty }-\frac{{\left({\sum }_{i\in J}{q}_i{\upsilon }_i{c}_i^{\infty }\right)}^2}{{\sum }_{i\in J}{\upsilon }_i^2{c}_i^{\infty }}\right].$$ (3.23)

The fact that the right-hand side of the above equation is nonnegative follows from the Cauchy-Schwarz inequality

$${\left(\sum _{i\in J}{q}_i{\upsilon }_i{c}_i^{\infty }\right)}^2\le \left(\sum _{i\in J}{q}_i^2{c}_i^{\infty }\right)\left(\sum _{i\in J}{\upsilon }_i^2{c}_i^{\infty }\right).$$

In the case that ɛ is a constant, ψ_D = 0 on Γ_D, and ${\sum }_{i\in J}{q}_i{c}_i^{\infty }=0$ which is the charge neutrality, the linearized equation (3.22) simplifies to the familiar equation

$$\mathrm{\Delta }\psi -{\widehat{\lambda }}_D^{-2}\psi =0.$$

We call λ̂_D the size-modified Debye length. Recall that the Debye length λ_D > 0 in the classical Debye–Hückel theory is defined by

$${\lambda }_D^{-2}=\frac{\beta }{\varepsilon }\sum _{i\in J}{q}_i^2{c}_i^{\infty }.$$ (3.24)

It is clear that λ̂_D > λ_D. This indicates that finite ionic sizes lead to a longer Debye screening length.

4 A Charged Spherical Molecule: Basic Properties

In this section, we consider a charged spherical molecule, centered at the origin and with radius R₀ > 0, immersed in a solvent that occupies the region defined by R₀< r < R_∞ in the spherical coordinates for some R_∞ > R₀, where R_∞ can be finite or infinite. Corresponding to the general setting described in the previous section, we have in this case that

$$\begin{array}{l}\mathrm{\Omega }=\{x\in {ℝ}^3:R_0<\left|x\right|<R_{\infty }\},\\ {\mathrm{\Gamma }}_{\mathrm{N}}=\{x\in {ℝ}^3:\left|x\right|R_0\},\\ {\mathrm{\Gamma }}_{\mathrm{D}}=\{\begin{array}{ll}\{x\in {ℝ}^3:\left|x\right|=R_{\infty }\}\hfill & \text{if}{R}_{\infty }<\infty ,\hfill \\ \{\infty \}\hfill & \text{if}{R}_{\infty }=\infty .\hfill \end{array}\end{array}$$

We consider the boundary-value problem of the PBE, classical and size-modified, for the electrostatic potential ψ (cf. (2.2) and (3.3)):

$$\{\begin{array}{ll}\varepsilon \mathrm{\Delta }\psi =V^{\prime }(\psi )\hfill & \text{in}\mathrm{\Omega },\hfill \\ \varepsilon \frac{\partial \psi }{\partial n}=\sigma \hfill & \text{on}{\mathrm{\Gamma }}_{\mathrm{N}},\hfill \\ \psi =0\hfill & \text{on}{\mathrm{\Gamma }}_{\mathrm{D}}.\hfill \end{array}$$ (4.1)

If ψ = ψ(r)(r = |x|) is radially symmetric, then this system is the same as

$$\{\begin{array}{ll}\varepsilon \left({\psi }^{″}+\frac{2}{r}{\psi }^{\prime }\right)=V^{\prime }(\psi )\hfill & \text{if}{R}_0<r<R_{\infty },\hfill \\ {\psi }^{\prime }(R_0)=-\frac{\sigma }{\varepsilon },\hfill \\ \psi (R_{\infty })=0.\hfill \end{array}$$ (4.2)

Here, we assume both the dielectric coefficient ɛ > 0 and surface charge density σ are constants. We can consider the inhomogeneous Dirichlet boundary condition ψ = ψ_∞ on Γ_D for a constant ψ_∞ by replacing ψ by ψ − ψ_∞. We assume that V : ℝ → ℝ is smooth, V″ > 0 in R, and inf_s∈ℝV(s)= 0. We then have one and only one of the three cases covered in Theorem 3.3: V′ > 0 in ℝ, V′ < 0 in ℝ, or there exists a unique ϕ₀ such that V (s)> V (ϕ₀) = 0 for any s ≠ 0. In the last case, V′ < 0 in (−∞,ϕ₀), V′(ϕ₀) = 0, and V′ > 0 in (ϕ₀, ∞). The term −V′(ψ) is the ionic charge density that is related to M ionic species with valences and volumes satisfying (3.4).

For the classical PBE, we have $V(\varphi )={\sum }_{i\in J}{c}_i^{\infty }(e^{-\beta qi\varphi }-1)$, where $c_i^{\infty }(i\in J,i\ne 0)$ are bulk ionic concentrations. Under the assumption on the charge neutrality ${\sum }_{i\in J}{q}_i{c}_i^{\infty }=0,$ this V satisfies the properties in the third case in Theorem 3.3 with ϕ₀ = 0. So our analysis covers this case.

We first consider the case that R_∞< ∞.

Theorem 4.1

Let ɛ > 0, σ ∈ ℝ, and 0 < R₀< R_∞< ∞. Assume that V : ℝ → ℝ is smooth, V″ > 0 in ℝ, and inf_s∈ℝ V(s) = 0. Then the boundary-value problem (4.1) has a unique weak solution. Moreover it is radially symmetric and smooth on Ω̄.

Proof

We assume that σ ≠ 0. The case that σ = 0 can be proved similarly. We denote H = {ϕ ∈ H¹(R₀, R_∞) : ϕ(R_∞) = 0} and define I:H→ (−∞, ∞] by

$$I[\varphi ]=4\pi {\int }_{R_0}^{R_{\infty }}\left[\frac{\varepsilon }{2}{\varphi }^{\prime }{(r)}^2+V(\varphi )\right]r^2\mathit{\text{dr}}-4\pi R_0^2\sigma \varphi (R_0)\forall \varphi \in H.$$ (4.3)

If ϕ ∈ H, then we have with δ = ɛ/(4σ) > 0 that

$$\left|\varphi (R_0)\right|=\left|{\int }_{R_0}^{R_{\infty }}{\varphi }^{\prime }(r)\mathit{\text{dr}}\right|\le \sqrt{R_{\infty }-R_0}{\left({\int }_{R_0}^{R_{\infty }}{\left|{\varphi }^{\prime }(r)\right|}^2\mathit{\text{dr}}\right)}^{1/2}\le \frac{R_{\infty }-R_0}{4\delta }+\delta {\int }_{R_0}^{R_{\infty }}{\left|{\varphi }^{\prime }(r)\right|}^2\mathit{\text{dr}},$$

leading to

$$\begin{array}{cc}I[\varphi ]\ge \pi \varepsilon R_0^2{\int }_{R_0}^{R_{\infty }}{\left|{\varphi }^{\prime }(r)\right|}^2\mathit{\text{dr}}-\frac{4\pi {\sigma }^2{R}_0^2(R_{\infty }-R_0)}{\varepsilon }& \forall \varphi \in H.\end{array}$$

Notice that by Poincare's inequality, I[ϕ] controls $\parallel \varphi {\parallel }_{H^1(R_0,R_{\infty })}^2$, up to an additive constant. By standard arguments of direct methods in the calculus of variations and by the fact that H¹(R₀,R_∞) can be compactly embedded into C([R₀, R_∞]), we obtain the existence of a minimizer ψ ∈ H of the functional I : H → [0,∞]. Moreover, the minimum value of I over H is finite. The uniqueness of this minimizer follows from the strict convexity of I. Clearly, the minimizer ψ = ψ(r) is a smooth solution to the corresponding Euler–Lagrange equation, the first equation in (4.2). Moreover, it satisfies the natural boundary condition ψ′(R₀) = −σ/ɛ. Since ψ ∈ H, ψ(R∞)= 0. If we also denote ψ(x)= ψ(|x|), then it is clear that this function is a radially symmetric solution to (4.1), and is smooth on Ω̄.

To prove the uniqueness of solution to (4.1), we first note that the solution ψ minimizes the functional

$$Q[\varphi ]={\int }_{\mathrm{\Omega }}\left[\frac{\varepsilon }{2}{\left|\nabla \varphi \right|}^2+V(\varphi )\right]\mathit{\text{dV}}-{\int }_{{\mathrm{\Gamma }}_{\mathrm{N}}}\sigma \varphi \mathit{\text{dS}}$$

over all ϕ ∈ H¹(Ω) such that ϕ = 0 on Γ_D. Indeed, for any η ∈ H¹(Ω) with η = 0 on Γ_D, we have by integration by parts, the convexity of V, and (4.1) that

$$\begin{array}{rr}Q[\psi +\eta ]-Q[\psi ]\hfill & ={\int }_{\mathrm{\Omega }}\left[\frac{\varepsilon }{2}{\left|\nabla \eta \right|}^2+\varepsilon \nabla \psi .\nabla \eta +V(\psi +\eta )-V(\psi )\right]\mathit{\text{dV}}-{\int }_{{\mathrm{\Gamma }}_{\mathrm{N}}}\sigma \eta \mathit{\text{dS}}\hfill \\ \hfill & \ge {\int }_{\mathrm{\Omega }}[\varepsilon \nabla \psi .\nabla \eta +V^{\prime }(\psi )\eta ]\mathit{\text{dV}}-{\int }_{{\mathrm{\Gamma }}_{\mathrm{N}}}\sigma \eta \mathit{\text{dS}}\hfill \\ \hfill & \ge {\int }_{\mathrm{\Omega }}[-\varepsilon \mathrm{\Delta }\psi +V^{\prime }(\psi )]\eta \mathit{\text{dV}}+{\int }_{{\mathrm{\Gamma }}_{\mathrm{N}}}\varepsilon \frac{\partial \psi }{\partial n}\eta \mathit{\text{dS}}-{\int }_{{\mathrm{\Gamma }}_{\mathrm{N}}}\sigma \eta \mathit{\text{dS}}\hfill \\ \hfill & =0.\hfill \end{array}$$ (4.4)

Now if ψ̂ is another solution to (4.1), which is not assumed to be radially symmetric, then both ψ and ψ̂ are minimizers of the functional Q. Since Q is strictly convex, it has at most one minimizer. Therefore, ψ̂ = ψ. Q.E.D.

We now study the behavior of the equilibrium potential ψ = ψ(|x|) that is the solution to the boundary-value problem (4.1). We denote by T(r) the total amount of ionic charges in the region {x ∈ Ω : R₀< |x| < r}:

$$\begin{array}{cc}T(r)=-4\pi {\int }_{R_0}^r{V}^{\prime }(\psi (s))s^2\mathit{\text{ds}}& \forall r\in [R_0,R_{\infty }].\end{array}$$ (4.5)

Then T_∞ := T(R_∞) represents the total amount of ionic charges in Ω. If we integrate both sides of Poisson's equation in (4.1), apply integration by parts, and use the boundary condition at r = R₀, then we have

$$T_{\infty }+4\pi R_0^2\sigma +4\pi R_{\infty }^2\varepsilon {\psi }^{\prime }(R_{\infty })=0.$$ (4.6)

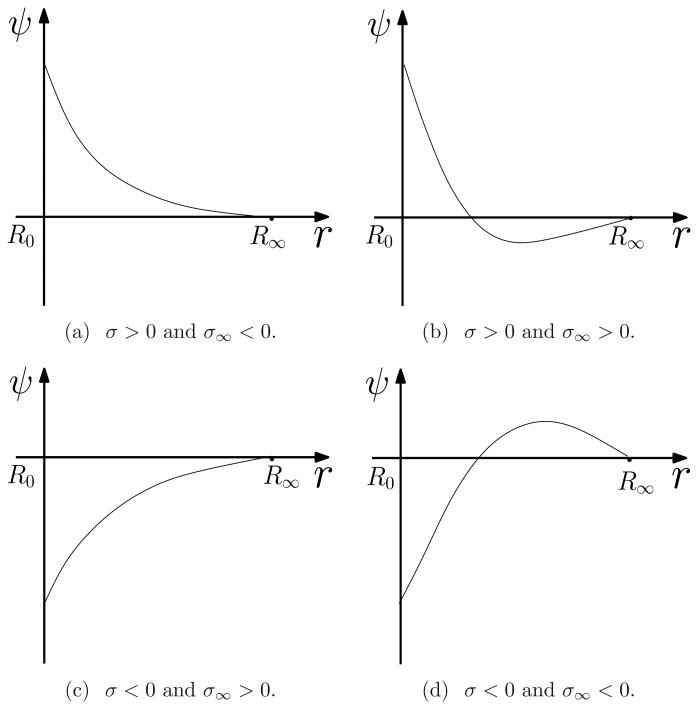
This is the charge neutrality of the system, as σ_∞ := ɛψ′(R_∞) can be viewed as the effective surface charge density on the part of the boundary Γ_D = {x ∈ ℝ³ : |x| = R_∞} which is set due to the finiteness of the underlying domain. The surface charge density σ in the boundary condition and the effective surface charge density σ_∞= ɛψ′(R_∞) are the key parameters that determine the monotonicity of the electrostatic potential ψ.

Theorem 4.2

Let ɛ > 0, σ ∈ ℝ, and 0 < R₀< R_∞ < ∞. Assume that V : ℝ → ℝ is smooth, V″ > 0 in ℝ, and inf_s∈ℝV(s)= 0. Consider V′ > 0 in ℝ, or V′ < 0 in ℝ, or V′(ϕ₀) = 0 for a unique ϕ₀∈ ℝ. Let ψ = ψ(|x|)be the solution to the boundary-value problem (4.1).

1. If σ > 0 and σ_∞> 0, then there exists a unique $R_c^{+}$∈ (R₀,R_∞) such that ψ′ < 0 in (R₀, $R_c^{+}$) and ψ′ > 0 in ( $R_c^{+}$,R_∞). If σ < 0 and σ_∞ < 0, then there exists a unique $R_c^{-}$∈ (R₀, R_∞) such that ψ′ > 0 in (R₀, $R_c^{-}$) and ψ′ < 0 in ( $R_c^{-}$,R_∞).

2. If σσ_∞ < 0 and at least one of σ and σ_∞ is nonzero, then ψ′ > 0 in (R₀, R_∞) if σ < 0 or σ_∞ > 0, and ψ′ < 0 in (R₀, R_∞) if σ > 0 or σ_∞ < 0.

3. The case that σ = σ_∞ = 0 can only occur when V′(0) = 0. In this case ψ = 0 identically in (R₀, R_∞).

The main results of Theorem 4.2 are illustrated in Figure 3. To prove the theorem, we first prove a lemma.

Lemma 4.1

Given the assumption as in Theorem 4.2 and A := {r ∈ (R₀,R_∞) : ψ′(r) = 0}.

1. If V′ > 0 in ℝ or V′ < 0 in ℝ, then either A = ∅ or A contains exactly one point.

2. If V′ (ϕ₀) = 0 at some unique ϕ₀ ∈ ℝ, then either A = ∅, or A contains exactly one point, or A = (R₀,R_∞) in which case we have additionally that ϕ₀ = 0 and ψ = 0 identically in (R₀, R_∞).

Proof

Suppose there exist r₁, r₂ ∈ ℝ such that R₀ ≤ r₁ < r₂ ≤ R_∞ and ψ′(r₁) = ψ′(r₂) = 0. Denote ω = {x ∈ ℝ³ : r₁ < |x| < r₂}. Then, by the same argument used in proving Theorem 4.1 (cf. (4.4)) and the strict convexity of V, the restriction of ψ onto ω is the unique minimizer among all ϕ ∈ H¹ (ω) of the functional

$$G[\varphi ]={\int }_{\omega }\left[\frac{\varepsilon }{2}{\left|\nabla \varphi \right|}^2+V(\varphi )\right]\mathit{\text{dV}}.$$ (4.7)

1. Suppose V′ > 0. Since inf_s∈ℝV (s)= 0, we have V (−∞) = 0. Thus, with ϕ_k = −k (k = 1,2,…), we have 0 ≤ G[ψ] ≤ G[ϕ_k] → 0 as k → ∞. Thus G[ψ] = 0. But this is impossible: If the integral of |∇ψ|² vanishes, then ψ is a constant. But V (s) > 0 for any s ∈ ℝ. Thus the integral of V (ψ) over ω is positive, a contradiction to G[ψ] = 0. For this case and similarly the case that V′ < 0, the set A can then contain at most one point.

2. In this case, V (ϕ₀) = min_{s ∈ℝ}V (s)= 0. Thus, the constant function ϕ = ϕ₀ also minimizes G over H¹(ω). By the uniqueness of minimizer which follows from the strict convexity of G, ψ = ϕ₀ in [r₁, r₂] and [r₁, r₂] ⊆A. Let s = inf A > R₀ and t = sup A < R_∞. At any point in (s, t), ψ = ϕ₀ and ψ′ vanishes. Moreover, ψ′ ≠ 0 in (R₀, s) if s > R₀ and in (t, R_∞) if t < R_∞. We prove now s = R₀ and t = R_∞. Assume s > R₀ and ψ′ > 0 in (R₀, s). Differentiating both sides of δψ = V′(ψ)/ɛ in R₀ < r < t, we obtain

   $${\psi }^{‴}(r)+\frac{2}{r}{\psi }^{″}(r)=\left[\frac{1}{\varepsilon }{V}^{″}(\psi (r))+\frac{2}{r^2}\right]{\psi }^{\prime }(r)\forall r\in (R_0,t).$$ (4.8)

   Hence, setting u = ψ′, we have Lu := Δu + c(r)u = 0 for R₀< r < t, where c(r) := − [(1/ɛ)V″(ψ(r)) + 2/r²] < 0 and c(r) is bounded in (R₀,s). Clearly u(s) = 0 ≤ u(r) for all r ∈ (R₀,t). Hence u attains its minimum with the minimum value 0 at any interior point x with |x| = s in {x ∈ ℝ³: R₀< |x| < t}. By the Strong Maximum Principle (cf. Theorem 3.5 of [13]), u must be a constant in R₀< r < t. This contradicts the fact that u > 0 in R₀< r < s and u = 0 in s < r < t. Hence ψ′ > 0 in (R₀,s) is impossible. Similarly, ψ′ < 0 in (R₀,s) is impossible. Therefore s =R₀. Similarly, we have t = R_∞. Finally A = (s,t) = (R₀,R_∞), and since ψ(R_∞)= 0, ψ = ϕ₀= 0 in (R₀, R_∞). Q.E.D.

Proof of Theorem 4.2

1. Assume σ > 0 and σ_∞ > 0. Then ψ′(R₀)< 0 and ψ′(R_∞) > 0. Hence by Lemma 4.1 there exists a unique $R_c^{+}$ ∈ (R₀,R_∞) such that ${\psi }^{\prime }(R_c^{+})=0.$ Clearly, ψ′ < 0 in (R₀, $R_c^{+}$) and ψ′ > 0 in ( $R_c^{+}$, R_∞). The case that σ < 0 and σ_∞ < 0 can be proved similarly.

2. Assume σ > 0 and σ_∞ ≤ 0, i.e., ψ′(R₀) < 0 and ψ′(R_∞) ≤ 0. The other cases can be treated similarly. It suffices to show that ψ′ does not vanish in (R₀,R_∞). Assume there existed r₀∈(R₀,R_∞) such that ψ′(r₀) = 0. By Lemma 4.1, ψ′ does not vanish at other points. Thus there are two cases. Case 1: ψ′ < 0 in (R₀,r₀) and ψ′ < 0 in (r₀,R_∞). Case 2: ψ′ < 0 in (R₀, r₀) and ψ′ > 0 in (r₀, R_∞). We show that each case is impossible.

   Consider Case 1. If V′ > 0 in ℝ, then Δψ > 0 on {x ∈ ℝ³ : r₀ < |x| < R_∞}. Let x₀∈ ℝ³ be such that |x₀| = r₀. Since ψ(|x₀|)> ψ(|x|) for any x with |x| ∈(r₀,R_∞). Lemma 3.4 in [13] then implies that ψ′(r₀) ≠ 0, a contradiction. If V′ < 0, we obtain the same contradiction ψ′(r₀) ≠ 0 by considering (R₀,r₀). Finally, assume V′(ϕ₀) = 0. If ψ(r₀) ≠ ϕ₀, then since ψ′(r₀) = 0, ψ″(r₀) = V′(ϕ(r₀))/ɛ ≠ 0. These imply that ψ′ changes its sign at r₀ which is a contradiction in Case 1. Thus Case 1 is impossible.

   Consider Case 2. In this case, we must have ψ′(R_∞)= 0, since ψ′(R_∞) > 0 would lead to ψ′(r₁) = 0 for some r₁∈(r₀, R_∞) which is impossible. Moreover, ψ reaches its minimum at r₀ and in particular ψ″(r₀) > 0. If V′ < 0 then Δψ < 0 in Ω. The Strong Maximum Principle then implies that ψ must be a constant for r ∈ (R₀, R_∞). This is impossible as ψ′(R₀) < 0. If V′ > 0, then Δψ > 0 and ψ(R_∞) = 0 > ψ(r) for r ∈ (r₀, R_∞). By Lemma 3.4 in [13], we must have ψ′(R_∞) > 0, leading to a contradiction. Assume V′(ϕ₀) = 0 at some unique ϕ₀. We cannot have ϕ₀ > ψ(r₀) as this would imply that V′(ψ(r₀)) < 0 and hence ψ″(r₀) < 0 which contradicts ψ″(r₀) > 0. We cannot have ϕ₀ ≤ ψ(r₀) neither, since otherwise we would have that V′(ψ) ≥ 0 and hence Δψ ≥ 0 on the region r₀< r < R_∞. But ψ(R_∞) = 0 > ψ(r) for any r ∈ (r₀, R_∞). Lemma 3.4 in [13] then implies ψ′(R_∞) > 0, a contradiction. Thus Case 2 is also impossible.

3. We have now ψ′(R₀)= 0 and ψ′(R_∞) = 0. As in the proof of Lemma 4.1 with r₁ = R₀ and r₂= R_∞,ψ is a constant in [R₀, R_∞]. Since ψ(R_∞) = 0, ψ = 0 identically in [R₀, R_∞]. Thus V′(0) = V′(ψ) = ɛΔψ = 0. Hence ϕ₀ = 0. Q.E.D.

   We recall that for any r ∈ (R₀, R_∞), T(r) is the total amount of ionic charges in the region {x ∈ Ω : R₀ < |x| < r}; cf. (4.5). In the following corollary, we consider a special and useful case but deliver more results:

Corollary 4.1

Let ɛ > 0, σ ∈ ℝ, and 0 < R₀< R_∞< ∞. Assume that V : ℝ → ℝ is smooth, V″ > 0in ℝ, and V (s) > V(0) = 0 for any s ∈ ℝ with s ≠ 0. Let ψ = ψ(|x|) be the solution to the boundary-value problem (4.1).

1. If σ = 0, then ψ = 0 and T = 0 identically.

2. If σ > 0, then ψ′ < 0, ψ″ > 0, and T′ < 0 in (R₀,R_∞).

3. If σ < 0, then ψ′ > 0, ψ″ < 0, and T′ > 0 in (R₀,R_∞).

Proof

1. It is easy to verify that in this case ψ = 0 is the unique solution to (4.1). Moreover, T′(r) = −V′(ψ(r)) = −V′(0) = 0 and T(R₀) = 0. Hence T = 0 in (R₀, R_∞).

2. Assume σ > 0. We then have σ_∞ = ɛψ′(R_∞) ≤ 0. Otherwise, σ_∞> 0. Then by Theorem 4.2 there exists R_c ∈ (R₀,R_∞) such that ψ′ < 0 in (R₀,R_c) and ψ′ > 0 in (R_c, R_∞). We have ψ(R_c)< min(ψ(R₀),ψ(R_∞)) < 0. Choose τ ∈ ℝ such that ψ(R_c) < τ < min(ψ(R₀),ψ(R_∞)). Define ψ̂ by ψ̂ = ψ if ψ ≥ τ and ψ̂ = τ if ψ < τ. Then I [ψ̂] < I [ψ], where I [·] is defined in (4.3). This is impossible as ψ minimizes I [·] over H = {ϕ ∈ H¹(R₀, R_∞) : ϕ(R_∞) = 0} that includes ψ̂. Now, since σ > 0 and σ_∞< 0, we have ψ′ < 0 by Theorem 4.2. Since ψ(R_∞) = 0, ψ > 0 in (R₀, R_∞). Hence V′(ψ) > 0 in (R₀, R_∞). Consequently,

   $$\begin{array}{cc}{\psi }^{″}(r)=-\frac{2}{r}{\psi }^{\prime }(r)+\frac{1}{\varepsilon }{V}^{\prime }(\psi (r))>0& \text{in}(R_0,R_{\infty }).\end{array}$$

   Since V′(ψ) > 0 in (R₀, R_∞), we also have T′(r)< 0 for all r ∈ (R₀, R_∞).

3. This can be proved similarly. Q.E.D.

   We now consider the case R_∞= ∞.

Theorem 4.3

Let ɛ > 0, σ ∈ ℝ, and R_∞ = ∞.

1. Suppose V : ℝ → ℝ is smooth and V′(0) ≠ 0. Then there is no solution to the boundary-value problem (4.1).

2. Suppose V : ℝ → ℝ is smooth, V″ > 0 on ℝ,V (−∞) = ∞ and V (∞) = ∞, and V (0) = inf_s∈ℝV (s) = 0. Then there exists a unique solution to the boundary-value problem (4.1). Moreover, this solution ψ = ψ (|x|)is radially symmetric and smooth on Ω̄, ψ′ < 0 and ψ″ > 0 in r = |x| if σ > 0, ψ′ > 0 and ψ″ < 0 in r = |x| if σ > 0, and ψ = 0 for all r if σ = 0.

We remark that in general all charges in an ionic system are in a finite region. Therefore it is natural to impose the far-field condition ψ(R_∞) = 0. The properties on V assumed in part (2) of the theorem are exactly those covered in the third case in Theorem 3.3 but with the minimum of V attained exactly at 0. The theorem states that the solution to (4.1) exists only when V′(0) = 0. This is precisely the condition of charge neutrality in the bulk. Therefore, for the three cases of V stated in Theorem 3.3, only the third case with V′(0) = 0 permits a solution to (4.1).

Proof of Theorem 4.3

1. (1) Assume V′(0) > 0. (The case that V′(0) < 0 is similar.) Assume ψ is a solution to (4.1), which is not assumed to be radially symmetric. Clearly, ψ is smooth on Ω̄. Since ψ(∞) = 0 and V′(0) > 0, there exist A ≥ R₀ and α > 0 such that ɛΔψ = V′(ψ) ≥ α for all x ∈ Ω with |x| ≥ A. In the spherical coordinates (r,θ, φ) (r ≥ 0, 0 ≤ θ ≤ π, 0 ≤ φ < 2π), ψ = ψ(r, θ, φ), and

   $$\begin{array}{cc}\mathrm{\Delta }\psi =\frac{1}{r^2}\frac{\partial }{\partial r}\left(r^2\frac{\partial \widehat{\psi }}{\partial r}\right)+\frac{1}{r^{2}sin\theta }\frac{\partial }{\partial \theta }\left(sin\theta \frac{\partial \widehat{\psi }}{\partial \theta }\right)+\frac{1}{r^2{sin}^2\theta }\frac{{\partial }^2\widehat{\psi }}{\partial {\phi }^2}\ge \alpha & \forall r\ge A.\end{array}$$ (4.9)

   Define

   $$\begin{array}{cc}u(r)=\frac{1}{4\pi }{\int }_0^{2\pi }{\int }_0^{\pi }sin\theta \widehat{\psi }(r,\theta ,\phi )d\theta d\phi & \forall r>R_0.\end{array}$$

   Clearly, u(∞)= 0, since ψ̂ → 0 as r → ∞. Multiplying both sides of the inequality in (4.9) by sinθ/(4π), and then integrating the resulting sides over θ ∈ [0,π] and ϕ ∈ [0,2π), we obtain (1/r²) (r²u′(r))′ ≥ α for all r ≥ A. This leads to

   $$\begin{array}{cc}{u}^{\prime }(r)\ge \frac{1}{3}\alpha r+\left[A^2{u}^{\prime }(A)-\frac{1}{3}\alpha A^3\right]\frac{1}{r^2}& \forall r\ge A.\end{array}$$

   With one more time of integration, we get

   $$\begin{array}{cc}u(r)\ge u(A)+\frac{1}{6}\alpha (r^2-A^2)-\left[A^2{u}^{\prime }(A)-\frac{1}{3}\alpha A^3\right]\left(\frac{1}{r}-\frac{1}{A}\right)& \forall r\ge A.\end{array}$$

   This contradicts u(∞)= 0. Part (1) is proved.

2. (2) We first show the uniqueness. Suppose ψ₁ and ψ₂ are both solutions to (4.1). Clearly they are smooth on Ω̄ = {x ∈ ℝ³ : |x| ≥ R₀}. Let η = ψ₁ − ψ₂. This function attains its maximum on Ω̄. Suppose P := max_x∈Ω̄η > 0. If there exists x₀∈ Ω = {x ∈ ℝ³ : |x| > R₀} such that η(x₀) = P > 0, then there exists an open ball B(x₀) ⊂ Ω centered at x₀ such that η > 0 on B(x₀). Since ψ₁ and ψ₂ are bounded on Ω̄, ψ₁(x), ψ₂(x) ∈ K for some compact subset of ℝ for all x ∈ Ω ¯. Setting γ₀ = min_s∈K V″(s) > 0, we obtain

   $$\begin{array}{cc}\varepsilon \mathrm{\Delta }\eta =V^{\prime }({\psi }_1)-V^{\prime }({\psi }_2)\ge {\gamma }_0\eta >0& \text{in}B(x_0)\end{array}.$$ (4.10)

   Since η(x₀) = max_x∈B(xo)η > 0, the Strong Maximum Principle (cf. Theorem 3.5 of [13]) then implies that η is a constant in B(x₀). Consequently Δη = 0 in B(x₀), and (4.10) implies η = 0 in B(x₀), contradicting to η(x₀) > 0. Thus there exists y₀ ∈ ∂Ω with |y₀| = R₀ such that η(y₀) = P > η(x) for all x ∈ Ω. But this and (4.10) imply ∂_nη(y₀) = 0 by Lemma 3.4 in [13], contradicting ∂_nη = 0 on Γ_D = {x ∈ ℝ³ : |x| = R₀}. Hence η ≤ 0 and ψ₁ ≤ ψ₂ in Ω. Similarly ψ₂≤ ψ₁ in Ω. Thus ψ₁ = ψ₂ in Ω.

   We now prove the existence of solution to (4.1) that satisfies all the properties. If σ = 0 then ψ = 0 in Ω is the desired solution. Assume σ > 0. (The case σ < 0 is similar.) Choose a sequence R_k ∈ ℝ (k = 1, 2,…) such that R₀< R₁< R₂< · · · < R_k< · · · and R_k → ∞ as k → ∞. For each integer k ≥ 1, let ψ_k = ψ_k(|x)|) be the unique solution to the boundary-value problem

   $$\{\begin{array}{ll}\varepsilon \mathrm{\Delta }{\psi }_k=V^{\prime }({\psi }_k)\hfill & \text{in}{\mathrm{\Omega }}^k=\{x\in {ℝ}^3:R_0<\left|x\right|<R_k\},\hfill \\ \varepsilon \frac{\partial {\psi }_k}{\partial n}=\sigma \hfill & \text{on}{\mathrm{\Gamma }}_{\mathrm{N}}^k=\{x\in {ℝ}^3:\left|x\right|=R_0\},\hfill \\ {\psi }_k=0\hfill & \text{on}{\mathrm{\Gamma }}_{\mathrm{D}}^k=\{x\in {ℝ}^{:}\left|x\right|=R_k\}.\hfill \end{array}$$ (4.11)

   By Corollary 4.1, ψ_k > 0, ψ′_k < 0, and ψ″_k > 0 in (R₀, R_k).

   Using the spherical coordinates, we have by the first equation in (4.11) that

   $$\begin{array}{cc}{\psi }_k^{″}(r)+\frac{2}{r}{\psi }_k^{\prime }(r)=V^{\prime }({\psi }_k(r))& \text{in}(R_0,R_k).\end{array}$$ (4.12)

   Multiply both sides of this equation by ${\psi }_k^{\prime }(r)$ and integrate the resulting sides over [R₀, R_k]. Noting that ${\psi }_k^{\prime }{\psi }_k^{″}=(1/2){({{\psi }^{\prime }}_k^2)}^{\prime }$and ${{\psi }^{\prime }}_k{V}^{\prime }\left({\psi }_k\right)=(d/\mathit{\text{dr}})V\left({\psi }_k\right)$, we then obtain by ${\psi }_k^{\prime }\left(R_0\right)=-\sigma /\varepsilon ,{\psi }_k\left(R_k\right)=0$ and V(0) = 0 that

   $$\frac{1}{2}\left[{({{\psi }^{\prime }}_k(R_k))}^2-{\left(\frac{\sigma }{\varepsilon }\right)}^2\right]+{\int }_{R_0}^{R_k}\frac{2}{r}{({{\psi }^{\prime }}_k(r))}^2\mathit{\text{dr}}=-V({\psi }_k(R_0)).$$

   Thus, since V (s) > 0 for any s > 0, we have 0 ≤ V (ψ_k(r)) ≤ σ²/(2ɛ²) for all r ∈ [R₀, R_k] and k ≥ 1. Since V is positive and strictly increasing in (0,∞) and V (∞) = ∞, and since ψ_k(R₀) ≥ ψ_k(r) for all r ∈ [R₀, R_k], we have by setting ϕ_c > 0 with V (ϕ_c)= σ²/(2ɛ²) that 0 ≤ ψ_k(r)≤ ϕ_cfor all r ∈ [R₀,R_k] and all k ≥ 1. Moreover, since ${\psi }_k^{″}>0$ in $(R_0,R_k),-\sigma /\varepsilon ={\psi }_k^{\prime }(R_0)\le {\psi }_k^{\prime }(r)\le {\psi }_k^{\prime }(R_{\infty })\le 0$ 0 for all r ∈ [R₀, R_k] and all k ≥ 1. Define ψ_k = 0 in (R_k, ∞) (k = 1, 2,…). Then the sequence ${\left\{{\psi }_k\right\}}_{k=1}^{\infty }$ is bounded in W^1,∞(R₀, ∞).

   There now exists a subsequence ${\left\{{\psi }_{k,1}\right\}}_{k=1}^{\infty }$ of ${\left\{{\psi }_k\right\}}_{k=1}^{\infty }$ such that ψ_k,1 → ψ in H¹(R₀, R₁) as k → ∞ for some ψ ∈ H¹(R₀, R₁). There exists a subsequence ${\left\{{\psi }_{k,2}\right\}}_{k=1}^{\infty }$ of ${\left\{{\psi }_{k,1}\right\}}_{k=1}^{\infty }$ such that ψ_k,2 → ϕ in H¹(R₀, R₂) as k → ∞ for some ϕ ∈ H¹(R₀, R₂) with ϕ = ψ on (R₀, R₁). We define ψ = ϕ in (R₁, R₂) so that ψ ∈ H¹(R₀, R₂). Repeating, we obtain ψ ∈ C([R₀, ∞)) with ψ ∈ H¹(R₀, R) for any R > R₀and subsequences ${\left\{{\psi }_{k,j}\right\}}_{k=1}^{\infty }$of ${\left\{{\psi }_k\right\}}_{k=1}^{\infty }$(j = 1,2,…), where for each j ≥ 1, the sequence ${\left\{{\psi }_{k,j+1}\right\}}_{k=1}^{\infty }$ is a subsequence of ${\left\{{\psi }_{k,j}\right\}}_{k=1}^{\infty }$. Moreover, ψ_k,j → ψ in H¹(R₀, R_j) as k ∞. Now ${\left\{{\psi }_{k,k}\right\}}_{k=1}^{\infty }$ is a subsequence of ${\left\{{\psi }_k\right\}}_{k=1}^{\infty }$, and ${\left\{{\psi }_{k,k}\right\}}_{k=1}^{\infty }$ converges to ψ in H¹(R₀, R), and hence also in C([R₀, R]), for any R > R₀.

   The limit ψ = ψ(|x|) is clearly radially symmetric. It is a (weak and hence strong) solution to the first two equations in (4.1). We show now that lim_r→∞ ψ(r) = 0. For each k ≥ 1, define

   $${\eta }_k(r)=\{\begin{array}{ll}{\psi }_k(R_0){\left(\frac{1}{R_0}-\frac{1}{R_k}\right)}^{-1}\left(\frac{1}{r}-\frac{1}{R_k}\right)\hfill & \text{if}{R}_0\le r\le R_k,\hfill \\ 0\hfill & \text{if}r>R_k.\hfill \end{array}$$

   One easily verifies that η_k(|x|) satisfies ɛΔη_k= 0 in Ω^k(cf. (4.11) for the definition of Ω^k) and η_k = ψ_k on the boundary of Ω^k. Since ɛΔ(ψ_k − ηk) ≥ 0 in Ω^k, we thus have by the Maximum Principle that ψ_k ≤ ηk in Ω^k and hence in Ω = {x ∈ ℝ³ : |x| > R₀}. Denote ${\left\{{\eta }_{k,k}\right\}}_{k=1}^{\infty }$ the subsequence of ${\left\{{\eta }_k\right\}}_{k=1}^{\infty }$ that corresponds to ${\left\{{\psi }_{k,k}\right\}}_{k=1}^{\infty }$. Then we have

   $$0\le \underset{r\to \infty }{\text{lim sup}}\psi (r)=\underset{r\to \infty }{\text{lim sup}}\underset{k\to \infty }{\text{lim}}{\psi }_{k,k}(r)\le \underset{r\to \infty }{\text{lim sup}}\underset{k\to \infty }{\text{lim}}{\eta }_{k,k}(r)=\underset{r\to \infty }{\text{lim sup}}\frac{R_0\psi (R_0)}{r}=0,$$

   implying lim_r→∞ ψ(r) = 0.

   Since ψ′_k ≤ 0 on (R₀, R_k) for all k ≥ 1 ψ′ ≤ 0 on (R₀, ∞). Let u(r)= ψ′(r). Then u(|x|) satisfies Δu−c(r)u = 0 on {x ∈ ℝ³ : |x| > R₀}, where c(r) = − [(1/ɛ)V″(ψ(r)) + 2/r²] < 0, cf. (4.8). Since u ≤ 0 in (R₀,∞), the Strong Maximum Principle implies that u ≠ 0 in (R₀,∞). Hence u = ψ′ < 0 in (R₀,∞). Since ψ ≥ 0 and V(ψ) ≥ 0, we also have ψ″(r) = −(2/r)ψ′ + V′(ψ) > 0 for all r > R₀. Q.E.D.

5 Numerical Results

We now present numerical results to illustrate our analysis. We consider minimizing numerically the free-energy functional (2.6) with (2.7) and (2.8). We chooseΩ = {x ∈ ℝ³ : R₀ < |x| < R_∞},Γ_N = {x ∈ ℝ³ : |x| = R₀},Γ_D = {x ∈ ℝ³ : |x| = R_∞},with R₀, R_∞ ∈ ℝ such that R₀ < R_∞. We assume the dielectric coefficient ɛ and surface charge density σ are both constants. We also set ψ_D = 0 on Γ_D. Note that our system is radially symmetric. We use the constrained optimization method developed in our previous work [38] to solve numerically our optimization problem.

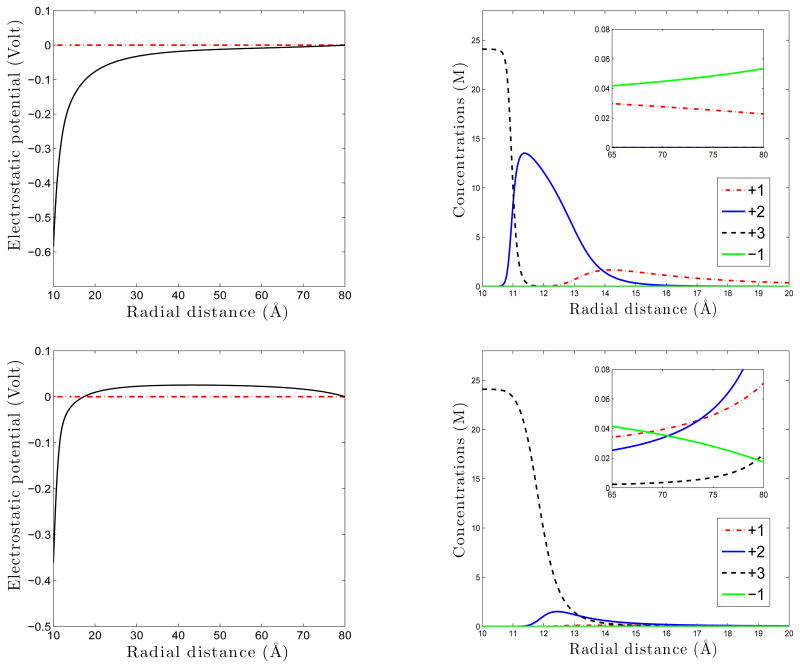
We set R₀ = 10 Å and R_∞ = 80 Å. We distribute uniformly some negative charges on the sphere r = R₀ with the surface charge density $\sigma =-150/(4{\pi }^2{R}_0^2)$ e/Ų. We consider three species of counterions K⁺, Ca²⁺, Al³⁺, and one species of coions Cl⁻in the solution that occupies Ω. So M = 4. We label these ionic species by 1, 2, 3, and 4, respectively. We label the solvent (water) by 0. The corresponding valences and ionic volumes are (z₁,z₂, z₃, z₄) = (+1,+2,+3,−1) and (υ₁,υ₂,υ₃,υ₄) = (5.51³, 4.75³, 4.1³, 6.37³) ų, respectively. The volume of a solvent (water) molecule is υ₀= 2.75³ ų. For these real ionic systems, it happens that α₄< α₀= 0 < α₁< α₂< α₃, where α_k = z_k/υ_k (0 ≤ k ≤ 4) with z₀= 0. We use a³ to approximate the volume of an ion of diameter a. The temperature is T = 300K. The dielectric coefficient is absorbed into the Bjerrum length l_B= e²/(4πɛk_BT). We set the Bjerrum length to be l_B= 7Å. We consider two cases of the numbers of ions in different species. Case I: (N₁,N₂, N₃, N₄) = (60, 30, 20, 50). By simple calculations, we have that in this case the effective surface charge density σ_∞ > 0. Case II: (N₁,N₂, N₃, N₄) = (50,50,50,50). In this case σ_∞ < 0.

In Figure 4, we plot our computational results of the electrostatic potential ψ and the equilibrium ionic concentrations. For Case I, the potential ψ is monotonically increasing. This is exactly predicted by Theorem 4.2, since in this case, σ < 0 and σ_∞ > 0. Notice that the concentration of +3, which has the largest ratio of valence-to-volume among all the species, monotonically deceases, while that of −1 monotonically increases. These are predicted by Theorem 3.2. For Case II, σ < 0 and σ_∞ > 0, and the behavior of the potential ψ is again as predicted by Theorem 4.2. We observe the stratification of the counterion concentrations in both cases.

6 Conclusions

We have analyzed a PB-like mean-filed model for electrostatic interactions of multivalent ions with finite ionic sizes in an ionic solution near a charged surface. Our work continues that presented in [22] but gives more details on how equilibrium ionic concentrations depend on the electrostatic potential and how the important parameters, the valence-to-volume ratios of ions, determine the properties of ionic concentrations near the charged surface.

Here we discuss some of our main results. First, our analysis shows that for a strong potential, the layering structure of counterion concentrations does depend on the valence-to-volume ratios. In general, the comparison of concentrations for different ionic species can be subtle. Instead of comparing c_i and c_j, one may need to compare (v_ic_i)^1/υi and (υ_jc_j)^1/υj. The difference of these quantities may only be observed for certain range of the potential. See part (2) of Theorem 3.2.

Second, we characterize the ionic charge density through the function V defined in (3.2); cf. also (3.15). We recall for the classical PB theory that the corresponding function V is defined by

$$V^{\prime }(\varphi )=-\sum _{i\in J,i\ne 0}{q}_i{c}_i^{\infty }{e}^{-\beta qi\varphi },$$

where $c_i^{\infty }$ (i ∈ J, i ≠ 0) are positive constants. An important difference here is that, when the ionic size effects are included, the function V grows linearly at the −∞ and ∞, rather than exponentially as in the classical PB theory.

Third, when the ionic size effects are included, the Debye length is then modified. In Table 1 and Table 2, we compare the size-modified Debye length λ̂_D defined in (3.23) with the classical Debye length λ_D defined in (3.24) for a few ionic systems. The difference between these two tables is that the diameters of ions listed in Table 1 do not, while those in Table 2, include the size of a hydration shell. It is clear that the modification of the Debye length due to the inclusion of the ionic size effect is not significant. This may suggest that a linearized system may not describe well the size effect. Clearly, further tests are needed on real ionic solutions and biomolecular systems.

Table 1.

A comparison of the Debye length λ_D and the ionic size-modified Debye length λ̂_D for a few systems of ionic solutions. The diameter of an ion does not include that of a water molecule (hydration shell). Concentrations mean bulk concentrations.

Ionic systems	Diameters of ions (Å)	Concentrations (M)	λD(Å)	λ̂D(Å)
Cl−, Na+	3.62, 2.04	0.1, 0.1	9.715	9.726
Cl−, K+	3.62, 2.76	0.1, 0.1	9.715	9.720
Cl−, Ca2+	3.62, 2.00	0.2, 0.1	5.609	5.618
Cl−, Na+, K+	3.62, 2.04, 2.76	0.2, 0.1, 0.1	6.870	6.880
Cl−, Na+, Ca2+	3.62, 2.04, 2.00	0.3, 0.1, 0.1	4.858	4.870
Cl−, Na+, K+, Ca2+	3.62, 2.04, 2.76, 2.00	0.4, 0.1, 0.1, 0.1	4.345	4.358
Cl−, K+, Ca2+, Al3+	3.62, 2.76, 2.00, 1.35	0.6, 0.1, 0.1, 0.1	3.072	3.085

Table 2.

A comparison of the Debye length λ_D and the ionic size-modified Debye length λ̂_D for a few systems of ionic solutions. The diameter of an ion includes that of a water molecule (hydration shell). Concentrations mean bulk concentrations.

Ionic systems	Diameters of ions (Å)	Concentrations (M)	λD(Å)	λ̂D(Å)
Cl−, Na+	6.37, 4.79	0.1, 0.1	9.715	9.847
Cl−, K+	6.37, 5.51	0.1, 0.1	9.715	9.763
Cl−, Ca2+	6.37, 4.75	0.2, 0.1	5.609	5.701
Cl−, Na+, K+	6.37, 4.79, 5.51	0.2, 0.1, 0.1	6.870	6.970
Cl−, Na+, Ca2+	6.37, 4.79, 4.75	0.3, 0.1, 0.1	4.858	4.974
Cl−, Na+, K+, Ca2+	6.37, 4.79, 5.51, 4.75	0.4, 0.1, 0.1, 0.1	4.345	4.449
Cl−, K+, Ca2+, Al3+	6.37, 5.51, 4.75, 4.10	0.6, 0.1, 0.1, 0.1	3.072	3.172

Finally, for a spherical charged molecule immersed in an ionic solution occupying a finite region, the qualitative behavior of the electrostatic potential—either it is monotonic or it changes the monotonicity only once in the entire range of the radial variable—is completely determined by the sign of two surface charge densities. One is the prescribed surface charge density and the other is the effective surface charge density. If the region of ionic solution is the entire complement of the sphere in ℝ³, then the ionic charge neutrality is necessary for the existence and uniqueness of the electrostatic potential that vanishes at infinity, governed by the PBE (classical or size-modified). Such a potential is always monotonic.

We conclude with two remarks on the mathematical model we have studied here. First, for the case of a uniform size for all ions and solvent molecules, the free-energy functional (2.1) can be derived from a lattice-gas model; cf. [20]. For a general and more interesting case where the sizes are different, such a derivation seems not available. It is therefore interesting to give a rigorous derivation of such a free-energy functional using the notion of equilibrium statistical mechanics. Second, we have assumed mainly that the region Ω of ionic solution is bounded. The case that Ω has an infinite volume is a tricky situation to define the functional F[c]; cf. (2.1) and (2.6). Usually, the concentration c_i is taken to be the bulk value $c_i^{\infty }(>0)$at infinity (or far away from the charged surface). Such a value can be experimentally determined. If Ω is unbounded with an infinite volume, then the integral of c_i over Ω will be just infinite if $c_i=c_i^{\infty }$ at infinity. The PBE, however, can be defined on an unbounded domain as the electrostatic potential ψ can exist in the entire space.