Distinguishing hair cell from neural potentials recorded at the round window

Abstract

Almost all patients who receive cochlear implants have some acoustic hearing prior to surgery. Electrocochleography (ECoG), or electrophysiological measures of cochlear response to sound, can identify remaining auditory nerve activity that is the basis for this residual hearing and can record potentials from hair cells that are no longer functionally connected to nerve fibers. The ECoG signal is therefore complex, being composed of both hair cell and neural signals. To identify signatures of different sources in the recorded potentials, we collected ECoG data across frequency and intensity from the round window of gerbils before and after treatment with kainic acid, a neurotoxin. Distortions in the recorded waveforms were produced by different sources over different ranges of frequency and intensity. In response to tones at low frequencies and low-to-moderate intensities, the major source of distortion was from neural phase-locking that was sensitive to kainic acid. At high intensities at all frequencies, the distortion was not sensitive to kainic acid and was consistent with asymmetric saturation of the hair cell transducer current. In addition to loss of phase-locking, changes in the envelope after kainic acid treatment indicate that sustained neural firing combines with receptor potentials from hair cells to produce the envelope of the response to tones. These results provide baseline data to interpret comparable recordings from human cochlear implant recipients.

almost all patients who receive cochlear implants have some acoustic hearing prior to surgery. This includes patients classed as profoundly deaf, as well as those with severe or only moderate hearing loss but poor speech understanding (Gifford et al. 2010). Electrocochleography (ECoG), or electrophysiological measures of cochlear response to sound, can identify remaining cochlear function that is the basis for this residual hearing (Choudhury et al. 2012; Fitzpatrick et al., in press; McMahon et al. 2008; Santarelli et al. 2008). In addition, ECoG can provide detailed information about spectral and temporal aspects of cochlear function that is not reflected in the audiogram. In the spectral domain, ECoG responses contain information about the magnitudes, frequency ranges, and thresholds of responses from cochlear hair cells and auditory nerve fibers. Cochlear responses traditionally considered to be from hair cells include the cochlear microphonic (CM) and summating potential (SP). The CM is produced from transducer currents through stereocilia and follows the fine structure of the stimulus (e.g., waveform of a tone). The SP results from sustained depolarization of the receptor potential within hair cells, primarily in response to high frequencies (Palmer and Russell 1986). Potentials produced by the auditory nerve include the compound action potential (CAP), the auditory nerve neurophonic (ANN), and a sustained response that is the neural correlate to the SP. The CAP is a specialized response to the onsets and offsets of sounds. These produce highly synchronized action potentials across large numbers of nerve fibers that produce a field response that also resembles the shape of an action potential. At low frequencies, the receptor potentials follow the fine structure with relatively little net depolarization. Action potentials in the nerve also phase-lock to the fine structure and have enough symmetry in phase to produce the ANN, or evoked potential correlate of neural phase-locking. The SP and the sustained firing of the auditory nerve can follow the envelope of the stimulus. Whether the sustained nerve firing contributes to the ECoG is not clear.

Although there are dozens of studies investigating the CM, SP, and CAP, the ANN has been examined in relatively few studies (examples include He et al. 2012; Henry 1995; Lichtenhan et al. 2013; Snyder and Schreiner 1984, 1987). This lack of information about neural vs. hair cell contributions to the ECoG is proving significant because ECoG is increasingly being used to monitor the damage caused by a cochlear implant electrode (Colletti et al. 2011; Radeloff et al. 2012) and to characterize the cochlear health of patients before implantation (Choudhury et al. 2012; Fitzpatrick et al., in press). In particular, the ANN and CM are both present in the ongoing response to tones and are difficult to cleanly separate. Overcoming this difficulty is one of the major motivations of the current study. To do this, we collected ECoG data across frequency and intensity from the round window of the gerbil. The round window is a popular site for ECoG recording in humans and animals because of its proximity to the internal cochlear environment. The data were collected from normal-hearing animals, and a neurotoxin (kainic acid, or KA) was used to separate hair cell from neural responses. The goal was to identify frequency ranges and intensities where hair cell and neural nonlinearities produce distortions in the ECoG, to identify markers that can be used to distinguish the contributions of each. The presence or absence of these markers could then be used to determine how pathological conditions have affected the cochlear environment.

Because the ANN has not been identified in most studies of the round window ECoG, the frequency and intensity ranges where hair cell or neural responses, either separately or together, produce distortions are not well defined. The two main sources of nonlinearities producing harmonic distortion are the transduction process in hair cells and the conversion to an action potential code in the auditory nerve. The expected distortions from these processes are shown in Fig. 1 at low, moderate, and high intensity. The transduction process in the stereocilia has the form of an asymmetric second-order Boltzmann function with earlier saturation to hyperpolarizing than to depolarizing motion of the stereocilia (e.g., Dallos 1986; Kros et al. 1992; Santos-Sacchi 1993). This pattern could be due to a greater proportion of closed than open channels when in the resting, or no sound, condition, the asymmetric starting position of the hair bundles, or other factors. For instance, the basilar membrane motion (Cooper 1998) and intracochlear pressure (Olson and Dong 2006) also have input-output functions in the shape of an asymmetric Boltzmann function, so these processes may contribute to distortions in the ECoG, as well. At a low intensity (Fig. 1, top), the transduction process is within its near-linear range so that the CM has a sinusoidal waveform and its spectrum has a single peak at the stimulus frequency. For the auditory nerve, the response at the round window is the unitary response to a single action potential convolved with the shape of the period histogram. The shape of an action potential (Prijs 1986) can be modeled as one cycle of a 1-kHz tone. The period histogram for a low-frequency tone is a rectified version of the signal because of phase-locking where the spike rate cannot go below zero (although it can go below the spontaneous rate). Rectification is asymmetric about the time axis, and when zero is the lower bound, the harmonics produced are predominantly even (Teich et al. 1989). Thus the spectrum of the ANN has most energy at the signal frequency and second harmonic. The details of the shape of the unitary response and cycle histogram matter as regards the harmonic distortion produced, as is further considered in the discussion. When the hair cell and auditory nerve responses are added together, the distortion in the ECoG at this low intensity is therefore expected to be primarily at the second harmonic, as well. At a moderate intensity, the asymmetry of the input-output function of hair cell transduction causes the response to saturate only in the hyperpolarizing direction, a form of rectification. The spectrum of a waveform where rectification is not at zero still emphasizes even harmonics, but unlike rectification at zero, there are odd harmonics, as well (Teich et al. 1989). The unitary response and synchrony are relatively constant with level and so were not shown again. Thus, at a moderate intensity, the distortion in the ECoG still emphasizes the second harmonic compared with the third. However, now the distortion is from both the CM and ANN, rather than just the ANN. At a high intensity, the transduction process saturates in both the hyperpolarizing and depolarizing directions. The expected waveform therefore begins to approximate a square wave, which, if symmetric around the time axis, would have only odd harmonics. Because the transduction process is not symmetric, there will still be even components. When the responses are added together to produce the ECoG, the second harmonic still contains both hair cell and nerve components, whereas the increased presence of a third harmonic is indicative of saturation of the transducer current in both directions of stereociliary movement.

Fig. 1.

Distortion from hair cell transduction and auditory nerve rectification in electrocochleography (ECoG) recordings. Schematic examples of distortion to the ongoing portion of a 500-Hz tone burst at low, moderate, and high intensities are provided. For each intensity, the hair cell transduction process is schematized as an asymmetric second-order Boltzmann function, with deflection (Defl) on the x-axis and current (Curr) on the y-axis (Chertoff et al. 2003; Corey and Hudspeth 1983; Hudspeth and Corey 1977; Santos-Sacchi 1993; Sirjani et al. 2004). The position on this input-output function for different intensities is shown by the thick line. The evoked potential correlate of these currents is the cochlear microphonic (CM). At the low intensity, the time waveform of the CM is a sinusoid and the spectrum is a single peak at the stimulus frequency. At the moderate intensity, the hair cell saturates only in the hyperpolarizing direction (negative currents), producing distortion primarily at the second harmonic (1,000 Hz). At the high intensity, the hair cell saturates to both directions but in an asymmetric fashion, yielding energy primarily at the third harmonic (1,500 Hz) with some at the second. For the auditory nerve, a representative unit response at the round window (RW) and a cycle histogram of the phase-locking are shown in response to the low intensity. The unit response is shown as cycle of a 1-kHz tone, and the histogram shows firing only to some phases of the stimulus, i.e., phase-locking. When these properties are convolved, the waveform expected at the RW from the auditory nerve neurophonic (ANN), or evoked correlate of phase-locking, is distorted compared with the unit response. The spectrum shows that the distortion is primarily second harmonic. The shape of the cycle histogram is constant across intensity and so is only shown once. The ECoG for each intensity is then the sum of these different sources. Amp, amplitude; APs, action potentials.

Note that in this schematic Fig. 1 there are many simplifications: the amplitudes of the CM and ANN are normalized to the peak, the CM and ANN signals are in phase, high-spontaneous-rate fibers at near-threshold stimulus levels can show energy only at the fundamental frequency, and details of the shapes of the unitary round window response and rectification can affect the harmonics in the ANN. However, this pattern serves as a useful framework to begin to analyze the contributions of each source to ECoG recordings in animals and humans. The effects of these additional factors are considered further in the discussion. For a high-frequency stimulus, the ANN should be small or absent because of the low-pass nature of neural phase-locking (Johnson 1980; Weiss and Rose 1988) so that the fine structure of the ECoG would be solely due to the CM. However, the sustained action potentials could contribute to the envelope of the ECoG. If so, the shape of the envelope could also help to distinguish the relative degree of SP and sustained neural firing in the envelope of the ECoG in pathological conditions.

MATERIALS AND METHODS

Twenty-three male Mongolian gerbils (Meriones unguiculatus) were used for round window ECoG recordings in response to tones. Experimental protocols were approved by the Institutional Animal Care and Use Committee according to the standards described by the National Institutes of Health Committee on Care and Use of Laboratory Animals.

Surgery.

The gerbils were anesthetized using 1.5 g/kg urethane and 6 mg/kg Nembutal delivered through an intraperitoneal injection. The Nembutal dose is 10% of anesthetic level and serves to sedate the animal. The urethane is a long-lasting anesthetic requiring no supplements during the course of the acute experiments (up to 8 h). A rectal probe was inserted and the animals were positioned on a heating pad to maintain their temperature between 36 and 38°C. After their heads were secured in a custom-made holder, a postauricular incision was made and soft tissue was dissected to access the bulla and clear the bony rim of the external auditory canal. The bone over the bulla was opened with the tip of a scalpel to provide access to the round window within its niche. A sound tube was placed at the bony ear canal, and a seal was made using ear impression material or dental cement.

Sound stimulation and evoked potential recording.

Both the sound tube and recording electrode were connected to a Bio-logic Navigator Pro (Natus Medical, San Carlos, CA), which generated acoustic stimuli and made recordings using its auditory evoked potential hearing diagnostics software. Acoustic stimuli were presented to the sealed ear canal using Etymotic ER-3 speakers. The speakers are calibrated for sound delivery to human listeners through insert earphones, so calibration for the animal studies was performed through a probe tube connected to the end of the sound tube at the entrance of the bony ear canal. Sounds were recorded at this location using a 0.25-in. Brüel & Kjær microphone (Nærum, Denmark). The amplitudes measured were corrected for the probe tube transfer function as determined before the experiments using couplers. Stimuli were tone bursts of varying frequency and intensity (in terms of sound pressure) presented in alternating condensation and rarefaction starting phases. Frequencies were 250, 375, 500, 750, 1,000, 1,500, 2,000, 3,000 and 4,000 Hz. These frequencies are relatively low for gerbils, which can hear up to ∼50 kHz (Ryan 1976), but contain the range most often encountered in human recordings from cochlear implant candidates (Choudhury et al. 2012; Fitzpatrick et al., in press). The ability to hear low frequencies is the major reason to use gerbils instead of more common laboratory animals such as mice and rats (Heffner and Heffner 2007). Intensities ranged from ∼10 to ∼90 dB SPL, depending on calibration corrections. The tone bursts had rise and fall times shaped by a Blackman window of either 1 cycle or 2 ms, depending on which was longer. The duration of the plateau was 6 to 24 ms, with longer durations for lower frequency stimuli. The stimulus rate was 23.3 Hz for the lower frequency tones and 29.3 Hz for the higher frequencies.

Recordings were made using a disposable tapered monopolar probe (Neurosign Surgical) with an exposed length of 200 μm and a tip diameter of 500 μm. This stainless steel electrode is the same as those used in human recordings from the round window (Choudhury et al. 2012). In both humans and gerbil the electrode can be placed by the surgeon to rest within the round window niche. Recordings consisted of 512 points collected with different sampling rates, depending on duration (8,000–48,000 samples/s). The averaged responses to condensation and rarefaction starting phases were stored in separate buffers. Recordings were started 4 ms before stimulus onset, and the recording epoch was 32 ms for each stimulus. Filters were high pass at 10 Hz and low pass at 5,000–15,000 Hz. Artifact rejection was set to 1.5 mV. The use of the same electrode and stimulus/recording system as the human recordings was intended to make comparisons between the data sets as similar as possible.

KA treatments.

KA is an analog of l-glutamate and has an excitotoxic effect on afferent auditory neurons (Bledsoe et al. 1981). It was shown by Dolan et al. (1989) to eliminate a residual potential leftover after application of tetrodotoxin (TTX) to the round window membrane. This residual potential, displaying similar properties to the CAP, was hypothesized to be the excitatory postsynaptic potential evoked by the hair cell neurotransmitter. Consequently, to completely remove nerve activity, KA is a better choice than TTX.

After evoked potentials were recorded, the recording electrode was removed from the round window niche and a syringe filled with 60 mM KA dissolved in lactated Ringer solution was placed in the same position at the niche. The warmed (37°C) KA solution was injected into the round window niche and covered the round window membrane. After 1 h, the solution was removed with the rolled tip of a tissue and flushed with lactated Ringer. The recording electrode was placed back into the niche, and post-KA evoked potentials were recorded in the same manner as before. Controls animals underwent the same procedure but received an injection of only lactated Ringer solution in the round window niche.

Data analysis.

The raw waveforms from condensation and rarefaction phases were stored separately. A difference curve was determined by subtracting the response to the condensation from the rarefaction phase and dividing by two, and a sum curve was obtained by summing the phases and dividing by two. The spectrum of each signal was obtained from the fast-Fourier transform (FFT), as implemented in MATLAB, using zero padding and a Blackman window. To avoid contributions from the CAP, windowing of the time waveform was used before the FFT was calculated. A response at the signal frequency or one of its harmonics was considered significant if the magnitude of the peak exceeded the noise level by three standard deviations (3 SD). The noise and its variance were determined from six bins, three on each side of the signal starting two bins away from the peak. In addition, circular statistics (Mardia and Jupp 1999) were used to determine if the distribution of response phase across cycles showed significant synchronization (Rayleigh test of uniformity, P < 0.001). This P value was chosen to be comparable to the 3 SD required of the spectral test. Both tests had to be met for the response to be considered significant.

RESULTS

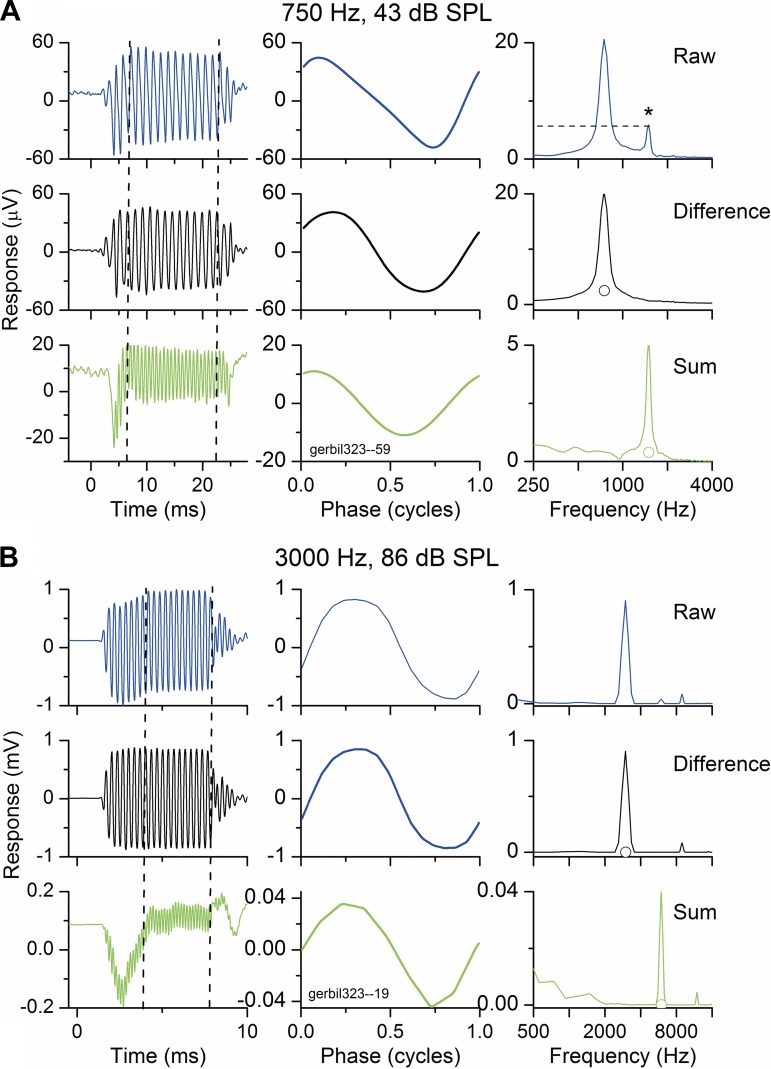
All animals had clean middle ears and normal thresholds for sounds in the round window recordings. Figure 2 shows results and analyses of recordings from the round window of a gerbil in response to a low-frequency (750 Hz) and a high-frequency (3,000 Hz) tone burst presented with alternating phases. The low frequency was at a moderate intensity (43 dB SPL), whereas the high frequency was more intense (86 dB SPL). For both frequencies, the response to each phase (called raw; only the condensation is shown for clarity) and the difference and summed signals are shown in different rows, and the time waveform, phase waveform, and frequency spectra are in different columns. These plots allow us to identify the magnitude and phase of the response and its distortions. For the low-frequency, moderate-intensity stimulus (Fig. 2A), the phase waveform (top middle) was a distorted version of the sine wave presented as the stimulus. This plot was derived by averaging each cycle in the ongoing part of the time waveform (between the dashed lines at top left). In the spectrum (top right), there was a large peak at the stimulus frequency and a smaller peak at the second harmonic (1.5 kHz, asterisk). Taking the difference between the condensation and rarefaction responses (middle row) emphasizes features that are dissimilar in the two phases. In this case, the only peak in the difference curve is at the first harmonic, or stimulus frequency, because these components are inverted in the two phases. Taking the sum (bottom row; equivalent to alternating the phase in most recording systems) emphasizes parts of the signal that are the same in each phase, because the parts that are out of phase add to zero. This is predominantly the second harmonic and so is twice the stimulus frequency. Note the difference in scale; the size of the second harmonic in this plot is the same as that of the raw signal (condensation phase alone, dashed line). In the time series of the summed response, a compound action potential is more evident than in the difference plot, indicating that at least part of this neural response was relatively insensitive to the phase of the stimulus.

Fig. 2.

Examples of recordings from the RW to 2 tone frequencies. The stimulus in each case was a tone burst presented in condensation and rarefaction phases with the raw waveforms to each phase stored in separate buffers. For both frequencies, the raw, difference, and summed signals are shown in different rows, and the time series, phase, and frequency spectra are shown in different columns. In the frequency spectra of the difference and sum curves, the response was considered significant if the peak was higher than the open circle, which represents the noise level plus 3 SD. Significance was also computed from the phase curve using circular statistics, with a Rayleigh coefficient of 13.66 (P < 0.01) as the criterion. A: response to a low frequency (750 Hz) and moderate intensity (43 dB SPL). The distortion in this case has only a second harmonic component (1,500 Hz). B: response to a high frequency (3,000 Hz) and high intensity (86 dB SPL). At this frequency/intensity combination there is distortion with both second and third harmonics.

For the high-frequency, more intense stimulus (Fig. 2B), the response was also distorted, but with a different harmonic content. In this case, there was energy at both the second and third harmonics in the raw waveform, with greater energy at the third harmonic. The third harmonic is also present in the difference curve, whereas in the sum curve, at an expanded scale compared with the raw waveform, there is a small fourth harmonic.

Following the scheme presented in Fig. 1, the interpretation of these results would be as follows. The ECoG, or raw waveform for each phase, contains the signal and its distortions derived from hair cell rectification and saturation and auditory nerve rectification. The difference curve in each case contains the CM and the ANN components at the signal frequency. The sum curve is that part of the ANN contributing a second harmonic due to rectification and any even-harmonic distortion due to the asymmetry of the input-output function. In the low-intensity case the hair cell contribution is likely to be small, whereas in the high-intensity case it may be substantial. If a third harmonic is present, as in the case in Fig. 1B, it will show up in the difference curve and indicates saturation of hair cell transduction in both directions of movement.

Responses across frequency and intensity in normal hearing animals.

Figure 3 shows the effect of frequency on input-output functions of the first and second harmonics, obtained from the FFT magnitudes in the difference and sum curves, respectively (see Fig. 1). The intensities shown are relative to that required to produce a 1-μV output at the fundamental frequency. This metric helps to normalize for differences in sensitivity across frequencies. The data for each frequency can be converted to SPL on the basis of other threshold data presented later. Examples of a low (750 Hz) and high frequency (3,000 Hz) show monotonic increases in the difference curves (Fig. 3A), with some saturation at the highest intensities. The minimum significant response is usually between 0.1 and 0.5 μV, or between −20 and −10 dB on the scale. For the sum curves (Fig. 3B), the function to 500 Hz is also monotonic, indicating second harmonic distortion at the lowest stimulus levels. In contrast, the function to 3,000 Hz did not begin to increase until the component at the fundamental frequency had reached 10 μV (20 dB on the scale), i.e., about 30 dB above the response threshold. This difference is consistent with the explanations provided above, i.e., the summed response to the low-frequency, low-intensity stimulus is primarily due to distortion produced by rectified, phase-locked nerve firing, whereas at high intensities, distortion does not occur until there is rectification in the hair cell response.

Fig. 3.

Responses across frequency and intensity in the difference and sum curves. To account for differences in sensitivity across frequency, the intensities are plotted relative to the level that produced a 1-μV response to the fundamental frequency from the difference curve for each frequency. A and B: example of responses across intensity to a low-frequency (500 Hz) and high-frequency (3,000 Hz) tone burst in 1 animal. Note that in the sum curve (B), the response to the low frequency has second harmonic distortion at the lowest intensities, whereas the high frequency has no distortion until the response becomes much larger. These responses indicate the ANN contributes to the ECoG at levels below those where rectification and saturation of hair cell transduction occurs. The reference 1-μV response level for 500 and 3,000 Hz occurred to stimuli of 45 and 22 dB SPL, respectively. C and D: responses to all frequencies and intensities averaged across animals (n = 13; error bars are SE). The transition from a “low-frequency” to a “high-frequency” pattern occurs between 1,000 and 1,500 Hz.

Next, we show similar data averaged across animals (n = 13). Again, the difference curves are relatively similar for each frequency (Fig. 3C). In the sum curves (Fig. 3D), the transition from the “low-frequency” pattern to the “high-frequency” pattern occurred between 1,000 and 1,500 Hz.

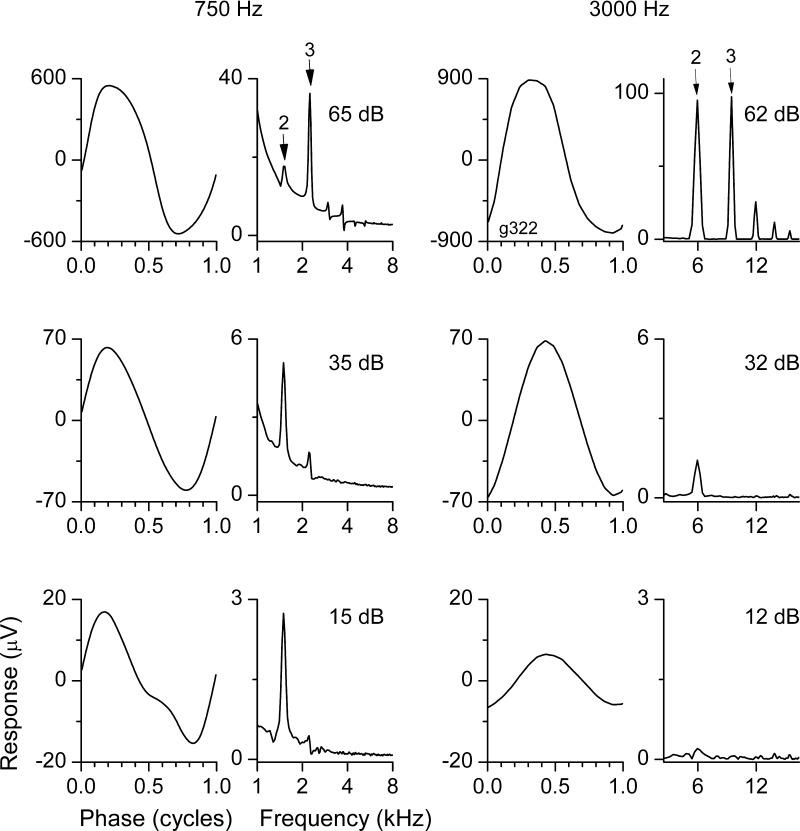
Third harmonics, produced by distortions that are symmetric around the zero axis, showed differential effects with intensity but not frequency (Fig. 4). For both the low- and high-frequency tones at a high intensity (top row), the third harmonic was prominent in the raw condensation curves. The presence of a prominent third harmonic at high intensities for both low and high frequencies indicates that hair cell saturation was the source of the distortion. At a moderate intensity (middle row), the distortion at both frequencies was dominated by the second harmonic. The second harmonic signal in the high frequency must be due to hair cell rectification, because it is above the range of the ANN. In the low-frequency signal, the second harmonic should contain both hair cell and ANN distortion. At a low intensity, the low frequency still showed a large second harmonic distortion, whereas the high frequency showed almost no distortion. The low-frequency distortion is presumed to be due to the ANN.

Fig. 4.

Signatures of neural vs. hair cell distortions. Stimulus levels are relative to that required to produce a 1-μV response to the fundamental frequency in the difference curves (18 dB SPL for 750 Hz and 15 dB SPL for 3,000 Hz). Top row: in response to a high intensity of both the low- and high-frequency tones, there was distortion in the waveform that included a large third harmonic as well as higher harmonics. The presence of a prominent third harmonic indicates that hair cell saturation contributed to the distortion (see Fig. 1C). Middle row: in response to a moderate intensity, the distortion to both frequencies was dominated by the second harmonic. Second harmonic distortion can arise from either hair cell or neural rectification (see Fig. 1B). Bottom row: at a low intensity, the low frequency still showed a large second harmonic distortion, whereas the high frequency showed almost no distortion. The distortion at low frequencies is presumed to be due the ANN (see Fig. 1A).

In the previous figures, stimulus levels were plotted relative to a constant response threshold to account for differences in sensitivity across frequencies. These differences are shown in Fig. 5, where the thresholds for a 1-μV response at each frequency are shown relative to the sound level (dB SPL) that produced them. For the first harmonic, taken from the difference curves, the 1-μV thresholds were higher for frequencies from 250 to 500 Hz than for 750 Hz and above. These increases at the lowest frequencies are consistent with the gerbils' audiogram (Ryan 1976). For the second harmonic, taken from the sum curves, thresholds were lowest at 750 and 1,000 Hz. These frequencies correspond to those where the ANN was present and thresholds were lowest. The second harmonic thresholds for lower frequencies increased despite the presence of the ANN because of lower sensitivity, whereas those for higher frequencies increased because of the lack of an ANN. The third harmonic threshold was at a high level across frequencies, consistent with the relatively high levels required for hair cell saturation.

Fig. 5.

Stimulus level required to reach the 1-μV response level for the first 3 harmonics. For first and third harmonics the difference curves were used, whereas the sum curves were used for measurement of the second harmonic. Error bars are SE.

Effects of KA.

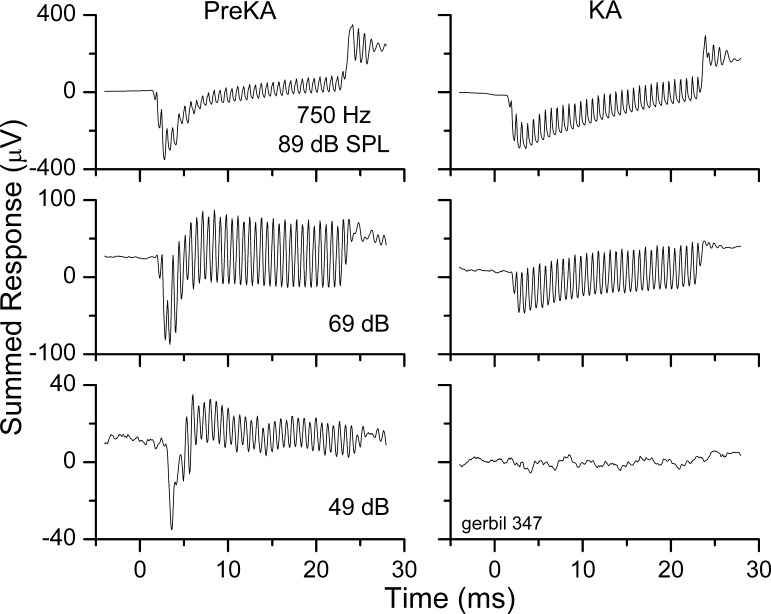
KA is a glutamate analog, and excitotoxicity caused by excess glutamate or KA severs the link between hair cell and nerve fiber. The effectiveness of the treatment can be seen in Fig. 6, which shows the summed response to a level series at 750 Hz before and after application of KA to the round window for 1 h. At each intensity, the treatment removed the CAP. At the lowest sound level (49 dB SPL), there was a large ongoing portion in the pre-KA response that was completely blocked by the treatment. This result indicates that the ongoing response in the sum curve at that intensity was due to the ANN. At higher sound levels, there was a considerable ongoing response both before and after KA. This result indicates that at higher levels, the second harmonic distortion has contributions derived from distortions in hair cell transduction. The third harmonic was also present at high intensities after KA treatment (not shown).

Fig. 6.

Time series of the summed responses to a 750-Hz tone before (pre-KA) and after kainic acid (KA) treatment. For all 3 intensities the KA removed the compound action potential (CAP). At the lowest sound level there was a large ongoing response pre-KA that was completely blocked by the KA, but at both of the higher levels there was a considerable ongoing response both before and after KA treatment.

Further examples and summary data are presented in Fig. 7. For a low-frequency tone burst (Fig. 7A), the KA caused a small loss of response at low and moderate levels to the first harmonic (left), whereas the loss of response to the second harmonic at low levels was nearly complete (right). The change in the second harmonic between the pre- and posttreatment data at this low frequency resembles the difference between low and high frequencies previously presented (Fig. 3B), i.e., the post-KA response to this low frequency resembles a high-frequency response. The change in the difference curve is presumably also due to the loss of the ANN, showing that neural phase-locking contributes a considerable proportion to the first harmonic in the ECoG at this low frequency. For a 3,000-Hz tone, the KA had virtually no effect on either the first or second harmonic (Fig. 7B). This result indicates no neural phase-locking contribution to the signal or its distortions at this high frequency. The post-KA response for all frequencies and intensities, averaged across animals, is shown in Fig. 7C. The first and second harmonic components of the responses were the same for both low and high frequencies. The similarity between high and low frequencies, particularly in the sum curves, is again consistent with the loss of phase-locked neural activity at the low frequencies (compare with Fig. 3D).

Fig. 7.

Effect of KA across frequencies and intensities. A: difference and summed responses to a low frequency (750 Hz) before and after KA treatment. The response at moderate intensities was smaller in the difference curve and essentially gone in the average curves. The reference 1-μV response level occurred to a stimulus of 21 dB SPL. B: difference and summed responses to a high frequency (3,000 Hz) before and after KA treatment. The response was relatively unaffected by the KA. The reference 1-μV response level occurred to a stimulus of 16 dB SPL. C: post-KA responses across frequency and intensity, averaged across animals (n = 6; error bars are SE). Unlike the responses without KA (see Fig. 2), there was no difference between high and low frequencies after treatment with KA.

In Fig. 8 the results in control and experimental animals are compared. Control animals had lactated Ringer solution placed at the round window for 1 h, whereas experimental animals had KA added. The metric was the change required to reach a 1-μV response criterion compared with the pretreatment condition. For the difference curves, a repeated-measures, two-way ANOVA showed that neither frequency (11 frequencies, F = 1.74, df = 10, P = 0.08) nor group (post-KA and control, F = 2.54, df = 1, P = 0.11), nor their interaction, had significant effects (P > 0.05). For the summed data, a larger loss of response was seen at frequencies of 1,000 Hz or lower in the KA animals compared with higher frequencies, resulting in a significant effect of frequency (F = 3.82, df = 10, P < 0.001). The effect of group was also significant (F = 6.73, df = 1, P = 0.010). The interaction between group and frequency just missed being significant (F = 1.84, df = 10, P = 0.06).

Fig. 8.

Comparison of the 1-μV threshold for the difference and sum curves for KA treatment and control animals. For control animals, lactated Ringer solution alone was placed at the RW, and there was little change in threshold in either the difference or sum curve across frequencies. With the KA treatment there was some overall loss of response at most frequencies, which was in general not significantly different from controls except at low frequencies. Error bars are SE. Level of significance in 2-tailed t-tests: *P < 0.05; **P < 0.01; ***P < 0.001.

Summating potential.

Treatment with KA also changed the envelope of the response. The envelope was measured as the DC shift in the ongoing portion of the sum curves compared with the prestimulus baseline response (Fig. 9A). In a response to 3,000 Hz at 90 dB SPL, the KA caused the envelope to change from positive to negative. At 80 and 70 dB SPL, the envelope was negative but became more so after the KA was applied. The change in the envelope across the range of stimulus levels is shown in Fig. 9B (red line).

Fig. 9.

Change in the envelope after KA treatment. A: responses are the sum curves for a high-frequency (3,000 Hz) tone at the intensities shown. The envelope (solid and dashed horizontal lines) became more negative after treatment with KA. B: curves from the same data as in A, including additional intensities. For the pre-KA and KA curves, the envelope was measured as the difference from baseline, and the Abs. Change curve is the absolute value of the difference between the 2 curves.

Whether the envelope was initially negative or positive varied with frequency and intensity within animals and could be different across animals. Consequently, to compare results across cases, the analysis used was to plot the absolute magnitude of the change due to KA, as in Fig. 9B. In Fig. 10, it can be seen that the change in the envelope after KA was greatest at high frequencies and high intensities. The two-way, repeated-measures ANOVA showed significant effects of frequency (F = 15.3, dF = 10, P < 0.001) and intensity (F = 18.2, df = 8, P < 0.001) and a significant interaction between them (F = 2.8, df = 96, P < 0.001).

Fig. 10.

Change in the magnitude of the envelope across frequencies and intensities and averaged across animals (n = 6; error bars are SE). The changes were greatest for high frequencies and high intensities.

DISCUSSION

The major results were that distortions in the waveforms recorded in response to tones from the round window have different sources over different ranges of frequency and intensity. At low frequencies and low-to-moderate sound intensities, the major source of distortion is from neural phase-locking, which is sensitive to KA. At high intensities at all frequencies, the distortion is not sensitive to KA and is therefore predominantly from rectification and saturation of hair cell stereociliary movements. An additional result was a large change in the envelope after KA treatment at high frequencies and intensities. This change after loss of nerve activity indicates that sustained neural firing combines with hair cell receptor currents (the SP) to produce the envelope of the response to tones.

Previous recordings from the round window.

Recording from the round window has a long history, including many studies using gerbils. Most studies in gerbils and other species have focused on the CM, CAP, and SP (reviewed by Dallos 1973). Each of these potentials is also seen in human audiological recordings and is of clinical importance. In contrast to these potentials, the ANN has received relatively less attention. Although it has been previously described in animal studies, including gerbils (e.g., He et al. 2012; Henry 1995, 1997; Snyder and Schreiner 1984, 1985), it has only recently been shown to be a prominent part of the signal in human ECoG recordings from the round window (Choudhury et al. 2012). In the animal studies, the frequency ranges and intensities over which harmonic distortion was obtained were identified and are consistent with those reported here. However, in studies from the round window by Henry (1995), the label “ANN” was used for second harmonic distortion across the intensity and frequency range. Instead, we show that only at low frequencies and low intensities is the ANN the main source of distortion, whereas at low frequencies and high intensities, and at high frequencies and all intensities, the CM is the main source. Interestingly, for all frequencies the hair cell transduction began to add to the distortion at a constant level of about 30–40 dB above the 1-μV response threshold (Figs. 3 and 7). In the gerbil, this corresponds to 40–50 dB SPL for frequencies of 1 kHz and above, and higher levels for lower frequencies where the response thresholds increase (Fig. 5). The pattern is roughly equivalent to the gerbil's audiogram (Ryan 1976), so the sound level where hair cell transduction begins to contribute to the distortions are expected to vary by species.

The fact that the distortions occur at a constant response level independent of frequency suggests that they are primarily due to hair cell transduction, rather than a result of nonlinear basilar membrane movement. The nonlinearities in basilar membrane movement depend on a functioning cochlear amplifier (Cooper 1998), and the strength of the compressive nonlinearity is much greater in the base than in the apex (Cooper and Rhode 1995). If basilar membrane motion were a primary source of non-KA-sensitive distortions in the ECoG, a different pattern across frequency, reflecting changes in the strength of the cochlear amplifier, would be expected.

The alternating phase method for canceling the CM to isolate the ANN.

Alternating the phase of the signal is often used to reverse the polarity of the CM so that when added together, any signal remaining is considered to be primarily neural. A popular model of why the ANN or frequency following a response recorded from the scalp produces a prominent second harmonic component after alternation is that the rectified signals to each phase will interleave. When they are added, the resulting signal has twice the stimulus frequency (Choudhury et al. 2012; Gardi et al. 1979; Henry 1995; Lichtenhan et al. 2013). However, for at least the ANN, inspection of Fig. 2 and results with KA suggest instead that the correct interpretation is the one given in Fig. 1. Recall that in Fig. 1, the second harmonic distortion in the “ECoG signal” includes any sources that produce rectification, whether of hair cell or neural origin. Thus, even at moderate intensities, alternating the stimulus does not produce a purely neural waveform in response to a low-frequency stimulus where phase-locking is present. In addition, at low levels where the auditory nerve does provide the only source of distortion, the greatest energy of the rectified signal is still at the signal frequency itself, not the second harmonic. The data in Fig. 2 support this view. In Fig. 2, the second harmonic distortion is present in the signals to each phase alone (A and B, top row, raw signals). The magnitudes of the peaks at the second harmonic to each phase are equivalent to the peak in the sum, or alternated, case (note the difference in scales). Thus there is no added benefit in detecting the second harmonic by alternating the signal. In addition, when the difference of two half-wave rectified signals to alternating phase is taken, the difference curve has greater energy than the alternated curves, and it is at the signal frequency (simulation not shown). Thus energy at the signal frequency is derived from the ANN, not only from the CM. This view is supported with the KA experiments, because with low-frequency stimuli it was often the case that the energy at the signal frequency increased or decreased after KA treatment. This can occur when the ANN and CM have different phases in relation to the stimulus, as would be expected due to synaptic delay, as well as differences in saturation and spread of excitation between the two signals (Patuzzi et al. 1979). Further simulations, similar to those shown in Fig. 1 but allowing the phase between the CM and ANN to vary, showed that the ANN can either add to or subtract from the energy at the signal frequency (not shown). No change in the energy at the signal frequency would be expected to occur in models where the ANN is considered to be entirely in the sum curves. This discussion shows that the utility of alternating the phase to separate the CM from the ANN is actually quite small. It does serve to “detrend” the waveforms if there is drift in the recordings, but otherwise the main information is contained in the response to each phase alone.

Range of phase-locking in single auditory nerve fibers compared with recordings from the round window.

The range of phase-locking in the current experiments showed an abrupt drop-off between 1,000 and 1,500 Hz (Figs. 3D and 7). This is a reasonable match for the cutoff frequency seen in populations of auditory nerve fibers in many species (e.g., Johnson 1980; Weiss and Rose 1988), including the gerbil (Versteegh et al. 2011). However, phase-locking is measureable above 1,000 Hz in single fibers of most species, again including the gerbil. In the cat, Lichtenhan et al. (2013) also found that the ANN cutoff frequency was about 1,000 Hz, despite the known presence of single-fiber phase-locking to higher frequencies. They argued that 1,000 Hz was a physical upper limit for field recordings of the second harmonic because the duration of the potential evoked by a single action potential is about 1 ms. This process should provide a low-pass filter on the response, and the similar results in the gerbil support this explanation. Other methods may be able to demonstrate phase locking above 1,000 Hz. By using a forward masking technique, reductions of response from the ANN at the stimulus frequency can be detected, which is in principle a more sensitive measure than detecting the distortion. The presence of an ANN at frequencies above 1,000 Hz were reported in both cats and normal-hearing humans with this method (Verschooten et al. 2011, 2013). Phase changes may also be a more sensitive measure than amplitude of the distortions. In a recent study in the gerbil, phase-frequency plots showed a flat slope for frequencies above about 2.5 kHz but an increasing phase lag for lower frequencies that disappeared after treatment with TTX (He et al. 2012).

Spread of excitation.

Previous articles have suggested that modeling the ANN and CM to any given stimulus is difficult because the response magnitudes and phases depend not only on changes in each responding element but also the extent of the cochlea contributing to the response and the geometry between active elements and the recording electrode (Chertoff et al. 2012; He et al. 2012; Snyder and Schreiner 1985). In this report we have clarified results that will need to be modeled, but we can offer only a few comments on the underlying parameters. First, the KA data show that hair cell transduction must be the predominant source of distortion in the round window ECoG at high frequencies and in all frequencies at high intensities (Fig. 7). Thus, despite spread of excitation to high-frequency regions where stereociliary movement would be expected to be in the linear range, the rectification and saturation of this movement is still a prominent component of the total signal. In animals or humans with high-frequency hearing loss, where the spread of excitation is limited, the distortion could be expected to make a larger proportion of the total response.

Spread of excitation is likely to affect the shape of the rectified response, which has a large influence on the harmonics in the ANN. In Fig. 1, the shape of the rectified response used in the convolution is less than is typical for an auditory nerve fiber, as can be seen because the response is spread across more than a half cycle. This shape is reasonable because the contributions from suprathreshold stimuli will include some extent of the cochlea, which due to traveling wave phase delays will spread the response in time. When modeling the convolution, we found that if the shape of the rectification had a synchrony more nearly approaching that of a single auditory nerve, the convolution produced more energy at the third harmonic, although the second always predominated.

Spread of excitation also makes determination of the source of the responses within the cochlea through phase-frequency plots unreliable. That is, because the source location of potentials varies for different frequencies, changes in phase do not reflect a travel-time delay. He et al. (2012) showed that for high frequencies, the phase delay of round window recordings from the gerbil relative to the stapes was flat. For low frequencies the slope was dominated by the ANN, because it became flat after application of TTX. He et al. (2012) also questioned the general view that the round window recordings are dominated by responses from the base of cochlea. Using suppressor tones to mask the response to a low-frequency probe, they showed that the response to a low-frequency tone arose from near the characteristic frequency (CF) region at low levels and above the CF region at high levels. Our previous observations using animals with noise-induced high-frequency hearing loss are also consistent with a large contribution from the apical cochlea to the round window response (Choudhury et al. 2011).

Envelope of the ECoG.

The envelope of the ongoing response was altered by KA. This shows that the envelope is not formed just by the SP, which is typically understood as a hair cell potential (Dallos et al. 1972; Durrant et al. 1998; Zheng et al. 1997). Instead, the results indicate that sustained neural potentials contribute to the round window response. This nerve contribution could be called the ANSP, for auditory nerve sustained potential. The ANSP is strongest at high frequencies and intensities. It is thus the continuation of the ANN to frequencies where phase-locking makes little contribution, and the neural continuum of ANN to ANSP is a close parallel to the receptor potential in inner hair cell transduction (Palmer and Russell 1986).

With one exception that we are aware of, the ANSP has generally not been considered to be an important component of the ECoG in mammals. Instead, the DC response has been considered to be due to the SP alone. The exception is a report by Kupperman (1966) that suggested the positive SP was produced by asynchronous neural activity based on a latency that was similar to the CAP, while the latency for the negative SP was shorter. In contrast, previous studies using KA or TTX in mammals have generally reported no change in the envelope (Bledsoe et al. 1981; Dolan et al. 1990; Jenison et al. 1986). However, studies in birds have shown a contribution from sustained firing in the nerve similar to our result (Sun et al. 2000). This potential was called the slow positive neural potential. Our results are not exactly equivalent, because the direction of the change was not uniformly in the positive direction. The previous mammalian studies generally illustrated a limited number of waveforms, and the quantitative data appear to be an average change across animals. As has been often reported, the sign of the envelope response is variable across frequencies, intensities, and preparations (e.g., Dallos et al. 1972; Davis et al. 1958). Therefore, averaging across cases can obscure changes to the envelope at high frequencies and intensities that occur in every case with KA. For this reason, we presented the average of the absolute change in the envelope, rather than the average including the sign. With this analysis, large changes across cases were evident. The contribution of the ANSP to the envelope response is not entirely surprising, since most frequency/intensity combinations, particularly those more than 40–50 dB above threshold, will activate a large fraction of auditory nerve fibers with many firing at a high rate.

Despite differences in absolute sign across cases, within cases the direction of change of the envelope response due to ANSP was consistent across intensity (see Fig. 9D). At some frequency/intensity combinations the sign of the envelope DC could change from positive to negative after KA (again, as in Fig. 9D). These results suggest that the variance in sign across cases may be due to electrode configuration. We placed the return electrode on an exposed neck muscle, but not consistently in the same location. The results also suggest that the potentials from the asynchronous nerve activity and from the receptor potential in hair cell transduction oppose each other. This finding is consistent with other literature suggesting two sources to the envelope, such as inner or outer hair cell transduction (Davis et al. 1958; Johnstone and Johnstone 1966). The results with KA, and earlier results from Kupperman (1966), suggest that one of the sources contributing to the envelope is the ANSP.

Implications for human round window ECoG recordings during cochlear implantation.

We have recently shown cochlear potentials can be recorded with high signal-to-noise ratio from nearly all cochlear implant recipients (Choudhury et al. 2012; Fitzpatrick et al. 2013). The recordings are relatively easy to obtain, requiring only about 10 min per case with the use of standard clinical audiometric instrumentation. If properly interpreted, this information could prove useful in fitting patients to their speech processor and in counseling on possible outcomes. A major motivation of the current study was to identify markers in an animal model that could determine the degree of hair cell and neural components within the signal recorded from the human round window. The animal model is valuable because of the ability to test a wide range of frequencies and intensities and to remove the neural contribution through the use of neurotoxins.

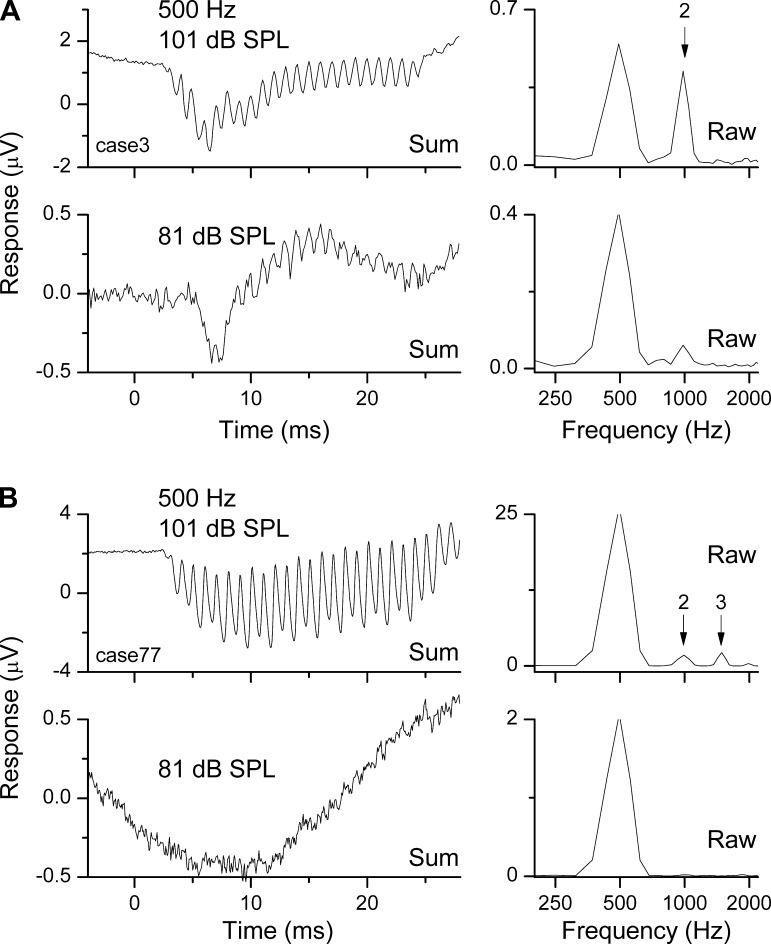
The animals used in the current study had normal cochlear function as assessed by their ECoG thresholds (first and second harmonics). These animals can provide baseline data for the physiological processes that contribute to the field potentials. A more accurate model of the clinical hearing condition typical of many cochlear implant candidates can be obtained through the use of damaging levels of high-pass noise, which produce a hearing condition with preserved low-frequency and loss of high-frequency sensitivity (Choudhury et al. 2011, 2013; Suberman et al. 2011). A future goal is to extend the experiments with KA to animals with this type of sloping hearing loss. However, examples of round window recordings from human cochlear implant patients show many of the features identified in the current study. Examples for two subjects are shown in Fig. 11. For one (Fig. 11A) there was a strong second harmonic to the 500-Hz stimulus and no third harmonic at two intensities, indicating that the potentials included neural phase-locking. In another, there were nearly equivalent-sized peaks for the second and third harmonic at the loudest intensity and no distortion at a lower intensity, indicating that the harmonic distortion at the louder intensity was from hair cell transduction with little if any contribution from the auditory nerve. These examples show that the round window ECoG can be a diagnostic tool for measuring the functional state of the cochlea with more precision than is possible by less invasive recording methods.

Fig. 11.

Responses from the RW of human subjects just prior to cochlear implantation. A: responses for a subject where the distortion was due primarily to the ANN. Responses to 2 intensities are shown in 2 rows. The summed time series for each intensity is shown at left, and the frequency spectrum for the raw condensation phase is shown at right. Note that the time series therefore does not contain energy at the signal frequency, but the spectrum does. For both intensities, the time series has a CAP and ongoing response at twice the tone frequency of 500 Hz. The dominant distortion is due to the second harmonic, which for the louder sound has nearly the same magnitude as the peak at the tone frequency. B: responses for a subject where distortion was due primarily to the hair cells; same format as in A. There is no CAP apparent in the time series, and little or no second harmonic is evident at the lower intensity. In the frequency spectrum of the more intense sound, there is both second and third harmonics.

Audiograms and auditory brain stem responses (ABRs) can only reflect the degree of fully functional hearing, i.e., complete connections between hair cells and nerve fibers. In contrast, the round window recordings can also detect hair cell responses not connected to auditory nerve fibers. Until recently, it was thought that hair cells were the part of the system most sensitive to noise damage, and so would contribute little information not available in audiograms and ABRs. However, subjects with auditory neuropathy spectrum disorder have functioning outer hair cells but disorders of transmission between inner hair cells and nerve fibers (Starr et al. 1996). In addition, it has been shown in mice and guinea pigs that the part of the system most sensitive to noise trauma is the synapse between hair cells and nerve fibers, rather than the hair cells themselves (Furman et al. 2013; Kujawa and Liberman 2009; Lin et al. 2011). As many as 50% of nerve fibers in these species may be lost after moderate noise exposures that cause temporary threshold shift. This loss of synapses is not associated with loss of basilar membrane/hair cell function and over extended periods of weeks or months leads to loss of spiral ganglion cells. Similar pathologies are evident in human temporal bone cases (Makary et al. 2011). Based on these considerations, a large fraction of cochlear implant recipients can be expected to have functioning hair cells unconnected to nerve fibers. A reasonable hypothesis then is that the presence or absence of responding hair cells can provide information about the overall health of the cochlea that could support nerve fibers and spiral ganglion cells that can be electrically stimulated. That is, for two patients with similar audiogram thresholds and ABR growth functions, if one shows evidence of preserved hair cells and the other does not, it might be expected that the one with more functional hair cells will also have more nerve fibers or ganglion cells able to be stimulated and better speech performance with the implant. Thus the magnitude of ECoG recordings could reflect speech outcomes, as has been seen in preliminary results (20 patients, ECoG magnitude summed across frequency vs. consonant-nucleus-consonant word scores, r² = 0.47; Fitzpatrick et al. 2013). Making the best use of the ECoG signal requires a better understanding of the proportion of the signal derived from hair cell and nerve, to which this and future studies in animals are intended to contribute.

Conclusions.

We reached the following conclusions from this study. 1) At low frequencies and low-to-moderate sound intensities, the major distortion in the round window ECoG is at the second harmonic and sensitive to KA, and is therefore caused by neural phase-locking that produces the ANN. 2) At high intensities at all frequencies, the distortion includes second and third harmonics that are not reduced by KA and are therefore predominantly from rectification and saturation of the hair cell transduction mechanism. 3) The envelope of the response at the round window contains a contribution from sustained firing in the auditory nerve. 4) Determining the relative contributions of hair cell and neural potentials evident in the ECoG should be useful in understanding the variance of outcomes with cochlear implants.

GRANTS

This work was supported by the National Institute for Deafness and other Communication Disorders (NIDCD) Grant 5T32 DC005360 (P. Manis), the Howard Holderness Distinguished Medical Scholars Program, and a research grant from the MED-EL Corporation.

DISCLOSURES

D. C. Fitzpatrick receives contractual research support from Advanced Bionics, Cochlear Corporation, and MED-EL Corporation.

C. A. Buchman receives contractual research support from Cochlear Corporation and MED-EL Corporation, research grant support from the NIDCD, and is an unpaid consultant for Advanced Bionics, Cochlear Corporation, MED-EL Corporation, and Anspach Corporation.

O. F Adunka receives contractual research support from Advanced Bionics, Cochlear Corporation, and MED-EL Corporation and is a consultant for MED-EL Corporation.

AUTHOR CONTRIBUTIONS

M.F., S.H.P., O.F.A., and D.C.F. conception and design of research; M.F., H.A.K., A.K.D., S.H.P., O.F.A., and D.C.F. performed experiments; M.F., H.A.K., A.K.D., and D.C.F. analyzed data; M.F., C.A.B., O.F.A., and D.C.F. interpreted results of experiments; M.F. and D.C.F. prepared figures; M.F., H.A.K., A.K.D., S.H.P., C.A.B., O.F.A., and D.C.F. edited and revised manuscript; M.F., H.A.K., A.K.D., S.H.P., C.A.B., O.F.A., and D.C.F. approved final version of manuscript; D.C.F. drafted manuscript.