Spatial frequency bandwidth of surround suppression tuning curves

Abstract

The contrast detection threshold of a grating located in the periphery is increased if a surrounding grating of the same frequency and orientation is present. This inhibition between center and surround has been termed surround suppression. In this work we measured the spatial frequency bandwidth of surround suppression in the periphery for different spatial frequencies (0.5, 1.1, 3, and 5 cycles/deg) of a sinusoidal grating (target) surrounded by a grating with different spatial frequencies (surround). Using a Bayesian adaptive staircase, we measured contrast detection thresholds in an 8AFC detection task in which the target (grating with a 2.3-deg Butterworth window) could appear in one of eight possible positions at 4° eccentricity. The target was surrounded by a grating (with a 18° Butterworth window) with the same or an orthogonal orientation. In each session we fixed the spatial frequency of the target and changed the spatial frequency and the orientation of the surround. When the surround was orthogonal to the target, the thresholds were similar to those obtained without surround, independent of the surrounding spatial frequency. However, when the target and surround had the same orientation and spatial frequency, the contrast threshold was increased by a factor ranging from 3 to 6 across subjects. This suppression reduced rapidly as the spatial frequency of the surround moved away from that of the target. The bandwidth of the suppressive effect depended on spatial frequency, declining from 2.9 octaves at 0.5 c/deg to 1 octave for frequencies above 3 c/deg. This is consistent with the bandwidth of individual simple cells in visual cortex and of spatial frequency channels measured psychophysically, both of which decline with increasing spatial frequency. This suggests that surround suppression may be due to relatively precise inhibition by cells with the same tuning as the target.

Introduction

A small patch of pattern can be harder to see when it is surrounded by a larger area of the pattern than when it is presented in isolation. In particular, the contrast threshold for detecting a target grating is increased if the target is surrounded by a grating of the same frequency and orientation (Ejima & Takahashi, 1985; Lev & Polat, 2011; Petrov, Carandini, & McKee, 2005; Polat & Sagi, 1993; Snowden & Hammet, 1998; Xing & Heeger, 2000; Yu & Levi, 2000). The psychophysical properties of this surround suppression have been studied by several workers. As a result, we know that the strongest surround suppression occurs when the target stimulus is located in the periphery and surrounded by an annular grating with a high contrast, the same orientation, and the same spatial frequency. Surround suppression tends to be weaker when the target is near the fovea (Petrov et al. 2005; Petrov & McKee, 2006; Snowden & Hammet, 1998; Xing & Heeger, 2000). It also depends on the relative orientation between the target and surround. Suppression is maximized if the surround grating has the same orientation as the target; surround gratings oriented orthogonal to the target have little or no effect on contrast detection thresholds (Petrov et al., 2005; Polat & Sagi, 1993). Under some conditions, orthogonally oriented surrounds can even improve contrast discrimination (Yu & Levi, 2000) and show a facilitation effect, enhancing the apparent contrast of the target (Cannon & Fullenkamp, 1993; Ejima & Takahashi, 1985; Polat & Sagi, 1993; Yu, Klein, & Levi, 2003).

The effect (suppression or facilitation) also depends on the relative contrast of the center and surround regions. For example, surround patterns with higher contrast than the target can reduce the apparent contrast of the target (Cannon & Fullenkamp, 1991, 1993; Chubb, Sperling, & Solomon, 1989; Olzak & Laurinen, 1999; Snowden & Hammet, 1998; Xing & Heeger, 2000; Yu, Klein, & Levi, 2001; Yu et al., 2003), potentially increasing the contrast detection threshold for the target. However, if the contrast of the target is higher than the surround, a facilitation effect can occur (Cannon & Fullenkamp, 1993; Ejima & Takahashi, 1985; Yu, Klein, & Levi, 2002; Yu et al., 2003).

We also have a fair idea of the underlying neuronal mechanisms responsible for surround suppression. Analogous behavior can be seen in physiological experiments recording from single neurons. We can distinguish the effect of non-overlapping surround patterns from the masking effect produced by patterns that are spatially overlapped (overlay suppression), both psychophysically (Petrov et al., 2005) and with physiological data (DeAngelis, Robson, Ohzawa, & Freeman, 1992; DeAngelis, Freeman, & Ohzawa, 1994). Broadly, center-surround suppression seems to occur because each neuron receives inhibitory input from a pool of surrounding neurons. However, the detailed functional architecture is still not clear. For example, we do not know how closely the tuning of the inhibitory pool matches that of the center neuron. One way of assessing this psychophysically is to determine the strength of surround suppression as a function of surround frequency, keeping the center frequency constant.

The effect of surround spatial frequency on surround suppression using iso-oriented surround has been investigated by only a small number of studies (Cannon & Fullenkamp, 1991; Petrov et al., 2005; Yu & Levi, 2000; Yu et al., 2001). The results have been conflicting, complicated by the fact that center-surround interactions clearly depend strongly on whether the stimulus is presented in the fovea or peripherally and on the contrasts involved. Ultimately, these psychophysical results will have to be related to the properties of visual neurons in order to gain a full understanding of the neuronal mechanisms involved.

In this paper, we contribute to this goal by measuring the effect of surround spatial frequency on the contrast detection threshold of a peripheral target grating, for both iso-oriented and cross-oriented surround gratings (Figure 1). Our objective is to determine the contrast detection thresholds of different center spatial frequencies, surrounded by gratings of different spatial frequencies, in order to measure the spatial frequency bandwidth of the suppression tuning curves.

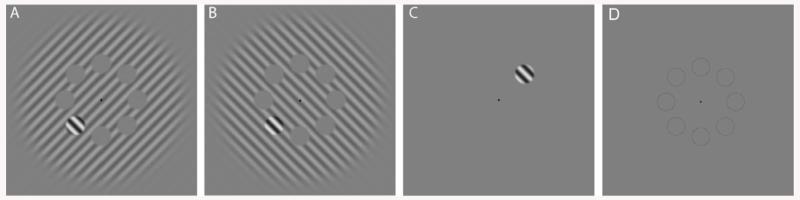
Figure 1.

Example stimuli. (A) Stimulus with orthogonal surround; (B) parallel surround; and (C) no surround. In this example surround and target have the same spatial frequency. (D) Image with the eight positions outlined that appear after the stimulus.

Methods

Subjects

Four human subjects (aged between 18 and 37 years) with experience in psychophysical experiments, took part in the experiments. The subjects KL, GY, and CB were not aware of the purpose of the study. All subjects had normal or corrected-to-normal refraction and normal visual acuity. Experimental procedures were approved by Newcastle University’s Faculty of Medical Sciences Ethics Committee. One author (ISP) and one experienced subject (MGC), who was not aware of the purpose of the study, took part in the control experiments. Experimental procedures were approved by Complutense University’s Ethics Committee.

Equipment

The experiments were carried out in a dark room. The stimuli were presented on a 16-inch monitor (SONY Trinitron Multiscan G200, Sony Corp., Tokyo, Japan) under the control of a PC running Matlab (MathWorks, Natick, MA) using the Psychophysics Toolbox extensions (Brainard, 1997; Pelli, 1997; www.psychtoolbox.org) and Bits++ (Cambridge Research Systems Ltd., Cambridge, UK), giving 14 bits of gray-scale resolution. The monitor was gamma corrected using a Minolta LS-100 photometer (Konica Minolta Optics, Inc., Osaka, Japan). It had a resolution of 800 × 600 pixels (horizontal × vertical) with vertical frame rate of 120 Hz, a mean luminance of 42 cd/m², and was observed binocularly from a distance of 50 cm. A chin rest (UHCOTech HeadSpot, Houston, TX) was used to stabilize the subject’s head and to control the observation distance. Stimuli were presented at the center of the monitor screen in a square of 19.5 cm per side (512 × 512 pixels), subtending an area of 22.1° × 22.1°, resulting in 23 pixels per degree of visual angle. The remainder of the screen was at the mean luminance. For the control experiments we used a 19-inch monitor EIZO Flexscan 720 (Eizo Corp., Japan) with a resolution of 1024 × 768 pixels (horizontal × vertical) with a vertical frame rate of 120 Hz under the control of an Apple Macintosh Pro (Cupertino, CA). The rest of conditions (Psychtoolbox extensions, gray-scale resolution, mean luminance, chin rest, distance, and stimulus size) were the same as used in the main experiment.

Stimuli

The stimuli combined elements from Yoon et al. (2010), Cannon and Fullenkamp (1991), and Petrov et al. (2005). We used a Bayesian adaptive staircase to measure contrast detection thresholds in an eight-alternative spatial forced-choice (8AFC) paradigm in which the target (grating with a 2.3-deg Butterworth spatial window of order 10, see González & Wintz [1987, p. 179, 181] and Sierra-Vazquez, Serrano-Pedraza, & Luna [2006]; a formal definition can be seen in their appendix A) appeared randomly in one of eight possible positions at 4.1° eccentricity (see Figure 1). We chose this eccentricity because it has been shown that surround suppression (when target and surround have the same orientation) is stronger in the periphery than in the fovea and reaches a plateau at eccentricities greater than 4° (Petrov et al., 2005). The values of the stimulus parameters given here were altered in control experiments 1 and 2; the parameters used the control experiments are described in the text.

We tested three general conditions in which the target could appear in a surround grating with an orthogonal orientation (Figure 1A), with the same (parallel) orientation (Figure 1B), or with no surround (Figure 1C). The surround gratings had a fixed Michelson contrast of 0.25 and a 18-deg Butterworth window of order 10. The orientation of the target and surround was randomly ±45°. The phase of the target and the surround was the same but randomized in each trial. Olzak and Laurinen (1999) found that stronger suppression occurs when targets and surrounds with same orientation are in phase, although Petrov and McKee (2006) found that surround suppression is not affected by the phase of the surround.

In order to control the target’s contrast independent of the surround’s contrast, we presented target and surround in different frames and temporally interleaved them (Schofield & Georgeson, 1999; Serrano-Pedraza & Sierra-Vázquez, 2006). Thus, although the frame rate of the monitor was 120 Hz, the stimuli were in practice presented at 60 Hz after frame interleaving. In the condition without surround we used the same technique but with zero contrast for the surround. Note that interleaving a grating of contrast 1 with gray frames reduces the final contrast of the grating by half (0.5). The contrast thresholds reported in the Results refer to the effective contrast after interleaving.

Procedure

Each trial started with a fixation cross displayed at the center of the screen using a Gaussian temporal function with standard deviation of σ_t = 80 ms truncated to give an overall duration of 500 ms. After the fixation cross the stimulus with the target (Figure 1) appeared modulated in time by a Gaussian temporal function with σ_t = 100 ms (duration of 200 ms, 2σ_t), truncated to give an overall duration of 500 ms. We chose a Gaussian temporal function to control the temporal presentation because its Fourier transform is a Gaussian function too (Bracewell, 1986, p. 98, 130) and therefore it does not introduce high-temporal frequency components in the spatiotemporal frequency domain as other temporal windows do (i.e., Heaviside unit step or ideal temporal window). The contrast of the target was controlled by an adaptive staircase procedure. Then the stimulus was followed by an image with the eight possible positions outlined (Figure 1D), and the subject’s task was to indicate the position of the target by pressing a mouse button. A new trial was initiated only after the observer’s response; thus the experiment proceeded at a pace determined by the observer.

In each session we fixed the spatial frequency of the target and the spatial frequency of the surround. We measured contrast detection thresholds for targets of different spatial frequencies (0.5, 1.1, 3, and 5 cycles/deg) and for surrounds of different spatial frequencies around the frequency of the target.

Contrast detection threshold was defined as the minimum Michelson contrast that is needed in order to achieve a performance of 55.37% correct, with chance being 12.5%. Contrast detection thresholds were measured using adaptive Bayesian staircases (Treutwein, 1995) using a 8AFC paradigm. In general between 4 and 6 min were required per contrast detection threshold estimation. The characteristics of the Bayesian staircases were (a) the prior probability density function was uniform (Emerson, 1986; Pentland, 1980) with a starting contrast of 0.495; (b) the logistic function was used as the model likelihood function adapted from García-Pérez (1998, appendix A) with a spread value of 1 (with delta parameter equal to 0.01, a lapse rate of 0.02, and a guess rate of 0.125); (c) the value of the target contrast in each trial was obtained from the mean of the posterior probability distribution (King-Smith, Grigsby, Vingrys, Benes, & Supowit, 1994); (d) the staircase stopped after a fixed number of trials (30 trials) (Anderson, 2003; Pentland, 1980); and (e) the final threshold was estimated from the mean of the final probability density function. Two contrast threshold estimations per condition were obtained for each subject when surround was present. Four contrast thresholds were obtained when the surround was not present. A total of 48 conditions (4 target spatial frequencies × 2 surround orientations × 6 surround spatial frequencies) were tested in the experiments with surround and 4 (4 target spatial frequencies) in the experiments without surround. The different conditions were counter-balanced across subjects. Practice sessions were performed previous to the experiment.

Data analysis

In order to obtain the bandwidth of the surround suppression, we used least squares estimation and the multidimensional Nelder-Mead simplex search algorithm (Nelder & Mead, 1965) to fit a log-Gaussian function (blue line in Figure 2) with three free parameters (A, f₀, and α) to the parallel data (red circles in Figure 2):

$${\log }_{10}\left[m_0\right(f\left)\right]=L+A\exp \left[-\frac{{\ln }^2(f∕f_0)}{2{\alpha }^2}\right]$$ (1)

where f₀ corresponds to the peak frequency of the fit, L corresponds to the contrast threshold of the target grating without surround, and m₀ corresponds to the contrast detection threshold of the target grating surrounded by a grating of spatial frequency f. This log-Gaussian model was chosen because it matched the shape of the data and has a well-defined bandwidth. The bandwidth (full-width at half maximum, in octaves) is:

$$B_{\mathrm{oct}}=\alpha \times \left(2\sqrt{2}∕\sqrt{\ln 2}\right)$$ (2)

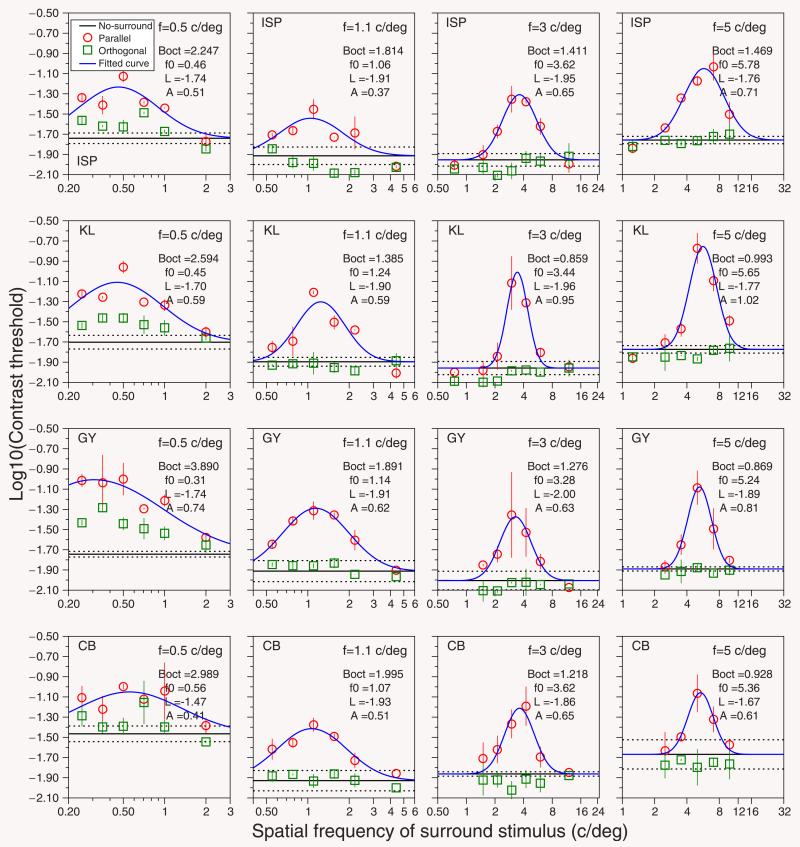
Figure 2.

Results for four subjects. Each panel shows logarithmic contrast thresholds as a function of the spatial frequency of the surround stimuli. Each file shows the results for one subject. Each column shows the results for one spatial frequency of the target (0.5, 1.1, 3, and 5 cycles/deg) presented within a spatial window of fixed size of 2.3°. Symbols show the mean of our two measurements of the contrast thresholds for detecting the target as a function of the spatial frequency of the surround; error bars go between the two measurements (equivalent to SD for n = 2). Red circles, parallel surround; green squares, orthogonal surround. Horizontal black line, represents the mean (± SD, dotted lines) of the contrast thresholds for detecting the target without surround. Gaussian-shape function represents the fitted curve (blue line). The target’s spatial frequency and the fitted parameters of the model are represented on the right side of each panel. The value of B_oct corresponds to the bandwidth (in octaves) of the model calculated using Equation 2 (see text).

Results

Contrast detection thresholds were measured for gratings (target) presented in the visual periphery surrounded by orthogonal (cross-oriented surround) gratings, parallel (iso-oriented surround) gratings, or with no surround. Four spatial frequencies were used (0.5, 1.1, 3, and 5 cycles/deg) for the target gratings and several different spatial frequencies for the surround, centered in each case on the spatial frequency of the target.

Figure 2 shows the results for our four subjects. Each row corresponds to one subject. Each panel shows the logarithmic contrast thresholds for detecting a target of a particular spatial frequency as a function of the spatial frequency of the surround grating. The horizontal black line represents the contrast threshold for detecting the target without any surround (base line), and the dotted lines above and below represent the standard deviation. Green squares represent the contrast thresholds for detecting the target in the presence of a surround with orthogonal orientation and different spatial frequencies (orthogonal data). Red circles represent the contrast thresholds for targets with surround of the same orientation (parallel data). Blue line represents the model (see Equation 1) fitted to the parallel data points.

Strength of surround suppression at different spatial frequencies

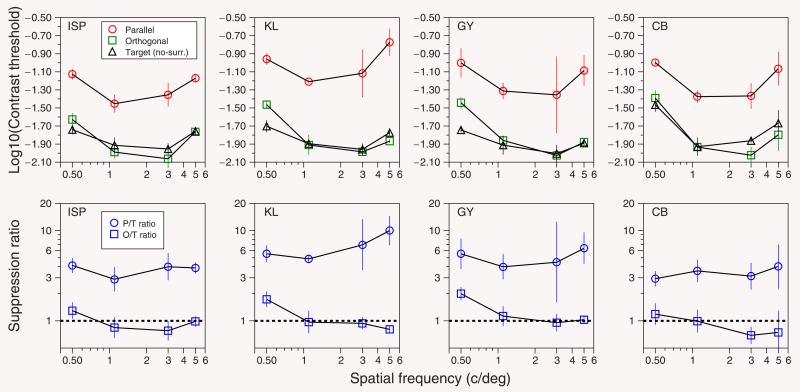
When the surround is parallel to the target (red circles in Figure 2), contrast thresholds are substantially higher, indicating suppression. In each case, the strongest suppression occurs when the center and surround have the same spatial frequency. When the surround is orthogonal to the target, the contrast thresholds are essentially unaffected by the presence of the surround, at least for frequencies above 0.5 cycles/deg. In Figure 3, we replot this same spatial frequency data for both parallel and orthogonal surrounds and also when there is no surround. The top row shows the contrast thresholds for our four subjects, for spatial frequencies of 0.5, 1.1, 3, and 5 cycles/deg. As expected from the human contrast sensitivity function, the curves have a similar U shape. The highest sensitivity is found for spatial frequencies between 1 and 3 cycles/degree, somewhat lower than at the fovea; a result that is expected given that contrast sensitivity declines with eccentricity (Robson & Graham, 1981) more rapidly for high spatial frequencies than for low spatial frequencies (Wright & Johnston, 1983). The bottom row shows the ratio of the contrast threshold for the parallel surround to that for the no-surround condition (blue circles) (i.e., the usual surround suppression factor [Petrov et al., 2005]) and also the ratio between orthogonal and no-surround conditions (blue squares). This shows that suppression of the target by a matching surround is almost independent of the target spatial frequency. When the surround is orthogonal to the target, there is essentially no suppression at the frequencies tested. The exception is the lowest frequency tested, 0.5 cycles/deg, for which there was some weak suppression (O/T ratio significantly greater than 1 in subjects KL and GY). This orthogonal–surround suppression was about a factor of 3 weaker than for a parallel surround.

Figure 3.

Results for four subjects. The results are obtained from Figure 2 in the conditions without surround (target alone) and with surround (parallel and orthogonal) for which the spatial frequency was the same for the target and surround. Each panel on the top row shows the logarithmic contrast thresholds (mean ± SD) as a function of the spatial frequency of the target. Red circles, parallel surround; green squares, orthogonal surround; and black triangles, target alone (no surround). The panels on the bottom row show the suppression ratio calculated in contrast units. Blue circles, ratio between contrast thresholds in parallel and target conditions; blue squares, ratio between contrast thresholds in orthogonal and target conditions. Error bars show the 70% confidence interval estimated by bootstrap resampling. We generated 20,000 thresholds using a normal random distribution with the mean and SD of the thresholds on the upper panels. We calculated the suppression ratios and took the percentiles 85% and 15% from the distribution of ratios.

Spatial frequency bandwidth of surround suppression

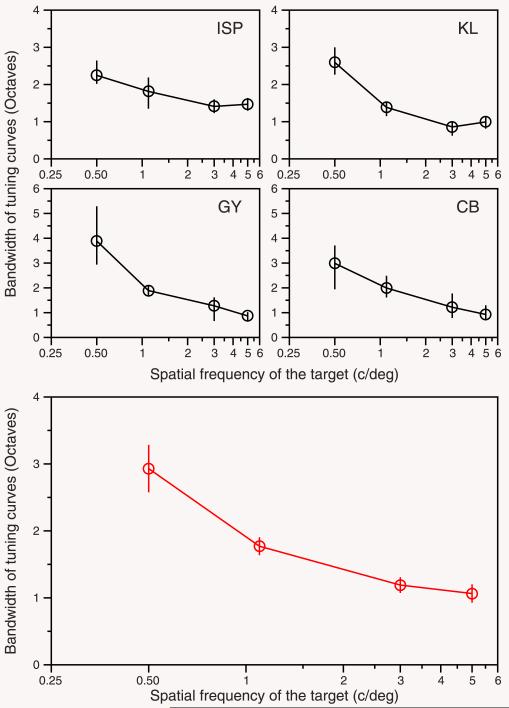
Figure 2 shows that the suppressive effect is stronger when target and surround have the same spatial frequency as well as orientation. When the frequency of the surround moves away from that of the target, the suppression is rapidly reduced. Contrast thresholds as a function of the spatial frequency of the surround have a Gaussian profile on our log-log axes. We therefore fitted a log-Gaussian function (blue line) to the contrast detection thresholds obtained in the parallel condition (see Data analysis, Methods section) in order to obtain the bandwidth of the surround suppression. Figure 4 shows this bandwidth (in octaves) for the four subjects as a function of the spatial frequency of the target. In every subject, the surround suppression bandwidth decreases with increasing spatial frequency of the target.

Figure 4.

Bandwidths of surround-suppression tuning curves. The four upper panels show the results for each subject. Black circles, data taken from the B_oct values in Figure 2. Error bars show the 70% confidence interval estimated by bootstrap resampling. We generate 2000 thresholds (in the parallel condition) using a normal random distribution with the mean and SD of the thresholds in the Figure 2. We fitted Equation 1 (as in Figure 2) to the resampled data and then we took the percentiles 85% and 15% from the distribution of estimated bandwidths (B_oct). The lower panel (red circles) shows the mean (± SEM) of bandwidths (in octaves) estimated from the four subjects.

Control experiments: effect of target size on the bandwidth of surround suppression tuning curves

The results of the main experiment show that the bandwidth of the surround suppression tuning curves declines from 2.9 octaves at 0.5 cycles/deg to 1 octave for frequencies above 3 cycles/deg (see Figure 4, bottom panel). However, there is a possibility that this result may be a direct consequence of the spatial window size used in the experiment. In particular, we used a fixed size (2.3° of diameter) for each spatial frequency of the target. This means that the spatial frequency bandwidth of the target stimuli also falls with increasing spatial frequency, potentially explaining our results. In order to disentangle this confound we performed two control experiments.

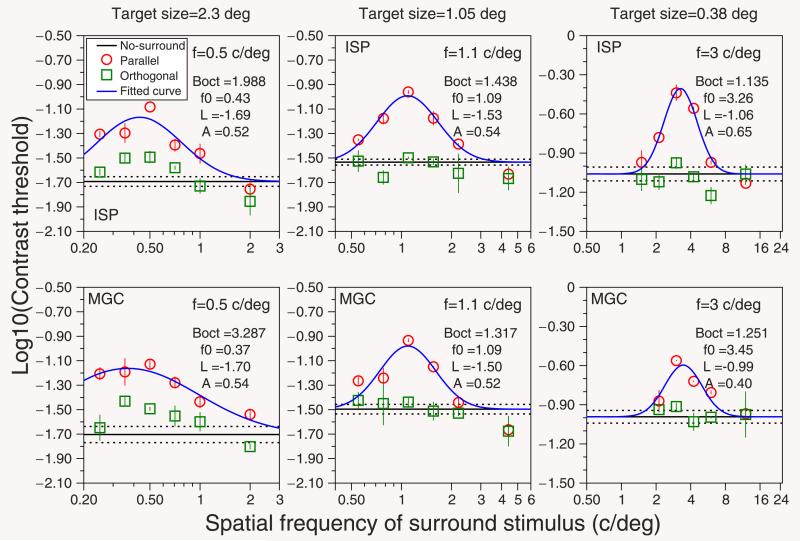
In control experiment 1 (see Figure 5), we replicated the main experiment using targets with the same number of visible cycles. We kept the center of the target at the same eccentricity, 4.1°, but reduced the size of the spatial window as the target spatial frequency increased. We used three target spatial frequencies (0.5, 1.1, and 3 cycles/deg) and three target sizes (2.3°, 1.05°, and 0.38°), respectively. We did not measure suppression curves for 5 cycles/deg given that the spatial window needed for this frequency would be just 0.23°, which is very difficult to perceive at 4.1° eccentricity. One author (ISP) and one new subject (MGC), who did not participate in the main experiment, took part in the experiment. Figure 5 shows the results of this control experiment. The bandwidths (B_oct, Equation 2) estimated from the fitted curve (Equation 1) decreased with increasing spatial frequency of the target, even when the same numbers of cycles were visible (see Figure 7). Note that contrast thresholds for 1.1 and 3 cycles/deg (subject ISP) were higher than in the main experiment, since the stimuli at those frequencies were smaller.

Figure 5.

Results of control experiment 1 for two subjects (ISP and MGC). Each panel shows logarithmic contrast thresholds as a function of the spatial frequency of the surround stimuli. Each file shows the results for one subject. Each column shows the results for one spatial frequency of the target: 0.5, 1.1, and 3 cycles/deg, presented within a spatial window of diameter 2.3°, 1.05°, and 0.38°, respectively. The value of B_oct corresponds to the bandwidth (in octaves) of the model calculated using Equation 2. Other details as in Figure 2.

Figure 7.

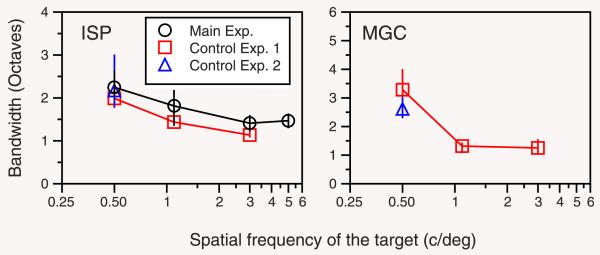
Bandwidths of surround-suppression tuning curves of control experiments. Each panel shows the results for each subject (ISP and MGC). Black circles, data taken from the main experiment for the same subject (Figure 2); red squares, data taken from the B_oct values in Figure 5 (control experiment 1); blue triangles, data taken from the B_oct values in Figure 6 (control experiment 2). Error bars show the 70% confidence interval estimated by bootstrap resampling. Other details as in Figure 4.

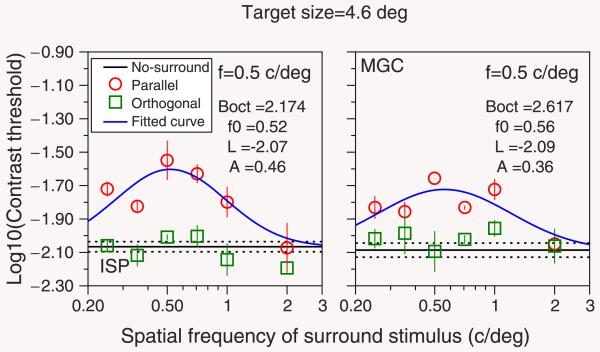
In control experiment 1, the window size for the target of 0.5 cycles/deg was the same as in the main experiment. We also asked whether increasing the target size for this spatial frequency would have an effect on the suppression bandwidth. In control experiment 2 (see Figure 6) we replicated the main experiment for a target with spatial frequency of 0.5 cycles/deg but doubling the size of the spatial window to 4.6°. In order to fit in such large targets, we had to change the experiment from a 8AFC task to a 4AFC (two vertical and two horizontal positions), and therefore the contrast threshold was defined as the Michelson contrast that is needed in order to achieve a performance of 61.75% correct, with chance being 25%. A window of 4.6° would of course extend much closer to the fovea if its center had the same eccentricity, potentially reducing the suppressive effect (Petrov et al., 2005). Thus, in order to maintain the same part of the retina free of stimulation, we now presented the target at 5.3° eccentricity. This means that, in both the main experiment and in control experiment 2, the innermost edge of the target had an eccentricity of 3°. The results presented in Figure 6 show that bandwidths of surround suppression tuning curves of targets of 0.5 cycles/deg were very similar to those obtained with smaller window sizes (2.3°) and bigger than for targets of higher spatial frequencies.

Figure 6.

Results of control experiment 2. Each panel shows logarithmic contrast thresholds as a function of the spatial frequency of the surround stimuli. Each column shows the results for one subject (ISP and MGC). The spatial frequency of the target was 0.5 cycles/deg, presented within a spatial window of diameter 4.6°. The value of B_oct corresponds to the bandwidth (in octaves) of the model calculated using Equation 2. Other details as in Figure 2.

Figure 7 summarizes the results of both control experiments, plotting the bandwidths obtained for the same subjects under different conditions. The suppression bandwidth at 0.5 cycles/deg is independent of the target window size, and the bandwidth of suppression falls as a function of frequency, even though the bandwidth of the target is now constant. This shows that the constant size of the target spatial window in the main experiment cannot explain the decreasing bandwidth of surround suppression tuning curves.

Discussion

In this research we have measured contrast detection thresholds of a target grating located at 4.1° eccentricity surrounded by gratings with same or orthogonal orientations. Our objective was to measure the surround tuning curves for different center spatial frequencies in order to estimate the bandwidth of these suppression tuning curves. Our results show that (a) surround suppression is strongest when the surround has the same spatial frequency and orientation as the target; (b) the strength of surround suppression is almost independent of the spatial frequency of the target over a 10-fold range; (c) suppression strength is a log-Gaussian function of the difference between the spatial frequency of target and surround; (d) the spatial-frequency bandwidth of suppression tuning curves falls with increasing spatial frequency of the target, from 2.9 octaves at 0.5 cycles/deg to 1 octave at 5 cycles/deg; this bandwidth fall has also been replicated for targets with different sizes and with targets with the same number of visible cycles (control experiments 1 and 2); and (e) orthogonal surrounds had no effect on contrast thresholds at frequencies above 1 cycle/deg but produced very weak suppression at 0.5 cycle/deg. We did not measure the orientation bandwidth of surround suppression, but our results could be explained if this also falls with increasing spatial frequency. For frequencies above 1 cycle/deg, an orientation bandwidth of less than ~70° (full-width half-maximum) would ensure that cross-oriented surrounds produce no suppression; for 0.5 cycles/deg, an orientation bandwidth of 140° would produce the observed weak cross-suppression.

Comparing our results with previous studies, we find considerable variation, suggesting that the particular task and stimulus parameters are critical. Cannon and Fullenkamp (1991) and Yu et al. (2001) both used a contrast-matching paradigm, surrounded by a grating with either iso- or cross-orientation (contrast-contrast phenomenon; Chubb et al., 1989; Olzak & Laurinen,1999), in order to measure the apparent contrast of a grating patch presented at the fovea. Because center-surround mechanisms depend on contrast and retinal location (Cannon & Fullenkamp, 1991, 1993; Chubb et al., 1989; Olzak & Laurinen, 1999; Petrov et al., 2005; Snowden & Hammet, 1998; Xing & Heeger, 2000; Yu et al., 2001, 2003), it is not surprising that there are differences with the results we obtained using threshold contrast in the periphery.

First, our bandwidths appear much narrower than those measured at the fovea. Cannon & Fullenkamp (1991) in their experiment 5, measured the apparent contrast for three center spatial frequencies (2, 4, and 8 cycles/deg) as a function of surround spatial frequency. Their results are qualitatively similar to ours, in that the bandwidth of iso-surround suppression decreases with increasing spatial frequency, but the bandwidths were broader. For iso-oriented surround when the target had a spatial frequency of 2 cycles/deg, suppression was uniform over the 2 octaves they tested (no tuning); at 4 cycles/deg, some tuning was evident; and at 8 cycles/deg, the tuning was narrower still, though still broader than our 1 octave bandwidth at 5 c/deg. Yu et al. (2001) found that surround suppression is very broadly tuned to spatial frequency. In our results at target spatial frequencies of 3 or 5 cycles/deg, surround suppression was nearly abolished when the surround had twice the spatial frequency of the target; whereas, in Yu et al. (2001), suppression was as strong as when the surround frequency matched that of the target.

Second, both Yu et al (2001) and Cannon and Fullenkamp (1991) found an asymmetry in the effect of spatial frequency mismatches. Cannon and Fullenkamp (1991) found more suppression for surround spatial frequencies above the target frequency than below. Yu et al. (2001) found that the apparent contrast of the target was enhanced by surrounds of lower spatial frequency, whether these had iso- or cross- orientation. Cross-oriented surrounds of higher frequency than the target had no effect, whereas iso-oriented higher frequency surrounds reduced the apparent contrast of the target. Thus, both studies found that surround frequencies that are higher than the target frequency have a relatively stronger suppressive effect, while surround frequencies lower than the target have weaker suppression and may even be facilitative. This implies that near the fovea, cells receive relatively more inhibition from their neighbors tuned to higher spatial frequencies (Cannon & Fullenkamp, 1991). In contrast, our tuning curves were symmetric for all target spatial frequencies tested; the strength of surround suppression also remained constant when expressed as a ratio of contrast thresholds with and without surround (Figure 3). In the periphery, therefore, it seems that there is no difference in the inhibitory weighting of low and high spatial frequencies, either in absolute terms or relative to the frequency of the center.

Third, as noted, previous workers have found that the surround can facilitate the target. As just noted, Yu et al. (2001) found that the apparent contrast of the target could be enhanced by a lower frequency surround. Yu and Levi (2000) examined the effect of a surround on the ability to perceive contrast increments on a pedestal, when surround and target both had a spatial frequency of 8 cycles/deg and were presented at the fovea. At a surround contrast of 25%, as used in our experiments, they found a facilitation effect for both iso- and cross-orientation surround. Iso-orientation surrounds became suppressive only at much higher contrasts (60%). With our peripheral stimuli, we found no evidence of iso-orientation facilitation and very little evidence of cross-orientation facilitation at any spatial frequency. Indeed, at the lowest frequency tested, two subjects showed cross-orientation suppression (KL and GY at 0.5 cycles/deg). These differences provided further evidence that center-surround mechanisms depend strongly on contrast and retinal location and probably on the nature of the psychophysical task as well.

The experiment of Petrov et al. (2005) is more directly comparable to ours. They measured contrast detection thresholds in order to determine a surround suppression tuning curve for iso-oriented surround at 68 eccentricity. They obtained a tuning curve peaking at the center spatial frequency (1.3 c/deg) of Gabor patches, with a spatial frequency bandwidth of 1.5 octaves. If we compare our result for the center spatial frequency of 1.1 c/deg, their bandwidth is not very different than ours (1.77 octaves) given that we obtained our results at 4.1° eccentricity and we used a different stimulus configuration. Both are narrower than the bandwidth obtained by Cannon and Fullenkamp (1991) for a target spatial frequency of 2 cycles/deg presented at the fovea.

Our results go beyond those of Petrov et al. (2005) by measuring this bandwidth as a function of center spatial frequency. Our results are consistent with the decreasing spatial frequency bandwidth of simple cells in striate cortex as a function of preferred spatial frequency (De Valois et al., 1982), and the decreasing bandwidth of psychophysical spatial frequency channels (Schofield & Georgeson, 2003; Serrano-Pedraza & Sierra-Vázquez, 2006; Solomon, 2000; Wilson, McFarlane, & Phillips, 1983). This suggests that surround suppression at contrast threshold may largely reflect inhibition by cells tuned to the same spatial frequency as the center. The bandwidth of the surround suppression would then reflect the bandwidth of the cells themselves, which decreases with increasing spatial frequency.