A Model for Tear Film Thinning With Osmolarity and Fluorescein

Abstract

Purpose.

We developed a mathematical model predicting dynamic changes in fluorescent intensity during tear film thinning in either dilute or quenching regimes and we model concomitant changes in tear film osmolarity.

Methods.

We solved a mathematical model for the thickness, osmolarity, fluorescein concentration, and fluorescent intensity as a function of time, assuming a flat and spatially uniform tear film.

Results.

The tear film thins to a steady-state value that depends on the relative importance of the rates of evaporation and osmotic supply, and the resulting increase of osmolarity and fluorescein concentrations are calculated. Depending on the initial thickness, the rate of osmotic supply and the tear film thinning rate, the osmolarity increase may be modest or it may increase by as much as a factor of eight or more from isosmotic levels. Regarding fluorescent intensity, the quenching regime occurs for initial concentrations at or above the critical fluorescein concentration where efficiency dominates, while lower concentrations show little change in fluorescence with tear film thinning.

Conclusions.

Our model underscores the importance of using fluorescein concentrations at or near the critical concentration clinically so that quenching reflects tear film thinning and breakup. In addition, the model predicts that, depending on tear film and osmotic factors, the osmolarity within the corneal compartment of the tear film may increase markedly during tear film thinning, well above levels that cause marked discomfort.

We solve a mathematical model for the thickness, osmolarity, fluorescein concentration and fluorescent intensity for a flat tear film thinning by evaporation. The model clarifies the regime where quenching occurs and predicts moderate to large increases in osmolarity depending on the parameters.

Introduction

Sodium fluorescein dye has been used clinically for more than 50 years to measure tear film stability and breakup^1,2 and assess corneal staining^3–5 and barrier properties.^6,7 The tear film breakup test (TBUT) involves instillation of the dye, usually via a saline-wetted dye-impregnated strip, followed by measurement of the time required for formation of so-called dry spots of tear breakup.⁸ Other tests, such as tear clearance, follow the elution of dye by monitoring decreased fluorescence over time as the dye is diluted by freshly secreted tears.^9–12 Thus, fluorescein dye has many uses in clinical practice associated with dry eye and is inextricably tied to our understanding of tear film instability, considered a core mechanism of the dry eye condition.

However, despite its widespread usage, many properties of fluorescein molecules, such as concentration quenching, are rarely considered when applied to the tear film. Concentration quenching is often used in cell biology to detect permeability and volume changes.^13,14 Cell shrinkage increases dye concentration, which leads to a decrease in fluorescence due to fluorescence energy absorption by more closely spaced adjacent molecules.¹⁵ Webber and Jones¹⁶ and Maurice¹ have introduced this concept for the tear film, producing a fixed thickness curve for fluorescence and introducing the idea of a critical concentration for maximum fluorescence (Fig. 1). Their work underscores the concept that both very low concentrations (dilute regime) and high (quenching regime) concentrations of fluorescein in the tear film should be avoided in clinical use because both would appear too dark for the tear film to be visualized. However, their experimental model is based on constant thickness and concentration and does not predict the changes in fluorescence when the tear film undergoes evaporative thinning, which is considered to be the major mechanism of water loss in the interblink interval.

Figure 1.

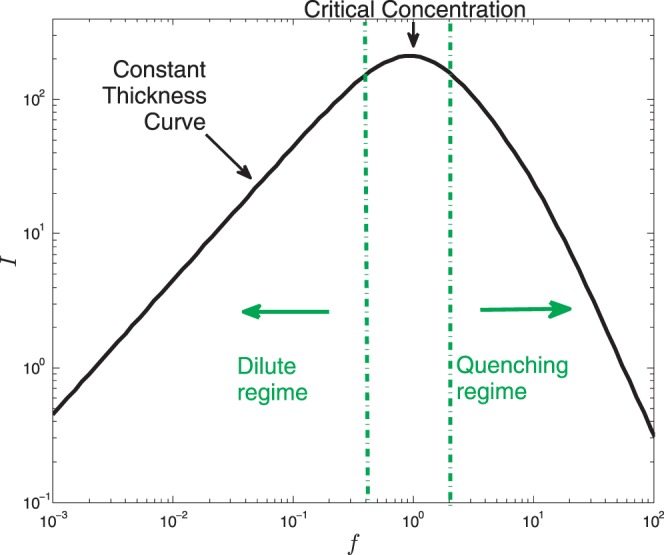
The solid curve shows the intensity I as a function of the normalized fluorescein concentration f for fixed tear film thickness h. This type of plot is after Webber and Jones¹⁶ and more recently by Nichols et al.²⁴ The maximum intensity occurs at f = 1 in this plot, which indicates the critical concentration. In the dilute regime, I ∝ f occurs at smaller concentrations, while in the quenching regime, I ∝ f⁻² occurs at larger concentrations. The regimes marked here are for constant thickness.

Tear film thinning by evaporation is also known to increase tear film hyperosmolarity, which is considered to be a core mechanism of dry eye disease, along with tear film instability.¹⁷ However, current methods do not allow direct measurement of tear film hyperosmolarity over the corneal compartment of the tear film.^18,19 Previous studies have suggested that tear film hyperosmolarity over the cornea may increase rapidly and perhaps, locally spike as high as 800 to 900 mOsm/kg, in the interblink interval, causing associated pain and pro-inflammatory stress to the ocular surface.^20–23 Thus, an understanding of dynamic changes in both tear film osmolarity and fluorescence over the cornea during the interblink interval would serve to address issues basic to our understanding of the dry eye condition.

For these reasons, we developed a mathematical model to predict the relationship between tear film fluorescence intensity, thickness, and osmolarity in the interblink interval as the tear film thins over time. The model expands upon the early fluorophotometer studies by Webber and Jones¹⁶ and Maurice,¹ but adds the critical component of tear film thinning to predict fluorescence and changes in osmolarity. It is also based on a more recent study by Nichols et al.,²⁴ who have experimentally measured tear film fluorescence changes with thinning and derived a mathematical expression linking fluorescence intensity, fluorescein concentration, and tear film thickness. Our model is designed to predict changes in fluorescence intensity and tear film osmolarity dynamically during tear film thinning. The results clarify which initial tear film fluorescein concentration ranges are useful relative to the critical concentration for maximum fluorescence.

Methods

Overview of Model and Assumptions

An overview of the model is presented below. Additional details of the derivation and mathematical aspects may be found in the Appendices.

Owing to the paucity of tear film mathematical models addressing evaporative thinning in the literature, this model represents a first-step simplification of the tear film during thinning (Fig. 2) and is based on certain assumptions noted below.

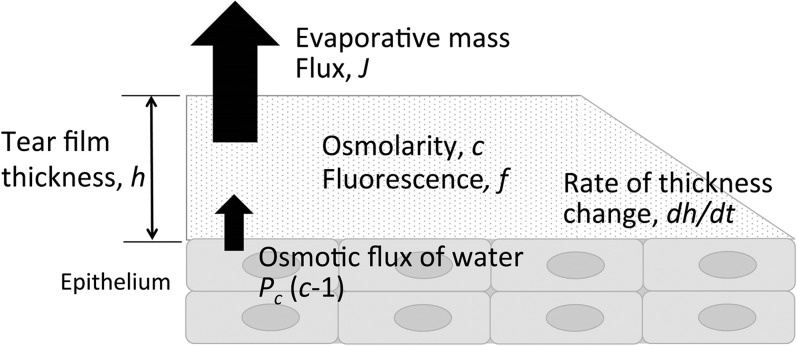
Figure 2.

A schematic of the tear film and epithelium. The mathematical model is for the tear film thickness (h), and the osmolarity (c) and fluorescein concentration (f) inside the tear film. The tear film loses water via evaporation (J) and gains water from the corneal epithelium via osmosis (P_c(c − 1)), causing thinning (dh/dt).

1. The tear film is simplified to an aqueous layer located between air and the corneal epithelium. There are no lipid or mucin layers in this model. The air/tear film interface is assumed to be flat on a flat substrate.^25,26 The thickness of the tear film is given by h′(t′) in micrometers (μm), which changes as a function of t′ (in seconds).

2. Tear film thinning is due to evaporation, as reported in the literature.^27,28 The evaporation rate is specified as the thinning rate from previously measured values using interferometry without goggles.²⁸ The tear film is assumed to lose water to the air via the evaporative mass flux J′(t′).

3. The tear film solutes that we consider in this model are tear film osmolarity and the fluorescein concentration (denoted by c′(t′) and f′(t′), in mOsM and weight %, respectively), and we assume that solutes inside the tear film cannot cross the air/tear film interface. As the tear film thins by evaporation, we assume that both the osmolarity and fluorescein increase in concentration, in a relationship to each other determined by known properties, so that fluorescein concentration can be used to model tear film changes in osmolarity during thinning. Both concentrations are assumed to be independent of depth, which can be justified mathematically for thin fluid films,²⁶ and we assume that solutes other than fluorescein molecules contribute to osmotic flow.

4. The corneal surface is included in the model as an osmotic membrane to account for osmotic flux from the tear film to the cornea and vice versa. Here it is modeled as a zero-thickness membrane because this approach can approximate the water transport across cell membranes²⁹ and the cornea.³⁰ The osmolarity on the corneal side of the boundary is assumed to be isosmolar²⁶ with a flux of water proportional to the concentration difference across it. Further, we assume that as the tear film thins by evaporation it can thin no further than the height of the glycocalyx.^31,32 We assume this height to be h_eq = 0.25 μm.^33,34

As the tear film undergoes evaporative thinning in the interblink interval, several critical concepts must be addressed in this mathematical model (Fig. 2). The first step is to ensure conservation of water and solutes in the tear film as water leaves by evaporation (J) to the air and enters by osmosis at the ocular surface (P_c(c − 1)). Both of these aspects would be expected to dynamically change as the tear film thins by evaporation (h) and solutes become more concentrated, increasing the osmolarity of the fluid (c). We enforce mass conservation by writing a set of equations, where some are differential and some are algebraic (shown below). To model changes in the fluorescence intensity with tear film thinning (f), the behavior of fluorescein molecules in the dilute and quenching regimes needs to be considered (Fig. 1). Our mathematical model begins with the fluorescent intensity as a function of fluorescein concentration as in Nichols et al.,²⁴ which describes the fixed-thickness observations of Webber and Jones¹⁶ and Maurice,¹ but then the model predicts dynamic changes with tear film thinning. Only major points of the model will be discussed below, with details presented in the Appendices. In the model, all variables are normalized in order to understand the common aspects of tear film dynamics for different parameter values.

Conservation of Mass: Water and Solutes

The following equations govern the effect of evaporation and osmosis on the tear film thickness, fluorescein concentration, and solutes (osmolarity):

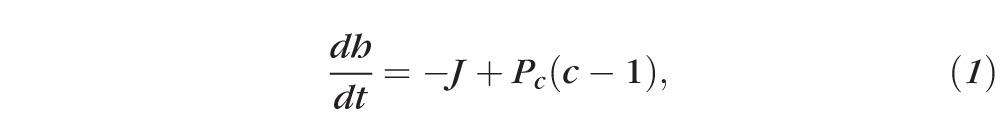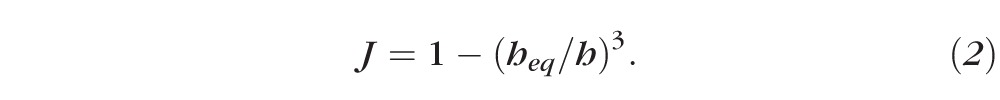Equation 1 represents the conservation of water as it leaves the tear film from evaporation and enters from osmosis. Equation 2 is an expression for the evaporative flux from the tear film to the air, which is often called a constitutive equation for evaporative mass flux in the physical science literature.³¹ Equations 3 and 4 ensure conservation of osmolarity (solutes) and fluorescein, respectively:

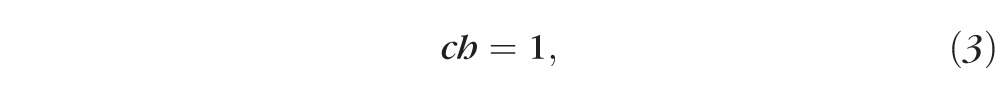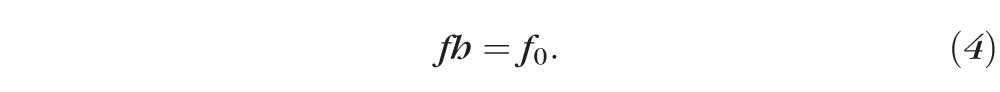The only differences in the model between osmolarity and fluorescein are that they have different initial conditions. Equation 3 uses an initial osmolarity normalized to one, but the initial fluorescein concentration is a ratio of actual concentration to the critical concentration to address the issue of fluorescence efficiency. In addition, fluorescein is assumed to not induce osmotic flow (this is implicit in tear turnover studies),^6,7 and we assume that fluorescein does not penetrate the cornea in the short time frame considered for dynamic tear film thinning (seconds rather than 30–40 minutes).¹⁶

We now turn to the osmotic interactions between the tear film and epithelium. As the tear film thins by evaporation,³⁵ solutes are expected to concentrate, inducing osmotic flow into the tear film from the epithelium. The normalized permeability of the cornea to water via osmosis is given by Equation 5.

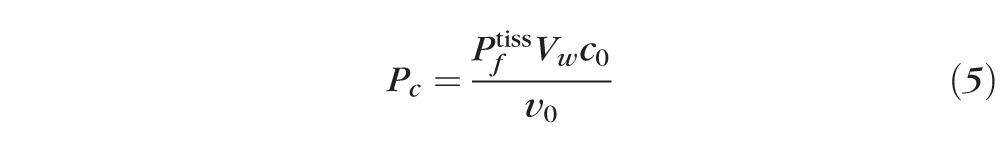Here P_f ^tiss is the permeability of the tissue to water²⁹ and V_w is the molar volume of water. Typical values for the physical parameters are given in Appendix B. There are few data available in the literature for the permeability of the cornea to water. Fatt and Weismann³⁰ have estimated the permeability from Ussing chamber data for animals and scaling those results with human dimensions. King-Smith et al.^35,36 have inferred the permeability from averaging thinning rate measurements on a set of 30 subjects. We use permeability values that range from 0 to the measured value in order to understand the range of possible dynamics. Solving for Equation 1 after eliminating J and c via Equations 2 and 3, one obtains Equation 6:

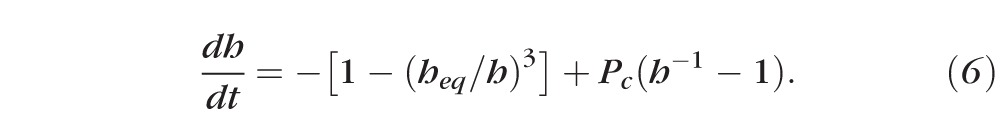The equation for the fluorescein concentration, f(t), has the same form as the osmolarity equation, except that it is expressed relative to the critical concentration. To compare closely with experimental data, we convert the fluorescein concentration to fluorescent intensity, using the formula developed in Nichols et al.²⁴ The fluorescent intensity is determined by two contributions. One contribution is the efficiency, E(f), which decreases with the second power of fluorescein concentration owing to fluorescence quenching. When normalized with the critical fluorescein concentration, f_cr, one obtains Equation 7.^15,24

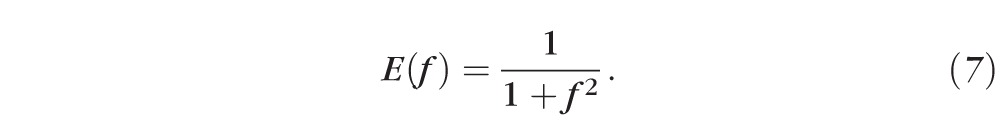The other contribution (Equation 8) is the absorptance of fluorescein (A).

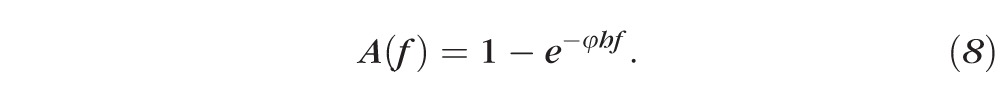For fixed thickness, h, we note that if the fluorescein concentration is high (i.e., f is large), then A ≈ 1 and E decreases toward zero quadratically, as quenching occurs. In contrast, if fluorescein concentrations are low (i.e., f is small), the opposite occurs. In Equation 9, the fluorescein concentration is then converted to intensity via the relation

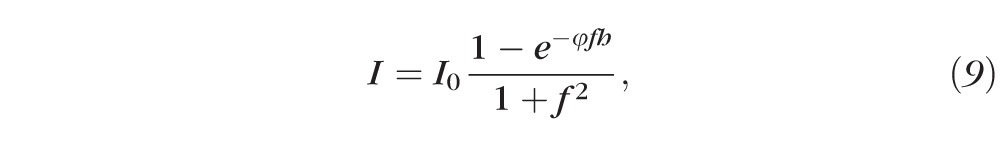which is valid over a wide range of fluorescein concentrations. Conservation of mass of fluorescein has important implications for the intensity, a function of fluorescein concentration, because it constrains h and f so that they do not change independently; specifically, their product must remain constant in this model. Thus, if we use Equation 4 to eliminate f from Equation 9, we obtain Equation 10.

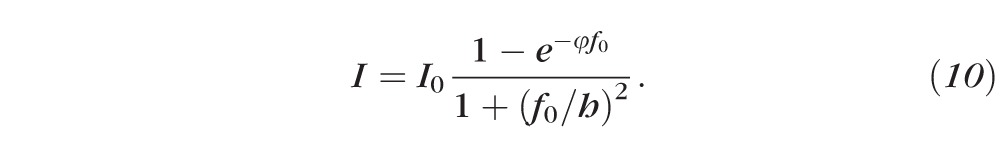We note that in this form, the fluorescence intensity decreases because of the denominator (h decreases during thinning), but the numerator is a constant, independent of h and f because their product is constant as a result of mass conservation of fluorescein molecules (Equation 4).

Results

Dynamics and Evaporation Rate

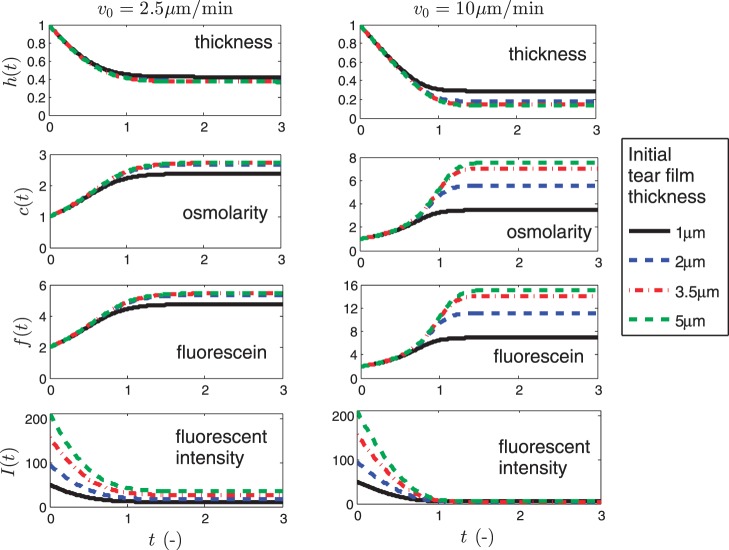
Figure 3 shows results of the model for evaporative thinning of the tear film, using normalized values to allow comparisons among initial tear thicknesses. We begin with the standard parameters for the problem, using previously published values for thinning rates²⁷ of v₀ = 2.5 μm/min and 10 μm/min and for four initial values of the film thickness d = 1, 2, 3.5, and 5 μm.³⁷ Here we assume P_f^tiss = 4 μm/s³⁶; thus, P_c = 0.52 or 0.13 for the two thinning rates, respectively. The initial concentrations are c = 1 (isosmotic) and f = 2 (twice the critical concentration) in both cases.

Figure 3.

The four normalized variables: h(t), c(t), f(t), and I(t) are shown as functions of normalized time t for v₀ = 2.5 μm/min and 10 μm/min. The legend applies to all of the plots.

The normalized results demonstrate that for all four initial thicknesses (d), the rate of thinning is the same and is effectively constant until osmosis becomes significant and an equilibrium thickness, h_∞, is reached for each thickness. This equilibrium thickness is expected to occur with evaporative thinning of the tear film because an osmotic flux of water is induced into the tear film from the epithelium by an increasingly hyperosmolar tear film with thinning. As some point, the two reach an equilibrium or steady-state thickness where evaporative flux out of the tear film and osmotic flux into the tear film are in balance (t ≈ d/v₀). If both the initial thickness and thinning rate (d and v₀) are known, it may be an estimate of TBUT due to overall thinning in this model. For d = 2 μm with v₀ = 2.5 μm/min, this estimate is approximately d/v₀ = 48 seconds, but the timing varies with faster thinning rates and/or thinner films.

Through conservation of mass (Equations 4 and 5), solute (osmolarity) and fluorescein concentrations increase proportionally to 1/h. In Figure 3 where v₀ = 2.5 μm/min, d = 1 μm, the osmolarity rises by a factor of approximately 2.4, to 720 mOsM. For the other three thicknesses, the increase is very similar, by a factor of approximately 2.7 to 810 mOsM dimensionally. These osmolarities are well above the threshold for sensation and, thus, are more than sufficient for subjects to perceive irritation or burning.²⁰ The fluorescein concentration (third row) rises by identical factors, so that the intensity decreases monotonically with decreasing h or increasing f, consistent with being in the quenching regime. Overall, Figure 3 shows the degree to which the osmolarity and fluorescein concentration increase as the tear film thins, but fluorescein intensity decreases owing to quenching of fluorescence.²⁴

Results for a larger evaporation rate of v₀ = 10 μm/min are shown in the right-hand column of Figure 3. One can see that the final relative thickness is now different for each initial thickness d; while these final thicknesses appear to differ by only small amounts, they have a significant effect on the osmolarity. For d = 1, 2, 3.5, and 5 μm, the osmolarity increases by factors of 4, 6, 7, and 8, to reach respective osmolarities of 1200, 1800, 2100, and 2400 mOsM. These osmolarities are quite high and would certainly cause marked discomfort.²⁰ The levels reached depend on the evaporation rate and the permeability of the cornea for osmosis, and in this case, we assumed a smaller permeability than that deduced by King-Smith et al.,³⁶ in order to explore the parametric behavior of the answers and because of likely variability of this parameter among subjects (may be similar to that for fluorophores, e.g., McNamara et al.⁷ and Araie and Maurice³⁸).

Dynamics and Initial Fluorescein Concentration

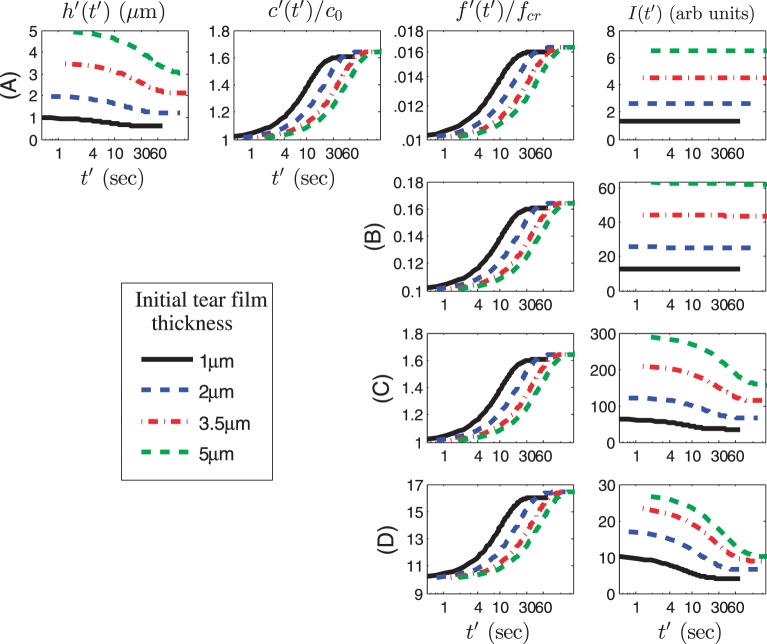
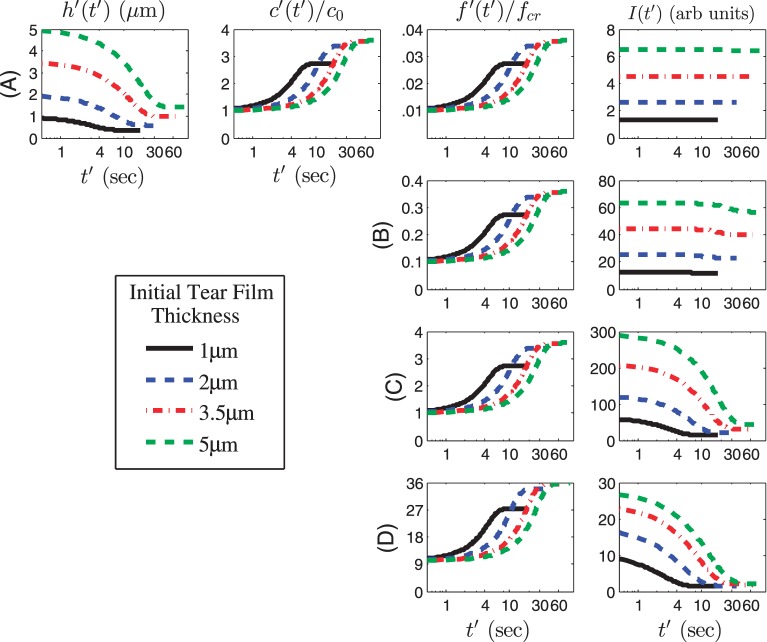
We now study the effect of initial fluorescein concentration on the time course of the dependent variables. We choose initial values of f ′(0) = 0.002%, 0.02%, 0.2% (critical concentration),^1,16 and 2% solution, which when normalized for our model yields f₀ = 0.01, 0.1, 1, and 10, respectively. Thus, we are using a wide range of initial concentrations that span the range from the dilute to the quenching regime,^16,24 with f = 1 indicating the critical normalized concentration. Thinning rates are v₀ = 2.5 μm/min (Fig. 4) and v₀ = 10 μm/min (Fig. 5), as before.²⁷ The permeability is P_f^tiss = 12 μm/s from King-Smith et al.³⁶ so that P_c = 1.55. Results are shown in Figures 4 and 5 using nonnormalized (dimensional) time, thickness, and intensity, and a logarithmic scale for the time axis (in seconds). The osmolarity and fluorescein concentration is given relative to the isosmotic and critical concentrations, respectively.

Figure 4.

Dimensional results for the dependent variables with a log scale for the dimensional time t′ axis (maximum value: 90 seconds). Row A: f₀ = 0.01. Row B: f₀ = 0.1. Row C: f₀ = 1. Row D: f₀ = 10. The results for h and c are identical for all four rows but only the first row is shown. Here v₀ = 2.5 μm/min and P_f^tiss = 12 μm/s, so that P_c = 1.55.

Figure 5.

Same as for Figure 4 except v₀ = 10 μm/min and P_f^tiss = 12 μm/s, so that P_c = 0.39.

In Figure 4, the tear film thickness and osmolarity change at the same rate for all initial tear film thicknesses, as tear film thinning due to evaporation occurs. As the tear film thins, fluorescein concentration increases proportionally to 1/h as is required for conservation of solutes. Thus, for all initial thicknesses, fluorescein concentration increases, but the changes in fluorescence intensity (I) depends on the proximity of the initial concentration to the critical concentration. Therefore, at the low concentrations of fluorescein in rows A and B (f₀ = 0.01 and 0.1, or 0.002% and 0.02% fluorescein, respectively), I does not change as the tear film thins, but there is a marked decrease in I once the critical concentration has been reached in the quenching regime in rows C and D (f₀ = 1 and 10, or 0.2% and 2% fluorescein, respectively). Note the strong dependence of I on the film thickness, not only the instantaneous value of h, but also the initial thickness d. The dependence on the initial thickness has been noted previously.²⁴ Figure 5 shows the same calculations, but with the increased thinning rate, v₀ = 10 μm/min, and associated P_c = 0.39. In this case, as expected, tear film thinning and associated increases in osmolarity and fluorescein concentration are more rapid and larger than in Figure 4. For the cases with d = 3.5 and 5 μm, the film thickness reduces by approximately a factor of four, so that the final osmolarity is approximately 1200 mOsM, and fluorescein concentration quadruples as well. The intensity shows some quenching with 0.02% fluorescein concentration (row B); 0.2% and 2% fluorescein (rows C and D, respectively) show clear quenching for all thicknesses.

Fluorescence Intensity During Thinning

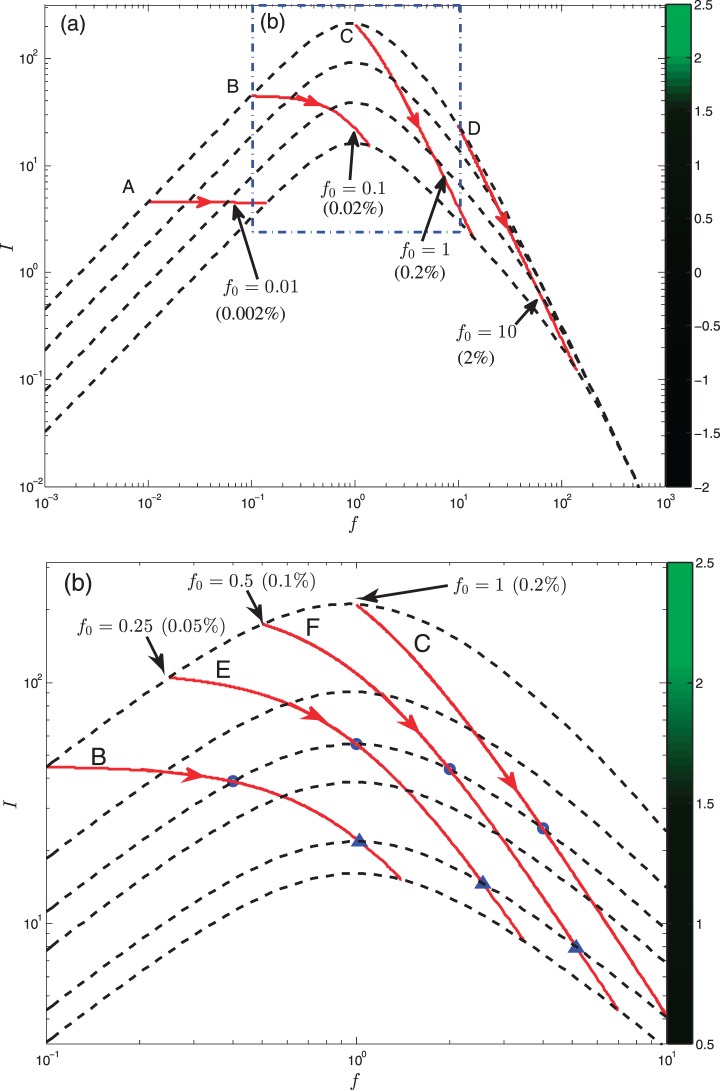
Figure 6a summarizes results of the model's predictions for changes in fluorescent intensity during tear film thinning for the same four initial normalized fluorescein concentrations as in Figures 4 and 5, which are labeled A through D. The difference between previous models (Fig. 1) and our model is that the prediction for fluorescence changes with tear film thinning. The dashed lines are fixed thickness (h) curves that represent increasing tear film thinning.^18,25 The red lines with arrows track changes in fluorescence intensity A through D with thinning (refer to color bar for intensity changes) for d = 3.5 μm and P_c = 0 with a thinning rate of 4 μm/min. When f is large enough (>10), then all of the dashed curves converge to the single curve I₀/(1 + f²) because the efficiency term's denominator is dominant in the expression for the intensity when in the high concentration quenching regime. The latter curve is only reached when P_c = 0 (no osmosis). When f is small (f₀ ≤ 0.01), there are no intensity changes with thinning.

Figure 6.

(a) Solution trajectories I(f) are shown as solid (red) curves, using different initial fluorescein concentrations f₀. The dashed curves are constant-h curves as in Figure 1 for several values of h (top is from Fig. 1). The (solid red) solution trajectories end on the dashed (constant h) curve for P_c = 0; the arrows indicate the direction of increasing time. (b) A zoom (blue box in [a]) on the solution trajectories I(f) for the thinning tear film near the critical concentration. The labels on the color bar are the logarithm of the intensity. Cases E and F are added to show the region near the critical concentration. The symbols indicate the steady states of the calculations on dashed (constant-h) curves with P_c = 0.361 (4 μm/min, circles) and P_c = 0.072 (20 μm/min, triangles), with P_f^tiss = 12 μm/s.

Figure 6b highlights results near the critical concentration for fluorescein (f₀ = 0.1, 0.25, 0.5, 1). While it appears that for f₀ = 0.25, for example, one will reach the quenching regime during some experiments with large concentration increase, quenching will not occur until later in the experiment and this may be for unrealistically long times. We conclude that it is wise to keep the initial concentration in the tear film at or above the critical concentration in order to stay in the quenching regime for evaporative thinning. This concentration is desirable clinically because it allows for initial high tear film fluorescence, but fluorescence decreases as the tear film thins owing to quenching.

The constant value of intensity that we calculate for small concentrations is in qualitative agreement with the in vivo measurements in the dilute regime shown in figure 3A of Nichols et al.²⁴ In that figure, the tear film thickness decreases rapidly (approximately 4 μm in 11 seconds), but the fluorescent intensity decreases only very slowly in the same time span. Our ideal model predicts exactly constant intensity, but some tangential flow and other effects may slightly change the intensity in vivo. In contrast, in the quenching regime, the measured intensity drops dramatically as the rapid thinning progresses in figure 3B of Nichols et al.²⁴ Our mathematical model predicts a similar result.

Discussion

This mathematical model greatly advances the small body of previous work on fluorescence quenching in the tear film,^1,16,24 by predicting dynamic changes in fluorescence intensity during tear film thinning and estimating concomitant increases in tear hyperosmolarity. Given the importance of tear film instability and hyperosmolarity to the dry eye condition,¹⁷ it provides an important link between thinning and local or spatial changes in tear film osmolarity. The model also allows a prediction of fluorescence changes, both above and below the critical concentration,^1,16 when the initial concentration of fluorescein is known or can be estimated.

The TBUT test involves instilling fluorescein dye into the eye, often with a moistened dye-impregnated strip.⁸ Using that system, an unknown amount of dye is instilled into a tear volume estimated as 7 μL,³⁹ but is actually unknown in each individual patient. Even when known volumes of dye are instilled, the final concentration of dye in the eye is unknown under most clinical conditions. For example, instillation of 1 μL 2% fluorescein into an eye with a 7-μL volume would lead to a concentration of 0.25% in eye,⁴⁰ which corresponds closely to curve C in Figure 6. Thus, in an eye with a normal tear volume, our model predicts that this concentration should fluoresce brightly upon instillation, but quench markedly as the tear film thins. This change in fluorescence provides the clinician with ample evidence of global or local tear thinning or breakup. However, if an increased concentration is instilled or if the tear volume is less, such as in dry eye,^41,42 initial fluorescence may be decreased and the tear film may undergo much more rapid quenching. Likewise, if a lesser concentration of dye is instilled, line B in Figure 6 shows that quenching may occur at a slower rate. These variables could affect judgment of TBUT and add to its variability as a clinical measure.⁴⁰ Although fluorescein dye is used commonly in clinical practice, quenching is rarely discussed in relation to tear film thinning or tear breakup. This model was designed to assist the development of fluorescence technology as a more quantitative technique than using time alone as a measure of tear instability.

During the process of tear breakup, dark areas appear in the fluorescent tear film and continue to darken until they appear black with a cobalt blue light source.⁸ Recently, it has been suggested that areas of tear breakup actually represent quenching of fluorescein dye, rather than an absence of aqueous.²⁴ If evaporation plays a major role in both tear film thinning and breakup,³⁵ then our model can be used to predict changes in fluorescence as the tear film thins in local areas of tear breakup. Thus, the model can be compared to experimental data for eye fluorescence to better understand the mechanism of tear breakup, which remains controversial.^43–46 Another potential clinical use for our model is to obtain an estimate tear volume among individual subjects. According to some reports, tear volume may be reduced markedly among dry eye patients.^41,42 Thus, if known volumes of fluorescein are instilled, the model could be further developed to predict initial tear film fluorescence and thus approximate individual tear volume.

In our model, we used fluorescence to model tear film osmolarity over the corneal surface, taking into account differences in concentration between the two solutes. Osmolarity of corneal compartment of the tear film⁴⁷ is clearly of interest in assessing ocular surface stress during tear instability,¹⁷ but, unfortunately, it cannot be measured directly with current technology.^18,19 Recently, it has been suggested that local changes in tear film osmolarity over the cornea could reach 800 to 900 mOsM during extended interblink intervals,²⁰ and ocular surface sensory measures link burning and stinging with tear breakup and thinning.^48,49 These data strongly imply local or global increases in hyperosmolarity of the tear film over the cornea. Our model allows an estimation of hyperosmolarity from experimental fluorescein images of tear film breakup and thinning, which may increase understanding of the stress that tear film hyperosmolarity may provide to the underlying corneal surface in the interblink interval.^17,22,23

Any mathematical model is limited by its assumptions and often simplifies complex interactions to generate predictions. For example, although a lipid layer was not explicitly present in this model, its effect was taken into account by setting evaporation rates to match in vivo thinning rates.²⁸ Similarly, we included an implicit mucin layer (van der Waals term),³² which halts thinning at the thickness of the glycocalyx.^33,34 Furthermore, this model is based on evaporation as the mechanism involved in tear film thinning,³⁵ but other forces, such as tangential flow, are not treated in this initial model.³¹ In addition, we assume a constant evaporation rate and do not include changes in evaporation that may occur by external conditions such as the relative humidity or wind.²⁸ Since our goal is to study the dynamics of thinning in controlled indoor conditions, as may occur during experimental conditions, we believe that this limited treatment of evaporation is acceptable here.

Another assumption of this model is that it assumes that the cornea is impermeable to fluorescein. While this may be very close to reality under normal conditions, in which approximately 0.002% fluorescein penetrates the epithelium,³⁷ it may not provide an accurate representation of an abnormal cornea with diminished barrier function.⁵⁰ The model does illuminate the dynamics of the osmolarity, fluorescein concentration, and fluorescence intensity during the thinning process, and illustrates more clearly than previous work where the dilute and quenching regimes are obtained during the evaporative thinning process.

A limitation of the fluorescence approach in studying the tear film is the fact that the concentration of fluorescein is usually unknown. To reach the dilute regime in their experiments, Nichols et al.²⁴ have measured each subject's tear film after a 2-minute rest period following an initial instillation of 1-μL drop of 2% solution into the eye. Because of the rest period, the tear turnover helps to lower the fluorescein concentration into the dilute regime. Immediate measurement of tear film fluorescence after instillation of fluorescein offers a better chance of having a good estimate of the fluorescein concentration in clinical settings, particularly for the quenching regime.

Appendix A

Derivation and Normalization of the Model

The equations for the tear film thickness, as well as conservation of osmolarity (ions) and fluorescein, are as follows.

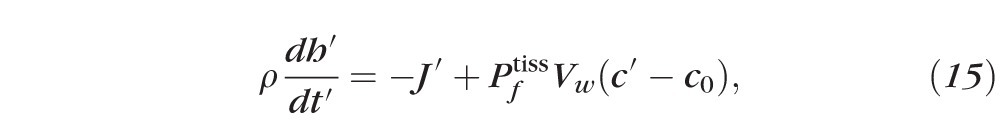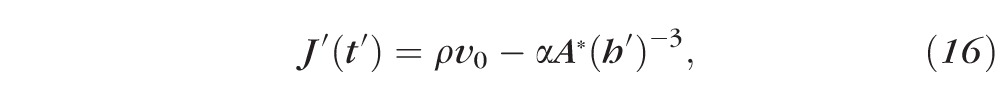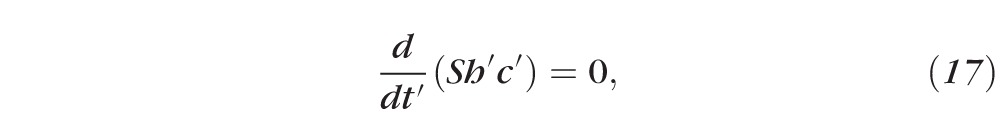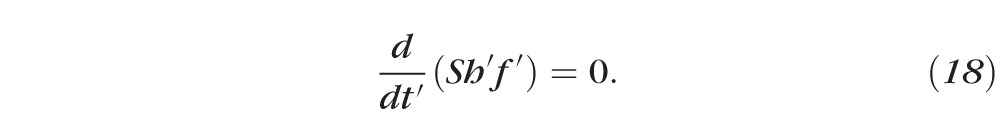Primes denote dimensional (nonnormalized) quantities. These equations result from conserving water and solutes. For example, Equation 15 has three terms representing, from left to right, the rate of change of the mass of water per unit area inside the tear film, the rate of mass of water per unit area leaving the air/tear interface via evaporation, and the rate of mass of water per unit area entering the tear film at the tear/epithelium interface owing to osmosis. Here the evaporative mass flux J′(t′) is simplified from that used previously^31,32,51; the term ρv₀ is determined by experimentally measured thinning rates.²⁷ The pressure at the free surface is given by the van der Waals term A*(h′)⁻³, and this term will prevent complete dewetting in the absence of osmosis from the cornea.³² This term is used to represent the wettable cornea and the fact that the glycocalyx strongly attracts water.³⁴ Equations 17 and 18 are conservation of mass for osmolarity and fluorescein, with S representing a constant unit area of the epithelium. The dimensional parameters are given in Table A1.

Table A1.

Dimensional Parameters Used in the Model*

Parameter	Value	Quantity
d	1, 2, 3.5, 5 μm	Tear film thickness52
v0	2.5, 10 μm min−1	Initial thinning rate27
ρ	1000 kg m−3	Aqueous layer density53
Vw	18 cm3/mol	Molar volume of water
A*	(1 × 10−17 m3 Pa)	Hamaker constant32
α	1.87 × 10−2 m2 K Pa−1	Evaporation pressure coefficient32
Pftiss	≤12 μm/s	Corneal permeability36,54
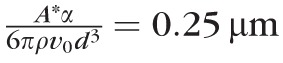
h′eq		Equilibrium film thickness32
c0	300 mOsm or 0.54 mol%	Isosmolar concentration19
Mf	376 g/mol	Molecular weight of sodium fluorescein55
ε	7.6 × 104 cm−1 M−1	Molar extinction coefficient55
fcr	0.2%	Critical fluorescein concentration24
f0	0.01%–10%	Initial fluorescein concentration24

^* The parameters α and A* were estimated. They are only needed in combination to get h_eq = 0.25 μm in this work.

We may integrate the mass conservation equations (Equations 17 and 18) with respect to time (t′), then divide out the common factor S to obtain:

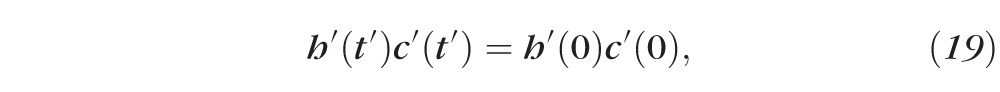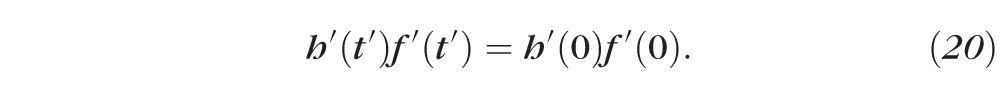Using the initial conditions, and normalizing, gives Equations 3 and 4. Applying the normalizations to Equations 15 and 16 gives Equations 1 and 2.

Appendix B

Parameter Values

The flow resistance of the corneal epithelium, in the notation and units of Fatt and Weissman,³⁰ may be given as R_t = R_gcT_eye/(P_f^tissS_cV_w) = 8.06 × 10¹¹ dyn·s/cm. Here we have used the following: S_c = 1.47 cm² is the corneal surface area,⁵⁶ T_eye = 308 K is the ocular surface temperature,^57,58 and R_gc = 8.314 × 10⁷ dyn·cm/mol/K is the universal gas constant. We note that R_t here is larger than the animal model estimate of Fatt and Weissman³⁰ (chapter 6), so that the rate of osmosis is smaller than their estimate. The normalization of the equations results in the nondimensional parameters given in Table B1.

Table B1.

Representative Nondimensional Parameters That Are Used Unless Otherwise Noted*

Parameter	Definition	d
		5 μm	3.5 μm	2 μm	1 μm
heq	h′eq/d	0.05	0.0714	0.125	0.25
Pc	PftissVwc0/v0	1.55	1.55	1.55	1.55
φ	εfcrd	0.202	0.141	0.0808	0.0404

^* These parameters are for a thinning rate of v₀ = 2.5 μm/min and P_f^tiss = 12 μm/s. If v₀ = 10 or 20 μm/min, then P_c = 0.388 or 0.194, respectively.