In vivo measurements of the effect of whole body vibration on spinal loads

Abstract

Purpose

It is assumed that whole body vibration (WBV) improves muscle strength, bone density, blood flow and mobility and is therefore used in wide ranges such as to improve fitness and prevent osteoporosis and back pain. It is expected that WBV produces large forces on the spine, which poses a potential risk factor for the health of the spine. Therefore, the aim of the study was to measure the effect of various vibration frequencies, amplitudes, device types and body positions on the loads acting on a lumbar vertebral body replacement (VBR).

Methods

Three patients suffering from a fractured lumbar vertebral body were treated using a telemeterized VBR. The implant loads were measured during WBV while the patients stood on devices with vertically and seesaw-induced vibration. Frequencies between 5 and 50 Hz and amplitudes of 1, 2 and 4 mm were tested. The patients stood with their knees straight, slightly bent, or bent at 60°. In addition, they stood on their forefeet.

Results

The peak resultant forces on the implant increased due to vibration by an average of 24 % relative to the forces induced without vibration. The average increase of the peak implant force was 27 % for vertically induced vibration and 15 % for seesaw vibration. The forces were higher when the legs were straight than when the knees were bent. Both the vibration frequency and the amplitude had only a minor effect on the measured forces.

Conclusions

The force increase due to WBV is caused by an activation of the trunk muscles and by the acceleration forces. The forces produced during WBV are usually lower than those produced during walking. Therefore, the absolute magnitude of the forces produced during WBV should not be harmful, even for people with osteoporosis.

Introduction

In gyms, physiotherapy practices and even in some private homes, whole body vibration (WBV) platforms are used at various intensities and frequencies. There are two main WBV platform types: one that moves both feet up and down synchronously, e.g., Power Plate, and another that induces seesaw vibration, e.g., Galileo. WBV is assumed to improve, among other things, muscle strength, bone density, blood flow and mobility [1–4]. The focuses during therapy are on preventing or reducing osteoporosis [5, 6], on muscle training [7] to prevent falls and the resulting bone fractures [8], and on the prevention of back pain [9]. WBV activates muscles and may lead to rapid accelerations of body segments [6, 10]. Therefore, it is often expected that large forces are acting on the spine and on the joints of the lower extremities during WBV. However, little information exists regarding the actual spinal loads during WBV.

Pel et al. [11] measured the acceleration at the levels of the ankle, knee and hip in eight healthy volunteers while they stood in a defined squat position on a Power Plate and a Galileo platform that were operating at a frequency of 25 Hz. They also measured the accelerations of the unloaded platforms. With increasing vibration frequency, they observed a large increase in the vertical platform acceleration. The maximum values were 8g for the Power Plate and 15g for the Galileo platform. The authors calculated the transmission of vibration in the vertical direction as a percentage of the measured acceleration divided by the unloaded platform acceleration. They found that the Power Plate and the Galileo platform transmitted 55 and 85 % of the vibration to the ankle, 9 and 8 % to the knee and only 3 and 2 % to the hip, respectively.

Pollock et al. [10] studied the effect of frequency and amplitude on the muscle activity and accelerations throughout the body during WBV. They found an increase in muscle activities of 5–50 % of the maximal voluntary contractions, with the greatest increase in the lower legs. Depending on the frequency and amplitude, the accelerations ranged from 0.2 to 9g. The measured accelerations decreased with the distance from the platform. At the toe and ankle, the acceleration increased linearly with the vibration frequency; at the knee, it increased with frequencies up to 15 Hz and decreased thereafter. The authors reported a trend toward greater acceleration at the toe, knee and head during high amplitude vibration.

An increase in the tensile muscle forces is necessarily accompanied by an increase in the compressive forces in the joint bridged by the muscles. Increased EMG activities during WBV would therefore inevitably cause increased contact forces on the hip and knee joint, as well as on the spine. Dynamic accelerations during WBV also increase the loads in the skeleton. However, as was shown by Pollock et al. [10], the various body parts experience particular accelerations. Therefore, predicting the spinal load increase due to dynamic accelerations and muscle activities is not an easy task. In addition, WBV changes the stiffness of the muscles, which may affect the damping properties and consequently the spinal load.

A telemeterized vertebral body replacement (VBR) allows in vivo measurement of the loads [12]. It has been used in several studies, e.g., to determine the loads for various sitting positions, during WBV in a car-driving simulation, while lifting and lowering weights at various levels, and to determine the effect of a brace [13–15].

The aim of this study was to determine the effect of the vibration frequency, amplitude, WBV platform type, and body position on the loads acting on a VBR during WBV. We expected a force increase of approximately 50 % due to vibration, that the device type would have only a minor effect on the forces, and that the vibration frequency and amplitude, as well as the body posture, would have a strong influence on the forces.

Methods

Instrumented vertebral body replacement and patients

To measure the spinal loads in vivo, a clinically proven implant (Synex, Synthes Inc., Bettlach, Switzerland) has been modified. Six strain gauges, a 9-channel telemetry unit and a coil for the inductive power supply were integrated within a hermetically sealed cylindrical tube. After extensive calibration, the implant can be used to measure all six of the load components acting on it. The average errors were lower than 2 % for the force and 5 % for the moment components, related to the maximum applied force (3,000 N) and moment (20 Nm). The sensitivity of the measuring implant is less than 1 N and 0.01 Nm. More detailed information on the telemeterized VBR can be found elsewhere [12].

Instrumented VBRs were implanted in five patients with A3-type compression fractures [16] who were aged 62–71 years. Each patient had a fracture of either the vertebral body L1 or L3. The fracture was first stabilized using an internal spinal fixation device, which was implanted from the posterior. In a second surgery, parts of the fractured vertebral body and of the adjacent discs were removed, and the telemeterized VBR was inserted into the corpectomy defect. To enhance fusion of the adjacent vertebrae, autologous bone material was added to the VBR. Three of the patients agreed to participate in the WBV study. Data on these patients and the surgical procedures are provided in Table 1.

Table 1.

Data on patients and surgical procedure

	Patient
	WP1	WP4	WP5
Sex	Male	Male	Male
Age (years)	62	63	66
Height (cm)	168	170	180
Weight (kg)	66	60	63
Fractured vertebra	L1	L1	L3
Level of internal fixation device	T12–L2	T11–L3	L2–L4
Bone material added	Yes	Yes	Yes
Time between implantation and measurement (months)	65	49	43

For the inductive power supply during the measurements, a coil was placed around the trunk of the patient at the level of the implant. A loop antenna, placed at the patient’s back, received the load-dependent telemetry signals and transmitted them to a notebook, in which the forces and moments were calculated and displayed in real time. The patients were videotaped during the measurements and the telemetry signals were stored on the same videotape. The telemetry and the external equipment have been described in detail elsewhere [17].

The Ethics Committee of our hospital approved the clinical implantation of telemeterized implants into the patients. All patients gave their written consent to undergo implantation of the instrumented VBR, subsequent load measurements, and publishing of their images.

Whole body vibration platforms

Two types of WBV platforms were used: The Power Plate ‘Pro 5’ (Performance Health Systems, Northbrook, IL, USA) creates vibrations between 25 and 50 Hz via an up-and-down movement of the platform with amplitudes of approximately 1 or 2 mm.

The Galileo Advanced (Novotec Medical GmbH, Pforzheim, Germany) oscillates up and down between 5 and 30 Hz around a central axis, producing a seesaw vibration [18]. This platform causes one leg to go up while the other goes down. The amplitude depends on the position of the feet on the platform. It is small when the feet are near the axis (position 1) and large when they are near the edge of the platform (position 4). The amplitudes for foot positions 1–4 are approximately 1, 2, 3 and 4 mm, respectively [19].

Sinusoidal platform motions and accelerations can be assumed for both platform types [11]. An acceleration of up to approximately 100 m/s² or 10g may occur at the surface of the Galileo platform [19].

Measurements and exercises

During measurements on both platforms, the patients stood on both legs and loaded their feet symmetrically. The investigated four postures were:

1. with the knees straight

2. with the knees slightly bent

3. with the knees 60° flexed

4. on the forefeet.

On the Power Plate, these four postures were investigated at 25 Hz with amplitudes of 1 mm (Table 2; exercises 1a–d) and 2 mm (exercises 2a–d).

Table 2.

Studied exercises

Exercise no.	WBV device	Amplitude (mm)	Frequency (Hz)	Posture
1a	Power Plate	1	25	Knees straight
1b	Power Plate	1	25	Knees slightly bent
1c	Power Plate	1	25	Knees 60° flexed
1d	Power Plate	1	25	On forefoot
2a	Power Plate	2	25	Knees straight
2b	Power Plate	2	25	Knees slightly bent
2c	Power Plate	2	25	Knees 60° flexed
2d	Power Plate	2	25	On forefoot
3a	Galileo	2	25	Knees straight
3b	Galileo	2	25	Knees slightly bent
3c	Galileo	2	25	Knees 60° flexed
3d	Galileo	2	25	On forefoot
4b	Galileo	4	25	Knees slightly bent
5b	Galileo	2	12.5	Knees slightly bent
6b	Galileo	4	12.5	Knees slightly bent
7b	Galileo	2	5–25–5	Knees slightly bent

WBV whole body vibration

On the Galileo platform measurements in the four postures were taken at 25 Hz with 2 mm amplitude (Table 2; exercises 3a–c). Measurements in posture (b) were repeated at 25 Hz/4 mm (4b), at 12.5 Hz/2 mm (5b) and 12.5 Hz/4 mm (6b). Furthermore, the vibration frequency was increased from 5 to 25 Hz and later decreased to 5 Hz with 2 mm amplitude and the knees slightly bent (7b).

Before the vibration started, the patients stood a few seconds on the platform in the desired position while the implant loads were recorded. The vibration was then switched on for 12–15 s. Exercise 7b lasted approximately 60 s. Between all exercises the patient stood relaxed for 10–30 s. However, the break was approximately 5 min long when the device was changed. To avoid overstressing the patients, each exercise was only measured once.

Evaluation

If not stated otherwise, the resultant force acting on the VBR is presented here. It is geometric sum of the three measured force components. The maximum force during a loading cycle was defined as the ‘peak force’, and the difference between the maximum and minimum force magnitude during vibration as the ‘force range’. To calculate the ‘mean force’ during vibration, the filtfilt function of Matlab (MathWorks, Ismaning, Germany) was used. In this function, the dataset was filtered forwards and backwards. Fast-Fourier transformations were applied to choose the cutoff frequencies of the bandpass filter for the various vibration frequencies of the devices.

The force on the VBR strongly depends on the position of the center of mass of the upper body and consequently on the posture [15]. Therefore, increases in the peak and mean forces during vibration were calculated in percentage relative to the values for the corresponding posture without vibration.

Results

Effect of whole body vibration

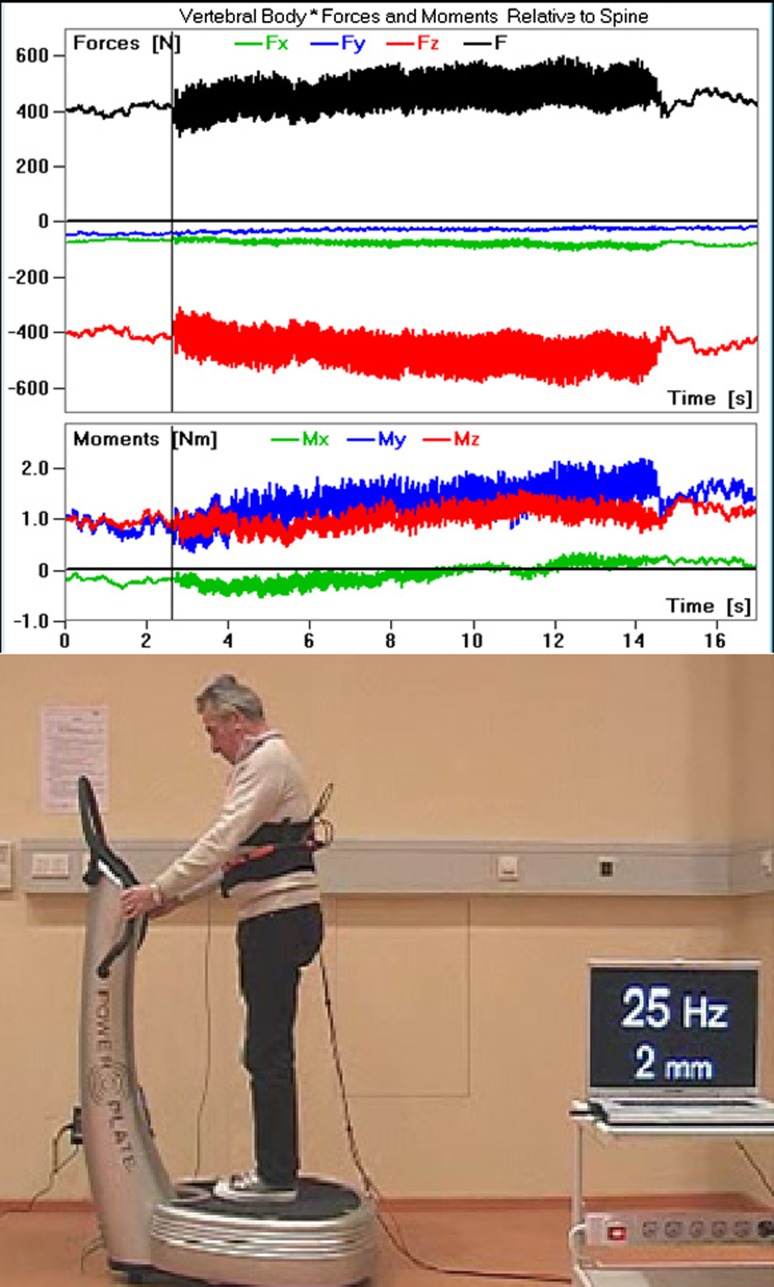
Figure 1 shows the six load components and the resultant force on the VBR when standing on the Power Plate platform with straight knees and a vibration amplitude of approximately 2 mm (exercise 2a). The vibration produced dynamic changes of all six load components and the resultant force. The peak forces during vibration were always higher than the force without vibration.

Fig. 1.

Measured load components and the resultant force F when patient WP1 was standing on the Power Plate platform with straight knees. Vibration was switched on between approximately 2.5 and 14.5 s. The amplitude was approximately 2 mm (exercise no. 2a)

In general, WBV with amplitudes of 1 or 2 mm caused an average peak force that was 24 % higher than the force for standing in the same posture without vibration. However, force increases of more than 40 % were also sometimes observed. The mean force during WBV increased by an average of only 8 % (maximum value 14 %), compared to the same posture without vibration.

Effect of frequency

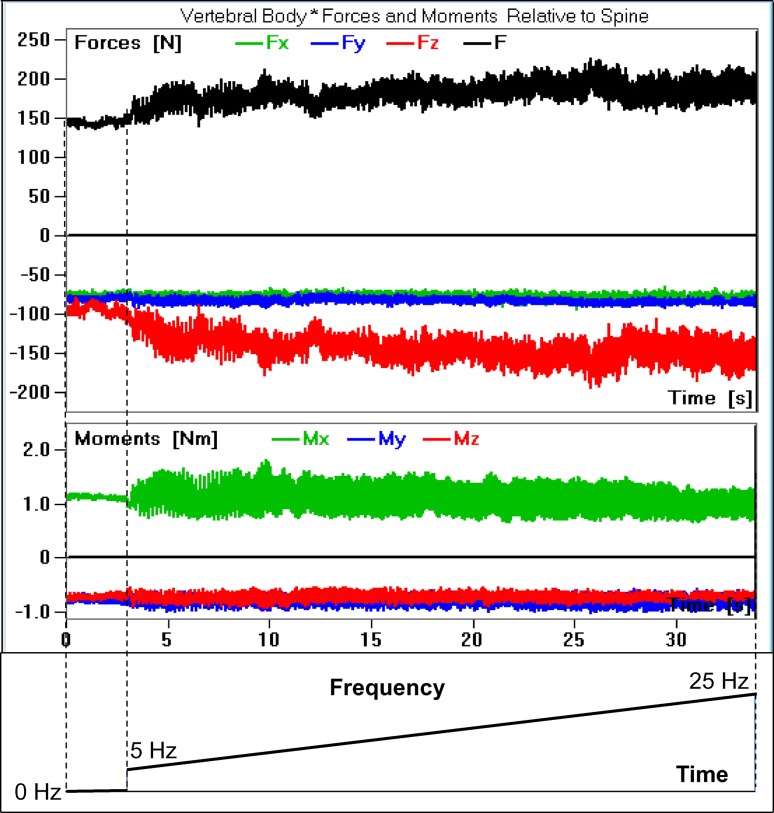
A frequency increase from 5 to 25 Hz (exercise 7b) had only a minor effect on the implant loads (Fig. 2). This effect is true for all six of the load components.

Fig. 2.

Measured load components and the resultant force F when patient WP5 was standing on the Galileo platform with slightly bent knees. The amplitude was approximately 2 mm. The vibration was switched on after approximately 3 s. The frequency was increased from 5 to 25 Hz (exercise no. 7b)

Effect of posture

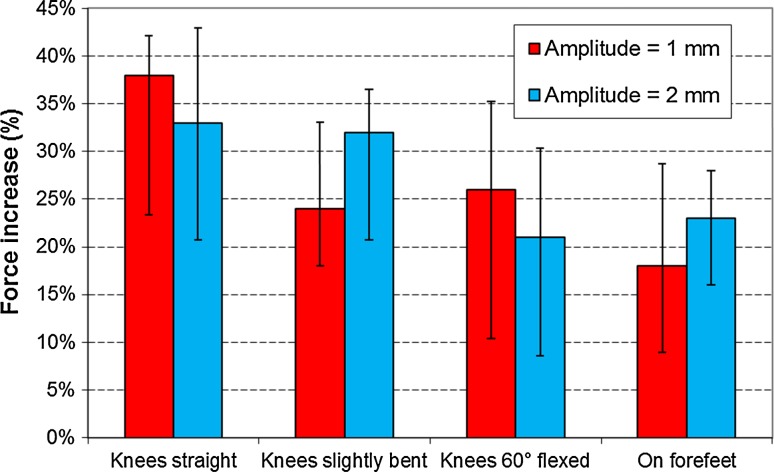
The posture (exercises 1 and 2, a–d) had an effect on the implant forces. As expected, the greatest force increases were measured with the knees straight (Fig. 3). When the knees were bent, their flexion angle had only a minor effect on the measured force increase. Standing on the forefeet caused a similar force increase to that observed standing with the knees bent. The difference between the peak and mean-force increases was on average approximately 25 % for standing with straight knees and 12 % for the other positions.

Fig. 3.

Effect of the position on the average peak-force increase due to vibration when standing on the Power Plate platform (exercise nos. 1 and 2, a–d). The frequency was 25 Hz. Medians and ranges for the three patients are shown

Comparison of the force increases for the two platform types

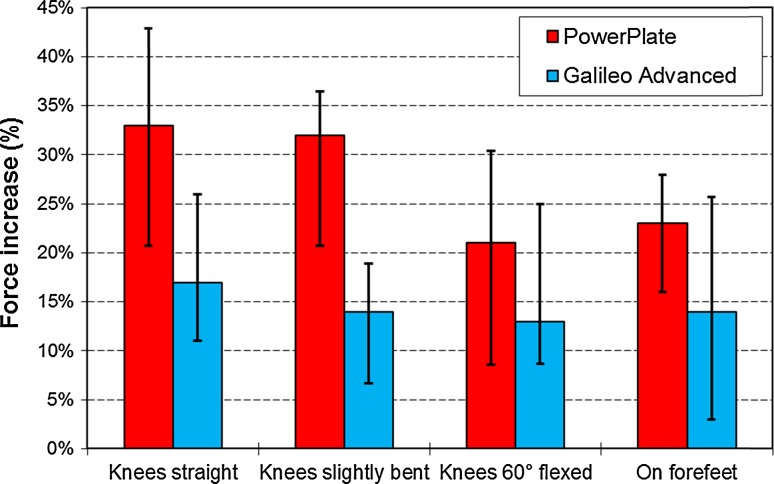
The force increase on the VBR was higher for the Power Plate platform with vertical vibration than for the Galileo platform with seesaw vibration. The average increase of the peak force for all exercises was 27 % for the Power Plate and 15 % for the Galileo platform (Fig. 4). The increase of the mean force due to vibration was 12 % for the Power Plate and 4 % for the Galileo platform.

Fig. 4.

Average peak-force increase due to vibration for standing in various positions (exercise nos. 2 and 3, a–d). The results of the Power Plate platform are compared to those of the Galileo platform. The vibration amplitude was 2 mm and the frequency was 25 Hz

Effect of vibration amplitude

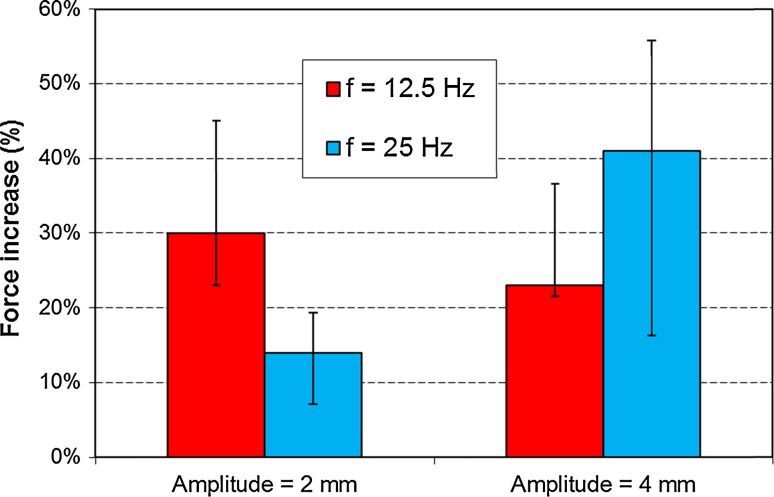
The vibration amplitude had only a minor effect on the increase of the peak force (Figs. 3, 5). In some cases, the force increase was even higher for the smaller vibration amplitudes. However, the force-range variation during vibration increased nearly linearly with the increasing vibration amplitude. The absolute values of the range varied for each patient. They also depended on the posture and were highest when the patient stood with straight knees.

Fig. 5.

Average peak-force increase due to vibration for two frequencies and amplitudes. The patients were standing on the Galileo platform with the knees slightly bent (exercise nos. 3d and 4b–6b)

Discussion

The loads on a telemeterized VBR were measured when the patients were standing in various postures on the WBV platforms and experienced vibrations of various frequencies and amplitudes.

WBV yielded small increases of the measured forces in the lumbar spine. The average peak-force increase of 24 % due to vibration represents only half of the expected value. During WBV, the muscle-activity increases, and the dynamic accelerations increase the weights of the body segment. Both effects increase the forces transmitted by the large joints and the vertebrae. The accelerations of the body segments decrease as the distance from the surface of the vibration platform increases. Pel et al. [11] observed that only 2–3 % of the acceleration at the platform reaches the hip. In other words, the vibration is mainly damped in the legs. The vibration experienced by the upper or middle lumbar spine, where the VBR is implanted, is even smaller and should therefore have only a minor effect on the force in that region. The muscle-activity increase during WBV varies strongly, depending on the muscle. Pollock et al. [10] measured values ranging from 5 to 50 % of the maximal voluntary contraction for muscles in the leg and hip region. The increased muscle activity is most likely reflected in the increase of the mean force, whereas the difference between the mean and peak forces is mainly due to accelerations of the upper body mass. However, the effect of the muscle activity during WBV is much lower than during stumbling. Bergmann et al. [20] observed an increase of the resultant force in the hip joint of approximately 150 % relative to the corresponding value for walking when patients stumbled without falling. During stumbling, all the muscles in the lower extremities are most likely activated to stabilize the body. Our results suggest that during WBV, co-contraction of the antagonistic muscles only plays a minor role.

The relatively small increases of the resultant force fit with the small accelerations measured at hip level [11] and the measured muscle-activity increase in the lower leg [10]. The magnitude of the measured force during WBV is usually less than that during walking, which is a crucial activity with high spinal loads.

The measured absolute implant forces varied strongly between individuals in this study. This finding is in agreement with those of previous load-measuring studies [13, 14, 21, 22]. Sato et al. [22], for example, measured the intradiscal pressure in eight healthy volunteers aged between 22 and 29 years and observed upright standing values between 215 and 747 kPa. The posture has a strong influence on the results. Therefore, small changes in the posture lead to large variations in the measured forces. For example, a change of the inclination angle of the upper body by 5° produces a change of the resultant implant force of approximately 16 %, and moving the head from a neutral to a flexed position increases the resultant implant force in a patient by approximately 13 % [15].

The variation of the vibration frequency between 5 and 25 Hz had only a minor influence on the measured force increases (Figs. 2, 5). This finding was unexpected. The resonance frequency of certain organs may fall within the studied frequency range, but this fact clearly has only a negligible effect on the spinal load. The small changes in the relative force increase are mainly due to small variations in the posture.

Straight knees led to the highest increase of the force on the VBR and the highest force range during vibration. When the knees are bent or when standing on the forefeet, the damping of the vibration should be higher and, consequently, the measured peak forces lower. Surprisingly, the knee flexion angle (slightly bent or 60° flexed) had only a minor effect on the relative spinal load increases. However, a knee flexion angle of 60° is usually accompanied by a large flexion of the upper body, which leads to higher spinal loads.

Standing on the Power Plate platform with vertical vibration produced greater VBR forces than did standing on the Galileo platform with seesaw vibration (Fig. 4). The medians of the peak and mean-force-increase values for the various postures were approximately twice as high for the Power Plate platform compared to the Galileo system. The up-and-down movement of the Power Plate platform produces a similar up-and-down movement of the center of mass of the patient. Conversely, the seesaw vibration of the Galileo system does not necessarily cause an up-and-down movement of the center of mass. Therefore, the higher forces for the Power Plate platform are likely due to mass inertia.

Surprisingly, the amplitude of the vibration did not have a clear effect on the measured peak forces. One would expect that the forces would increase with the vibration amplitude, but the opposite was often observed (Figs. 3, 5). However, the force-range changes during vibration increased as the vibration amplitude increased. The large variation of the results is mainly caused by small changes in the posture, both when the vibration started and during the vibration. In elderly patients, not all of the parameters can be controlled.

The study has some limitations. Only a small cohort of three patients with a unique telemeterized VBR was available. Therefore, only descriptive statistics could be performed. The patients were between 67 and 71 years old at the time of the measurements. Therefore, only a limited number of exercises could be studied, particularly because the patients were also involved in several additional load-measuring studies. The patients were not accustomed to standing on a vibration platform. This new situation most likely led to an increased muscle tonus and therefore higher forces. After switching on and during the vibration, the patients often slightly shifted their posture (e.g., the flexion angle of the knees, the position of the head, and the inclination of the upper body), which affected the measured forces. For the most part, these small changes could only be detected in the video in a fast motion mode. There was more often a trend toward slightly higher than toward lower forces with increasing vibration time during an exercise.

Conclusions

The average peak force on a VBR increases by approximately 24 % during WBV compared to the same posture without vibration. The maximum force during WBV is mostly less than that for walking. Therefore, the magnitude of the force during WBV should not be harmful, even for people with osteoporosis. Bending the knees or standing on the forefeet leads to lower force increases relative to standing with straight legs. The Power Plate platform caused a higher force increase than did the Galileo platform.