Design of chiral ligands for asymmetric catalysis: From C₂-symmetric P,P- and N,N-ligands to sterically and electronically nonsymmetrical P,N-ligands

Abstract

For a long time, C₂-symmetric ligands have dominated in asymmetric catalysis. More recently, nonsymmetrical modular P,N-ligands have been introduced. These ligands have been applied successfully in various metal-catalyzed reactions and, in many cases, have outperformed P,P- or N,N-ligands.

Most asymmetric catalysts that have been developed so far are metal complexes with chiral organic ligands. The chiral ligand modifies the reactivity and selectivity of the metal center in such a way that one of two possible enantiomeric products is formed preferentially. Based on this concept, many metal complexes have been found that catalyze various reactions with impressive enantioselectivity. Despite impressive progress in this field, the design of suitable chiral ligands for a particular application remains a formidable task. The complexity of most catalytic processes precludes a purely rational approach based on mechanistic and structural criteria. Therefore, most new chiral catalysts are still found empirically, with chance, intuition, and systematic screening all playing important roles. Nevertheless, for certain reactions such as Rh-catalyzed hydrogenation (1, 2) or Pd-catalyzed allylic substitution (3, 4), the mechanism is known, allowing at least a semirational approach to catalyst development. Moreover, useful general concepts have been developed during the last three decades that greatly facilitate the development of new chiral ligands, even in the absence of mechanistic information. Some of these concepts are described in the following sections, mainly from the perspective of our own research.

C₂-Symmetric Ligands

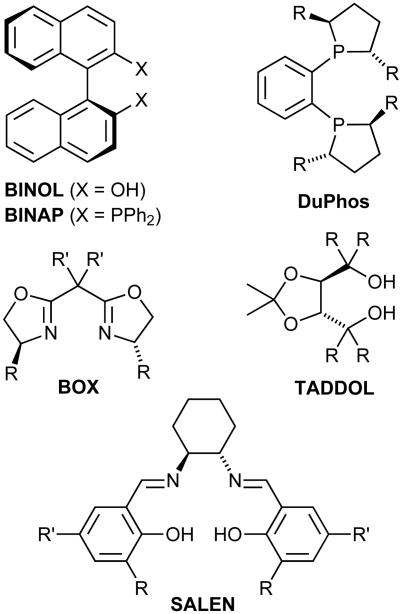
Of the thousands of chiral ligands prepared so far, a relatively small number of structural classes stand out because of their broad applicability. These “privileged ligands,” as they may be called (5), allow high levels of enantiocontrol in many different metal-catalyzed reactions. A survey of their structures reveals that, at first sight, a surprisingly large number of them possess C₂ symmetry (Fig. 1).

Fig. 1.

Privileged ligand structures.

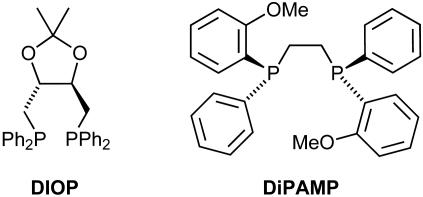
The C₂-symmetric ligand DIOP (Fig. 2) was introduced by Dang and Kagan in 1971 (6). The reason for choosing a C₂-symmetric ligand with two equivalent P atoms was to reduce the number of possible isomeric metal complexes, as well as the number of different substrate–catalyst arrangements and reaction pathways, when compared with a nonsymmetrical ligand. This consequence of C₂ symmetry can have a beneficial effect on enantioselectivity because the competing less-selective pathways are possibly eliminated. Because fewer reaction intermediates must be taken into account, C₂ symmetry is of particular advantage in mechanistic studies because it facilitates analysis of the ligand–substrate interactions that may be responsible for enantioselection.

Fig. 2.

Kagan's DIOP and Knowles' DiPAMP ligands.

The design principles that led Dang and Kagan to this ligand had a marked influence on the course of research in asymmetric catalysis, and many diphosphine ligands that were introduced subsequently were patterned after DIOP. Knowles (7), for example, prepared a dimeric analogue of one of his previously synthesized monophosphines, which he termed DiPAMP (Fig. 2). Based on this ligand, he developed an industrial catalytic asymmeric process, an Rh-catalyzed hydrogenation of a dehydro-amino acid derivative, used as the key step in the production of 3,4-dihydroxy-l-phenylalanine (l-Dopa). Since then, the concept of C₂ symmetry has led to many further highly efficient diphosphines, such as BINAP (8) and DuPhos (9), and it has been applied successfully to other ligand classes with coordinating N or O atoms (Fig. 1) (5, 10–13).

We, too, were attracted by the advantages and aesthetics of C₂ symmetry when we introduced the semicorrins (Fig. 3) as a new type of ligand. To our delight, these ligands gave excellent results in the Cu-catalyzed cyclopropanation of olefins and Co-catalyzed conjugate reduction of α,β-unsaturated carboxylic acid derivatives (10). The planar π-system and the two five-membered rings confine the conformational flexibility, which simplifies a prediction of the 3D structure of semicorrin metal complexes. The two substituents at the stereogenic centers are positioned in close proximity to the coordination site, and they shield the metal center from two opposite directions (Fig. 3). Therefore, these substituents are expected to have a strong direct effect on a reaction taking place in the coordination sphere.

Fig. 3.

Structure of a semicorrin metal complex.

Variation of the semicorrin structure led to analogous bisoxazoline (BOX) ligands; Fig. 1).† Ligands of this type were reported independently by research groups in 1990–1991 (14–21), and since then they have been established as one of the most versatile ligand classes for asymmetric catalysis (10, 11). Bisoxazolines are attractive because various derivatives can be readily prepared from simple amino alcohols as chiral precursors, allowing the ligand to be tailored to a specific catalytic process. It is unrealistic to expect that one particular ligand will exert perfect enantiocontrol in many reactions for many different substrates. Therefore, it is crucial that the synthesis is flexible and simple, to allow structural optimization of a ligand for a particular application.

C₂ Versus C₁ Symmetry

Although the concept of C₂ symmetry has been very successful, there is no fundamental reason why C₂-symmetric ligands should necessarily be superior to their nonsymmetrical counterparts. In fact, efficient nonsymmetrical ligands have been found that in some reactions give even higher enantioselectivities than the best C₂-symmetric ligands. Moreover, convincing arguments can be made for certain reactions as to why nonsymmetrical ligands with two electronically and sterically divergent coordinating units should, in principle, permit more effective enantiocontrol than C₂-symmetric ligands.

Asymmetric hydrogenation with Rh catalysts provides an instructive example. As pointed out by Achiwa and coworkers (22), the intermediates in the catalytic cycle are nonsymmetrical and, consequently, the two phosphine groups interact with a metal-bound substrate in an electronically and sterically different manner. In a substrate complex (Fig. 4), the interaction between P^cis and the coordinated substrate is primarily steric in nature, whereas P^trans exerts mainly an electronic effect (electronic effects of a ligand are transmitted preferentially to the trans-coordination site). Similar arguments can be given for other intermediates in the catalytic cycle. Because the two phosphine groups influence the reactivity and selectivity of the metal catalyst in different manners, their structures should be optimized individually, to obtain a perfect ligand.

Fig. 4.

Achiwa's hydrogenation studies with desymmetrized DIOP.

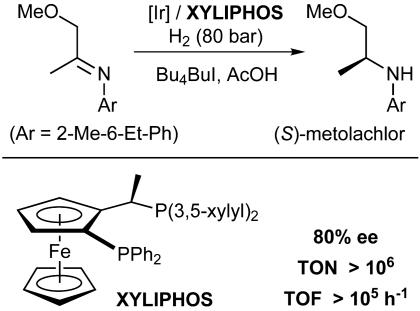
Achiwa and coworkers have illustrated this by desymmetrizing the DIOP ligand (Fig. 4). Indeed, replacing one of the diphenylphosphine units by a more electron-rich dicyclohexylphosphino group resulted in a significant increase of both catalyst activity and enantioselectivity. Although this strategy of desymmetrizing C₂-symmetric ligands appears to be straightforward, it does not necessarily guarantee an improvement of the performance of the catalyst. If catalytically active, isomeric metal complexes are formed in which the coordinating atoms (e.g., P^cis and P^trans of ligand B; Fig. 4) have exchanged positions, all efforts to individually optimize the coordinating groups are futile. However, if this problem can be avoided (which is often difficult), the results may be spectacular, as illustrated by the industrial synthesis of the important herbicide metolachlor (Fig. 5) (23). The key step, an Ircatalyzed imine hydrogenation, was improved dramatically by systematic variation of the individual substituents at the P atoms. In contrast to applications in the pharmaceutical sector, extremely high enantioselectivity was not required here. The crucial issues in this case were the turnover number and rate. Under carefully optimized conditions with the chiral ligand XYLIPHOS, more than a million turnovers and extremely high rates could be achieved in very concentrated solution, making this commercial process highly attractive.

Fig. 5.

Industrial process for the production of (S)-metolachlor.

From C₂-Symmetric Bisoxazolines to Nonsymmetrical P,N-Ligands

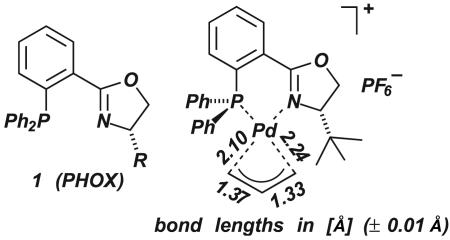
An even more effective way to desymmetrize a P,P- or N,N-ligand is to switch to mixed donor P,N-ligands, because of the distinctly different characteristics of a “soft” P-ligand with π-acceptor properties and a “hard” N-ligand acting primarily as an σ-donor.‡ This line of thought led us and, independently, Helmchen (24) and Williams (42) to a new, highly versatile class of ligands, the phosphinooxazoline (PHOX) ligands 1 (Fig. 6).

Fig. 6.

Structure of PHOX ligands and x-ray data of an allyl–Pd complex.

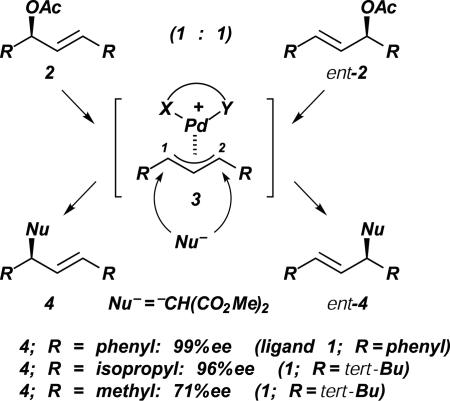
The reaction that we were investigating at that time, an enantioselective Pdcatalyzed allylic substitution, is shown in Fig. 7 (3, 4). Starting from a racemic mixture of allylic acetates 2 and ent-2, containing two identical substituents at the allylic termini, an allyl complex 3 is formed. Because both allylic acetate 2 and ent-2 are converted to the same intermediate, the stereochemical information is lost in this step. It is the subsequent step, a nucleophilic addition to the allyl system, that determines which enantiomer is formed. Nucleophilic attack at C (1) leads to product 4, attack at C (3) to the enantiomer ent-4. Consequently, the problem of inducing enantioselectivity is equivalent to controlling the regioselectivity of nucleophilic attack.

Fig. 7.

Mechanism of enantioselective Pdcatalyzed allylic substitution.

Initially, we tested bisoxazolines and related C₂-symmetric ligands in this reaction. Although good results were obtained in certain cases, the scope of this ligand class proved to be limited. Therefore, we turned our attention to other ligands such as the PHOX ligands 1 (Fig. 6) (24). From this ligand class, we hoped to gain an additional means of controlling the regioselectivity, based on electronic effects. In contrast to allyl complexes with C₂-symmetric N,N- or P,P-ligands, complexation by P,N-ligands should result in effective electronic discrimination of the allylic termini because of the different trans-influence of the two electronically dissimilar hetero atoms. (For other effective approaches to enantiocontrol in Pd-catalyzed allylic substitution, see refs. 3 and 4.) Electronic differentiation of this type had been demonstrated by Faller et al. (25) (stoichiometric reaction of allyl–Mo complexes with CO and (NO)⁺ as trans ligands) and by Åkermark et al. (26) (NMR studies of allyl–Pd complexes). Crystal structure and NMR data confirmed that complexation with a PHOX ligand results in a strong electronic differentiation of the allylic termini, as reflected by the different Pd–C distances (Fig. 6) (3, 24). As we had hoped, Pd–PHOX complexes were found to be highly reactive and selective catalysts, providing up to 99% enantiomeric excess with a range of C-terminal and N-terminal nucleophiles (Fig. 7). The selectivities could be rationalized by a combination of steric and electronic effects, the preference of nucleophilic attack at the allylic C atom trans the phosphino group being a key factor (24).

The Importance of a Modular Ligand Structure

Although PHOX ligands gave excellent results in reactions of diphenyl- and diisopropyl-allyl acetates, only moderate enantioselectivities were recorded for the “smaller” dimethyl- and diethyl-substituted analogues. In this respect, the PHOX ligands display opposite reactivity when compared with the diphosphine ligands, developed by Trost, that give excellent results with “small” substrates but unsatisfactory enantiomeric excess and yield with 1,3-diphenylallyl acetate (3, 4). However, because of the modular nature of the PHOX ligands (Fig. 8), many different derivatives could be readily synthesized, which made it possible to optimize the ligand structure for certain substrates that initially had given unsatisfactory results. In this way, Helmchen (24) achieved high enantioselectivities with small substrates such as 1,3-dimethylallyl or cylopentenyl and cylohexenyl acetate.

Fig. 8.

Modular construction of PHOX ligands 1.

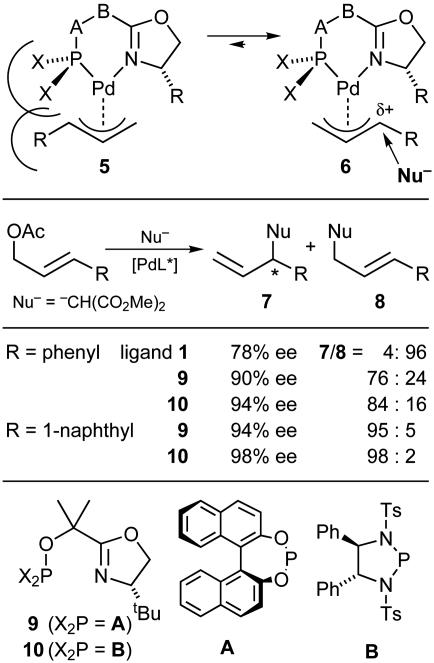
We wondered whether the ligand structure could also be adapted to the problems of regiocontrol and enantiocontrol for monsubstituted allyl esters (Fig. 9). In general, Pd catalysts induce the formation of the linear, achiral product 8 with high preference over the branched, chiral regioisomer 7. [Other metal catalysts (e.g., Mo, W, and Ir) show opposite regioselectivity in favor of the branched product. Examples of chiral catalysts of this type are W–PHOX and Ir–PHOX complexes, as well as Mo complexes with N,N-ligands (3, 4).] By rendering the transition state more cationic in character, nucleophilic attack at the substituted allyl terminus should become more favorable. Consequently, we introduced electronegative substituents on the P atom to increase the electrophilicity at the Pd center. Also, by introduction of sterically demanding P-substituents, we wanted to shift the equilibrium between the intermediate allyl complexes toward isomer 6. Because of the trans influence of the P atom, the predominance of this isomer should promote nucleophilic attack at the substituted terminus. These considerations led us to ligands 9 and 10, which in contrast to the original PHOX ligands 1 induced formation of the branched products with high regioselectivity and excellent enantioselectivity (Fig. 9) (24, 27). The analogous methylallyl ester gave unsatisfactory results. However, a further modification of P,N-ligands was reported recently, which resulted in high regioselectivity and enantioselectivity for this substrate as well (28).

Fig. 9.

Ligand optimization for regioselective and enantioselective allylic substitution.

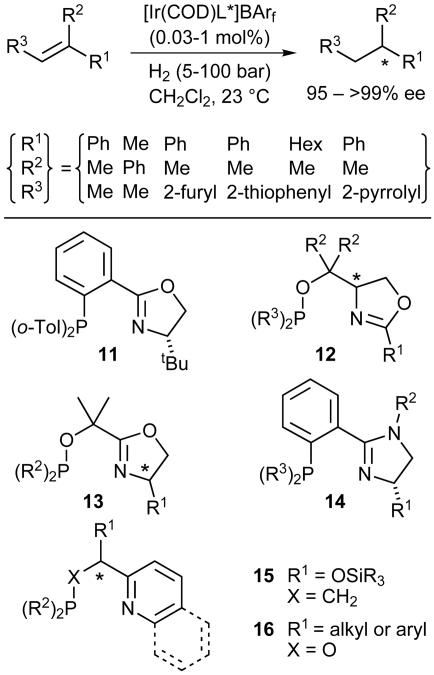
Although the PHOX ligands were developed originally for Pd-catalyzed allylic substitution, they could be applied successfully to various other metalcatalyzed processes, including Heck reactions, Cu-catalyzed 1,4-additions, and Ru-catalyzed transfer hydrogenation of ketones (24). Another reaction class that gave very promising results was Ircatalyzed asymmetric hydrogenation of CC and CN bonds (29, 30). We thought that Ir–PHOX complexes might behave like chiral analogues of the Crabtree catalyst, an achiral (tricyclohexylphosphine)(pyridine)Ir(I) complex that displays unusually high reactivity toward trisubstituted and tetrasubstituted olefins (31). Pleasingly, after optimization of the catalyst structure and the reaction parameters, good to excellent enantiomeric-excess values and high turnover numbers could be obtained in the hydrogenation of imines and unfunctionalized aryl-substituted olefins (Fig. 10). Until now, olefins of this kind could not be hydrogenated with high enantioselectivity at such low catalyst loadings. In this respect, Ir-PHOX complexes clearly distinguish themselves from Ru and Rh catalysts that require a polar coordinating group near the CC bond.

Fig. 10.

Ir-catalyzed asymmetric hydrogenation of olefins.

Again, the modular nature of the PHOX ligands made it possible to extend the application range of these catalysts. Among the many PHOX analogues that we tested, readily available phosphinites of type 12 and 13 proved to be the most versatile ligands. Subsequently, we added phosphinoimidazolines, such as type 14, to our collection of ligands (32, 33), as well as pyridine- and quinoline-derived P,N-ligands of type 15 and 16 (34), all of which gave very promising results. Recently, other research groups have become interested in this class of catalysts and have reported additional variants of Ir complexes with P,N-ligands (35–40) and, as a further modification, oxazoline ligands with a heterocyclic carbene unit instead of a phosphino group (41). Clearly, Ir complexes derived from P,N-ligands represent a new class of catalysts that significantly expands the application range of asymmetric hydrogenation. Several types of functionalized and nonfunctionalized olefins, for which no suitable catalysts were known previously, can now be hydrogenated with high efficiency and good to excellent enantioselectivity.

Conclusion

The concept of steric and electronic desymmetrization has led to a new class of chiral ligands, the PHOX ligands 1 and related P,N-ligands. Because of the modular construction, these ligands could be adapted to many metalcatalyzed reactions and in many cases, they outperformed P,P- or N,N-ligands. Chiral ligands based on other combinations of coordinating atoms, such as the P,S; P,O; or N,S varieties, have also been reported and, considering the enormous diversity of possible ligand structures of this type, further work in this area seems worthwhile. Although the concept of C₂ symmetry will remain an attractive design principle for new ligands, sterically and electronically nonsymmetrical heterobidentate ligands are likely to play an increasing role in the development of asymmetric catalysis.