Abstract
Laser scanning is a non-invasive method for three-dimensional assessment of facial morphology and symmetry. The aim of this study was to quantify facial symmetry in healthy adolescents and explore if there is any gender difference. Facial scans of 270 subjects, 123 males and 147 females (aged 15.3 ± 0.1 years, range 14.6–15.6), were randomly selected from the Avon Longitudinal Study of Parents and Children. Facial scans were processed and analysed using in-house developed subroutines for commercial software. The surface matching between the original face and its mirror image was measured for the whole face, upper, middle, and lower facial thirds. In addition, 3 angular and 14 linear parameters were measured. The percentage of symmetry of the whole face was significantly lower in males (53.49 ± 10.73 per cent) than in females (58.50 ± 10.27 per cent; P < 0.01). There was no statistically significant difference in the amount of symmetry among facial thirds within each gender (P > 0.05). Average values of linear parameters were less than 1 mm and did not differ significantly between genders (P > 0.05). One angular parameter showed slight lip line asymmetry in both genders. Faces of male 15-year-old adolescents were less symmetric than those of females, but the difference in the amount of symmetry, albeit statistically significant, may not be clinically relevant. Upper, middle, and lower thirds of the face did not differ in the amount of three-dimensional symmetry. Angular and linear parameters of facial symmetry did not show any gender difference.
Introduction
A desire to improve facial aesthetics is very often the reason for seeking orthodontic treatment (Kiyak 2000, 2008; Miguel et al., 2010). Recent papers in evolutionary psychology suggest that the perception of facial attractiveness is, among other factors, influenced by facial symmetry (Zaidel and Cohen, 2005; Rhodes, 2006; Komori et al., 2009). With increased concern about facial appearance, more patients complain of even slight asymmetry (Hwang et al., 2007), which justifies an inclusion of objective and thorough facial symmetry analysis in routine orthodontic examination. However, the problem arises when the boundaries of normal facial asymmetry are to be defined (Rossi et al., 2003; Liukkonen et al., 2005).
Albeit the need for three-dimensional assessment of facial symmetry was recognized more than six decades ago (Fischer, 1954), until the early nineties available methods were time consuming and not fully automated (Moss et al., 1991). O’Grady and Antonyshyn (1999) evaluated six different techniques for quantitative analysis of facial symmetry in three dimensions. Two of these techniques relied on the identification of anthropometric landmarks (asymmetry in the location of anthropometric landmarks and Euclidean distance matrix analysis), two relied on interactive identification of anatomic features or boundaries (e.g. scalar measurements of the lower ciliary margin and palpebral fissure area), and two described the differences in whole surfaces (clearance vector mapping and determination of the volume of asymmetry). Landmark-dependent methods in facial symmetry analysis have dominated orthodontic literature (Ferrario et al., 1994, 1995, 2001, 2003; Ras et al., 1994a,b, 1995). However, these methods have been criticized due to unreliable identification of landmarks, questionable validity of the symmetry plane, and incapability of depicting asymmetries in regions where landmarks are few and far between (Hartmann et al., 2007; Stauber et al., 2008). In recent studies, facial symmetry has been quantified by means of landmark-independent methods, which take into account all available facial points and allow a full face analysis (Nkenke et al., 2006; Hartmann et al., 2007; Primožič et al., 2009, 2011; Meyer-Marcotty et al., 2010; Djordjevic et al., 2011).
In orthodontic literature, the majority of research on facial symmetry of normal individuals has been conducted using two-dimensional methods. Hard tissue asymmetry has been analysed on panoramic (Kambylafkas et al., 2006; Kurt et al., 2008) and postero-anterior radiographs (Peck et al., 1991; Haraguchi et al., 2002; Rossi et al., 2003; Hwang et al., 2007), whereas soft tissue asymmetry has been evaluated using anthropometry (Farkas and Cheung, 1981; Skvarilova, 1993; Farkas, 1994) and photography (Ercan et al., 2008; Haraguchi et al., 2008). With the exception of a few studies, three-dimensional imaging methods were mainly applied in the analysis of facial asymmetry in cleft lip and palate patients (Ras et al., 1994a,b, 1995; Ferrario et al., 2003; Nkenke et al., 2006; Stauber et al., 2008; Meyer-Marcotty et al., 2010). There is a knowledge gap on the amount of three-dimensional facial symmetry in healthy individuals.
Previous studies have shown that age and gender do not have an effect on facial asymmetry (Burke and Healy, 1993; Skvarilova, 1993; Ferrario et al., 2001). On the other hand, there are authors who demonstrated sexual dimorphism in the amount (Ercan et al., 2008) and direction of facial asymmetry (Smith, 2000; Hardie et al., 2005). Furthermore, it has been reported that different regions of the face have different degree of asymmetry (Farkas and Cheung, 1981; Severt and Proffit, 1997; Ferrario et al., 1994, 2001; Shaner, 2000; Haraguchi et al., 2002; Ercan et al., 2008). The present study aimed to quantify facial symmetry and investigate gender differences in a cohort of 15-year-old British adolescents, using relatively novel method of three-dimensional assessment.
Subjects and methods
Sample
The study population comprised adolescents participating in the Avon Longitudinal Study of Parents and Children (ALSPAC), which is an ongoing research project based at the University of Bristol, UK. The study was designed to understand the ways in which the physical and social environment interact, over time, with the genotype to affect health, behaviour, and development (Golding and ALSPAC study team, 2004). The 14 541 enrolled pregnancies (expected date of delivery 1 April 1991 to 31 December 1992) represented about 85 per cent of the eligible population in the region of Avon, England, UK. Up to date information on this study and abstracts of publications can be found on the ALSPAC website (http://www.bristol.ac.uk/alspac).
During one of the follow-ups in 2006/2007, 4747 participants, 2233 males (average age 15.4 ± 0.3 years, range 14.5–17.0) and 2514 females (average age 15.4 ± 0.3 years, range 14.3–16.9), provided consent for facial laser scanning, which was organized in collaboration with Cardiff University. Only healthy normal growing individuals of Caucasian origin, without history of trauma, and operation in the maxillofacial region were included. Relevant ethics committees approved the protocol for laser scanning. For the purpose of this cross-sectional study, participants were randomly selected from the ALSPAC database. Sample size calculation was carried out using computer software G*Power 3 (Faul et al., 2007). One hundred thirty-three males and 133 females were required for the unpaired t-test to have a 90 per cent chance of detecting a 5 per cent difference in three-dimensional facial symmetry, at the 5 per cent level of significance. An estimate of the variation (SD 12 per cent) was based on the pilot study. The number of participants was rounded up from 266 to 270 in the protocol.
Image acquisition and processing
Laser scanning protocol has been described previously (Kau et al., 2005a). In this paper, a summary is provided. Two Minolta Vivid 900 laser scanners (Konica Minolta, Tokyo, Japan) were used to scan subjects in natural head position, which has proven to be reliable (Kau et al., 2005b). After calibration, scanning was performed with medium range lenses (focal length 14.5 mm), at a distance of 135 cm from the subjects, and controlled with multi-scan software (Cebas Computer; GmBH, Eppelheim, Germany). The system acquired more than 307 000 points of the facial surface in approximately 8 seconds. The procedure was repeated if the subject moved, opened mouth, or changed facial expression during the scanning. Left and right halves of the face were scanned simultaneously for each subject and saved in the computer memory in a vivid file format (VVD). In-house developed subroutines for reverse engineering software Rapidform 2006® (INUS Technology, Seoul, Korea) were used for image processing and facial symmetry analysis. Image processing comprised removal of extraneous data, smoothing of the shells, filling small holes, registering, and merging (Zhurov et al., 2005). Before merging, scan quality was assessed. Left and right scans were merged only if there was at least 70 per cent match between them in the overlap area, within 0.5 mm of tolerance (Toma et al., 2008; Djordjevic et al., 2011; Figure 1).
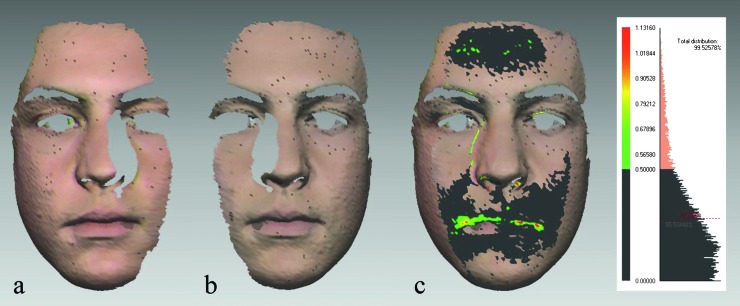
Figure 1.
Evaluation of laser scan’s quality. (a and b) Right and left facial halves (facial shells) in Rapidform 2006® (INUS Technology, Seoul, Korea) after four-stage processing. (c) Absolute colour map and the histogram were used to evaluate scan quality before merging. Surface matching of the two shells in the overlap area was 85.53 per cent. Deviations less than 0.5 mm are presented in dark grey, 0.51–0.79 mm in light green, 0.80–0.90 mm in yellow, and 0.91–1.13 mm in red. Internally developed subroutine automatically determined average distance between the facial shells in the overlap area: 0.28 mm (SD 0.24 mm). Therefore, laser scans were suitable for merging.
Three-dimensional facial symmetry parameters
Facial symmetry was quantified by means of the mirroring approach (Figure 2). For each subject, a mirror facial shell was created in Rapidform 2006 (INUS Technology, Seoul, Korea) using internally developed set of subroutines. The original facial shell was divided into the upper, middle, and lower thirds in order to compare facial symmetry in different regions of the face (Primožič et al., 2009, 2011; Djordjevic et al., 2011). The upper third was defined as the part of the face above the inner canthus plane, the middle ranged from the inner canthus plane to the plane through the outer commissures of the lips, and the lower was below this plane (Figure 3). The surface matching between the original and the mirror facial shells was assessed by the best-fit superimposition method for the whole face, upper, middle and lower thirds, within 0.5 mm of tolerance, and expressed as percentages. The lower the percentage, the lower facial symmetry, i.e. higher facial asymmetry. Average and maximum distances between the two shells were also computed. Shell-to-shell deviations were presented graphically as colour maps and quantitatively on histograms.
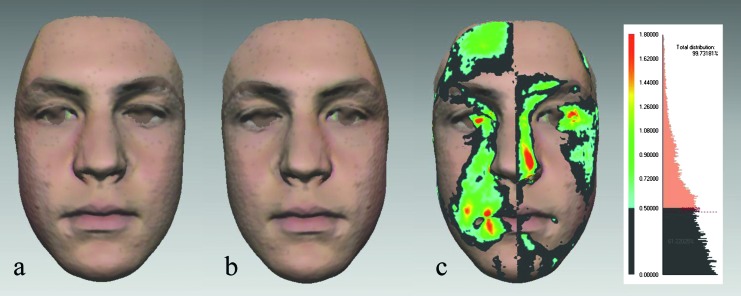
Figure 2.
Three-dimensional facial symmetry analysis in a 15-year-old male (same as in Figure 1): (a) the original facial shell, (b) the mirror facial shell, and (c) the colour map and the histogram. Symmetric areas of the face (within tolerance level 0.5 mm) are presented in dark grey. The colours indicate the range of deviations between the original and the mirror facial shells: 0.50–0.72 mm in turquoise, 0.73–1.26 mm in light green, 1.27–1.44 mm in yellow, and 1.45–1.80 mm in red. The percentage of symmetry of the whole face read from the histogram is 61.22 per cent.
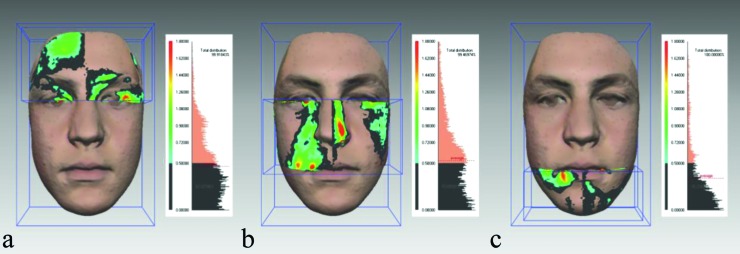
Figure 3.
The original facial shell was divided into the upper, middle, and lower thirds. The upper third (a) was defined as the part of the face above the inner canthus plane, the middle third (b) ranged from the inner canthus plane to the plane through the outer commissures of the lips, and the lower third (c) was below this plane. The percentage of symmetry for the upper, middle, and lower thirds in this male subject (same as in Figures 1 and 2) was 62.03, 53.66, and 78.23 per cent, respectively. The colours indicate the same range of deviations as in Figure 2.
Linear and angular facial symmetry parameters
Twenty-one reliable facial landmarks (Toma et al., 2009; Djordjevic et al., 2011) were manually identified on each facial scan by one operator. Based on these landmarks, 3 angular and 14 linear measurements were made in order to assess facial symmetry, as previously reported (Djordjevic et al., 2011; Figure 4). The midsagittal plane of the structure created by the superimposition of the original and mirror facial shells (Zhurov et al., 2010) was adopted as the symmetry plane for linear measurements.
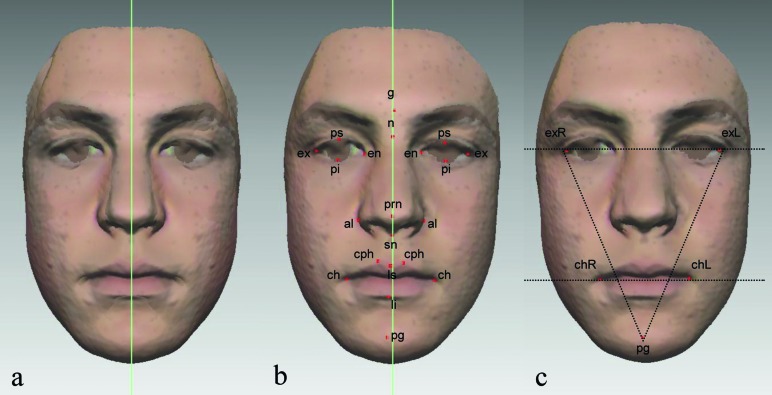
Figure 4.
(a) The symmetry plane adopted for the study was the midsagittal plane of the structure created by the superimposition (best-fit registration) of the original and mirror facial shells. (b) For median landmarks [glabella (g), nasion (n), pronasale (prn), subnasale (sn), labiale superius (ls), labiale inferius (li), and pogonion (pg)], the distance from the symmetry plane was calculated. For bilateral landmarks [palpebrale superius (ps), palpebrale inferius (pi), exocanthion (ex), endocanthion (en), alare (aal), crista philtri (cph), and cheilion (ch)), the midpoint between two corresponding landmarks was first determined and then its deviation from the symmetry plane was measured. (c) Three angular parameters were measured: the exR–exL–pg, exL–exR–pg, and exRexL–chRchL. ‘R’ and ‘L’ denote ‘right’ and ‘left’. These angles were projections of spatial angles onto the frontal plane.
Statistical analysis
All statistical analyses were performed using the Statistical Package for Social Sciences Software version 17.0 (SPSS Inc., Chicago, Illinois, USA). Descriptive statistics was used to assess the scan quality. The assumption of normality was checked by Shapiro–Wilk test, frequency histograms, and normal probability plots, and homogeneity of variances by Levene’s test. The data for the three-dimensional symmetry (whole face, upper, middle, and lower facial thirds) and angular parameters were normally distributed. The data for linear parameters of facial symmetry were positively skewed and square root transformed in order to obtain normal distribution. Therefore, mean, standard deviation, and range (minimum and maximum) were presented. The data for the average and maximum distances between the original and the mirror facial shells were positively skewed. After logarithmic transformation, normal distribution was obtained and results presented as geometric mean, geometric standard deviation, and range (minimum and maximum). Unpaired t-test was performed to compare all facial symmetry parameters between genders. One-way analysis of variance (ANOVA) was used to compare three-dimensional symmetry of the upper, middle, and lower facial thirds in each gender. P values of 0.05 or less were considered statistically significant, with the exception of comparisons of facial thirds (P < 0.017) and linear parameters (P < 0.004), for which Bonferroni correction was applied.
Results
Facial symmetry analysis was performed on facial scans of 270 adolescents, 123 males (average age 15.3 ± 0.1 years, range 15.0–15.6) and 147 females (average age 15.3 ± 0.1 years, range 14.6–15.6). Although the sample size was calculated for equally sized groups of males and females, the power of the study was not affected by the change in the proportion.
All facial scans fulfilled predefined criteria. On average, surface matching of facial halves in the overlap area before merging was 87.5 ± 6.8 per cent and the average distance between them 0.3 ± 0.1 mm.
The results of quantitative analysis of facial symmetry for both genders are presented in Table 1. On average, the percentage of symmetry of the whole face in males was 53.49 ± 10.73 per cent and in females 58.50 ± 10.27 per cent. Unpaired t-test revealed that the difference of 5.01 per cent was statistically significant. Upper and middle thirds of male faces had significantly lower symmetry than those of female faces (P < 0.01). There was no statistically significant gender difference in the amount of symmetry of the lower third of the face (P > 0.05). Average distance between the original and the mirror facial shells was generally higher in males than in females, and the difference was statistically significant (P < 0.01). There was no statistically significant difference in the amount of maximum asymmetry between genders (P > 0.05). The ANOVA did not reveal any statistically significant difference in three-dimensional facial symmetry among the upper, middle, and lower facial thirds within each gender (P > 0.05; Table 2).
Table 1.
Descriptive statistics and comparison of facial symmetry parameters between genders (independent samples t-test). OS, original facial shell; MS, mirror facial shell; Max., maximum; exR-exocanthion right; exL-exocanthion left; chR-cheilion right; chL-cheilion left; msp, midsagittal plane; mid (subscripted), the middle of the distance between bilateral landmarks; g, glabella; n, nasion; prn, pronasale; sn, subnasale; ls, labiale superius; li, labiale inferius; pg, pogonion; ps, palpebrale superius; pi, palpebrale inferius; ex, exocanthion; en, endocanthion; al, alare; cph, crista philtri; ch, cheilion; SD, standard deviation; range, minimum to maximum; diff., difference; CI, confidence interval; n.s., not significant.
| Parameters | Males (n = 123) | Females (n = 147) | Mean difference/ratio (95% CI) | P value | ||
| Mean (SD) | Range | Mean (SD) | Range | |||
| Three-dimensional facial symmetry | ||||||
| Whole face (%) | 53.49 (10.73) | 31.01–79.55 | 58.50 (10.27) | 31.99–83.14 | −5.01 (−7.54 to −2.48) | <0.001* |
| Upper third (%) | 53.41 (13.03) | 22.23–83.50 | 59.84 (14.52) | 24.17–91.34 | −6.43 (−9.78 to −3.08) | <0.001* |
| Middle third (%) | 54.07 (13.53) | 18.14–84.43 | 58.87 (12.07) | 30.49–85.10 | −4.80 (−7.88 to −1.72) | 0.002* |
| Lower third (%) | 54.69 (21.92) | 10.34–98.10 | 57.26 (18.25) | 10.26–99.10 | −2.57 (−7.39 to 2.24) | 0.294 (n.s.) |
| Mean OS–MS (mm) | 0.61 (1.28)** | 0.33–1.14 | 0.54 (1.27)** | 0.28–1.05 | 1.14 (1.07 to 1.20) | <0.001* |
| Max. OS–MS (mm) | 2.85 (1.35)** | 1.46–4.98 | 2.67 (1.37)** | 1.34–4.92 | 1.07 (0.99 to 1.14) | 0.084 (n.s.) |
| Angular parameters | ||||||
| 1. exR–exL–pg (°) | 65.32 (1.35) | 62.15–68.73 | 64.59 (1.51) | 61.32–68.98 | 0.73 (0.38 to 1.08) | <0.001* |
| 2. exL–exR–pg (°) | 65.85 (1.44) | 62.26–69.68 | 64.97 (1.49) | 61.41–69.32 | 0.88 (0.53 to 1.23) | <0.001* |
| Difference 1−2 (°) | 1.31 (1.12) | 0.01–5.47 | 1.34 (0.93) | 0.01–5.53 | −0.03 (−0.27 to 0.22) | 0.840 (n.s.) |
| exRexL–chRchL (°) | 2.07 (1.18) | 0.14–6.23 | 2.34 (1.29) | 0.24–7.32 | −0.27 (−0.57 to 0.03) | 0.080 (n.s.) |
| Linear parameters | ||||||
| g–msp (mm) | 0.81 (0.35)*** | 0.01–3.01 | 0.77 (0.33)*** | 0.00–2.27 | 0.04 (−0.04 to 0.13) | 0.286 (n.s.) |
| n–msp (mm) | 0.73 (0.29)*** | 0.04–2.23 | 0.67 (0.30)*** | 0.00–1.74 | 0.06 (−0.02 to 0.13) | 0.135 (n.s.) |
| prn–msp (mm) | 0.78 (0.35)*** | 0.01–4.44 | 0.74 (0.33)*** | 0.00–2.94 | 0.04 (−0.04 to 0.13) | 0.296 (n.s.) |
| sn–msp (mm) | 0.67 (0.32)*** | 0.00–3.42 | 0.62 (0.30)*** | 0.01–2.03 | 0.05 (−0.02 to 0.13) | 0.181 (n.s.) |
| ls–msp (mm) | 0.76 (0.34)*** | 0.00–4.06 | 0.74 (0.33)*** | 0.00–2.70 | 0.02 (−0.06 to 0.10) | 0.557 (n.s.) |
| li–msp (mm) | 0.81 (0.36)*** | 0.00–3.14 | 0.80 (0.36)*** | 0.00–3.25 | 0.01 (−0.07 to 0.10) | 0.816 (n.s.) |
| pg–msp (mm) | 0.94 (0.42)*** | 0.00–6.14 | 0.88 (0.39)*** | 0.00–4.40 | 0.06 (−0.05 to 0.15) | 0.312 (n.s.) |
| psmid–msp (mm) | 0.67 (0.29)*** | 0.00–2.37 | 0.68 (0.31)*** | 0.02–1.95 | −0.01 (−0.08 to 0.06) | 0.827 (n.s.) |
| pimid–msp (mm) | 0.67 (0.30)*** | 0.00–1.98 | 0.71 (0.29)*** | 0.01–1.80 | −0.04 (−0.11 to 0.03) | 0.273 (n.s.) |
| exmid–msp (mm) | 0.68 (0.30)*** | 0.01–1.84 | 0.73 (0.29)*** | 0.00–1.79 | −0.05 (−0.12 to 0.02) | 0.160 (n.s.) |
| enmid–msp (mm) | 0.67 (0.30)*** | 0.00–1.91 | 0.63 (0.30)*** | 0.00–2.83 | 0.04 (−0.03 to 0.11) | 0.274 (n.s.) |
| almid–msp (mm) | 0.52 (0.27)*** | 0.01–3.48 | 0.54 (0.27)*** | 0.00–1.75 | −0.02 (−0.08 to 0.05) | 0.540 (n.s.) |
| cphmid–msp (mm) | 0.83 (0.36)*** | 0.01–4.07 | 0.78 (0.32)*** | 0.00–2.69 | 0.05 (−0.03 to 0.14) | 0.175 (n.s.) |
| chmid–msp (mm) | 0.81 (0.31)*** | 0.00–2.80 | 0.77 (0.34)*** | 0.01–3.19 | 0.04 (−0.03 to 0.13) | 0.227 (n.s.) |
Statistically significant.
Geometric mean and geometric standard deviation.
Square root transformed.
Table 2.
Comparison of facial thirds in the amount of symmetry. n.s., not significant.
| Upper third (%) | Middle third (%) | Lower third (%) | ANOVA | ||
| Mean (SD) | Mean (SD) | Mean (SD) | F | P | |
| Males | 53.41 (13.03) | 54.07 (13.53) | 54.69 (21.92) | 0.175 | 0.839 (n.s.) |
| Females | 59.84 (14.52) | 58.87 (12.07) | 57.26 (18.25) | 0.885 | 0.414 (n.s.) |
The average difference between the exR–exL–pg and the exL–exR–pg angles (Figure 4) was 1.31 ± 1.12 degrees in males and 1.34 ± 0.93 degrees in females. The third angle, exRexL–chRchL, showed slight lip line asymmetry of 2.07 ± 1.18 degrees in males and 2.34 ± 1.29 degrees in females. There was no statistically significant gender difference in angular parameters of facial symmetry (P > 0.05).
Average values of linear parameters of facial symmetry were less than 1 mm. In general, ‘alare’ was the least asymmetric and ‘pogonio’ the most asymmetric landmark. Maximum horizontal asymmetry ranged from 1.84 mm (‘exocanthion’) to 6.14 mm (‘pogonion’) in males and from 1.74 mm (‘nasion’) to 4.40 mm (‘pogonion’) in females. The amount of horizontal asymmetry of the landmarks did not differ significantly between genders (P > 0.05).
Discussion
Facial symmetry has been attracting attention of orthodontists and maxillofacial surgeons for decades. Progress of science and technology enables accurate and meticulous analysis of facial soft tissue anatomy, which was not feasible previously. In order to further improve diagnosis of facial symmetry, it is important to analyse faces objectively in three dimensions. Laser surface scanning provides such analysis in an accurate, reliable, and non-invasive manner (Kau et al., 2004, 2005a,b; Zhurov et al., 2005).
Different methods for three-dimensional analysis of facial symmetry have been suggested, but none of them is universally accepted. In this study, the focus was on the surface matching of the original and the mirror facial shells obtained by laser surface scanning. Once an image of a subject has been captured and processed, a mirror image can be generated and superimposed on the original one using iterative closest point algorithm. Theoretically, a face is perfectly symmetric if it is identical to its mirror image. Similar concept has been applied in two-dimensions, using composite photographs consisting of left–left and right–right facial halves, in order to investigate the effects of asymmetry on human perception (Penton-Voak et al., 2001; Zaidel and Cohen, 2005).
The absolute distances between all pairs of points on the surfaces of the original and mirror facial shells have been automatically calculated and the average and maximum distances used in the subsequent analysis. The percentage of surface matching between the two shells was also measured. Deviations up to 0.5 mm were considered insignificant. This tolerance level was chosen according to the results of previous investigation, which showed that the accuracy of Minolta Vivid laser scanner is 0.56 ± 0.25 mm (Kau et al., 2004). This method is independent of any symmetry plane and not influenced by the size of the face. One of the potential limits is the absence of the overlap between the original face and the mirror face in the marginal areas. Special consideration should be given to the image processing stages. Furthermore, laser scanning quality should be consistently checked prior to merging facial halves captured by two devices.
The results for three-dimensional parameters of facial symmetry showed that, on average, slightly more than half of the male face was symmetric. Mean symmetry of female faces was significantly higher, but the difference of 5 per cent, albeit statistically significant, may not be clinically relevant (95 per cent confidence interval between 2.5 and 7.5 per cent). Statistically significant gender difference was found for the upper and middle thirds of the face but not for the lower third. When facial thirds were compared within each gender, no statistically significant differences were revealed. Slight lip line asymmetry was revealed by measuring the exRexL–chRchL angle. On average, linear parameters did not exceed 1 mm and ‘pogonion’ was the most asymmetric landmark on the face. Angular and linear parameters of facial symmetry did not show any significant gender difference.
These findings can be directly compared to our recent prospective study on facial symmetry in Finnish adolescents (Djordjevic et al., 2011). The results generally coincide, except that the gender difference in three-dimensional symmetry was not found in the later study. One of the explanations for this might be that the sample size was small and non-parametric tests not sensitive enough to reveal subtle difference, which was detected in the present study. Lip line asymmetry in general population has been previously analysed using the same angle on frontal facial photographs in 1282 Korean young adults, 18–29 years of age (Song et al., 2007). The average values of the angle (0.2 ± 1.4 degrees in males and 0.3 ± 1.3 degrees in females) were less than in this study
There is no consensus in the published literature on the most asymmetric part of the face. Some authors stated that the upper third is the most asymmetric (Farkas and Cheung, 1981; Farkas, 1994), whereas others found the middle (Ercan et al., 2008) and the lower third (Ferrario et al., 1994; Severt and Proffit, 1997; Shaner et al., 2000; Haraguchi et al., 2002) to be the most asymmetric. The differences can be explained by different methodological approaches (two-dimensional or three-dimensional) and selection of participants (in some studies orthodontic patients), contrary to the random sampling from general population performed in this study.
The finding that the upper, middle, and lower parts of the face do not differ significantly in terms of the amount of facial symmetry may have important clinical implications. An orthodontist should bear in mind that patients’ perceptions of facial attractiveness may depend on the appearance/symmetry of features in the upper and middle thirds of the face, which are out of reach of orthodontic treatment. As patients’ expectations might be high, good communication prior to undertaking treatment is essential. Colour maps can enhance patient’s understanding of the problem and possibly impede unrealistic expectations.
When facial symmetry of a particular patient is to be compared with the average values, obtained from this or any other study, the difference must be cautiously interpreted. For example, a face with a visible deviation of the nose or chin may have the same degree of three-dimensional symmetry as a face with a barely noticeable asymmetry in the cheeks or forehead or a face with asymmetry scattered around the whole surface. It underlines the importance of further research to investigate the relationship between objective measurement and individual perception of facial symmetry. In the last few years, such research has been conducted mainly on photographs (van Keulen, et al., 2004; Evans et al., 2005; Chatrath et al., 2007) and in a recent study on the three-dimensional images obtained by optical sensor (Meyer-Marcotty et al., 2011). Facial laser scans could be applied in a similar manner in future studies.
To our knowledge, this is the first study to analyse facial symmetry in a large cohort of healthy adolescents using laser surface scanning. We believe that it is important to establish age- and gender-specific three-dimensional norms for facial morphology and symmetry in a given population. Therefore, it is hoped that this research would initiate further investigation in other populations and among different age groups in order to create databases, which will be applied clinically.
Conclusions
Faces of male 15-year-old adolescents were less symmetric than those of females, but the difference in the amount of symmetry, albeit statistically significant, may not be clinically relevant. Upper, middle, and lower thirds of the face did not differ in the amount of three-dimensional symmetry. Angular and linear parameters of facial symmetry did not show any gender difference.
Funding
The UK Medical Research Council, the Wellcome Trust, and the University of Bristol provide core support for ALSPAC.
Acknowledgments
We are extremely grateful to all the families who took part in this study, the midwives for their help in recruiting them, and the whole ALSPAC team, which includes interviewers, computer and laboratory technicians, clerical workers, research scientists, volunteers, managers, receptionists, and nurses. This publication is the work of the authors (Jelena Djordjevic, Arshed M. Toma, Alexei I. Zhurov, and Stephen Richmond), who will serve as guarantors for the contents of this paper, and does not reflect the views of the ALSPAC executive.
References
- Burke PH, Healy MJ. A serial study of normal facial asymmetry in monozygotic twins. Annals of Human Biology. 1993;20:527–534. doi: 10.1080/03014469300002932. [DOI] [PubMed] [Google Scholar]
- Chatrath P, De Cordova J, Nouraei SA, Ahmed J, Saleh HA. Objective assessment of facial asymmetry in rhinoplasty patients. Archives of Facial Plastic Surgery. 2007;9:184–187. doi: 10.1001/archfaci.9.3.184. [DOI] [PubMed] [Google Scholar]
- Djordjevic J, et al. Three-dimensional longitudinal assessment of facial symmetry in adolescents. European Journal of Orthodontics. 2011;35:143–151. doi: 10.1093/ejo/cjr006. [DOI] [PubMed] [Google Scholar]
- Ercan I, et al. Facial asymmetry in young healthy subjects evaluated by statistical shape analysis. Journal of Anatomy. 2008;213:663–669. doi: 10.1111/j.1469-7580.2008.01002.x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Evans CA, Viana G, Anderson NK, Giddon DB. Tolerance of deviations in eye and mouth position. Orthodontics and Craniofacial Research. 2005;8:75–84. doi: 10.1111/j.1601-6343.2005.00317.x. [DOI] [PubMed] [Google Scholar]
- Farkas LG, editor. Anthropometry of the head and face. New York: Raven Press; 1994. pp. 20–25. 103–111. [Google Scholar]
- Farkas LG, Cheung G. Facial asymmetry in healthy North American Caucasians. An anthropometrical study. Angle Orthodontist. 1981;51:70–77. doi: 10.1043/0003-3219(1981)051<0070:FAIHNA>2.0.CO;2. [DOI] [PubMed] [Google Scholar]
- Faul F, Erdfelder E, Lang AG, Buchner A. G*Power 3: a flexible statistical power analysis program for the social, behavioral, and biomedical sciences. Behavior Research Methods. 2007;39:175–191. doi: 10.3758/bf03193146. [DOI] [PubMed] [Google Scholar]
- Ferrario VF, Sforza C, Ciusa V, Dellavia C, Tartaglia GM. The effect of sex and age on facial asymmetry in healthy subjects: a cross-sectional study from adolescence to mid-adulthood. Journal of Oral and Maxillofacial Surgery. 2001;59:382–388. doi: 10.1053/joms.2001.21872. [DOI] [PubMed] [Google Scholar]
- Ferrario VF, Sforza C, Dellavia C, Tartaglia G, Colombo A, Carù A. A quantitative three-dimensional assessment of soft tissue facial asymmetry of cleft lip and palate adult patients. Journal of Craniofacial Surgery. 2003;14:739–746. doi: 10.1097/00001665-200309000-00026. [DOI] [PubMed] [Google Scholar]
- Ferrario VF, Sforza C, Poggio CE, Tartaglia G. Distance from symmetry: a three-dimensional evaluation of facial asymmetry. Journal of Oral and Maxillofacial Surgery. 1994;52:1126–1132. doi: 10.1016/0278-2391(94)90528-2. [DOI] [PubMed] [Google Scholar]
- Ferrario VF, Sforza C, Miani A, Jr, Serrao G. A three-dimensional evaluation of human facial asymmetry. Journal of Anatomy. 1995;186:103–110. [PMC free article] [PubMed] [Google Scholar]
- Fischer B. Asymmetry of the dentofacial complex. Angle Orthodontist. 1954;24:179–192. [Google Scholar]
- Golding J ALSPAC Study Team. The Avon Longitudinal Study of Parents and Children (ALSPAC)—study design and collaborative opportunities. European Journal of Endocrinology. 2004;151:U119–U123. doi: 10.1530/eje.0.151u119. [DOI] [PubMed] [Google Scholar]
- Haraguchi S, Iguchi Y, Takada K. Asymmetry of the face in orthodontic patients. Angle Orthodontist. 2008;78:421–426. doi: 10.2319/022107-85.1. [DOI] [PubMed] [Google Scholar]
- Haraguchi S, Takada K, Yasuda Y. Facial asymmetry in subjects with skeletal Class III deformity. Angle Orthodontist. 2002;72:28–35. doi: 10.1043/0003-3219(2002)072<0028:FAISWS>2.0.CO;2. [DOI] [PubMed] [Google Scholar]
- Hardie S, Hancock P, Rodway P, Penton-Voak I, Carson D, Wright L. The enigma of facial asymmetry: is there a gender-specific pattern of facedness? Laterality. 2005;10:295–304. doi: 10.1080/13576500442000094. [DOI] [PubMed] [Google Scholar]
- Hartmann J, Meyer-Marcotty P, Benz M, Häusler G, Stellzig-Eisenhauer A. Reliability of a method for computing facial symmetry plane and degree of asymmetry based on 3D-data. Journal of Orofacial Orthopedics. 2007;68:477–49. doi: 10.1007/s00056-007-0652-y. [DOI] [PubMed] [Google Scholar]
- Hwang HS, Youn IS, Lee KH, Lim HJ. Classification of facial asymmetry by cluster analysis. American Journal of Orthodontics and Dentofacial Orthopedics. 2007;132:279.e1–e6. doi: 10.1016/j.ajodo.2007.01.017. [DOI] [PubMed] [Google Scholar]
- Kambylafkas P, Murdock E, Gilda E, Tallents RH, Kyrkanides S. Validity of panoramic radiographs for measuring mandibular asymmetry. Angle Orthodontist. 2006;76:388–393. doi: 10.1043/0003-3219(2006)076[0388:VOPRFM]2.0.CO;2. [DOI] [PubMed] [Google Scholar]
- Kau CH, Knox J, Zhurov AI, Richmond S. Proceedings of the 7th European Craniofacial Congress, Bologna, Italy, Monduzzi Editore, International Proceedings Division. 2004. Validation of a portable 3D optical scanning device for field studies; pp. 41–45. [Google Scholar]
- Kau CH, et al. Natural head posture for measuring three-dimensional soft tissue morphology. In: Middleton J, Shrive MG, Jones ML, editors. Computer methods in biomechanics and biomedical engineering—5 FIRST. Cardiff: Numerics Ltd; 2005a. [Google Scholar]
- Kau CH, et al. Reliability of measuring facial morphology with a 3-dimensional laser scanning system. American Journal of Orthodontics and Dentofacial Orthopedics. 2005b;128:424–430. doi: 10.1016/j.ajodo.2004.06.037. [DOI] [PubMed] [Google Scholar]
- Kiyak HA. Cultural and psychologic influences on treatment demand. Seminars in Orthodontics. 2000;6:242–248. [Google Scholar]
- Kiyak HA. Does orthodontic treatment affect patients’ quality of life? Journal of Dental Education. 2008;72:886–894. [PubMed] [Google Scholar]
- Komori M, Kawamura S, Ishihara S. Averageness or symmetry: which is more important for facial attractiveness? Acta Psychologica. 2009;131:136–142. doi: 10.1016/j.actpsy.2009.03.008. [DOI] [PubMed] [Google Scholar]
- Kurt G, Uysal T, Sisman Y, Ramoglu SI. Mandibular asymmetry in Class II subdivision malocclusion. Angle Orthodontist. 2008;78:32–37. doi: 10.2319/021507-73.1. [DOI] [PubMed] [Google Scholar]
- Liukkonen M, Sillanmäki L, Peltomäki T. Mandibular asymmetry in healthy children. Acta Odontologica Scandinavica. 2005;63:168–172. doi: 10.1080/00016350510019928. [DOI] [PubMed] [Google Scholar]
- Meyer-Marcotty P, Alpers GW, Gerdes AB, Stellzig-Eisenhauer A. Impact of facial asymmetry in visual perception: a 3-dimensional data analysis. American Journal of Orthodontics and Dentofacial Orthopedics. 2010;137:168.e1–e.8. doi: 10.1016/j.ajodo.2008.11.023. [DOI] [PubMed] [Google Scholar]
- Meyer-Marcotty P, Stellzig-Eisenhauer A, Bareis U, Hartmann J, Kochel J. Three-dimensional perception of facial asymmetry. European Journal of Orthodontics. 2011;33:647–653. doi: 10.1093/ejo/cjq146. [DOI] [PubMed] [Google Scholar]
- Miguel JA, Sales HX, Quintão CC, Oliveira BH, Feu D. Factors associated with orthodontic treatment seeking by 12–15-year-old children at a state university-funded clinic. Journal of Orthodontics. 2010;37:100–106. doi: 10.1179/14653121042957. [DOI] [PubMed] [Google Scholar]
- Moss JP, Coombes AM, Linney AD, Campos J. Methods of three dimensional analysis of patients with asymmetry of the face. Proceedings of the Finnish Dental Society. 1991;87:139–149. [PubMed] [Google Scholar]
- Nkenke E, et al. Determination of facial symmetry in unilateral cleft lip and palate patients from three-dimensional data: technical report and assessment of measurement errors. Cleft Palate-Craniofacial Journal. 2006;43:129–137. doi: 10.1597/04-138.1. [DOI] [PubMed] [Google Scholar]
- O’Grady KF, Antonyshyn OM. Facial asymmetry: three-dimensional analysis using laser surface scanning. Plastic and Reconstructive Surgery. 1999;104:928–937. doi: 10.1097/00006534-199909040-00006. [DOI] [PubMed] [Google Scholar]
- Peck S, Peck L, Kataja M. Skeletal asymmetry in esthetically pleasing faces. Angle Orthodontist. 1991;61:43–48. doi: 10.1043/0003-3219(1991)061<0043:SAIEPF>2.0.CO;2. [DOI] [PubMed] [Google Scholar]
- Penton-Voak IS, et al. Symmetry, sexual dimorphism in facial proportions and male facial attractiveness. Proceedings of the Royal Society B Biological Sciences. 2001;268:1617–1623. doi: 10.1098/rspb.2001.1703. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Primožič J, Ovsenik M, Richmond S, Kau CH, Zhurov A. Early crossbite correction: a three-dimensional evaluation. European Journal of Orthodontics. 2009;31:352–356. doi: 10.1093/ejo/cjp041. [DOI] [PubMed] [Google Scholar]
- Primožič J, Richmond S, Kau CH, Zhurov A, Ovsenik M. Three-dimensional evaluation of early crossbite correction: a longitudinal study. European Journal of Orthodontics. 2011;35:7–13. doi: 10.1093/ejo/cjq198. [DOI] [PubMed] [Google Scholar]
- Ras F, Habets LL, Van Ginkel FC, Prahl-Andersen B. Facial left-right dominance in cleft lip and palate: three-dimensional evaluation. Cleft Palate-Craniofacial Journal. 1994a;31:461–465. doi: 10.1597/1545-1569_1994_031_0461_flrdic_2.3.co_2. [DOI] [PubMed] [Google Scholar]
- Ras F, Habets LL, Van Ginkel FC, Prahl-Andersen B. Three-dimensional evaluation of facial asymmetry in cleft lip and palate. Cleft Palate-Craniofacial Journal. 1994b;31:116–121. doi: 10.1597/1545-1569_1994_031_0116_tdeofa_2.3.co_2. [DOI] [PubMed] [Google Scholar]
- Ras F, Habets LL, Van Ginkel FC, Prahl-Andersen B. Logitudinal study on three-dimensional changes of facial asymmetry in children between 4 to 12 years of age with unilateral cleft lip and palate. Cleft Palate-Craniofacial Journal. 1995;32:463–468. doi: 10.1597/1545-1569_1995_032_0463_lsotdc_2.3.co_2. [DOI] [PubMed] [Google Scholar]
- Rhodes G. The evolutionary psychology of facial beauty. Annual Review of Psychology. 2006;57:199–226. doi: 10.1146/annurev.psych.57.102904.190208. [DOI] [PubMed] [Google Scholar]
- Rossi M, Ribeiro E, Smith R. Craniofacial asymmetry in development: an anatomical study. Angle Orthodontist. 2003;73:381–385. doi: 10.1043/0003-3219(2003)073<0381:CAIDAA>2.0.CO;2. [DOI] [PubMed] [Google Scholar]
- Severt TR, Proffit WR. The prevalence of facial asymmetry in the dentofacial deformities population at the University of North Carolina. International Journal of Adult Orthodontics and Orthognathic Surgery. 1997;12:171–176. [PubMed] [Google Scholar]
- Shaner DJ, Peterson AE, Beattie OB, Bamforth JS. Assessment of soft tissue facial asymmetry in medically normal and syndrome-affected individuals by analysis of landmarks and measurements. American Journal of Medical Genetics. 2000;93:143–154. doi: 10.1002/1096-8628(20000717)93:2<143::aid-ajmg12>3.0.co;2-q. [DOI] [PubMed] [Google Scholar]
- Skvarilova B. Facial asymmetry: type, extent and range of normal values. Acta Chirurgia Plastica. 1993;35:173–180. [PubMed] [Google Scholar]
- Smith WM. Hemispheric and facial asymmetry: gender differences. Laterality. 2000;5:251–258. doi: 10.1080/713754376. [DOI] [PubMed] [Google Scholar]
- Song WC, et al. Horizontal angular asymmetry of the face in Korean young adults with reference to the eye and mouth. Journal of Oral and Maxillofacial Surgery. 2007;65:2164–2168. doi: 10.1016/j.joms.2006.11.018. [DOI] [PubMed] [Google Scholar]
- Stauber I, et al. Three-dimensional analysis of facial symmetry in cleft lip and palate patients using optical surface data. Journal of Orofacial Orthopedics. 2008;69:268–282. doi: 10.1007/s00056-008-0746-1. [DOI] [PubMed] [Google Scholar]
- Toma AM, Zhurov A, Playle R, Richmond S A three-dimensional look for facial differences between males and females in a British-Caucasian sample aged 15 ½ years old. Orthodontics and Craniofacial Research. 2008;11:180–185. doi: 10.1111/j.1601-6343.2008.00428.x. [DOI] [PubMed] [Google Scholar]
- Toma AM, Zhurov A, Playle R, Ong E, Richmond S. Reproducibility of facial soft tissue landmarks on 3D laser-scanned facial images. Orthodontics and Craniofacial Research. 2009;12:33–42. doi: 10.1111/j.1601-6343.2008.01435.x. [DOI] [PubMed] [Google Scholar]
- van Keulen C, Martens G, Dermaut L. Unilateral posterior cross-bite and chin deviation: is there a correlation? European Journal of Orthodontics. 2004;26:283–288. doi: 10.1093/ejo/26.3.283. [DOI] [PubMed] [Google Scholar]
- Zaidel DW, Cohen JA. The face, beauty, and symmetry: perceiving asymmetry in beautiful faces. International Journal of Neuroscience. 2005;115:1165–1173. doi: 10.1080/00207450590914464. [DOI] [PubMed] [Google Scholar]
- Zhurov AI, Kau CH, Richmond S. Computer methods for measuring 3D facial morphology. In: Middleton J, Shrive MG, Jones ML, editors. Computer methods in biomechanics and biomedical engineering—5. Cardiff: First Numerics Ltd; 2005. ISBN 0-9549670-0-3. [Google Scholar]
- Zhurov AI, Richmond S, Kau CH, Toma AM. Averaging facial images. In: Kau CH, Richmond S, editors. Three-dimensional imaging for orthodontics and maxillofacial surgery. London: Wiley-Blackwell; 2010. ISBN 978–1405162401. [Google Scholar]