Continuous Cervical Spine Kinematics During In Vivo Dynamic Flexion-Extension

Abstract

BACKGROUND CONTEXT

A precise and comprehensive definition of “normal” in vivo cervical kinematics does not exist due to high inter-subject variability and the absence of mid-range kinematic data. In vitro test protocols and finite element models that are validated using only end range of motion data may not accurately reproduce continuous in vivo motion.

PURPOSE

The primary objective of this study was to precisely quantify cervical spine intervertebral kinematics during continuous, functional flexion-extension in asymptomatic subjects. The advantages of assessing continuous intervertebral kinematics were demonstrated by comparing asymptomatic controls with single-level anterior arthrodesis patients.

STUDY DESIGN

Cervical spine kinematics were determined during continuous in vivo flexion-extension in a clinically relevant age group of asymptomatic controls and a group of C5/C6 arthrodesis patients.

PATIENT SAMPLE

6 single-level (C5/C6) anterior arthrodesis patients (average age: 48.8 ± 6.9 yrs; 1 M, 5 F; 7.6±1.2 mo. post-surgery) and 18 asymptomatic control subjects of similar age (average age: 45.6 ± 5.8 yrs; 5 M, 13 F).

OUTCOME MEASURES

The physiologic measure of continuous kinematic motion paths at each cervical motion segment (C2 to C7) during flexion-extension.

METHODS

Participants performed flexion-extension while biplane radiographs were collected at 30 images per second. A previously validated tracking process determined three-dimensional vertebral positions with sub-millimeter accuracy. Continuous flexion-extension rotation and anterior-posterior translation motion paths were adjusted for disc height and static orientation of each corresponding motion segment. This study was funded by NIH/NIAMS Grant #1R03AR056265 and The Cervical Spine Research Society 21^st Century Development Grant. The authors have no potential financial conflict of interest biases related to this study.

RESULTS

Inter-subject variability in flexion-extension angle was decreased 15% to 46% and inter-subject variability in anterior-posterior translation was reduced 14% to 33% after adjusting for disc height and static orientation angle. Average inter-subject variability in continuous motion paths was 1.9° in flexion-extension and 0.6 mm in translation. Third-order polynomial equations were determined to precisely describe the continuous flexion-extension and anterior-posterior translation motion path at each motion segment (all R² > .99).

CONCLUSIONS

A significant portion of the inter-subject variability in cervical kinematics can be explained by the disc height and the static orientation of each motion segment. Clinically relevant information may be gained by assessing intervertebral kinematics during continuous functional movement rather than at static, end range of motion positions. The fidelity of in vitro cervical spine mechanical testing protocols may be evaluated by comparing in vitro kinematics to the continuous motion paths presented.

Introduction

Cervical spine kinematics are commonly assessed using static, end-range of motion radiographs collected with the head in full flexion and full extension[1-5]. Previous studies have demonstrated significant inter-subject variability in intervertebral flexion-extension range of motion (ROM), ranging from 20% to 60% of the overall motion at each motion segment[4-8]. This large variability makes it difficult to identify “abnormal” motion that may develop following injury, degeneration, or surgical intervention. Furthermore, restricting data collection and analysis to static endpoint positions prohibits the characterization of mid-range kinematics that make up the majority of spine motion during activities of daily living[9, 10]. While several studies have recorded two-dimensional, continuous cervical motion during dynamic flexion-extension, these studies failed to report intervertebral kinematics throughout the continuous motion[8, 11-13]. Thus, the high inter-subject variability in flexion-extension ROM, combined with the lack of any quantitative continuous kinematic data throughout the entire movement cycle, precludes the development of a precise and comprehensive definition of “normal” in vivo cervical kinematics during flexion-extension.

The mechanical effects of arthrodesis and disc arthroplasty on adjacent segments are often evaluated by in vitro testing of cervical specimens[14-19] and finite element models derived from in vitro tests[20-24]. The preferred in vitro testing protocol is one that most closely follows the in vivo kinematic pattern for all segments of the cervical spine[25]. Thus, the fidelity of in vitro testing is often evaluated by comparing in vivo and in vitro total range of motion measures over the entire subaxial spine or at each intervertebral motion segment[15],[26],[27]. The high variability in segmental in vivo range of motion, as well as the lack of data describing mid-range kinematics during in vivo functional loading, make it unclear how accurately the kinematic response of in vitro tests and finite element models represent actual in vivo motion during dynamic functional loading.

The current study was carried out to address the aforementioned limitations to our current knowledge of in vivo cervical spine kinematics during functional loading. The objective of this study was to quantify intervertebral kinematics during continuous, functional flexion-extension in a group of asymptomatic control subjects. As part of this objective, subject-specific disc height and static intervertebral orientation at each cervical level were used to more precisely define “normal” in vivo kinematics. The hypothesis associated with this objective was that static disc height and static orientation would be associated with kinematics at each motion segment during functional dynamic loading. Finally, in order to demonstrate the benefit of assessing intervertebral kinematics using continuous data, continuous intervertebral kinematics were compared between single-level anterior arthrodesis patients and asymptomatic controls.

Materials and Methods

Following Institutional Review Board approval, data was analyzed from 6 single-level (C5/C6) anterior arthrodesis patients (average age: 48.8 ± 6.9 yrs; 1 M, 5 F; 7.6±1.2 mo. post-surgery) and 18 asymptomatic control subjects of similar age (average age: 45.6 ± 5.8 yrs; 5 M, 13 F) who provided informed consent to participate in this research study. Arthrodesis subjects who were at least 18 years of age and scheduled to undergo (or recently received) single-level ACDF surgery were identified during clinic visits. Pregnant women, patients diagnosed with osteoporosis, and patients with any other injury or disease that interferes with spine function were excluded. Asymptomatic controls who had no history of cervical spine dysfunction or pain were recruited to approximately match the age and gender distribution of the arthrodesis subjects. Control subject recruitment was accomplished through an advertisement in an employee newsletter and word of mouth.

High-resolution CT scans (0.29×0.29×1.25 mm voxels) of the cervical spine (C2-C7) were acquired on each participant (GE Lightspeed 16). Bone tissue was segmented from the CT volume using a combination of commercial software (Mimics software, Materialise, Leuven, Belgium) and manual segmentation[28]. A three-dimensional (3D) model of each vertebra was generated from the segmented bone tissue. Markers were interactively placed on the 3D bone models to define bone-specific anatomic coordinate systems (Figure 1).

Figure 1.

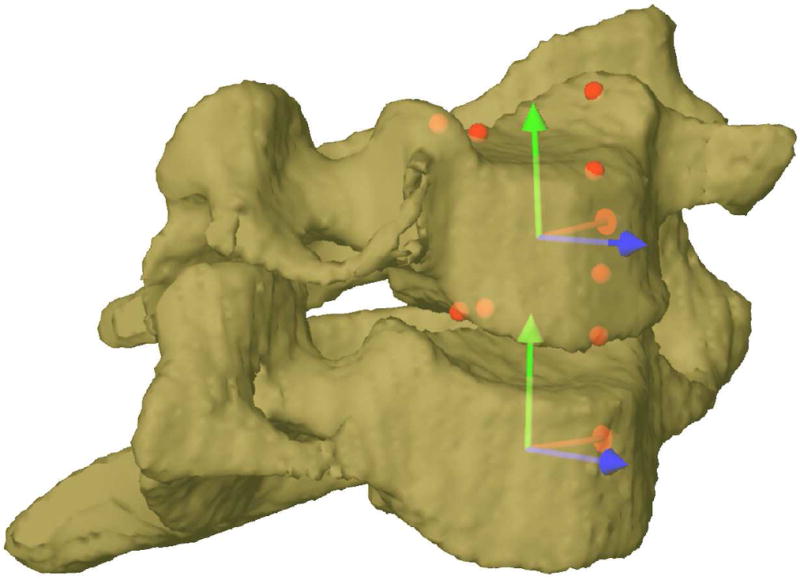
A C6/C7 motion segment demonstrating anatomic coordinate system definitions. Eight markers were placed on each bone (red spheres, shown only on C6 for clarity). Anatomic coordinate systems (red, green and blue arrows) were created within each bone using the eight markers. Relative rotation of the superior bone relative to the inferior bone was divided into flexion-extension (about the red axis), rotation (about the green axis), and lateral bend (about the blue axis) components. Relative translation was determined by the displacement between anatomic coordinate system origins and expressed relative to the inferior bone anatomic coordinate system (anterior-posterior = blue, medial-lateral = red, and superior-inferior = green).

Subjects were seated within a biplane X-ray system and directed to continuously move their head and neck through their entire range of flexion-extension (Figure 2). A metronome set at 40 to 44 beats per minute was used to ensure the participants moved at a continuous, steady pace to complete each full movement cycle in 3 seconds or less. Radiographs were collected at 30 frames per second for 3 seconds for each trial of continuous flexion-extension (X-ray generator parameters: 70 KV, 160 mA, 2.5 ms X-ray pulses; source-to-subject distance 140 cm). Radiographs were collected for 2 or 3 trials for each subject, resulting in a total of 63 movement trials analyzed for this study.

Figure 2.

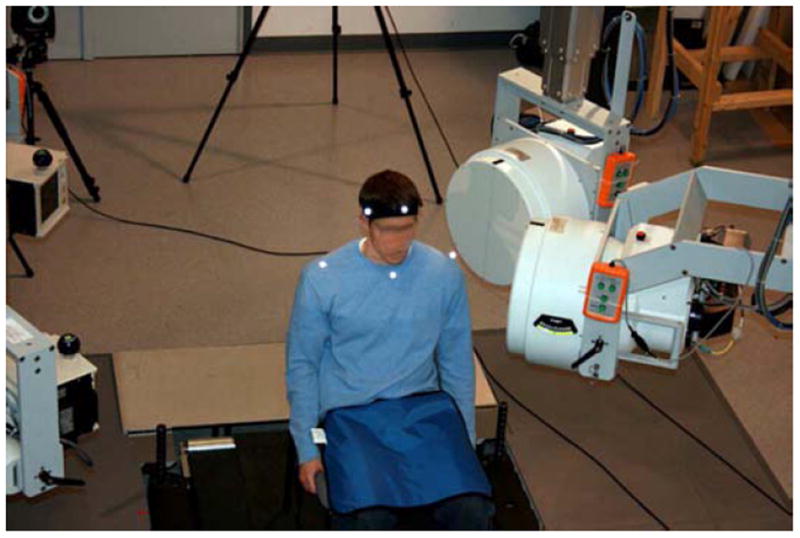
Biplane x-ray data collection system. X-ray tubes (left) directed X-rays through the subject to image intensifiers (right). 2.5 ms X-ray pulses (70 kV, 160 mA) were generated by cardiac cine-angiography generators at a rate of 30 Hz and images were collected by high-speed cameras synchronized to the x-ray pulses.

Multiple trials from the same subject were averaged to yield a single average dataset for each subject used for statistical analysis. A static trial with the subject looking forward with the head in the neutral position was also collected for each participant. The effective radiation dose for each dynamic flexion-extension motion trial was estimated to be 0.16 mSv (determined using PCXMC simulation software, STUK, Helsinki, Finland). The effective dose of a cervical spine CT scan has been reported to be between 3.0 mSv and 4.36 mSv[29, 30].

A previously validated tracking process determined three-dimensional vertebral position with sub-millimeter accuracy[31] for all static and dynamic trials. Details describing the volumetric model-based tracking process, including hardware and software specifications, calibration and distortion correction procedures, and computational algorithms have been described previously[31-34]. Tracked data was filtered at 1.0 Hz using a fourth-order, low-pass Butterworth filter with the filter frequency determined using residual analysis[35]. Six degree-of-freedom (DOF) kinematics between adjacent vertebrae (3 translations, 3 rotations) were calculated following established standards for reporting spine kinematics[36],[37]. Intervertebral position and orientation in each frame of the continuous dynamic trial were normalized to the static neutral trial for each subject. Only the flexion-extension component of vertebral rotation and the anterior-posterior component of vertebral translation were included in the current analysis, as we have previously demonstrated that intervertebral motion out of the flexion-extension plane during in vivo flexion-extension is relatively small (an average 2.1° of rotation ROM and 0.6 mm of translation ROM)[38].

C2 motion relative to C7 (C2/C7) was interpolated to obtain C2/C7 motion at 1% increments of the total cervical ROM for each participant. In this way, the total cervical spine flexion-extension ROM of each participant was standardized to 100%, allowing for comparisons among subjects. Segmental kinematics were then interpolated to obtain relative intervertebral motion for every 1% increment of C2/C7 spine motion. Flexion and extension portions of the overall movement were analyzed separately.

The static neutral trial was used to define intervertebral orientation and disc height of each motion segment for each participant. An automated computer algorithm determined disc height at each level. This algorithm identified the nucleus region of the disc on each bone surface model according to previous reports[39, 40]. Disc height measurements were then acquired within the nucleus region and across the entire central 1/3^rd of the disc width. Therefore, the disc height recorded for each disc was the average disc height within the central nucleus region when the head was in a static, upright neutral position.

A regression equation relating range of motion to disc height at each motion segment was determined (Appendix 1). A second regression equation relating static orientation to average flexion-extension angle at each motion segment was also determined (Appendix 1).

The regression equations developed using control subject data were applied to the continuous kinematic data from arthrodesis subjects to adjust flexion-extension and anterior-posterior translation according to disc height and static orientation at each motion segment.

Analysis of variance (ANOVA) was used to identify statistically significant differences in flexion-extension angle between arthrodesis and control groups at each motion segment at every 10% interval of C2/C7 ROM.

Results

Disc height was significantly correlated to ROM at the C5/C6 and C6/C7 motion segments in control subjects (Appendix Table A1). A significant relationship between static orientation and average flexion-extension angle was identified at the C2/C3, C3/C4, C4/C5 and C5/C6 motion segments during flexion and extension in control subjects (Table A2). Similarly, significant correlations between disc height and AP translation were identified at C5/C6 and C6/C7 motion segments (correlation coefficients (r) from 0.650 to 0.690; p-values from .002 to .004) and between static positioning and average AP translation value at all motion segments (correlation coefficients (r) from -0.486 to -0.776; p-values from ≤.001 to .041) in control subjects.

The average inter-subject variability in flexion-extension angle over the continuous movement path for the control group decreased 15% to 46% after accounting for differences in disc height and static orientation angle (Table 1). Similarly, the average inter-subject variability in AP translation was reduced 14% to 33% after adjusting for disc height and static orientation angle (Table 1).

Table 1.

Inter-subject variability in flexion-extension and anterior-posterior translation before and after accounting for disc height and static orientation in 18 control subjects.

	Inter-Subject Variability
	Flexion-Extension Rotation			Anterior-Posterior Translation
Motion Segment	Before Adjustment	After Adjustment	Decrease in Variability	Before Adjustment	After Adjustment	Decrease in Variability
C2/C3	2.1°	1.3°	38%	0.9 mm	0.7 mm	22%
C3/C4	3.5°	2.5°	29%	1.0 mm	0.8 mm	20%
C4/C5	2.9°	1.9°	34%	0.7 mm	0.6 mm	14%
C5/C6	2.6°	1.4°	46%	0.6 mm	0.4 mm	33%
C6/C7	2.9°	2.6°	10%	0.5 mm	0.4 mm	20%

Continuous motion path data revealed nearly identical motion paths in arthrodesis and control groups at the C2/C3 motion segment (Figure 3A, 3B). The motion paths for the two groups diverged when in a flexed orientation (at the C3/C4 motion segment) (Figure 3C, 3D) and when in an extended orientation (at the C6/C7 motion segment) (Figure 3I, 3J). The consistent offset between groups at the C4/C5 motion segment (Figure 3E, 3F) approached significance during flexion from 40% to 90% of the C2/C7 ROM (all p < .10) and during extension from 30% to 70% of the C2/C7 ROM (all p < .10). As expected, statistically significant differences between groups were identified at the C5/C6 motion segment (0% through 40% and 70% through 100%, all p < .002 for flexion and all p < .009 for extension). Significant differences were also identified at the C6/C7 motion segment near full extension (p = .013 at 0% and 10% of ROM during flexion and p = .047 at 100% ROM during extension). Differences between arthrodesis and control in AP translation continuous motion paths were consistent with these flexion-extension differences.

Figure 3.

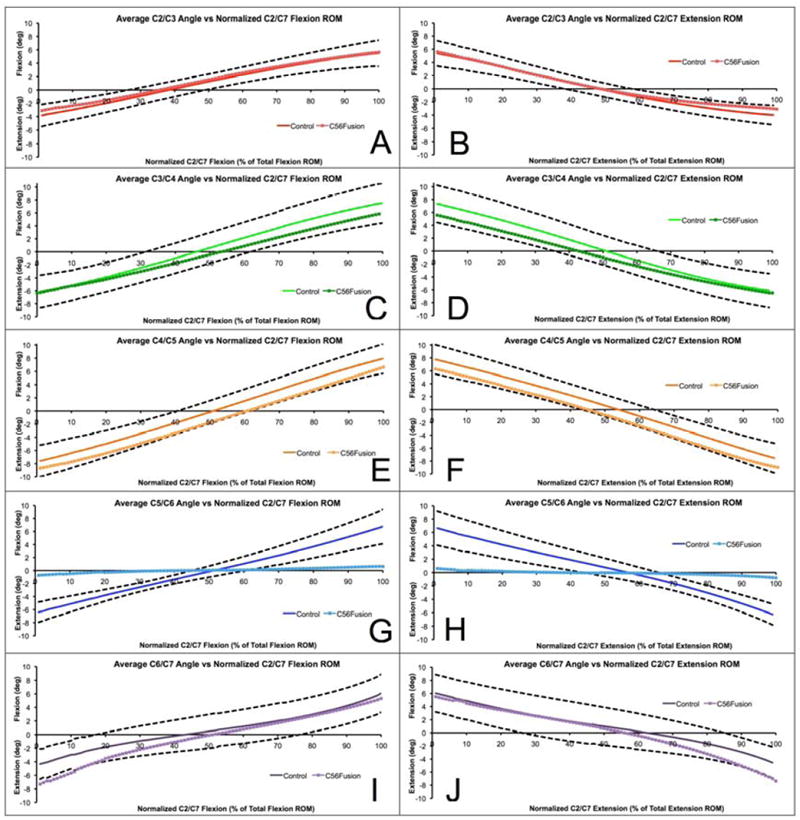
Average intervertebral flexion-extension curves for each cervical motion segment from C2/C3 (top) to C6/C7 (bottom) for the control group (solid lines) and fusion group (square markers) from full extension to full flexion (left column) and from full flexion to full extension (right column). Vertical axis represents flexion-extension angle at each individual motion segment, normalized to the neutral position. Horizontal axis represents percent of C2/C7 total ROM in flexion (left column) and extension (right column). Dashed black lines denote plus/minus one standard deviation in control group curves. Third-order polynomials fit to each segmental flexion-extension curve for the control group are provided in Table A3.

Discussion

This is believed to be the initial report of continuous cervical kinematic data during in vivo flexion-extension. The results indicate that disc height and static orientation are parameters that explain a significant portion of the inter-subject variability in cervical kinematics. The logic behind evaluating these two variables was that increased disc height would allow for increased ROM at each motion segment, and that subjects with more extended (or flexed) static orientation angles would tend to be in more extension (or flexion) during their complete active motion cycle after standardizing each subject’s ROM to 100%. This study has shown that 27% to 38% of the inter-subject variability in C5/C6 and C6/C7 flexion-extension ROM is associated with differences in disc height at C56 and C67. This finding is in agreement with a previous multivariate analysis study that determined cervical ROM was associated with the severity of disc degeneration[41]. The lack of association between disc height and ROM at more superior disc levels most likely occurred due to little variability in disc height at C23, C34 and C45 in our healthy, asymptomatic subjects. The present study also demonstrated that 31% to 48% of the inter-subject variability in average flexion-extension angle is associated with differences in static orientation at the upper motion segments (C2/C3 through C5/C6). This result indicates static orientation should be taken into account when flexion ROM and extension ROM are measured relative to the static neutral position[8],[38].

After accounting for disc height and static orientation, the average inter-subject variability in the continuous flexion-extension motion paths was 1.9°. In contrast, previous studies have reported inter-subject variability from 3.4° to 7.2° in flexion-extension range of motion[4-8]. Adjusting for disc height and static orientation reduced inter-subject variability and helped achieve the primary study objective, to develop a more precise description of intervertebral kinematics during continuous, functional flexion-extension for asymptomatic control subjects.

As demonstrated in Figure 3, the continuous intervertebral motion paths may provide useful clinical information that cannot be obtained from static flexion-extension radiographs. For example, at the C4/C5 motion segment, if only end ROM data points were selected to measure ROM, the likely conclusion would be there is no difference in kinematics one level above the arthrodesis in comparison to controls. However, the continuous motion data indicates a consistent offset toward more extension in the arthrodesis group relative to control subjects at C4/C5. This preliminary result suggests the mechanical loading applied to the C45 disc may be altered by C5/C6 arthrodesis, providing a potential explanation for the occurrence of adjacent segment degeneration superior to the arthrodesis. Similarly, the continuous motion paths for the C6/C7 motion segment indicate that from full flexion to the neutral orientation, the C6/C7 motion segment kinematics are nearly identical in control subjects and arthrodesis patients. However, as the spine moves into extension, the control group and arthrodesis patient motion paths begin to diverge. This suggests C5/C6 arthrodesis may alter the mechanics of the C6/C7 motion segment in extension, implying a potential explanation for adjacent segment degeneration inferior to the operated motion segment. The continuous kinematic results suggest that studies that report only adjacent segment total range of motion following arthrodesis or arthroplasty[11, 42, 43] may overlook potential long-term clinically significant effects of these procedures (e.g. an offset toward extension in C4/C5 found in the present study). Furthermore, static, end-range images cannot be used to identify specific ranges of motion where kinematics are altered following surgery (e.g. extreme extension affected C6/C7 kinematics in the present study).

As previously noted, data from cervical arthrodesis patients was included in the current analysis to highlight the advantages of collecting and analyzing in vivo kinematics continuously, throughout the entire flexion-extension movement. Due to the small sample of arthrodesis patients, differences between arthrodesis and control groups should only be viewed as potentially suggesting that anterior cervical arthrodesis may affect adjacent segment kinematics. A larger sample of arthrodesis patients will be necessary to conclusively establish in vivo kinematics in arthrodesis patients. Furthermore, arthrodesis patient testing occurred relatively soon after surgery. Differences between arthrodesis and control groups may become more apparent with longer-term follow-up testing. An additional limitation of the current study is the relatively narrow age range for the asymptomatic control subjects. Although the control subjects in this study were within a clinically relevant age range, continuous kinematics from younger and older asymptomatic cohorts may differ from the current results. This study was not biased in any way by the study sponsors who had no influence on the study design, data analysis or interpretation of the results.

In conclusion, inter-subject variability in cervical flexion-extension kinematics is influenced by disc height and the static orientation of each motion segment. The continuous kinematic technique described may help in the design of the next generation of motion-preserving technology, perhaps allowing for patient-specific implant designs. Continuous kinematic techniques may also detect clinically important differences between arthrodesis and arthroplasty that are not apparent at static, end range of motion positions. Finally, the continuous motion path curves may be used to evaluate and improve the fidelity of in vitro cervical spine mechanical testing protocols.

Supplementary Material

06. Figure A1.

C5/C6 flexion-extension curves before (A) and after (B) adjusting for disc height during the flexion motion in 18 control subjects. C5/C6 flexion-extension angle versus percent of C2/C7 flexion-extension ROM curves for each control subject are shown by solid lines while group means are identified by black circles. Accounting for disc height reduced inter-subject variability by adjusting the slope of the curves for individual subjects while not affecting the group mean.

07. Figure A2.

C4/C5 flexion-extension curves before (A) and after (B) adjusting for static orientation during the flexion motion in 18 control subjects. Motion paths from each individual subject are represented by solid lines, group means are identified by black circles. Accounting for static orientation reduced inter-subject variability by shifting the entire curve up or down for each subject while not affecting the group mean.

Appendix 1

Materials and Methods

Relationship Between Disc Height and Range of Motion

Pearson correlation was used to identify significant relationships between disc height and flexion-extension range of motion and between static orientation and average flexion-extension angle during the dynamic movement for control subjects. A regression equation relating disc height and flexion-extension ROM at each disc level was determined when significant correlations between these two variables were identified. This regression equation was used to predict ROM at each motion segment given the disc height. A vertebral level-specific scale factor was then calculated for each motion segment for every subject using the predicted and actual ROM using the following equation:

$$\mathit{ScaleFactor}=(\mathit{Predicted}\_\mathit{ROM}-\mathit{Actual}\_\mathit{ROM})/\mathit{Actual}\_\mathit{ROM}.$$ (1)

The scale factor and average flexion-extension angle over the entire flexion (or extension) movement were then input to equation (2), below, to determine the adjusted intervertebral flexion-extension angle at each 1% interval of C2/C7 ROM.

$$\mathit{Adjusted}\_\mathit{Angle}=\mathit{Measured}\_\mathit{Angle}+(\mathit{Measured}\_\mathit{Angle}-\mathit{Average}\_\mathit{Angle})\ast \mathit{ScaleFactor}$$ (2)

In this way, the slope of the segmental flexion-extension angle versus percent of C2/C7 ROM curves were adjusted to account for inter-subject differences in disc height (Figure A1).

Relationship Between Static Orientation and Average Flexion-Extension Angle

A second regression equation relating static orientation and average flexion-extension angle at each motion segment was determined when significant correlations between these two variables were identified. This regression equation was used to predict the average intervertebral flexion-extension angle at each motion segment given the static orientation angle. A vertebral level-specific offset value was then calculated for each motion segment for every subject using the predicted and actual average flexion-extension angle using the following equation:

$$\mathit{OffsetValue}=\mathit{Measured}\_\mathit{Average}\_\mathit{Angle}-\mathit{Predicted}\_\mathit{Average}\_\mathit{Angel}.$$ (3)

The offset value was then used to determine the adjusted intervertebral flexion-extension angle at each 1% interval of C2/C7 ROM using the equation:

$$\mathit{Adjusted}\_\mathit{Angle}=\mathit{Measured}\_\mathit{Angle}-\mathit{OffsetValue}.$$ (4)

In this way, segmental flexion-extension angle versus percent C2/C7 ROM curves were adjusted to account for inter-subject differences in static orientation (Figure A2). After segmental flexion-extension angles were adjusted to account for disc height and static orientation, as just described, a 3^rd order polynomial was fit to the group mean flexion-extension angle versus percent of C2/C7 ROM curve for each motion segment.

The process described above was repeated using anterior-posterior translation curves in place of flexion-extension rotation curves to more precisely define the anterior-posterior translation motion path during flexion-extension.

Results

Applying regression equations relating disc height to ROM reduced inter-subject variability in C5/C6 and C6/C7 flexion-extension curves by adjusting the slope of the flexion-extension curve for each participant (Figure A1).

Applying regression equations relating static orientation to average flexion-extension angle (Table A2) reduced inter-subject variability in C2/C3, C3/C4, C4/C5 and C5/C6 flexion extension curves by adjusting the mean value of the flexion-extension curve for each participant (Figure A2).

Third-order polynomials fit to flexion-extension versus percent of C2/C7 rotation ROM precisely described the mean continuous flexion-extension motion path at each motion segment for the control group (all R² > .99) (Table A3). Third-order polynomials fit to anterior-posterior translation versus percent of C2/C7 rotation ROM precisely described the mean AP translation motion path at each motion segment for the control group (all R² > .99) (Table A4).