Sparse parallel transmission on randomly perturbed spiral k-space trajectory

Abstract

Combination of parallel transmission and sparse pulse is able to shorten the excitation by using both the coil sensitivity and sparse k-space, showing improved fast excitation capability over the use of parallel transmission alone. However, to design an optimal k-space trajectory for sparse parallel transmission is a challenging task. In this work, a randomly perturbed sparse k-space trajectory is designed by modifying the path of a spiral trajectory along the sparse k-space data, and the sparse parallel transmission RF pulses are subsequently designed based on this optimal trajectory. This method combines the parallel transmission and sparse spiral k-space trajectory, potentially to further reduce the RF transmission time. Bloch simulation of 90° excitation by using a four channel coil array is performed to demonstrate its feasibility. Excitation performance of the sparse parallel transmission technique at different reduction factors of 1, 2, and 4 is evaluated. For comparison, parallel excitation using regular spiral trajectory is performed. The passband errors of the excitation profiles of each transmission are calculated for quantitative assessment of the proposed excitation method.

Introduction

Parallel transmission can reduce the excitation duration using the coil sensitivity pattern of a RF coil array (1-13). Recently, sparse undersampled k-space trajectory inspired by compressed sensing technique demonstrates the unique capability in shortening the excitation pulse width and thus accelerating the excitation (14-28). Unlike the parallel transmission technique, this method is simple and does not require specialized RF hardware. Studies show that the sparse parallel transmission, a technique combining parallel transmission and sparse undersampling, would be advantageous in fast excitation, providing augmented capability of reducing excitation time while maintaining the excitation fidelity (29,30). However, the conventional k-space trajectories take much time in traveling k-space samples. In implementing sparse parallel excitation, how to design an optimal k-space trajectory is an overarching issue needed to be addressed, which is technically challenging. Methods of designing a short k-trajectory to connect all the sparse excitation samples have been explored (30). In this study, we propose and investigate a randomly perturbed spiral k-space trajectory for sparse parallel transmission. The spiral trajectory is firstly designed using the k-space method (31,32) and then randomly perturbed by utilizing the variable density and Monto-Carlo sampling schemes (33) which are commonly used in compressed sensing MRI (34-49). Finally the gradient waveforms and parallel transmission pulses are designed. The feasibility of this method is evaluated by taking Bloch simulation of the sparse parallel transmission at different reduction rates. The accuracy of the excitation profile of each transmission is also investigated by calculating the maximum error of the passband.

Theory and methods

Figure 1 describes the design procedure of the proposed method. In this procedure, a conventional spiral k-space trajectory was designed firstly. Then, the Mote-Carlo incoherent sampling strategy was used to sample the k-space. These sparse sampling data were used to modify the path of the previous designed spiral k-space trajectory. The new trajectory was along the spiral trajectory. But if a sample generated by the sparse sampling strategy was outside the spiral trajectory, a new path with shortest distance would be generated to connect the spiral trajectory and the sparse sampling data. Thus by connecting all the sparse samples with the spiral trajectory, a randomly perturbed spiral trajectory was generated. Finally, the corresponding gradient waveforms on two dimensions were designed based on the proposed randomly perturbed spiral trajectory by using the time-optimal gradient method (50) which is able to design gradient waveforms with minimum time for arbitrary trajectory. The RF pulses of each channel for the sparse parallel transmission was then designed by using the spatial domain method (15).

Figure 1.

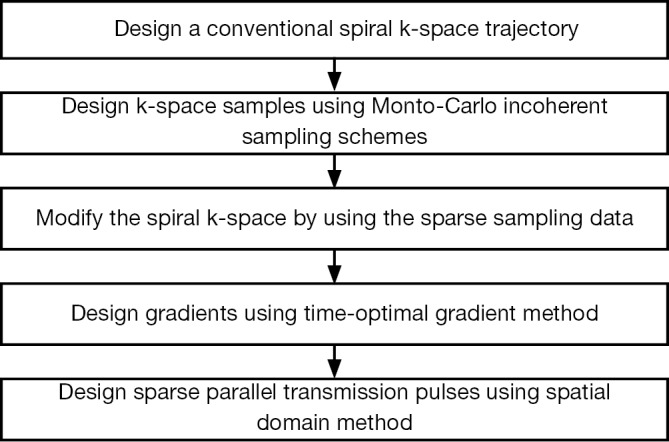
Design procedure of the sparse transmission pulses on randomly perturbed spiral k-space trajectory.

An example of the 2-dimensional sparse parallel transmission pulses on the proposed randomly perturbed spiral k-space trajectory was designed and a numerical calculation of the block equation was performed to investigate the feasibility of the proposed method and the accuracy of the excitation profile. In this experiment, the desired excitation pattern was a cylinder with 6 cm diameter and the flip angle was 90°. The excitation was performed by using a 4-element RF coil array. The sensitivity pattern of each element of the coil array is shown in Figure 2. The k-space extension was 0.5 cycle/cm and 1,200 samples were chosen using the random undersampling schemes. The sparse parallel transmission with the reduction factor of 4 was performed to maximize the acceleration capability of the design. Sparse parallel transmission with the reduction factor of 2 and a non-parallel transmission were also performed for comparison in terms of excitation accuracy. Furthermore, for validation of the proposed sparse parallel excitation method, excitation using regular spiral trajectory which had a similar length to that of sparse parallel excitation at the reduction factor of 4 was performed.

Figure 2.

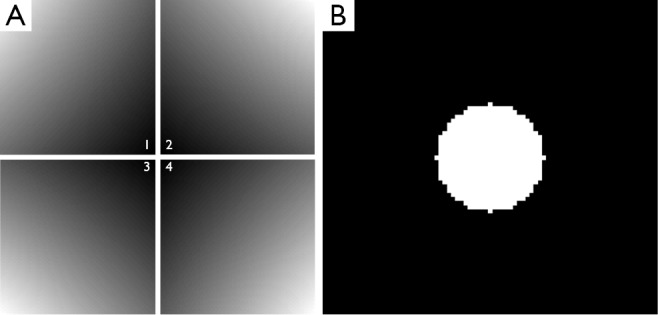
Sensitivity patterns of four elements of the RF coil array used in this study and the desired excitation profiles.

To fairly evaluate the excitation accuracy and the variation, the maximum value of the passband error was used. For 90° excitation, the desired normalized magnetization in passband is 1. Therefore the maximum passband error is defined as:

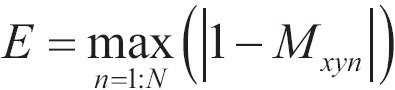[1]

In this equation, M_xyn denotes the magnetization of each position in the passband of the transverse plane of the excitation profile, while N denotes the number of the excitation positions in the passband. The maximum value reflects the worst case in the excitation profile, indicating the performance of the excitation. The smaller the error is, the more accurate the excitation is.

Results

In the sparse parallel transmission at the reduction factor of 4, the pattern of the randomly perturbed spiral k-space trajectory is shown in Figure 3A. It is illustrated that the samples are along a spiral trajectory, but randomly perturbed by incoherent data. The corresponding gradient waveforms designed based on this optimal trajectory are shown in Figure 3B. Both the gradients and the RF pulses satisfied the limitation of hardware of commercially available human MRI scanners. It is noticed that the rapid change of the gradient waveforms may present a challenging requirement on the hardware performance. Results of the excitation profiles of 4 individual RF pulses and the combined excitation profile are shown in Figure 4. The 1D plot along the central line of the 2D excitation pattern (combined) is shown in Figure 4B. The excitation error is approximately 0.2 calculated by using the Eq. [1].

Figure 3.

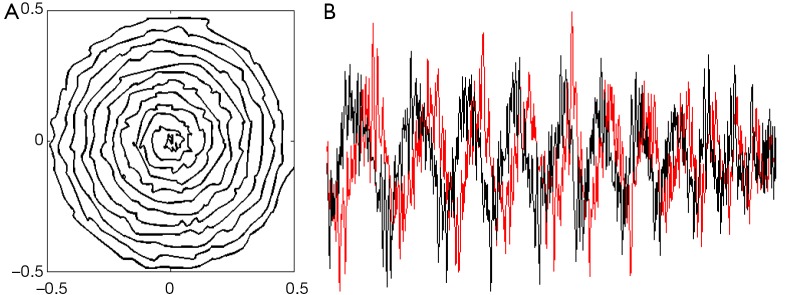
The randomly perturbed spiral k-space trajectory with the range from –0.5 to 0.5 on both directions (A), and (B) x (black) and y (red) gradient waveforms generated based on the proposed perturbed spiral k-space trajectory.

Figure 4.

Excitation profiles of the 4 individual RF pulses; (A) their combined excitation profile; (B) 1D excitation profile along the central line. The excitation error of its passband based on Eq. [1] was ~0.2.

In the comparison study, sparse parallel transmission at the reduction factor of 1 (i.e., no reduction) and 2 were also performed using the same RF coil array and the same parameters. The 1D and 2D excitation profiles of the two experiments are shown in Figure 5. At the reduction factors of 1 and 2, the excitation errors are all in the range of 0.2 calculated by using Eq. [1]. This result indicates that the excitation errors of the sparse parallel transmission pulses are at the same level of the conventional non-parallel transmission pulses, demonstrating that the proposed perturbed spiral k-space trajectory is feasibility and efficiency in fast MR excitations.

Figure 5.

(A,B) 2D and 1D excitation profiles of the non-accelerated transmission (i.e., reduction rate of 1); (C,D) 2D and 1D excitation profiles of the sparse parallel transmission at the reduction rate of 2 using proposed randomly perturbed spiral trajectory; (E,F) 2D and 1D excitation profiles of an RF pulse on regular spiral k-space trajectory for comparison purpose. The passband error of the regular spiral RF pulse was in the region of 0.3, which was worse than that of the proposed method (0.2).

Figure 5E,F shows the results of excitations using conventional spiral k-space trajectory which has a similar length to that of sparse parallel excitation at the reduction rate of 4. Its passband error calculated from Eq. [1] was approximately 0.3, showing a degraded excitation accuracy over that of the proposed sparse parallel transmission on randomly perturbed spiral trajectory.

Discussion and conclusions

The method of designing sparse parallel transmission RF pulses on a randomly perturbed spiral sparse k-space trajectory is proposed and investigated. The optimal k-trajectory traveling through the sparse k-space samples shortens the corresponding gradient waveforms and pulse width. The promising result of Bloch simulation has demonstrated the feasibility and efficiency of this method. The small ripples on both the in-slice and out-of-slice regions are partially due to the imperfection of the k-space undersampling and RF pulses, which need to be improved for better excitation accuracy. The comparison result of the sparse parallel transmissions at the reduction factors of 2 and 4, and the non-parallel transmission demonstrates that the performance of the sparse parallel transmission at high reduction factors is at the same level of the non-parallel transmission strategy. Furthermore, this method can be also applied to non-parallel pulse designs and 3D spatial selective pulse designs.