Titin-mediated control of cardiac myofibrillar function

Abstract

According to the Frank-Starling relationship, ventricular pressure or stroke volume increases with end-diastolic volume. This is regulated, in large part, by the sarcomere length (SL) dependent changes in cardiac myofibrillar force, loaded shortening, and power. Consistent with this, both cardiac myofibrillar force and absolute power fall at shorter SL. However, when Ca²⁺ activated force levels are matched between short and long SL (by increasing the activator [Ca²⁺]), short SL actually yields faster loaded shortening and greater peak normalized power output (PNPO). A potential mechanism for faster loaded shortening at short SL is that, at short SL, titin becomes less taut, which increases the flexibility of the cross-bridges, a process that may be mediated by titin's interactions with thick filament proteins. We propose a more slackened titin yields greater myosin head radial and azimuthal mobility and these flexible cross-bridges are more likely to maintain thin filament activation, which would allow more force-generating cross-bridges to work against a fixed load resulting in faster loaded shortening. We tested this idea by measuring SL-dependence of power at matched forces in rat skinned cardiac myocytes containing either N2B titin or a longer, more compliant N2BA titin. We predicted that, in N2BA titin containing cardiac myocytes, power-load curves would not be shifted upward at short SL compared to long SL (when force is matched). Consistent with this, peak normalized power was actually less at short SL versus long SL (at matched force) in N2BA-containing myocytes (N2BA titin: ΔPNPO (Short SL peak power minus long SL peak power) = −0.057 ± 0.049 (n=5) versus N2B titin: ΔPNPO = +0.012 ± 0.012 (n=5)). These findings support a model whereby SL per se controls mechanical properties of cross-bridges and this process is mediated by titin. This myofibrillar mechanism may help sustain ventricular power during periods of low preloads, and perhaps a breakdown of this mechanism is involved in impaired function of failing hearts.

INTRODUCTION

Muscles in vivo shorten against a load and, thus, generate power (which is work capacity per unit time). The velocity that muscle shortens is inversely related to the load on (or force produced by) the muscle with the relationship between force and shortening velocity generally expressed by a rectangular hyperbola [1]. Each point on the force-velocity relationship can be used to estimate power output (by simply multiplying force x velocity), where power is zero at the two extremes of the force-velocity relationship and reaches a maximum at intermediate loads. During oscillatory activity, in vivo skeletal muscles [2] and presumably cardiac muscle operate at intermediate forces and velocities where power is close to maximum. In the heart, ventricular stroke volume is determined by myocardial power output, which dictates the amount that the myocardium shortens against external loads arising from arterial impedance and ventricular wall stress. Since the ventricles act as a functional syncytium and presumably all myocytes are electrically activated during each heartbeat, stroke volume is determined by the power generated by individual cardiac myocytes. However, the exact mechanisms that determine power output of individual cardiac myocytes remain unanswered and have been the focus of several studies. One important determinant of myocyte power is myofibrillar sarcomere length, which is thought to underlie the Frank-Starling relationship, whereby greater end-diastolic ventricular volume increases stroke volume. We previously investigated sarcomere length dependence of loaded shortening and power output and found slower loaded shortening and less power at short sarcomere length over all absolute loads and this sarcomere length dependence persisted even when force-velocity curves were normalized for differences in isometric force, i.e., loaded shortening velocity was slower at short sarcomere length at loads less than ~40% isometric force [3]. A plausible mechanism to explain slower loaded shortening and decreased power output at short sarcomere length is the coincident decrease in thin filament activation levels at short sarcomere length given that force was lower due to the well described sarcomere length dependence of Ca²⁺ sensitivity of force [4]. However, when Ca²⁺ activated force was matched at short sarcomere length to those at long SL (by increasing the activator [Ca²⁺]) short sarcomere length actually yielded slightly faster loaded shortening velocities and greater peak normalized power output [3]. This suggests a myofibrillar mechanism that tends to speed loaded cross-bridge cycling to minimize the fall of power at short sarcomere length. Interestingly, treatment of myocytes with 2% dextran to compress the myofilament lattice at short sarcomere length also caused faster loaded shortening compared to long sarcomere lengths, again implicating a myofibrillar mechanism that leads to faster loaded cross-bridge cycling at short sarcomere length [3]. This finding of faster loaded shortening at short sarcomere length challenges classic models of cross-bridge and muscle mechanics [5] and suggests that the mechanical state of one population of cross-bridges affects the activity of other cross-bridge populations by, for example recruitment and subsequent retention of non-cycling cross-bridges into the cycling force-generating population. Physiologically, these results implicate a myofibrillar mechanism that may help sustain ventricular power during periods of lower preloads, and perhaps a breakdown of this mechanism may contribute to impaired function of failing hearts.

Our working hypothesis for faster loaded shortening at short sarcomere length (when force is matched) is that as sarcomere length is shortened, titin becomes less taut which reduces the constraint of cross-bridges, a process that may be mediated in part by titin's interaction with myosin binding protein-C (MyBP-C) on the thick filament [6] (see Figure 1). According to this model, a slackened titin yields greater myosin head radial and azimuthal mobility [3]. This leads to faster cross-bridge cycling by creating more flexible cross-bridges that are more likely to maintain thin filament activation, which allows more force-generating cross-bridges to work against a fixed load. Importantly, though, this mechanism alone cannot overcome the decrease in the number of cross-bridges induced, in part, by increased lattice spacing that normally occurs with short sarcomere length, which is why loaded shortening is slower at short sarcomere length when activator [Ca²⁺] is the same between long and short SL. These mechanistic ideas are consistent with findings that (i) titin binds C-terminal domains of MyBP-C [7], (ii) gene targeted ablation of MyBP-C caused radial displacement of cross-bridges away from the thick filaments [8], and (iii) ablation of MyBP-C yielded faster loaded shortening in cardiac myocyte preparations [9].

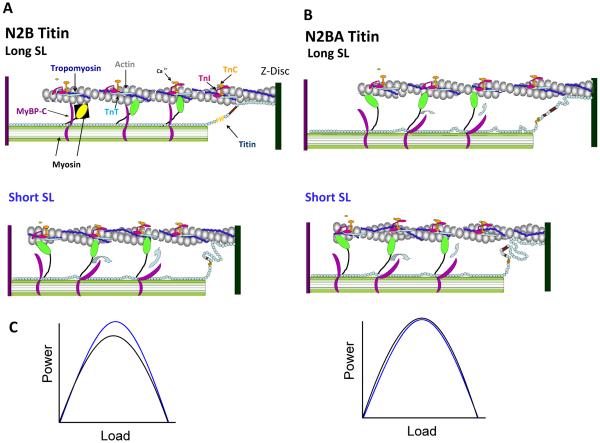
Figure 1.

Myofibrillar model for altered cross-bridge kinetics mediated by sarcomere length and titin. A. Representation of a half-sarcomere containing N2B titin at two different sarcomere lengths. At long sarcomere length titin is extended and provides passive tension that may help maintain extension of MyBP-C from the thick filament to constrain the myosin cross-bridges. At short sarcomere length, titin is slackened and this coincident decrease in passive tension may alleviate MyBP-C constraint of the cross-bridge, leading to more flexible crossbridges that act to more readily retain thin filament activation during active shortening. B. Half sarcomere depiction in the presence of N2BA titin. Even at long sarcomere length titin is slightly slackened, which would increase flexibility of the cross-bridges and sustain thin filament activation allowing more cross-bridges to work against the load yielding less load per bridge so each cross-bridge cycles faster. C. The augmented shortening speed at long sarcomere length in N2BA myofibrils is predicted to eliminate faster loaded shortening at short sarcomere length (right panel) observed in N2B-containing myofibrils (left panel) when force is matched between long and short SL. (Short SL is represented by the blue line; long SL is represented by the black line.) (Sarcomeric protein and protein domains are not drawn to scale.)

We tested titin's role in modulating loaded shortening and power output by measuring SL dependence of power at matched forces in rat skinned cardiac myocytes containing either N2B titin or the longer, more compliant N2BA titin. We predicted that a more compliant titin would augment loaded shortening at long sarcomere length leading to less overshoot in loaded shortening and power at short sarcomere length (and matched force) in N2BA titin myocytes.

We also tested the hypothesis that faster rates of force development observed at short sarcomere lengths [10–12] is mediated by titin compliance, which may affect the extent of cooperative thin filament activation. We predicted faster rates of force development in myocyte preparations containing N2BA titin due to less spread of cooperative activation along thin filament and recruitment of force-generating cross-bridges (which takes time and slows force development) following a mechanical slack-re-stretch maneuver.

METHODS

Experimental animals

Adult mutant N2BA titin rats (n = 9) (which are 50% Brown-Norway, 25% Fischer, 25% Sprague-Dawley hybrid rats) [13] and control littermates (n = 7) were housed in groups of 2 or 3 and provided access to food and water ad libitum. All procedures involving animal use were performed according to the Animal Care and Use Committee of the University of Missouri.

Solutions

The compositions of relaxing and activating solutions were as follows (in mmol/L, chemicals obtained from Sigma at highest possible purity): free Mg²⁺ 1, EGTA 7, MgATP 4, imidazole 20, and creatine phosphate 14.5 (pH 7.0); specific [Ca²⁺] between 10^−4.5 (maximal Ca²⁺ activating solution) and 10⁻⁹ (relaxing solution); and sufficient KCl to adjust ionic strength to 180 mmol/L. Preceding each Ca²⁺-activation, myocyte preparations were immersed for 30 sec in a solution of reduced Ca²⁺-EGTA buffering capacity, which was identical to normal relaxing solution except that EGTA was reduced to 0.5 mM. This protocol resulted in more rapid development of steady state force during subsequent Ca²⁺ activation. Relaxing solution, in which the ventricles were mechanically disrupted and myocytes were resuspended, contained 2 mM EGTA, 5 mM MgCl₂, 4 mM ATP, 10 mM imidazole, and 100 mM KCl at pH 7.0 with the addition of a protease inhibitor cocktail (Set I Calbiochem, San Diego, CA).

Myocardial preparations

Skinned cardiac myocytes were obtained by mechanical disruption of hearts from rats as described previously [14]. Briefly, rats were anaesthetized by inhalation of isoflurane (20% v/v in olive oil), and their hearts were excised and rapidly placed in ice cold relaxing solution. The left ventricle was separated from the right ventricle and dissected from the atria, cut into 2–3 mm pieces and further disrupted for 5 seconds in a Waring blender. The resulting suspension of cells was centrifuged for 105 sec at 165 × g, after which the supernatant fluid was discarded. The myocytes were skinned by suspending the cell pellet for 5 min in 0.3 % ultrapure Triton X-100 (Pierce Chemical Co.) in cold relaxing solution. The skinned cells were washed twice with cold relaxing solution, suspended in 10 ml of relaxing solution and kept on ice.

Experimental Apparatus

The experimental apparatus for mechanical measurements of myocyte preparations was similar to one previously described [14]. Briefly, a myocyte was attached between a force transducer and high speed motor by gently placing the ends of the myocyte into stainless steel troughs (25 gauge). The ends of the myocyte were secured by overlaying a 0.5 mm long piece of 3-0 monofilament nylon suture (Ethicon, Inc.) onto each end of the myocyte, and then tying the suture into the troughs with two loops of 10-0 monofilament suture (Ethicon, Inc.). The attachment procedure was performed under a stereomicroscope (~100× magnification) using fine tipped forceps.

Prior to mechanical measurements the experimental apparatus was mounted on the stage of an inverted microscope (model IX-70, Olympus Instrument Co., Japan), which rested on a pneumatic anti-vibration table with a cut-off frequency of ~1 Hz. Force measurements were made using a capacitance-gauge transducer (Model 403-sensitivity of 20 mV/mg plus a x10 amplifier and resonant frequency of 600 Hz; Aurora Scientific, Inc., Aurora, ON, Canada). Length changes during mechanical measurements were introduced at one end of the preparation using a DC torque motor (model 308, Aurora Scientific, Inc.) driven by voltage commands from a personal computer via a 12-bit D/A converter (AT-MIO-16E-1, National Instruments Corp., Austin, TX, USA). Force and length signals were digitized at 1 kHz using a 12-bit A/D converter and each was displayed and stored on a personal computer using custom software based on LabView for Windows (National Instruments Corp.). Sarcomere length was set using IonOptix SarcLen system (IonOptix, Milton, MA), which used a fast Fourier transform algorithm of the video image of the myocyte. For these experiments, muscle length recordings were used to calculate velocity of shortening (in units of um/sec) since previous work indicated that muscle length and sarcomere length traces are closely matched over the entire range of relative loads [3]. Video microscopy was completed using a 40x objective (Olympus UWD 40) and 25x intermediate lenses.

Force-velocity and power-load measurements

The protocol to obtain force-velocity and power-load measurements has been described in detail [14] and all measurements were done at 12 ± 1°C. An attached myocyte was first transferred into maximal Ca²⁺ activating solution (pCa 4.5) and allowed to obtain maximal steady-state isometric force. It was then transferred to a submaximal Ca²⁺ activating solution (which yielded ~50% maximal force) and a series of sub-isometric force clamps were applied to determine isotonic shortening velocities. The isotonic force was maintained using a servo system for 150–250 msec while muscle length changes during this time were monitored. Following the force clamp, the myocyte was slackened to near zero force to estimate the relative load sustained during the isotonic shortening, after which the myocyte was re-extended to its starting length. The myocytes were kept in submaximal Ca²⁺ activating solution 2–4 minutes during which 10–20 force clamps were performed without significant loss of force. After obtaining a force-velocity relationship, the myocyte preparation was activated again in maximal Ca²⁺ activation solution and if force fell below 80% of initial force, data from that myocyte were discarded. Force-velocity measurements were obtained in this manner for all experimental conditions.

Measurement of rate constant of force development

Measurement of force development kinetics was accomplished using a protocol previously described [15]. Briefly, a myocyte in activating solution was allowed to develop steady-state force after which it was rapidly slacked by 15–20% of original myocyte length (L₀) and held for 20 msec and then was rapidly re-stretched to a value slightly greater than L₀ for 2 msec before it was returned to L₀. This slack-re-stretch maneuver is thought to cause dissociation of crossbridges and redistribution to pre-force generating states, and thus force redevelopment arises from re-attachment of crossbridges to the thin filament and/or subsequent transition to force generating states.

Data analyses

Myocyte length traces, force-velocity curves, and power-load curves were analyzed as previously described [14]. Myocyte length and sarcomere length traces during loaded shortening were fit to a single decaying exponential equation:

$$\mathrm{L}={\mathrm{Ae}}^{-kt}+\mathrm{C},$$ (1)

where L is cell length at time t, A and C are constants with dimensions of length, and k is the rate constant of shortening (k_shortening). Velocity of shortening at any given time, t, was determined as the slope of the tangent to the fitted curve at that time point. In this study velocities of shortening were calculated by extrapolation of the fitted curve to the onset of the force clamp (i.e., t = 0).

Hyperbolic force-velocity curves were fit to the relative force-velocity data using the Hill equation [1]

$$(\mathrm{P}+a)(\mathrm{V}+b)=({\mathrm{P}}_{\mathrm{o}}+a)b,$$ (2)

where P is force during shortening at velocity V; P_o is the peak isometric force; and a and b are constants with dimensions of force and velocity, respectively. Power-load curves were obtained by multiplying force x velocity at each load on the force-velocity curve. The optimum force for mechanical power output (F_opt) was calculated using the equation [16]

$${\mathrm{F}}_{\text{opt}}={({\mathrm{a}}^2+\mathrm{a}\cdot {\mathrm{P}}_{\mathrm{o}})}^{1∕2}-a.$$ (3)

Curve fitting was performed using a customized program written in Qbasic, as well as commercial software (Sigmaplot).

Tension redevelopment following slack-re-stretch maneuver was fit by a single exponential equation:

$$\mathrm{F}={\mathrm{F}}_{\text{max}}(1-{\mathrm{e}}^{-ktr\mathrm{t}}),$$ (4)

Where F is tension at time t, F_max is maximal tension, and k_tr is the rate constant of force development.

To determine if SL significantly affected loaded shortening velocity, power, andk_tr, paired t tests were used. Student's t test was used to test for differences in velocity, power, and k_tr between N2B titin and N2BA titin cardiac myocyte preparations. In all cases, P < 0.05 was accepted as a statistically significant difference. n = the number of myocyte preparations.

RESULTS

Rat model of varied titin isoform content in myofilaments

An autosomal dominant rat mutation model was utilized that phenotypically alters the splicing patterns of titin in order to investigate the role that titin plays in mediating the sarcomere length dependence of loaded shortening and power output in cardiac myocytes. The mutation results in a natural expression of a larger titin molecule (N2BA, 3.9 MDa) compared to the normally expressed (N2B, 3.0 MDa) [13]. The use of this model offered three important experimental design advantages to test titin's role in myocyte power generation; (i) it precludes the use of trypsin that has been previously used to clip titin [17–19] but likely has confounding effects due to putative ubiquitous cleavage targets, (ii) it allows for comparison between ventricular myocytes as opposed to comparing myocytes from ventricles versus atria (which have varied muscle regulatory isoforms), and (iii) since the rat model is a genetic variant on Sprague Dawley hybrid background (25% Sprague-Dawley , 25% Fischer, 50% Brown-Norway), this allows for better comparisons with much of our previous mechanistic work on myocyte power that used Sprague Dawley rat cardiac myocyte preparations [3].

Titin-mediated effects on loaded shortening and power in skinned cardiac myocytes

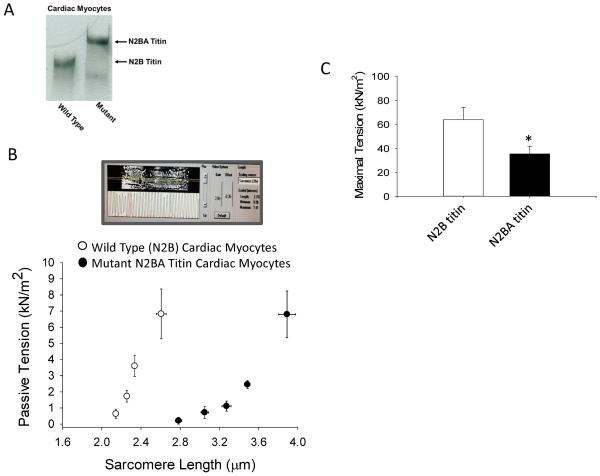
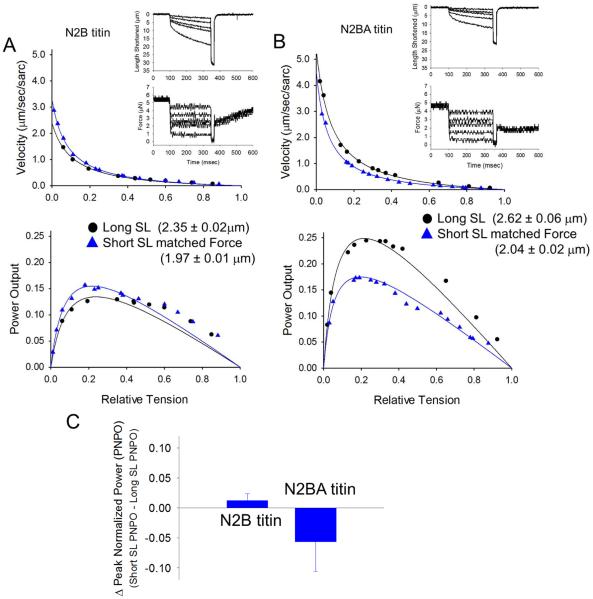
We tested a conceptual model by which sarcomere length mediates loaded shortening velocity, whereby cross-bridge constraint is mediated by titin strain (Figure 1). According to this scheme, at short sarcomere length titin strain is reduced and consequently cross-bridges are more flexible to maintain thin filament activation thereby speeding loaded shortening. If this is in fact the case, a more compliant (longer N2BA) titin should attenuate the greater power output at short sarcomere length (at matched force) by essentially normalizing cross-bridge flexibility at the two sarcomere lengths. For these experiments myocyte preparations were used from wild-type (N2B titin) and mutant (N2BA titin) rats. Titin was evaluated in myocytes by SDS-agarose gels [13], which separated the smaller N2B and the larger N2BA titin exclusively in wild-type and mutant rats, respectively (Figure 2). Even though both N2B and N2A elements are extensible, the N2BA isoform is considerably more compliant since: (i) it has more Ig domains than N2B titin; (ii) the PEVK region is longer in N2BA titin than in N2B titin; and (iii) the N2BA titin has the N2A region that is also extensible [13]. Consistent with this, sarcomere length-passive tension relationships were shifted markedly to the right in N2BA-titin containing myocytes (Figure 2B). N2BA myocytes also developed less force during maximal Ca²⁺ activations (Figure 2C), which is consistent with a previous report that incorporated permeabilized multi-cellular myocardial preparations from wild-type and mutant N2BA rats [12]. Regarding isotonic contractions, N2B myocytes exhibited faster loaded shortening and greater power at short sarcomere length (SL = 1.97 ± 0.01 μm ) than long sarcomere (SL = 2.35 ± 0.02 μm) when force was matched (Figure 3A) (a result consistent with previous work [3]). In contrast, loaded shortening velocity and power were less at short sarcomere length (SL = 2.04 ± 0.02 μm ) (matched force) than long sarcomere length (SL = 2.62 ± 0.06 μm ) in N2BA myocytes (Figure 3B). Interestingly, loaded shortening and power were similar at short sarcomere length between groups while both variables where greater at long sarcomere length in N2BA myocytes compared to N2B myocytes. This result is consistent with a model by which sarcomere length regulates loaded shortening velocity by altering cross-bridge flexibility that is mediated by titin.

Figure 2.

A. SDS-agarose gel analysis of titin isoforms from wild-type (N2B titin) and mutant RBM20 (N2BA titin) rat ventricular myocytes using methods described in [17]. B. Top panel, N2BA myocyte preparation image and sarcomere length measurement in pCa 9.0. Bottom panel, sarcomere length-passive tension relationships for N2B (n=5) and N2BA (n=5) skinned cardiac myocyte preparations. C. Bar plot of maximal Ca²⁺-activated tension (mean ±SEM, p<0.05) in N2B titin (n=10) and N2BA titin (n=8) containing skinned cardiac myocyte preparations.

Figure 3.

Force-velocity and power-load curves from an N2B titin-containing myocyte (A) and N2BA titin-containing myocyte (B) at long sarcomere length (black circles) and short sarcomere length at matched calcium activated force levels (blue triangles). Insets are force clamps (for 250ms) and length traces during near half-maximal Ca²⁺ activations. C. Bar plot shows the difference (means ± SD) in peak power between short SL (matched force) minus long SL for N2B (n=5) and N2BA (n=5) myocytes.

Titin-mediated effects on rates of force development in skinned cardiac myocytes

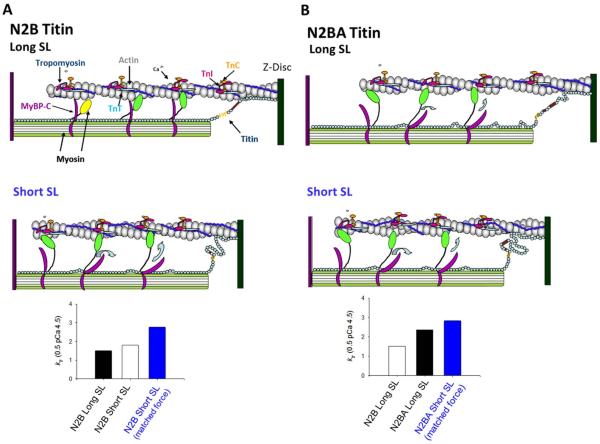
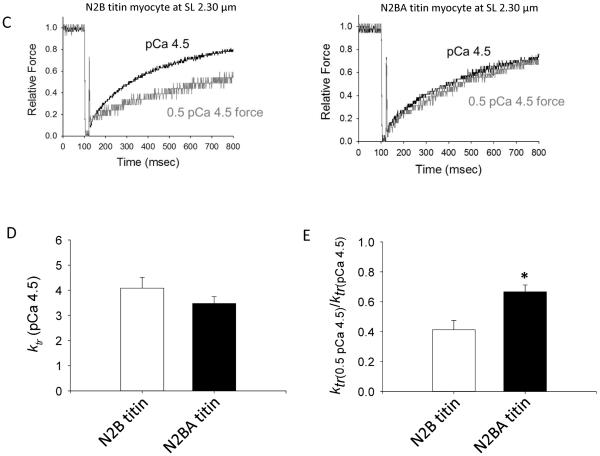
Several studies have observed that the rate of myocardial force development is faster at short sarcomere length than at long sarcomere length [3, 10–12, 20, 21]. This myofibrillar biophysical response is not necessarily intuitive because shorter sarcomere length reduces active force, thus, sarcomere length appears to override the well characterized Ca²⁺ activation dependence of force development rates (often assessed by the rate constant of force development (k_tr)) [10, 22–25]. We propose a theoretical model by which the axial propagation of thin filament activation, due perhaps to tropomyosin persistence length (i.e., bending rigidity), changes with sarcomere length and varied titin isoforms (Figure 4A & B). This is speculated to arise from mechano-transduction of titin radial forces both toward the Z disk (barbed end of actin filament) and toward the H zone (pointed end of the actin filament), as well as titin's direct interaction with the thin filament in its PEVK region [26] and possibly its indirect interaction via cMyBP-C [27]. According to this model, faster force development at short SL arises from decreased tropomyosin persistence length (i.e., less bending rigidity) and, thus, less cooperative recruitment of force-generating cross-bridges. The extent of cooperative activation or recruitment of cross-bridges from the non-cycling population to the cycling force-generating pool has been both shown experimentally [28] and modeled [29] to limit force development rates. According to this idea, force development rates (i.e., k_tr) during submaximal Ca²⁺ activations should be greater in myocyte preparations with longer more-compliant N2BA titin (theoretical bar plots in Figure 4B), whereby less taut titin yields less cooperative axial spread of cross-bridge recruitment and higher k_tr values. Consistent with this, even though k_tr values were not different between N2B and N2BA myocytes during maximal Ca²⁺ activation (Figure 4D), k_tr was faster in N2BA myocytes during submaximal Ca²⁺ activations (assessed by the k_tr ratio between half-maximal Ca²⁺ activated force (0.5 pCa 4.5) and maximal Ca²⁺ activation (pCa 4.5) (Figure 4E). These results support a model whereby titin mediates kinetics of force development in cardiac myofilaments by altering cooperative spread of thin filament activation.

Figure 4.

A & B. Theoretical model of thin filament compliance (e.g., tropomyosin persistence length) with sarcomere length and longer titin isoforms. Faster force development at short SL (bar plots in A) may arise from greater thin filament compliance and less cooperative recruitment of force-generating cross-bridges. According to this idea force development rates (indexed by k_tr) should be greater in myocytes with longer more-compliant N2BA titin (theoretical bar plots in panels A & B). C–E. While k_tr during maximal Ca²⁺ activation was not different between N2B and N2BA myocytes at sarcomere length 2.30 μm (C & D), k_tr was faster in N2BA myocytes during submaximal Ca²⁺ activations (C & E). This difference is more evident when assessed by the k_tr ratio between half-maximal Ca²⁺ activated force (0.5 pCa 4.5) and maximal Ca²⁺ activation (pCa 4.5) (E). Bar plots are means ± SEM; *p<0.05, n = 7 for each group.

DISCUSSION

The power output generated by cardiac myocytes is a key determinant of the beat-to-beat regulation of ventricular stroke volume. Myocyte power output has been determined to be regulated by a number of biochemical, biophysical, and physiological factors. For instance, power output is tightly regulated by physiological levels of activator Ca²⁺ concentrations and this appears to be due, at least in part, to the number of strongly bound cross-bridges made available by thin filament activation [14, 30]. Also, during submaximal Ca²⁺ activations, loaded shortening velocity slows during a force clamp, a process most likely regulated by cooperative deactivation of the thin filament during shortening as cross-bridges detach [14, 21, 30]. In addition, power output is modulated in a complex manner by sarcomere length, which is a biophysical factor that is likely a major contributor to the Frank-Starling relationship. We previously found a sarcomere length dependence of loaded shortening and power over the entire range of absolute loads and this sarcomere length dependence persisted even when force-velocity curves were normalized for differences in isometric force [3]. A plausible mechanism to explain slower loaded shortening and decreased power output at short sarcomere length is the coincident decrease in thin filament activation levels (defined as sites on thin filament made available for myosin cross-bridges) at short sarcomere length given that force was lower due to the well described length dependence of Ca²⁺ sensitivity of force (for review see [4]). Consistent with this, when Ca²⁺ activated force was matched at short sarcomere length and long sarcomere length, short sarcomere length yielded faster loaded shortening velocities and greater peak normalized power output [3]. Physiologically, these results implicate a myofibrillar mechanism to help sustain ventricular power during periods of lower preloads and considerable myocyte shortening. In this study we tested a potential mechanism for faster loaded shortening at short sarcomere length (when force is matched). The proposed mechanism is, as sarcomere length is shortened, titin becomes less taut and this reduces the constraint of the cross-bridges. The increase in myosin head radial and azimuthal mobility leads to faster cross-bridge cycling either directly or by these more flexible cross-bridges having a longer actin interaction distance that tends to maintain thin filament activation; this would yield a greater number of cross-bridges in the cycling, force-generating pool providing more cross-bridges to work against a fixed load (i.e., less load per cross-bridge). This proposed mechanism was tested by utilizing myofilaments that contain a larger, more compliant titin molecule (N2BA type 3.9 MDa) compared to the normally expressed (N2B 3.0 MDa) [13]. Myocytes with N2BA titin were predicted to have power-load curves that were shifted upward at long sarcomere length compared to N2B myocytes. We indeed found that N2BA myocytes had elevated loaded shortening and power at long sarcomere length compared to N2B myocytes, which is consistent with the idea that titin modulates cross-bridge mobility and contributes to sarcomere length control of loaded shortening and power. This mechanism may help ensure adequate ejection and pressure and could be especially important to help balance titin's other role of controlling lattice spacing as a function of sarcomere length [18]. In this capacity, titin mediates the radial force between thin and thick filaments such that when SL is shortened the titin-mediated force between filaments is reduced and interfilament lattice spacing is increased, which would tend to reduce the number of force-generating cross-bridges and slow loaded shortening. A final point in support of the cross-bridge flexibility model is that PKA-mediated phosphorylation of cMyBP-C also has been shown to increase both cross-bridge radial movement (i.e., flexibility) [31–33] and myocyte power output [34, 35].

Cardiac myofibrillar mechanics studies have observed faster force development rates at short sarcomere length [3, 10–12, 20, 21]. This creates an interesting conundrum; how does short sarcomere length and its consequential force decline supersede the well-defined Ca²⁺ activation dependence of force development rates [10, 22–25])? According to our thick filament model (Figure 1), cross-bridge mobility increases at short sarcomere but this would tend to increase the extent of cooperative activation, which is predicted to slow, not speed, force development rates. Thus, we propose that at short sarcomere, titin is less taut and elicits less strain on thin filament proteins. This may cause a decrease in the persistence length of tropomyosin via subtle conformational changes in tropomyosin by mechanical forces transduced toward the pointed end of actin filaments by thin filament proteins. This, in turn, would yield less cooperative spread of activation (Figure 4), which is modeled to limit rates of force development [29]. According to this idea, force development rates should be faster in myocyte preparations containing N2BA titin because of attenuated cooperative spread of activation along the thin filament. Consistent with this idea, k_tr values were greater in N2BA myocytes during submaximal Ca²⁺ activations, this was evident when k_tr during submaximal Ca²⁺ activations were expressed as fraction of k_tr values during maximal Ca²⁺ activations. A qualitatively similar increase in the ratio of k_tr during submaximal Ca²⁺ activations to k_tr during maximal Ca²⁺ activations was observed in skinned multicellular myocardial preparations containing N2BA titin compared to those containing N2B titin [12]. Taken together, these results support a model whereby titin mediates kinetics of force development in cardiac myofilaments by perhaps altering tropomyosin persistence length and subsequent cooperative spread of thin filament activation, which is generally consistent with the mechanism by which titin is projected to be a stress/strain sensor for a plethora of cellular signals [36]. However, force redevelopment rates may change as a function of titin strain independent of any effects on tropomyosin. For example, it is possible that more flexible cross-bridges induced by either short sarcomere length and/or more compliant titin molecules may not be stiff enough to cooperatively activate thin filaments (and slow force development), but once bound to actin they may be sufficient to sustain thin filament activation during load clamps (or, more physiologically, during mid-to-late ejection), a process that would tend to speed loaded shortening.

Last, it remains unclear whether changes in N2BA/N2B titin ratios are beneficial or maladaptive for ventricular performance [37, 38]. Interestingly, the expression of N2BA was found to increase in failing hearts of some patients with coronary artery disease [39, 40] and with non-ischemic dilated cardiomyopathy [41]. The physiological consequence of greater N2BA titin content in mammalian ventricular performance is debatable. On the one hand, greater myofibrillar N2BA titin would tend to reduce passive tension over the working sarcomere length of the ventricle; this may assist in ventricular filling by lowering ventricular stiffness and, thus, lowering diastolic ventricular filling pressure. On the other hand, greater N2BA may hinder diastolic filling by (i) reducing cooperative deactivation of thin filaments by more flexible cross-bridges and (ii) lowering the elastic recoil of myofilaments after systole. These two biophysical processes would hinder ventricular filling by prolonging systole and reducing suction of blood early in diastole, respectively. When optimized, both these processes could be very important for ideal filling and supplying adequate end-diastolic volumes to permit the ventricles to work near the plateau of the Frank-Starling relationship. This is especially important in times of high peripheral demands, where sympathetic drive is heightened and heart rates are elevated to diminish diastolic time.

From a systolic ejection standpoint, more compliant N2BA titin may (i) diminish the steepness of the Frank-Starling relationship by attenuating the changes in lattice spacing and consequent changes in cross-bridge binding probability; (ii) assist ejection by yielding more flexible cross-bridges that would help sustain thin filament activation and cross-bridge binding in mid-to-late ejection, and/or (iii) decrease cooperative activation of the thin filament by less spread of thin filament activation yielding fewer cycling cross-bridges that generate force to work against the afterload thereby slowing loaded shortening in accordance with force-velocity relationship. In summary, as with diastole, there seems to be some biophysical benefits and detriments of more compliant titin on the dynamics of cardiac systole. Nevertheless, the biophysical findings from this study suggest that more N2BA titin yields reduced thin filament cooperative activation and deactivation, which may compromise systolic function due to fewer cross-bridges recruited to work against a given afterload and diastolic function due to reduced filling time, respectively. Overall, it seems that ventricular function and cardiac reserve are likely worsened if the N2BA:N2B titin ratio becomes too high. These ideas are consistent with the observation that RBM 20 deficient rats that contain N2BA titin develop dilated cardiomyopathy [42].

• We tested titin's role on myocyte force development, loaded shortening, and power output.

• N2BA titin yielded faster rates of force development, faster loaded shortening, and greater power.

• We suggest that titin modulates both cross-bridge flexibility and thin filament activation.