Abstract
Birds in the cormorant (Phalacrocoracidae) family dive tens of metres into water to prey on fish while entraining a thin layer of air (a plastron film) within the microstructures of their feathers. In addition, many species within the family spread their wings for long periods of time upon emerging from water. To investigate whether wetting and wing-spreading are related to feather structure, microscopy and photographic studies have previously been used to extract structural parameters for barbs and barbules. In this work, we describe a systematic methodology to characterize the quasi-hierarchical topography of bird feathers that is based on contact angle measurements using a set of polar and non-polar probing liquids. Contact angle measurements on dip-coated feathers of six aquatic bird species (including three from the Phalacrocoracidae family) are used to extract two distinguishing structural parameters, a dimensionless spacing ratio of the barbule (D*) and a characteristic length scale corresponding to the spacing of defect sites. The dimensionless spacing parameter can be used in conjunction with a model for the surface topography to enable us to predict a priori the apparent contact angles of water droplets on feathers as well as the water breakthrough pressure required for the disruption of the plastron on the feather barbules. The predicted values of breakthrough depths in water (1–4 m) are towards the lower end of typical diving depths for the aquatic bird species examined here, and therefore a representative feather is expected to be fully wetted in a typical deep dive. However, thermodynamic surface energy analysis based on a simple one-dimensional cylindrical model of the feathers using parameters extracted from the goniometric analysis reveals that for water droplets on feathers of all six species under consideration, the non-wetting ‘Cassie–Baxter’ composite state represents the global energy minimum of the system. By contrast, for other wetting liquids, such as alkanes and common oils, the global energy minimum corresponds to a fully wetted or Wenzel state. For diving birds, individual feathers therefore spontaneously dewet once the bird emerges out of water, and the ‘wing-spreading’ posture might assist in overcoming kinetic barriers associated with pinning of liquid droplets that retard the rate of drying of the wet plumage of diving birds.
Keywords: superhydrophobicity, wetting of bird feathers, oil repellency, binodal and spinodal
1. Introduction
The water-repellent nature of various bird feathers is typically attributed to a combination of a natural hydrophobic coating (preen oil) coupled with the microstructural topography of the feathers [1]. A droplet that is deposited on a water-repellent feather resides in a solid–liquid–air non-wetting composite state, in which tiny air pockets are trapped within the barbules of the feathers. These air pockets appear shiny when immersed under a liquid and are referred to as a plastron. While the term plastron was initially formulated by Brocher [2] to describe a general thin film of gas, it has primarily been studied in the context of aquatic insects where the utility of the plastron-like air layer in hiding from predators, for respiration, for mating or for providing a safe habitat for their young has been extensively investigated [3–9].
In aquatic birds, the existence of this plastron air layer inhibits the complete wetting of the feather and is thought to be critical in maintaining its water repellency. A plastron layer around the plumage also enhances insulation, ensures adequate thermoregulation, and can provide additional buoyancy to aquatic birds [10,11]. Birds in the cormorant family routinely dive in water up to many tens of metres for food and are known to subsequently dry their wings by spreading them in sunlight for extended periods of time. Noting this behaviour, researchers have attempted to correlate the diving and wing-spreading phenomena to the structure of bird feathers, with notable efforts for the cormorant and darter, but there is lack of a clear consensus [12–15]. In their seminal paper on the wettability of porous substrates, Cassie & Baxter [16] recognize the applicability of their idealized cylindrical model in describing the wetting on the barbules of bird feathers. Subsequently, Rijke [12] correlated the feather structure and wing-spreading phenomenon by documenting the wing-spreading behaviours of cormorants and studying feather barbs and barbules, which he characterized by applying the Cassie–Baxter (CB) model to feather texture.
Bird feathers have cylindrically shaped barbs and barbules that emerge from the main shaft (rachis) of the feather. Rijke used optical microscopy and photography to measure barb spacing, 2D, and diameter, 2R, from which he calculated a spacing ratio D* = (R + D)/R for various bird species [12]. In previous literature, researchers have argued both in favour of, and against, a correlation between the spacing ratio D* for the feathers and diving, swimming and wing-spreading behaviour [17–20]. Recently, Bormashenko et al. [21] obtained an estimate of the water breakthrough pressure (Pb) on typical feathers as approximately 10 kPa (corresponding to a depth of approx. 1 m of water). Past studies have also relied on photographic and microscopic techniques in attempts to characterize feather structure and wettability [12,14,17,18,22,23].
In this work, we apply a technique that enables us to use a contact angle goniometer [24] as a quantitative structural probe by making contact angle measurements with a number of polar and non-polar liquids on feathers that have been dip-coated with a low surface energy coating used in our past work [25]. We focus primarily on wing feathers, as those are most relevant during diving and wing-spreading phenomena. From these contact angle data, we self-consistently estimate an effective spacing ratio and a characteristic length scale of the critical flaws or defects in these complex structures. This systematic approach also enables us to verify the consistency of the various models used to estimate breakthrough pressures and to rationalize the diving behaviour of different bird species. Finally, we use a thermodynamic analysis which shows that the CB non-wetting state on feathers immersed in water is the globally stable state and thus connects the measured values of the spacing ratio, the hydrophobicity of the waxy oil coating and the observed behaviour of deep (more than 10 m) diving birds.
2. Experimental procedure
2.1. Collection of bird feathers
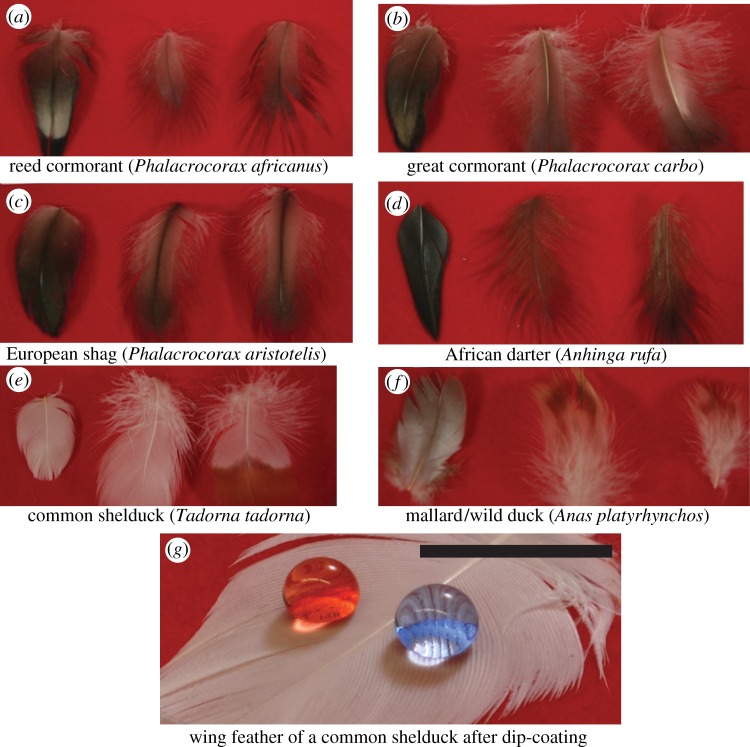
The wing feathers are obtained from six different species of aquatic birds, three from the same Phalacrocoracidae family: the reed cormorant, the great cormorant and the European shag. The remaining species each come from distinct families and were chosen because of their diving and wing-spreading behaviour and include: the African darter, the common shelduck and the mallard. Feather samples from 12 birds (two from each species) were furnished by the Natural History Museum, London, UK. No birds were sacrificed specifically for this study. After pruning, wing, breast and belly feathers of these birds were selected and representative samples are shown in figure 1a–f.
Figure 1.
Optical photographs of wing, breast and belly feathers for the six bird species. (a) Reed cormorant, (b) Great cormorant, (c) European shag, (d) African darter, (e) Common shelduck and (f) Mallard are shown. Feathers are typically 2–3 cm in length and wing feathers (leftmost among the three feathers) are typically more regular than breast and belly feathers (middle and right feather respectively). (g) A wing feather of a common shelduck after dip-coating in 50–50 fluorodecyl POSS/Tecnoflon solution is not wetted by water (γlv = 72.1 mN m−1, blue), or rapeseed oil (γlv = 35.5 mN m−1, red). The scale bar in the figure corresponds to 1 cm. (Online version in colour.)
2.2. Coating protocol
Fluorodecyl POSS (polyhedral oligomeric silsesquioxane) molecules consist of silsesquioxane cages surrounded by eight 1H,1H,2H,2H-heptadecafluorodecyl groups [26]. Owing to the high density of perfluorinated carbon atoms, a smooth fluorodecyl POSS surface has one of the lowest solid-surface energy values reported to date (γsv ≈ 10 mN m−1) [27]. The very low surface energy of POSS makes it an ideal material for conferring ultrathin, perfectly conformal coatings on topographically complex structures down to the submicrometre length scale [25]. Building on our earlier experience with woven fabrics and meshes, we were able to coat the feathers with thin, uniform, flexible and conformal layer of fluorodecyl POSS mixed with a commercially available fluoroelastomer (Tecnoflon BR 9151, Solvay Solexis, γsv ≈ 18 mN m−1). Asahiklin AK225 (Asahi Glass Company) was used as the common solvent for the fluorodecyl POSS and Tecnoflon. We provide further details of the coating procedure, sample preparation for microscopy and elemental fluorine maps verifying a conformal coating in the electronic supplementary material.
2.3. Contact angle measurements and tensiometry
Contact angle measurements and sliding angle measurements were obtained with a Ramé-Hart 590-F1 goniometer fitted with a tilt stage. Advancing and receding contact angles were measured using approximately 5 µl (approx. 3–3.5 mm diameter) droplets of various liquids (purchased from Aldrich and used as received). At least six contact angle measurements were performed with each liquid on each wing sample over the inner and outer vanes of the feathers. The liquid surface tension was measured using a Data Physics (DCAT 11) tensiometer with a platinum–iridium Wilhelmy plate at 20°C.
3. Results and discussion
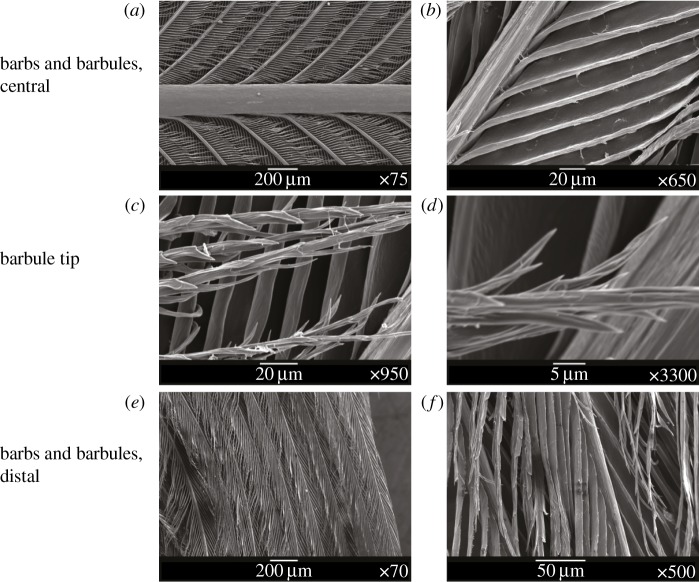
Feather structures are ‘quasi-hierarchical’ [21] and involve multiple distinct characteristic length scales, as shown in figure 2 for wing feathers of the common shelduck (Tadorna tadorna). The generic features of feathers consist of a main shaft (rachis), barbs (ramus) that branch out of the main shaft, and barbules that extend from the barbs and often form interlocking microstructures. The feathers are coated by preening oils secreted from the uropygial glands of aquatic birds. These oils are typically hydrophobic and consist of a mixture of waxes, esters and fatty acids that determine the local surface energy of the bird feathers [1,28–31]. On a smooth, chemically homogeneous surface, a liquid droplet exhibits a contact angle at equilibrium (θE) given by Young's relation cos θE = (γsv − γsl)/γlv, where γ is the pairwise interfacial tension between the solid (s), liquid (l) and vapour (v) phases, respectively [32]. However, the waxy secretions of the uropygial glands can result in a heterogeneous coating [33] on the feather resulting in an experimental advancing contact angle (θadv) that is typically used in lieu of the Young's equilibrium contact angle (θE). Rijke [12] reports an advancing water contact angle (θadv) on a flat surface comprising this waxy coating of approximately 90°. Elowson [17] measured values of θadv on the central primary rachises of the feathers of the African darter as θadv = 95° and mallard as θadv = 88°, again indicating the intrinsic hydrophobicity of the waxy coating on aquatic birds.
Figure 2.
Scanning electron micrographs of the topography of a wing feather of a common shelduck are shown. Pairs of images at different magnifications for the central, tip and distal parts of the feather indicate the complexity and hierarchical nature of its texture.
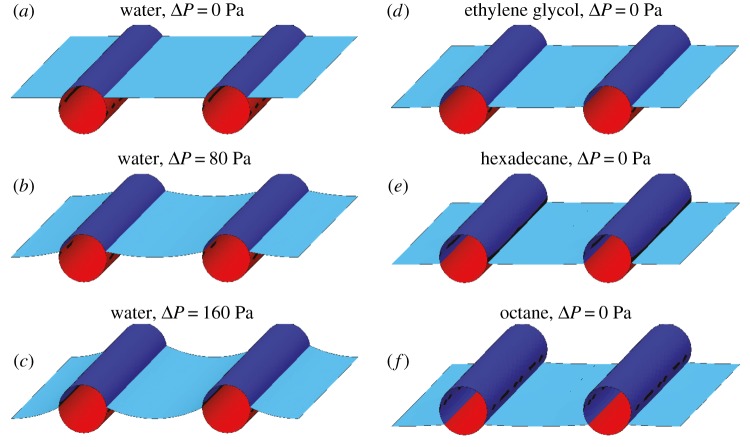
On rough surfaces, liquid droplets can exhibit one of the following two states—(i) either a composite CB state where the droplets partially rest on the solid elements and partially on the trapped air pockets between the asperities or (ii) a fully wetted (Wenzel) state where the droplets wet and penetrate the topography of the surface [16,34]. The topographical details along with the surface chemistry of the feathers are critical in determining whether a bird will maintain the non-wetting CB state as it dives into water [12,14,35]. A wing feather of a common shelduck is moderately hydrophobic in the uncoated state and water droplets (γlv = 72.1 mN m−1) have macroscopic advancing contact angles of 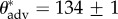 ° (see figure 1g and the electronic supplementary material, figure E1). When a hydrostatic pressure differential is applied on the air–water interface (illustrated in figure 3a–c), the contact line advances along the solid cylindrical textural elements [37]. However, when probed with lower surface tension liquids such as hexadecane or dodecane, the shelduck wing feather in the uncoated state is instantaneously wetted. We can confer enhanced repellency on feathers to these low surface tension liquids by dip-coating the feather with the 50 : 50 mixture of fluorodecyl-POSS/Tecnoflon. This now enables us to perform contact angle measurements using liquids with decreasing surface tensions and forms the basis for an alternative probing technique to quantify the texture and wettability of feathers. If the surface tension (γlv) of the contacting liquid is lowered (as shown in figure 3d–f) at a fixed pressure differential, the fraction of wetted solid once again increases. The close correspondence between these two sets of images suggests that probing a feather with water at increasing pressures or immersion depths is analogous to probing the same feather with liquids of successively lower surface tension. We therefore present an approach to quantitatively characterize bird feathers and other textured surfaces by first modifying the surfaces with an ultrathin conformal low energy coating that amplifies the natural liquid repellency and then performing contact angle measurements using a set of probing liquids. By dip-coating the feathers in our low surface energy coating (based on fluorodecyl-POSS/Tecnoflon), we eliminate complications arising from local variations in preen oil as well as sample-to-sample variations. We can then exclusively focus on the structural length scales that characterize the quasi-hierarchical feather structure. These parameters completely characterize the important wetting aspects of the feather texture. Finally, we seek to elucidate correlations between the details of feather texture and the behavioural response of these birds.
° (see figure 1g and the electronic supplementary material, figure E1). When a hydrostatic pressure differential is applied on the air–water interface (illustrated in figure 3a–c), the contact line advances along the solid cylindrical textural elements [37]. However, when probed with lower surface tension liquids such as hexadecane or dodecane, the shelduck wing feather in the uncoated state is instantaneously wetted. We can confer enhanced repellency on feathers to these low surface tension liquids by dip-coating the feather with the 50 : 50 mixture of fluorodecyl-POSS/Tecnoflon. This now enables us to perform contact angle measurements using liquids with decreasing surface tensions and forms the basis for an alternative probing technique to quantify the texture and wettability of feathers. If the surface tension (γlv) of the contacting liquid is lowered (as shown in figure 3d–f) at a fixed pressure differential, the fraction of wetted solid once again increases. The close correspondence between these two sets of images suggests that probing a feather with water at increasing pressures or immersion depths is analogous to probing the same feather with liquids of successively lower surface tension. We therefore present an approach to quantitatively characterize bird feathers and other textured surfaces by first modifying the surfaces with an ultrathin conformal low energy coating that amplifies the natural liquid repellency and then performing contact angle measurements using a set of probing liquids. By dip-coating the feathers in our low surface energy coating (based on fluorodecyl-POSS/Tecnoflon), we eliminate complications arising from local variations in preen oil as well as sample-to-sample variations. We can then exclusively focus on the structural length scales that characterize the quasi-hierarchical feather structure. These parameters completely characterize the important wetting aspects of the feather texture. Finally, we seek to elucidate correlations between the details of feather texture and the behavioural response of these birds.
Figure 3.
A surface evolver simulation of the wetting phenomena on a one-dimensional model a bird feather by different liquids [36]. As the pressure differential across the air–water interface increases from (a) zero, (b) 80 Pa and (c) 160 Pa, higher and higher fractions of the solid texture is wetted by water. The response of the same feather in contact with (d) ethylene glycol (γlv = 44 mN m−1, θadv = 100°), (e) hexadecane (γlv = 27.5 mN m−1, θadv = 80°) and (f) octane droplet (γlv = 21.6 mN m−1, θadv = 60°) with negligibly small pressure differential is depicted. (Online version in colour.)
The quasi-hierarchical structure of the feather [21] implies that there are multiple length scales accessible to the liquid drop as it probes the topography of the feather. Because the non-wetting CB state for low surface tension liquids on the dip-coated feathers is metastable, a single defect site on the feather can act locally as a nucleation centre that induces a local transition to the Wenzel state, which then propagates to the rest of the feather. This nucleation and subsequent propagation of the metastable CB to Wenzel transition on the dip-coated feathers is specific to low surface tension liquids (with values of θE < 90°). A similar wetting transition at the defect sites is not observed for water (θE > 90°), and the transition to the Wenzel state is instead determined by the smallest length scale (i.e. barbules) of the structure as we show in §3.3.
As we describe below, the goniometric measurements of 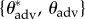 (provided in the electronic supplementary material, table E2) allow us to
(provided in the electronic supplementary material, table E2) allow us to
(1) provide a wetting-based quantification of D*, the effective cylinder spacing ratio of the feathers that was investigated by Rijke [12];
(2) predict the hydrostatic breakthrough pressure for each feather structure;
(3) estimate ℓdefect, the characteristic length-scale of defects that governs the Wenzel transition for droplets of low surface tension liquids.
Within this global framework, the contact angle data characterize the average topographical details, and allow us to use wetting as a ‘goniometric microscope’ that augments existing microscopic studies.
3.1. Characterization of feathers using advancing contact angles and a dimensionless spacing ratio
In the electronic supplementary material, table E2, we provide the apparent advancing (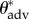 ) and receding (
) and receding (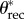 ) contact angles of liquid droplets on the dip-coated wing feathers of each of the six bird species. The advancing and receding contact angles (θadv and θrec) on a perfectly flat, fluorodecyl-POSS-coated surface are also provided for comparison. The apparent contact angles in the composite CB state decrease with decreasing surface tension (γlv) and below a threshold value, the droplets irreversibly transition from a composite non-wetting state and spread rapidly across the structure to establish a wetted state with a much lower apparent contact angle. For example, a liquid drop of hexadecane (γlv = 27.5 mN m−1) exhibits an apparent contact angle of
) contact angles of liquid droplets on the dip-coated wing feathers of each of the six bird species. The advancing and receding contact angles (θadv and θrec) on a perfectly flat, fluorodecyl-POSS-coated surface are also provided for comparison. The apparent contact angles in the composite CB state decrease with decreasing surface tension (γlv) and below a threshold value, the droplets irreversibly transition from a composite non-wetting state and spread rapidly across the structure to establish a wetted state with a much lower apparent contact angle. For example, a liquid drop of hexadecane (γlv = 27.5 mN m−1) exhibits an apparent contact angle of 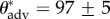 ° on a dip-coated feather of the African darter. However, a drop of dodecane (γlv = 25.3 mN m−1) placed on the same dip-coated feather immediately transitions to the fully wetted state and spreads across the feather resulting in an apparent contact angle of
° on a dip-coated feather of the African darter. However, a drop of dodecane (γlv = 25.3 mN m−1) placed on the same dip-coated feather immediately transitions to the fully wetted state and spreads across the feather resulting in an apparent contact angle of 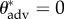 ° .
° .
We use a one-dimensional cylindrical framework to model the wettability of the feathers. The apparent macroscopic contact angle is related to the topography of the feathers and the coating chemistry by the CB relation, which can be expressed for this model as [17]
| 3.1 |
Here, 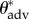 represents the measured macroscopic apparent contact angle, θadv is the measured advancing value of the contact angle on a flat, fluorodecyl-POSS-coated surface and D* = (R + D)/R is a dimensionless geometrical parameter defined in terms of the cylinder radius (R) and half-spacing (D). Using the CB relation for a cylindrical texture (equation (3.1)), a nonlinear regression was performed with D* as the only regression parameter (cf. the solid curves in electronic supplementary material, figure E2). A 95% confidence interval is used as a metric of uncertainty for the value of the spacing ratio D* (and values are listed in table 1). The spacing ratio characterizing the wing feather is denoted
represents the measured macroscopic apparent contact angle, θadv is the measured advancing value of the contact angle on a flat, fluorodecyl-POSS-coated surface and D* = (R + D)/R is a dimensionless geometrical parameter defined in terms of the cylinder radius (R) and half-spacing (D). Using the CB relation for a cylindrical texture (equation (3.1)), a nonlinear regression was performed with D* as the only regression parameter (cf. the solid curves in electronic supplementary material, figure E2). A 95% confidence interval is used as a metric of uncertainty for the value of the spacing ratio D* (and values are listed in table 1). The spacing ratio characterizing the wing feather is denoted 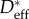 and was found to be significantly different for the various birds, ranging from a value as low as
and was found to be significantly different for the various birds, ranging from a value as low as 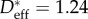 for the reed cormorant to as high as
for the reed cormorant to as high as 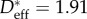 for the European shag (table 1).
for the European shag (table 1).
Table 1.
Effective values of the spacing ratio (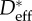 ) measured on wing feathers from this work are compared against values obtained from microscopy (
) measured on wing feathers from this work are compared against values obtained from microscopy (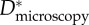 ) of barbs and barbules from the literature for wing feathers and breast feathers. In the last column, an estimate of the half-spacing of defect sites (ℓdefect) deduced from contact angle measurements is also reported, and its significance is detailed in §3.5 in the main text.
) of barbs and barbules from the literature for wing feathers and breast feathers. In the last column, an estimate of the half-spacing of defect sites (ℓdefect) deduced from contact angle measurements is also reported, and its significance is detailed in §3.5 in the main text.
| bird species |
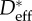 wing feathers wing feathers |
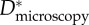 wing feathers wing feathers |
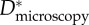 breast feathers breast feathers |
ℓdefect (µm) | ||
|---|---|---|---|---|---|---|
| barbs | barbules | barbs | barbules | |||
| African darter | 1.28 ± 0.10 | 1.7a | 1.6a | 4.5b, 9.9a | 7.6a | 220 ± 50 |
| reed cormorant | 1.24 ± 0.14 | 4.2a | 2.4a | 4.3b, 8.5a | 2.9a | 190 ± 70 |
| great cormorant | 1.57 ± 0.15 | — | 4.8b | — | 290 ± 30 | |
| mallard | 1.84 ± 0.13 | 5.7a | 1.9a | 5.9b, 10.6a | 2.9a | 250 ± 30 |
| European shag | 1.91 ± 0.16 | — | — | — | — | 350 ± 30 |
| common shelduck | 1.89 ± 0.12 | 6.7a | 2.1a | 11.1a | 3.5a | 280 ± 30 |
Estimating an appropriate value of this geometric spacing ratio (D*) using micrographs (such as those in figure 2) is difficult owing to the complexity of the feather structures. As a result, there are conflicting reports in the literature of the values of D* obtained from microscopy on barbs and barbules (henceforth denoted 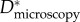 ). In table 1, we list the effective values of the spacing ratio we obtain from fitting to contact angle data alongside values of
). In table 1, we list the effective values of the spacing ratio we obtain from fitting to contact angle data alongside values of 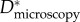 from the early work of Rijke [12] on breast feathers and subsequent work of Elowson [17] on breast and wing feathers. A comparison between the two columns indicates that our small values of
from the early work of Rijke [12] on breast feathers and subsequent work of Elowson [17] on breast and wing feathers. A comparison between the two columns indicates that our small values of 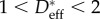 are consistent with Elowson's microscopy-based estimates at the barbule scale of wing feathers. In the following sections, we demonstrate that the values of D* obtained from the wetting of the barbules are consistent with the overall observed wetting, de-wetting and breakthrough pressure (Pb) of typical wing feathers whereas the larger literature values of
are consistent with Elowson's microscopy-based estimates at the barbule scale of wing feathers. In the following sections, we demonstrate that the values of D* obtained from the wetting of the barbules are consistent with the overall observed wetting, de-wetting and breakthrough pressure (Pb) of typical wing feathers whereas the larger literature values of 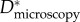 (corresponding to barbs) lead to erroneous predictions.
(corresponding to barbs) lead to erroneous predictions.
3.2. Predicting breakthrough pressures of water interface on feathers
The preceding structural analysis used the CB relation (equation (3.1)) to obtain a goniometric measure of the dimensionless spacing ratio (D*) on the feathers, which we can then use, in turn, to predict the breakthrough pressure of water for each bird species. As the bird dives underwater to a depth H, a hydrostatic pressure differential ΔP = ρgH builds up across the composite air–liquid interface. As a result, the interface must become curved to sustain this pressure differential. In figure 4, we show an illustration of the liquid meniscus between parallel cylinders of radius R and half-spacing D under the influence of a dimensionless pressure differential 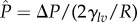 . The position of the contact line is characterized by the angle ϑ subtended between the contact line and the vertical line through the centre of the cylinder. In the absence of an external pressure differential and negligible gravitational effects (ΔP = 0), the liquid/air meniscus is flat, and is located at ϑ = θE. For ΔP > 0, the position of the contact line on the cylinder is determined by a balance of the capillary force and the pressure force as detailed in the electronic supplementary material. Beyond a certain threshold, a transition to the fully wetted (Wenzel) state can occur by either a depinning, sagging or coalescence mechanism [38]. Determining which of the various modes of failure occurs first involves knowledge of the geometry as well as making specific assumptions about the model that represents the barbs and barbules of the feathers. Bormashenko et al. [21] developed an analytical expression for the pressure differential at which a depinning transition occurs on a single layer of freely suspended parallel cylinders. As we show explicitly in the electronic supplementary material, this depinning transition requires a barbule spacing D* < |tanθE|. Therefore, if the barbules are spaced widely apart, this depinning transition does not occur. In the absence of this transition, Bormashenko assumes the liquid meniscus transitions to the fully wetted state only when it coalesces around the cylinder. However, the dense underlying structure on a feather (as seen in figure 2b) can also permit a sufficiently curved liquid meniscus to interact with additional underlying layers of solid features leading to a Cassie to Wenzel transition. We can describe this possibility by also allowing for a ‘sagging’ transition (in the place of Bormashenko's coalescence transition) resulting from meniscus contact with an underlying planar solid substrate (i.e. h → 0 in figure 4). This allows us to combine Bormashenko's results on the depinning transition with independent calculations of the sagging transition to obtain a single framework for determining the breakthrough pressure Pb according to electronic supplementary material, equation SI-8. We also provide a state space plot (electronic supplementary material, figure F3) to indicate the actual mode of transition for various pairs of D*, θE.
. The position of the contact line is characterized by the angle ϑ subtended between the contact line and the vertical line through the centre of the cylinder. In the absence of an external pressure differential and negligible gravitational effects (ΔP = 0), the liquid/air meniscus is flat, and is located at ϑ = θE. For ΔP > 0, the position of the contact line on the cylinder is determined by a balance of the capillary force and the pressure force as detailed in the electronic supplementary material. Beyond a certain threshold, a transition to the fully wetted (Wenzel) state can occur by either a depinning, sagging or coalescence mechanism [38]. Determining which of the various modes of failure occurs first involves knowledge of the geometry as well as making specific assumptions about the model that represents the barbs and barbules of the feathers. Bormashenko et al. [21] developed an analytical expression for the pressure differential at which a depinning transition occurs on a single layer of freely suspended parallel cylinders. As we show explicitly in the electronic supplementary material, this depinning transition requires a barbule spacing D* < |tanθE|. Therefore, if the barbules are spaced widely apart, this depinning transition does not occur. In the absence of this transition, Bormashenko assumes the liquid meniscus transitions to the fully wetted state only when it coalesces around the cylinder. However, the dense underlying structure on a feather (as seen in figure 2b) can also permit a sufficiently curved liquid meniscus to interact with additional underlying layers of solid features leading to a Cassie to Wenzel transition. We can describe this possibility by also allowing for a ‘sagging’ transition (in the place of Bormashenko's coalescence transition) resulting from meniscus contact with an underlying planar solid substrate (i.e. h → 0 in figure 4). This allows us to combine Bormashenko's results on the depinning transition with independent calculations of the sagging transition to obtain a single framework for determining the breakthrough pressure Pb according to electronic supplementary material, equation SI-8. We also provide a state space plot (electronic supplementary material, figure F3) to indicate the actual mode of transition for various pairs of D*, θE.
Figure 4.
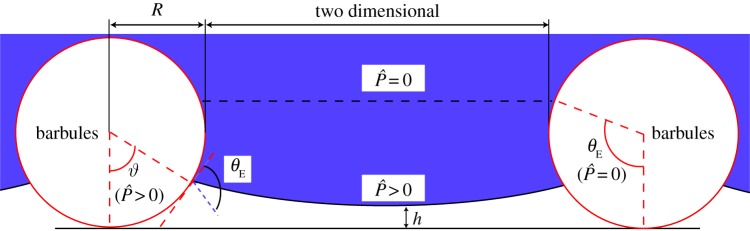
Schematic of the Cassie–Baxter composite interface resting on an array of parallel barbules of radius R and half-spacing D under a dimensionless external pressure differential 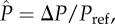 where Pref = 2γlv/R. Here, θE is the equilibrium contact angle of the liquid, ϑ is the angular coordinate characterizing the location of the contact line at a given pressure and h is the altitude of the bottom of the curved meniscus above the substrate. The horizontal dashed line indicates the location of the meniscus in the absence of any external pressure differential. (Online version in colour.)
where Pref = 2γlv/R. Here, θE is the equilibrium contact angle of the liquid, ϑ is the angular coordinate characterizing the location of the contact line at a given pressure and h is the altitude of the bottom of the curved meniscus above the substrate. The horizontal dashed line indicates the location of the meniscus in the absence of any external pressure differential. (Online version in colour.)
As expected (cf. electronic supplementary material, equation SI-8), the critical pressure for breakthrough Pb is inversely proportional to the length scale of the texture (R), and therefore the selection of R is critical in the design of robust non-wetting textures. In a typical bird feather, there are many different length scales corresponding to the barb, barbules and tiny offshoots from the barbules. Because barbules occupy most of the area fraction of the feather, the length scale of these barbules is expected to be the dominant length scale. Motivated by the scanning electron micrographs in figure 2a–f, and the data of Elowson [17], we select a typical value of the barbule length scale of R ∼ 5 μm. We also use the advancing contact angle (θadv) in lieu of the equilibrium contact angle (θE) to account for inhomogeneities on the surface of the feather [39]. With this value of the characteristic feature size along with knowledge of 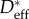 and θadv for the feathers of the various bird species, we can predict the breakthrough pressure of water by using the electronic supplementary material, equation SI-8.
and θadv for the feathers of the various bird species, we can predict the breakthrough pressure of water by using the electronic supplementary material, equation SI-8.
To obtain the unknown value of θadv for each feather, we make use of the fact that although our FluoroPOSS coating significantly modifies the intrinsic surface chemistry of the feathers, the thin (less than 200 nm) and conformal nature of the coating ensures that the values of the effective spacing ratio (D*) remain the same as the uncoated feather. In addition, apparent advancing contact angles for water droplets (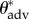 ) on the uncoated feathers can be measured. Using the CB relation (equation (3.1)), and the values of
) on the uncoated feathers can be measured. Using the CB relation (equation (3.1)), and the values of 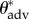 and
and 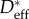 from table 1 and electronic supplementary material, table E2, an estimate of values of θadv for water droplets on the inherent waxy coating of the feathers can be calculated (presented in the second column of table 2). These values of θadv characterize the wettability of the heterogeneous waxy secretions (preen oil) from the uropygial gland that is deposited onto the feathers. We use these advancing contact angles in place of the equilibrium contact angles for each feather to determine the value of Pb and these values are listed in table 2.
from table 1 and electronic supplementary material, table E2, an estimate of values of θadv for water droplets on the inherent waxy coating of the feathers can be calculated (presented in the second column of table 2). These values of θadv characterize the wettability of the heterogeneous waxy secretions (preen oil) from the uropygial gland that is deposited onto the feathers. We use these advancing contact angles in place of the equilibrium contact angles for each feather to determine the value of Pb and these values are listed in table 2.
Table 2.
Estimated values of advancing contact angles (θadv) for water on the waxy preen oil coating that is present on a feather, critical angular location (ϑc), predicted breakthrough pressure for water droplets (Pb) and the corresponding transition mode are compiled for the uncoated wing feathers of the six bird species. The last two columns contain empirically observed diving depth and wing-spreading behaviour for the same bird species.
| bird species | estimated CA θadv (°) | critical angular location ϑc (°) | predicted breakthrough pressure, Pb (kPa) | predicted transition mode | diving depth (m) | wing-spreading |
|---|---|---|---|---|---|---|
| African darter | 112 ± 2 | 68 | 28 | depinning | <5 | Y |
| reed cormorant | 118 ± 2 | 73 | 40 | depinning | 5–6a | Y |
| great cormorant | 117 ± 6 | 62 | 19 | depinning | 4.7, <10b | Y |
| mallard | 105 ± 3 | 57 | 13 | sagging | dabbling | N |
| European shag | 106 ± 3 | 58 | 12 | sagging | 33–35 | Y |
| common shelduck | 116 ± 2 | 61 | 13 | sagging | dabbling | N |
aFrom neutral buoyancy experiments, not natural observation.
bUsually < 10 m, but can dive to depths of 35 m [40]; wing-spreading: Y, predictably; N, never; dabbling, dabbling species, not primarily divers.
We observe in table 2 that the predicted Pb for the Cassie to Wenzel transition on the feathers of the various species is in the range of 10–40 kPa for all six species, which corresponds to a maximum diving depth in water of 1 ≤ H ≤ 4 m, consistent with the independent estimate obtained by Bormashenko et al. [21]. Therefore, from our calculations of Pb, the diving depths at which the non-wetting Cassie state can be maintained are towards the lower end of the spectrum of the typical diving depths reported for the African darter and reed cormorant. In addition, for the great cormorant and the European shag, which reach depths of up to 35 m while hunting for fish, our calculations predict that the individual bird feathers will indeed become fully wetted during a typical dive. Using our analysis, the barbules would need to be as small as R ∼ 0.3 µm to achieve diving depths of 35 m while maintaining the non-wetting state, which is inconsistent with the observed structural length scales shown in the scanning electron micrographs in figure 2. This suggests that evaluating the resistance to meniscus breakthrough is not sufficient by itself to completely explain the diving and wing-spreading behaviour of deep-diving aquatic birds.
In the SEM image shown in the electronic supplementary material, figure C2, we can identify the presence of large defect sites in the feather structure. We can quantify these defect sites by introducing a local effective half-spacing (ℓdefect) on the quasi-hierarchical feather. These defect sites correspond to the initial nucleation site at which low surface tension liquid droplets initiate transition to the fully wetted Wenzel state. By equating the Laplace pressure of the probe liquid droplet with the breakthrough pressure Pb (cf. Section G in the electronic supplementary material) an expression for the defect size (ℓdefect) can be obtained
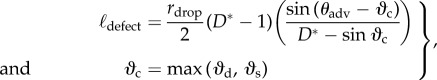 |
3.2 |
where rdrop is the radius of the probe liquid drop and ϑc is the critical angular location at which the Cassie to Wenzel transition occurs either by depinning (ϑd) or sagging (ϑs), whichever occurs first. For the wing feather of a common shelduck, we find the defect length scale, ℓdefect = 280 µm. The same exercise is repeated for the wing feathers of other five bird species, and the results are summarized in the last column of table 1. All six bird species have similar values of the half-spacing of defect sites (ℓdefect ∼ 200–300 µm). These values of the defect length scale are much larger than the barb or barbules length scale (i.e. 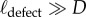 ), highlighting the quasi-hierarchical structure of the feather. Our goniometric measurements thus suggest that the scale of the defects controlling the Cassie to Wenzel transition for low surface tension metastable liquids is set by topographic features that are an order of magnitude larger than those typically considered in optical microscopy of the feather.
), highlighting the quasi-hierarchical structure of the feather. Our goniometric measurements thus suggest that the scale of the defects controlling the Cassie to Wenzel transition for low surface tension metastable liquids is set by topographic features that are an order of magnitude larger than those typically considered in optical microscopy of the feather.
In summary, the overall wetting behaviour of the wing feathers of each of the six bird species has been characterized in terms of two structural parameters—(i) an effective spacing ratio (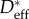 ) that governs the value of Pb required to disrupt the non-wetting water/air interface on the smallest features on the feathers (i.e. the barbules with R ∼ 5 µm) and (ii) a larger defect length scale ℓdefect that governs the breakthrough of the metastable composite interface formed with low surface tension liquids. The difference between the characteristic length scales that govern the breakthrough of the composite interface with water, as opposed to low surface tension liquids, arises from the global thermodynamic stability of the non-wetting CB state formed with water when the equilibrium contact angle is above a certain critical contact angle, as we now proceed to show.
) that governs the value of Pb required to disrupt the non-wetting water/air interface on the smallest features on the feathers (i.e. the barbules with R ∼ 5 µm) and (ii) a larger defect length scale ℓdefect that governs the breakthrough of the metastable composite interface formed with low surface tension liquids. The difference between the characteristic length scales that govern the breakthrough of the composite interface with water, as opposed to low surface tension liquids, arises from the global thermodynamic stability of the non-wetting CB state formed with water when the equilibrium contact angle is above a certain critical contact angle, as we now proceed to show.
3.3. Surface energies of Cassie–Baxter and Wenzel state interfaces
An expression for the surface energy of the composite CB interface relative to an initial reference state can be obtained for the array of parallel cylinders. The reference state is the initial planar interface that exists in the absence of a pressure differential (i.e. 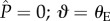 ). The total surface energy is the sum of two terms (i) the evolving solid/air and solid/liquid interactions that accrue as the contact line descends along the barbule (governed by the Young–Dupré equation), and (ii) the incremental energy of the increasing liquid/air interface resulting from the curvature of the meniscus. In the electronic supplementary material, we derive the following expressions for the surface energies for the CB state (ΔEC) and the Wenzel state (ΔEw) relative to the initial reference state:
). The total surface energy is the sum of two terms (i) the evolving solid/air and solid/liquid interactions that accrue as the contact line descends along the barbule (governed by the Young–Dupré equation), and (ii) the incremental energy of the increasing liquid/air interface resulting from the curvature of the meniscus. In the electronic supplementary material, we derive the following expressions for the surface energies for the CB state (ΔEC) and the Wenzel state (ΔEw) relative to the initial reference state:
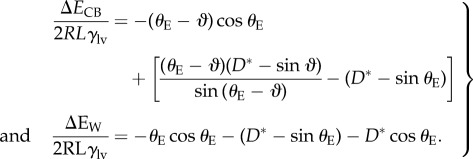 |
3.3 |
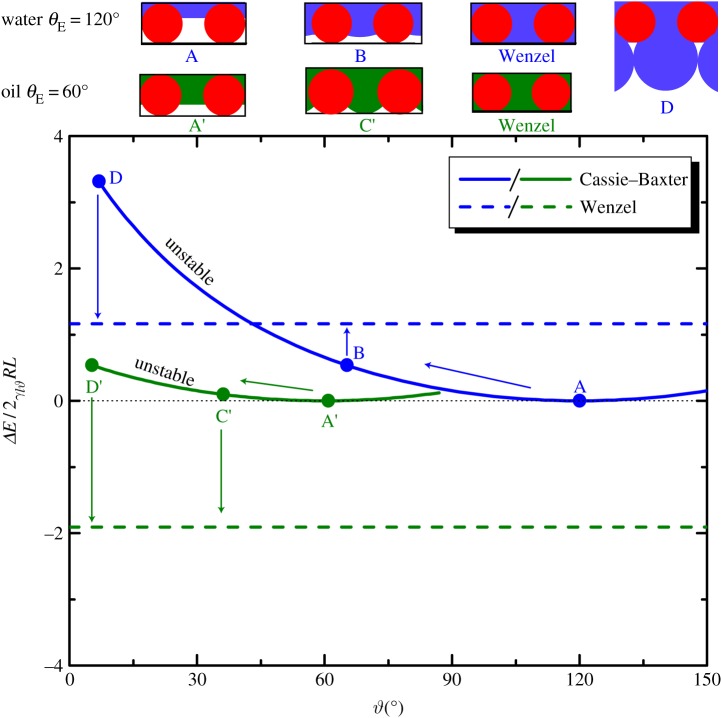
In each case, the surface energy is scaled by a reference energy 2RLγlv, where L is the length of the cylindrical element. The final term in the expression for the energy of the Wenzel state is introduced to account for the interactions on the underlying flat substrate. In figure 5, we show the free energy of the interface on an array of cylindrical barbules as it evolves with increasing applied pressure differential for a fixed value of D* = 1.5, and for two values of equilibrium contact angles of θE = 120° and θE = 60° (representative of the behaviour of water and oils, respectively). The solid line corresponds to the surface energy of the CB state (ΔECB), and the dashed line is the energy of the fully wetted Wenzel state (ΔEw), determined from equation (3.3). For both curves, the initial non-wetting reference state A (i.e. 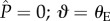 ) is located at a local minimum of the surface energy. The surface energy of the composite interface increases with increasing external pressure differential as the meniscus descends along the cylindrical features. When θE = 120° and D* = 1.5 (blue curve), the liquid meniscus transitions to the Wenzel state by a depinning mechanism, indicated by state B (
) is located at a local minimum of the surface energy. The surface energy of the composite interface increases with increasing external pressure differential as the meniscus descends along the cylindrical features. When θE = 120° and D* = 1.5 (blue curve), the liquid meniscus transitions to the Wenzel state by a depinning mechanism, indicated by state B (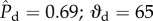 °) in figure 5. Beyond this point
°) in figure 5. Beyond this point 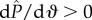 and the interface is mechanically unstable to pressure fluctuations. The dimensionless surface energy just before this transition occurs is ΔEc/(2γlvRL) = 0.54. The numerical value of the surface energy at the transition state can be interpreted as an energy barrier that must be overcome before transition. Further, we observe that when θE = 120° and D* = 1.5, the final fully wetted Wenzel state (dashed line) is at a higher energy (ΔEw/(2γlvRL) = 1.16) than the transition state B and the initial CB state. This indicates that the globally stable equilibrium state is the CB non-wetting state; therefore, upon decreasing the external pressure differential, the Wenzel state will spontaneously dewet and re-establish the composite non-wetting CB interface.
and the interface is mechanically unstable to pressure fluctuations. The dimensionless surface energy just before this transition occurs is ΔEc/(2γlvRL) = 0.54. The numerical value of the surface energy at the transition state can be interpreted as an energy barrier that must be overcome before transition. Further, we observe that when θE = 120° and D* = 1.5, the final fully wetted Wenzel state (dashed line) is at a higher energy (ΔEw/(2γlvRL) = 1.16) than the transition state B and the initial CB state. This indicates that the globally stable equilibrium state is the CB non-wetting state; therefore, upon decreasing the external pressure differential, the Wenzel state will spontaneously dewet and re-establish the composite non-wetting CB interface.
Figure 5.
Plot of the total surface energy of the composite solid/liquid system against the angular position ϑ of the interface on a one-dimensional array of parallel cylinders of diameter 2R, half-spacing D with a dimensionless geometric spacing D* = (R + D)/R = 1.5. The solid curves indicate the energies of the composite interface corresponding to the Cassie–Baxter non-wetting state, and the dashed line indicates the surface energy of the fully wetted Wenzel state. The blue and green curves are evaluated for water (θE = 120°) and a typical oil (θE = 60°) respectively. A schematic of the liquid meniscus corresponding to the initial state A, depinning transition state B, sagging transition state C and the Wenzel state are shown above the plots. State D is associated with the coalescence transition proposed by Bormashenko, when a rigid, underlying substrate below the feather is absent, as shown in the sketch. (Online version in colour.)
By contrast, the wetting behaviour of an oil (green curve; θE = 60°) on the cylindrical features with the same structural spacing ratio (D* = 1.5) is markedly different. The surface energy once again increases from the initial reference state A′. However, in this case, the meniscus transitions to the Wenzel state by a sagging mechanism, indicated by state C′ 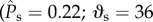 °). The dimensionless surface energy barrier just prior to transition is only ΔEc/(2γlvRL) = 0.09, much smaller than the corresponding transition value obtained for water. The most characteristic feature of the wetting of the oil is the much lower energy of the Wenzel state (ΔEw/(2γlvRL) = −1.9) relative to both the transition state C′ and the initial state A′. The negative value of the surface energy for the Wenzel state indicates that the wetting transition is irreversible for this oil, and the liquid will not recover to the initial non-wetting state even upon complete removal of the external pressure difference.
°). The dimensionless surface energy barrier just prior to transition is only ΔEc/(2γlvRL) = 0.09, much smaller than the corresponding transition value obtained for water. The most characteristic feature of the wetting of the oil is the much lower energy of the Wenzel state (ΔEw/(2γlvRL) = −1.9) relative to both the transition state C′ and the initial state A′. The negative value of the surface energy for the Wenzel state indicates that the wetting transition is irreversible for this oil, and the liquid will not recover to the initial non-wetting state even upon complete removal of the external pressure difference.
The irreversibility of the wetting transition highlights the metastable nature of the non-wetting state for oils [41,42], and an estimate of the magnitude of the energy barrier prior to transition can be obtained from equation (3.3). So far, we have considered the wetting energies of two liquids with different values of θE on the same structural element. A similar irreversible wetting transition can occur for water by simply increasing the value of the dimensionless spacing (D*). An understanding of the energetics of wetting, and the global stability of the CB non-wetting state on the cylindrical elements of the feathers, is therefore essential in interpreting the diving and wing-spreading behaviour of birds.
3.4. Thermodynamics of wetting: binodal and spinodal
To test whether water droplets on a given bird feather are in a thermodynamically stable CB state, values of the contact angle on a smooth surface with identical chemistry (θE) are needed. By using the best-fit value of D* from our goniometric analysis on the dip-coated feather, and measuring the apparent advancing contact angle for water on each uncoated feather, we can estimate the advancing contact angles on the waxy preen oil that covers natural feathers using equation (3.1), as discussed in §3.2. We have listed these values in the second column of table 2.
For a feather with a hydrophilic coating (θE < 90°), the fully wetted state represents the global equilibrium state with minimum free energy, whereas on a feather with a strongly hydrophobic coating 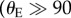 °), the composite solid–liquid–air interface is the global equilibrium state. The thermodynamic crossover condition between the two states is analogous to a binodal transition [43] that can be found by equating the two expressions (CB and Wenzel) for apparent contact angles to give
°), the composite solid–liquid–air interface is the global equilibrium state. The thermodynamic crossover condition between the two states is analogous to a binodal transition [43] that can be found by equating the two expressions (CB and Wenzel) for apparent contact angles to give
| 3.4 |
Equation (3.4) gives an implicit expression for the binodal line for the critical angle θc on a feather with a specific value of the spacing ratio D*. These critical values are evaluated for each of the six species studied in this paper in table 3 and equation (3.4) is shown as the broken line in figure 6.
Table 3.
Values of the critical angle for the Cassie–Baxter to Wenzel transition (θc) on wing feathers of various species obtained using effective values of the spacing ratio (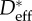 ) in equation (3.4).
) in equation (3.4).
| bird species | D* | θc (°) |
|---|---|---|
| African darter | 1.28 ± 0.10 | 96 ± 2 |
| reed cormorant | 1.24 ± 0.14 | 95 ± 3 |
| great cormorant | 1.57 ± 0.15 | 100 ± 2 |
| mallard | 1.84 ± 0.13 | 104 ± 2 |
| European shag | 1.91 ± 0.16 | 105 ± 2 |
| common shelduck | 1.89 ± 0.12 | 104 ± 1 |
Uncertainty in θadv is computed using Monte Carlo simulation with 10 000 repeats and by sampling values of D* and θ* as random normal distributions.
Figure 6.
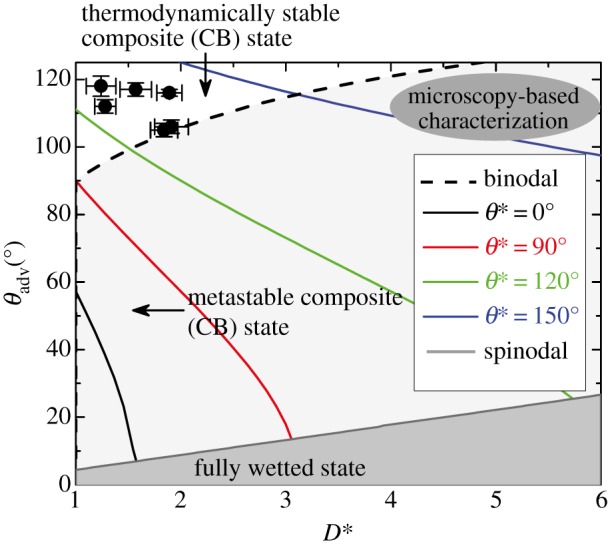
A phase diagram of surface wettability is plotted with measured advancing contact angles (θadv) for water on the ordinate and a best-fit value of the effective spacing ratio (D*) on the abscissa. Isocontours of constant apparent contact angle (evaluated using equation (3.1)) are shown by solid coloured lines. The dashed line corresponds to the binodal line (equation (3.4)) separating the thermodynamically stable Cassie–Baxter (CB) states and the metastable CB states (light grey). The measured water contact angle data for the six bird species under consideration all lie above the binodal where the composite interface is the equilibrium state. The metastable region of the chart below the binodal (light grey) is obtained by equating the Laplace pressure of a water drop with radius equal to its capillary length, to the Pb calculated from equation (SI-8) with R = 5 µm. The solid grey line indicates the spinodal. In the region below the spinodal, the CB state is unstable and will undergo a spontaneous transition to the fully wetted Wenzel state. (Online version in colour.)
The broken line demarcating the stable and metastable regions in figure 6 is analogous to a binodal curve and can also be obtained by setting ΔEw = 0 in equation (3.3), when the energy of the fully wetted Wenzel state is exactly equal to the lowest energy of the CB state (when the pressure difference 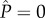 ).
).
For a given barbule spacing D*, the composite interface (or CB state) is globally the lowest energy state if θadv > θc; this corresponds to the region above the binodal in figure 6. When θadv < θc, the fully wetted Wenzel state has a lower free energy than the CB state. However, because of the energy barrier shown in figure 5, a liquid droplet on a feather can remain trapped in a metastable non-wetting CB state, in the region shaded light grey in figure 6 until a defect nucleates and grows leading to an irreversible wetting transition. Organic liquids with θE < 90° that produced non-zero contact angles on the coated feathers all lie in this shaded region between the binodal and spinodal in figure 6. Liquid droplets in metastable CB states eventually transition into the more stable Wenzel state under pressure perturbations. From the electronic supplementary material, equation SI-8, the breakthrough pressure required depends on the spacing ratio (D*) and the barbule length scale (R). The dark grey region in figure 6 below the spinodal curve indicates the region where the transition to the thermodynamically stable fully wetted Wenzel transition state is spontaneous, and the non-wetting CB state is thermodynamically unstable. A representative spinodal curve, evaluated with a barbule length scale of R = 5 µm is plotted as the dark grey line in figure 6, where the characteristic length scale that sets the magnitude of the Laplace pressure for a large drop sitting on a feather corresponds to the capillary length of water (rdrop = 1.7 mm).
On comparing the second column of table 2 with the last column of table 3, it is clear that for all six bird species, the value of the advancing contact angle (θadv ∼ 105–118°) that we compute for each feather (by using the best-fit value of D* obtained from our goniometric analysis) is larger than the corresponding critical contact angle θc (i.e. θadv > θc). Therefore, based on our goniometric analysis, we conclude that water droplets sitting on the quasi-hierarchical feathers of all six birds are in fact, in a thermodynamically stable CB state, as shown in figure 6. The wetting behaviour of the natural waxy coating (or preen oil) on aquatic bird feathers is, at best, moderately hydrophobic (θadv ∼ 105–118°), and therefore a low value of the effective spacing ratio between barbules (1 < D* < 2) is essential to push the feathers above the binodal (dashed line) in figure 6. Various microscopy-based estimations reported in the literature [12,14,17–19,22], particularly those based on barb dimensions, lead to much larger reported values of the spacing ratio (D* ≈ 3–10). This would erroneously locate the feathers in the top right corner of figure 6, in a region where the fully wetted Wenzel state is the global equilibrium state for water–feather interactions.
3.5. Connection between wetting, thermodynamics and avian diving
Based on our analysis, the predicted values of Pb for feathers immersed in water are toward the lower end of the typical hydrostatic pressure that the feathers are subjected to during a dive. With increasing hydrostatic pressure, the air between the barbs and barbules is gradually replaced by water as the air–water interface bulges (figure 3). Therefore, the individual feathers are expected to ultimately be fully wetted in a typical dive.
However, as the birds return to the surface, this Wenzel state becomes energetically unfavourable compared with the solid–liquid–air composite state. Drainage induced de-wetting of the texture is expected, but this energetically favourable transition behaviour may be retarded by kinetic barriers that trap some of the water temporarily in the feather texture. Spreading of the wings will help to facilitate the transition from the Wenzel to the CB state by reducing contact line pinning of droplets. The rate of transition from a fully wetted state to a composite state will depend on the geometry and wetting characteristics of the feather (e.g. the barb spacing and the advancing contact angle). However, for the feathers of all six aquatic birds under consideration here, our goniometric analysis suggests that the non-wetting state is indeed thermodynamically favourable. By contrast, Bormashenko [30] has determined the equilibrium contact angle of water on the central rachis of pigeon feathers to be θE = 72° (i.e. the pigeon feather is mildly hydrophilic). Therefore, our framework implies that although pigeon feathers repel water, the corresponding CB state with water is, at best, metastable. The enhanced hydrophobicity of the waxy coating and preening oil used by aquatic diving birds (θE > 90°), coupled with low values of D*, are essential for the feathers to spontaneously dewet after each dive.
4. Conclusion
In this work, we have extended and applied our understanding of the wetting of fibrous textured surfaces to bird feathers. From the thermodynamic analysis, we show that a combination of the structural details of the feathers (i.e. densely spaced barbules with low values of D*) along with the hydrophobic surface chemistry of preen oil (i.e. θE > 90°) together can help establish the CB composite state as the global equilibrium state when feathers are in contact with water. By contrast, for lower surface tension liquids or for widely spaced barbules, this CB state is, at best, metastable. The characteristic length scale of the barbules determined from microscopy (R ∼ 5 µm) sets the breakthrough pressure for the case of water, while the much larger defect length scale (ℓdefect) controls the loss of metastability for droplets of organic liquids placed on the same feather structures.
The estimated hydrostatic breakthrough pressures of water on the textures of all six wing feathers indicated that individual feathers are expected to become wet at moderate depths (on the order of a few metres) smaller than the typical diving depths attained by the African darter, reed cormorant and the European shag. Using our dip-coating approach, we are able to locate the various feather textures on a wetting state phase diagram and thereby show that a non-wetted solid–liquid–air composite interface is the global equilibrium state of water droplets on these feathers at atmospheric pressure. Once the birds emerge out of water, the dewetting transition is thermodynamically favourable. The wing-spreading behaviour demonstrated by these species might help facilitate this dewetting/water-shedding if there are pinning sites or other kinetic traps that delay the spontaneous drying of feathers and their return to the lowest energy CB state.
We have also demonstrated that feathers of aquatic bird species with a thin, conformal POSS/Tecnoflon coating are not wetted by low surface tension liquids like hexadecane and dodecane. Dip-coating thus enables us to focus exclusively on the role of physical structure or texture on the observed wetting behaviour. From goniometric measurements of the apparent advancing contact angle data (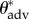 ) on the dip-coated feathers, we extracted a dimensionless spacing ratio (1 < D* < 2) and a measure of the half-spacing of defect sites (190 µm < ℓdefect < 350 µm) that characterize the quasi-hierarchical structure of the wing feathers of each bird species. These two structural parameters capture the most important structural aspects of the complicated texture of bird feathers in terms of a simple one-dimensional wetting cylindrical model. This approach provides a simple and quantitative method to investigate bird feathers, compared with previous work based on microscopic or photographic evaluation alone. Applying this systematic and self-consistent framework to measurements on feathers of other avian species will help to correlate the structure of bird feathers with observed aquatic behaviour.
) on the dip-coated feathers, we extracted a dimensionless spacing ratio (1 < D* < 2) and a measure of the half-spacing of defect sites (190 µm < ℓdefect < 350 µm) that characterize the quasi-hierarchical structure of the wing feathers of each bird species. These two structural parameters capture the most important structural aspects of the complicated texture of bird feathers in terms of a simple one-dimensional wetting cylindrical model. This approach provides a simple and quantitative method to investigate bird feathers, compared with previous work based on microscopic or photographic evaluation alone. Applying this systematic and self-consistent framework to measurements on feathers of other avian species will help to correlate the structure of bird feathers with observed aquatic behaviour.
Acknowledgements
The authors thank the Center for Materials Science and Engineering and the Institute of Soldier Nanotechnologies at MIT for the use of experimental facilities and Mr Justin Kleingartner for helpful discussion during the preparation of the manuscript.
Funding statement
The authors acknowledge financial support from the Army Research Office (ARO), the Office of Naval Research (ONR) and the MIT-Legatum Centre for Development and Entrepreneurship.
References
- 1.Salibian A, Montalti D. 2009. Physiological and biochemical aspects of the avian uropygial gland. Braz. J. Biol. 69, 437–446. ( 10.1590/S1519-69842009000200029) [DOI] [PubMed] [Google Scholar]
- 2.Brocher F. 1912. Recherches sur la respiration des insectes aquatiques adultes. Les Haemonia. Ann. Biol. Lacustre. 5, 5–26. [Google Scholar]
- 3.Thorpe WH. 1950. Plastron respiration in aquatic insects. Biol. Rev. 25, 344–390. ( 10.1111/j.1469-185X.1950.tb01590.x) [DOI] [PubMed] [Google Scholar]
- 4.Schutz DTM. 2003. Adaptations to an aquatic life may be responsible for the reversed sexual size dimorphism in the water spider, Argyroneta aquatica. Evol. Ecol. Res. 5, 105–117. [Google Scholar]
- 5.Anderson DS. 1960. The respiratory system of the egg-shell of Calliphora erythrocephala. J. Insect Physiol. 5, 120–128. ( 10.1016/0022-1910(60)90037-8) [DOI] [Google Scholar]
- 6.Hebets EA, Chapman RF. 2000. Surviving the flood: plastron respiration in the non-tracheate arthropod Phrynus marginemaculatus (Amblypygi: Arachnida). J. Insect Physiol. 46, 13–19. ( 10.1016/S0022-1910(99)00096-7) [DOI] [PubMed] [Google Scholar]
- 7.Hinton HE. 1960. Plastron respiration in the eggs of blowflies. J. Insect Physiol. 4, 176–183. ( 10.1016/0022-1910(60)90079-2) [DOI] [Google Scholar]
- 8.Thorpe WH, Crisp DJ. 1947. Studies on plastron respiration. J. Exp. Biol. 24, 227–269. [DOI] [PubMed] [Google Scholar]
- 9.Hsu SH, Woan K, Sigmund W. 2011. Biologically inspired hairy structures for superhydrophobicity. Mater. Sci. Eng. R Rep. 72, 189–201. ( 10.1016/J.Mser.2011.05.001) [DOI] [Google Scholar]
- 10.Grémillet D, Tuschy I, Kierspel M. 1998. Body temperature and insulation in diving great cormorants and European shags. Funct. Ecol. 12, 386–394. ( 10.1046/j.1365-2435.1998.00199.x) [DOI] [Google Scholar]
- 11.Wilson RP, Hustler K, Ryan PG, Burger AE, Noldeke EC. 1992. Diving birds in cold water: do Archimedes and Boyle determine energetic costs? Am. Nat. 140, 179–200. ( 10.1086/285409) [DOI] [Google Scholar]
- 12.Rijke AM. 1968. The water repellency and feature structure of cormorants, phalogrocorcidae. J Exp. Biol. 48, 185–189. [Google Scholar]
- 13.Gremillet D, Chauvin C, Wilson RP, Le Maho Y, Wanless S. 2005. Unusual feather structure allows partial plumage wettability in diving great cormorants Phalacrocorax carbo. J. Avian Biol. 36, 57–63. ( 10.1111/j.0908-8857.2005.03331.x) [DOI] [Google Scholar]
- 14.Rijke AM. 1970. Wettability and phylogenetic development of feather structure in water birds. J. Exp. Biol. 52, 469. [Google Scholar]
- 15.Henyemann WW. 1984. Spread-winged behaviour of double-crested and flightless cormorants Phalacrocorax auritus and P. harrisi: wing drying or thermoregulation? Ibis 126, 230–239. [Google Scholar]
- 16.Cassie ABD, Baxter S. 1944. Wettability of porous surfaces. Trans. Faraday Soc. 40, 546–551. ( 10.1039/TF9444000546) [DOI] [Google Scholar]
- 17.Elowson AM. 1984. Spread-wing postures and the water repellency of feathers: a test of Rijke's hypothesis. Auk 101, 371–383. [Google Scholar]
- 18.Rijke AM. 1987. The water repellency of water-bird feathers. Auk 104, 140–142. ( 10.2307/4087247) [DOI] [Google Scholar]
- 19.Rijke AM, Jesser WA. 2010. The feather structure of dippers: water repellency and resistance to water penetration. Wilson J. Ornithol. 122, 563–568. ( 10.1676/09-172.1) [DOI] [Google Scholar]
- 20.Rijke AM, Jesser WA. 2011. The water penetration and repellency of feathers revisited. Condor 113, 245–254. ( 10.1525/cond.2011.100113) [DOI] [Google Scholar]
- 21.Bormashenko E, Gendelman O, Whyman G. 2012. Superhydrophobicity of lotus leaves versus birds wings: different physical mechanisms leading to similar phenomena. Langmuir 28, 14 992–14 997. ( 10.1021/la303340x) [DOI] [PubMed] [Google Scholar]
- 22.Rijke AM, Jesser WA, Mahoney SA. 1989. Plumage wettability of the African darter Anhinga-melanogaster compared with the double-crested cormorant Phalacrocorax auritus. Ostrich: J. Afr. Ornithol. 60, 128–132. ( 10.1080/00306525.1989.9633739) [DOI] [Google Scholar]
- 23.Rijke AM, Jesser WA, Evans SW, Bouwman H. 2000. Water repellency and feather structure of the Blue Swallow Hirundo atrocaerulea. Ostrich 71, 143–145. ( 10.1080/00306525.2000.9639893) [DOI] [Google Scholar]
- 24.Berg JC. 1993. Wettability. New York, NY: M. Dekker. [Google Scholar]
- 25.Choi W, Tuteja A, Chhatre S, Mabry JM, Cohen RE, McKinley GH. 2009. Fabrics with tunable oleophobicity. Adv. Mater. 21, 2190–2195. ( 10.1002/adma.200802502) [DOI] [Google Scholar]
- 26.Mabry JM, Vij A, Iacono ST, Viers BD. 2008. Fluorinated polyhedral oligomeric silsesquioxanes (F-POSS). Angew. Chem. 47, 4137–4140. ( 10.1002/anie.200705355) [DOI] [PubMed] [Google Scholar]
- 27.Chhatre SS, Guardado JO, Moore BM, Haddad TS, Mabry JM, McKinley GH, Cohen RE. 2010. Fluoroalkylated silicon-containing surfaces−estimation of solid-surface energy. ACS Appl. Mater. Interfaces 2, 3544–3554. ( 10.1021/am100729j) [DOI] [PubMed] [Google Scholar]
- 28.Elder WH. 1954. The oil gland of birds. Wilson Bull. 66, 6–31. ( 10.2307/4158258) [DOI] [Google Scholar]
- 29.Ruiz-Rodríguez M, Valdivia E, Soler JJ, Martín-Vivaldi M, Martín-Platero AM, Martínez-Bueno M. 2009. Symbiotic bacteria living in the hoopoe's uropygial gland prevent feather degradation. J. Exp. Biol. 212, 3621–3626. ( 10.1242/jeb.031336) [DOI] [PubMed] [Google Scholar]
- 30.Jacob J, Ziswiler V. 1982. The uropygial gland. Avian Biol. 6, 199–324. ( 10.1016/B978-0-12-249406-2.50013-7) [DOI] [Google Scholar]
- 31.Dekker MH, Piersma T, Damste JS. 2000. Molecular analysis of intact preen waxes of Calidris canutus (Aves: Scolopacidae) by gas chromatography/mass spectrometry. Lipids 35, 533–541. (doi:papers2://publication/uuid/BE51BF84-01A0-4F9F-B0E6-6B4C7A6B78EB) [DOI] [PubMed] [Google Scholar]
- 32.Young T. 1805. An essay on the cohesion of fluids. Phil. Trans. R. Soc. Lond. 95, 65–87. ( 10.1098/rstl.1805.0005) [DOI] [Google Scholar]
- 33.Brockway LO, Jones RL. 1964. Electron microscopic investigation of the adsorption of long-chain fatty acid monolayers on glass. Contact angle, wettability, and adhesion. Adv. Chem. 43, 275–294. [Google Scholar]
- 34.Wenzel RN. 1936. Resistance of solid surfaces to water by wetting. Ind. Eng. Chem. 28, 988–994. ( 10.1021/ie50320a024) [DOI] [Google Scholar]
- 35.Bormashenko E, Bormashenko Y, Stein T, Whyman G, Bormashenko E. 2007. Why do pigeon feathers repel water? Hydrophobicity of pennae, Cassie–Baxter wetting hypothesis and Cassie–Wenzel capillarity-induced wetting transition. J. Colloid Interface Sci. 311, 212–216. ( 10.1016/j.jcis.2007.02.049) [DOI] [PubMed] [Google Scholar]
- 36.Brakke KA. 1992. The surface evolver. Exp. Math. 1, 141–165. ( 10.1080/10586458.1992.10504253) [DOI] [Google Scholar]
- 37.Tuteja A, Choi W, Mabry JM, McKinley GH, Cohen RE. 2008. Robust omniphobic surfaces. Proc. Natl Acad. Sci. USA 105, 18 200–18 205. ( 10.1073/pnas.0804872105) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 38.Butt H-J, Semprebon C, Papadopoulos P, Vollmer D, Brinkmann M, Ciccotti M. 2013. Design principles for superamphiphobic surfaces. Soft Matter 9, 418–428. ( 10.1039/C2SM27016A) [DOI] [Google Scholar]
- 39.Marmur A. 2009. Solid-surface characterization by wetting. Annu. Rev. Mater. Res. 39, 473–489. ( 10.1146/annurev.matsci.38.060407.132425) [DOI] [Google Scholar]
- 40.Great Cormorant (Phalacrocorax carbo) 2000. The Birds of North America Online (A. Poole, Ed.). Ithaca: Cornell Lab of Ornithology. 2000 (accessed 08 August 2012). See http://bna.birds.cornell.edu/bna/species/553/articles/introduction .
- 41.Marmur A. 2003. Wetting on hydrophobic rough surfaces: to be heterogeneous or not to be? Langmuir 19, 8343–8348. ( 10.1021/la0344682) [DOI] [Google Scholar]
- 42.Tuteja A, Choi W, Ma ML, Mabry JM, Mazzella SA, Rutledge GC, McKinley GH, Cohen RE. 2007. Designing superoleophobic surfaces. Science 318, 1618–1622. ( 10.1126/science.1148326) [DOI] [PubMed] [Google Scholar]
- 43.Lafuma A, Quere D. 2003. Superhydrophobic states. Nat. Mater. 2, 457–460. ( 10.1038/nmat924) [DOI] [PubMed] [Google Scholar]