The Proximate Determinants of Educational Homogamy: The Effects of First Marriage, Marital Dissolution, Remarriage, and Educational Upgrading

Abstract

This paper adapts the population balancing equation to develop a framework for studying the proximate determinants of educational homogamy. Using data from the National Longitudinal Survey of Youth on a cohort of women born between 1957 and 1964, we decompose the odds of homogamy in prevailing marriages into four proximate determinants: (1) first marriages, (2) first and later marital dissolutions, (3) remarriages, and (4) educational attainment after marriage. The odds of homogamy among new first marriages are lower than among prevailing marriages, but not because of selective marital dissolution, remarriage, and educational attainment after marriage, as has been speculated. Prevailing marriages are more likely to be educationally homogamous than new first marriages because of the accumulation of homogamous first marriages in the stock of marriages. First marriages overwhelmingly account for the odds of homogamy in prevailing marriages in this cohort. Marital dissolutions, remarriages, and educational upgrades after marriage have relatively small and offsetting effects. Our results suggest that, despite the high prevalence of divorce, remarriage, and continued schooling after marriage in the United States, the key to understanding trends in educational homogamy lies primarily in variation in assortative mating into first marriage.

Introduction

Unions between men and women with varying social and biological characteristics establish the families that bear and raise the next generation of children. Thus assortative mating, the patterns of resemblance of marital partners and parents, is of central interest to social scientists. Because marriage creates intimate ties between individuals and families, intermarriage across social boundaries is a measure of the social distance between groups and the “openness” of societies (Kalmijn 1998). Moreover, the extent to which husbands and wives resemble each other on traits such as educational attainment, family wealth, and earnings may be a key component of inequality among families (Fernández and Rogerson 2001; Schwartz 2010b). Given the importance of assortative mating patterns, a key question is how and why they vary across populations and over time.

To investigate this question, many scholars have examined the extent to which variation in broad social and economic forces, including cultural mores, the roles and statuses of women, macroeconomic conditions, and patterns of social inequality can explain variation in spousal resemblance (e.g., Fernández et al. 2005; Raymo and Xie 2000; Smits et al. 1998; Torche 2010). Demographers and other social scientists have yet to perform a more basic exercise, however: they have yet to understand the mechanics behind how spousal resemblance in populations is generated. With respect to fertility, Bongaarts (1978) showed that all social and economic factors must work through one or more “proximate determinants.” For example, for economic change to influence fertility it must affect one of its proximate determinants, such as the proportion of women who marry or have sexual partners, the proportion of couples using contraception, the prevalence of abortion, or the length of postpartum infecundability.

In this paper, we adapt the population balancing equation to develop a framework for studying the proximate determinants of the educational resemblance of spouses. Although the framework we develop could be applied to assortative mating on any characteristic, we focus on education as this measure dominates studies concerned with the connection between assortative mating and inequality (e.g., Blau and Duncan 1967; Mare 1991; Smits et al. 1998). We use this framework to decompose the extent to which changes in spouses’ educational resemblance among all married couples, or in the stock of marriages, arise from four flows: (1) entry into first marriages, (2) first and later marital dissolutions, (3) remarriages, and (4) postmarital educational upgrades.

Differences in educational homogamy—or the tendency for spouses to resemble each other on education—across times and places can always be traced to one of these components. For example, the liberalization of divorce laws may have made it less costly for mismatched couples to divorce, but this would be reflected in an increase in the numbers of heterogamous couples who divorce. In this sense, the four flows identified above can be viewed as the proximate determinants of changes in spousal resemblance, whereas the social and economic forces behind these changes can be viewed as indirect determinants. We apply our framework to the marriage patterns of a single cohort of American women born between 1957 and 1964 using data from the National Longitudinal Survey of Youth (NLSY79).

This paper contributes to the literature in several ways. First, past research has recognized the contributions of first marriages, marital dissolutions, remarriages, and postmarital educational upgrades to spousal resemblance (Kalmijn 1991a; Raymo and Xie 2000; Schwartz and Mare 2005), but no study has quantified the contribution of these effects. In so doing, our paper integrates a series of disconnected yet intimately related literatures on factors affecting spousal resemblance (e.g., Clarkwest 2007; Jacobs and Furstenberg 1986; Lichter 1990; Weiss and Willis 1997), and formalizes past work examining couple resemblance from a stock and flow perspective (Schwartz 2010a). Schwartz (2010a) performed a stock and flow analysis of differences in educational homogamy between marital and cohabiting unions using NLSY79 data, but did not formally decompose these differences. Second, our findings have practical implications for researchers who are interested in studying entry into first marriages but have data only on existing marriages, and for those who compare spousal resemblance across countries or groups with different divorce, remarriage, and postmarital educational upgrading rates. Third, our study sets the stage for future research on how changes in marriage timing, remarriage, and divorce have affected trends in the resemblance between spouses. Once we understand the dynamics of assortative mating across one cohort’s life, we can show how these demographic changes have contributed to changes in assortative mating across time and space and what basic demographic trends may portend for the future.

Educational Homogamy as a Dynamic Process

Assortative mating has largely been conceptualized as a process of sorting into marriage (e.g., Becker 1974; Kalmijn 1998; Mortensen 1988). However, changes that occur at multiple points in individuals’ lives may affect the resemblance of spouses in the population. Couples who have made a “bad match” may offset this via “postmarital adaptive socialization” (Oppenheimer 1988), or may be more weakly attached to marriage and thus more likely to divorce (Becker et al. 1977).

If educational homogamy is a dynamic process that changes over people’s lives as individuals make decisions about marriage, education, and divorce, how should we measure spousal resemblance? The answer to this depends on the question of interest. If we are interested in how marriage markets or particular historical or national contexts influence who marries whom, examining assortative mating among recently wed couples, or newlyweds, is appropriate. However, if we are interested in the implications of spousal resemblance for the intergenerational transmission of inequality or the distribution of resources across families, it may be preferable to examine a wide cross section of marriages, or prevailing marriages, because they are representative of all marriages in the population at a given time (Schwartz and Mare 2005). This approach is used by studies that examine the impact of changes in marriage patterns on increases in economic inequality in the United States (e.g., Cancian and Reed 1999; Karoly and Burtless 1995; Schwartz 2010b).

This discussion assumes, however, that it is necessary to pick one cross section of the data to examine assortative mating. Past research has primarily relied on cross-sectional data to examine trends and thus cannot assess the extent to which the resemblance of spouses is due to the way in which people sort into or out of marriage or change their characteristics within marriage. The typical response to these issues has been to control for the effects of changes that occur after marriage by examining the educational characteristics of spouses as closely as possible to the time of their weddings—that is, among newlyweds (e.g., Kalmijn 1994; Mare 1991; Qian 1998; Qian and Preston 1993). When data on newlyweds are not available, researchers often restrict their samples to young couples to avoid the effects of divorce, remarriage, and postmarital educational upgrades (Blackwell and Lichter 2000; Qian 1997; Qian and Lichter 2007; Rosenfeld 2008) or use data on wide cross sections of marriages (Schwartz and Mare 2005; Smits et al. 1998; Torche 2010; Ultee and Luijkx 1990).

Researchers who use cross-sectional data on prevailing marriages, however, are often interested in making inferences about sorting into unions (e.g., Blackwell and Lichter 2000; Qian 1997; Rosenfeld 2008; Smits et al. 1998). Thus, determining how well samples of prevailing marriages reflect patterns of entry into marriage is of crucial interest to many scholars of assortative mating. By examining assortative mating as a dynamic process that does not end upon entry into first marriage, this paper shows how newlyweds become prevailing marriages, thus providing an empirical link between studies that use data on prevailing marriages and those that use data on newlyweds. Given the high incidence of divorce, remarriage, and educational attainment after marriage in the United States (Bumpass and Call 1989; Bumpass et al. 1990; Goldstein 1999), homogamy among new first marriages may be quite different than among prevailing marriages. These factors may also affect scholars’ interpretations of differences in assortative mating across countries or groups.

Stocks and Flows of Educational Homogamy

How might the proximate determinants of educational homogamy (the flows) affect the resemblance of prevailing marriages (the stock)? Previous research offers clues about their likely effects, but no study has quantified the contribution of each to the resemblance of spouses.

First Marriage

Empirical evidence suggests that educational homogamy declines at older ages. Lichter (1990) found that women who marry after the age of 30 are more likely to marry less-educated men, perhaps because there are fewer potential partners from which to choose or because marriage markets become more structured by educationally heterogeneous environments, such as work, with age (Lewis and Oppenheimer 2000; Mare 1991; Shafer and Qian 2010). But homogamy among those who marry at younger ages may also be lower than among those who marry at older ages if young people are more likely match on expected rather than completed education. College sweethearts who marry after one partner has graduated while the other is still in school differ in their educational attainment at marriage but become homogamous upon graduation. Assortative mating may also be less important for young people because they are less established in their careers and identities than older adults (Oppenheimer 1988).

A reconciliation of these hypotheses, which is consistent with empirical research (Schwartz 2010a; Weiss and Willis 1997:S318), is that age patterns of educational homogamy follow an inverted-U pattern, with lower odds of homogamy among those who marry young and among those who marry relatively late. Given these hypotheses and findings, we expect that first marriages increase the odds of homogamy in the stock of marriages at young ages but reduce them at older ages.

Marital Dissolution

Most studies have found that heterogamous marriages are more likely to dissolve, especially when wives have more education than their husbands (Bumpass et al. 1991; Clarkwest 2007; Kalmijn 2003; Schwartz 2010a; Tzeng and Mare 1995). This suggests that couples leaving the stock of marriages are less alike than couples who remain, and thus that marital dissolutions increase the odds of homogamy in the stock of marriages.

Remarriage

Remarriages tend to be less educationally homogamous than first marriages (Dean and Gurak 1978; Jacobs and Furstenberg 1986). These findings may partially be the result of selection, whereby women who have had only one marriage have higher odds of homogamy than do remarried women in either their first or second marriages (Dean and Gurak 1978). Nevertheless, given that remarriages tend to be less homogamous than first marriages on average, we expect that remarriage has a negative impact on educational homogamy.

Postmarital Educational Upgrading

Previous research has examined the potential convergence of spouses’ attitudes, values, and personality traits (Caspi and Herbener 1993), but no study to our knowledge has examined whether spouses’ educational characteristics tend to converge. Educational changes may result in greater homogamy if heterogamous couples become homogamous as the result of continued schooling. By contrast, educational changes may result in lower odds of homogamy if initially homogamous couples pursue a specialization strategy in which one partner returns to school to increase the family’s earnings.

In sum, prior research suggests that opposing forces may be at work in the assortative mating process. First marriages may increase the odds of homogamy at younger ages and decrease them at older ages. Marital dissolutions may increase homogamy in prevailing marriages as dissimilar marriages dissolve, but remarriages may have the opposite effect.

Data and Measurement

We use the National Longitudinal Survey of Youth (NLSY79), a panel study of 12,686 men and women aged 14 to 21 as of December 31, 1978, to conduct our analysis. We restrict our analysis to the cross-sectional sample and the Hispanic and black over-samples, which consist of 9,763 respondents. Respondents were first interviewed in 1979 and were reinterviewed yearly through 1994 and then biennially. We use interviews conducted from 1979 to 2002.

Because the spouses of NLSY79 respondents may not be part of the cohort of youth aged 14 to 21 as of December 31, 1978, we follow female respondents from age 16 to 41 (n = 4,922) regardless of their husbands’ age, which preserves the cohort interpretation of the analysis. This age range roughly correspondents to the ranges used in past research (Schwartz and Mare 2005; Smits et al. 1998). Women aged 16 to 41 contribute 87,004 person-years to the analysis.

Our data are structured such that we know the exact ages of women at the time of their interviews, but we do not know the exact timing of all marital and educational transitions. Thus, we consistently know only whether an event occurred between interviews. Because we are examining status changes between interviews at ages x and x + 1, the last interview year, on its own, does not inform our analysis. Dropping the last interview year reduces the number of person-years to 86,314. Moreover, a small number of women had large gaps between interviews that make identifying the precise timing of events difficult. To reduce error introduced by these gaps, we restrict the sample to person-years for which the next interview occurred within three years (n = 82,672). In addition, a small percentage of marital status transitions (about 5%) were illogical or inconsistent, such as moving from married to never married. We limit our analysis to person-years between which logical transitions or no transitions occurred (n = 82,224).

We classify education into four categories based on years of schooling completed: <12, 12, 13 to 15, and≥16 years.¹ Missing or invalid data on women’s or spouses’ education were imputed from adjacent years where possible. We drop person-years in which respondents’ or spouses’ education characteristics are missing or when respondents’ or spouses’ education category declines, for a final sample of 81,589 person-years.

Methods

Balancing Equation

We formalize how the proximate determinants of educational homogamy affect resemblance in the stock of marriages by adapting the population balancing equation, which states that the population in year t + 1 is equal to the previous year’s population, plus births and in-migration, minus deaths and out-migration (Preston et al. 2001). Similarly, as a cohort ages, there are two ways in which the number of marriages between husbands of education i and wives of education j can increase. Couples can either be “born” into the education category, that is, they can enter the category by forming a new first or later marriage; or they can “in-migrate” by continuing their schooling. Likewise, there are two ways in which the number of marriages between husbands and wives in joint education category ij can decrease. Couples can exit the category through the “death” of a first or later marriage via separation, divorce, or widowhood; or they can leave the joint education category through “out-migration” via postmarital educational attainment. This can be represented formally as

$$l_{x+1ij}^M=l_{xij}^M{+}_1{d}_{xij}^F{-}_1{d}_{xij}^{D1}{+}_1{d}_{xij}^R{-}_1{d}_{xij}^{D2}{+}_1{d}_{xij}^I{-}_1{d}_{xij}^O,$$ (1)

where i = husband’s education (i = <12, 12, 13-15,≥16), j = wife’s education (j = <12, 12, 13-15,≥16), x = wife’s age (x = 16, . . ., 41), $l_{xij}^M$ = the number of prevailing marriages at age x among couples with joint education ij, ${}_1{d}_{xij}^F$ = the number of new first marriages between ages x and x + 1 among couples with joint education ij, ${}_1{d}_{xij}^{D1}$ = the number of dissolutions from first marriages (divorce, separation, or widowhood) between ages x and x + 1 among couples with joint education ij, ${}_1{d}_{xij}^R$ = the number of new remarriages between ages x and x + 1 among couples with joint education ij, ${}_1{d}_{xij}^{D2}$ = the number of dissolutions from remarriages (divorce, separation, or widowhood) between ages x and x + 1 among couples with joint education ij, ${}_1{d}_{xij}^I$ = the number of couples between ages x and x + 1 who enter the ijth education category as a result of an educational upgrade (in-migration), and ${}_1{d}_{xij}^O$ = the number of couples between ages x and x + 1 who exit the ijth education category as a result of an educational upgrade (out-migration).

Thus, the number of marriages between husbands of education i and wives of education j at wife’s age x + 1 ($l_{x+1ij}^M$) is made up of those marriages that existed at age x ($l_{xij}^M$), plus the number of new first marriages between ages x and x + 1 (${}_1{d}_{xij}^F$), minus the number of marital dissolutions (${}_1{d}_{xij}^{D1}$ + ${}_1{d}_{xij}^{D2}$), plus the number of remarriages (${}_1{d}_{xij}^R$), plus the in-migration of couples to joint education category ij (${}_1{d}_{xij}^I$), minus the out-migration of couples from joint education category ij (${}_1{d}_{xij}^O$).

The balancing equation provides the conceptual basis for our analysis, but the numbers of marriages at exact age x ($l_{xij}^M$) are not analogous to stock measures of marriage from survey data. Cross-sectional data contain information on marriages in a given age range—say, those who are at least 18 years old but younger than 19— whereas $l_{xij}^M$ refers to the number of marriages at exact age x. For comparability with data used in previous empirical research, we decompose the impact of the flows on the stocks, that is, the number of married person-years spent in education category ij between ages x and x + n, where n is the width of the age interval. This quantity is estimated as ${}_n{L}_{xij}^M$, where ${}_n{L}_{xij}^M=(l_{x+1ij}^M+l_{xij}^M)∕2$ (Preston et al. 2001).

Multistate Life Tables

We estimate the stocks and flows into and out of marriage shown in Eq. (1) using multistate life tables. The building blocks of the multistate life tables are transition rates into and out of marriage and education categories. To estimate the rates, we first classify women into one of four marital states as shown in Fig. 1: (1) never married, (2) married, (3) separated, divorced, or widowed, or (4) remarried. Women have education i, and couples have joint education ij. We calculate the age- and education-specific rates with which women transition (a) from singlehood to first marriage (${\lambda }_{ij}^F$), (b) from first marriage to separated, divorced, or widowed (${\lambda }_{ij}^{D1}$), (c) from separated, divorced, or widowed to remarriage (${\lambda }_{ij}^R$) and (d) from remarried to separated, divorced, or widowed (${\lambda }_{ij}^{D2}$). (See Table 4 in the appendix for definitions and sample sizes.) Within each of these states, we also calculate educational upgrading rates. The numerators for the rates are the numbers of events between ages x and x + n, and the denominators are the numbers of person-years at risk. Separate rates are calculated for interviews separated by one, two, and three years; they are weighted using the 1979 sampling weights. These rates are averaged proportionate to the number of person-years they contribute, thereby producing a single set of transition rates by female respondent’s age. Because few marital events occur before age 18, we present results for female respondents aged 18 to 41 (n = 78,220), but the radix for our multistate life table is the weighted distribution of women in the each of the states shown in Fig. 1 at age 16.²

Fig. 1.

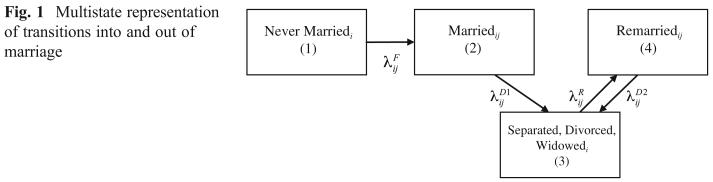
Multistate representation of transitions into and out of marriage

The multistate life table uses the transition rates to produce implied marital status and joint education distributions by age. The disadvantage of using the raw data for our analysis is that the numbers of prevailing marriages observed from one age to the next are not only a function of the processes of interest to us here, but are also affected by censoring and survey attrition. Multistate life table methods are preferred because the implied marriage distributions are a function of only the transition rates of interest. Thus, when using the distributions estimated from the multistate life table, the balancing equation adds up exactly, and because the multistate life table represents a complete and interrelated system of states and transitions, counterfactual rates can easily be substituted for the observed rates to perform simulations and decompositions.³

Log-Linear Models

To summarize patterns of educational homogamy, we use data from our multistate life table and log-linear models for contingency tables. Our contingency table is produced by cross-classifying female respondent’s education by spouse’s education and respondent’s age. We use log-linear models because they allow us to examine the association between couples’ education while controlling for changes in the distribution of education by age. This feature is particularly important for this analysis because there are large shifts in the education distribution of married couples across the ages we examine.

To summarize the association, we use homogamy models, which describe the association between spouses’ education in terms of the odds that husbands and wives have the same rather than different levels of education. Homogamy is the dominant concept of association in the assortative mating literature, and these models allow us to describe and decompose spousal resemblance using a single intuitive measure. Interested readers should refer to Online Resource 1 for results of analyses using alternative measures of educational resemblance.

We use two types of homogamy models. The first allows the odds of homogamy to vary by wife’s age:

$$\log \left({\mu }_{ijk}\right)=\lambda +{\lambda }_i^H+{\lambda }_j^W+{\lambda }_k^A+{\lambda }_{ik}^{HA}+{\lambda }_{jk}^{WA}+{\delta }_1{d}^O+{\delta }_2{d}^{O}A+{\delta }_3{d}^O{A}^2,$$ (2)

where H is husbands’ education (i = 1, …, 4), W is wives’ education (j = 1, …, 4), A is wives’ age category (k = 18–21, …, 38–41), d^O is a dummy variable for homogamy (d^O = 1 where i = j, and 0 otherwise), and ${\mu }_{ijk}$ is the weighted expected number of marriages between husbands and wives with joint education ij among wives in age category k. This model parameterizes changes in the log odds of homogamy by age with a quadratic function.

The second homogamy model estimates the “average” odds of homogamy across wife’s age by omitting the interaction terms between homogamy and age (δ₂d^OA, δ₃d^OA²). We present results from these models for consistency with prior research because most studies have estimated their association parameters across a relatively wide age range (e.g., Kalmijn 1991b; Qian 1998; Schwartz and Mare 2005; Smits et al. 1998) and because sample sizes by age for some transitions are small. We also use this model to perform our decomposition of the extent to which the odds of homogamy across this age span are affected by each of the flows.

Simulation and Decomposition

To conduct the decomposition, we perform a series of simulations in which we successively increase the number of flows that may affect the stock of marriages.

$$\begin{array}{cc}{l}_{x+1ij}^M=l_{xij}^M{+}_1{d}_{xij}^F\hfill & (\mathrm{S}1:\text{First marriages only})\hfill \\ l_{x+1ij}^M=l_{xij}^M{+}_1{d}_{xij}^F{-}_1{d}_{xij}^{D1}\hfill & (\mathrm{S}2:+\text{First dissolutions})\hfill \\ l_{x+1ij}^M=l_{xij}^M{+}_1{d}_{xij}^F{-}_1{d}_{xij}^{D1}{+}_1{d}_{xij}^R\hfill & (\mathrm{S}3:+\text{Remarriages})\hfill \\ l_{x+1ij}^M=l_{xij}^M{+}_1{d}_{xij}^F{-}_1{d}_{xij}^{D1}{+}_1{d}_{xij}^R{-}_1{d}_{xij}^{D2}\hfill & (\mathrm{S}4:+\text{Later dissolutions})\hfill \\ l_{x+1ij}^M=l_{xij}^M{+}_1{d}_{xij}^F{-}_1{d}_{xij}^{D1}{+}_1{d}_{xij}^R{-}_1{d}_{xij}^{D2}{+}_1{d}_{xij}^I{-}_1{d}_{xij}^O\hfill & (\mathrm{S}5:+\text{Educational upgrades})\hfill \end{array}$$

In S1, the stock of marriages among spouses with joint education ij at wife’s age x + 1 is the sum of the number of existing marriages with joint education ij at age x and the number of new first marriages among spouses with joint education ij (${}_1{d}_{xij}^F$). This creates a new distribution of marriages under the counterfactual that the stock of marriages is affected only by new first marriages. The quantities in S1 are estimated using a multistate life table in which all the transition rates shown in Fig. 1 are set to zero after age 18 except for transition rates into first marriage (${\lambda }_{ij}^F$). We then estimate log-linear homogamy models using the simulated data. The coefficients from these models give the odds of homogamy if first marriages were the only flow to have affected the stock of marriages.

In S2, the stock of marriages is affected by both first marriages (${}_1{d}_{xij}^F$) and dissolutions from first marriages (${}_1{d}_{xij}^{D1}$). To estimate these quantities, we use a multistate life table in which all of the rates shown in Fig. 1 are set to zero after age 18 except for transitions to first marriages and first marital dissolutions (${\lambda }_{ij}^F$ and ${\lambda }_{ij}^{D1}$). As in the first simulation, we estimate log-linear homogamy models using the simulated data. The difference in the log odds of homogamy estimated from S2 and S1 is an estimate of the impact of first marital dissolutions on homogamy.

S3 to S5 proceed in an analogous manner; each simulation adds a new marital or educational transition, and the difference in the odds of homogamy between successive simulations is an estimate of the impact of the added component.

Results

Marital and Educational Status Transitions

The relative impact of first marriages, marital dissolutions, remarriages, and postmarital educational upgrading on spousal resemblance is determined by two components: (1) how selective the transition is of homogamous couples and (2) how frequent these events are. For example, even if divorce is highly selective of heterogamous couples, divorce will have little impact on homogamy in the stock of marriages if it is very rare. Thus, to provide context for the log-linear model results to come, we use life tables to describe the marital and educational experiences and characteristics of women in the NLSY79 cohort.

Table 1 shows that the vast majority of women in the NLSY79 cohort married before the age of 41 (88%). The median age of first marriage for women was 23.7, which is consistent with other estimates from around this period (U.S. Census Bureau 2006). During this cohort’s prime marrying years, divorce rates were at near all-time highs in the United States (Goldstein 1999). The high rate of marital dissolution is reflected in the NLSY79: about 37% of first marriages had dissolved after 10 years of marriage, an estimate similar to those using other data from around this time (Martin 2006). Although remarriage rates after divorce declined somewhat in the 1980s and 1990s, Table 1 also shows that more than two-thirds of women remarried within 10 years of a marital dissolution. Finally, a nontrivial percentage of couples increased their education after marriage. Bumpass and Call (1989:10, Table 4) estimated that more than 40% of married men and women who turned 30 in the late 1980s had been enrolled in school at some point after marriage. Our measure differs somewhat from theirs in that we measure education transitions from one category to the next rather than in single years, but we nevertheless find that in about 24% of marriages, at least one spouse experienced an educational transition within 10 years of marriage.

Table 1.

Life table estimates of women’s marriage and education transitions and characteristics

Percentage First Married by Age 41	87.5
Median Age at First Marriage	23.7
Percentage of First Marriages Dissolved Within 10 Years of Marriage	37.2
Percentage Remarried Within 10 Years of a Marital Dissolution	68.9
Percentage of Marriages With an Education Category Upgrade Within 10 Years of Marriage
Either upgrades	24.2
Wife upgrades	13.6
Husband upgrades	14.0
Wife’s Years of Schooling at First Marriagea (%)
<12	6.8
12	37.2
13–15	28.5
≥16	27.5
Husband’s Years of Schooling at First Marriagea (%)
<12	10.3
12	40.5
13–15	21.7
≥16	27.5

Note: Data are weighted using 1979 sampling weights.

Source: National Longitudinal Survey of Youth (NLSY79), 1979–2002.

^aCalculated for couples in which wives are aged 18 to 41.

Table 1 also shows husbands’ and wives’ education at the time of wives’ first marriage. The modal category for both sexes is 12 years of schooling, but substantial fractions had either “some college” or college. These education distributions are quite similar to those estimated for newlyweds from June Current Population Survey (CPS) data between 1980 and the mid-1990s (authors’ calculations).

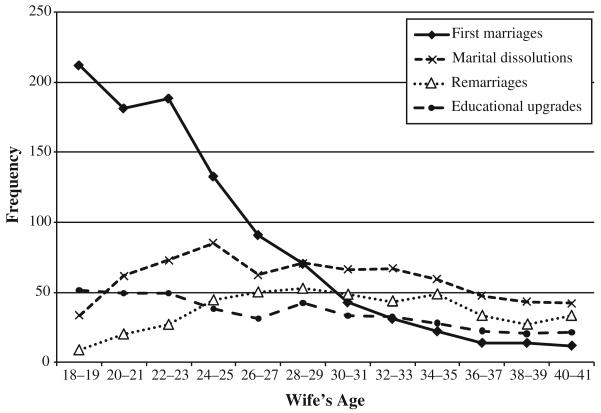
Figure 2 shows the frequency of these events by wife’s age estimated from multistate life tables (beginning with 1,346 16-year-old female respondents (l₀)), and illustrates which flows have the most potential to affect resemblance in the stock of marriages. First marriages are by far the most common transition through women’s late 20s. Marital dissolutions, remarriages, and educational upgrades are less common, although their numbers exceed the numbers of new first marriages after women’s mid-30s. Overall, the prevalence of these events suggests that each of the proximate determinants may substantially affect spousal resemblance in this cohort.

Fig. 2.

Multistate life table estimates of numbers of marital and educational transitions. Data are weighted using 1979 sampling weights. l₀ = 1,346 16-year-old female respondents. Source: National Longitudinal Survey of Youth (NLSY79), 1979–2002

Educational Homogamy in the Stocks and Flows

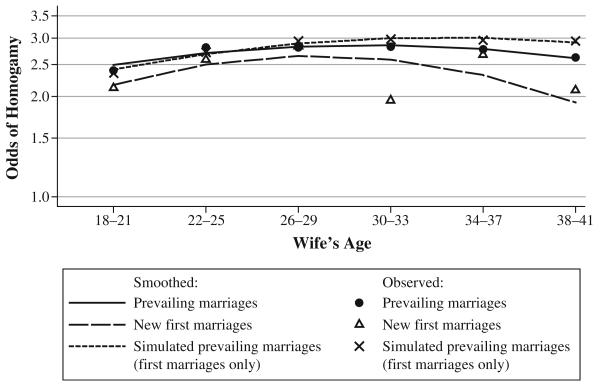
Figure 3 presents the results of our log-linear models by age using data from the multistate life tables. Age patterns are smoothed with a quadratic function (Eq. (2)) and are also shown unsmoothed (observed). We show these patterns for three samples: prevailing marriages, new first marriages, and a simulated distribution of prevailing marriages. We focus on the odds of homogamy in prevailing and new first marriages here and discuss the simulated age pattern in the next section. Figure 3 shows that the odds of homogamy range from about 2:1 to 3:1, depending on wife’s age and the sample. The figure also shows that younger and older wives have somewhat lower odds of homogamy than wives in their late 20s and early 30s. Although the odds of homogamy in prevailing marriages vary only slightly by wife’s age, large upward or downward shifts in these odds across the entire age range may still be generated by the flows into and out of marriage.

Fig. 3.

Odds of educational homogamy in prevailing marriages, new first marriages, and simulated prevailing marriages. Data are weighted using 1979 sampling weights. Estimates are from log-linear models using data from multistate life tables. Source: National Longitudinal Survey of Youth (NLSY79), 1979–2002

The inverted-U shape of the relationship between the odds of homogamy and age is more pronounced for new first marriages, consistent with our expectations based on past research.⁴ Another notable feature of Fig. 3 is that at every age, the odds of homogamy among new first marriages are lower than they are for prevailing marriages. Our decomposition (discussed below) reveals the reasons for these differences.

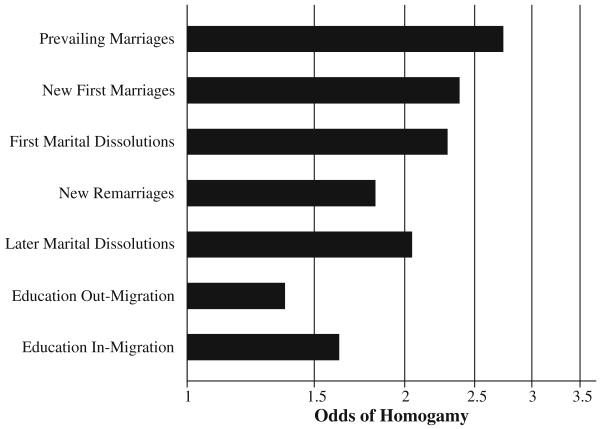
Because of the relatively small sample sizes by age for the other transitions, Fig. 4 summarizes the odds of homogamy for remarriages, first and later marital dissolutions, and postmarital educational upgrades across the 18–41 age span. For comparison, we also show the odds of homogamy pooled across wife’s age for prevailing marriages and new first marriages. As in Fig. 3, Fig. 4 shows that prevailing marriages are more likely to be homogamous than new first marriages. Couples who dissolve their first marriages are somewhat less likely to be homogamous than those in prevailing and new first marriages, a result consistent with past findings (Clarkwest 2007; Kalmijn 2003; Schwartz 2010a). This implies that first marital dissolutions increase the likelihood of homogamy in the stock of marriages as dissimilar couples exit their marriages. Remarriages are also less likely to be homogamous than new first marriages and prevailing marriages. This implies that remarriages contribute to a decline in the odds of homogamy among prevailing marriages as these couples enter the stock of marriages. Likewise, couples who dissolve their remarriages are less likely to be homogamous than those in prevailing marriages, again suggesting that later marital dissolutions may increase the odds of homogamy in the stock. Because there are relatively few dissolutions from remarriages, however, we expect the impact of dissolutions from first marriages to dominate the impact of dissolutions from remarriages. Finally, couples in which one or both spouses change their education are less likely to be homogamous before their educational transitions than after, suggesting that educational changes increase the odds of homogamy in the stock of marriages. To summarize, our results thus far suggest that remarriages decrease the resemblance of the stock of marriages, but marital dissolutions and educational upgrades increase its resemblance. Next, we show the extent to which each of these flows affects homogamy in the stock of marriages.

Fig. 4.

Odds of educational homogamy in the stocks and flows of marriages. Data are weighted using 1979 sampling weights. Estimates are from log-linear models using data from multistate life tables. Wives are aged 18 to 41. Source: National Longitudinal Survey of Youth (NLSY79), 1979–2002

Simulation and Decomposition

First Marriages

In addition to age patterns of homogamy for prevailing and new first marriages, Fig. 3 shows results using a simulated distribution of prevailing marriages generated under the assumption that first marriages were the only marital or educational transition to have occurred after age 18 (S1). Figure 3 shows that, if first marriages were the only flow to have affected the stock, the level and age pattern of homogamy in prevailing marriages would have been very similar to that observed, with the exception of somewhat higher odds of homogamy at older ages. This simulation reveals an important finding: marital dissolutions, remarriages, and educational upgrades are unnecessary to produce higher odds of educational homogamy in the stock of marriages than among newlyweds. The accumulation of first marriages alone can account for the higher odds of homogamy among prevailing marriages.

Why does the accumulation of first marriages produce higher odds of homogamy among prevailing marriages than new first marriages? To understand this, we turn to age patterns of homogamy among new first marriages and entry into first marriage. Most first marriages in this cohort took place before women were about 27 years old (Fig. 2). At these ages, the odds of homogamy among new first marriages trend upward (Fig. 3). In S1, once women marry for the first time, they do not divorce, change their education, or remarry, but remain in their first marriages through age 41. Because relatively few new first marriages occur in the downward portion of the trend and many homogamous marriages have already accumulated in the stock of marriages, the downward trend among newlyweds has little effect on the odds of homogamy in the stock. By contrast, because each new first marriage is weighted equally in the cross section, the odds of homogamy among new first marriages are lower than among prevailing marriages. Therefore, even if there were no educational changes, remarriages, or marital dissolutions, the odds of homogamy would still be higher in the stock of marriages than among new first marriages.

Marital Dissolutions, Remarriages, and Educational Upgrades

Table 2 shows the odds and log odds of homogamy in observed prevailing marriages using data from each of the five simulated marriage distributions. We use the counterfactual log odds to decompose the impact of the proximate determinants on the log odds of homogamy in the stock of marriages among wives aged 18 to 41. We decompose the log odds rather than the odds because the decomposition is additive in the log odds but multiplicative in the odds. Table 2 also shows the percentage contribution of each flow to the log odds of homogamy in prevailing marriages and gives a bootstrapped 95% confidence interval for these quantities.⁵

Table 2.

Decomposition of the log odds of educational homogamy in prevailing marriages

Sample or Simulation	Odds	Log Odds	Contribution to Log Odds	Percentage Contribution (%)	95% CI for Percentage Contribution (%)a
Observed Prevailing Marriages	2.737	1.007	—	—	—
First Marriages (S1)	2.882	1.058	1.058	105.10	[95.0, 115.2]
+ First Dissolutions (S2)	2.942	1.079	0.021	2.07	[−3.95, 8.09]
+ Remarriages (S3)	2.773	1.020	−0.059	−5.90	[−10.9, −0.88]
+ Later Dissolutions (S4)	2.761	1.015	−0.004	−0.43	[−3.65, 2.79]
+ Educational Upgrades (S5)	2.737	1.007	−0.009	−0.86	[−7.74, 6.02]
Total			1.007	100.00

Notes: Data are weighted using 1979 sampling weights. Estimates are from log-linear models using data from multistate life tables. Wives are aged 18 to 41.

Source: National Longitudinal Survey of Youth (NLSY79), 1979–2002.

^a95% confidence intervals (CIs) are estimated using 1,000 bootstrapped samples.

As is evident in Fig. 3, Table 2 shows that the odds of homogamy for observed prevailing marriages are slightly lower than if first marriages were the only flow to have affected the stock (S1). Under this counterfactual, the log odds of homogamy would have been 1.058, rather than 1.007 as observed. Thus, first marriages explain 105% (1.058 / 1.007 × 100) of the log odds of homogamy in prevailing marriages. The 95% confidence interval for this estimate indicates that although we can say with relative certainty that first marriages overwhelmingly account for the odds of homogamy in prevailing marriages, we cannot claim with great confidence that the odds of homogamy would be higher than observed if first marriages alone had affected the odds of marriage.

The contribution of first marital dissolutions to the log odds of homogamy in the stock of marriages can be calculated by subtracting S1 from S2 (1.079 – 1.058 = 0.021). These results imply that first marital dissolutions increase the log odds of homogamy in the stock of marriages by about 2% (0.021 / 1.007 × 100).⁶ By comparison, remarriages, later marital dissolutions, and educational upgrades decrease the odds of homogamy in the stock. Of these flows, remarriage has the largest impact, contributing to about a 6% reduction in the log odds. The impact of later marital dissolutions and educational upgrades are both quite small (<1%) and negative.⁷ Again, the 95% confidence intervals suggest that we cannot be highly confident in the direction of these impacts (except perhaps for the negative impact of remarriage). Nevertheless, what is clear is that the effects of dissolutions, remarriages, and educational upgrades are small relative to the effects of first marriages.

Newlyweds Versus Prevailing Marriages

Samples of newlyweds in their first marriages are often viewed as the gold standard in assortative mating research, but researchers who do not have information on newlyweds or who are interested in overall patterns of resemblance use data on prevailing marriages, either using a wide age range of couples (e.g., Schwartz and Mare 2005; Smits et al. 1998; Torche 2010) or younger couples—for example, those 20 to 29 years old (Blackwell and Lichter 2000; Qian 1997; Rosenfeld 2008). The methods developed here can be used to assess by how much and why homogamy among new first marriages differs from homogamy among prevailing marriages.

Panel A of Table 3 decomposes the difference in the log odds of educational homogamy among prevailing and new first marriages in which wives are aged 18 to 41 into parts due to (a) age patterns of homogamy into new first marriages and age patterns of entry into first marriages, and (b) marital dissolutions, remarriages, and educational upgrades. The first row shows the observed difference in the log odds among prevailing and new first marriages using multistate life table data. These results indicate that researchers who use samples of prevailing marriages across a wide age range to make inferences about sorting into first marriages overestimate the odds of homogamy by about 15%. Our confidence interval for this estimate suggests we can be relatively confident that the odds of homogamy in prevailing marriages are higher than those among new first marriages for wives in this age range. Row 2 of Panel A shows that if first marriages were the only flow to have affected the stock of marriages, we would overestimate the odds of homogamy among new first marriages by an even larger amount—by 21%. The point estimates indicate that changes occurring after first marriages actually reduce the difference in homogamy between new first marriages and prevailing marriages (Row 3). In terms of the percentage contributions, age patterns of homogamy and entry into first marriage are responsible for all the higher odds of homogamy among prevailing marriages than among newlyweds (137%).

Table 3.

Decomposition of the difference in the log odds of educational homogamy in prevailing and new first marriages

	Difference in Log Odds	Odds Ratio	95% CI for Odds Ratioa	Percentage Contribution (%)b
Panel A. Wives Aged 18 to 41
(1) Total Difference in Log Odds
Observed Prevailing Marriages – 1.007 – 0.868 = 0.139  Observed New First Marriages		1.15	[1.04, 1.26]	—
(2) Portion Due to Age Patterns of Homogamy and First Marriage
First Marriages (S1) – 1.058 – 0.868 = 0.190  Observed New First Marriages		1.21	[1.08, 1.34]	137.1
(3) Portion Due to Marital Dissolutions, Remarriages, Educational Upgrades
Observed Prevailing Marriages – 1.007 – 1.058 = −0.052  First Marriages (S1)		0.95	[0.85, 1.05]	−37.1
Panel B. Wives Aged 18 to 29
(1) Total Difference in Log Odds
Observed Prevailing Marriages – 1.006 – 0.885 = 0.121  Observed New First Marriages		1.13	[1.00, 1.26]	—
(2) Portion Due to Age Patterns of Homogamy and First Marriage
First Marriages (S1) – 1.014 – 0.885 = 0.129  Observed New First Marriages		1.14	[0.97, 1.30]	106.6
(3) Portion Due to Marital Dissolutions, Remarriages, Educational Upgrades
Observed Prevailing Marriages – 1.006 – 1.014 = −0.008  First Marriages (S1)		0.99	[0.89, 1.09]	−6.6

Note: Data are weighted using 1979 sampling weights.

Source: National Longitudinal Survey of Youth (NLSY79), 1979–2002.

^a95% confidence intervals (CIs) are estimated using 1,000 bootstrapped samples.

^bEstimated using the difference in log odds.

Does restricting the sample to young couples reduce the discrepancy between the odds of homogamy among prevailing and new first marriages? Panel B of Table 3 performs this exercise for couples in which wives are aged 18 to 29, an age range similar to that used by previous research lacking information on newlyweds. Row 1 of Panel B shows that restricting the sample to relatively young couples does not substantially reduce the discrepancy: the odds of homogamy in prevailing marriages are about 13% higher than among new first marriages, compared with 15% for the wider age range. Again, age patterns of sorting into first marriages and entry into first marriage are responsible for this difference, rather than marital dissolutions, remarriages, and educational upgrades, but the 95% confidence intervals indicate that we can be less confident in the direction of our point estimates for young wives.

Discussion

Despite the high incidence of divorce, remarriage, and continued schooling after marriage in the United States, we find that these factors have small and offsetting impacts on the odds of educational homogamy in prevailing marriages among a cohort of women aged 14 to 22 in 1979. Consistent with other work, our point estimates indicate that marital dissolutions slightly increase educational resemblance, remarriages decrease resemblance, and educational upgrades have very little impact (e.g., Clarkwest 2007; Jacobs and Furstenberg 1986; Weiss and Willis 1997). Our contribution has been to show how these components work together to determine the observed odds of homogamy in prevailing marriages.

Our primary finding is that the odds of homogamy in prevailing marriages are overwhelmingly attributable to new first marriages. This suggests that the key to understanding between-cohort trends in educational homogamy in the United States lies in changes in assortative mating into first marriage, rather than changes in divorce, remarriage, or continued schooling after marriage, but research using data on more than one cohort is needed to fully investigate this claim. Although the odds of homogamy in prevailing marriages in this cohort are due mainly to new first marriages, this does not mean that samples of newlyweds and prevailing marriages are interchangeable. We find that the odds of homogamy among prevailing marriages in which wives are aged 18 to 41 are 15% higher than the odds of homogamy among newlyweds in the same age range. Restricting the sample to younger couples does not substantially increase the comparability of the two samples, although our point estimates are not as precise for this sample. Contrary to previous speculations (Kalmijn 1991a; Schwartz and Mare 2005), differences in homogamy between these samples do not appear to reflect selective marital dissolution, remarriage, and continued schooling, at least in this cohort. Rather, they are a product of the accumulation of homogamous first marriages in the stock of marriages.

This accumulation occurs because of age patterns of homogamy into first marriages and age patterns of entry into marriage. We find that age patterns of homogamy among new first marriages follow an inverted-U pattern, with lower odds of homogamy among younger and older wives. Because relatively few new first marriages occurred in the downward portion of the trend (after age 30) and many homogamous marriages had already accumulated in the stock of marriages, the downward trend among newlyweds has little effect on the odds of homogamy in prevailing marriages. By contrast, because every new first marriage is weighted equally in the cross section, the odds of homogamy among newlyweds are lower than among prevailing marriages. These findings have (non-)implications for our interpretation of the social distance between educational groups across samples. The higher odds of homogamy among prevailing marriages than among newlyweds do not reflect meaningful differences in the social distance between educational groups; they are almost entirely a product of entry into first marriages.

We can use our understanding of the relationship between age patterns of homogamy into new first marriages and age patterns of first marriage to think about the implications of shifts in these patterns for future trends in educational homogamy. Suppose that the inverted-U shape of the odds of homogamy among first-married newlyweds has remained constant over time. Then an increase in the average age at marriage for women from 22 to 27—which was roughly the shift from 1970 to 1995 (Schoen and Standish 2001)—would result in an increase in homogamy among prevailing marriages. If the average age at marriage increases beyond age 30, then the odds of homogamy in prevailing marriages will begin to decline. At present, the average age at first marriage is moving toward the downward slope of the inverted U. This suggests that spouses’ educational resemblance may decrease among cohorts to come if the shape of age patterns of homogamy remains stable.

A more likely scenario is that the shape of the inverted U is not constant over time, but is affected by changes in marriage timing. This could occur if the number of eligible partners of a given age affects the odds of homogamy. For example, if the observed odds of homogamy decline after women are older than about 30 because the pool of eligible mates is increasingly sparse (Lichter 1990), we would expect that as the number of eligible mates increase at older ages, so would the odds of homogamy. This would shift the peak of the inverted U to the right. Future research could examine how shifts in age patterns of homogamy and marriage timing have affected past trends in spousal resemblance and simulate how different changes may affect future trends.

Although their impacts are small, selective marital dissolutions, remarriages, and educational upgrades may affect comparisons between groups or countries where differences in homogamy are also relatively small. For example, differences in educational homogamy between cohabitors and married couples are modest, and marital dissolution has been found to play a role in accentuating these differences (Schwartz 2010a). Differences in the educational resemblance of spouses across countries tend to be large (e.g., Smits and Park 2009; Smits et al. 1998; Torche 2010); thus, if the effects of the proximate determinants in other countries are similar to those in the United States, many cross-national comparisons will not be affected. Of course, the impact of these demographic factors may vary considerably from context to context. For instance, divorce is more prevalent in the United States than in most other countries (OECD 2008), suggesting that the effects of divorce on homogamy should be larger in the United States than elsewhere. On the other hand, if divorce is more selective of heterogamous couples in other countries, then its effects on homogamy could be just as large.

The lesson we draw from this analysis is that researchers of assortative mating should exercise caution when drawing conclusions about the mechanisms that generate differences in spousal resemblance on the basis of data on prevailing marriages. We have shown that the proximate determinants of educational homogamy work in relatively complex ways using data from one cohort of American women. Future research should examine how these factors vary across times and places, and how variation in the indirect determinants of educational homogamy—that is, variation in broad social and economic factors—affects not only sorting into first marriages but also selective marital dissolution, remarriage, and continued schooling after marriage.

Appendix

Table 4.

Transition measures and sample sizes

Measure	Definition	n
New First Marriage	Respondent transitions from single (never married) to married  between interview years separated by no more than three years.	3,058
First Marital Dissolution	Respondent transitions from first marriage to separation, divorce,  or widowhood between interview years separated by no more  than three years.a	1,664
New Remarriage	Respondent transitions from separated, divorced, or widowed to  married or reunited between interview years separated by no more  than three years.b	1,186
Later Marital Dissolution	Respondent transitions from remarriage to separation, divorce,  or widowhood between interview years separated by no more  than three years.a	555
Educational Upgrade	Either partner in a marriage or remarriage increases their  education category between interview years separated by no  more than three years.	1,160

Note: Female respondents are aged 16 to 41.

Source: National Longitudinal Survey of Youth (NLSY79), 1979–2000.

^a3% of first marital dissolutions and 2% of later marital dissolutions were attributable to widowhood.

^b18% of new remarriages were reunions with a former spouse following a separation or divorce.