Skip to main content
PMC home page
Proceedings of the Royal Society B: Biological Sciences logoLink to Proceedings of the Royal Society B: Biological Sciences
. 2014 Jun 7;281(1784):20140149. doi: 10.1098/rspb.2014.0149

Physiological regulation of evaporative water loss in endotherms: is the little red kaluta (Dasykaluta rosamondae) an exception or the rule?

Philip C Withers 1,2,✉, Christine E Cooper 1,2
PMCID: PMC4043090  PMID: 24741015

Abstract

It is a central paradigm of comparative physiology that the effect of humidity on evaporative water loss (EWL) is determined for most mammals and birds, in and below thermoneutrality, essentially by physics and is not under physiological regulation. Fick's law predicts that EWL should be inversely proportional to ambient relative humidity (RH) and linearly proportional to the water vapour pressure deficit (Δwvp) between animal and air. However, we show here for a small dasyurid marsupial, the little kaluta (Dasykaluta rosamondae), that EWL is essentially independent of RH (and Δwvp) at low RH (as are metabolic rate and thermal conductance). These results suggest regulation of a constant EWL independent of RH, a hitherto unappreciated capacity of endothermic vertebrates. Independence of EWL from RH conserves water and heat at low RH, and avoids physiological adjustments to changes in evaporative heat loss such as thermoregulation. Re-evaluation of previously published data for mammals and birds suggests that a lesser dependence of EWL on RH is observed more commonly than previously thought, suggesting that physiological independence of EWL of RH is not just an unusual capacity of a few species, such as the little kaluta, but a more general capability of many mammals and birds.

Keywords: evaporative water loss, relative humidity, water vapour pressure deficit, ambient temperature, mammal

1. Introduction

Understanding the effects of relative humidity (RH) on physiological parameters, particularly evaporative water loss (EWL), is important because of the theoretical effect of RH on EWL and consequent effects on other physiological variables (e.g. body temperature, Tb; metabolic rate, MR and thermal conductance, C), especially for mammals and birds. It is a central paradigm of comparative physiology, for mammals and birds at Ta in and below thermoneutrality and over the short-term (i.e. hours), that EWL is determined essentially by physics [1–3], and not by physiological regulation, although EWL is often actively enhanced for thermoregulation at high ambient temperature (Ta) and can be modified in the longer term by development and acclimatization.

Based on Fick's law of diffusion [1–3], EWL depends on the difference in water vapour concentration (χ) between the body surface (χsat; assumed to be saturated, RH = 100%) and ambient air (χo) i.e. EWL α (χsat − χo). Ambient water vapour concentration depends on RH and χsat; χo = (RH/100)χsat) EWL and χsat are expected to increase exponentially with Ta (°K), e.g. χsat = 9.16 108 e−(5218/Ta) [4]. Water vapour pressure (wvp) is an equivalent measure of water potential to χ [1], so EWL should be inversely and linearly related to ambient wvp and positively linearly related to water vapour pressure deficit (Δwvp = wvpsat − wvpo). Various studies have reported that EWL changes inversely with RH and wvp, or linearly with Δwvp, for various small endotherms at and below thermoneutrality [5–13], as expected from physical principles. However, a few studies have reported a lower or even opposite relationship between EWL and RH, wvp or Δwvp [12–14]. This raises the important question of whether these endotherms have physiological regulation of EWL at low and moderate Ta, which could confer substantial advantages for balancing their water budget. However, complexities with these studies, such as use of RH, wvp or Δwvp, heterothermy [13], postural adjustments [14], measurement method and protocols [13,15], and use of skin or ambient temperature to calculate Δwvp [16] make it difficult to interpret these patterns.

Here, we measure the effect of varying ambient Ta and RH on hygric, metabolic and thermal physiology for the little red kaluta (Dasykaluta rosamondae). This small (35 g) dasyurid marsupial has several physiological characteristics of arid-habitat mammals, including a low and thermolabile Tb, low basal metabolic rate and EWL, high point of relative water economy and ready use of torpor [17]. We might expect the EWL of little red kaluta to deviate from a theoretical linear relationship with Δwvp, if such deviation has adaptive value such as water conservation. We explore the effects of RH and Δwvp on EWL for the kaluta and other small endotherms in comparison with biophysically predicted expectations, then we consider the implications of effects of RH on EWL for thermal and metabolic physiology.

2. Material and methods

(a). Study animals

Eight little red kalutas (seven males, one female) were collected in northern Western Australia [see 17]. They were housed individually at Ta approximately 20°C, with a 12 L : 12 D cycle, and were fed mince, tinned cat food and mealworms, with ad libitum water.

(b). Respirometry

MR (oxygen consumption, VO2; carbon dioxide production, VCO2) and EWL were measured by flow-through respirometry [18]. An Aalborg GFC17 mass flow controller regulated compressed air flow through a 265 ml chamber at 350 ml min−1, inside a temperature-controlled room at a Ta of 25°C, 30°C and 35°C. RH was controlled at each Ta (approx. 17%, 36%, 56% and 78% RH) by saturating inlet air at known temperatures, using a Lauda K-2/R refrigerated water bath, then warming to Ta; RH was calculated from saturation wvp at the water bath temperature and Ta, using standard equations [19]. Excurrent RH and Ta were measured with a Vaisala HMP45A probe, and a subsample was drawn through a column of drierite then a Servomex OA174 or A184 O2 analyser and a Hereus–Leybold Binos or Hartmann and Braun Uras 10E CO2 analyser. Analysers were interfaced to a PC via digital multimeters and serial ports. Excurrent O2, CO2, RH and Ta were recorded every 20 s throughout the experiment, using custom-written software (Visual Basic v. 6). Tb was measured immediately at the end of each experiment using a RadioSpares 611–234 thermocouple meter, with a plastic-tipped thermocouple inserted approximately 2 cm into the cloaca.

The O2 analysers were two-point calibrated with compressed nitrogen (BOC Gases) and dry ambient air (20.95%). The CO2 analysers were calibrated with compressed N2 and a certified gas mix (0.53% CO2; BOC Gases). The RH probes were calibrated over a wide range (using air saturated at a known temperature then warmed to ambient Ta as described above), and calibration was routinely confirmed using 1% RH air (dried with Drierite) and 100% RH air (saturated; by breathing on the RH probe). Flow meters were calibrated using a Sensidyne Gilibrator 2.

Kalutas were fasted for 24 h before commencement of experiments, then measured for no less than 6 h at each Ta and RH combination (measured in random order) until O2, CO2 and RH were stable and minimal for at least 20 min (see [15]). VO2, VCO2 and EWL were calculated after [18] using a custom-written analysis program (Visual Basic v. 6). Respiratory exchange ratio (RER) was calculated as VCO2/VO2. Metabolic heat production (MHP) was calculated from MR using the measured RER after [2], and evaporative heat loss (EHL) was calculated from EWL using 2.4 J mg−1 H2O [3]. Wet thermal conductance (Cwet; J g−1 h−1 °C−1) was calculated as MR/(Tb − Ta) and dry thermal conductance (Cdry; J g−1 h−1 °C−1) as (MHP – EHL)/(Tb − Ta), for (Tb − Ta) > 1°C.

EWL was also corrected for Δwvp, which was calculated as the difference between saturation wvp and ambient wvp at the measured RH and Ta. It is customary to calculate Δwvp as the difference between wvp at 100% RH and ambient Ta and wvp at ambient RH and Ta [7,14,20]. Skin temperature is more appropriate than Ta to calculate Δwvp [16], but its measurement is more difficult, so ambient temperature is generally used as a proxy. The Δwvp will be similar, particularly at low ambient wvp, unless there is a very large difference between Ta and Tskin.

(c). Statistics

Values are presented as mean ± standard error, with N the number of individuals and n the number of measurements. Multivariate repeated measures ANOVA (mRMANOVA) with two levels of within-individual repeat (Ta and RH) and a priori polynomial contrasts were used to analyse the repeated measurements of individuals. Statistical analyses were conducted using a custom-written Excel spread sheet [21] and SPSS (v. 21 for Windows).

3. Results

Mean body mass of kalutas was 33 ± 0.6 g over all experiments (N = 8, n = 84). Kalutas rested quietly in the chamber, at all Ta and RH combinations. There was no significant difference in body mass with either Ta or RH treatments.

(a). Effects of Ta and relative humidity on evaporative water loss

There was a significant effect of Ta on EWL (F2,5 = 20.5, p = 0.004), but no significant overall effect of RH (F3,4 = 6.03; p = 0.058) by mRMANOVA (figure 1). However, a significant quadratic contrast for EWL (p = 0.045) over all RH treatments, combined with a very insignificant mRMANOVA effect (F2,3 = 0.059; p = 0.943), and no significant polynomial contrasts (p > 0.678) for the three lowest RH treatments (RH = 78% removed from the model) indicate that EWL was significantly lower at the highest RH but did not differ between the three lower RHs. The insignificant interaction between Ta and RH (F6,1 = 5.23; p = 0.317) suggests the patterns in EWL with RH were consistent for each Ta.

Figure 1.

Figure 1.

Open in a new tab

Evaporative water loss (EWL) of little red kalutas (Dasykaluta rosamondae), over a range of ambient relative humidities (RHs), measured at ambient temperatures of 25°C, 30°C and 35°C. Values are mean ± s.e., N = 8.

When EWL was expressed per Δwvp (EWL/Δwvp; figure 2), there was a significant effect of Ta (F2,5 = 15.5; p = 0.007) and RH (F3,4 = 10.5; p = 0.023) by mRMANOVA. A significant contrast (p = 0.012) indicated that EWL/Δwvp was significantly higher at elevated RH. A significant Ta×RH interaction term (p = 0.011) suggests that the EWL/Δwvp pattern with RH was different for the three Tas. EWL/Δwvp values for kalutas at low RH, from Ta = 11–38°C [17] are included in figure 2.

Figure 2.

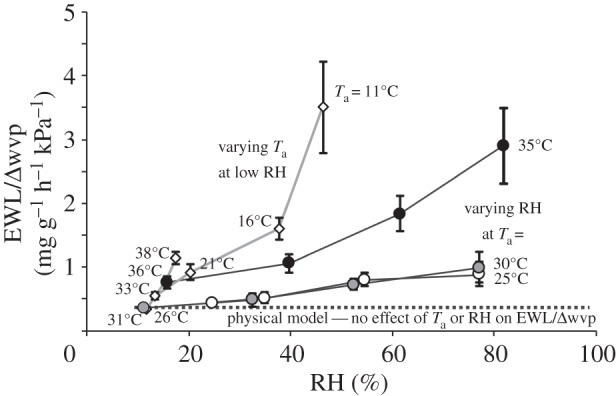
Figure 2.

Open in a new tab

Evaporative water loss (EWL) relative to water vapour pressure deficit, of little red kalutas (Dasykaluta rosamondae), over a range of ambient humidities (RHs) and different ambient temperatures (Ta; values in figure). Values from this study (circles) are for four RH at three Ta; values from Withers & Cooper [17] (diamonds) are for Ta from 11°C to 38°C, at low RH. A simple physical model of evaporation predicts no effect of Ta or RH on EWL/Δwvp (see text).

(b). Effects of Ta and relative humidity on other physiological variables

There were significant Ta effects for Tb (F2,5 = 93.3, p < 0.001), VO2 and VCO2 (F2,5 ≥ 33.4, p ≤ 0.007) and Cwet and Cdry (F1,6 ≥ 13.3, p ≤ 0.011). We did not determine Cwet or Cdry at Ta = 35°C, because (Tb − Ta) was less than 1. There were no overall RH effects for Tb (F3,4 = 2.50, p = 0.198), VO2 and VCO2 (F3,4 ≤ 3.39, p ≥ 0.134) or Cwet and Cdry (F3,4 ≤ 2.34; p ≥ 0.215). For all RH data, there was a weak linear effect (p = 0.020) of RH on Tb, with higher Tb at the higher RH. There was no significant effect of RH on Tb (p = 0.173) when the highest RH data were excluded, indicating that there was no effect of RH on Tb, except at the highest RH where Tb increased slightly.

4. Discussion

Previous studies have shown that EWL changes with Ta, RH and Δwvp in a complex manner [5–14]. Our findings for kalutas of considerable independence of EWL from RH, suggest physiological control. Ta influences on EWL also differ from biophysical predictions. Furthermore, re-examination of effects of RH and Δwvp on EWL data from previous studies suggests that our results for kalutas are not unusual among small endotherms. Maintaining constancy of EWL at low RH not only conserves water, but also affects thermal and metabolic physiology.

(a). Effect of Ta on evaporative water loss

Above the thermoneutral zone (TNZ), EWL of endotherms typically increases more rapidly than predicted from Ta alone, reflecting augmented EHL for thermoregulation when Tb > Ta [2,3,22,23]. This physiological response is also apparent for kalutas; at Ta above thermoneutrality, EWL is enhanced, with a successively higher EWL/Δwvp between 31°C and 38°C [17, fig. 2] and a higher EWL/Δwvp at 35°C compared with 25°C and 30°C (this study; figure 2).

At Ta below TNZ, EWL of endotherms is often constant or even decreases with increasing Ta [24,25], rather than increasing exponentially with Ta as would be predicted (by the physical effect). Kaluta are no exception. EWL is relatively constant at Ta in and below thermoneutrality (30°C; [17]), so EWL/Δwvp decreases as Ta increases from 11°C to 31°C (figure 2); this pattern differs from the physical model that EWL is proportional to Δwvp. This non-exponential effect of Ta on EWL below thermoneutrality is generally attributed to the counteracting effects of Ta on respiratory and cutaneous EWL. Thermoregulatory adjustments in MR increase respiratory ventilation and EWL at low Ta, which counterbalances the expected decrease in cutaneous EWL [24,25]. In the light of our findings for EWL constancy at low RH, an alternative interpretation of this EWL constancy at low Ta is that EWL is acutely regulated over this Ta range. Whatever the explanation for this EWL constancy below the TNZ, marked deviations in EWL from the simple physical effects of Ta indicate some form of physiological regulation, for thermoregulation via proportionally increased MHP or water conservation by direct regulation of EWL.

(b). Effect of relative humidity on evaporative water loss

Just as deviations of EWL from the physical effects of Ta reflect regulatory processes, comparison of EWL responses with variation in RH at a constant Ta with a biophysical model also provides evidence of physiological regulation of EWL. Various studies have reported that EWL changes inversely with RH and wvp (or linearly with Δwvp) for small endotherms [5–13], consistent with a simple physical model. However, some studies have reported a lower or no relationship between EWL and RH or Δwvp, generally at low or moderate Ta [12–14]. EWL is independent of wvp at 20°C for rock pigeons; adjustments in respiratory ventilation or expired air temperature might account for this [12]. For heterothermic male and post-lactating female little brown bats, there was no wvp effect on EWL at Ta of 28°C or 33°C [13], suggesting that EWL is a controlled rather than a physical process, and there was an unexpected linear relationship between EWL and wvp at Ta = 37°C. We [14] previously reported that EWL of brushtail possums was constant at low RH, at a thermoneutral Ta (25°C); we attributed this EWL constancy at low RH to postural changes and a body core to extremity thermal gradient. Our data for kalutas, showing an unexpected constancy of EWL under environmental conditions that would be expected to perturb water balance, suggest that EWL was under active physiological regulation, reducing their EWL at low RH (high Δwvp).

Only these few species have been reported to deviate from the expected physical EWL–RH–Δwvp model, whereas most others apparently conform to it. However, we have re-analysed data from other studies examining the effect of RH, wvp or Δwvp, on EWL to evaluate how well those data fit the expectation of a linear physical effect of Δwvp on EWL. We found that the expected linear relationship between Δwvp and EWL is less universal than first thought.

(c). Biophysical model for evaporative water loss and relative humidity

We use here a simple conceptual framework for the biophysical relationship between EWL and Δwvp (at Tas at or below 35°C) to assess how EWL data from published studies conform to this model. According to the simple Fickian model for diffusion, we would expect a positive linear relationship between EWL and Δwvp that passes through the origin (no EWL when there is no Δwvp). To compare data from these disparate studies, we normalized the data, so that EWL at the lowest Δwvp would fit on an arbitrary ‘relative EWL’ line; data from higher Δwvp should then also fit on the line if the relationship was Fickian. We found that results from some studies conform to the Fickian model, but many results do not (figure 3). In particular, for many species, the relative EWL decreases substantially below the expected linear relationship at high Δwvp. The potential significance of these deviations from the simple Fickian model has not previously been evaluated in a conceptual framework and was often not even recognized in the original studies. Our re-evaluation of previous data for mammals and birds shows that a lesser dependence of EWL on Δwvp is more common than previously thought. Our interpretation is that physiological independence of EWL of Δwvp is not just an unusual capacity of a few species, such as the little kaluta, but is a more general capability of many mammals and birds.

Figure 3.

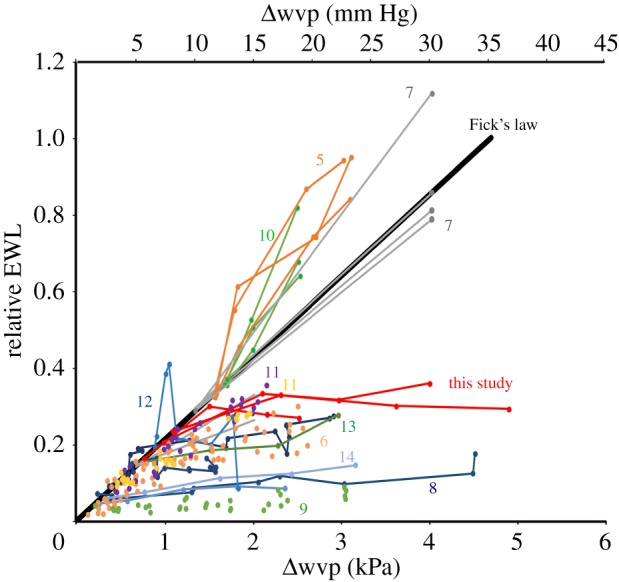
Figure 3.

Open in a new tab

Conceptual model (thick black line) for the expected physical relationship between evaporative water loss (EWL) and water vapour pressure deficit (Δwvp), based on diffusion (Fick's law), compared with the relative EWL for various endotherms from the literature (numbers indicate source). The Δwvp is calculated as ((100 − ambient RH)/100)*saturation water vapour pressure at the ambient temperature. EWL is scaled for each species, so that the EWL for the lowest measured Δwvp fits on the expected physical model line. Data that fall below the expected physical line are consistent with a regulated decrease in EWL (i.e. water conservation), whereas data that fall above the expected physical line are consistent with an increased EWL at higher Δwvp (i.e. enhanced water loss).

Decreasing curvilinear relationships for EWL–Δwvp are of particular interest, because they suggest physiological regulation of EWL at low RH. That EWL is plastic and under physiological control over periods of weeks to months in response to developmental and/or acclimatory changes in water requirements has been demonstrated for birds and mammals [26–29]. However, active regulation of EWL over a period of only 6 h has not, to our knowledge, been previously considered. Although mechanisms that acutely enhance EWL for thermoregulatory purposes at high Ta or high RH are well documented, e.g. salivation, sweating, panting [2–3], mechanisms that could acutely modify EWL in and below thermoneutrality are not. Potential mechanisms for decreasing EWL at high Δwvp will depend on the relative partitioning of cutaneous and respiratory avenues of EWL, and may include decreased cutaneous EWL, e.g. by modification of skin lipids [30–32], and/or reduced respiratory EWL, e.g. by lowered expired air temperature by nasal counter-current heat and water exchange [25,33–36].

We propose that our description of acute deviation of EWL from the expected physical model for EWL at moderate and low Ta is evidence for acute physiological regulation, e.g. over a few hours. This EWL regulation at low RH is presumably an adaptation to conserve body water when EHL is not required for thermoregulation. This probably has considerable adaptive significance, particularly for species with limited access to free water such as the arid-habitat kaluta. A conservative estimate of the water savings for kalutas (calculated by assuming a linear relationship between EWL values at the highest RH, and EWL = 0 at RH = 100%, and extrapolating this relationship to the lowest measured RH then subtracting the observed EWL) indicates that the EWL of kalutas at about 20% RH is only about 40–50% of that predicted if EWL increased as expected biophysically, saving the kaluta at least 1.0–4.6 mg H2O g−1 h−1, depending on Ta.

(d). Other physiological implications of evaporative water loss–relative humidity effects

There is a paucity of data for endotherms that examine the effects of RH on other physiological variables that impact homeostatic thermoregulation, such as Tb, MR and C. If RH had the expected biophysical effect on EWL, then we would expect compensatory thermoregulatory changes in MR, or impacts of changes in EHL on Tb. For example, wvp may affect Tb for kangaroo rats, particularly at high Ta [37]. For brushtail possums [14], there was a significant RH effect on MR at Ta = 30°C, sufficient to maintain a constant Tb when EWL (and thus EHL) was reduced at higher RH. However, RH does not affect Tb or MR for some rodents [6,7,38]. For kalutas, Tb increased significantly only at the highest RH, where EWL (and thus EHL) were significantly reduced; at lower RH, EWL as well as Tb, MR and C were independent of RH. Kalutas did not adjust MR or C to maintain constant Tb at the high RH, reflecting their general thermolability [17].

This observation highlights another important consequence of EWL constancy with varying RH/wvp/Δwvp. Constancy of EWL avoids thermoregulatory impacts that would occur as a result of changing EHL. Constant EWL (and thus EHL) at differing RH keeps Tb constant without the need for compensatory changes in MR and/or C.

Acknowledgements

We thank Graham and Scott Thompson, and Phil Runham, for providing us with the kalutas. We thank Shane Maloney for loan of the Hartmann and Braun Uras 10E carbon dioxide analyser.

Experimental work was approved by the Animal Ethics Committee of the University of Western Australia, and animals were held under licence from the West Australian Department of Environment and Conservation.

Data accessibility

Raw data are provided online as electronic supplementary material.

Funding statement

This study was supported by an Australian Research Council Discovery Grant to C.E.C. and P.C.W.

References

  • 1.Monteith JL, Campbell GS. 1980. Diffusion of water vapour through integuments – potential confusion. J. Therm. Biol. 5, 7–9. ( 10.1016/0306-4565(80)90033-9) [DOI] [Google Scholar]
  • 2.Withers PC. 1992. Comparative animal physiology. Philadelphia, PA: Saunders College Publishing. [Google Scholar]
  • 3.McNab BK. 2002. The physiological ecology of vertebrates. Ithaca, NY: Cornell University Press. [Google Scholar]
  • 4.Daniels F, Alberty RA. 1975. Physical chemistry. New York, NY: Wiley. [Google Scholar]
  • 5.Chew RM, Dammann AE. 1961. Evaporative water loss of small vertebrates, as measured with an infrared analyser. Science 133, 384–385. ( 10.1126/science.133.3450.384) [DOI] [PubMed] [Google Scholar]
  • 6.Baudinette RV. 1972. Energy metabolism and evaporative water loss in the California ground squirrel. Effects of burrow temperature and water vapour pressure. J. Comp. Physiol. 81, 57–72. ( 10.1007/BF00693550) [DOI] [Google Scholar]
  • 7.Edwards RM, Haines H. 1978. Effects of ambient water vapour pressure and temperature on evaporative water loss in Peromyscus maniculatus and Mus musculus. J. Comp. Physiol. 128, 177–184. ( 10.1007/BF00689482) [DOI] [Google Scholar]
  • 8.Lasiewski RC, Acosta AL, Bernstein MH. 1966. Evaporative water loss in birds – I. Characteristics of the open flow method of determination, and their relation to estimates of thermoregulatory ability. Comp. Biochem. Physiol. 19, 445–457. ( 10.1016/0010-406X(66)90153-8) [DOI] [Google Scholar]
  • 9.Lasiewski RC, Weathers WW, Bernstein MH. 1967. Physiological responses of the giant hummingbird, Patagonia gigas. Comp. Biochem. Physiol. 23, 797–813. ( 10.1016/0010-406X(67)90342-8) [DOI] [PubMed] [Google Scholar]
  • 10.Christian DP. 1978. Effects of humidity and body size on evaporative water loss in three desert rodents. Comp. Biochem. Physiol. A 60, 425–430. ( 10.1016/0300-9629(78)90011-7) [DOI] [Google Scholar]
  • 11.Webb PI, Speakman JR, Racey PA. 1995. Evaporative water loss in two sympatric species of vespertilionid bat, Plecotus auritus and Myotis daubentoni: relation to foraging mode and implications for roost site selection. J. Zool. 235, 269–278. ( 10.1111/j.1469-7998.1995.tb05143.x) [DOI] [Google Scholar]
  • 12.Bernstein MH, Hudson DM, Stearns JM, Hoyt RW. 1977. Measurement of evaporative water loss in small animals by dew-point hygrometry. J. Appl. Physiol. 43, 382–385. [DOI] [PubMed] [Google Scholar]
  • 13.Procter JW, Studier EH. 1970. Effects of ambient temperature and water vapor pressure on evaporative water loss in Myotis lucifugus. J. Mammal 51, 799–804. ( 10.2307/1378307) [DOI] [Google Scholar]
  • 14.Cooper CE, Withers PC. 2008. Allometry of evaporative water loss in marsupials: implications of the effect of ambient relative humidity on the physiology of brushtail possums (Trichosurus vulpecula). J. Exp. Biol. 211, 2759–2766. ( 10.1242/jeb.019463) [DOI] [PubMed] [Google Scholar]
  • 15.Cooper CE, Withers PC. 2009. Effects of measurement duration on the determination of basal metabolic rate and evaporative water loss of small marsupials: how long is long enough? Physiol. Biochem. Zool. 82, 438–446. ( 10.1086/603654) [DOI] [PubMed] [Google Scholar]
  • 16.Lillywhite HB, Menon JG, Menon GK, Sheehy CM, Tu MC. 2007. Water exchange and permeability properties of the skin in three species of amphibious sea snakes (Laticauda spp.). J. Exp. Biol. 212, 1921–1929. ( 10.1242/jeb.028704) [DOI] [PubMed] [Google Scholar]
  • 17.Withers PC, Cooper CE. 2009. Thermal, metabolic, and hygric physiology of the little red kaluta, Dasykaluta rosamondae (Dasyuromorphia: Dasyuridae). J. Mammal 90, 752–760. ( 10.1644/08-MAMM-A-286R.1) [DOI] [Google Scholar]
  • 18.Withers PC. 2000. Design, calibration and calculation for flow-through respirometry systems. Aust. J. Zool. 49, 445–461. ( 10.1071/ZO00057) [DOI] [Google Scholar]
  • 19.Parish OO, Putnam TW. 1977. Equations for the determination of humidity from dewpoint and psychrometric data. NASA Technical Note D-8401. Dryden Flight Research Centre, CA: NASA. [Google Scholar]
  • 20.Coulombe HN. 1970. Physiological and physical aspects of temperature regulation in the burrowing owl Speotyto cunicularia. Comp. Biochem. Physiol. 35, 307–337. ( 10.1016/0010-406X(70)90599-2) [DOI] [Google Scholar]
  • 21.Withers PC, Cooper CE. 2011. Using a priori contrasts for multivariate repeated-measures ANOVA to analyse thermoregulatory responses of the dibbler (Parantechinus apicalis; Marsupialia, Dasyuridae). Physiol. Biochem. Zool. 84, 514–521. ( 10.1086/661637) [DOI] [PubMed] [Google Scholar]
  • 22.Moritmoto T. 1998. Heat loss mechanisms. In Physiology and pathophysiology of temperature regulation (ed. Blatteis CM.), pp. 80–90. Singapore: World Scientific. [Google Scholar]
  • 23.Tattersall GJ, Sinclair BJ, Withers PC, Fields PA, Seebacher F, Cooper CE, Maloney SK. 2012. Coping with thermal challenges: physiological adaptations to environmental temperatures. Comp. Physiol. 2, 2151–2202. [DOI] [PubMed] [Google Scholar]
  • 24.Withers PC, Cooper CE. 2009. Thermal, metabolic, hygric and ventilatory physiology of the sandhill dunnart (Sminthopsis psammophila; Marsupialia, Dasyuridae). Comp. Biochem. Physiol. 153, 317–323. ( 10.1016/j.cbpa.2009.03.006) [DOI] [PubMed] [Google Scholar]
  • 25.Withers PC, Cooper CE, Nespolo RF. 2012. Evaporative water loss, relative water economy and evaporative partitioning of a heterothermic marsupial, the monito del monte (Dromiciops gliroides). J. Exp. Biol. 215, 2806–2813. ( 10.1242/jeb.070433) [DOI] [PubMed] [Google Scholar]
  • 26.Tracey RL, Walsberg GE. 2001. Developmental and acclimatory contributions to water loss in a desert rodent: investigating the time course of adaptive change. J. Comp. Physiol. B 171, 669–679. ( 10.1007/s003600100218) [DOI] [PubMed] [Google Scholar]
  • 27.McKechnie AE, Wolf BO. 2004. Partitioning of evaporative water loss in white-winged doves: plasticity in response to short-term thermal acclimation. J. Exp. Biol. 207, 203–210. ( 10.1242/jeb.00757) [DOI] [PubMed] [Google Scholar]
  • 28.Muñoz-Garcia A, Williams JB. 2008. Developmental plasticity of cutaneous water loss and lipid composition in stratum corneum of desert and mesic nestling house sparrows. Proc. Natl Acad. Sci. USA 105, 15 611–15 616. ( 10.1073/pnas.0805793105) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 29.Muñoz-Garcia A, Cox RL, Williams JB. 2008. Phenotypic flexibility in cutaneous water loss and lipids of the stratum corneum in house sparrows (Passer domesticus) following acclimation to high and low humidity. Physiol. Biochem. Zool. 81, 87–96. ( 10.1086/522651) [DOI] [PubMed] [Google Scholar]
  • 30.Elias PM. 2004. Epidermal permeability barrier: from the early days at Harvard to emerging concepts. J. Invest. Dermatol. 122, xxxvi–xxxix. ( 10.1046/j.0022-202X.2004.22233.x) [DOI] [PubMed] [Google Scholar]
  • 31.Muñoz-Garcia A, Williams JB. 2005. Cutaneous water loss and lipids of the stratum corneum in house sparrows Passer domesticus from arid and mesic environments. J. Exp. Biol. 208, 3689–3700. ( 10.1242/jeb.01811) [DOI] [PubMed] [Google Scholar]
  • 32.Ro J, Williams JB. 2010. Respiratory and cutaneous water loss of temperate-zone passerine birds. Comp. Biochem. Physiol. A 156, 237–246. ( 10.1016/j.cbpa.2010.02.008) [DOI] [PubMed] [Google Scholar]
  • 33.Bartholomew GA. 1972. The water economy of seed-eating birds that survive without drinking. In Proceedings of the XVth International Ornithological Congress (ed. Voous KH.), pp. 237–254. Leiden, The Netherlands: Brill. [Google Scholar]
  • 34.Schmidt-Nielsen K, Hainsworth FR, Murrish DE. 1970. Counter-current heat exchange in the respiratory passages: effect on water and heat balance. Respir. Physiol. 9, 263–276. ( 10.1016/0034-5687(70)90075-7) [DOI] [PubMed] [Google Scholar]
  • 35.Collins JC, Pilkington TC, Schmidt-Nielsen K. 1971. A model of respiratory heat transfer in a small mammal. Biophys. J. 11, 886–914. ( 10.1016/S0006-3495(71)86262-8) [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 36.Geist NR. 2000. Nasal respiratory turbinate function in birds. Physiol. Biochem. Zool. 73, 581–589. ( 10.1086/317750) [DOI] [PubMed] [Google Scholar]
  • 37.Kay FR. 1975. Environmental physiology of the banner-tailed kangaroo rat. I. Influence of ambient temperature, humidity and carbon dioxide on body temperature. Comp. Biochem. Physiol. A 50, 483–488. ( 10.1016/0300-9629(75)90305-9) [DOI] [Google Scholar]
  • 38.Ewing WG, Studier EH. 1973. A method for control of water vapour pressure and its effects on metabolism and body temperature in Mus musculus. Comp. Biochem. Physiol. A 45, 121–125. ( 10.1016/0300-9629(73)90012-1) [DOI] [PubMed] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Data Availability Statement

Raw data are provided online as electronic supplementary material.

Articles from Proceedings of the Royal Society B: Biological Sciences are provided here courtesy of The Royal Society

RESOURCES