Skip to main content
PMC home page
Scientific Reports logoLink to Scientific Reports
. 2014 Jun 4;4:5165. doi: 10.1038/srep05165

Critical role of γ-phosphate in structural transition of Na,K-ATPase upon ATP binding

Irina Yu Petrushanko 1,3, Vladimir A Mitkevich 1,3, Anastasia A Anashkina 1, Elizaveta A Klimanova 2, Elena A Dergousova 2, Olga D Lopina 2, Alexander A Makarov 1,a
PMCID: PMC4044624  PMID: 24893715

Abstract

Active transport of sodium and potassium ions by Na,K-ATPase is accompanied by the enzyme conformational transition between E1 and E2 states. ATP and ADP bind to Na,K-ATPase in the E1 conformation with similar affinity but the properties of enzyme in complexes with these nucleotides are different. We have studied thermodynamics of Na,K-ATPase binding with adenine nucleotides at different temperatures using isothermal titration calorimetry. Our data indicate that β-phosphate is involved in complex formation by increasing the affinity of adenine nucleotides to Na,K-ATPase by an order of magnitude, while γ-phosphate does not affect it. ATP binding to Na,K-ATPase in contrast to ADP binding generates a structural transition in the enzyme, which is consistent with the movement of a significant portion of the surface area to a solvent-protected state. We propose that ATP binding leads to convergence of the nucleotide-binding and phosphorylation domains transferring the enzyme from the “E1-open” to “E1-closed” conformation ready for phosphorylation.

In all animal cells, active transport of sodium and potassium ions across the plasma membrane is facilitated by Na,K-ATPase (sodium pump, EC 3.6.3.9). The transport is followed by conformational transition between the two main states of the enzyme, E1 and E2, that bind three Na+ and two K+ ions, respectively1. Na,K-ATPase in the E1 conformation has a high affinity to ATP and Na+, while the enzyme in the E2 conformation has a low affinity to ATP and high affinity to K+ ions1. Functional monomer of the Na,K-ATPase consists of the catalytic α-subunit and β-subunit, the latter is required for routing of the α-subunit to the plasma membrane and for the K+ ions binding2,3. The α-subunit contains sites for the binding of nucleotide, Na+ and K+, and is responsible for the cation translocation as well as for the ATP hydrolysis. A major part of α-subunit is localised in the cytosol and consists of the large cytosolic loop forming the nucleotide binding (N) and phosphorylation (P) domains, and the small cytosolic loop, which together with the N-terminus of the molecule forms the actuator (A) domain. Transition from the E1 to E2 conformation is accompanied by formation of the phosphorylated intermediate: at first γ-phosphate of ATP bonded to the N domain is transferred to the carboxyl group of Asp369 located in the P domain, and then the acyl-phosphate bond is hydrolyzed1. Recently a crystal structure of the protein in intermediate [Na3]E1P-ADP state (pig renal α1β1γ enzyme) at 4.3 Å4 and 2.8 Å5 resolution has been solved. The structure of Na,K-ATPase in the E1 free state is absent and it remains unclear which conformational transitions the enzyme undergoes when passing from the free E1 state to the nucleotide-binding state.

ATP and ADP bind to Na,K-ATPase in the E1 conformation with similar affinity6,7, however ATP and ADP affect Na,K-ATPase in a different way. ATP was shown to protect the enzyme from inactivation by hydroxyl radicals and ADP does not produce such effect8. Structural organization of the free Na,K-ATPase and as complexes with ATP or ADP may be different. This assumption is supported by the fact that crystal structures of the Na,K-ATPase homolog – sarco (endo) plasmic reticulum Ca2+-ATPase (SERCA) in the Ca2+-bound E1 state9,10, and in complex with a nonhydrolyzable ATP analog - adenosine (β - γ methylene)-triphosphate11, differ from each other by the arrangement of N and A domains with respect to the P domain.

In this work, we have used isothermal titration calorimetry (ITC) to estimate the equilibrium constants along with the enthalpic and entropic components for the A-nucleotides (AMP, ADP and ATP) binding to Na,K-ATPase in the E1 state at different temperatures. Previously the Na,K-ATPase interactions with adenine nucleotides have been studied by ITC, at a single temperature value and the thermodynamic parameters of the enzyme binding with ATP were not reported6,12. Thus no conclusions could be made regarding conformational changes in the protein upon binding of nucleotides. In this study we have found that β-phosphate is involved in complex formation by increasing the affinity of nucleotides to Na,K-ATPase by an order of magnitude, while γ-phosphate does not affect it. Furthermore, we have demonstrated that ATP binding to Na,K-ATPase in contrast to the ADP binding generates a structural transition in the enzyme, which is compatible with the movement of molecular surface area (ranging from 4021 to 5891 Å2) from a solvent-exposed to solvent-protected state. Comparison of the ITC data with the structure modeling results for Na,K-ATPase in the E1 free state and in the ATP-bound state allowed us to conclude that this structural change is caused by the locking of the N domain of Na,K-ATPase to the P domain induced by the γ-phosphate of ATP, thereby reducing the exposed surface area of the protein.

Results

Affinities of Na,K-ATPase to adenine nucleotides

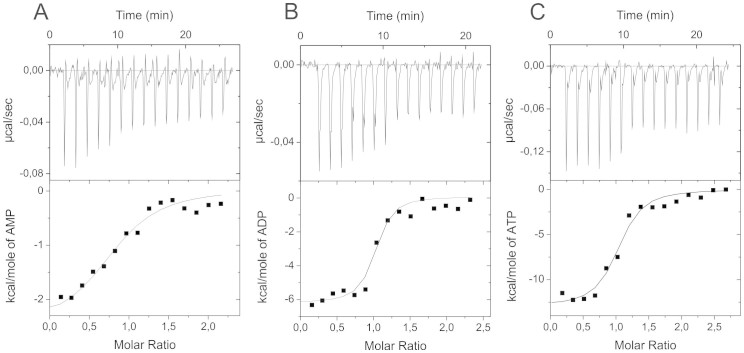
We used ITC to estimate the affinities (association (Ka) and dissociation (Kd) equilibrium constants) of AMP, ADP and ATP to duck ATPase at different temperatures (Table 1). It should be noted that the experimental conditions exclude ATP hydrolysis and formation of phosphorylated intermediate (see Methods). A typical set of ITC data for AMP, ADP and ATP binding to ATPase at 37°C is shown in Figure 1, including the “raw” calorimetric data for the ligand-into-protein titration (upper parts) and the binding curves (lower parts). The latter were fitted to a model with one adenine nucleotide binding site per ATPase molecule.

Table 1. Thermodynamic parameters of the duck Na,K-ATPase binding to AMP, ADP and ATP determined by isothermal titration calorimetrya.

Ligand T, °C Kab, M−1 Kdc, μM ΔHd, kcal/mole TΔSe, kcal/mole ΔGf, kcal/mole
AMP 25 1.1 × 106 0.91 −1.40 6.83 −8.23
AMP 30 1.8 × 106 0.56 −1.81 6.86 −8.67
AMP 37 1.7 × 106 0.59 −2.19 6.65 −8.84
ADP 25 1.4 × 107 0.07 −5.68 4.06 −9.74
ADP 30 2.4 × 107 0.04 −6.00 4.23 −10.23
ADP 37 2.6 × 107 0.04 −6.21 4.31 −10.52
ATP 10 1.2 × 107 0.08 7.70 16.87 −9.17
ATP 25 1.6 × 107 0.06 −4.00 5.82 −9.82
ATP 37 2.0 × 107 0.05 −12.80 −2.44 −10.36
Open in a new tab

aAll measurements were performed three to four times in imidazole buffer (25 mM imidazole, 1 mM DTT, 1 mM EDTA, 3 mM NaCl and 250 mM sucrose, pH 7.5).

bKa – affinity constant; standard deviation did not exceed ±20%.

cKd – dissociation constant; calculated as 1/Ka.

dΔH – enthalpy variation; standard deviation did not exceed ±10%.

eTΔS– entropy variation; calculated from the equation ΔG = ΔH − TΔS.

fΔG – Gibbs energy; calculated from the equation ΔG = −RTlnKa.

Figure 1. Na,K-ATPase interaction with adenine nucleotides measured by ITC.

Figure 1

Open in a new tab

Titration curves (upper panels) and binding isotherms (lower panels) for duck Na,K-ATPase interaction with AMP (A), ADP (B) and ATP (C) at 37°C and pH 7.5.

In the presence of 3 mM NaCl we observed a 13-fold and 15-fold higher affinity for the binding of ATPase to ADP compared to AMP at 25°C (Kd values equal to 0.07 and 0.9 μM) and at 37°C (Kd values equal to 0.04 and 0.6 μM), respectively (Table 1). Affinities of ATPase to ADP and ATP were similar (Table 1). The data show that β-phosphate significantly contributes to the affinity of nucleotides to ATPase, while γ-phosphate of ATP does not affect the affinity during complex formation. These data confirm previous suggestions6,13 regarding the key role of β-phosphate in the formation of Na,K-ATPase complexes with nucleotides. The obtained binding constants for ADP and ATP, are close to the values determined previously7,14.

In the presence of 3 mM KCl instead of NaCl (E2 state), we failed to observe any measurable ATP or ADP binding to ATPase either at 25 or 37°C, in agreement with the previous data6. To determine binding constants by ITC it is necessary to use protein concentration exceeding the Kd value15. According to14, the Kd value for ATP binding with Na,K-ATPase in the E2 state is equal to 450 μM. The concentrations of Na,K-ATPase used in this study render it not possible to determine the Kd values by ITC.

Energetics of complex formation between Na,K-ATPase and adenine nucleotides

In addition to the binding constant estimates, obtained from the shape of the binding curves, we also estimated the change of enthalpy, ΔH, upon binding of an adenine nucleotide to Na,K-ATPase (Table 1). Plotting the enthalpy of the interaction versus temperature we obtained heat capacity change (ΔCp) values for the ATPase interactions with adenine nucleotides as d(ΔH)/dT (Figure 2, Table 2). Na,K-ATPase binding both to AMP and ADP had low ΔCp of −66 and −43 cal·mol−1·K−1, respectively, and the ATP binding to Na,K-ATPase was characterized by massive ΔCp of −760 cal·mol−1·K−1 (Table 2). On the assumption of additive contributions of the different phosphor groups to the ΔCp values16, we found that the contribution of γ-phosphate is equal to −717 cal·mol−1·K−1.

Figure 2. Thermodynamic analysis of Na,K-ATPase interactions with adenine nucleotides.

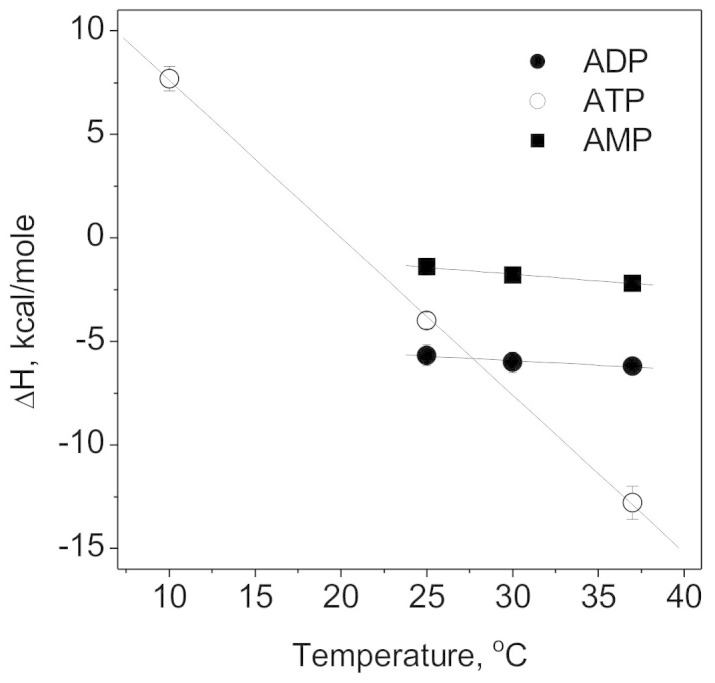
Figure 2

Open in a new tab

Temperature dependence of enthalpy of the duck Na,K-ATPase interactions with AMP (filled squares), ADP (filled circles) and ATP (empty circles) at pH 7.5.

Table 2. Heat capacity changes and change in the solvent-accessible surface area for the duck Na,K-ATPase binding to AMP, ADP and ATPa.

Ligand ΔCp, cal·mole−1·K−1 ΔAtot_minb, Å2 ΔAtot_maxb, Å2
AMP −66 349 551
ADP −43 228 333
ATP −760 4021 5891
Open in a new tab

aHeat capacity changes were obtained as d(ΔH)/dT from Figure 2.

bChanges in solvent-accessible surface area were estimated using the following formula: ΔCp = k × ΔAtot, where ΔAtot is the total change in solvent accessible surface area in Å2. ΔAtot_max and ΔAtot_min are calculated for the min and max k values (0.129 and 0.189 cal/(K·mol·Å2), as shown in21).

Changes in heat capacity are believed to reflect the change in the solvent accessible surface area (SAA) during the process of complex formation17. The large decrease in ΔCp upon complex formation between Na,K-ATPase and ATP suggests that ATP binding to Na,K-ATPase significantly decreases its SAA18. To assess changes in SAA by ITC a number of empirical formulas are used, which connect the changes in ΔCp and the changes of water-accessible polar and nonpolar surface area in proteins19,20. In the review21 it was shown that formulas representing individual contributions of polar and nonpolar groups can be replaced by the formula with a single coefficient: ΔCp = k × ΔAtot, where ΔAtot is the total change in SAA in Å2. The value of k reported in different studies varies from 0.129 to 0.189 cal/(K·mol·Å2)21. Using these values we estimated the total decrease in protein SAA when enzyme complexes with nucleotides are formed (Table 2).

Molecular modeling of the Na,K-ATPase structure changes upon ATP binding

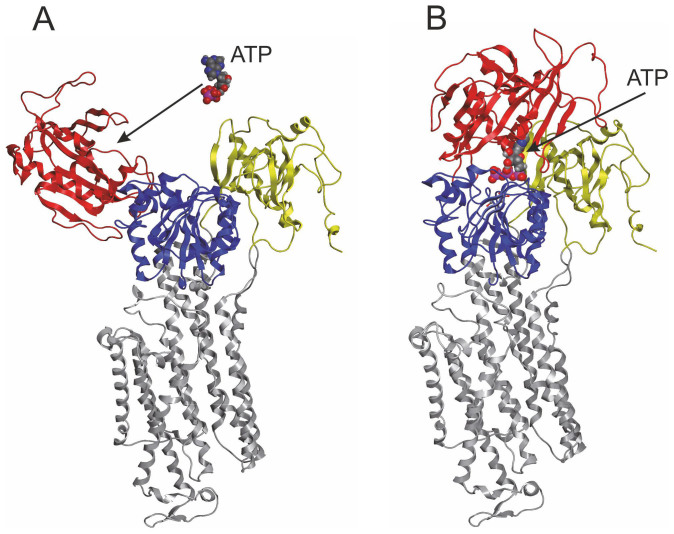
Models have been constructed of the Na,K-ATPase in the “open” E1 free state and “closed” ATP-bound state (Figure 3). In the E1 “open” state the N and P domains are separated in space. In the E1 “closed” state the N domain is positioned closer to the boundary between the P and A domains, with the ATP γ-phosphate in the N domain interacting with the side chain of residue Asp369 in the P domain. The SAAs, estimated for an “open” E1 and “closed” E1 conformations were 39436 Å2 and 34687 Å2, respectively. Thus, the difference in SAA between the “open” and “closed” ATP-binding state conformations is 4749 Å2. This is in good agreement with the data of ΔAtot obtained from ITC (Table 2).

Figure 3. Conformational changes of Na,K-ATPase upon ATP binding.

Figure 3

Open in a new tab

(A) Cartoon representation of the Na,K-ATPase α1-subunit model, built on the basis of 2.8 Å structure of the porcine α1-subunit (Protein Data Bank code 3wgu). (B) Complex of the Na,K-ATPase with ATP. The ATP was docked to the protein as described5. Color coding of the Na,K-ATPase refers to individual domains, with the A domain (residues 1 to 77 and 149 to 270) in yellow, the P domain (residues 363 to 376 and 589 to 753) in blue, the N domain (residues 377 to 588) in red, and the transmembrane region (M1–M10) in grey. The ATP nucleotide is shown as ball-and-stick representation with standard color coding for atom type (carbon, gray; oxygen, red; nitrogen, blue; sulfur, yellow; phosphorus, pink; magnesium, brown). This figure was prepared with MOE version 2013.08 modeling software.

Discussion

The classical scheme of Na,K-ATPase function consists of the two major conformational transitions: between the phosphorylated forms of the enzyme E1P-E2P and between the non-phosphorylated forms E2-E1. From the crystal structure of Na,K-ATPase it is known that in the E2 conformation the N and P domains are positioned close to each other22, while limited proteolysis results showed that in the E1 conformation these domains reside apart in three-dimensional space23. Conformational transitions in the Na,K-ATPase molecule when binding nucleotides up to now has not been established.

AMP and ADP binding to Na,K-ATPase is followed by small changes in the ΔH and ΔS values with temperature and there are no indications of a structural transition in the enzyme. The large ΔS variation with temperature upon ATP binding to Na,K-ATPase, the decrease of ΔH with increasing of temperature, and the numerically large, negative heat capacity change upon complex formation (Figure 2, Tables 1,2), suggest that ATP binding to Na,K-ATPase induces a structural transition of the enzyme that transfers a part of the surface area from a solvent exposed to solvent protected state18,24. Thus the ATP γ-phosphate plays a key role in the structural rearrangement essential for the enzyme function. Similarly, binding of GTP, but not GDP, leads to a global conformational transition in the structures of elongation (EF-G) and translation termination (eRF3) factors19,25. Modeling of the Na,K-ATPase complex with ATP, produces results demonstrating convergence of the ATP γ-phosphate in the N domain and Asp369 in the P domain of Na, K-ATPase (Figure 3B), and shows the change of solvent accessible surface area of the protein similar to those that were obtained experimentally for ATP binding (Table 2). Thus, the structural changes occurring upon ATP binding can be attributed to the convergence of N and P domains of Na,K-ATPase. For structural homolog of the catalytic subunit of Na,K-ATPase, SERCA, it was demonstrated that domain arrangements in the Ca2+-bound E1 form and in the complex with a nonhydrolyzable ATP analog AMPPCP (Ca2-E1-AMPPCP) are different11. Nucleotide binding assembles the cytoplasmic domains by a 90° rotation of the N domain toward the P domain, converting the open Ca2+-bound E1 form into a compact structure.

To summarize, it has been shown that Na,K-ATPase in the E1 form complexed with ATP and ADP have different conformations. When complexed with ADP the enzyme does not undergo structural changes and remains in the E1-open conformation, whereas when binding ATP it undergoes a structural transition resulting in a significant decrease of the solvent accessible surface area. Replacement of ADP by ATP leads to convergence of the Na,K-ATPase nucleotide binding and phosphorylation domains, resulting in the protein adopting the E1-closed conformation, ready for phosphorylation. Thus, γ-phosphate is a trigger for the structural change in Na,K-ATPase, converting it from the relaxed state to the ratcheted state.

Methods

Na,K-ATPase purification and activity measurements

Na,K-ATPase (α1β1 isozyme26) was purified from duck salt glands (for details see27,28,29) to the purity grade of 99% of total protein as confirmed by polyacrylamide gel electrophoresis in the presence of SDS. The enzyme preparations also contain a 13 kDa protein that is similar to proteins of the FXYD family30. Specific activity of the duck Na,K-ATPase reached ~1800–2000 μmol Pi (mg protein × h)−1 at 37°C.

Hydrolytic activity of the duck Na,K-ATPase was estimated as ATP cleavage in the medium containing (in mM): 130 NaCl, 20 KCl, 3 MgCl2, 3 ATP, and 30 imidazole, pH 7.4 at 37°C31,32,33. Na-K-ATPase-induced ATP hydrolysis was measured as a difference in ATP cleavage rate in the presence or absence of the Na-K-ATPase specific inhibitor ouabain (1 mM)29. Reaction of the ATP cleavage was carried out in the volume 0.5 ml. It was initiated by the addition of the protein (2 μg) and stopped 2 min later by the addition of 0.5 ml of the 3 M cold acetate buffer. The method developed by Rathbun and Betlach33 was used to quantify the amount of inorganic phosphate (Pi) spectrophotometrically. In the presence of ouabain, ATPase activity in the enzyme preparation was not detected, indicating the absence of other ATPases in the preparation.

Isothermal titration calorimetry (ITC)

The thermodynamic parameters of adenine nucleotides (ATP, ADP and AMP) binding to duck Na,K-ATPase were measured using a MicroCal iTC200 instrument (MicroCal, Northampton, MA), as described elsewhere34. Experiments were carried out at 10, 25, 30 and 37°C in imidazole buffer containing 25 mM imidazole, 1 mM EDTA, 250 mM sucrose, 0.1 mM DTT, and either 3 mM NaCl or 3 mM KCl, pH 7.5. Experiments were conducted in the absence of Mg2+, preventing the formation of phosphorylated intermediate13. Absence of ATP hydrolysis by the enzyme was monitored by detecting Pi. Aliquots (2.6 μl) of ligands were injected into a 0.2-ml cell containing the protein solution to achieve a complete binding isotherm. Protein concentration in the cell ranged from 5 to 20 μM, and ligand concentration in the syringe ranged from 50 to 200 μM. The heat of dilution was measured by injection of the ligand into the buffer solution or by additional injections of ligand after saturation; the values obtained were subtracted from the heat of reaction to obtain the effective heat of binding. The resulting titration curves were fitted using the MicroCal Origin software, assuming one set of binding sites. Affinity constants (Ka) and enthalpy variations (ΔH) were determined and the Gibbs energy (ΔG) and entropy variations (ΔS) were calculated from the equation: ΔG = −RTlnKa = ΔH − TΔS.

Modelling

Three-dimensional models of the Na,K-ATPase catalytic α-subunit in the E1 “opened” and “closed” state were created on the basis of the previously published 2.8 Å structure of the porcine α-subunit in the [Na3]E1P-ADP state (PDB code 3wgu)5. For modeling of the complex of Na,K-ATPase in the ATP E1 “closed” state the ATP molecule was placed into the cavity of the ATP-binding site of the enzyme similarly to the ADP molecule5. Modeling the enzyme in the “opened” E1 free state was carried out by changing the geometry of the polypeptide chain of the boundary segments 375–379 and 586–590 of the N-domain, by analogy to the changes observed in SERCA upon nucleotide binding, specifically exemplified by the rigid body motion of N-domain relative to the A and P-domains11. The resulting model structures of Na, K-ATPase were minimized in the force field MMFF94x using the MOE version 2013.08 modeling software (Chemical Computing Group Inc., Montreal, Quebec, Canada). The solvent accessible surface area of the α-subunit of Na,K-ATPase was calculated with the server http://structure.pitt.edu/anchor.

Author Contributions

I.Yu.P. and V.A.M. conceived the project, coordinated the study and drafted the paper with contributions from A.A.A., O.D.L. and A.A.M. I.Yu.P., V.A.M., E.A.K. and E.A.D. performed experiments. A.A.A. analyzed the structural data. A.A.M. coordinated the study and contributed materials and reagents.

Acknowledgments

This study was funded by the Molecular and Cellular Biology Program of the Russian Academy of Sciences and by the Russian Foundation for Basic Research (grants 14-04-01737 and 12-04-00403).

References

  1. Schainer-Bobis G. The sodium pump. Its molecular properties and mechanics of ion transport. Eur. J. Biochem. 269, 2424–2433 (2002). [DOI] [PubMed] [Google Scholar]
  2. Apell H. J. & Karlish S. J. Functional properties of Na,K-ATPase, and their structural implications, as detected with biophysical techniques. J. Membr. Biol. 180, 1–9 (2001). [DOI] [PubMed] [Google Scholar]
  3. Geering K. The functional role of beta subunits in oligomeric P-type ATPases. J. Bioenerg. Biomembr. 33, 425–438 (2001). [DOI] [PubMed] [Google Scholar]
  4. Nyblom M. et al. Crystal structure of Na+, K(+)-ATPase in the Na(+)-bound state. Science 342, 123–127 (2013). [DOI] [PubMed] [Google Scholar]
  5. Kanai R., Ogawa H., Vilsen B., Cornelius F. & Toyoshima C. Crystal structure of a Na+-bound Na+,K+-ATPase preceding the E1P state. Nature 502, 201–206 (2013). [DOI] [PubMed] [Google Scholar]
  6. Grell E., Lewitzki E., Schacht A. & Stolz M. Nucleotide/Protein Interaction: Energetic and Structural Features of Na, K-ATPase. J. Therm. Anal. Calorim. 77, 471–481 (2004). [Google Scholar]
  7. Fedosova N. U., Champeil P. & Esmann M. Rapid filtration analysis of nucleotide binding to Na,K-ATPase. Biochemistry 42, 3536–3543 (2003). [DOI] [PubMed] [Google Scholar]
  8. Xu K. Y., Zweier J. L. & Becker L. C. Oxygen-free radicals directly attack the ATP binding site of the cardiac Na+, K(+)-ATPase. Ann. N.Y. Acad. Sci. 834, 680–683 (1997). [DOI] [PubMed] [Google Scholar]
  9. Toyoshima C., Nakasako M., Nomura H. & Ogawa H. Crystal structure of the calcium pump of sarcoplasmic reticulum at 2.6 A resolution. Nature 405, 647–655 (2000). [DOI] [PubMed] [Google Scholar]
  10. Winther A. M. et al. The sarcolipin-bound calcium pump stabilizes calcium sites exposed to the cytoplasm. Nature 495, 265–269 (2013). [DOI] [PubMed] [Google Scholar]
  11. Sørensen T. L., Møller J. V. & Nissen P. Phosphoryl transfer and calcium ion occlusion in the calcium pump. Science 304, 1672–1675 (2004). [DOI] [PubMed] [Google Scholar]
  12. Pilotelle-Bunner A. et al. ATP binding equilibria of the Na(+), K(+)-ATPase. Biochemistry 47, 13103–13114 (2008). [DOI] [PubMed] [Google Scholar]
  13. Grycova L. et al. ATP and magnesium drive conformational changes of the Na+/K+-ATPase cytoplasmic headpiece. Biochim. Biophys. Acta, 1081–1091 (2009). [DOI] [PubMed] [Google Scholar]
  14. Skou J. C. The Na,K-pump. Methods Enzymol. 156, 1–25 (1988). [DOI] [PubMed] [Google Scholar]
  15. Ladbury J. E. & Doyle M. L. Biocalorimetry 2. Applications Calorimetry In The Biological Sciences. (The Sussex John Wiley & Sons, Ltd, 2004). [Google Scholar]
  16. Hedwig G. R. & Hinz H. J. Group additivity schemes for the calculation of the partial molar heat capacities and volumes of unfolded proteins in aqueous solution. Biophys Chem 100, 239–260 (2003). [DOI] [PubMed] [Google Scholar]
  17. Jelesarov I. & Bosshard H. R. Isothermal titration calorimetry and differential scanning calorimetry as complementary tools to investigate the energetics of biomolecular recognition. J Mol Recognit. 12, 3–18 (1999). [DOI] [PubMed] [Google Scholar]
  18. Kairane C., Mahlapuu R., Ehrlich K., Zilmer M. & Soomets U. The effects of different antioxidants on the activity of cerebrocortical MnSOD and Na,K-ATPase from post mortem Alzheimer's disease and age-matched normal brains. Curr. Alzheimer Res. 11, 79–85 (2014). [DOI] [PubMed] [Google Scholar]
  19. Hauryliuk V. et al. The pretranslocation ribosome is targeted by GTP-bound EF-G in partially activated form. Proc. Natl. Acad. Sci. U S A 105, 15678–15683 (2008). [DOI] [PMC free article] [PubMed] [Google Scholar]
  20. Perozzo R., Folkers G. & Scapozza L. Thermodynamics of protein-ligand interactions: history, presence, and future aspects. J Recept Signal Transduct Res. 24, 1–52 (2004). [DOI] [PubMed] [Google Scholar]
  21. Robertson A. D. & Murphy K. P. Protein Structure and the Energetics of Protein Stability. Chem Rev. 97, 1251–1268 (1997). [DOI] [PubMed] [Google Scholar]
  22. Shinoda T., Ogawa H., Cornelius F. & Toyoshima C. Crystal structure of the sodium-potassium pump at 2.4 A resolution. Nature 459, 446–450 (2009). [DOI] [PubMed] [Google Scholar]
  23. Segall L., Javaid Z. Z., Carl S. L., Lane L. K. & Blostein R. Structural basis for alpha1 versus alpha2 isoform-distinct behavior of the Na,K-ATPase. J. Biol. Chem. 278, 9027–9034 (2003). [DOI] [PubMed] [Google Scholar]
  24. Fuller W., Parmar V., Eaton P., Bell J. R. & Shattock M. J. Cardiac ischemia causes inhibition of the Na/K ATPase by a labile cytosolic compound whose production is linked to oxidant stress. Cardiovasc Res. 57, 1044–1051 (2003). [DOI] [PubMed] [Google Scholar]
  25. Kononenko A. V. et al. GTP-dependent structural rearrangement of the eRF1:eRF3 complex and eRF3 sequence motifs essential for PABP binding. Nucleic Acids Res. 38, 548–558 (2010). [DOI] [PMC free article] [PubMed] [Google Scholar]
  26. Boldyrev A. A., Lopina O. D., Kenney M. & Johnson P. Characterization of the subunit isoforms of duck salt gland Na/K adenosine triphosphatase. Biochem Biophys Res Commun. 216, 1048–1053 (1995). [DOI] [PubMed] [Google Scholar]
  27. Petrushanko I. Y. et al. S-glutathionylation of the Na,K-ATPase catalytic α subunit is a determinant of the enzyme redox sensitivity. J. Biol. Chem. 287, 32195–32205 (2012). [DOI] [PMC free article] [PubMed] [Google Scholar]
  28. Jørgensen P. L. Purification of Na+, K+-ATPase: enzyme sources, preparative problems, and preparation from mammalian kidney. Methods Enzymol. 156, 29–43 (1988). [DOI] [PubMed] [Google Scholar]
  29. Smith T. W. Purification of Na+, K+-ATPase from the supraorbital salt gland of the duck. Methods Enzymol. 156, 46–48 (1988). [DOI] [PubMed] [Google Scholar]
  30. Yakushev S. S., Kumskova E. M., Rubtsov A. M. & Lopina O. D. Effect of colchicine on sensitivity of duck salt gland Na,K-ATPase to Na+. Biochemistry (Mosc) 73, 990–994 (2008). [DOI] [PubMed] [Google Scholar]
  31. Petrushanko I. et al. Na-K-ATPase in rat cerebellar granule cells is redox sensitive. Am. J. Physiol. Regul. Integr. Comp. Physiol. 290, R916–925 (2006). [DOI] [PubMed] [Google Scholar]
  32. Komniski M. S., Yakushev S., Bogdanov N., Gassmann M. & Bogdanova A. Interventricular heterogeneity in rat heart responses to hypoxia: the tuning of glucose metabolism, ion gradients, and function. Am. J. Physiol. Heart Circ. Physiol. 300, H1645–1652 (2011). [DOI] [PubMed] [Google Scholar]
  33. Rathbun W. B. & Betlach M. V. Estimation of enzymically produced orthophosphate in the presence of cysteine and adenosine triphosphate. Anal. Biochem. 28, 436–445 (1969). [DOI] [PubMed] [Google Scholar]
  34. Mitkevich V. A. et al. Termination of translation in eukaryotes is mediated by the quaternary eRF1*eRF3*GTP*Mg2+ complex. The biological roles of eRF3 and prokaryotic RF3 are profoundly distinct. Nucleic Acids Res. 34, 3947–3954 (2006). [DOI] [PMC free article] [PubMed] [Google Scholar]

Articles from Scientific Reports are provided here courtesy of Nature Publishing Group

RESOURCES