Mechanical limits to maximum weapon size in a giant rhinoceros beetle

Abstract

The horns of giant rhinoceros beetles are a classic example of the elaborate morphologies that can result from sexual selection. Theory predicts that sexual traits will evolve to be increasingly exaggerated until survival costs balance the reproductive benefits of further trait elaboration. In Trypoxylus dichotomus, long horns confer a competitive advantage to males, yet previous studies have found that they do not incur survival costs. It is therefore unlikely that horn size is limited by the theoretical cost–benefit equilibrium. However, males sometimes fight vigorously enough to break their horns, so mechanical limits may set an upper bound on horn size. Here, I tested this mechanical limit hypothesis by measuring safety factors across the full range of horn sizes. Safety factors were calculated as the ratio between the force required to break a horn and the maximum force exerted on a horn during a typical fight. I found that safety factors decrease with increasing horn length, indicating that the risk of breakage is indeed highest for the longest horns. Structural failure of oversized horns may therefore oppose the continued exaggeration of horn length driven by male–male competition and set a mechanical limit on the maximum size of rhinoceros beetle horns.

1. Introduction

Sexual selection drives the evolution of many of Nature's most conspicuous morphologies and displays [1,2]. A central tenet of sexual selection theory is that sexual traits are costly, and that these traits will evolve to be increasingly exaggerated until naturally selected survival costs balance the reproductive benefits of further trait elaboration [2–4].

In many systems, the size or intensity of sexual traits and displays does indeed reflect an equilibrium between mating benefits and survival costs [5]. For example, male field crickets with longer calling bouts and higher call rates are favoured by choosy females, but are also more likely to be attacked by parasitoid flies that use these calls to locate their hosts [6]. Similarly, male guppies with brighter colour patterns are more attractive to females, but are also more conspicuous to predators [7]. As a result, these sexual traits represent a selective balance between mating benefits and survival costs.

In other systems, however, sexual traits do not appear to reflect a balance between benefits and costs. In fact, surprisingly few studies have found evidence that sexual traits incur evolutionarily significant costs [8–10], and there are now several examples of exaggerated ornaments and weapons that are not nearly as costly as we might expect [11–13]. These observations suggest that the evolution of sexual traits is constrained by other factors before reaching the theoretical cost–benefit equilibrium.

The horns of the Asian rhinoceros beetle, Trypoxylus dichotomus, are one such example of an elaborate, but inexpensive sexually selected trait. Males have a long, forked head horn that they use to pry rival males away from wounds or sap sites on trees, where females come to feed (figure 1) [14,15]. As in other rhinoceros beetles [16,17], T. dichotomus horns are positively allometric, such that large males have disproportionately long horns, and small males have disproportionately short horns [14,15]. Males with longer horns are more likely to win fights and gain ownership of these sap sites, and therefore achieve higher mating success [18,19]. Surprisingly, although horns can reach nearly two-thirds the length of the body in the largest males, they appear to incur no costs. Specifically, horns do not impair the beetles' ability to fly [20–23]; horns do not stunt the growth of other body structures [24]; horns do not weaken the beetles' immune function [24]; and, most importantly, horns do not reduce male survival [24,25]. Thus, there appears to be directional sexual selection for increasing horn size, yet little or no counterbalancing selection due to low survival. What prevents T. dichotomus males from evolving even longer horns?

Figure 1.

Trypoxylus dichotomus males use their long forked head horn to fight over a hornless female feeding at a natural sap site.

I hypothesized that mechanical limits set an upper bound on horn size. That is, the continued exaggeration of horn size may be constrained by the risk of structural failure of the horn itself. T. dichotomus males fight vigorously enough to break their horns [14] (EL McCullough 2011, personal observation), which suggests that horns are sometimes pushed to their structural limits. Furthermore, because males cannot repair or replace a broken horn, injured males are effectively removed from the mating pool. Yet, whether unusually large weapons are more susceptible to failure is currently unknown.

To test this mechanical limit hypothesis, I estimated the safety factors of horns across the full range of horn sizes, using both laboratory and field measurements. Safety factors quantify how ‘safe’ a structure is relative to the loads it is likely to experience and are defined in this study as the ratio between the load that causes a horn to fail and the maximum expected load [26].

During fights, a T. dichotomus male inserts his head horn underneath an opponent and uses it like a pitchfork to pry the rival up and off the substrate (electronic supplementary material, movie S1). Thus, to win a fight, a male must overcome his rival's ability to hold on to the tree, or his grasping force. Game theory predicts that males will only escalate to intense, sustained fights when the contestants are of equal size [27–29], and field observations indicate that this is true for T. dichotomus [15,30]. Because the maximum loads a horn must withstand occur during fights with a size-matched rival, a male's own grasping force can be used as a proxy for the maximum fighting forces likely to be experienced by his horn. If mechanical limits are important in determining maximum horn size, safety factors should decrease with increasing horn length.

2. Material and methods

Field observations were conducted at the National Chi Nan University campus in central Taiwan. The campus grounds contain many ash (Fraxinus spp.) trees, which is the exclusive host plant of T. dichotomus in Taiwan. The study was conducted in June and July when adults are most abundant. All males found in the study area were collected from their natural sap sites and individually marked with quick-drying paint pens. Head horn length (hereafter simply referred to as horn length) and body size were measured to the nearest 0.01 mm using dial calipers. Horn length was measured from the base of the head horn to the tip of the lateral-most tine, and body size was measured as prothorax width. Males were scored as having no visible injuries, moderate injuries (i.e. scrapes or small wounds on their horns, pronota or elytra) or severe injuries (i.e. several broken tines or entirely broken horns).

I estimated how much force a male would exert and transmit through his horn to dislodge a typical size-matched rival (hereafter referred to as ‘fighting force’) by measuring that male's own grasping force. I measured the fighting forces of field-collected males (n = 278) by clamping a spring scale onto their short thoracic horn (which is just above the beetle's centre of mass [23]), and slowly pulling on the scale until the beetle was dislodged from the tree. Fighting force was measured five times for each individual, with a 1 min rest between trials. Beetles were observed closely to ensure that no trials resulted in obviously submaximal performance, and there was no evidence that they fatigued over time (repeated-measures ANOVA: F_4,1230 = 0.62, p = 0.65). The maximum force from these trials was used in the statistical analyses (see [31] for justification for using maximal performance values). All trials were conducted at natural sap sites between 20.00 and 04.00 when the beetles are most active.

To estimate the loads that cause horn failure, I purchased beetles as final instar larvae from a commercial insect vendor and raised them to adulthood in the laboratory. Laboratory-reared beetles were used in the breaking tests to control for differences in age and thus variation in the wear and fatigue of horns. Adult males were housed in individual glass containers, and experiments were conducted when beetles were approximately 10 days post-eclosion. Horn length and body size were measured to the nearest 0.01 mm using dial calipers. The heads of cold-euthanized males (n = 76) were dissected from the body and fixed at the base to a support block using fast-drying epoxy. The distal tip of the horn was also embedded in a thin strip of epoxy to minimize stress concentrations. To prevent desiccation, the horns were wrapped with wet paper towels while the epoxy hardened.

The maximum force supported by a horn before failure (hereafter referred to as ‘breaking force’) was measured using an Instron In-Spec 2200 mechanical tester. Horns were loaded in cantilever bending to mimic the loading regime experienced during a typical fight. The safety factor for each horn was then calculated as the measured value of breaking force divided by the estimated value of fighting force. Fighting force estimates for beetles used in the breaking force tests were derived from the best-fit line from ordinary least-squares regression: fighting force = 0.12 × horn length + 0.94 (see also Results). The results are qualitatively the same when fighting forces were estimated as a function of body size instead of horn length, because body size and horn length are strongly correlated (R² = 0.91).

After horn failure, the maximum stress experienced within the horn cuticle before fracture was estimated using classic beam theory [32]. The distance along the antero-posterior axis of the horn between the tip (i.e. point of force application) and the fracture margin was measured, and then the free end of the horn was embedded in clear acrylic resin and sanded until smooth to measure the cross-sectional geometry of the horn (e.g. height and second moment of area) as close as possible to the site of failure. The outer and inner margins of the cuticle at this location were traced manually from digital photographs using ImageJ (National Institutes of Health), and the second moment of area (a shape factor that describes the distribution of mass in the cross section about the neutral bending axis) was measured with the BoneJ plugin [33]. The ultimate bending stress (σ) of the horn cuticle at the site of failure was then calculated as

where F is the breaking force, r is the distance between the fracture margin and the point of force application, c is half the height of the horn cross section and I is the second moment of area.

3. Results

Of the 1012 males collected and measured in the field, 174 (17%) showed some sign of injury, and 44 (4%) had severely damaged horns (figure 2). Injured males were significantly larger than uninjured males (ANOVA: F_1,1010 = 19.14, p < 0.001). The average body size was 24.66 ± 2.27 mm (mean ± s.d.) for severely injured males, 23.89 ± 2.77 mm for injured males and 23.04 ± 2.70 mm for uninjured males. These observations are consistent with those of Siva-Jothy [14], who also found that severe horn damage was most common among the largest males.

Figure 2.

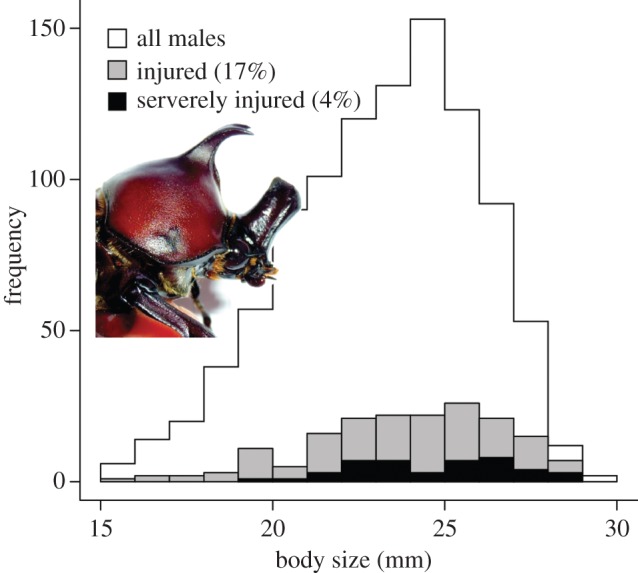
Body size histograms of field-collected males with varying degrees of injury; 17% of the population showed some sign of injury and 4% had severely damaged horns. Inset: example of a male with a broken head horn.

Maximum fighting forces increased with horn length (figure 3; R² = 0.48, F_1,275 = 252.2, p < 0.001), indicating that longer horns experience higher loads. There was also a weak, but statistically significant relationship between breaking force and horn length (figure 3; R² = 0.08, F_1,74 = 6.05, p = 0.02), indicating that longer horns can withstand higher loads. Horns broke at various locations between the middle and base of the horn shaft, but there was no relationship between horn length and the location of failure along the antero-posterior axis of the horn (R² = 0.001, F_1,74 = 0.04, p = 0.85). The ultimate bending stress of the horn cuticle at the point of fracture ranged from 92.5 to 394.6 MPa (201.4 ± 75.2 MPa). There was no relationship between bending stress and horn length (R² = 0.02, F_1,70 = 1.07, p = 0.30), but there was a significant relationship between bending stress and the location of failure along the length of the horn (R² = 0.54, F_1,70 = 83.28, p < 0.001). Ultimate bending stress decreased from the proximal base to the middle of the horn shaft, which may be largely explained by the corresponding decrease in second moment of area (EL McCullough 2013, unpublished data).

Figure 3.

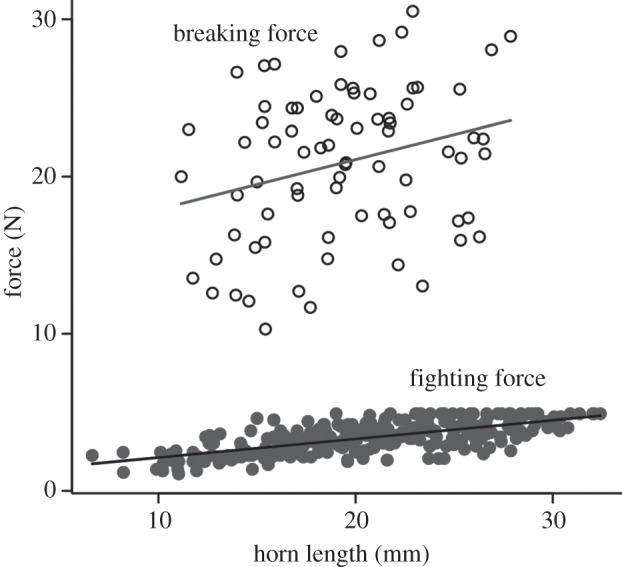
Relationships between horn length and fighting force (dark grey points; R² = 0.48, F_1,275 = 252.2, p < 0.001; fighting force = 0.12 × horn length + 0.94) and breaking force (open circles; R² = 0.08, F_1,74 = 6.05, p = 0.02; breaking force = 0.32 × horn length + 14.74).

Safety factors ranged from 3.5 to 10.3 (6.5 ± 1.6). There was a significant negative relationship between safety factor and horn length (figure 4; R² = 0.13, F_1,74 = 10.9, p < 0.01), indicating that longer horns are more susceptible to failure.

Figure 4.

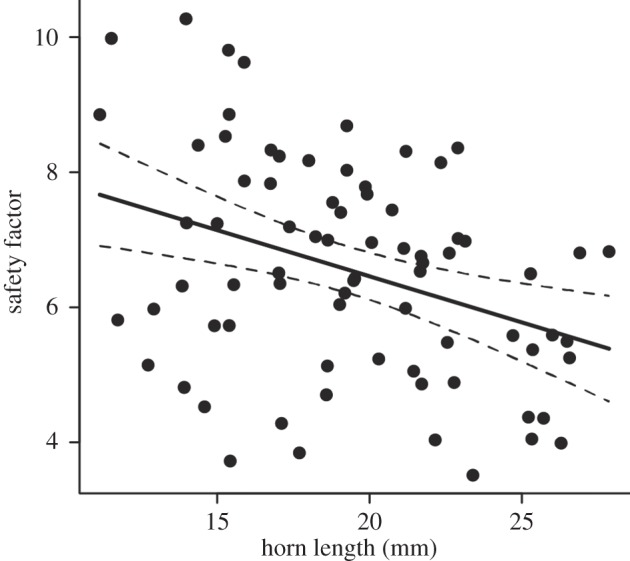
Relationship between horn length and safety factor (R² = 0.13, F_{1, 74} = 10.9, p < 0.01; safety factor = −0.14 × horn length + 9.19). The dashed lines indicate the 95% confidence intervals.

4. Discussion

The results presented here support the hypothesis that mechanical limits set an upper bound on horn size in the rhinoceros beetle T. dichotomus. The safety factors of horns, calculated as the ratio of absolute breaking force to maximum expected load, decreased with increasing horn length, indicating that the risk of breakage is highest for the longest horns. Furthermore, field observations indicate that males do indeed push horns to their structural limits, and that large males with long horns are the most susceptible to horn damage (figure 2) [14]. Mechanical failure of large horns may therefore ‘put the brakes on’ the continued exaggeration of horns driven by male–male competition.

Although it is possible that horns also experience high loads when a male falls to the ground after an unsuccessful fight, these forces are likely to be much lower than the forces exerted on horns during fights. The estimated impact force of a ‘worst case scenario’ fall, based on high-speed videos of beetles falling to the floor in the laboratory, is approximately 2.5 N, which is no greater than the measured fighting forces. In the field, beetles falling into leaf litter will experience significantly lower impact forces. I therefore expect that the fighting forces estimated here are the maximal loads exerted on horns, and thus the most biologically relevant forces for determining the safety factors of beetle horns.

Biological safety factors should vary depending on the structure's contribution to fitness, the predictability of the loads it experiences, and how costly the structure is to produce and maintain [26]. Based on this theory, beetle horns are expected to have very high safety factors. Horns are critically important to a male's reproductive success; males cannot repair or replace a broken horn; and the loads exerted on horns during fights are likely to be very unpredictable. Additionally, males should be able to afford a robust horn because they are inexpensive to produce and maintain. In agreement with these predictions, I found that T. dichotomus horns have high safety factors, ranging from 3.5 to 10.3. Nevertheless, these estimates suggest that even the horns with the lowest safety factors are at least three times stronger than needed to withstand the maximum forces incurred during fights, so the probability of fracture should be very low. The observation that horns do fail in the field at fairly high frequencies indicates that they must be subjected to loads or conditions that differ from those measured in this study.

It is important to note, however, that the safety factors of beetle horns are comparable to safety factors measured for other biological structures: 2.6–7.4 for crab claws [34,35], 4.8 for the leg bones of a galloping horse [26], 6 for the wing bones of a flying goose [26] and 9–17 for the flight feathers of a flying pigeon [36]. And, like beetle horns, these structures can and do fail. Approximately 6% of wild crab populations have broken claws [34], and the incidence of fracture for leg and wing bones of mammals and birds ranges from 0.2 to 3% [37]. Thus, even structures with high measured safety factors are susceptible to mechanical failure under natural conditions.

There are at least three aspects of loading in biological structures that often are not accounted for in safety factor estimates, and which may contribute to the seemingly high rates of failure under natural conditions: complex loading regimes, material fatigue and viscoelasticity of biological materials. These factors may also explain the apparent mismatch between the measured safety factors of beetle horns and the relatively high incidence of breakage in natural populations.

First, in this study, breaking forces were measured by loading horns in pure cantilever bending, yet horns are likely to experience more complex loading regimes in typical fights. In particular, T. dichotomus horns—with their broad, forked tips—are expected to be both bent and twisted when a male tries to pry his opponent up and off of a tree. One of the broken horns collected in the field had a spiral-shaped fracture, indicating that horns can be subjected to significant torsional loads during fights.

Second, the horns used in the breaking tests were from males that were kept in individual containers, and that were therefore in nearly pristine condition. In natural populations, however, horns accrue scratches, abrasions and other types of wear (figure 5) that can act as local stress concentrators and significantly lower the theoretical strength of a structure [38–40]. Furthermore, horns are probably very susceptible to material fatigue due to repetitive loading and the accumulation of micro-cracks. Fatigue failure has been reported for a number of biological structures, including mammalian bones [32] and mollusc shells [40], and recent work on the fatigue properties of locust wing cuticle indicates that cyclic loading can cause failure at loads that are only a third of the cuticle's ultimate breaking strength [41]. These observations indicate that older horns may fail at loads significantly lower than those that would cause failure the first time the horns are loaded [32,40,41]. This fatigue hypothesis is supported by seasonal patterns of horn damage in the field: broken horns were more common at the end of the breeding season, presumably after males had engaged in many nights of intense fighting, subjecting their horns to high, repeated loads.

Figure 5.

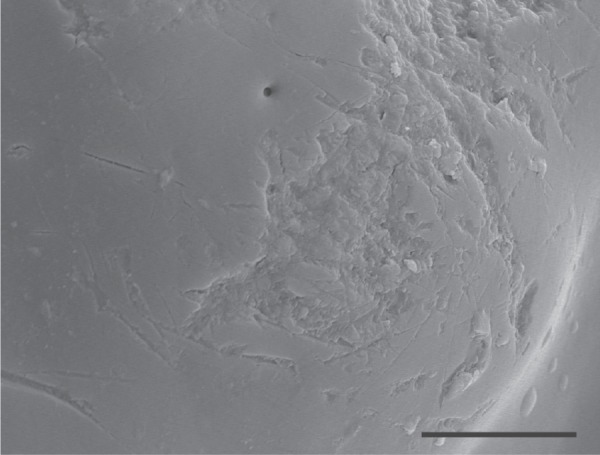
Scanning electron micrograph of the outer surface of a horn from a field-collected beetle, showing substantial abrasion and numerous scratches and micro-cracks. Scale bar, 10 µm.

Third, like other biomaterials, horn cuticle is expected to be at least somewhat viscoelastic, so a horn's ability to dissipate energy and withstand crack propagation during impacts may be highly sensitive to the rate at which it is loaded [32,38]. High loading rates are known to make viscoelastic materials more rigid and brittle [32], so a very rapid flick during a fight may put horns at a higher risk of failure, especially in the presence of scratches, cracks and other flaws.

Water content can also significantly affect the material properties of insect cuticle [42–44]. Specifically, desiccation increases the strength and stiffness of cuticle, but decreases fracture toughness, or the ability to withstand defects and crack propagation [42]. The horns of T. dichotomus males are significantly drier than other body parts; their relative moisture content is only 26%, compared with 40% in elytra and 54% in legs [23]. Intriguingly, because horns are so dry, the material properties of ‘fresh’ samples of horn cuticle are remarkably similar to those of ‘dry’ samples of cuticle from other insects: ultimate bending stress is 201.4 ± 75.2 MPa for fresh horn cuticle, compared with 217.4 ± 48.2 MPa for dry locust leg cuticle [42]. Previous authors have suggested that insects could increase the stiffness and strength of their exoskeletons by simply reducing the cuticle's moisture content [42]. The low moisture content of T. dichotomus horns may be an example of such an adaptation. However, while the increase in strength and stiffness makes horns more resistant to bending and thus more effective at transmitting fighting forces [45], these changes also make the horns more brittle, and therefore more likely to fail, particularly in the presence of surface defects and other types of wear [42]. Whether the horns of other rhinoceros beetle species also have low moisture contents, or whether other species trade off the strength, stiffness and toughness of horns in different ways remains to be tested.

I propose that mechanical limits are important in setting the maximum size of horns in rhinoceros beetles in general. In all species studied to date, males use their horns as weapons in male–male competitions [17,46,47], and males with broken horns are not uncommon in museum collections (electronic supplementary material, figure S1). Thus, it appears that many rhinoceros beetle species push horns to their mechanical limits, so the competitive advantage of increased horn size may be opposed by the increased vulnerability of horn failure. Quantifying the structural properties of different horns, and identifying how intensely and predictably each species uses its horns during fights may be critical in understanding variation in horn size among species. Future studies should also consider other factors (e.g. genetic and developmental constraints) that may contribute to the limits on horn size. Furthermore, understanding how variation in horn shape, in addition to horn size, affects performance during combat is likely to provide important insights into patterns of diversity in rhinoceros beetle horns, and potentially other sexually selected animal weapons [45].

Funding statement

This project was funded by grants from the Society for Integrative and Comparative Biology, Sigma-Xi and the National Science Foundation (DGE 0809127, OISE 0912433 and IOS 1310235).