Skip to main content
PMC home page
Nature Portfolio logoLink to Nature Portfolio
. 2014 Jun 2;5:3990. doi: 10.1038/ncomms4990

Resistive switching and its suppression in Pt/Nb:SrTiO3 junctions

Evgeny Mikheev 1,a, Brian D Hoskins 1,2, Dmitri B Strukov 2, Susanne Stemmer 1,a
PMCID: PMC4059928  PMID: 24886761

Abstract

Oxide-based resistive switching devices are promising candidates for new memory and computing technologies. Poor understanding of the defect-based mechanisms that give rise to resistive switching is a major impediment for engineering reliable and reproducible devices. Here we identify an unintentional interface layer as the origin of resistive switching in Pt/Nb:SrTiO3 junctions. We clarify the microscopic mechanisms by which the interface layer controls the resistive switching. We show that appropriate interface processing can eliminate this contribution. These findings are an important step towards engineering more reliable resistive switching devices.

Inline graphicUnderstanding the resistive switching mechanism in oxide-based memories is an ongoing challenge. Here, the authors isolate an unintentional interfacial layer as the origin of resistive switching in Pt/Nb:SrTiO3 junctions, and show that suitable processing can remove this unwanted contribution.

Non-volatile resistive switching devices have attracted considerable attention, owing to the promise of a non-volatile, continuously tunable (as opposed to binary) memory and new computing approaches1,2,3. The two-terminal nature of such devices allows for lateral scaling to nanometre dimensions4. Typical devices show, however, poor reproducibility and device-to-device variability, which are the main barriers towards creating a viable technology3,5. One extensively studied type of resistive switching device consists of a Schottky junction between a doped, wide-band gap oxide, such as SrTiO3, and high work-function metals such as Pt, Au or metallic oxides such as SrRuO3 and YBa2Cu3O7−x6,7,8,9. In such devices, resistive switching is accompanied by a modulation of the effective Schottky barrier height7,8,10,11,12. These devices combine technologically attractive features, such as hysteretic current–voltage (I–V) characteristics with large on/off ratios, bipolar switching and continuously tunable resistance states. Unlike other types of resistive switching memories, they do not require an initial forming step, which is a significant advantage for practical applications. To effectively address the issues of reproducibility and variability, the microscopic origins of resistive switching must be understood.

Here, we report a systematic study of resistive switching of metal/oxide interfaces formed between Pt and Nb-doped SrTiO3. The results demonstrate that resistive switching is controlled by an interfacial layer, as revealed by a parasitic interfacial capacitance, an increased ideality factor of the Schottky barrier and an extended depletion width within the SrTiO3. A charge trapping-based model can fully explain the Schottky barrier lowering that accompanies the resistive switching. The interfacial layer capacitance is crucial in controlling the magnitude of the effect. We show that the contribution of such unintentional layers to the resistive switching process can be minimized by appropriate processing, thus providing a pathway towards engineering more reliable resistive switching devices.

Results

I–V characteristics

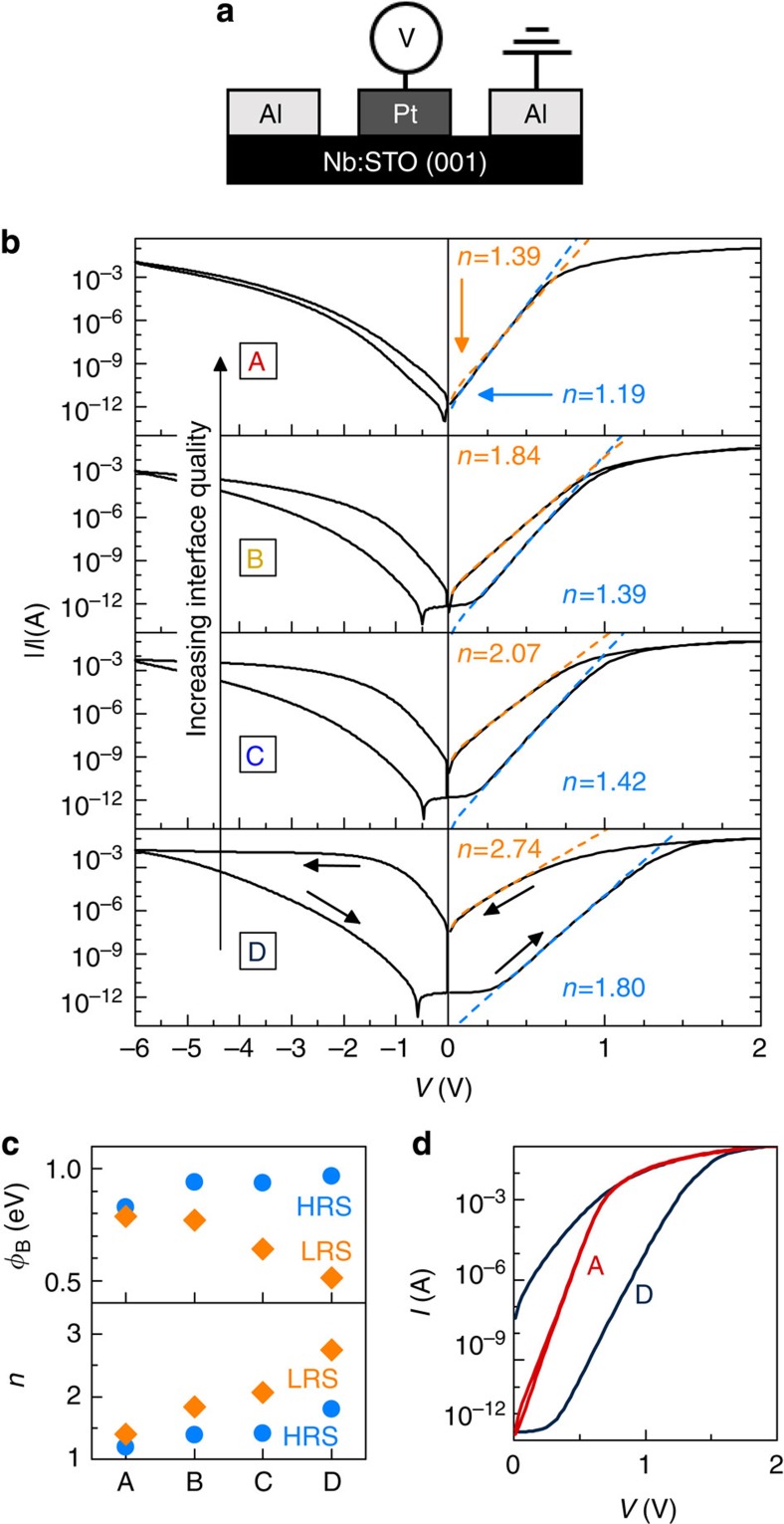
A series of Pt/Nb:SrTiO3 devices (Fig. 1a) were investigated, as summarized in Table 1. The interface quality was varied intentionally across this series. The samples are labelled A–D, in the order of decreasing interface quality. Sample A (highest quality interface) consists of an all-epitaxial junction of (001)Pt grown by high-temperature sputtering13. The interface quality of samples B, C and D was progressively reduced by sputtering Pt at room temperature (B), removing the in situ pregrowth anneal (C) and using highly energetic deposition (D). All samples were annealed in O2 to eliminate any possible contributions from oxygen vacancies.

Figure 1. I–V characteristics of the devices.

Figure 1

Open in a new tab

(a) Schematic of the device. (b) I–V characteristics for all samples. The blue and orange lines are fits to equation (1) for the HRS and the LRS, respectively, in each case. (c) Extracted barrier heights φB and ideality factors n. (d) Forward bias I–V for samples A and D.

Table 1. Summary of the Pt/Nb:SrTiO3 junction fabrication.

Sample A B C D
Pt deposition technique DC sputtering DC sputtering DC sputtering E-beam evaporation
In situ pregrowth anneal 2 h, 825 °C 2 h, 825 °C 5 min, 120 °C None
Pt growth temperature 825 °C Room temperature Room temperature Room temperature
Pt microstructure Epitaxial, (001)-oriented Polycrystalline Polycrystalline Polycrystalline
Post-growth anneal 30 s, 800 °C in flowing O2 30 s, 800 °C in flowing O2 30 s, 800 °C in flowing O2 30 s, 800 °C in flowing O2
Open in a new tab

Figure 1b shows the I–V characteristics of the four Pt/Nb:SrTiO3 junctions. The I–V hysteresis was probed by sweeping from −6 V to +2 V, then back to −6 V. All samples show bipolar resistive switching, with a high positive bias increasing the junction current and negative bias reversing the effect. The device states are referred to as low- and high-resistance states (LRS and HRS). The HRS is identical to the initial state of the device if an appropriate switching protocol is chosen. The conductivity of the LRS can be tuned by modifying this protocol7. The magnitude of the resistive switching clearly decreases as the junction quality is improved (that is, from sample D to A).

At low forward bias, the junction current can be described by thermionic emission theory:

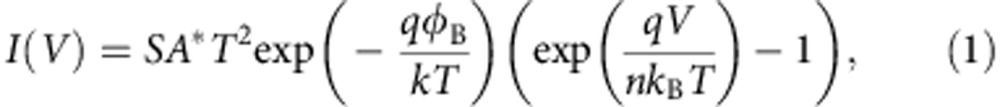graphic file with name ncomms4990-m1.jpg

where S is the junction area, A* the Richardson constant, q the electron charge, k the Boltzmann constant, T the temperature, n the ideality factor and φB the Schottky barrier height. The ideality factor n describes the deviation from ideal thermionic emission:

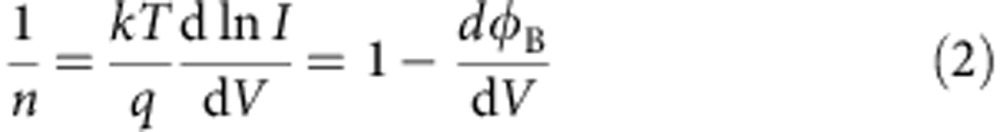graphic file with name ncomms4990-m2.jpg

Here, we use Equation (1) with φB and n as fit parameters to describe the lower and upper branches of the forward bias loop, corresponding to the HRS and LRS, respectively. The results are shown in Fig. 1c, from which several systematic trends are evident. Specifically, higher quality junctions have lower n in both HRS and LRS and slightly lower φB in HRS. Switching to LRS increases n and reduces φB, and this effect is much more pronounced in lower quality junctions.

The value of n=1.19 for the junction with epitaxial Pt (sample A) in LRS is very close to the ideal value (n=1). The near-ideal value is particularly noteworthy considering the high-doping level in the SrTiO3 (ND=1020 cm−3), because n typically increases with ND8,14,15. The lowest reported value at room temperature is n=1.1416 for an in situ ozone-cleaned Au/Nb:SrTiO3 junction, albeit at a much lower doping of ND=1018 cm−3. At high-doping levels (ND>1018 cm−3), typically reported values of n are above 1.48,9, similar to our samples B, C and D.

Two possible origins exist for n>1: (i) electron tunnelling through the barrier and (ii) a voltage-dependent barrier height8,14. In case of (i), the forward bias current should increase with n. Figure 1d shows that the opposite is true: the forward current is higher at low n. This suggests mechanism (ii), which is generally linked to the presence of an insulating interface layer and/or surface states15,17, the combination of which causes n>1. Next, we further analyse n, which allows for insights into the origins of resistive switching.

The applied bias V is partitioned by the parasitic capacitance of the interface layer Ci, with Vi=V(n−1)/n applied across the interface layer. The voltage across the depletion region is thus reduced to VD=V/n. A complete description of n15,17 involves not just Ci, but also the surface state charge, separated into two parts: the charge density in equilibrium with the metal (Dsa) and with the doped SrTiO3 (Dsb):

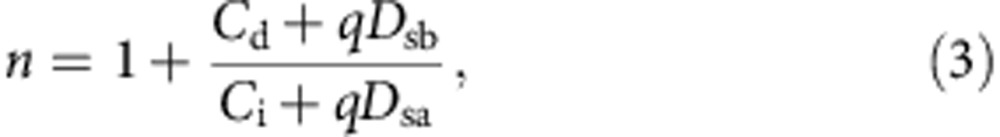graphic file with name ncomms4990-m3.jpg

where Cd is the depletion region capacitance. An adequate description of metal/Nb:SrTiO3 junctions can usually be obtained by neglecting the interface trapped charge9,10,14, which does not play a dominant role in C–V data measured at high frequencies (discussed next). In this case, n is given as:

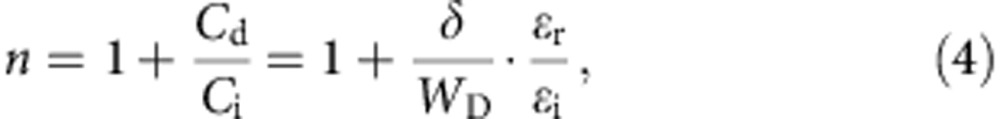graphic file with name ncomms4990-m4.jpg

where δ and WD are the interface layer and depletion width thicknesses, and εi and εr are their respective dielectric constants. From Equation (4), we see that the increase of n with decreasing interface quality can be rationalized in terms of a smaller interface capacitance caused by an increased thickness of the interfacial layer (δ). The increase in n and the lowering of φB on switching the junction to the LRS are generally observed for this type of resistive switching memory. It has been ascribed to barrier inhomogeneity8,11,18,19,20, because the global φB as measured in photocurrent experiments is insensitive to resistive switching19,21.

Capacitance–voltage characteristics

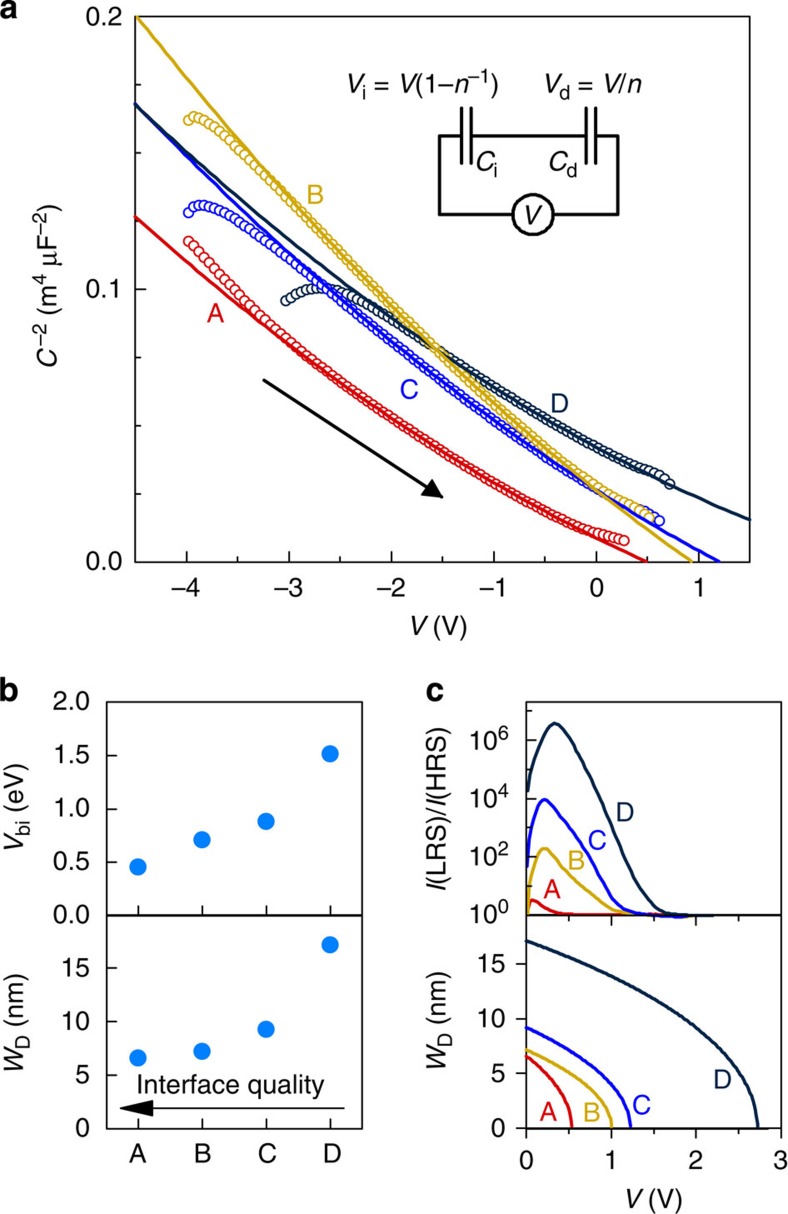
Further evidence of the interface layer is provided by capacitance–voltage (C–V) measurements. As discussed next, C–V clearly show the effects of applied bias partitioning between the depletion width and the interface layer. Figure 2a shows C−2 as a function of bias for all junctions (HRS), measured at 1 MHz frequency. On switching to LRS (not shown), the capacitance is hardly affected, in contrast to the large effect on I–V characteristics. The capacitance in LRS is increased by only a few per cent and decays with time, similar to the trend observed in the I–V measurements6,11.

Figure 2. C–V characteristics of the devices.

Figure 2

Open in a new tab

(a) C–V data for all samples in the HRS, plotted as C−2 versus V. The lines are fits to Equation (6). The inset shows the equivalent circuit model and the voltage partitioning between the depletion and interface layer capacitances, Cd and Ci. (b) Extracted built-in potentials Vbi and depletion widths WD at zero bias. (c) Ratio between the currents in LRS and HRS as the function of voltage (top) and the calculated depletion width under forward bias (bottom).

For Schottky junctions with a conventional semiconductor, C−2 is linearly dependent on V, as given by dC−2/dV=−2/(eε0ε1ND), where ε0 and εr are the vacuum dielectric permittivity and semiconductor relative dielectric permittivity. SrTiO3 has an electric field (E) dependent permittivity, which can be described as:

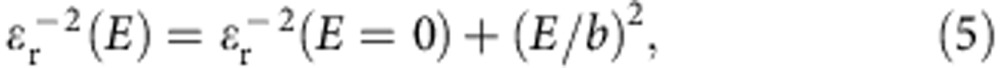graphic file with name ncomms4990-m5.jpg

where εr(E=0) and b are temperature-dependent constants. The field-dependent permittivity is responsible for the curvature of the C−2–V curves seen in Fig. 2a. The reduced curvature of the junctions with high n can thus be explained by the presence of an interface layer, which reduces the electric field that drops over the depletion region. Quantitatively, the measured capacitance is C=Cd/n, and for a field-tunable εr it can be written as14,17:

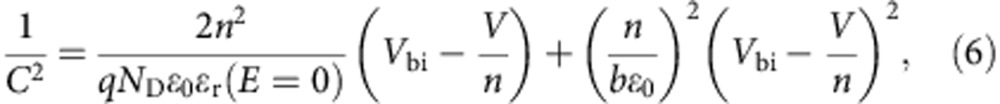graphic file with name ncomms4990-m6.jpg

where Vbi is the built-in voltage of the junction. We use this expression to fit the data in Fig. 2a, using Vbi and ND as the fit parameters. The constants εr(E=0) and b were extracted from the voltage dependence of C as described in refs 14, 22 and in the Methods Section. Using n obtained in the HRS from the I–V curves, the extracted values for ND are within 4% of ND determined by Hall measurements. The good description provided by Equation (6) confirms the interface layer model and shows that Equation (4) is appropriate. In particular, the analysis shows that n increases with decreasing interface quality because of the decrease of Ci in Equation (4), and not because of the interface states (see Equation (3)). Specifically, δ determines the Ci and the magnitude of n, and results in partitioning of the applied bias between the depletion width and the interface layer. We note that we make no claims that the interface states are absent (see also below), only that they are not the cause for the observed trend of increasing n in low-quality junctions.

Figure 2b shows that the extracted Vbi decreases with junction quality, similar to the trend observed for φB. The magnitude of the resistive switching clearly scales with Vbi, similar to prior reports as a function of electrode metal work function9. V=nVbi represents the forward bias at which the depletion region vanishes. The depletion width, WD, can be calculated from Vbi14,17:

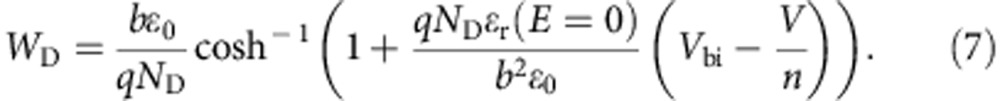graphic file with name ncomms4990-m7.jpg

The zero-bias value of WD is shown in Fig. 2b for all junctions. It scales with junction quality, similar to Vbi. Figure 2c (bottom graph) shows the calculated WD as a function of forward bias. The difference between I(LRS) and I(HRS) goes through a maximum with applied voltage, and the hysteresis loop closes (I(LRS)=I(HRS)) approximately at the same voltage when WD vanishes (see top graph in Fig. 2c).

Switching between resistance states

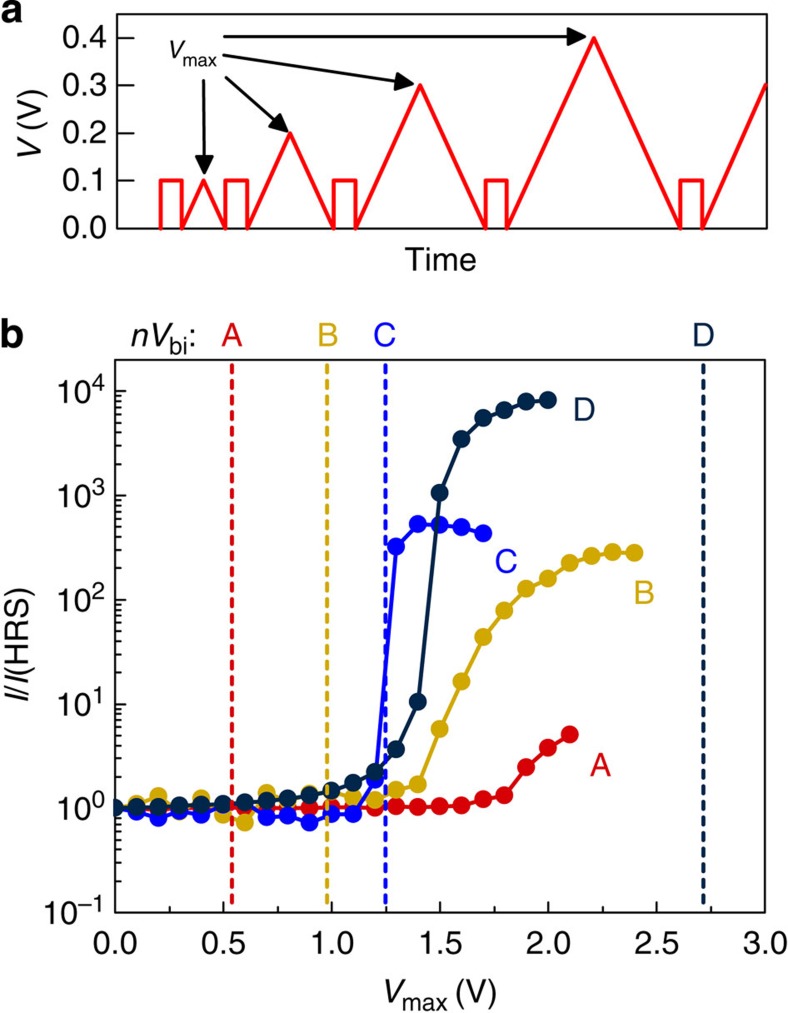
It is important to note that the switching from HRS to LRS occurs at a higher forward bias than that required for WD=0. This is illustrated in Fig. 3, where a sequence of positive bias loops with increasing peak voltages (Vmax) was applied (top graph), measuring the low-signal current (at +100 mV, bottom graph) between each cycle. As Vmax is increased, switching from HRS to LRS is observed for all devices. Except for junction D (lowest quality), the threshold for resistive switching is clearly above V=nVbi (dashed lines in Fig. 3c). The threshold voltage for switching is larger for high-quality junctions, showing the opposite trend as Vbi. As there is no more depletion region when the junction transitions from HRS to LRS, it is unlikely that defects that are located within the depletion region at zero bias are responsible for large resistive switching.

Figure 3. Switching characteristics of the devices.

Figure 3

Open in a new tab

(a) Applied voltage sequence used for testing the switching from HRS to LRS. The sequence consisted of alternating between 0.1 V read pulses and increasingly large switching biases. (b) Normalized small-signal currents plotted as a function of peak voltage in the switching loop. The effective built-in voltages, V=nVbi, are indicated by the dashed lines.

Discussion

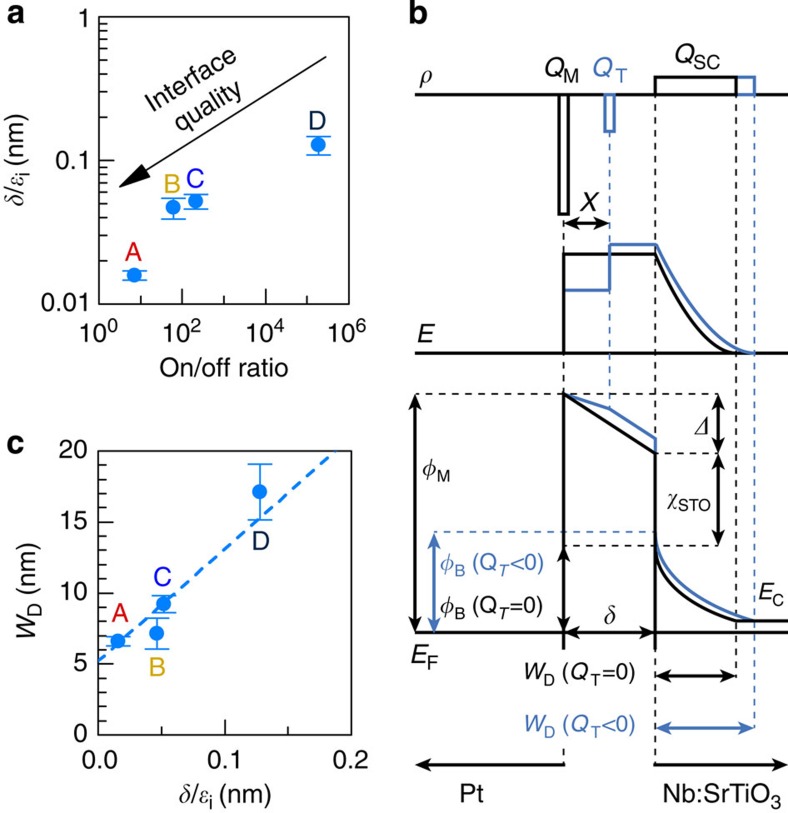
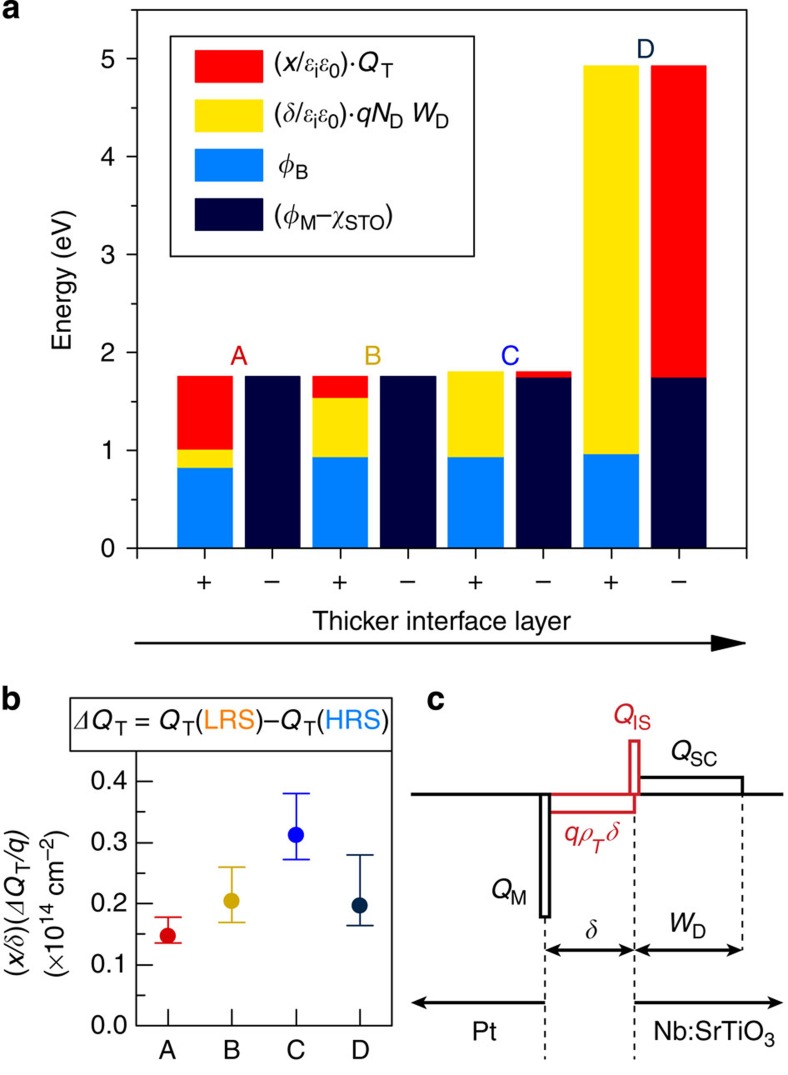
To briefly recap, the main result so far is the trend shown in Fig. 1a, namely the progressive suppression of resistive switching in high-quality junctions. From the combined analysis of I–V and C-V data, this trend can be explained by the reduction of an interface layer thickness δ. This correlation is illustrated in Fig. 4a, where the on/off ratio (I(LRS):I(HRS) measured at 0.1 V immediately after switching to LRS and HRS), is plotted against δ/εi, calculated from Equation (4). For interfaces with near-ideal n, resistive switching and the interface layer are (nearly) absent.

Figure 4. Influence of the interface layer on the Schottky junction parameters.

Figure 4

Open in a new tab

(a) Correlation between the magnitude of resistive switching and the interface layer thickness. (b) Effect of a negative trapped charge QT on a Pt/Nb:SrTiO3 junction. Top: charge density (ρ) distribution profile with and without QT. Middle: electric field (E) profile with and without QT. Bottom: band profile. The Schottky barrier is increased by a negative QT. (c) Correlation between the zero-bias depletion width and the interface layer thickness.

Possible physical origins of the interface layer are: (i) unintentional contamination, (ii) growth-induced damage and/or disorder and (iii) an intrinsic deadlayer arising from surface reconstructions23. Regarding (i), it is known that oxide surfaces such as SrTiO3 chemisorb carbon-hydroxyl layers upon air exposure and removal requires high temperatures and/or oxygen containing atmospheres24,25,26, as applied to sample A. Secondary ion mass spectrometry (SIMS; see Methods section) indicates significant amounts of carbon at the Pt/Nb:SrTiO3 interface for samples B, C and D, consistent with an interface contamination layer. (ii) may be an additional factor for sample D, as e-beam evaporation is known to cause more damage from energetic deposition than sputtering27. Intrinsic deadlayers, (iii), are unlikely to play a significant role, because of the systematic trend with interface processing. Oxygen vacancy concentrations were minimized by post-growth annealing in oxygen and, even if present, are the same for all samples. Consequently, a mobile defect migration-based resistive switching mechanism is highly unlikely to be relevant for resistive switching in the material system studied here (though it may, of course, play a role in other types of resistive switching memory devices).

The most likely mechanism for resistive switching involves charge trapping within the interface layer, as will be discussed next. Figure 4b shows the electric field profile across a junction that contains an effective trapped charge QT with a centroid position x, which could be within the interface layer or at the interface or both. QT alters the electric field profile across the junction. For example, a negative QT results in increased φB and WD. The effect of QT is accounted by an energy Δ, which modifies φB from its ideal value28, as described by:

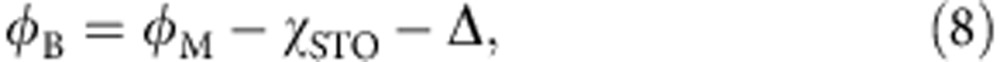graphic file with name ncomms4990-m8.jpg

where φM is the metal work function and χSTO is the electron affinity of SrTiO3. Both QT and the space charge QSC=qNDWD in the depletion layer contribute to Δ. For a charge centroid at x (see Fig. 4b):

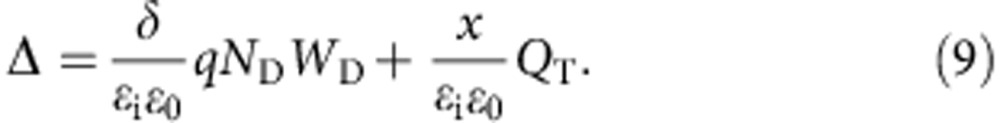graphic file with name ncomms4990-m9.jpg

The value of φB is thus dependent on the spatial distribution of the trapped charge. Combining Equations (8) and (9), we obtain:

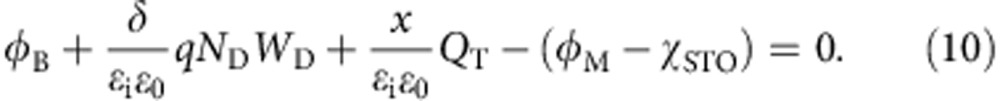graphic file with name ncomms4990-m10.jpg

The first two terms in Equation (10) are known from the I–V and C–V measurements. The last term is constant across the series, and we take φM=5.65 eV29 and χSTO=3.9 eV30. The third term, which contains QT, can thus be obtained from the experimental data. Figure 5a illustrates the contributions of all four terms in Equation (10) for the four samples. They are grouped into the negative [(φM−χSTO)=1.75 eV] and positive (φB and 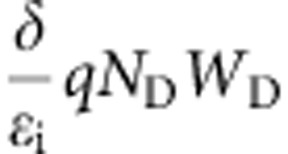Inline graphic) terms in Equation (10). QT can of course be negative or positive, and furthermore, it may contain contributions from interface traps and trapped charge within the interface layer. From Fig. 5a, we see an increasingly large contribution from the depletion layer for the samples with high δ. Because (φM−χSTO) is constant, Equation (10) yields a contribution from QT that switches from positive to negative sign across the series. The crossover from positive to negative can be rationalized by the presence of two distinct contributions to QT, with opposite signs. One contribution must come from the interface states (QIS) at the interface with SrTiO3. In general, QIS is determined by defects that are intrinsic to a specific semiconductor’s surface and is relatively insensitive to the specific overlayer31,32. Depending on the position with respect to the charge neutrality level (CNL) results, QIS can be negative or positive28. In SrTiO3, the CNL is high in the upper half of the band gap, approximately 0.7 eV below the conduction band30,33. Given the measured φB heights in Fig. 1c and the degenerate nature of doped SrTiO334, this implies that the Fermi level is below CNL and QIS is positive. This suggests a second contribution to QT, which is negative, and increases with decreasing interface quality. Figure 5a shows that it scales with δ, which implies a negative trapped charge, qδρT, with a volume density ρT in the interface layer. The negative trapped charge also explains the linear scaling of depletion width with δ. As shown in Fig. 4c, in the presence of a large negative charge in the interface layer, WD increases to satisfy charge neutrality.

Figure 5. Contributions of trapped and interface charges.

Figure 5

Open in a new tab

(a) Magnitude of the positive and negative terms (+ and − columns, respectively) in Equation (10) for all junctions in HRS. (b) Calculated modulation of trapped charged centroid during resistive switching, ΔQT=QT(LRS)−QT(HRS). (c) Simplified charge density profile for a Pt/Nb:SrTiO3 junction with interface states and trapped charge in the interface layer.

The charge profile resulting from the two contributions to QT is illustrated in Fig. 5c. The positive QIS and negative ρT produce the effective charge centroid QT, plotted in Fig. 5a,b:

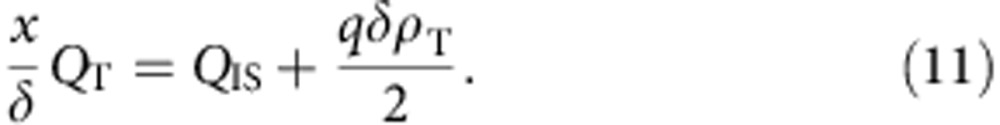graphic file with name ncomms4990-m12.jpg

Figure 5c shows the simplest charge profile that can be used to rationalize the scaling of QT with δ. Although more complex, inhomogeneous distributions of trapped charge in the interface layer are possible35, their role in modifying φB is still correctly described by a charge centroid QT.

As shown in Fig. 5b, the analysis also lends itself to an explanation of the switching between HRS and LRS. Switching is accomplished by a change in QT towards a more positive value in LRS, which can happen in two ways: (i) trapping of additional positive charge at the SrTiO3 surface, increasing QIS, or, (ii) reduction of the negative charge in the interface layer, decreasing δρT. Mechanism (ii) is more likely, given the nature of the time-dependent decay of LRS towards HRS.

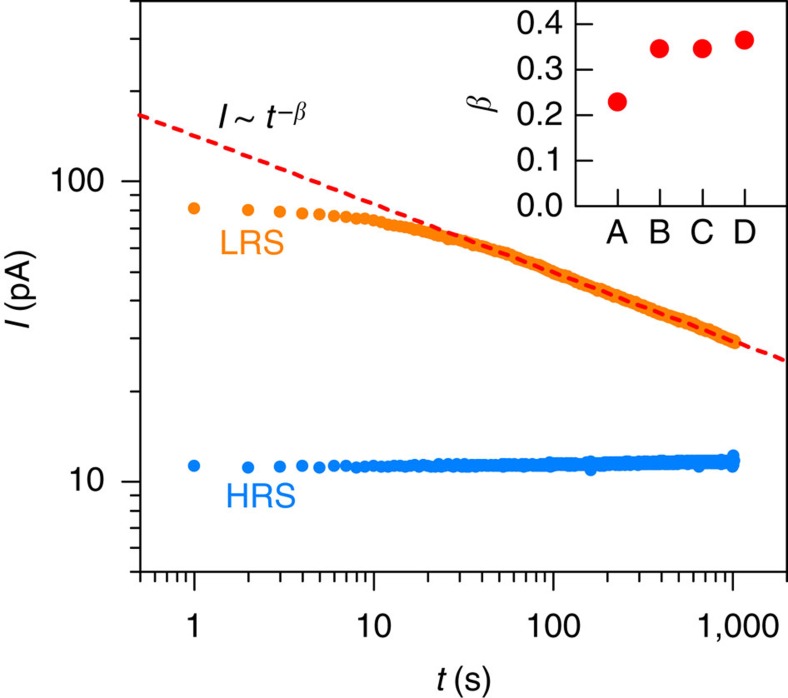
The state retention characteristics of the junctions are shown in Fig. 6. After a positive or negative voltage loop (for HRS and LRS respectively), the small-signal current is monitored (at V=+100 mV). With the appropriate switching protocol, the HRS is a stable state, whereas the current in LRS decays with time, eventually returning back to HRS. The decay of the LRS follows a power law with time after switching (I~tβ) after the junction is switched to LRS11,36. This so-called Curie-von-Schweidler behaviour is typical for capacitive charging37 and it is commonly observed for charge trapping under bias in high-k dielectrics38,39. The decay is due to a progressive increase in negative charges, which were previously de-trapped upon switching to LRS, implying mechanism (ii). The decay rates are similar for all junctions, with a slight trend towards slower decay in high-quality junctions, as revealed by the exponent β (see the inset in Fig. 6). The similarity in the retention properties of all junctions suggests a common origin of their resistive switching properties.

Figure 6. Resistance state retention characteristics.

Figure 6

Open in a new tab

Time-dependence of the small-signal (+0.1 V) current after switching to HRS and LRS, shown for sample A. The red dashed line is a fit to a power law for the decay of LRS. The inset shows the power law exponent for all junctions.

In contrast to the large resistive switching effect in the I–V measurement, ΔQT, the change of QT between HRS and LRS (Fig. 5b), does not correlate with δ. Instead, it scales with the threshold voltage for resistive switching, shown in Fig. 3. The variation of ΔQT across the series is quite small. This implies rather similar defect chemistry across the series that drives the resistive switching, and it further emphasizes the crucial roles of the interface layer thickness (capacitance): The Schottky barrier modulation (Fig. 1c) is caused by ΔQT, but the magnitude of the effect is determined by interface layer capacitance (thickness), as is evident from Equations (9) and (10). The interfacial layer capacitance (εi/δ) determines the voltage drop due to the trapped charge, thus controlling the degree of Schottky barrier modulation, and the magnitude of the resistive switching effect, upon the voltage-induced modulation of the trapped charge. Consequently, the magnitude of the resistive switching effect directly scales with δ, as seen experimentally in Figs 1b and 4a. Fluctuations in interface capacitance between devices, caused by variations in δ, can readily explain the commonly observed non-uniformity of resistive switching parameters.

In summary, the results presented here establish a strong connection between the suppression of resistive switching and reduction of an interfacial layer. To date, research on this type of device has largely focused on Schottky contacts using metals deposited at room temperature. As shown here, such interfaces readily provide large resistive switching effects. The effect is, however, due to an unintentional defective layer that is difficult to control, and thus likely responsible for poor reproducibility and device-to-device variations. The fact that this is now understood, and that it can furthermore be suppressed, using appropriate fabrication methods, opens the way towards engineering resistive switching based on intentional modification of interfaces or defect densities. To produce reliable and reproducible results, and thus a practical technology, high-quality interfaces, such as the epitaxial Pt junctions developed here, are essential to minimize contributions from unintentional layers.

Methods

Device processing

Devices were fabricated using (001) SrTiO3 single crystals doped n-type with 0.7 wt % Nb. To obtain a flat, stepped surface, the substrates were etched in Aqua Regia and annealed at 1,000 °C for 2 h in air. For sample A, which had the highest interface quality, the substrate was annealed at 825 °C in 10 mTorr O2, in situ, before growth of epitaxial, (001) Pt by DC sputtering at 825 °C in 10 mTorr Ar using a sputter power of 30 W. A detailed characterization such Pt films has been published elsewhere13. X-ray data is shown in Supplementary Fig. 1. For sample B, the substrate was cooled after the in situ anneal and Pt growth was performed at room temperature. For sample C, the pregrowth anneal was omitted. For sample D, Pt was deposited by standard e-beam evaporation at room temperature. Compared to sputtering, e-beam evaporation produces more damage from energetic deposition27. All samples were post-Pt deposition annealed at 800 °C in flowing O2 to eliminate any possible contributions from oxygen vacancies that may have formed during Pt deposition. Square 30 × 30 μm2 Pt electrodes were patterned by standard photolithography, following by selective oxidation of the top Pt surface by a room temperature plasma in 300 mTorr O2 at 100 W. The Pt oxide was then used as a hard mask for wet etching Pt in Aqua Regia at 60 °C40. A ground-signal-ground electrode configuration was then completed with Ohmic Al contacts made to the Nb:SrTiO3, which was defined by a standard lift-off process (see Fig. 1a for the completed device). I–V measurements were performed using a needle probe station and a HP 4155 semiconductor parameter analyser. C–V measurements were performed using a Cascade Microtech probe station with GGB 100-μm ground-signal-ground probes and a HP 4294 impedance analyser at 1 MHz.

Fitting procedures

Fitting in Fig. 1b was done in the data range below 1 μA to minimize the effect of series resistance. Fitting in Fig. 2a was performed in the range between −2 V and −0.25 V, where the AC conductance was low for all junctions. The s.d. of the extracted fit parameters were <2% for n and <1% for φB and Vbi, error bars in Figs 4 and 5 were calculated using error propagation.

To account for the field dependence of the dielectric constant, εr, of Nb:SrTiO3, we follow the procedure described in refs 14, 22. For a metal/semiconductor Schottky junction, the electric field decreases with the distance z from the interface. For a non-linear dielectric, this results in a spatial variation of εr, which is reduced at the interface (z=0) and increases to its zero-field value across the depletion width. The voltage dependence of capacitance can be used to extract the electric field E and εr at z=0:

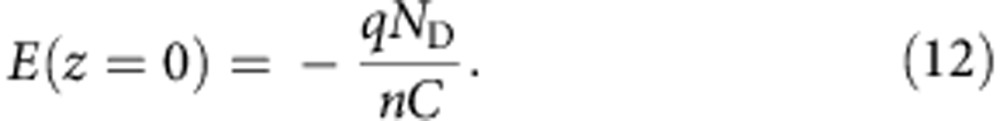graphic file with name ncomms4990-m13.jpg
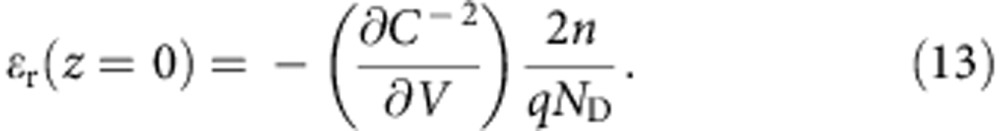graphic file with name ncomms4990-m14.jpg

The field dependence of the tunable dielectric constant εr can be parameterized as:

graphic file with name ncomms4990-m15.jpg

where a and b are materials constants. The zero-field dielectric constant is then given as: 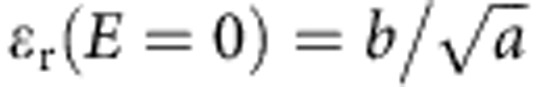Inline graphic, and one can rewrite Equation (14) as a linear relationship between 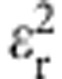Inline graphic and E2:

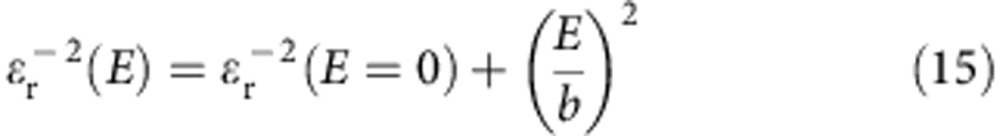graphic file with name ncomms4990-m18.jpg

Supplementary Fig. 2 shows 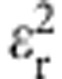Inline graphic as a function of E2 at z=0, obtained from the experimental C–V measurements. Fitting to equation (15) in the low-conductance region was used to obtain the materials parameters b and εr(E=0). We also performed fits using a simplified expression, which neglects the field dependence of εr, but does account for the voltage partitioning:

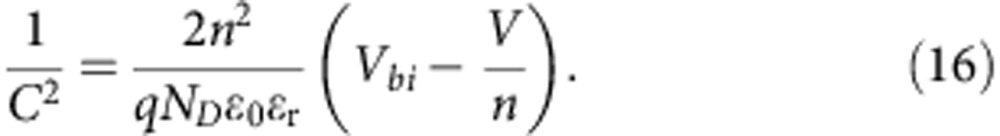graphic file with name ncomms4990-m20.jpg

The fit parameters here are εr and Vbi. The values of ideality factors n are taken from HRS in the I–V measurement. As shown in Supplementary Fig. 3, this linear model provides a good description only in the low negative voltage region. However, the extracted values and trends for εr and Vbi are fairly close to the ones deduced from the non-linear model. Consequently, the εr obtained with the linear model is a good approximation for the integrated effect of the depletion width capacitance, although in reality it has a non-uniform profile of εr14. These εr values are used in the main text for the purpose of quantifying the effect of trapped charges. Supplementary Table I summarizes the dielectric properties extracted from the fits of C–V data for all junctions, using both models outlined above. Supplementary Table II summarizes the Schottky barrier and state retention properties extracted from I–V measurements.

SIMS

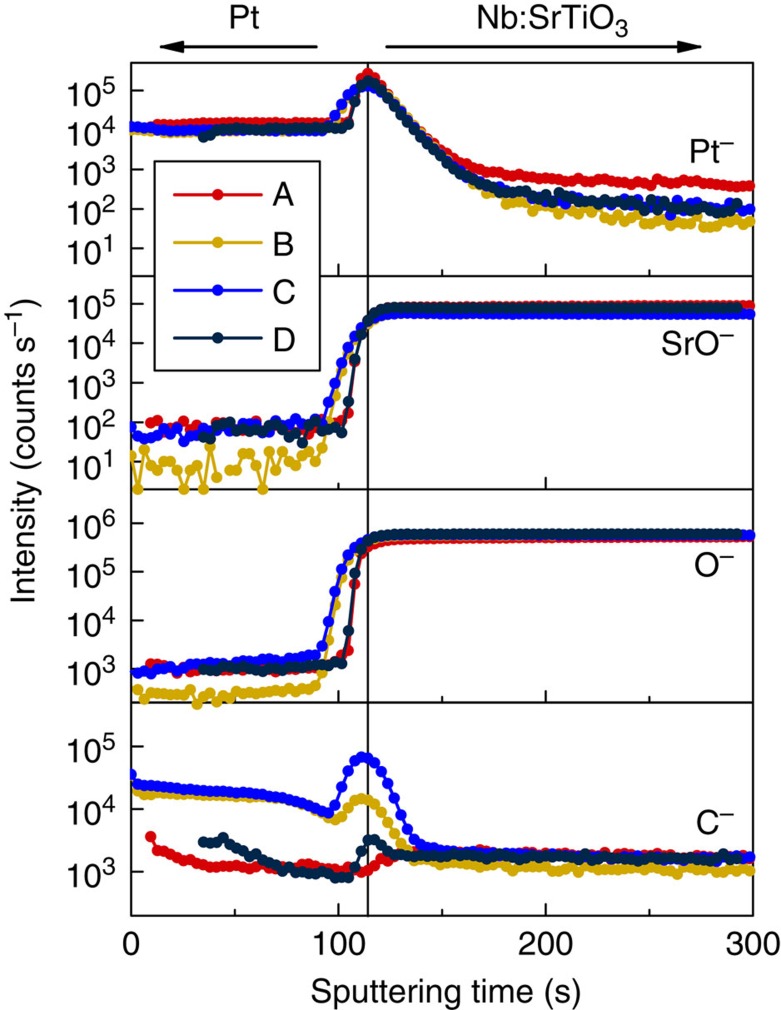
SIMS was performed using a Physical Electronics 6650 Quadrupole instrument (Physical Electronics, Chanhassen, MN). A 6 kV, 100 nA caesium primary ion beam with a spot size of 60 μm was rastered over a 300-μm-diameter area, and secondary ions were accepted from the centre 15 per cent of the rastered areas. A low-voltage electron beam was used for charge neutralization. Fig. 7 shows SIMS Pt, Sr, O and C profiles for all junctions. Sr and O show a sharp transition at the SrTiO3/Pt interface. Pt shows a rise in intensity at the interface, most likely associated with increased ionization yield in presence of oxygen sputtered from SrTiO3 at the interface. The magnitude of this rise is uniform across the studied sample series. The carbon profiles in samples B, C and D show increased intensity at the interface due to an unintentional interface contamination layers. It is unrelated to the increased Pt ionization yield near the interface. The absence of the carbon peak at the interface of sample A is consistent with the high quality of the interface, which was grown at 825 °C, which is likely sufficient to remove contamination layers.

Figure 7. Interface chemistry of all four samples.

Figure 7

Open in a new tab

Shown are the SIMS depth profiles of Pt−, SrO−, O− and C− for all samples. For samples B, C and D, a carbon peak is detected at the Pt/Nb:SrTiO3 interface.

Author contributions

E.M., B.D.H. and S.S. conceived and designed the experiments. E.M. and B.D.H. developed the device processing. E.M carried out the experiments and analysed the data. All authors discussed the results.

Additional information

How to cite this article: Mikheev, E. et al. Resistive switching and its suppression in Pt/Nb:SrTiO3 junctions. Nat. Commun. 5:3990 doi: 10.1038/ncomms4990 (2014).

Supplementary Material

Supplementary Information

Supplementary Figures 1-3 and Supplementary Tables 1-2

ncomms4990-s1.pdf (183.9KB, pdf)

Acknowledgments

We gratefully acknowledge Thomas Mates for help with the SIMS measurements. This work was supported by the Air Force Office of Scientific Research under a MURI grant (grantno. FA9550-12-1-0038). E.M. was also partially supported by the FAME Center, one of the six centres of STARnet, a Semiconductor Research Corporation program sponsored by MARCO and DARPA. The work made use of the UCSB Nanofabrication Facility, a part of the NSF-funded NNIN network.

References

  1. Sawa A. Resistive switching in transition metal oxides. Mater. Today 11, 28–36 (2008). [Google Scholar]
  2. Linn E., Rosezin R., Kugeler C. & Waser R. Complementary resistive switches for passive nanocrossbar memories. Nat. Mater. 9, 403–406 (2010). [DOI] [PubMed] [Google Scholar]
  3. Yang J. J. S., Strukov D. B. & Stewart D. R. Memristive devices for computing. Nat. Nanotechnol. 8, 13–24 (2013). [DOI] [PubMed] [Google Scholar]
  4. Zhirnov V. V., Meade R., Cavin R. K. & Sandhu G. Scaling limits of resistive memories. Nanotechnology 22, 254027 (2011). [DOI] [PubMed] [Google Scholar]
  5. Wong H. S. P. et al. Metal-Oxide RRAM. Proc. IEEE 100, 1951–1970 (2012). [Google Scholar]
  6. Karg S. F. et al. Transition-metal-oxide-based resistance-change memories. IBM J. Res. Dev. 52, 481–492 (2008). [Google Scholar]
  7. Fujii T. et al. Hysteretic current-voltage characteristics and resistance switching at an epitaxial oxide Schottky junction SrRuO3/SrTi0.99Nb0.01O3. Appl. Phys. Lett. 86, 012107 (2005). [Google Scholar]
  8. Fujii T. et al. Electrical properties and colossal electroresistance of heteroepitaxial SrRuO3/SrTi1-xNbxO3 (0.0002≤x≤0.02) Schottky junctions. Phys. Rev. B 75, 165101 (2007). [Google Scholar]
  9. Park C., Seo Y., Jung J. & Kim D. W. Electrode-dependent electrical properties of metal/Nb-doped SrTiO3 junctions. J. Appl. Phys. 103, 054106 (2008). [Google Scholar]
  10. Li J. Y., Ohashi N., Okushi H. & Haneda H. Temperature dependence of carrier transport and resistance switching in Pt/SrTi1-xNbxO3 Schottky junctions. Phys. Rev. B 83, 125317 (2011). [Google Scholar]
  11. Kan D. & Shimakawa Y. Transient behavior in Pt/Nb-doped SrTiO3 Schottky junctions. Appl. Phys. Lett. 103, 142910 (2013). [Google Scholar]
  12. Wang Y. H. et al. Investigation of the resistance switching in Au/SrTiO3:Nb heterojunctions. Appl. Phys. Lett. 103, 031601 (2013). [Google Scholar]
  13. Son J., Cagnon J. & Stemmer S. Strain relaxation in epitaxial Pt films on (001) SrTiO3. J. Appl. Phys. 106, 043525 (2009). [Google Scholar]
  14. Suzuki S. et al. Fabrication and characterization of Ba1-xKxBiO3/Nb-doped SrTiO3 all-oxide-type Schottky junctions. J. Appl. Phys. 81, 6830–6836 (1997). [Google Scholar]
  15. Card H. C. & Rhoderic E. h. Studies of tunnel MOS diodes I. Interface effects in silicon Schottky diodes. J. Phys. D 4, 1589 (1971). [Google Scholar]
  16. Shimizu T. & Okushi H. The properties of a metal/oxide semiconductor junction prepared using a high‐purity ozone surface treatment. Appl. Phys. Lett. 67, 1411–1413 (1995). [Google Scholar]
  17. Yamamoto T., Suzuki S., Kawaguchi K. & Takahashi K. Temperature dependence of the ideality factor of Ba1-xKxBiO3 Nb-doped SrTiO3 all-oxide-type Schottky junctions. Jpn J. Appl. Phys. Part 1 37, 4737–4746 (1998). [Google Scholar]
  18. Kim H., Park C., Lee S. & Kim D. W. Inhomogeneous barrier and hysteretic transport properties of Pt/SrTiO3 junctions. J. Phys. D 42, 055306 (2009). [Google Scholar]
  19. Lee E., Gwon M., Kim D. W. & Kim H. Resistance state-dependent barrier inhomogeneity and transport mechanisms in resistive-switching Pt/SrTiO3 junctions. Appl. Phys. Lett. 98, 132905 (2011). [Google Scholar]
  20. Shang D. S. et al. Electronic transport and colossal electroresistance in SrTiO3:Nb-based Schottky junctions. Appl. Phys. Lett. 94, 052105 (2009). [Google Scholar]
  21. Shang D. S., Sun J. R., Shi L. & Shen B. G. Photoresponse of the Schottky junction Au/SrTiO3: Nb in different resistive states. Appl. Phys. Lett. 93, 102106 (2008). [Google Scholar]
  22. Kahng D. & Wemple S. H. Measurement of nonlinear polarization of KTaO3 using Schottky diodes. J. Appl. Phys. 36, 2925–2929 (1965). [Google Scholar]
  23. Stengel M. & Spaldin N. A. Origin of the dielectric dead layer in nanoscale capacitors. Nature 443, 679–682 (2006). [DOI] [PubMed] [Google Scholar]
  24. Baniecki J. D. et al. Chemisorption of water and carbon dioxide on nanostructured BaTiO3-SrTiO3(001) surfaces. J. Appl. Phys. 106, 054109 (2009). [Google Scholar]
  25. Yoshimoto M., Ohkubo H., Kanda N. & Koinuma H. Two-dimensional epitaxial growth of SrTiO3 films on carbon-free clean surface of Nb-doped SrTiO3 substrate by laser molecular beam epitaxy. Jpn J. Appl. Phys. Part 1 31, 3664–3666 (1992). [Google Scholar]
  26. Jalan B., Cagnon J., Mates T. E. & Stemmer S. Analysis of carbon in SrTiO3 grown by hybrid molecular beam epitaxy. J. Vac. Sci. Technol. A 27, 1365–1368 (2009). [Google Scholar]
  27. Chen C. H., Hu E. L., Schoenfeld W. V. & Petroff P. M. Metallization-induced damage in III-V semiconductors. J. Vac. Sci. Technol. B 16, 3354–3358 (1998). [Google Scholar]
  28. Tung R. T. Recent advances in Schottky barrier concepts. Mater. Sci. Eng. Rep. 35, 1–138 (2001). [Google Scholar]
  29. Michaelson H. B. Work function of elements and its periodicity. J. Appl. Phys. 48, 4729–4733 (1977). [Google Scholar]
  30. Robertson J. & Chen C. W. Schottky barrier heights of tantalum oxide, barium strontium titanate, lead titanate, and strontium bismuth tantalate. Appl. Phys. Lett. 74, 1168–1170 (1999). [Google Scholar]
  31. Spicer W. E., Chye P. W., Skeath P. R., Su C. Y. & Lindau I. New and unified model for schottky-barrier and III-V insulator interface states formation. J. Vac. Sci. Technol. 16, 1422–1433 (1979). [Google Scholar]
  32. Spicer W. E., Lindau I., Skeath P. & Su C. Y. Unified defect model and beyond. J. Vac. Sci. Technol. 17, 1019–1027 (1980). [Google Scholar]
  33. Ohtomo A. & Hwang H. Y. Surface depletion in doped SrTiO3 thin films. Appl. Phys. Lett. 84, 1716–1718 (2004). [Google Scholar]
  34. Spinelli A., Torija M. A., Liu C., Jan C. & Leighton C. Electronic transport in doped SrTiO3: conduction mechanisms and potential applications. Phys. Rev. B 81, 155110 (2010). [Google Scholar]
  35. Yao Y. et al. In situ electron holography study of charge distribution in high-kappa charge-trapping memory. Nat. Commun. 4, 2764 (2013). [DOI] [PubMed] [Google Scholar]
  36. Zhang H. J., Zhang X. P., Shi J. P., Tian H. F. & Zhao Y. G. Effect of oxygen content and superconductivity on the nonvolatile resistive switching in YBa2Cu3O6+x/Nb-doped SrTiO3 heterojunctions. Appl. Phys. Lett. 94, 092111 (2009). [Google Scholar]
  37. Westerlund S. & Ekstam L. Capacitor theory. IEEE T. Dielect. El 1, 826–839 (1994). [Google Scholar]
  38. Zafar S., Callegari A., Gusev E. & Fischetti M. V. Charge trapping related threshold voltage instabilities in high permittivity gate dielectric stacks. J. Appl. Phys. 93, 9298–9303 (2003). [Google Scholar]
  39. Wolters D. R. & Vanderschoot J. J. Kinetics of charge trapping in dielectrics. J. Appl. Phys. 58, 831–837 (1985). [Google Scholar]
  40. Kollensperger P. A., Karl W. J., Ahmad M. M., Pike W. T. & Green M. Patterning of platinum (Pt) thin films by chemical wet etching in Aqua Regia. J. Micromech. Microeng. 22, 067001 (2012). [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information

Supplementary Figures 1-3 and Supplementary Tables 1-2

ncomms4990-s1.pdf (183.9KB, pdf)

Articles from Nature Communications are provided here courtesy of Nature Publishing Group

RESOURCES