Social learning in cooperative dilemmas

Abstract

Helping is a cornerstone of social organization and commonplace in human societies. A major challenge for the evolutionary sciences is to explain how cooperation is maintained in large populations with high levels of migration, conditions under which cooperators can be exploited by selfish individuals. Cultural group selection models posit that such large-scale cooperation evolves via selection acting on populations among which behavioural variation is maintained by the cultural transmission of cooperative norms. These models assume that individuals acquire cooperative strategies via social learning. This assumption remains empirically untested. Here, I test this by investigating whether individuals employ conformist or payoff-biased learning in public goods games conducted in 14 villages of a forager–horticulturist society, the Pahari Korwa of India. Individuals did not show a clear tendency to conform or to be payoff-biased and are highly variable in their use of social learning. This variation is partly explained by both individual and village characteristics. The tendency to conform decreases and to be payoff-biased increases as the value of the modal contribution increases. These findings suggest that the use of social learning in cooperative dilemmas is contingent on individuals' circumstances and environments, and question the existence of stably transmitted cultural norms of cooperation.

1. Introduction

A major challenge for the evolutionary sciences is to explain how cooperation is maintained in large populations with high levels of migration (henceforth referred to as large-scale cooperation). The evolution of cooperation requires mechanisms that allow cooperators to selectively interact with other cooperators; these include common ancestry [1], prior interaction [2] or assortment [3] between interacting individuals. However, the efficacy of these mechanisms is questionable in large populations with high levels of migration, where cooperators may therefore be vulnerable to exploitation by selfish defectors. Cultural group selection models posit that large-scale cooperation evolves via selection acting on populations among which behavioural variation is maintained by the cultural transmission of cooperative norms. In this case, shared cooperative norms ensure that individuals in the same population exhibit similar levels of cooperation. Thus, in these models, social learning at the individual level, via mechanisms such as conformity [4,5], has population-level consequences by maintaining stable, heritable behavioural variation between populations despite migration [4,6–8]. The behavioural variation between populations may then be subject to natural selection if populations with higher frequencies of cooperators outcompete those with lower frequencies; this process leads to the evolution of large-scale cooperation [4,9–13]. However, the key assumption that people acquire cooperative behavioural strategies via social learning remains empirically untested. Here, I test this assumption by examining whether individuals faced with a public goods dilemma employ social learning to make their decisions. Furthermore, I compare the prevalence of social learning across 14 populations to assess whether individuals vary in their use of social learning based on their characteristics and features of their environments. I find that overall individuals did not show a clear tendency to either conform or to be payoff-biased learners in a public goods dilemma, and that any tendency to do so depends considerably on their circumstances and the environment that they live in.

I focus on two social learning strategies, regarded as important for the evolution of large-scale cooperation due to their predicted population-level effects [4,12]. The first is conformity, the disproportionate tendency to acquire the behaviour exhibited with the highest frequency in a group of sampled individuals [4]. The second strategy is payoff-biased learning, the tendency to acquire behaviour that has produced the highest payoff or greatest success for an observed individual exhibiting the behaviour relative to other observed behaviours [4]. A large body of empirical work demonstrates the use of social learning in humans [5,14–16]. However, previous studies (reviewed in the electronic supplementary material, section 1) do not address whether individuals employ conformist and payoff-biased learning specifically in the context of a cooperative dilemma. Social learning is expected to be employed selectively in different task domains [17,18]. Hence, in order to test cultural group selection models of the evolution of large-scale cooperation, we need to determine whether humans specifically employ social learning to acquire behavioural strategies in the context of a cooperative dilemma.

I implemented public goods games (PGGs) experiments in 14 populations of a small-scale forager–horticulturist society, the Pahari Korwa living in India (see [19] for a description of the study populations). In each village, two rounds of an anonymous, one-shot PGG were played (see Material and methods for details of the experimental set-up). Participants were only informed that there would be a second round after they had played the first round. For each round, participants were divided into groups of six players. Each player received an endowment of 20 rupees and decided how much of it he/she wished to contribute to a group pot in divisions of 5 rupees. Once all six players had made their decisions, the total amount in the pot was doubled and then split equally between all six players. Each player's earnings consisted of the money he/she retained from his/her endowment plus an equal share of the earnings from the group pot. In this game, the income-maximizing strategy entails that a player contribute nothing to the group pot. Groups were reconstituted in round two so that a player's group composition in round two was different to his/her group composition in round one. Players were explicitly informed about the reconstitution of groups in round two and told that their group in round two would be different to their group from round one; all information and instructions about round two were provided only when round one had been completed. This process ensured that there were no repeated interactions between players.

The difference between the first (PGG1) and second (PGG2) round is that in the second round each player was presented two pieces of information prior to deciding how much he/she wished to contribute to his/her new group pot. Each player was told (i) the modal contribution (MC), i.e. the contribution made most frequently by the players in his/her group from round one and (ii) the highest earner's contribution (HEC), i.e. the contribution made by the player who had earned the highest amount in his/her group from round one. Note that the MC and HEC could only take on one of five values (0, 5, 10, 15 or 20) as player contributions were restricted to divisions of 5 rupees in both rounds. Once a player was told the MC and HEC for his/her group from round one, he/she decided how much of his/her new endowment he/she wished to contribute to his/her new group pot. Players were only informed of their earnings from each round at the end of both rounds. Hence, they did not know how much they or anyone else had earned in round one prior to making their decisions in round two.

To test whether individuals copied the MCs and HECs in round two, i.e. whether they employed conformist and/or payoff-biased learning, respectively, in making their PGG2 decisions, I compare the variance in PGG1 and PGG2 contributions. There is significant variation in PGG1 contributions between villages [19]. If individuals copied the MC and/or HEC, then we should expect the overall variance in contributions to be lower in round two compared with round one. However, as players were told the MCs and HECs only for their respective villages, we should also expect the variance of PGG2 contributions to increase between villages while it decreases within villages compared to the variance of PGG1 contributions. Thus, if players employed conformist and/or payoff-biased learning in the PGG2, we should expect a higher ratio of between-village to total (between-village and within-village) variance for PGG2 contributions, compared to PGG1 contributions. Although I did not provide players feedback about their earnings from the PGG1 before they made their decisions in the PGG2, they did have opportunities for individual learning from prior experience with the game structure, since they were playing the game for the second time in the PGG2 (albeit with different players). However, such individual learning is not expected to increase between-village variance in contributions, even though it may potentially decrease overall variance in contributions.

I also present more detailed analyses examining the extent to which individuals display a tendency to conform or to be payoff-biased. Following [20], a player's ‘tendency to conform’ is measured as the degree to which they changed their PGG2 contribution to be more similar to the MC and is calculated using the expression |PGG1 contribution—MC|—|PGG2 contribution—MC| (see Material and methods for details). The magnitude of the tendency to conform indicates the extent of the change. Positive values of the tendency to conform mean that a player's PGG2 contribution was closer to the MC than their PGG1 contribution, while negative values indicate the opposite. A value of zero indicates that relative to the MC, there was no change in the player's contribution across the two rounds. Similarly, a player's ‘tendency to be payoff-biased’ is calculated using the expression (PGG1 contribution—HEC)—(PGG2 contribution—HEC) and has the same attributes as the ‘tendency to conform’.

Note that since groups were reconstituted in round two and players were told the MC and HEC only for their respective groups from round one and not for the whole village, the experimental design minimized the chance that players who copied the MC or HEC did so out of any strategic considerations rather than simple social learning. Table 1 presents sample sizes of individuals who played both PGG1 and PGG2, for the 14 villages.

Table 1.

Number (n) of players (total n = 285) who played both PGG1 and PGG2 from each of 14 study villages. Mean age ± s.d. of participants was 34.59 ± 12.13 years and 46% were female.

village number	population sizea	percentage of migrants in sampleb	PGG players (n)
1	27	92 (12)	12
2	61	32 (22)	18
3	73	48 (21)	15
4	97	52 (31)	22
5	102	41 (32)	18
6	111	44 (36)	24
7	117	37 (30)	16
8	125	42 (38)	24
9	141	40 (30)	19
10	157	25 (44)	22
11	194	31 (42)	24
12	207	33 (43)	30
13	254	54 (39)	15
14	957	15 (47)	26

^aIncludes all adults and children residing in the focal village.

^bNumbers in parentheses indicate size of sample used to estimate the proportion of migrants. Migrants are individuals (Pahari Korwas) currently residing in the focal village but born in another village. Migration often follows marriage, particularly for females.

2. Material and methods

The following is a summary of the methods and analyses. Further details are provided in the electronic supplementary material.

(a). Public goods games

All games were played between 2 February and 16 May 2008. All games in most villages were administered on the third day after arrival in the village (the second day in two villages and the fourth day in one village) and completed in one day. All participants collected at a common location in the village on the day of the games. They were instructed about the game rules and examples both collectively and then individually at the private location where they played the game (scripts available upon request from the author). Players were tested both collectively and individually for their understanding of the game rules and of the anonymity of their decisions in each round of the game. Only a player who individually answered all test questions correctly played the game in round one. Only a player who had played in round one and answered all test questions for round two correctly played in round two. The full dataset from round one of the game (played in 16 villages) is presented in [19]. In all 14 villages where both rounds of the PGG were played, all individuals (n = 285) who understood the game rules in round one did so in round two; they therefore played in both rounds. Participants made their decisions in each round by manipulating real 5 rupee coins and depositing their contribution into a money box. In round two, prior to making their contribution decisions, players were informed about the HEC and the MC for their group from round one; this was done by placing 5 rupee coins summing to the relevant amount on the right and left side of the money box, respectively. Eleven of 49 round one groups across 14 villages generated two MCs. For players from these groups, I presented the MC that was most different to the HEC.

(b). Calculation of tendency to conform or be payoff-biased

In order to measure a player's tendency to conform I calculated two quantities. First, the absolute difference between the player's round one contribution and the MC, representing deviation from the MC before social learning. Second, I calculated the absolute difference between the player's round two contribution and the MC, representing deviation from the MC after social learning. The second difference was subtracted from the first difference to obtain the degree to which the deviation between a player and the MC changed after social learning [|PGG1 contribution—MC|—|PGG2 contribution—MC|]; this is defined as the tendency to conform. The tendency to be payoff-biased was similarly measured as the degree to which the deviation between a player and the HEC changed after social learning. However, in this case, I simply calculated the difference between the deviations from the HEC before and after social learning and not the difference between the absolute deviations [(PGG1 contribution—HEC)—(PGG2 contribution—HEC)]. According to this formulation, players whose PGG2 contributions were even lower than the HEC have positive values for the ‘tendency to be payoff-biased’ as they are following the general logic of payoff-maximization, which is central to a payoff-biased learning strategy.

(c). Statistical analyses

One hundred and fifty-four out of a total 285 players had a PGG1 contribution that was equal to the MC, and 95 out of 285 had a PGG1 contribution that was equal to the HEC. These individuals were already coordinated with the MC or HEC, respectively, even before they knew what this was; in other words, they gave rise to the MC or HEC in round one. Hence, explicitly finding out the value of the MC and HEC in round two provided very different information to them compared with individuals who were not coordinated with the MC and HEC already. For the coordinated individuals, the decision in round two was not whether they should move closer to or further away from the MC or HEC but rather whether they should stay coordinated with these or not. Since the decision structure faced by these individuals was completely different to that faced by individuals who were not already coordinated with the MC or HEC in round one, I analysed these two sets of individuals separately. Hence, I present two sets of analyses investigating the variation in and descriptors associated with a tendency to conform: (i) for individuals coordinated with the MC in round one and (ii) for individuals not coordinated with the MC in round one. Similarly, I present two sets of analyses investigating the variation in and descriptors associated with a tendency to be payoff-biased: (i) for individuals coordinated with the HEC in round one and (ii) for individuals not coordinated with the HEC in round one.

Non-parametric statistics were used to analyse the distribution of different learning strategies pooled across villages and to compare player PGG1 and PGG2 contributions. Multilevel normal linear models [21] were used to explicitly analyse variation in PGG2 contributions at the village and individual levels as well as to analyse variation in the tendency to conform and to be payoff-biased and the association of population and individual descriptors with this variation. Individuals (level 1) were nested within villages (level 2). All multilevel analyses were conducted in MLwiN, v. 2.14 [22]. I mainly use an information-theoretic model-fitting approach [23] to analyse data and interpret results. Analyses proceeded in four stages (details provided in the electronic supplementary material, section 2.5.1) and included a series of domain-wise (sets of related variables, such as those measuring wealth and kin, as described in the electronic supplementary material, section 2.5.1) models in view of the large number of variables analysed and the potential correlations between them.

3. Results

(a). Do individuals use information on the modal contribution and the highest earner's contribution in making their PGG2 contributions?

Total variance in PGG2 contributions is significantly lower than that in PGG1 contributions, but a significantly larger proportion of the total variance occurs between villages in PGG2 contributions when compared to PGG1 contributions (table 2 summarizes variance components for PGG1 and PGG2 contributions). Two per cent of the variance in PGG1 contributions is between villages when compared to 9.4% in PGG2 contributions across the same 14 villages (table 2; electronic supplementary material, figure S1). Hence, the proportion of between-village variance in contributions increased by 7.4% between the PGG1 and the PGG2. Variances of the absolute values of village level residuals differ significantly for PGG1 and PGG2 contributions (table 2). These results suggest that some individuals did use information on the MC and HEC in making their PGG2 contributions (recall that MC refers to the contribution made most frequently by the players in a group from round one, and HEC refers to the contribution made by the player who had earned the highest amount in his/her group from round one).

Table 2.

Null model (intercept only) variance components for PGG1 and PGG2 contributions. Variances of the absolute values of village level residuals differ significantly for PGG1 and PGG2 contributions (Levene's test for equality of variances: F = 7.397, p = 0.011).

game	variance ± s.d.			VPCa ± s.d.
	village level	individual level	total
PGG1	0.603 ± 1.006	29.341 ± 2.548	29.944	0.020 ± 0.031
PGG2	2.132 ± 1.745	19.730 ± 1.777	21.862	0.094 ± 0.066

^aVPC = village level variance/(village level variance + individual level variance).

The overall distributions of PGG1 and PGG2 contributions pooled across all villages are significantly different (Wilcoxon signed ranks test: Z = −2.143, n = 285, Monte Carlo simulated p = 0.032). Players made smaller contributions in the PGG2 (mean ± s.d. = 9.81 ± 4.60) than they did in the PGG1 (mean ± s.d. = 10.51 ± 5.44).

(b). Do individuals demonstrate a tendency to conform when they are not coordinated with the modal contribution?

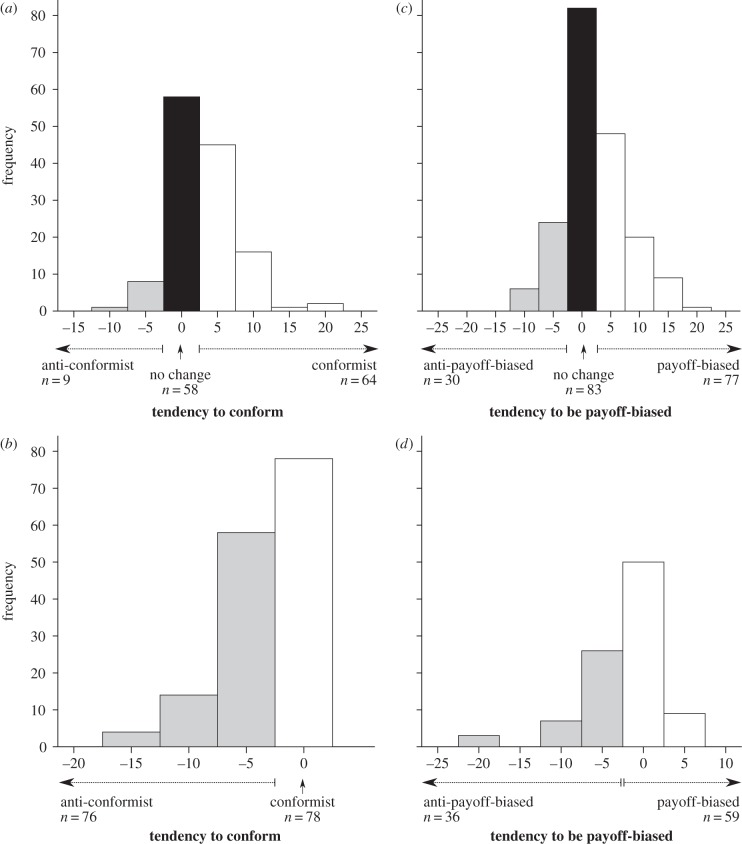
There is significant variation in the tendency to conform for individuals who were not coordinated with the MC in round one (figure 1a; electronic supplementary material table S1). Forty-four per cent of individuals did not change their contribution between rounds one and two compared to 49% who moved towards the MC and 7% who moved away from it (figure 1a). Pair-wise tests demonstrate that the number of players who moved towards the MC does not differ significantly from the number of players who did not change the value of their contribution between rounds one and two (electronic supplementary material, Table S1). However, the number of individuals who moved away from the MC is significantly lower than the number of individuals who did not change their contribution and is also lower than the number of players who moved towards the MC (electronic supplementary material, table S1). In summary, individuals do not show a clear tendency to move towards the MC but also do not move away from it.

Figure 1.

Frequencies of players' tendency to conform pooled across 14 villages for individuals who were (a) not coordinated with the MC in round one and (b) coordinated with the MC in round one. Frequencies of players' tendency to be payoff-biased pooled across 14 villages for individuals who were (c) not coordinated with the HEC in round one and (d) coordinated with the HEC in round one. The tendency to conform is measured as the degree to which players' PGG2 contributions shifted towards or away from the MC using the expression |(PGG1 contribution—MC)|—|(PGG2 contribution—MC)|. The tendency to be payoff-biased is measured as the degree to which players' PGG2 contributions shifted towards or away from the HEC using the expression (PGG1 contribution—HEC)—(PGG2 contribution—HEC). For figures (a,b), grey bars represent anti-conformists, and black bars represent individuals who did not change relative to the MC and white bars represent conformists. For figures (c,d), grey bars represent anti-payoff-biased individuals, and black bars represent individuals who did not change relative to the HEC and white bars represent payoff-biased individuals.

The tendency to conform does not vary significantly across the 14 villages. The DIC value for the null model with village-level intercepts (multilevel) is barely different from that for the null model without village-level intercepts (single level), indicating that the multilevel model accounting for village effects does not provide a better fit to the data (electronic supplementary material, table S2; null models).

Two variables are significantly associated with the tendency to conform, namely the number of people from the focal village that an individual invited to wine and dine at his/her home for the harvest festival (Cherta) held in the year of the study, and whether the individual's father is living in the village or not (electronic supplementary material, table S2; full model (multilevel)). An individual's PGG2 contribution shifts towards the MC by 1 rupee for every 10 people she/he invited home for the harvest festival. Individuals’ PGG2 contributions move away from the MC by about 4 rupees if their father is living in the village. Hence, players are more likely to conform if their social networks are larger and less likely to do so if their father is living in the village.

(c). Do individuals demonstrate a tendency to conform when they are already coordinated with the modal contribution?

Individuals who were already coordinated with the MC in round one could either choose to stay coordinated with it or move away from it. Fifty-one per cent of individuals stayed coordinated with the MC and their number did not differ significantly from the 49% of players who moved away from it (figure 1b; electronic supplementary material, table S1). Hence, individuals do not show a clear tendency to stay coordinated with the MC and an approximately equal number of individuals actively moved away from it.

The tendency to stay coordinated does not vary significantly across the 14 villages (electronic supplementary material, table S3; null models). Two variables are significantly associated with the tendency to conform, namely the number of monthly visits an individual makes to the nearest town and the value of the MC (electronic supplementary material, table S3; full model (multilevel)). An individual's PGG2 contribution shifts away from the MC by over 1.5 rupees for every additional visit that s/he makes to town. Individuals’ PGG2 contributions move away from the MC by about 4 rupees for every 10 rupee increase in the value of the MC. Hence, players are less likely to conform as the number of visits they make to town increases or the value of the MC increases.

(d). Do individuals demonstrate a tendency to be payoff-biased when they are not coordinated with the highest earner's contribution?

There is significant variation in the tendency to be payoff-biased for individuals who were not coordinated with the HEC in round one (figure 1c; electronic supplementary material table S1). Forty-four per cent of individuals did not change their contribution between rounds one and two compared to 40% who lowered their contributions to move towards or even lower than the HEC and 16% who raised their contributions to move away from it (figure 1c). Pair-wise tests demonstrate that the number of players who moved towards the HEC does not differ significantly from the number of players who did not change the value of their contribution between rounds one and two (electronic supplementary material, table S1). However, the number of individuals who moved away from the HEC is significantly lower than the number of individuals who did not change their contribution and is also lower than the number of players who moved towards the HEC (electronic supplementary material, table S1). In summary, individuals do not show a clear tendency to move towards the HEC but also do not move away from it.

The tendency to be payoff-biased does not vary significantly across the 14 villages (electronic supplementary material, table S4; null models). Two variables are significantly associated with the tendency to be payoff-biased, namely the value of the MC and the value of the HEC (electronic supplementary material, table S4; full model (multilevel)). An individual's PGG2 contribution shifts towards the HEC by about 3 rupees for every 10 rupee increase in the value of the MC and by about 5 rupees for every 10 rupee increase in the value of the HEC. Hence, players are more likely to be payoff-biased as the value of the MC and HEC increases.

(e). Do individuals demonstrate a tendency to be payoff-biased when they are already coordinated with the highest earner's contribution?

Individuals who were already coordinated with the HEC in round one could choose to stay coordinated with it, raise their contributions from the HEC or lower their contributions from the HEC. Fifty-three per cent of individuals stayed coordinated with the HEC compared to 38% who raised their contributions and 9% who lowered their contributions (figure 1d). The number of players who were payoff-biased (62%), i.e. stayed coordinated with the HEC or lowered their contributions, is significantly greater than the number of players who were not (38%), i.e. raised their contributions from the HEC (electronic supplementary material, table S1). Hence, individuals show a clear tendency towards payoff-biased behaviour by either staying coordinated with the HEC or lowering their contributions even further.

The tendency to stay coordinated with the HEC varies significantly across the 14 villages (electronic supplementary material, figure S2). Fifty-one per cent of the variation in the tendency to stay coordinated with the HEC occurs between villages. The DIC value for the null model with village-level intercepts (multilevel) is 37 units lower than that for the null model without village-level intercepts (single level), indicating that the multilevel model accounting for village effects provides a much better fit to the data (electronic supplementary material, table S5; null models). Once village and individual descriptors are included in the full model, the unexplained between-village variance in the tendency of staying coordinated with the HEC decreases to 48% (electronic supplementary material, table S5; full model (multilevel)).

Five variables are significantly associated with the tendency to be payoff-biased, namely sex, the percentage of the village population who were not Pahari Korwas, education, household size and the value of the HEC (electronic supplementary material, table S5; full model (multilevel)). Women tend to move about 2 rupees closer to the HEC than men do on average. Individuals tend to raise their contributions from the HEC by 0.7 rupees for every 10% increase in the percentage of non-Korwas in the village. Note that non-Korwas did not participate in the games in any village. Education has a nonlinear effect such that although literate individuals tend to raise their contributions by about 0.8 rupees away from the HEC, compared to illiterate individuals, those who have had some schooling tend to lower their contributions and are payoff-biased. Both household size and the value of the HEC show a positive association with the tendency to be payoff-biased; individuals lower their contributions by about 0.3 rupees for every additional person in their household and by about 0.4 rupees for every 1 rupee increase in the value of the HEC. Hence, players are more likely to be payoff-biased when they are women, have had some schooling, have larger households or the value of the HEC is higher. They are less likely to be payoff-biased if they are literate but not schooled and as the percentage of people from other ethnicities residing in their village increases.

(f). Are individuals’ PGG1 contributions associated with their tendency to conform or to be payoff-biased?

There is no significant relationship between PGG1 contributions and the tendency to conform for individuals who were not coordinated with the MC in round one (Spearman's ρ_{MC not coordinated} = 0.073, p = 0.41). In contrast, there is a significant negative relationship between PGG1 contributions and the tendency to conform for individuals who were coordinated with the MC in round one (Spearman's ρ_{MC coordinated} = −0.303, p < 0.01). PGG1 contributions are positively associated with the tendency to be payoff-biased for both individuals who were not coordinated with the HEC and those who were (Spearman's ρ_{HEC not coordinated} = 0.631, p < 0.01; Spearman's ρ_{HEC coordinated} = 0.356, p < 0.01).

4. Discussion

(a). Evidence for social learning in a cooperative dilemma

The ratio of between-village to total (between-village and within-village) variance in contributions increased significantly by 7.4% in round two compared to round one of the PGG. In other words, individuals were behaviourally more similar within villages and less similar between villages in round two of the PGG. These results suggest that at least some individuals used the information that was provided in round two about the behaviour of other players from their respective villages. I therefore infer that some individuals did employ social learning in making decisions in a cooperative dilemma.

However, more detailed analyses examining individuals' tendency to conform or to be payoff-biased suggest that individuals employ social learning to a very limited extent. Individuals do not display a clear tendency to either move towards the MC or to stay coordinated with it; about half of the individuals who were already coordinated with the MC in round one, moved away from it in round two. Individuals also do not show a clear tendency to move towards the HEC, but those who were already coordinated with it in round one did tend to either stay coordinated with it or lower their contributions even further. Hence, there is little evidence that individuals are conformist but some suggestion that they may be payoff-biased. It remains a possibility that individuals use social learning strategies other than the ones investigated in this study.

(b). Correlates of learning strategies

Properties of both individuals and villages are associated with the tendency to conform and to be payoff-biased. Individuals are more likely to conform if their social networks are larger. They are less likely to conform if their father is living in the village, as the number of visits they make to town increases or as the value of the MC increases. Individuals are more likely to be payoff-biased as the value of the MC and HEC increases, if they are women, have had some schooling or have larger households. They are less likely to be payoff-biased if they are literate but not schooled and as the percentage of people from other ethnicities residing in their village increases.

Large social networks may allow individuals to sample a large proportion of the population and obtain more accurate information about what others are doing, thereby making social learning more effective. At the same time, if fathers are important models of socially learned behaviour in these patrilineal populations, then a co-resident father may make individuals less sensitive to the behaviour of the majority. This might explain the positive association between conformity and social network size and its negative association with a co-resident father. Admittedly, these are speculations to guide future research but they highlight the idea that the extent to which individuals can sample the behaviour of others, and the composition of their sample, may have major effects on the degree of conformity and its effectiveness in a population.

Individuals are less likely to conform as the number of visits they make to town increases. Visits to towns may provide people increased opportunities for individual learning especially in the context of economic exchange. Hence, individuals who frequently visit towns may feel less of a need to rely on social information and conform to the behaviour of others. Greater experience with economic exchanges may even make them more sensitive to the costs and benefits of cooperation itself and therefore to the use of social learning within the context of cooperative dilemmas. Some authors have argued that the integration of individuals into market exchange economies should accelerate the spread of cooperative norms [24,25]; my finding questions their hypothesis.

It is notable that players' tendency to conform decreases and their tendency to be payoff-biased increases as the actual value of the MC increases, indicating that the conformist strategy may be sensitive to the cost of conformity; individuals may avoid conforming if it is too expensive to do so, especially in the context of a cooperative dilemma. A study on conformity in a perceptual task reported similar results—individuals' tendency to copy the majority decreased when incentives to make accurate judgements were introduced [26]. It is, however, puzzling that individuals are more likely to remain payoff-biased as the HEC increases; if payoff copiers are motivated by a desire to increase pay-offs, then they should favour lower HEC values which, given the structure of the PGG, will provide higher pay-offs. Nonetheless, these results raise the possibility that conformist and payoff-biased learning is conditional on the cost incurred by adopting a trait. This idea is bolstered by the finding that the tendency to be payoff-biased varies across villages and increases for individuals with larger households who presumably have to provision more people; cooperation may be more costly for these individuals making them more pay-off focused in cooperative dilemmas. A previous study found that proposer offers in the ultimatum game were also negatively associated with household size in the same populations [27].

Together, my results support the idea that individuals' learning strategies are sensitive to the relative costs of individual versus social learning and the relative likelihood that each of these strategies will result in the adoption of the optimal behaviour in different environments. Certainly, they suggest that people do not use a single learning strategy across all environments and irrespective of their circumstances even when performing the same task. The learning strategy used should depend on many factors, including, among others, the task domain [17,18,28], the availability of information about the choice of cultural/behavioural variants, the number of different variants available [17] and the rate of environmental change [7,29–31]. However, in cultural group selection models of cooperation [4,10,12,13,32] the use of conformity is not assumed to be flexible. Rather it is assumed to be an all-purpose learning strategy that individuals employ across task-domains, even though it leads to the acquisition of sub-optimal behaviour in some domains; the argument made is that conformity is advantageous to individuals averaged across several domains and its averaged benefit across domains mitigates the costs incurred on account of it in some domains (e.g. in cooperative dilemmas). My findings suggest that the latter assumption of a fixed all-purpose conformist strategy that is co-opted to the cooperative task domain is empirically unsupported and should be revised.

(c). The impact of social learning on cooperation

Models of cultural evolution assume that people acquire cooperative behavioural strategies via social learning and that this process leads to the stability of cooperative norms [4,12]. I find no evidence that social learning leads to the acquisition or maintenance of more cooperative behaviour. Individuals displayed a lower tendency to conform and a higher tendency to be payoff-biased as the value of the MC increased suggesting that individuals are less likely to acquire increasingly cooperative behavioural strategies via social learning. Concurrently, individuals who made large contributions in round one had a lower tendency to conform and a higher tendency to be payoff-biased in round two and, overall, players made smaller contributions in round two compared with round one.

(d). Cooperative norms

I do not find evidence for a clear tendency towards either conformity or payoff-biased learning in the context of a cooperative dilemma. Moreover, whether or not individuals use social learning in a cooperative dilemma is contingent on their circumstances and the environment that they live in. Theoretical work is required to clarify whether these low levels of social learning can maintain the stable between-population behavioural differences that are essential for selection at the group-level, despite the high rates of migration between my study villages (table 1).

Crucially, the extent to which behavioural variation between populations is maintained by social learning depends on the likelihood with which social learners selectively sample the behaviour of only those residing in their population. If individuals are more likely to sample behaviour across populations, then even high levels of social learning would in fact reduce behavioural variation between populations. Empirical work is required to establish whether individuals are more likely to learn from co-residents of the same population or not.

It is also worth noting that demographic factors such as migration [4,33,34] and population size [35] are expected to inversely affect the prevalence of social learning in populations; the same factors, i.e. large populations and high rates of migration, impede the evolution of cooperation [12,36]. Hence, while cultural group selection models invoke social learning to explain the evolution of cooperation in large populations with high rates of migration, theory predicts that social learning itself is less likely to be employed in such populations.

My finding that individuals' learning strategies are sensitive to the costs of social learning, combined with the intrinsically costly nature of cooperation, questions the existence of stably transmitted cultural norms of cooperation. We must not assume that modal or average behaviour represents a culturally transmitted norm in human populations, just as we do not do so when studying the behaviour of other animals. More generally, these findings emphasize the critical constraints placed by the environment on the degree to which traits are culturally transmitted.

This study has full approval from the Ethics Committee at University College London, and informed consent was obtained from all participants. The data are available from the author upon request.

Funding statement

The Cogito Foundation provided financial support for this study. Many thanks to the Wissenschaftskolleg zu Berlin for support during the writing of this paper.