Sex-biased survival predicts adult sex ratio variation in wild birds

Abstract

Adult sex ratio (ASR) is a central concept in population demography and breeding system evolution, and has implications for population viability and biodiversity conservation. ASR exhibits immense interspecific variation in wild populations, although the causes of this variation have remained elusive. Using phylogenetic analyses of 187 avian species from 59 families, we show that neither hatching sex ratios nor fledging sex ratios correlate with ASR. However, sex-biased adult mortality is a significant predictor of ASR, and this relationship is robust to 100 alternative phylogenetic hypotheses, and potential ecological and life-history confounds. A significant component of adult mortality bias is sexual selection acting on males, whereas increased reproductive output predicts higher mortality in females. These results provide the most comprehensive insights into ASR variation to date, and suggest that ASR is an outcome of selective processes operating differentially on adult males and females. Therefore, revealing the causes of ASR variation in wild populations is essential for understanding breeding systems and population dynamics.

1. Introduction

Adult sex ratio (ASR; the ratio of adult males to adult females in a population) is a fundamental variable in demography and population biology [1–3]. Functionally, ASR plays a major role in influencing mating competition, sex roles, mating systems and parental care across animals [4–6] including humans, as indicated by recent studies showing that ASR influences mate choice, and predicts economic behaviour, divorce rates, extra-marital affairs, rape and violence [7–10]. Therefore, determining causes and implications of ASR variations in wild animal populations provides important comparative insights for a comprehensive understanding of the evolutionary processes affecting social behaviour of animals and humans [11,12], as well as their implications for population demography, biodiversity conservation and health.

ASR varies widely among species, and theoretical, experimental and comparative studies suggest that variations in ASRs impact behaviour, ecologies and life histories [11–13]. For example, at male-biased ASR, rates of aggression increase; males harass females, which in turn induces increased female mortality [14]. Moreover, in species with male-biased ASR, courtship behaviour and male–male competition intensify [15,16], and/or males are more likely to provide care for their young than at female-biased ASRs [5,13,17]. Furthermore, ASR is a significant predictor of sex roles: birds with female-biased (or even) ASR typically exhibit conventional sex roles whereby males compete for females and females look after the young, whereas species with male-biased ASR often exhibit sex role reversal: males care for the young, whereas females compete for access to males [13]. These results, together with experimental manipulation of ASR [18,19], provide a convincing case that sex ratios influence mate choice behaviour. Nevertheless, while ASR is an important predictor of various aspects of social behaviour, mating competition and breeding systems, the causes of ASR variation remain poorly understood in wild populations [6,11,20].

Variation in ASR is due to four mutually non-exclusive factors [11,21]: variation in sex ratios at conception or at birth, sex difference in survival of juveniles and/or adults, different maturation time of males and females, and sex bias in dispersal movements of young or adults (e.g. emigration and immigration). First, biased ASR may emerge as a consequence of biased juvenile sex ratios. Sex ratios may already be biased at conception (primary SR), or at birth or hatching (secondary SR), or male and female juveniles may die at different rates [17,21–23]. Second, differential adult survival may bias ASR, because one sex may be predated more often than the other [24,25], or be more susceptible to parasites and pathogens [26]. Consistent with the latter argument, adult survival is sex-biased in a number of fishes, birds and mammals [27,28]. Third, maturation often depends on growth rates, and if one sex is larger than the other, the larger sex may take longer to mature, for instance in sexually dimorphic copepods, raptors or mammals [25,27]. This difference also influences the time each sex spends as adults. Fourth, emigration and immigration are often sex-specific: for instance in birds, males tend to stay in the natal territory and females disperse, whereas in mammals the reverse tends to be the case [29]. Note, however, that differential immigration and emigration only influence local ASR, whereas at the meta-population level their sex-specific effects are expected to cancel out.

Here, we provide the first comparative evidence that ASR correlates with adult mortality bias using data from 187 bird species from 59 avian families in phylogenetically controlled analyses [30,31]. Specifically, we test whether biased ASRs emerge via different sex ratios at birth, differential juvenile (fledgling) sex ratios and/or sex-specific adult survival. We then further investigate the causes of sex-specific adult survival and test three major hypotheses: mating competition may lead to increased mortality of the competing sex; the cost of parental care might be sex-biased, leading to higher mortality of the sex that provides more care; and higher direct costs of reproduction (e.g. egg production) may increase female mortality [17,22,28].

2. Material and methods

(a). Data collection

We collected ASR data from published sources by extensively searching reference works (e.g. Birds of Western Palearctic and Birds of North America) and the primary literature through the Web of Knowledge (using keywords like ‘bird*’, 'avian’, and English and Latin names of specific taxa, in combination with ‘sex ratio*’ or ‘ASR’). We did not restrict search to any avian taxon; nor did we prefer certain taxa over others, except to maximize the completedness of the dataset with regard to the research hypotheses. Thus we only searched for a species if other key variables (e.g. offspring sex ratio and sex-specific adult mortality) were already available for that species. The final number of species (187; see below) closely matches the number of species in an ASR dataset gathered independently by Donald [20] (173 species—data not publicly available). Frequency distributions of ASR suggest that these two datasets are very similar (e.g. compare our electronic supplementary material, figure S1 to fig. 2 in [20]). Therefore, we are confident that our dataset reflects the information currently available for birds.

We express ASR as the proportion of adult males in the population, the number of adult males/(number of adult males + number of adult females). When several estimates were available for a species (e.g. from different years or from different studies) we used their mean value. Data from different populations were only retained for calculations of repeatability (see below). In intensively studied bird populations, ASR was often based on censuses of individually marked breeding adults. We also included ASR estimates from studies using a variety of other methods, like capturing birds (both breeding and non-breeding), counting dead birds (e.g. killed by storms) or demographic studies [2,32]. We showed previously that using ASRs gathered by different methods provide consistent results [13,33]. In three species we used the estimate that was least likely to be influenced by anthropogenic effects such as habitat loss [34–36]. We excluded ASR estimates that were likely to be based on biased sampling, such as hunting bags of ducks [37]; instead, we used pre-breeding or breeding season counts.

For most populations, ASR was provided by the original source, although for 14 species we calculated ASR using the data provided in the original sources (e.g. from tables or figures presenting the number of adult males and females). Our final dataset includes 187 species, of which 132 species also had data on offspring sex ratio and/or sex-specific adult survival. ASRs were arcsine-square-root-transformed before analysis. The full dataset, together with their sources, is provided in the electronic supplementary material, table S1. As ASR estimates are often criticized on the basis that they are prone to sampling error [20], we also report the consistency of ASR estimates between studies of a given species (see below). Note that the latter studies were usually carried out in different populations and used different methodologies, so they are not repeatabilities in the strict sense.

Hatching and fledging sex ratios were collected from [38] and augmented by an extensive search in the primary literature. In birds, the major life-history stages (i.e. hatchling, fledging and adult) are usually straightforward to distinguish by different plumage patterns. The majority of these data on hatching and fledging sex ratios (proportion of males in all sexed offspring) were obtained using molecular methods, although in a few studies offspring were sexed morphologically. We divided offspring sex ratios into two groups: (i) hatching sex ratios refer to both freshly hatched chicks and unhatched eggs with embryos if data on the latter were available; and (ii) fledging sex ratios refer to chicks that survived to a certain age, usually close to (or at) fledging. Fledging sex ratios usually refer to chicks just before they actually fledge (or leave the nest) in most species, because of the difficulty of capturing chicks around the time of fledging (in precocial birds), or to avoid premature leaving of the nests caused by handling (in altricial birds).

Fledging sex ratios therefore reflect both primary and secondary sex ratios as moulded by sex-specific mortalities of male and female hatchlings. Following [38], we excluded data from experimental studies when the treatments aimed to influence offspring sex ratios, and we also did not include estimates from captive (aviary) studies. When several offspring sex ratio estimates were available for a species, for example from different years or different populations, we used their mean value. As with ASR, offspring sex ratios were arcsine-square-root-transformed prior to the analyses. All data and their sources are provided in the electronic supplementary material, table S1.

To obtain data on sex-specific annual mortalities, we augmented the data assembled by Liker & Székely [28] with more recent estimates. We used data from field studies in which mortality rates for both adult males and adult females were estimated in the same population and by the same method. Three main methods were used to estimate mortality rates: capture–recapture, ringing recoveries and local return rates. We intended to use the best data available, and we are not aware of any systematic bias in these estimates that would undermine the tested hypotheses. Sex differences in probabilities of recaptures may potentially influence the latter two estimates, although this bias is unlikely because recapture probabilities are not consistently different between adult male and female birds [28,39]. When several mortality estimates were available for a species, we used the ones that were based on capture–recapture analyses, larger samples and/or longer study periods. We always recorded sex-specific mortalities as given in the primary source, even if the difference between the sexes was not statistically significant (for a similar approach, see [39]). When separate sex-specific mortalities were not reported in the original source and mortalities were not statistically different between the sexes, we used the same mortality estimates for both sexes. We express sex bias in mortality as log(adult female mortality/adult male mortality); thus, we expect a positive correlation with ASR if mortality bias predicts ASR bias. Our dataset includes mortality estimates for 117 species that also have ASR data (see below).

To measure the relationship between mortality and the intensity of sexual competition, we followed previous studies and scored the frequency of polygamy in both sexes on a five-point scale (0–4; see rationale in [13,28]), with 0 corresponding to no (or very rare) polygamy (less than 0.1% of individuals), 1 to rare polygamy (0.1–1%), 2 to uncommon polygamy (1–5%), 3 to moderate polygamy (5–20%) and 4 to common polygamy (more than 20%). When the frequency of polygamy was not provided in the original source but a sufficient description of the species’s mating system was available, we estimated mating system using verbal descriptions of mating behaviour and pair-bonds. Scoring was a necessity, rather than a preference, to include as many species as possible in the analyses. The polygamy scores were significantly repeatable between two independent observers (intraclass correlation, r_ICC = 0.914, F = 22.2, p < 0.001, n = 28 species). Mating system bias was then calculated as the difference between the male and female mating scores, consistently with previous studies [13,33].

Male involvement in paternal care relative to female provisioning was scored in six types of care behaviour (nest building, incubation, nest guarding, chick brooding, chick feeding and chick guarding; see rationale in [40]). We scored male participation in each of these activities (0: no male care; 1: 1–33% male care; 2: 34–66% male care; 3: 67–99% male care; 4: 100% male care). Pre-hatching care was calculated as the average score of nest building, incubation and nest guarding, whereas post-hatching care was expressed as the average score of chick brooding, chick feeding and chick guarding (for a similar approach, see [28,40,41]).

Body size, sexual size dimorphism (SSD) and offspring developmental mode potentially confound relationships between demographic variables (such as mortality estimates and offspring sex ratios) and ASR [13,33]. In addition, body size may influence detectabilities, and thus ASR estimates; for instance, the larger sex may be more conspicuous [11,20]. Therefore, we also collected data on body mass, SSD and offspring development, and included these potentially confounding variables in phylogenetically corrected multiple regressions (see similar approaches in [13,28]). Body mass was included as the log-transformed mean body mass (in grams) of adult males and adult females, and SSD was estimated as log(adult male mass/adult female mass) [42]. Offspring development was categorized as (i) altricial, (ii) semi-precocial or semi-altricial, and (iii) precocial, based upon avian developmental modes [43,44]. Female reproductive output was estimated as average clutch size × fresh egg mass (in grams) and was log-transformed. We provide the mean ± s.e., unless otherwise stated. All data and their sources are provided in the electronic supplementary material, table S1.

(b). Phylogenetic comparative analyses

We used phylogenetic generalized least squares (PGLS) with maximum likelihood to find the best fitting λ [30,31]. To represent the phylogenetic relationships between species, we used the most recent comprehensive avian phylogeny [45] that included all but one species in our dataset. Following a recent molecular phylogeny [46], we added Charadrius nivosus to these trees as a sister taxon of Charadrius alexandrinus. To test the sensitivity of results to the phylogenetic hypotheses, we used 100 randomly extracted phylogenetic trees from the 10 000 alternative avian phylogenies (http://birdtree.org). All phylogenetic trees were fully resolved (i.e. had no polytomy), and included branch lengths (see [45] for details). From the PGLS models used with 100 trees, we calculated the mean slope of the phylogenetic regressions and mean two-tailed significance levels. Owing to missing data in one or more variables for a given species, we use different models to investigate the effects of potential confounds. We calculated the variance inflation factor (VIF) for all models (tables 1–3), although VIF was less than 5 for most models, suggesting that multi-collinearity may not inflate the results. All analyses were carried out in R (v. 2.15.2), using the packages ‘ape’ and ‘caper’ [47].

Table 1.

Adult sex ratio (ASR) in relation to hatching and fledging sex ratios, and sex bias in adult mortality. ASR (response variable) = proportion of adult males in the population. Hatching and fledging sex ratios = proportion of males among hatchlings and pre-fledging juveniles, respectively. Sex bias in adult mortality = log(adult female mortality/adult male mortality). Mean values, s.e. and p-values are given from PGLS models with 100 different phylogenetic hypotheses.

predictor variable	b (s.e.)a	p (s.e.)	no. species
hatching sex ratio	0.518 (0.333)	0.127 (0.004)	48
fledging sex ratio	0.470 (0.264)	0.082 (<0.001)	47
sex bias in adult mortality	0.263 (0.055)	<0.001 (<0.001)	117

^astandard errors include both sampling error and phylogenetic variance across the set of 100 trees. Estimates of standard errors for parameters were generated by averaging the variances of the estimates across the 100 trees, and adding the variance of the estimates across the 100 trees as well. This accounts for both the statistical and phylogenetic uncertainty in parameter estimates.

Table 2.

ASR in relation to (a) hatching sex ratio, (b) fledging sex ratio and (c) adult mortality bias. (d) Model that includes all predictors. ASR (response variable in all models) = proportion adult males in the population. Hatching sex ratio and fledging sex ratio = proportion male offspring at hatching or fledging, n = 47 and 46 species, respectively. Adult mortality bias = log(adult female mortality/adult male mortality), n = 115 species. Model that includes all predictors, n = 18 species. Mean values, s.e. and p-values are given from PGLS models with 100 different phylogenetic hypotheses.

predictor variables	b (s.e.)a	p (s.e.)
(a)
hatching sex ratio	0.541 (0.285)	0.065 (<0.001)
body mass	−0.001 (0.026)	0.950 (0.001)
development mode	−0.034 (0.037)	0.362 (0.003)
sexual size dimorphism	−0.623 (0.148)	<0.001 (<0.001)
(b)
fledging sex ratio	0.375 (0.254)	0.148 (<0.001)
body mass	0.019 (0.025)	0.447 (0.001)
development mode	−0.021 (0.033)	0.530 (0.003)
sexual size dimorphism	−0.505 (0.148)	0.001 (<0.001)
(c)
sex bias in adult mortality	0.234 (0.051)	<0.001 (<0.001)
body mass	−0.013 (0.010)	0.214 (<0.001)
development mode	0.012 (0.011)	0.265 (<0.001)
sexual size dimorphism	−0.280 (0.066)	<0.001 (<0.001)
(d)
hatching sex ratio	−0.094 (0.500)	0.855 (<0.001)
fledging sex ratio	1.009 (0.770)	0.216 (<0.001)
sex bias in adult mortality	0.630 (0.194)	0.008 (<0.001)
body mass	0.004 (0.042)	0.923 (<0.001)
development mode	0.038 (0.051)	0.468 (<0.001)
sexual size dimorphism	−0.152 (0.066)	0.472 (<0.001)

^astandard errors include both sampling error and phylogenetic variance across the set of 100 trees. See table 1 for details.

Table 3.

Adult mortality in relation to social mating system, parental care and reproductive output. (a) Male mortality = log(annual adult male mortality), n = 250 species. (b) Female mortality = log(annual adult female mortality), n = 234 species. (c) Sex-biased mortality = (log(adult female mortality/adult male mortality), n = 241 species. Social mating system = male mating system, female mating system and bias in mating system (i.e. male mating system − female mating system) in (a), (b) and (c), respectively. Parental care = involvement of male in care provisioning relative to female provisioning (see Material and methods). Body mass = male body mass (log(g)), female body mass (log(g)) and average body mass of males and females (log(g)) in (a), (b) and (c), respectively. Reproductive output = log(clutch size × egg mass). Mean values, s.e. and p-values are given from PGLS models with 100 different phylogenetic hypotheses.

predictor variables	b (s.e.)a	p (s.e.)
(a)
social mating system	0.020 (0.010)	0.055 (<0.001)
pre-hatching care	0.019 (0.025)	0.428 (0.002)
post-hatching care	−0.068 (0.024)	0.005 (<0.001)
body mass	−0.175 (0.031)	<0.001 (<0.001)
(b)
social mating system	0.008 (0.018)	0.667 (0.007)
pre-hatching care	−0.004 (0.025)	0.880 (0.006)
post-hatching care	−0.043 (0.023)	0.065 (0.002)
reproductive output	0.301 (0.086)	<0.001 (<0.001)
body mass	−0.390 (0.067)	<0.001 (<0.001)
(c)
mating system bias	−0.019 (0.006)	<0.003 (<0.001)
pre-hatching care	−0.018 (0.014)	0.185 (0.002)
post-hatching care	0.002 (0.015)	0.878 (0.009)
reproductive output	0.082 (0.036)	0.023 (0.001)
body mass	−0.079 (0.029)	0.008 (<0.001)

^astandard errors include both sampling error and phylogenetic variance across the set of 100 trees. See table 1 for details.

(c). Consistency of adult sex ratio estimates

We analysed up to six ASR estimates per species, and for species with more than six estimates (e.g. house sparrow Passer domesticus; see below), we selected six estimates that had the largest sample sizes. As only five species had more than six estimates available, this was a practical cut-off point to reduce over-representation of a small number of species with many ASR estimates. We tested the repeatability of the ASR estimates using data from 55 species in total (number of ASR estimates per species: 2.9 ± 0.2, mean ± s.e.). We tested the variability of intra- versus interspecific variation in ASR by fitting a PGLS model in which we allowed for intra-specific variance in traits. We did this by estimating a variance component for intra-specific variation in addition to allowing for phylogenetic signal by estimating λ. According to the latter analysis, about 56% of the variance in ASR measurements was intra-specific, implying that nearly half (44%) of the variation was interspecific, which indicates considerable variation in species-level mean ASR. Importantly, the direction of ASR (i.e. male- or female-biased) was highly conserved: in 44 species out 55 (80%), the direction of ASR bias was the same for all repeated estimates.

We tested whether ASR estimates depend on sample sizes in two ways. First, we used 28 estimates from house sparrows obtained with at least six different methods (counting birds killed by storm or poisoning, trapping, baiting, mist-netting, observing feeding birds and unspecified [48]). Nevertheless, ASR was unrelated to sample sizes (electronic supplementary material, figure S4a; b = −0.0186, t = 1.356, p = 0.187). Second, ASR was unrelated to sample size using a single datum for each species (electronic supplementary material, figure S4b; b = −0.011, t = 1.097, p = 0.275, n = 109 species).

Finally, we found no evidence that variance in ASR was related to sample sizes, because deviations from median ASR was not different between estimates that used fewer than 100 individuals, 100–999 individuals and 1000 or more individuals (modified robust Brown–Forsythe Levene-type test, F = 0.120, p = 0.887, n = 109 species).

3. Results

(a). Predictors of adult sex ratio bias

Avian sex ratios are significantly male-biased for hatchlings and adults (electronic supplementary material, figure S1; one-sample t-tests with phylogenetic control using 0.5 expectation; hatching SR = 0.517 ± 0.01, t = 3.27, p = 0.002, n = 83 species; ASR = 0.544 ± 0.006, t = 7.17, p < 0.001, n = 187 species), whereas fledging sex ratios are not different from 0.5 (0.509 ± 0.006, t = 0.7, p = 0.489, n = 86 species). Hatching, fledging and adult sex ratios are not different from each other in those species in which all three estimates were available from the same species (electronic supplementary material, S2). Note that the latter estimates may refer to different populations of the same species and/or different time periods of study.

Neither hatching nor fledging sex ratios predict ASR (figure 1 and table 1). However, mortality bias in adults is a strong predictor of ASR: female-biased adult mortalities are associated with male-biased ASR (figure 1 and table 1). These results remain consistent after including potentially confounding variables in the models (table 2a–c). SSD is significantly related to ASR in all three models (table 2a–c), suggesting that SSD predicts ASR in addition to mortality bias: species in which the males are larger than the females exhibit female-biased ASR even when sex-biased adult mortalities are controlled for.

Figure 1.

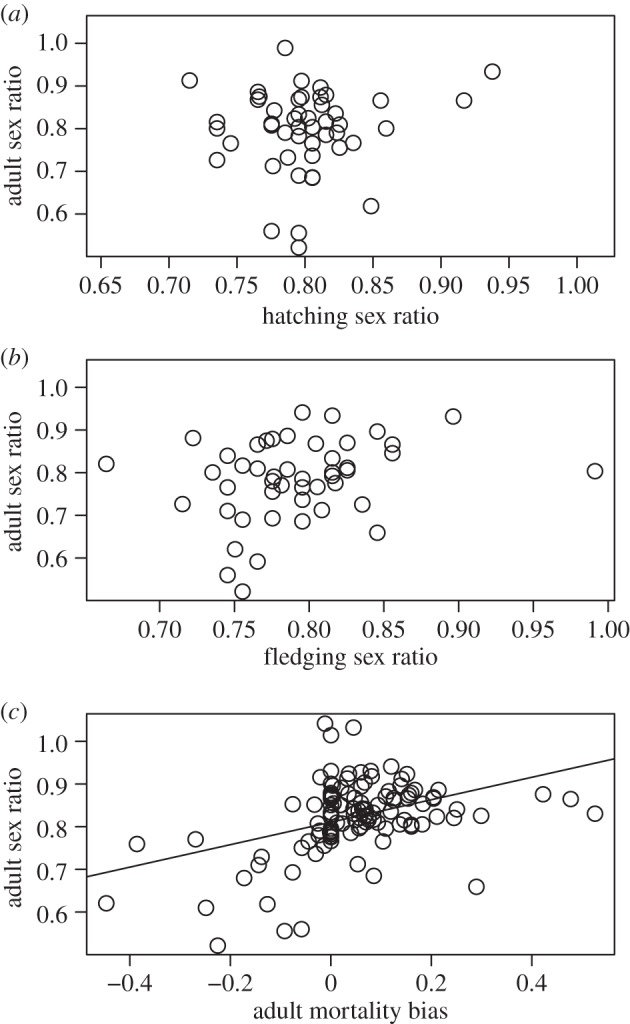
ASR in relation to hatching sex ratio, fledging sex ratio and adult mortality bias in birds. (a) Hatching sex ratio (b = 0.518, p = 0.127), (b) fledging sex ratio (b = 0.470, p = 0.082) and (c) sex bias in adult mortality (log(adult female mortality/adult male mortality), b = 0.263, p < 0.001). Sex ratios are expressed as no. of males/(no. of males + no. of females), and their arcsine-square-root-tranformed values are shown. Mean slope (b) and probability (p) of slope of phylogenetic regressions using PGLS with 100 different phylogenetic hypotheses are given. For statistical models, see table 1 (n = 48, 47 and 117 species, respectively).

When both hatching and fledging sex ratios and adult mortality bias are included in a multi-predictor PGLS together with potentially confounding variables, adult mortality bias remains the only significantly predictor of ASR (table 2d).

(b). Cost of sexual selection, parental care and reproduction

Adult mortality is significantly female-biased (female mortality − male mortality: 0.046 ± 0.01, one-sample t-test with phylogenetic control, t = 20.2, p < 0.001, n = 265 species; electronic supplementary material, figure S3). The strongest predictor of adult mortality is mating system, although parental care and cost of reproduction are also related to mortality of males or females, respectively (table 3). First, as expected, male mortality rates tend to increase with the frequency of male polygamy (figure 2a and table 3; b = 0.020, p < 0.055, n = 250 species). Furthermore, higher levels of male polygamy (relative to female) predict significantly higher male mortality relative to females (figure 2c and table 3; b = −0.019, p < 0.003, n = 241 species). Second, increased post-hatch care (but not pre-hatch care) by males is associated with reduced mortality in males (b = −0.068, p = 0.005) and tended to do so in females (b = −0.043, p = 0.065, table 3). Third, female mortality increased with reproductive output (b = 0.301, p < 0.001, table 3). Finally, mortality of both males and females decreased with body size, consistent with life-history expectations that larger birds have lower annual mortality than smaller ones (table 3).

Figure 2.

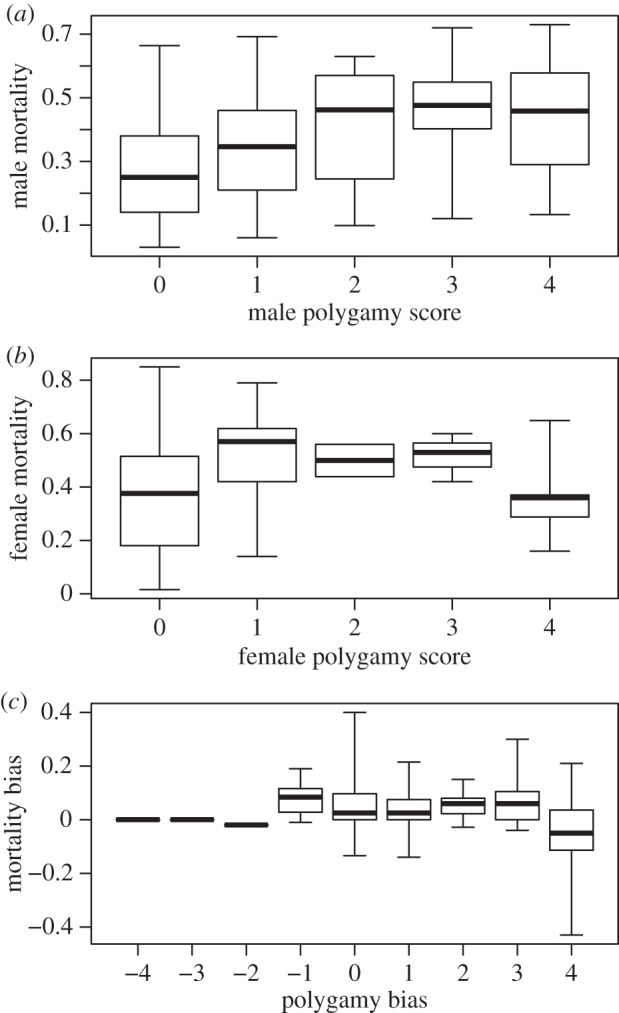
Annual adult mortality in relation to social mating system in birds. (a) Male mortality (b = 0.017, p < 0.010), (b) female mortality (b = 0.0028, p = 0.827) and (c) sex-biased mortality (log(adult female mortality/adult male mortality); b = −0.021, p = 0.0015). Mean slope (b) and probability (p) of slope of phylogenetic regressions using PGLS with 100 different phylogenetic hypotheses are given. For statistical models, see table 3 (n = 250, 234 and 241 species, respectively).

4. Discussion

Taken together, we show for the first time that ASRs in wild bird populations are significantly predicted by sex-biased adult mortality rates, rather than by hatching or juvenile sex ratios. We also show that intense sexual selection, primarily on males, predicts male-biased adult mortalities. Therefore, selections operating on adults may have more influence on ASR than sex-biased sex ratios at birth and/or any sex difference in juvenile mortalities [20,49,50].

Avian sex ratios are already male-biased at hatching, although the bias at hatching does not predict bias in adults. Although species with a male-biased offspring sex ratio may exhibit male-biased ASR (e.g. Kentish plover C. alexandrinus [32]), whereas species with female-biased offspring sex ratio may exhibit female-biased ASR (ruff Philomachus pugnax, great bustard Otis tarda [51,52]), the lack of a significant relationship suggests that the pattern is not general across birds. The latter biases are consistent with Fisher–Trivers’ frequency-dependent argument that in sexually dimorphic species (such as the ruff and great bustard where males are substantially larger than females) parents should produce more of the ‘cheaper’ sex (i.e. females) [53]. Juvenile mortalities may be sex-specific for several reasons: male offspring—which are often larger than female offspring—may die more often than females if they are more sensitive to food shortage [23]; such costs of sexual selection for males have also been documented at the juvenile stage in primates [54].

Furthermore, we found that SSD was associated with ASR independently from the effects of offspring sex ratio, adult mortality and other confounding variables. SSD may influence ASR through juvenile mortality or maturation rates, which were not included in our analyses due to a lack of data. SSD can potentially be associated with both of these factors; the larger sex may suffer higher juvenile mortality (see above) than the smaller one, or may delay breeding, as has been reported for males in several polygynous birds [55].

Our results are important from a theoretical perspective because they suggest that parents do not adjust offspring sex ratio with regards to ASR. This notion is consistent with Fisher's and Hamilton's arguments that parental compensation is not expected if skewed sex ratios emerge after the end of parental investment period [56,57]. As Fisher asserted [56], if differential mortalities of adult males and females produce biased ASR, then frequency-dependent selection on its own is unlikely to revert biased ASR to even, as long as the expected fitness of daughters and sons are on average the same. Although various studies have investigated whether females may bias the sex ratio of their offspring to social and ambient environments [58–60], our study appears to be the first to investigate the relationship between offspring sex ratios and ASR across a broad range of taxa.

The best predictor of ASR bias was mortality in adults: female-biased mortalities tended to produce more male-biased ASR, and vice versa. This result is robust to various additional effects (table 2) and consistent with single-species studies that show sex difference in mortalities: males and females often have different behaviour, ecology and life histories [42], and these differences may produce biased exposure to predators and other agents of mortality [61,62]. Consistently with previous works in birds and mammals [27,28], we found that sexual selection predicts sex-biased moralities: species in which males compete more intensely for mates exhibit higher male-biased mortality than species where male competition is weak or females compete for males. In addition, heavy clutches were associated with elevated female mortality, suggesting that egg production is costly, in line with broad-scale phylogenetic analyses of avian reproduction [63,64]. The negative correlation between post-hatch male care and male mortality may suggest that male involvement in post-hatch care occurs in species where males enjoy high survival. The overall mortality bias (and thus ASR) emerges as the outcome of these different processes that may produce male-biased, female-biased or even ASR.

While our study goes a long way towards establishing the causes of vertebrate ASR bias using birds as model organisms, we identify three major challenges. First, it is increasingly recognized that the relationships between ASR, social behaviour and mortalities may be complex [8,11,65,66]. On the one hand, Fisher [56] conjectured that there are feedbacks on an evolutionary time scale by arguing that ASR may be self-correcting. If ASR is heavily male-biased, this intensifies mate competition, and as such increases male mortality. This process could counterbalance the biased ASR producing a more even ASR. Following Fisher, Trivers [67] noted that sex-specific mortality patterns tend to coevolve with sex-specific patterns of parental investment; for instance, the male-biased adult mortalities in mammals are often credited to intense male–male competition [27]. On the other hand, other models suggest positive feedbacks between ASR and breeding system (e.g. reduced survival of the rarer sex due to increased mating activity) [6,17], which in turn may produce even more biased ASRs. Testing the complex relationships between social behaviour, mortality implications and ASR will probably reveal novel aspects of breeding system evolution [1,6,11].

Second, consistent with previous studies [28,41], we show that female birds have higher mortality than males. Trivers [67] argued that the heterogamic sex (in birds, the female) suffers higher mortality than the homogamic due to exposed deleterious mutations on the sex chromosome, although given the limited power of a two-taxon comparison (birds versus mammals), further ASR data are needed from taxa that exhibit variable sex determination systems (e.g. fishes, amphibians and reptiles). Third, ageing and maturation rates may differ between the sexes, and adult males and females may exhibit different susceptibility to heat stress, food shortage, parasites and predators [25,26]. Spatial or temporal variation in these factors should be investigated to test whether they are involved in corresponding changes in ASR [11,20].

ASRs are often criticized as potentially erroneous, because the published estimates are derived using a variety of methods, and often using modest sample sizes, which may lead to biased estimates [11,20]. However, the high repeatability between estimates of the same bird species (which were often collected from different populations using different methodologies) suggests that this problem—at least among the studies where multiple estimates were available—is not acute (see Material and methods). ASR estimates from 28 house sparrow populations that were derived with six different methodologies also suggest no systematic deviation in ASR with sample size [48] (electronic supplementary material, figure S4a). Variance in ASR among the studies in our dataset is not different between small, medium and large sample sizes (electronic supplementary material, figure S4b), although at very large sample sizes (over 10 000 individuals) ASR appears to be less variable than below 1000 individuals (see Material and methods). A potential explanation for the latter pattern may be that the bird species that had unusually large sample sizes somehow exhibit less variable ASRs (e.g. they may be large and long-lived species that are accessible for large-scale studies). Nevertheless, we concur with demographic studies that the most reliable estimates of ASR should involve modelling age-specific survival of hatchlings [2,32], although at the moment such data are only available for fewer than a handful of bird species.

In conclusion, we show that ASRs are better predicted by adult mortality than offspring sex ratios in birds. This suggests that selection operating on adults is more likely to impact on ASR than selection acting on females to bias offspring sex ratios, and/or on male and female juveniles to reach adulthood. ASR bias, in turn, has knock-on effects on social behaviour, sex roles and parental care. Future work should investigate further sources of sex-specific mortality, reveal the feedback relationships between ASRs, social behaviour and mortality costs, and test the generality of these findings using other taxa (e.g. mammals, fishes and insects).

Data accessibility

All data used in this paper are fully available (see the electronic supplementary material).

Funding statement

T.S. was financially supported by a Humboldt Award, and A.L. by a Marie Curie Intra-European Fellowship. R.P.F. is a University Research Professor.