Beam characteristics in two different proton uniform scanning systems: A side-by-side comparison

Abstract

Purpose:

To compare clinically relevant dosimetric characteristics of proton therapy fields produced by two uniform scanning systems that have a number of similar hardware components but employ different techniques of beam spreading.

Methods:

This work compares two technologically distinct systems implementing a method of uniform scanning and layer stacking that has been developed independently at Indiana University (IU) and by Ion Beam Applications, S. A. (IBA). Clinically relevant dosimetric characteristics of fields produced by these systems are studied, such as beam range control, peak-to-entrance ratio (PER), lateral penumbra, field flatness, effective source position, precision of dose delivery at different gantry angles, etc.

Results:

Under comparable conditions, both systems controlled beam range with an accuracy of 0.5 mm and a precision of 0.1 mm. Compared to IBA, the IU system produced pristine peaks with a slightly higher PER (3.23 and 3.45, respectively) and smaller, symmetrical, lateral in-air penumbra of 1 mm compared to about 1.9/2.4 mm in the inplane/crossplane (IP/CP) directions for IBA. Large field flatness results in the IP/CP directions were similar: 3.0/2.4% for IU and 2.9/2.4% for IBA. The IU system featured a longer virtual source-to-isocenter position, which was the same for the IP and CP directions (237 cm), as opposed to 212/192 cm (IP/CP) for IBA. Dose delivery precision at different gantry angles was higher in the IBA system (0.5%) than in the IU system (1%).

Conclusions:

Each of the two uniform scanning systems considered in this work shows some attractive performance characteristics while having other features that can be further improved. Overall, radiation field characteristics of both systems meet their clinical specifications and show comparable results. Most of the differences observed between the two systems are clinically insignificant.

I. INTRODUCTION

Technological advances in proton therapy in the recent years have led to the development of several methods of beam scanning. A simpler method among them, uniform scanning, has matured enough to be adopted for clinical application at a number of facilities.

Offering certain advantages over the more established, double-scattering technique by eliminating the need of scatterers and thus providing higher beam penetration range, less scattering and potentially a reduction in secondary radiation background, the method of uniform scanning may have more than one technological solution.

Historically, most of the clinical proton facilities have used such unique delivery systems that it was difficult to make a meaningful comparison. However, since 2007 and 2009, two US centers, Indiana University (IU) in Bloomington, Indiana, and the University of Florida Proton Therapy Institute (UFPTI) in Jacksonville, Florida, have started clinical use of uniform scanning on a gantry. The system developed at IU was developed for the Midwest Proton Radiotherapy Institute (presently IU Health Proton Therapy Center) while uniform scanning at IUFPTI was originally developed by Ion Beam Applications SA (IBA) in collaboration with physicists at Francis H. Burr Proton Therapy Center (Boston, Massachusetts). Besides UFPTI, the IBA system has been installed at a number of proton therapy facilities built by IBA worldwide, including ProCure Proton Therapy Center (PPTC) in Oklahoma City, Oklahoma, Westdeutsches Protonentherapiezentrum Essen (WPE) in Essen, Germany, and elsewhere. Both of the uniform scanning solutions are in routine clinical use. While other active beam delivery systems are available worldwide (e.g., a beam wobbling system implemented at Ion Beam Medical Center in Hyogo, Japan), this work focuses on a comparison of two uniform scanning systems developed at IU and IBA.

On the one hand, the uniform scanning system developed at the Indiana University (referred hereafter as the IU system) and the system developed by IBA have many similar, and even identical, hardware components; on the other hand, the technological implementations are different (e.g., the use and positioning of scanning magnets, range modulator design, etc.). It was therefore of interest to compare the two solutions to identify their differences in terms of resulting radiation field characteristics. Our comparison focused chiefly on properties such as longitudinal and transversal field characteristics and on dosimetric properties. Other system parameters such as safety, user interfaces, patient throughput, etc., while being important, were not considered in this work.

This work was focused on the measurement and analysis of the longitudinal and transversal characteristics, effective and virtual source position relative to the gantry isocenter, and on several dosimetric characteristics of the radiation fields.

II. METHODS AND MATERIALS

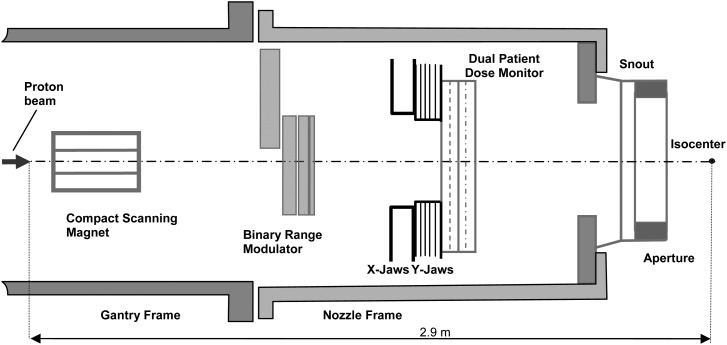
Schematic diagrams of the IU and IBA uniform scanning nozzles are shown in Figs. 1 and 2. Detailed descriptions of these systems have been reported elsewhere.^1,2 The basic approach to the production of clinical fields in both systems is the same: the beam of a certain energy (and penetration range) is scanned laterally, “painting” the dose over the prescribed area and the dose coverage in depth is achieved, through the use of a range modulator, by adding individual Bragg peaks (layers) with incrementally shorter penetration ranges (Bragg peak pullbacks). Both systems provide a requested beam penetration range through the use of a range shifter device located upstream of the gantry. The IBA system can produce a full depth-dose modulation delivering equal dose to all depths, from the maximum penetration range to the surface. The maximum commissioned depth modulation size in the IU system is 17 cm in water. Depth-dose delivery in both systems is controlled by specially designed computer control files or programs.

FIG. 1.

Schematic diagram of the Indiana University uniform scanning nozzle.

FIG. 2.

Schematic diagram of the IBA uniform scanning nozzle.

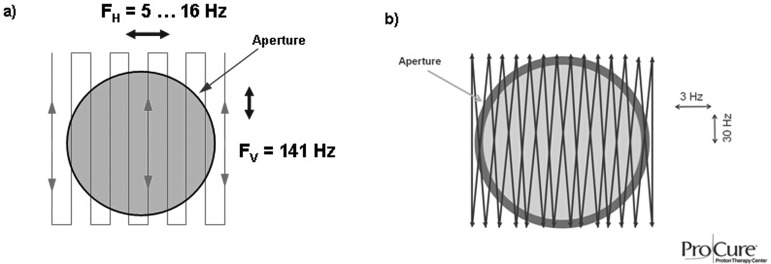
Typical scanning patterns used in the two systems are shown in Fig. 3. It must be noted that while the gantry and the nozzle frame hardware for the IU system was identical to the IBA system (see Figs. 1 and 2), key beam and dose delivery components such as the scanning magnet, range modulator, and beam monitoring detectors were developed at IU independently. All field measurements for the IU system reported in this work were conducted at IU; the data for the IBA system were mostly obtained at UFPTI with additional measurements done at WPE and PPTC.

FIG. 3.

Examples of uniform scanning patterns for the IU (a) and IBA (b) systems.

The choice of IBA centers for measurements was determined by beam accessibility, and the use of several facilities rather than just one was justified by the fact that once accepted by medical physicists for clinical commissioning and use, the facility’s characteristics meet clinical specifications for field flatness, range, penumbra size, etc.³ The major differences between the two systems are the initial energy (230 MeV for the IBA vs 208 MeV for the IU system) due to the use of different accelerators and the size of the largest commissioned field (25 cm diameter for IBA (Ref. ⁴) vs 30 cm diameter for IU).

Wherever possible, similar or identical conditions were created for measurements in the two systems. For example, pristine peaks were characterized at the range of 16 cm in water, which was chosen as being close to the mean range attainable by either system; equal spread-out Bragg peak (SOBP) modulation extents were chosen. Unless otherwise stated, reference conditions were used for field characterization. Reference conditions were characterized by the 16 cm range in water, 10 cm diameter aperture, 5 cm air gap between the aperture and water tank, and a SOBP with a 10 cm modulation extent, with the middle of the SOBP located at the gantry isocenter. It is important to note that under the reference conditions, the average dose rate in the IU system was 2 Gy/min and in the IBA system, 1 Gy/min.

The following subsections describe the methods used to obtain the data.

II.A. Longitudinal characteristics

Proton beams used in the IU and IBA beam delivery systems have a well defined range, which depends on the beam energy. Beam range and peak-to-entrance ratio (PER) are important radiation beam parameters that are used in treatment planning algorithms and are some of the key characteristics to be measured during commissioning and acceptance tests of any treatment system in proton therapy.

II.A.1. Pristine beam range accuracy and precision

The accuracy of prescribed beam penetration in water was verified by requesting a number of different penetration ranges near the nominal 16 cm value (namely, 15.9, 16.0, and 16.1 cm) and measuring the depth of the distal 80% of the maximum dose in a 10 cm-diameter filed. The measurements were performed either in a water phantom with a small-volume parallel plane ion chamber (Markus PTW Model 23343 or equivalent) or with multielement detectors such as multilayer ionization chambers,^5,6 whose water equivalent thickness had been previously determined.

Range delivery precision in this work was studied by requesting the same penetration depth several (usually three) times and calculating the average of beam penetration results. Additionally, range verification in the IU system is performed with a multilayer Faraday cup each time a new beam penetration range is requested. This procedure is described in detail for the double scattering system in Ref. 7, but it is also implemented in the beam lines feeding each of the two IU uniform scanning nozzles. Consistency of penetration range in the IBA system is verified on a daily basis as part of quality assurance (QA) program and the range accuracy is measured during annual QA checks. The QA data are not presented in this work, but they were included in the analysis of beam range accuracy and precision measurements described in this section.

II.A.2. Pristine beam peak-to-entrance ratio

Pristine beam peak-to-entrance ratio was determined for the pristine Bragg peak with penetration depth of 16 cm (about 150 MeV). Measurements were done in a water phantom using a Markus ion chamber and the entrance point was defined at a depth of 3 cm on the beam central axis. In all cases, the depth interval for these measurements was 2 mm or less.

II.A.3. Pristine beam full width at half maximum and distal fall-off

Full width at half maximum (FWHM) was measured for the pristine Bragg peak described in Sec. II A 2. Pristine beam distal fall-off, defined as the difference in depth between the distal 80% and 20% isodose, was determined from the measurements described in Sec. II A 2.

II.A.4. SOBP uniformity and distal fall-off

The SOBP uniformity in depth was measured within the dose reference volume (DRV) with dose intervals of about 2 mm. The DRV (see Fig. 4 and definitions therein) is defined in Ref. 1 as two penumbra widths (B) inside the 50% isodose laterally, 1.5 distal fall-off widths (A) inside the 50% isodose distally, and 1 total pullback water-equivalent thickness inside the (distal) 50% isodose proximally. The total pullback is defined as the number of longitudinal steps or layers within the volume multiplied by the water equivalent step width.

FIG. 4.

Definitions of dose reference volume, penumbra, and lateral flatness.

The SOBP longitudinal uniformity is defined as the maximum difference in the dose within the DRV expressed as the percentage of the average dose within the reference volume.

The SOBP distal fall-off was defined as described in Sec. II A 3 and was measured using a multielement ion chamber array (MLIC detector at IU and the Zebra detector at UFPTI).

II.B. Transversal characteristics

Transversal characteristics such as field flatness and penumbra may depend on the method of field production and therefore may differ between proton treatment delivery systems. Similar to longitudinal characteristics, transversal field characteristics are used in treatment planning and therefore need to be studied well. In this work, transversal characteristics were determined for two directions, both orthogonal to the central beam axis, one coinciding with the gantry plane (inplane, IP) and the other orthogonal to the gantry plane (crossplane, CP). Unless stated otherwise, measurements were done at the gantry isocenter.

II.B.1. Large field size, uniform area, flatness, and penumbra

The largest field commissioned at WPE and UFPTI for the IBA system at the time of this work was created with an aperture of 23.8 cm in diameter. While the largest field size commissioned for clinical use in the IU system is about 30 cm in diameter (at the 50% isodose), for a more direct comparison of large field characteristics, an aperture with a 24 cm diameter was used in the IU system. For a more clinically realistic beam delivery scenario, the beam range for these fields was set to 20 cm in water and SOBP modulation to 10 cm. For both systems, apertures with straight edges (no tapering) were used. The measurements were done at IU and WPE using radiochromic film (EBT and EBT2, International Specialty Products, Inc.) exposed in a plastic phantom at the proximal end of the SOBP (8 cm water equivalent). Assuming that the scanning patterns are optimized for the center of the SOBP (as described, for example, in Ref. 1), the choice of depth was driven by the desire to examine the large field’s characteristics in the “worst-case” clinically relevant conditions, where the differences between the systems and their scanning delivery imperfections, if any, would be least concealed by multiple Coulomb scattering in the phantom. Several film sheets were required to cover the fields and the sheets were “stitched” together during the analysis. The film was read out on a flatbed scanner (Epson Expression 10000XL); the signal in the red channel was converted to optical density and to dose using film calibration curves. The film analysis was done using ImageJ software package. Field flatness parameters were determined from the film using the following method: the lateral flatness is measured over the region of interest (ROI) along the line perpendicular to the beam central axis. The ROI is taken as 80% of the field size, where the field size is equal to the size of the 50% isodose. Field lateral flatness is then calculated as

$$F=\frac{{(|D-D_{\mathrm{mean}}|)}_{\max }}{D_{\mathrm{mean}}}·100,$$

where D is the dose value at a given point along the line perpendicular to the beam central axis within the ROI, and D_mean is the mean dose value over the ROI.

Penumbra for the large field were determined for IP and CP from the film measurements at the proximal end of the DRV.

Because clinical requirements for both systems call for ±2.5% uniformity, this number was used as a criterion for measuring the attainable uniform field size. In contrast to the field flatness characteristic described above (with a tolerance value of 4%), field uniformity is defined as the maximum deviation of dose from the mean value over the dose reference volume.

II.B.2. Pristine beam penumbra in air

In order to study the properties of the pristine beam, lateral penumbra of a field produced with a scanned pristine beam was measured in the IP and CP directions for a 10 cm diameter field in air, at a distance of 20 cm from the aperture. The beam range was chosen to be 27 cm in water, the largest penetration range common to both systems. The measurements were done using radiochromic film and analyzed as described in Sec. II B 1.

II.B.3. Reference field flatness and penumbra

Flatness and penumbra for the reference field in each system were determined in the same way as for the large field, except that penumbra measurements were done at the center of the SOBP (11 cm water equivalent).

II.B.4. Source-to-axis distance under reference conditions

Because no published recommendations exist that specifically address measurements of proton beam characteristics such as effective and virtual source distance from the gantry axis (isocenter), these characteristics were determined following the procedures described in Ref. 8 for electron beams.

To find the effective source position, a series of measurements were taken with a Markus ion chamber (on the beam central axis) as a function of the air gap between the aperture and the phantom surface. With the chamber response at the minimum air gap taken as reference value Q₀, the square root of the ratio Q₀/Q_g at each air gap, g, was plotted as a function of the air gap, and the effective source-to-axis distance (SAD) was calculated as the slope of the straight line representing the inverse-square-law assumption.

Virtual source position, as defined in Ref. 8, was determined by back projection of the 50% width of the field profiles obtained at several distances (−10 and 10 cm for IBA and 0, 10, and 20 cm for IU) from the isocenter, along the beam central axis. Field profiles were obtained using radiochromic film, which was read out and analyzed as described in Sec. II B 1. The 50% field width data were plotted as a function of distance and extrapolated to zero.

II.C. Dosimetric characteristics

As mentioned in Sec. II, in both systems, the dose at each layer is painted laterally, and is repainted multiple times (e.g., at least 100 times for the distal layer in the IU system and 30 times in the IBA system under reference conditions, when 100 monitor units (MU) are to be delivered to the field) before starting the next layer. In the IU system, the dose delivery to a layer is complete when the prescribed number of MU for that layer are delivered. The IBA system can be configured either to complete the field on MU completion or to complete the field when the layer painting is completed. The systems do not offer repainting in depth: the dose to each layer is only delivered once, and the beam is turned off between the layers. Because the dose under uniform scanning is delivered in a succession of layers, there is a minimum dose per layer that can be detected by the treatment delivery system with a required accuracy. Clearly, the minimum detectable dose and the number of repaintings are related to dose rate. In this work, we studied the minimum deliverable dose under the reference conditions, defining it as the dose corresponding to the smallest number of MU that the system will allow the user to deliver at the center of 10 cm modulation and at the isocenter. This number characterizes the precision of dose calculation and beam monitoring as well as the flexibility of beam delivery control.

In order to evaluate dosimetric properties of each uniform scanning system, the systems were studied for dose reproducibility throughout the week, dose overrun per 100 MU delivered, minimum delivered dose (in MU) at reference conditions, and dose delivery precision at different gantry angles. The time required to deliver 150 MU at reference conditions was also recorded.

Dose reproducibility was defined as the coefficient of variation

$$s=\frac{100}{⟨R⟩}\sqrt{\sum _{i=1}^n\frac{{(⟨R⟩-R_i)}^2}{n-1}},\%,$$

where n is the number of measurements, R_i is the ratio of measured values of monitor units and absorbed dose in the ith measurement, ⟨R⟩ is the average value of the ratios R_i determined from

$$⟨R⟩=\frac{1}{n}\sum _{i=1}^n{R}_i.$$

In this work, s was determined in five measurements (n = 5) taken over a period of one week, as recommended in Ref. 9.

Dose overrun was defined as

$$d=(1-\frac{{\displaystyle \sum _{i=1}^n}{\mathit{MUdel}}_i}{{\displaystyle \sum _{i=1}^n}{\mathit{MUreq}}_i})·100,$$

where ${\mathit{MUreq}}_i$ and ${\mathit{MUdel}}_i$ are the number of MUs requested and delivered during ith exposure, respectively, and n is the number of consecutive MU deliveries (n = 10).

Dose delivery precision as a function of gantry angle characterizes the robustness of a beam delivery and monitoring system. Because dose calibrations are usually performed at one gantry angle, it is important to know how accurately the dose can be delivered at other angles. Dose delivery precision was measured by delivering a preset number of MU, recording the charge collected in a reference Markus ion chamber installed in a plastic phantom attached to the 10 cm diameter aperture, and expressing it as percentage of the average charge delivered at all angles. Thus, the reference chamber was not positioned at isocenter and was rotating with the gantry. This detector position was chosen to eliminate the possible effect of gantry eccentricity and study only the effect of beam monitor sensitivity to the gantry angle, if any. The measurements were repeated at several gantry angles.

III. RESULTS AND DISCUSSION

Measurement results for the IU and IBA systems are summarized in Table I. A cursory look at the table reveals that many of the characteristics are similar; this is logical as the driving clinical performance requirements for the systems (e.g., field flatness, penumbra, etc.) and the general gantry designs are very similar, too. In fact, the gantry support structure and the nozzle frame at IU are identical to those used by IBA, but the gantry and beam control hardware, the scanning system, and beam monitoring components are different. For each characteristic discussed, we illustrate it with typical results obtained in one of the scanning systems.

TABLE I.

Comparison of longitudinal, transversal, and dosimetric characteristics of the IU and IBA uniform scanning systems.

System characteristic	IU system		IBA system
A. Longitudinal
Range accuracy,a mm	±0.5		± 0.5
Range precision,a mm	±0.1		± 0.1
Peak-to-entrance ratiob	3.45		3.23
FWHM—pristine peak, cm	1.9		2.0
Distal fall-off—pristine peak, cm	0.4		0.4
Uniformity—SOBP 10 cm, %	1		1.5
Distal fall-off—SOBP 10 cm, cm	0.4		0.4
B. Transversal	Inplane	Crossplane	Inplane	Crossplane
Large field—size (at 50%), cm	25.7	25.7	25.3	25.5
Large field—uniform size (within ±2.5%), cm	24.5	24.5	24.3	24.3
Large field—flatness within ROI, %	3.0	2.4	2.9	2.4
Large field—penumbra (at proximal SOBP), mm	6.2	6.2	2.5	3
Pristine, 10 cm field, penumbra in air,c mm	1	1	1.9	2.4
Reference conditions, at 11 cm water
Flatness, %	2.13	2.30	1.42	1.73
Penumbra, mm	3.9	3.9	3.8	4.2
SAD
Virtual (field size back projection), cm	237	237	211.7	191.8
Effective (dose vs distance), cm	244.8		234
C. Dosimetric
Dose reproducibility, throughout the week, %	0.27		0.3
Dose overrun per 100 MU (avg.), MU	0.04		0 – 2
Min. deliverable dose at reference conditions, MU	4		130
Time required to deliver 150 MU, min	0.7		3.2
Dose delivery accuracy vs gantry angle, %	± 1		± 0.5

^aPristine peak, R₈₀ = 16 cm.

^bMeasured at 3 cm water.

^cAt 20 cm from the aperture, pristine peak, R₈₀ = 27 cm.

III.A. Longitudinal characteristics

III.A.1. Pristine beam range accuracy and precision

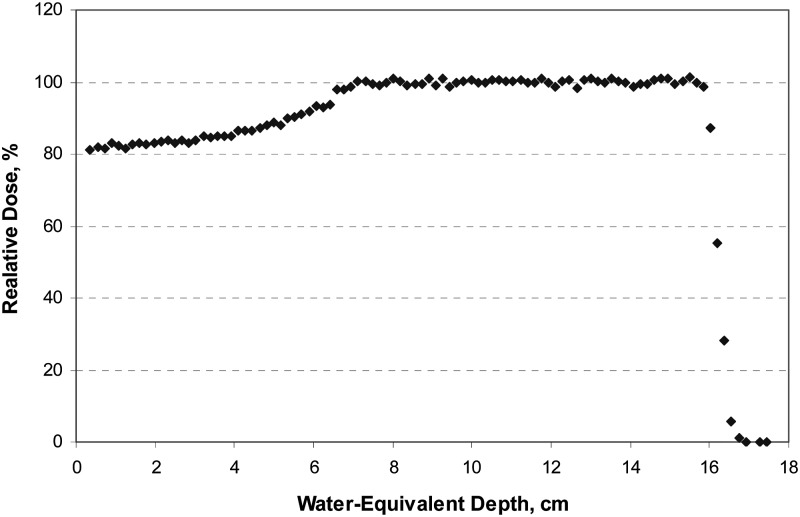
Range accuracy and precision characterize how well the delivered beam’s penetration depth in the patient can be controlled. In contrast with the IU system, where the beam range is defined at the 80% of maximum dose on the distal slope of the Bragg peak, in the IBA system, the beam range is defined for the 90% isodose on the distal slope of the modulated beam. Due to the range modulation technique used to create SOBPs, the depth of the 90% distal isodose in the pristine beam is always 1 mm larger than in the modulated beam. This difference in range definitions at IU and IBA facilities has been accounted for in the data analysis. Table I shows that both systems have similar range control accuracy and precision capabilities, and can control the beam range accurately and within the required tolerance. Figure 5 shows the results of range measurement at UFPTI for three requested ranges.

FIG. 5.

Beam range at UFPTI measured with a multielement detector for three requested ranges: 15.9, 16.0, and 16.1 cm on water.

Despite the fact that only a limited number of beam range measurements were taken as described in Sec. II A 1 and presented in Table I, viewed in the context of a much larger QA statistic spanning a few years and available for each system (not shown), the range data in Table I is in agreement with the range accuracy tolerance of ±1 mm and precision tolerance of ±0.5 mm in water as specified by QA acceptance criteria. We therefore regard the range measurements reported in Table I as supporting the conclusion of good range accuracy and reproducibility offered by the IU and IBA beam delivery systems.

III.A.2. Pristine beam peak-to-entrance ratio

PER measured with a small-volume detector may be used as a measure of pristine beam’s monochromaticity at the patient surface. For a given beam energy and field size, PER may be a function of the beam transport system and of the nozzle design. Clearly, higher PER values result in lower doses to patient’s skin both in the pristine peak and SOBP field irradiations. From Table I, it can be seen that the IU system has a slightly higher PER value compared with that of IBA, suggesting that the IU pristine beam at 16 cm range has a smaller contribution from low-energy protons. This may be explained by the differences in the maximum (and constant) beam energy values provided by the accelerators (208 and 230 MeV for the IU and IBA cyclotrons, respectively), requiring the use of a (thicker) range shifter in the IBA system, and differences in the design of beam transport lines (beam optics, magnet bore sizes, design and placement of the range shifter material, etc.).

III.A.3. Pristine beam full width at half maximum and distal fall-off

The distal fall-off, along with the PER and FWHM values, can be viewed as a measure of pristine beam’s monochromaticity or its “contamination” with lower-energy particles. The width of the pristine peak also determines the thickness of dose deposition layer and thus the size and the number of pullbacks in delivering a complete SOBP field under uniform scanning.

Analysis of FWHM results in Table I shows that Bragg peak widths in the IU and IBA systems are very similar, the IU value being slightly lower. This difference is in agreement with the PER comparison discussed in Sec. II A 1, indicating a slightly larger component of low-energy protons in the 16-cm range pristine beam produced by the IBA system. The similarity in FWHM results is explained by the similarities in the gantry beam line, which removes most of the differences that may have been caused by the different designs of beam lines upstream of the gantries.

The distal fall-off data at the 16 cm range are identical in both systems, providing a high dose gradient at the end of the beam range.

III.A.4. SOBP uniformity and distal fall-off

Figure 6 illustrates a 16-cm range beam with a 10 cm modulation at IU measured with a MLIC detector. Table I shows that the longitudinal uniformity numbers for the IBA system were slightly higher than those for IU. This may be explained by the differences in the method of SOBP production and monitoring. While in both systems the delivery of MUs is based on the individual layer weights, the MU overrun effect in the IBA system (see Sec. III C) is more pronounced and may contribute to the resulting longitudinal uniformity. In both systems, longitudinal uniformity values shown in Table I meet clinical performance requirements (±2.5% for the IU system and ±3% for the IBA system), and the difference between them is regarded as clinically insignificant.

FIG. 6.

Depth dose profile for a beam with the 16 cm range and 10 cm SOBP modulation at IU measured with a multielement detector (MLIC).

In both systems, the size of the SOBP distal fall-off between the 80 and 20% dose was found nearly the same as in the pristine peak for the same penetration depth, which indicates that proximal dose layers have insignificant contribution to the distal dose fall-off and the latter remains almost as steep as that of a pristine beam with the same penetration depth.

III.B. Transversal characteristics

Transversal characteristics results reported in Table I are given for IP and CP for both systems.

III.B.1. Large field size, uniform area, flatness, and penumbra

From the data on large field characteristics presented in Table I, it can be observed that field size values in the IP and CP directions for the IBA system are slightly different, with the CP value being 0.2 cm larger than the IP value (as illustrated in Fig. 7). The same trend, with a 0.5 mm difference, is seen for the IBA field penumbra, whereas the IU field size and penumbra characteristics are identical for IP and CP. The constant field and penumbra sizes in the IU system, as well as the variation of field and penumbra sizes in the IBA system, are explained by the fact that the IU system uses a compact scanning magnet, which deflects the beam in both IP and CP directions, while the IBA uniform scanning is implemented with two magnets. The magnet deflecting the beam in the CP direction is located closer to the gantry isocenter giving rise to larger field size and penumbra values.

FIG. 7.

Lateral profiles produced with the 23.8 cm aperture at WPE and the 24 cm aperture at IU, measured with radiochromic film.

The large field’s flatness values determined at the proximal end of the SOBP are, again, similar for both nozzles. It can be seen from Table I that in each system the CP and IP flatness values are not equal. The reason for that is the scanning pattern implementation in the scanning systems. The smaller value in the IU system (2.4%) is measured along the scan line while the IP value (3.0%) is the result of measurement along the axis orthogonal to a set of parallel scan lines.

Table I shows that the large field penumbra in the IU system are about 3.5 mm larger than the penumbra in the IBA system. This difference is explained by the different positioning of the range modulator devices as shown in Figs. 1 and 2, with the IBA range modulator wheel being about 85 cm farther from the isocenter and thus producing smaller penumbra than those found in the IU system (Fig. 7). The smaller dose gradient below the 20% dose level in the IU system may be explained by the more oblique, on average, angle of protons striking the edges of the IU aperture. This is due to the shorter distance between the IU system’s binary range modulator and the isocenter, compared with the IBA system.

III.B.2. Pristine beam penumbra in air

Pristine beam penumbra results in Table I show that for the IU system lateral penumbra values are equal for the IP and CP directions. For the IBA system, the CP results are about 25% larger than the IP data. This difference results from the use of the dual scanning-magnet design as discussed in Sec. III B 1 and is in reasonable agreement with the difference in distances from the isocenter to the centers of the two scanning magnets (about 20%). The IBA in-air penumbra in both IP and CP directions are larger than the respective IU penumbra. Since range modulator devices are not used during pristine beam delivery, the larger IBA penumbra may be caused by the larger source (beam spot) size, a possible result of degrading the beam energy from 230 MeV. Although no direct measurements of the IBA beam’s FWHM were available, its size in air at the isocenter can be estimated from the treatment planning data to be about 2.4 cm for a beam with a range of 27 cm in water. Under similar conditions, in the IU system, the beam spot FWHM size is about 2 cm.

III.B.3. Reference field flatness and penumbra

The data for reference field flatness and penumbra reported in Table I show that both scanning systems produce a uniform reference field, with the IBA system providing slightly better flatness. However, both systems meet field flatness requirements (see Sec. II B 1). Penumbra values are also quite similar. One can conclude that under reference conditions the difference in field penumbra between the two systems is masked by increased size of the scanned beam’s FWHM (compared with a smaller, at IU, beam size at 27 cm range in water) and the use of range modulation, along with multiple Coulomb scattering in the phantom (11 cm).

III.B.4. Source-to-axis distance for reference conditions

Comparison of effective SAD values shown in Table I reveals that the effective SAD determined from the Markus ion chamber measurements (see example in Fig. 8) are about 10 cm larger for the IU system compared with those of IBA. The data in Table I also indicate that the virtual source position of the IU system is the same in the IP and CP directions whereas the IBA scanning nozzle has two different values, as shown in Fig. 9. The two different values for the IBA system are explained by the use of two scanning magnets as opposed to one compact magnet in the IU nozzle. The IU magnet is also located further upstream giving rise to higher SAD values. The larger SAD values in the IU nozzle are seen as an advantage as they facilitate smaller in-air field penumbra and a smaller absolute dose variation along the beam central axis (inverse square of SAD). An additional advantage of the IU scanning solution is perceived in the symmetry of the field around the beam central axis reducing the uncertainty in the beam dose calculation model in the treatment planning software.

FIG. 8.

Determination of the effective source position at IU by extrapolation of Markus ion chamber measurements.

FIG. 9.

Virtual source position at UFPTI determined from field size measurements at two distances from gantry isocenter.

III.C. Dosimetric characteristics

Dose reproducibility results shown in Table I are similar for the IU and IBA nozzles. They indicate a high stability and precision of dose delivered by each system, well below the 2% acceptance threshold recommended by international standards.^9,10

It can be seen from Table I that the dose overrun effect at IU is much smaller than that at IBA. Ideally, these values should be zero, i.e., no extra dose should be delivered beyond the prescribed MUs, but due to finite reaction time of the control devices, minor radiation “leakage” may be expected, especially at higher dose rates. For the IU system at reference conditions, dose overrun contributes about 0.04% of the prescribed dose, a negligible amount. In the IBA system studied in this work, dose overrun depends on the dose rate, and under reference conditions, it contributed about 2% to the prescribed dose. This effect is explained by a requirement for completing a layer before turning the beam off.¹¹ If the amount of dose overrun can be predicted, and properly accounted for in the dose delivery process, it does not present a major problem, although smaller values are of course more preferable.

Minimum deliverable dose under reference conditions (and notably, at different dose rates) was smaller in the IU system. By definition, 1 MU at reference conditions corresponds to 1 cGy; therefore, the minimum deliverable dose in the IBA system is approximately equal to the typical dose per field in a prostate treatment. It was also found that the minimum deliverable MU in the IBA system strongly depended on the dose rate, suggesting that there was a governing requirement in the dose delivery algorithm for providing a certain minimum number of beam paints at the distal dose layer. For example, according to the IBA dose delivery algorithm, the minimum deliverable dose can be as low as 19 MU at 0.1 Gy/min. However, dose delivery at such a low rate, besides relying on the good linearity of beam monitors, requires much longer delivery time and therefore does not seem suitable for clinical use. An additional concern is intrafractional organ motion, which becomes more difficult to mitigate at longer irradiation times.

Predictably, the time required to deliver 150 MU at reference conditions in the IU system was approximately 4 to 5 times shorter than that required in the IBA system, since the IBA dose rate under the reference conditions is lower by a factor of 2 and the system takes a few seconds to align the beam laterally at low dose rate at the beginning of field delivery. It should be noted that the dose rates quoted for both systems are only approximate values since they include the time intervals with no beam during the switching of the layers. For example, in the IU system, the typical layer-switching time is about 0.5 s, adding a total of about 8 s to a 15-layer SOBP delivery time. In the IBA system, the time between layers is about 0.5–1 s per layer. The actual instantaneous dose rates at isocenter in both systems may vary in a complex manner due to the facts that (i) the dose is delivered in layers, (ii) the beam is turned off between the layers, and (iii) the beam spot is scanned laterally across the field.

The data on dose delivery precision as a function of gantry angle for both systems are shown in Table I and in Fig. 10. (The data for the IBA system were obtained at Procure PTC in Oklahoma.) Figure 10 plots the variation of dose as a function of gantry angle expressed as a fraction of dose averaged over all gantry angles. Dose delivery precision in a gantry is an important characteristic since machine calibrations (output factors) are usually performed at one angle and the machine output is assumed to be constant for all gantry angles. The results for IU indicate higher output sensitivity to the gantry angle, which means that the absorbed dose at isocenter per 100 MU delivered may vary by ±1%. The higher sensitivity of the IU system to gantry orientation is explained by the IU beam monitor (wide aperture ion chamber) design: when the gantry rotates, the flexing of the monitor’s thin walls under gravity (estimated at ±30 μm as discussed in Ref. 12) gives rise to relatively larger variations in the monitor output due to a smaller separation of the electrodes in the IU beam monitor (3 mm vs about 10 mm in the IBA beam monitor). The IU gantry dependence data reported in Table I is for the commissioned system. This issue was studied in detail,¹² and a new dose monitor ion chamber was designed and tested,¹³ reducing the gantry angle sensitivity in the IU system to ±0.5%, a result comparable with the IBA data. The larger of the two numbers is reported in Table I for the IU system because the redesigned dose monitor has not been yet commissioned for clinical use.

FIG. 10.

Dose calibration as a function of gantry angle for the IU and IBA systems.

While the larger separation of the electrodes in the IBA beam monitor mitigates the effect of gantry angle dependence, extra care is advisable during beam delivery at high dose rates with the IBA system since the recombination effect, which is inversely proportional to the applied bias voltage (800 V in the IBA system vs 1500 V in the IU system) and increases proportionally to the square of the distance between the ion chamber electrodes, may become the cause of a relatively narrow dose rate interval where the beam monitor is linear. Although the study of beam monitor’s linearity in dose rate for either system was beyond the scope of this work, we think it is worth reminding the reader of potential limitations the increased electrode separation may cause.

IV. CONCLUSIONS

We have compared radiation field characteristics of two uniform scanning systems on a gantry, one developed at IU and the other produced by IBA. While the two systems exhibit similar performance characteristics, a few differences may be identified.

The IBA system has a greater penetration range and the capability of full SOBP modulation. The IU system, although providing the maximum commissioned SOBP of only 17 cm in water, can be easily upgraded to full modulation (within its range of 27 cm in water) by creating a corresponding range modulator control file with appropriate dose layer weights. The IU system has greater SAD and beam spot FWHM values leading to smaller lateral in-air penumbra at the 27 cm range, and unlike the IBA system, the virtual source position values are equal for both IP and CP directions, reducing the uncertainty of the dose planning model. Pristine beam properties (PER, FWHM, distal fall-off) are very similar for both systems. The IU lateral penumbra in the large modulated field are larger than those in the IBA system but are smaller for pristine beams and always are equal in the IP and CP planes. The IU scanning system can deliver a dose under reference conditions faster, has higher scanning frequency and a more flexible algorithm of selecting scanning patterns and scanning field size, thus reducing the aperture overscan margin and providing a means of reducing secondary neutron background.

Considering the differences in the implementation of scanning, in the future, it would be of interest to perform a direct comparison of neutron background near the clinical dose distributions provided by the two systems.

Radiation field characteristics of both uniform scanning systems meet their clinical specifications and show comparable results. Our general conclusion is that most of the differences observed between the two systems are clinically insignificant.

The successful implementation of the IBA and IU systems demonstrates that the problem of uniform scanning has more than one solution. While other, more sophisticated methods such as intensity-modulated pencil beam scanning have already been implemented in proton radiotherapy, we believe that uniform scanning, which evolved as a logical step forward from passive beam spreading, will remain a useful and viable option in proton therapy, especially in situations where organ motion is insignificant or mitigated.