An empirical test of the aggregation model of coexistence and consequences for competing container-dwelling mosquitoes

Abstract

We investigated the aggregation model of coexistence as a potential mechanism explaining patterns of coexistence between container mosquitoes Aedes albopictus and Aedes aegypti in southern Florida. Aedes aegypti coexists with the invasive A. albopictus in many locations despite being an inferior resource competitor under most conditions. In agreement with aggregation theory we observed significant intraspecific aggregation of A. albopictus in all six field sites sampled in southern Florida in 2009. Quantitative results suggest that larval distributions of A. albopictus across containers are sufficiently aggregated to permit persistence of the inferior competitor A. aegypti. We tested whether observed levels of A. albopictus aggregation would significantly improve A. aegypti population performance in a controlled laboratory competition experiment manipulating A. albopictus aggregation while holding mean densities constant. We quantified A. aegypti’s estimated rate of population change for replicate, multi-container cohorts in response to increasing A. albopictus aggregation across the cohorts. Aedes albopictus aggregation treatments produced J statistics for aggregation that spanned the range observed in the field study. We demonstrate a positive linear relationship between intraspecific aggregation of the superior competitor A. albopictus and estimated rate of population change for cohorts of the inferior A. aegypti. Thus, aggregation of A. albopictus at levels comparable to those observed in nature appears to be sufficient to reduce significantly the competitive impact of A. albopictus on multi-container cohorts of A. aegypti, and may therefore contribute to local coexistence of these competitors.

Introduction

When competing species occupy discrete habitat patches or resources, distributions of individuals among those patches are predicted to influence competitive interactions and the likelihood of coexistence (Atkinson and Shorrocks 1981, Ives 1988, Chesson 2000, Inouye 2005). For example, if a superior competitor is aggregated in a fraction of available patches, unoccupied patches may serve as refuges for inferior competitors, facilitating coexistence via decreased interspecific competition. Intraspecific competition also necessarily increases with spatial aggregation of a species. These conditions, increasing intraspecific competition and decreasing interspecific competition, are widely accepted as general stabilizing mechanisms that facilitate coexistence of competitors (Chesson 2000, Chase and Leibold 2003, Adler et al. 2007).

Aggregation theory was developed to clarify this relationship between spatial distributions and competitor coexistence in discrete habitats. Under the aggregation model of coexistence, intraspecific aggregation describes the degree to which a species is clumped among a set of available patches while interspecific aggregation is the degree to which different species covary or occur in the same patch (Ives 1991). Theory suggests that when intraspecific aggregation increases relative to interspecific aggregation, the strength of interspecific competition decreases compared to intraspecific competition and it should be easier for species to coexist (Atkinson and Shorrocks 1981, Hanski 1981, Ives 1991). These predictions arise from multiple bodies of theory (Atkinson and Shorrocks 1981, Ives 1988, 1991) and aggregated distributions have been observed, and suggested to be important, in dung beetles (Hanski 1986), carrion flies (Ives 1991), and drosophilids occupying fruit and mushroom patches (Jaenike and James 1991, Wertheim et al. 2000). Aggregation has also been positively correlated with community diversity (e.g., Krijger and Sevenster 2001), suggesting that this may be an important and general mechanism structuring natural communities distributed among discrete habitat units. Despite these observations and theoretical support for aggregation as a coexistence mechanism, the specific prediction of the aggregation model of coexistence, that aggregation of the superior competitor reduces interspecific competitive impact, has not been tested experimentally for organisms in discrete resource patches (but see Stoll and Prati 2001, Idjadi and Karlson 2007 for empirical tests of effects of aggregation on competition using sessile organisms in continuous habitat). One of the goals of this paper is to provide such a test in an appropriate model system.

The aggregation model depends on nonrandom distributions of species across patches such that individuals encounter conspecifics more than heterospecifics (Ives 1991). This situation is similar to what is required for coexistence via classical resource partitioning, except that in aggregation theory patches are considered identical, whereas resource partitioning depends on species differentially using multiple resources (Ives 1988, 1991). This has made aggregation an attractive alternative to resource partitioning for explaining high species-diversity in communities with patches unlikely to differ greatly. There is considerable evidence that aggregated distributions do occur naturally in systems with similar patches (e.g., carrion, rotting fruit, mushrooms; see references above). Natural and artificial water-holding containers similarly support a high diversity of aquatic invertebrates in a community of discrete and often similar habitat patches with limited resources (e.g., Srivastava et al 2004, Blaustein and Chase 2007, Yee et al. 2007) and sometimes intense local competition (e.g., Juliano 1998, Juliano et al. 2004).

In this paper we test aggregation as a potential mechanism of coexistence using two medically-important, container-dwelling mosquitoes, Aedes albopictus and Aedes aegypti as a model system. Both species are vectors of dengue virus and multiple other arboviral zoonoses (Lounibos 2002), and have similar life histories, occupying water-filled, human-made containers such as cemetery vases and discarded tires as larvae (Juliano et al. 2004). Such human-made containers are ideal for testing the aggregation model because there is strong evidence for competition in nature (Juliano et al. 2004), discrete habitats can be created and manipulated in realistic ways in both the field and the laboratory (e.g., Juliano 1998, Costanzo et al. 2005), and a role of aggregation in coexistence in this system has been suggested by observational studies (Leisnham and Juliano 2009). Srivastava et al. (2004) described many advantages of using natural microcosms for testing ecological theory, including aggregation models. Although the containers used in our system are human-made, and hence not “natural microcosms,” the competitors involved are natural colonists of these wide-spread, common habitats, thus constituting a natural assemblage of interacting species.

Aedes albopictus originated in Asia and invaded the United States in 1985 (reviewed by Lounibos 2002). It has spread throughout the southeastern US where it has interacted with resident A. aegypti. Aedes aegypti populations were locally extirpated in rural and suburban locations shortly after the arrival of A. albopictus in Florida and elsewhere (O’Meara et al. 1995, Lounibos 2002), a result consistent with laboratory and field experiments showing clear competitive superiority of A. albopictus larvae under limited resource conditions (reviewed by Juliano 2009, 2010). However, in many urban locations, A. albopictus and A. aegypti have coexisted over multiple years (O’Meara et al. 1995, Rey et al. 2006). Several alternative explanations of this pattern have been postulated, with varying degrees of support (e.g., Costanzo et al. 2005, Murrell and Juliano 2008, Lounibos et al. 2010), yet the mechanisms contributing to coexistence are still incompletely understood. Despite obvious similarities to other patchy systems (e.g., fruit and mushrooms; Hartley and Shorrocks 2002), the aggregation model of coexistence has not been evaluated as a potential contributor to coexistence of A. albopictus and A. aegypti (but see Leisnham and Juliano 2009).

Our study has two goals: First, to determine if natural larval distributions of A. albopictus and A. aegypti are aggregated and if this aggregation is sufficient to contribute to observed coexistence and exclusion patterns in southern Florida, USA. To test this we sampled distributions at three cemeteries in which A. aegypti disappeared following A. albopictus invasion, and three in which A. albopictus and A. aegypti coexisted for multiple years following invasion. Standard aggregation indices derived from theory on aggregation and coexistence (Ives 1991, Sevenster 1996) were estimated for both species at each site. The second goal was to test the primary predictions of aggregation theory: that intraspecific aggregation of a superior competitor reduces competitive impact on an inferior competitor and simultaneously increases the intraspecific competitive impact of the superior competitor (Stoll and Prati 2001). To test these predictions, we conducted a controlled, laboratory competition experiment in which we manipulated aggregation of the superior competitor, A. albopictus, across a set of homogeneous containers, and then tested for effects of aggregation on population performance of the inferior competitor, A. aegypti, or the superior competitor, A. albopictus. Quantitative measures of A. albopictus aggregation in this experiment (see Methods) spanned levels observed in the field study, so that the relevance of aggregation and potential for coexistence in nature could be inferred. Thus, we provide the first experimental test of predictions of the aggregation model of coexistence in an animal system occupying discrete habitats.

Methods

Field Sampling

Proportion of A. aegypti-positive containers and coexistence vary along an urban-rural landscape gradient, with high A. aegypti densities and coexistence with A. albopictus in urban locations and low densities or exclusion of A. aegypti at rural sites (O’Meara et al. 1995, Rey et al. 2006). To represent A. albopictus aggregation at both site types, three urban and three rural Florida cemeteries with known history of invasion by A. albopictus were sampled (see Murrell et al. 2011 for maps of cemetery locations). Rose Hill Memorial Park, Tampa (RH), Palmetto City Cemetery (PM), and City of Fort Myers Cemetery (FM) were selected as urban/coexistence sites based on long-term persistent A. aegypti populations following invasion of A. albopictus (O’Meara et al. 1995, Juliano et al. 2004). Oak Hill Cemetery, Lakeland, (OH), Joshua Creek Cemetery near Arcadia, (JC), and Fort Denaud Cemetery near Fort Denaud, (FD) were selected as rural/exclusion sites where A. aegypti disappeared following A. albopictus invasion, and has been consistently absent in sampling at these sites for multiple years, while large A. albopictus populations have persisted (O’Meara et al. 1995, Juliano et al. 2004, Lounibos et al. 2010). Cemeteries were sampled once during the early rainy season (June 3–10) and again during mid rainy season (July 20–24), 2009.

Water-holding containers at each location were sampled using the wandering-quarter method (Catana 1963) until 30 containers were collected or no water-holding containers remained. Each container’s contents were transferred to plastic bottles and taken to the laboratory for processing. Abiotic characteristics recorded for each container included: container type (plastic, metal, ceramic, glass, stone), water volume, detritus types (herbaceous, coniferous, deciduous, insect), total volume of detritus, canopy cover (quantified using spherical densiometer), and total nitrate, phosphorous, and carbon content of fine particulate organic matter in the water (methods described by Murrell et al. 2011, APHA 1998). Macroinvertebrates were identified and counted. Mosquito larvae and pupae were identified to species. Mosquitoes were predominantly A. albopictus and A. aegypti (> 90% of individuals overall) although small numbers of A. triseriatus, Culex sp., and Toxorhynchites rutilus were present (32/326 total samples contained mosquitoes other than A. albopictus and A. aegypti). Non-mosquito macroinvertebrates were: Telmatoscopus sp. (Psychodidae), Culicoides sp. (Ceratopogonidae), Phoridae sp., Chironomidae sp., Oligochaeta sp., Ostracoda sp., Dytiscidae sp., and muscoid Diptera sp.

Quantifying Aggregation

We used the J-index (Ives 1991) to quantify intraspecific aggregation of A. albopictus and A. aegypti immatures at each cemetery:

$$J=\frac{\frac{\sum n_i(n_i-1)}{N}-m}{m}$$ Eq. 1

where n_i is the number of individuals in vase i, m is the mean of n_i (and the expected number of competitors per vase for a random distribution) and N is the total number of individuals across all vases. J in eq. 1 measures the proportional increase in conspecifics encountered by an individual relative to a random or Poisson distribution. When J = 0, individuals are randomly distributed, whereas J = 0.75 indicates a 75% increase in the expected number of conspecifics encountered in a vase (Ives 1991). J < 0 describes a uniform distribution (Inouye 2005).

The formulation of J in eq. 1 assumes that all patches are identical (Ives 1991). However, patches in nature are variable and may differ in size or carrying capacity despite being functionally similar. Thus Sevenster (1996) proposed a modification of J in eq. 1 to account for ‘the experience of density’ within a patch:

$$J_M=\left(\frac{\sum e_i}{N^2}·\frac{\sum n_i(n_i-1)}{e_i}\right)-1$$ Eq. 2

where e_i is the size of patch i expressed in any unit that is proportional to carrying capacity. In cemetery vases, the experience of density is likely to depend on multiple factors such as water volume, nutrient availability, and the presence and abundance of competitors. As we have no a priori function for determining a species’ experience of density in a vase, we used stepwise model selection of observed vase characteristics (GLMSELECT procedure, SAS v.9.2) to develop a linear model predicting the expected number of Aedes larvae (A. albopictus+A. aegypti+A. triseriatus) in a vase. We used AICc as the selection criterion and ‘leave-one-out’ or ‘n-fold’ cross validation as a stopping criterion (SAS Institute 2008). This approach omits a single observation from the n total observations, and fits a model on the remaining n − 1 parts. The fitted model is then used to compute the predicted residual sum of squares on the omitted observation. This process is repeated for each of n parts, and the sum of the n predicted residual sum of squares is used as the prediction error at each step of the model selection to determine when to stop (see SAS Institute 2008 for details). All abundance variables were log transformed to improve normality and homogeneity of variances of residuals. From a total of 5 class variables and 15 continuous variables (Appendix B), we arrived at a model predicting total Aedes larval abundance in a vase based on 3 class (Cemetery, Sampling Period, Vase Type) and 9 continuous variables (Total Carbon, Percent Canopy Cover, Water Volume, Detritus Volume, log₁₀(C. quinquefasciatus+1), log₁₀(Culicoides+1), log₁₀(Oligochaeta+1), log₁₀(Phoridae+1), log₁₀(Ostracoda+1); see Appendix B). Interestingly, several invertebrate-abundance coefficients indicate a positive relationship with Aedes larval abundance. These positive relationships may suggest facilitation pathways, perhaps via processing chains (Paradise 1999, 2000) or may simply serve as indicators of container quality (e.g., if certain taxa are positively associated with resource-rich containers). We assume that predicted total Aedes abundance from this final model is proportional to the carrying capacity of the vase and use that prediction as our estimate of e_i in the calculation of J_M in eq. 2.

We used Ives’ (1991) C-index to quantify interspecific association between A. albopictus and A. aegypti at each cemetery:

$$C_{xy}=\frac{\frac{\sum n_{xi}{n}_{yi}}{N_x}-m_y}{m_y}=\frac{{\mathit{Cov}}_{xy}}{m_x{m}_y}$$ Eq. 3

where subscripts x and y indicate the two species and Cov_xy is the covariance between the species across all vases at a site. C_xy is comparable to J in that C_xy > 0 indicates an increase in the number of heterospecifics encountered relative to expectations of a random distribution of both species (Ives 1991). C_xy < 0 indicates that both species encounter each other less frequently than if they were randomly distributed (Ives 1991, Sevenster 1996). As with J, Sevenster (1996) developed a modification of C_xy to incorporate the experience of density:

$$C_M=\left(\frac{\sum e_i}{N_x{N}_y}·\sum \frac{n_{xi}{n}_{yi}}{e_i}\right)-1$$ Eq. 4

As in Eq. 2., predicted Aedes abundance from our stepwise model is used for e_i in Eq. 4.

We used Sevenster’s T_xy (1996) to quantify the ‘relative effect of aggregation of y on x’:

$$T_{xy}\equiv \frac{1+C_{xy}}{1+J_y}$$ Eq. 5

T_xy is a persistence criterion that determines whether a focal species x could persist given aggregation of its competitor (J_y) and its association (C_xy) with that competitor (Sevenster 1996) and T_xy < 1.0 is interpreted as a necessary and sufficient condition for the persistence of species x given J_y and C_xy (Sevenster 1996). As our interest is whether aggregation of A. albopictus facilitates persistence of A. aegypti, we calculated T_xy (T_aeg·albo) for A. aegypti alone (for both original and modified aggregation indices using J_M and C_M from eqs. 2 and 4 respectively).

To test further whether A. aegypti persistence is related to aggregation we calculated Pearson correlation coefficients between both J_albo and C_xy and the number of A. aegypti larvae per cemetery. We also tested whether A. aegypti dominance at a cemetery is related to aggregation by pairwise Pearson correlations between J_albo and C_xy and the proportion of all larvae that were A. aegypti (SAS Institute 2002, PROC CORR). We tested whether A. albopictus aggregation differs between rural/exclusion and urban/coexistence sites using a t-test (SAS Institute 2002, PROC TTEST).

We used the accelerated bootstrap (SAS Institute 2010; Dixon 2001) to estimate 95% confidence intervals for all aggregation indices and to test whether the parameters estimated by these indices differed significantly from 0 (or 1 for T_aeg·albo) at α = 0.05. The accelerated technique (SAS Institute Inc., 2010) was chosen because it corrects for bias and skewness, characteristics we observed in bootstrap distributions of J and C_xy.

Competition Experiment

Experimental replicates consisted of cohorts of 80 first-instar larvae per species distributed across eight-container arrays, with treatments consisting of different degrees of larval aggregation of A. albopictus. Our goal was to test whether increasing aggregation of A. albopictus affects performance of an 80-larva cohort of A. aegypti. Aedes albopictus aggregation across containers was manipulated while A. aegypti larvae were uniformly distributed across each eight-container array (i.e., containers 1–8 each contain 10 A. aegypti larvae). Four A. albopictus aggregation treatments were: zero (10, 10, 10, 10, 10, 10, 10, 10 larvae/container), low (20, 20, 20, 20, 0, 0, 0, 0 larvae/container), medium (40, 40, 0, 0, 0, 0, 0, 0 larvae/container), and high (80, 0, 0, 0, 0, 0, 0, 0 larvae/container) aggregation of A. albopictus larvae. Thus, each 80-larva cohort of A. aegypti encounters the same mean density of A. albopictus, but that density is distributed differently among the individual containers. A control with zero A. albopictus larvae (0, 0, 0, 0, 0, 0, 0, 0 larvae/container) was also included. The experiment was conducted in two temporal blocks with four replicates per treatment per block.

Experimental containers were 400 ml plastic beakers with 250 ml distilled water and 100 μl of tree-hole water as an inoculum for microorganisms. Each container received 0.713 g (±0.003 g) of senescent Quercus virginiana (live oak) leaves collected in 2009 from Vero Beach, FL and 0.038 g (±0.002 g) Gryllodes sigillatus (decorated cricket) carcasses obtained from a laboratory colony at Illinois State University. Leaves and crickets were dried at 60°C for 48 hours before addition to containers. Aedes albopictus and A. aegypti eggs from laboratory colonies of field collected mosquitoes from Tampa, FL (block 1: F1 generation; block 2: F2 generation) were synchronously hatched in a 0.15 g/l mixture of yeast/lactalbumin and added to experimental containers in the above combinations four days after the containers had been established. All containers were housed at 28°C and a photoperiod of 14:10 h (L:D).

Pupae were collected daily and transferred individually to glass vials for eclosion. We recorded date of eclosion, sex, and species, dried adults at 60°C for > 48 hours, then determined dry mass and wing length of all female A. aegypti. We continued until all A. aegypti had eclosed. In the first block of the experiment, we also collected eclosing adults of A. albopictus until all had eclosed or died (62 days). In the second block collecting all A. albopictus was not possible due to time constraints. For the first block only we were able to test for effects of A. albopictus aggregation on performance of A. albopictus.

We estimated the instantaneous rate of increase (r′) for each 80-larva cohort of A. aegypti across each eight-container array using Livdahl and Sugihara’s (1984) composite index of performance:

$$r^{\prime }=\left[\frac{ln\left[\left(\frac{{\sum }_x{A}_{x}f(w_x)}{N_0}\right)\right]}{D+\left[\frac{{\sum }_{x}x{A}_{x}f(w_x)}{{\sum }_x{A}_{x}f(w_x)}\right]}\right]$$ Eq. 6

where N₀ is the initial number of females per 80-larva cohort (assumed to be 50% of the larvae), A_x is the number of females eclosing on day x, w_x is mean wing length of females eclosing on day x, and D is the estimated time between eclosion and adult reproduction, assumed to be 12 days for A. aegypti (Livdahl and Sugihara 1984, Juliano 1998). The function f(w_x) describes size-dependent fecundity and is: f(w_x) = 2.50(w_x)³ − 8.616 for A. aegypti (Briegel 1990).

The effect of A. albopictus aggregation on A. aegypti estimated rate of increase was analyzed in two ways. First, we performed a one-way randomized block ANOVA, treating A. albopictus aggregation treatments as fixed categorical variables and block as a random effect. We evaluated significant treatment effects by pairwise comparisons of treatment means adjusted for multiple comparisons using Ryan-Einot-Gabriel-Welsch multiple range at experimentwise α = 0.05 (SAS Institute 2008, PROC GLM).

We also tested for a linear relationship between A. albopictus aggregation and A. aegypti r′ using a linear model (SAS Institute 2008, PROC GLM), with intraspecific aggregation of A. albopictus quantified as unmodified J_albo from eq. 1 (as in this experiment, all containers should have identical carrying capacities) treated as a continuous variable and block treated as a class variable. J_albo values for the four aggregation treatments were: zero, −0.1; low, 0.9; medium, 2.9; and high, 6.9. We first tested a model including treatment×block interaction. As that interaction was not significant (see Results) we removed it forcing a common slope between the blocks, and resulting in an ANCOVA-like model but with the focus on the slope (SAS Institute 2008, PROC GLM). We then tested if the common slope differed significantly from zero as a test for the effect of aggregation of A. albopictus on performance of A. aegypti. We also tested a quadratic function to see if this resulted in improved fit compared to a simple linear relationship.

Overall impact of aggregation on interspecific competition for the 80-larva cohort across the eight–container array is a result of the combined effects of interspecific competition within single containers having different A. albopictus densities. We evaluated the relationship of interspecific competition in a single container to A. albopictus density by determining the effect of A. albopictus density on A. aegypti estimated rate of increase within single containers. We had five unique A. albopictus: A. aegypti density combinations (0:10, 10:10, 20:10, 40:10, 80:10), which were treated as classes in a randomized block ANOVA. The design was unbalanced due to unequal replication of density treatments and thus F-tests were calculated using Satterthwaite’s approach (Juliano et al. 2004, SAS Institute 2008). For r′ for individual containers, no transformation yielded data that met assumptions of normality and homogeneity of variance; hence we also used a randomization ANOVA (Cassell 2002). The same significant effects were identified by both parametric and randomization ANOVAs, hence we only report the results of the parametric analysis. We evaluated significant treatment effects with pairwise comparisons of least squares means (Tukey-Kramer method, experimentwise α = 0.05; SAS Institute 2008).

We also determined the index of performance r′ (eq. 6) for each 80-larva cohort of A. albopictus in block 1 of the experiment. For A. albopictus, the function f(w_x) for size-dependent fecundity is: f(w_x) = 78.02w_x − 121.24 (Lounibos et al. 2002), and time from eclosion to oviposition is estimated to be D = 14 days (Livdahl & Willey 1991). We analyzed effects on A. albopictus performance by one way randomization ANOVA (Cassell 2002) on a transformation of r′: λ′= exp(r′), which estimates the finite rate of increase for a cohort. This transformation has the advantageous property of being estimable even when no females survive to reproductive age (λ′ = 0), a condition that was common for A. albopictus in this experiment, and which renders r′ inestimable (r′ = −∞) (Juliano 1998). Randomization tests were necessary because of extreme non-normality of the residuals. This analysis thus tests the effect of aggregation of the superior competitor on its own performance.

Results

Field Sampling

Larval densities of both species were generally higher in the second sampling period (Table 1). Aedes aegypti densities were highly variable across cemeteries with very low numbers in rural cemeteries and relatively high densities in urban locations (Table 1). We also report the first recorded reappearance of a small number of A. aegypti in all three rural cemeteries (FD, JC, OH) since its disappearance following invasion by A. albopictus (O’Meara et al. 1995).

Table 1.

Aggregation of resident Aedes larvae in 6 Florida cemeteries. Cemeteries were sampled once in early rainy season (1) and in the middle to late rainy season (2) of 2009. Thirty water-filled vases per cemetery were sampled (15 at Palmetto). Aggregation indices were calculated for A. albopictus and A. aegypti and modified to account for patch-size using predicted Aedes abundance (see text for details).

	Larvae per Cemetery		Aggregation
	A. albopictus	A. aegypti	Jalbo	Jaeg	Caeg·albo	Taeg·albo
FD1	332	26	0.86 (0.29, 5.94)*	1.74 (0.78, 7.20)	0.72 (0.16, 4.83)	0.93 (†, 1.31)
FD2	1428	16	4.58 (1.56, 17.20)	17.07 (−0.76, 78.20)	1.34 (−0.01, 5.56)	0.42 (0.07, 0.95)
FM1	220	44	21.25 (1.64, 48.00)	2.69 (0.28, 7.03)	−0.34 (−0.91, 0.36)	0.03 (†, 0.56)
FM2	905	255	6.37 (2.47, 17.60)	8.37 (2.53, 29.70)	5.43 (0.99, 20.40)	0.87 (0.32, 1.45)
JC1	604	7	4.10 (1.49, 11.70)	84.18 (36.20, 140.50)	16.93 †	3.51 (2.34, 4.70)
JC2	1099	0	1.31 (0.71, 2.41)	NA	NA	NA
OH1	449	0	3.79 (1.89, 8.81)	NA	NA	NA
OH2	1489	23	2.05 (1.16, 3.84)	54.78 (24.40, 89.70)	4.68 †	1.86 (†, 3.01)
PM1	0	107	NA	2.55 (0.33, 32.70)	NA	NA
PM2	2	111	5.15 (1.97, 14.90)	0.88 (0.16, 3.90)	1.33 †	0.38 (†, 1.52)
RH1	186	147	1.41 (0.59, 3.57)	2.00 (0.81, 4.64)	0.17 (−0.33, 0.99)	0.49 (0.18, 0.79)
RH2	494	604	6.83 (0.66, 22.80)	4.32 (0.92, 19.70)	5.08 (0.52, 19.10)	0.78 (0.54, 1.00)

^*Numbers in parentheses are 95% confidence intervals determined using accelerated bootstrap method from 2000 bootstrap samples per statistic.

^†Confidence intervals inestimable due to skewed bootstrap distributions.

Bootstrapped 95% confidence intervals indicated significant intraspecific aggregation of A. albopictus (i.e., lower bound of CI for J > 0) for both sampling periods at all cemeteries with sufficient larvae for calculations (Table 1). A. aegypti larvae were also highly aggregated with significant aggregation in all but one instance (Fort Denaud in the mid rainy season; Table 1). Unmodified J-indices were similar with significant aggregation at all sites for both species for both sampling periods (Appendix A). Low larval abundances at several cemeteries resulted in non-estimable confidence intervals for interspecific aggregation between A. albopictus and A. aegypti (C_M) at six of twelve cemetery-season samples (7/12 for unmodified calculation). Of samples in which C_M was estimable, association between these species was not distinguishable from random (i.e., lower bound of CI for C_M < 0) at Fort Myer’s and Rose Hill Cemeteries in the early rainy season and at Fort Denaud in the mid rainy season (Table 1). At Fort Myer’s and Rose Hill, lower confidence limit bounds exceeded zero in the second sampling period (mid rainy season 2009), suggesting a shift to a positive association between A. albopictus and A. aegypti later in the year (Table 1, Appendix A).

Confidence intervals for the persistence criterion of A. aegypti in the presence of A. albopictus (T_aeg·albo) were estimable in 5/12 cemetery-season combinations (4/12 for unmodified calculations). Of these, upper bounds were ≤ 1.0 for both Rose Hill samples and the second Fort Denaud sample, suggesting favorable conditions for A. aegypti persistence at these sites (Table 1). These same confidence intervals were not significantly less than 1.0 in the calculation of T_aeg·albo from unmodified indices, though the actual estimates were less than 1.0 (Appendix A).

Abundances of the two species across cemetery samples were not significantly correlated (r = −0.171, P = 0.5955, n=12). For the modified aggregation indices (eqs. 2, 4), abundance of A. aegypti was not significantly correlated with intraspecific aggregation of A. albopictus (J_albo), r = 0.113 (n = 11), P = 0.7404, or interspecific aggregation (C_M), r = 0.004 (n = 9), P = 0.9913. There was also no significant correlation between J_albo or C_M and proportion of larvae that were A. aegypti at a cemetery: J_albo, r = 0.09 (n = 11), P = 0.7922; C_M, r = −0.288 (n = 9), P = 0.4526. A t-test indicated no significant difference in J_albo between rural/exclusion and urban/coexistence sites (t₉ = 1.72, P = 0.1193). Conclusions from correlations and t-test using the unmodified indices were identical and are not shown.

Competition Experiment

ANOVA revealed a significant effect of A. albopictus aggregation on A. aegypti estimated rate of increase (r′) as well as a significant block effect (Table 2). There was no significant interaction of treatment and block; hence pairwise comparisons were conducted on treatment means averaged across blocks. Although r′ was > 0 for all treatment groups, A. aegypti performance was greatest in the control treatment, indicating that all cohorts with A. albopictus present were negatively affected by interspecific competition (Fig. 1). Among cohorts that were exposed to A. albopictus competition, the uniform distribution of A. albopictus (no aggregation) resulted in the poorest performance by A. aegypti while the high aggregation treatment affected A. aegypti performance the least (Fig. 1). Two intermediate aggregation treatments (low and medium) were indistinguishable and fell between the uniform and high aggregation treatments.

Table 2.

(a) Randomized block ANOVA on estimated rate of increase (r′) of cohorts of 80 A. aegypti larvae in response to intraspecific aggregation treatments of A. albopictus. The degrees of freedom for each denominator MS are listed as the second value under ‘df.’ (b) Randomized block ANOVA on A. aegypti rate of increase (r′) within single cups in response to A. albopictus density treatments. The degrees of freedom for each denominator MS are listed as the second value under ‘df.’ Non-integer degrees of freedom result from using Satterthwaite’s Formula for F-tests which uses weighted sums of mean squares as the denominator of the F-test. This formulation is necessary due to an unbalanced design incorporating random effects.

Source	df	F	P
(a) A. albopictus aggregation (across cohort)	4, 4	111.40	0.0002
Block	1, 4	284.44	<0.0001
Treatment × Block	4, 30	0.14	0.9668
Error	30
(b) A. albopictus density (single cup)	4, 4	178.63	<0.0001
Block	1, 71.29	19.69	<0.0001
Treatment × Block	4, 309	0.32	0.8662
Error	309

Figure 1.

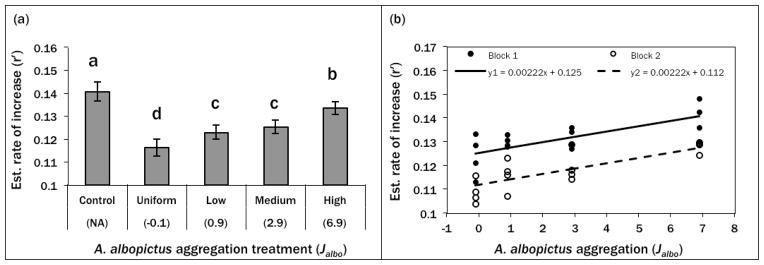
(a) Summary means of randomized block ANOVA testing effect of A. albopictus aggregation on A. aegypti rate of increase (r′). Bars are combined treatment means for 2 experimental blocks and error bars indicate standard error. Means with same letter are not significantly different (P > 0.05). (b) Linear model testing relationship between A. albopictus aggregation and A. aegypti estimated rate of increase (r′). Absent block*treatment interaction permitted forced common slope for both blocks. Significant positive relationship (P < 0.001) and block effect (P < 0.001) with R² = 0.7039.

The common-slope linear model treating A. albopictus aggregation as a continuous variable was significant with R² = 0.70. The slope was significantly greater than zero (t₂₉ = 5.82, P = <0.0001) indicating a positive linear relationship between J_albo and A. aegypti r′ (Fig. 1). Fitting a quadratic function did not yield a significant 2^nd order coefficient (t₂₈ = −0.82, P = 0.4174) indicating that the linear model is the most parsimonious description of the relationship between J_albo and A. aegypti r′.

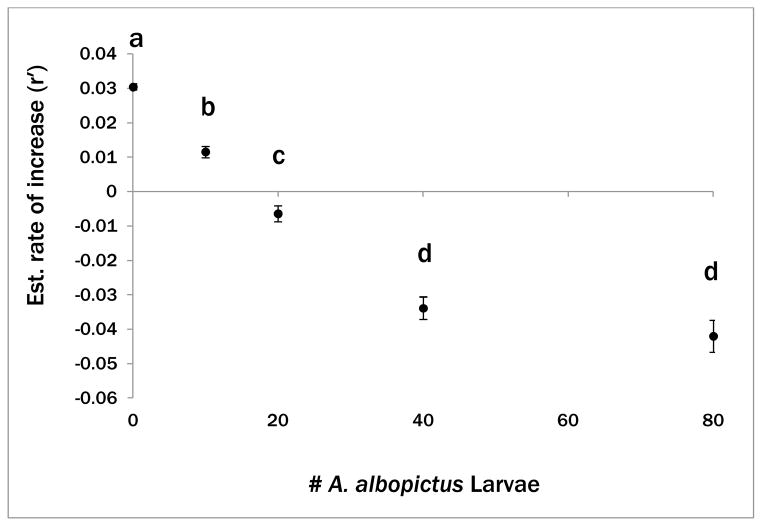
There was no significant interaction for the single-cup analysis and thus pairwise comparisons were again between treatments averaged over the two blocks (Table 2). Aedes aegypti performance was greatest in the 0:10 treatment and declined with increasing density of A. albopictus (Fig. 2). The two greatest densities, 40:10 and 80:10 resulted in the lowest estimated rate of increase for A. aegypti but were not distinguishable from each other.

Figure 2.

Summary means of randomized block ANOVA testing effect of A. albopictus density on A. aegypti rate of increase (r′) within a single container. Points are combined treatment means for two blocks and error bars indicate standard error. Means with the same letter are not significantly different (P > 0.05).

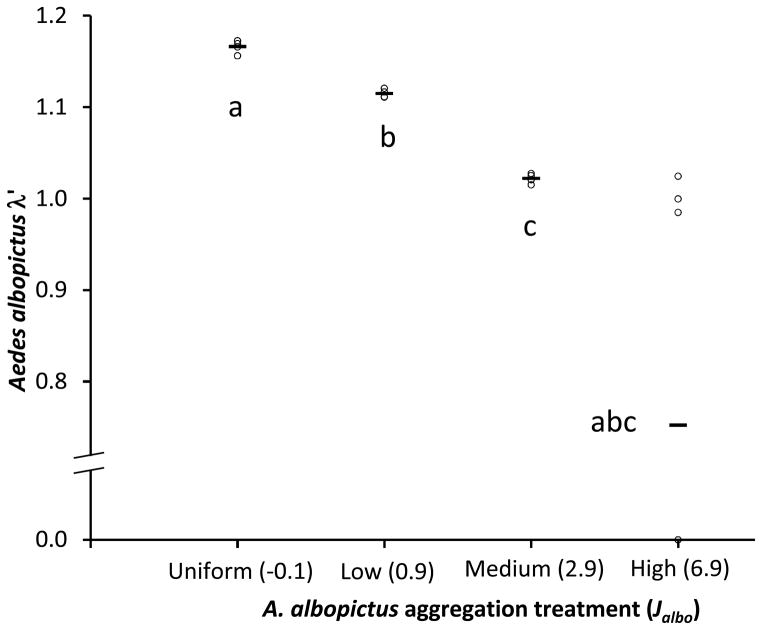
In block 1, A. albopictus λ′ was significantly affected by aggregation (P=0.0001) and declined as aggregation increased (Fig. 3). When level of aggregation was at its highest (J_albo = 6.9), λ′ was highly variable, and the mean fell well below 1.0, suggesting these cohorts of A. albopictus would be expected to decline (Fig. 3). Three of four replicate cohorts at this level yielded λ′ < 1.0 and one yielded no surviving adult females (λ′ = 0) (Fig. 3). For uniform, low, and medium aggregation, λ′ values did not overlap, and all differed significantly, but none differed from the mean for high aggregation (Fig. 3).

Figure 3.

Effect of Aedes albopictus aggregation on an index of performance λ′ for 80-larva cohorts of A. albopictus. Horizontal bars are means of N=4 observations, and open circles are individual replicates for block 1 only (See text). Randomization ANOVA (Cassel 2002) is significant (P<0.0001). Means associated with the same letters are not significantly different by pairwise unequal variance t-tests, with Bonferroni adjustment for 6 tests.

Discussion

Although under most conditions A. albopictus is superior in resource competition to A. aegypti (Juliano 2009), these two species coexist in some locations in the southern US, particularly in the part of south Florida that we sampled (O’Meara et al. 1995, Juliano et al. 2004). Our samples of natural larval distributions at six cemeteries in southern Florida with a range of A. albopictus and A. aegypti densities, and our laboratory experiment on the effects of aggregation, suggest that aggregation of these species may contribute to coexistence in nature.

Distributions of both species were highly aggregated in the field, with A. albopictus larvae significantly aggregated for every sample site and time (for both modified (J_M•albo) and unmodified (J_albo) aggregation indices). Aggregation of a superior competitor is predicted to facilitate the persistence of an inferior competitor (Ives 1991, Sevenster 1996, Wertheim et al. 2002); however, we did not observe a significant correlation between aggregation of A. albopictus and A. aegypti abundance. We also calculated a persistence criterion T_aeg·albo to determine if A. aegypti would be expected to persist given A. albopictus aggregation, however T_aeg:albo was also not clearly associated with A. aegypti abundance. Although all estimates of T_aeg:albo at coexistence/persistence sites were less than 1.0, a necessary and sufficient condition for competitor persistence, these were only significantly less than 1.0 at Rose Hill Cemetery. Interestingly, despite the long-term absence of A. aegypti at Fort Denaud, T_aeg·albo was also significantly less than 1.0 for the second sample at this cemetery. Whether this is related to the reappearance of A. aegypti at this site is unclear.

Interspecific association also influences competitive interactions and the likelihood of coexistence such that a negative or independent association between competitors facilitates coexistence while a positive association makes persistence of an inferior competitor more difficult (Ives 1991, Hartley and Shorrocks 2002). Leisnham and Juliano (2009) observed a negative association between these species in south Florida which is consistent with the aggregation hypothesis of coexistence, although they determined association only based on presence/absence, rather than larval abundances within individual vases. In contrast, though we were unable to estimate reliably interspecific aggregation at sites where one species was rare, three out of six estimates suggest independent association between A. albopictus and A. aegypti while the remaining observations suggest a positive association. In cemeteries with estimable values of C_aeg·albo for both sampling periods (FM and RH), the degree of interspecific association increased from independent association early in the rainy season to a positive association by the mid rainy season. This suggests that overlap between these species increases as larval densities increase throughout the rainy season, perhaps due to limited habitat availability. Increased overlap is expected to make coexistence more difficult, however, coexistence is still possible despite high positive correlations between competitors if the degree of intraspecific aggregation J is sufficiently high (Ives 1991).

These field results suggest that aggregation by itself does not provide a full explanation of patterns of persistence and exclusion of A. aegypti in nature. We observed no clear differences in A. albopictus aggregation or the persistence criterion T_aeg·albo in comparisons between urban/coexistence and rural/exclusion sites, as would be expected if aggregation fully determined coexistence patterns; though we note that the unexpected presence of A. aegypti at the 3 rural/exclusion cemeteries in which it has historically been absent, opens the question of the stability of apparent exclusion or coexistence. Nonetheless, our clear demonstration of A. albopictus aggregation in nature, and of the impact of A. albopictus aggregation on both interspecific competitive impact on A. aegypti larvae, and the performance of A. albopictus, is consistent with our hypothesis that aggregation of A. albopictus may create conditions favorable for persistence of the inferior resource competitor A. aegypti (Leisnham and Juliano 2009). Our results indicate that aggregation can be one stabilizing mechanism (Chesson 2000, Adler et al. 2007) that contributes to coexistence of these competitors. Other contributing mechanisms may include seasonal fluctuations in abiotic conditions or resources (Costanzo et al. 2005, O’Neal and Juliano 2012), local variation in resource availability (Murrell and Juliano 2008, Murrell et al. 2011), or local variation in abiotic conditions (Lounibos et al. 2010).

Our controlled laboratory experiment, which mimicked the degree of A. albopictus aggregation in the field, demonstrates that increasing aggregation of a superior competitor significantly releases an inferior competitor from competition, and also reduces performance of the superior competitor. Both effects potentially facilitate coexistence (Chesson 2000, Stoll and Prati 2001). We examined the performance of uniformly distributed A. aegypti larvae in response to increasing intraspecific aggregation of A. albopictus larvae, at a constant mean density of A. albopictus across the 8-container array. Our design enabled us to manipulate intraspecific aggregation of A. albopictus while holding intraspecific aggregation of A. aegypti (J_aeg = −0.1) and interspecific association (C_aeg·albo = 0) constant. Aedes aegypti larvae were not, of course, uniformly distributed in the field, and thus the effect of specific degrees of A. albopictus aggregation cannot be directly extrapolated to effects in the field. Nonetheless, A. aegypti performance consistently increased as A. albopictus aggregation increased (Fig. 1), exactly as predicted by aggregation theory (Atkinson and Shorrocks 1981, Ives 1991, Sevenster 1996). We note also that for all treatments, A. aegypti performance was significantly lower than the control with no A. albopictus, indicating that even the most extreme aggregation of a competitor may not result in full competitive release.

Our range of treatments produced J indices that spanned the majority of observed degrees of A. albopictus aggregation from the field, and even our low level of aggregation yielded a significant increase in A. aegypti performance compared to the uniformly distributed A. albopictus treatment (Fig. 1). These effects were observed despite overall high performance of A. aegypti cohorts (i.e., r′ > 0 for all treatments). This result suggests that increased aggregation of a competitor will benefit a species even under relatively favorable conditions. The observed increase in performance of one competitor in response to increased aggregation of another is likely a general phenomenon, independent of the relative competitive abilities of the two species. We chose to investigate A. aegypti as the focal species in this experiment because one of our goals is to understand the potential for this mechanism to explain patterns of coexistence in nature. As A. aegypti is predicted to be excluded by A. albopictus under many circumstances (reviewed by Juliano 2009), it was more interesting to test the response of A. aegypti to A. albopictus aggregation (rather than the opposite) to determine if this mechanism may contribute to persistence of the weaker competitor (see Idjadi and Karlson 2007).

Our experiment was designed to test for the effect of aggregation, and was not designed to quantify competitive effects or parameters of a particular competition model (i.e., we had only one density of A. aegypti, and except for the controls, only one density of A. albopictus). A more elaborate experiment manipulating both densities and degree of aggregation would be desirable; nevertheless, our experiment clearly indicates that aggregation of the superior competitor at levels observed in nature should facilitate coexistence of these competitors by the dual effect of releasing A. aegypti from the effects of interspecific competition, and reducing the performance of A. albopictus, probably via enhanced intraspecific competition, as has been documented in other systems (Stoll and Prati 2001).

We observed a positive linear relationship between the estimated rate of increase of A. aegypti and degree of A. albopictus aggregation as quantified by J (Fig. 1). To our knowledge this is the first report of the type of relationship between aggregation of one competitor and performance of another. It is unclear whether the linearity of this relationship is general or is instead particular to the species and conditions of our experiment. Similar experiments manipulating aggregation under other conditions or with other species will be essential for determining whether such linearity should be regarded as general for modeling effects of aggregation on coexistence.

Our analysis of individual containers shows that some A. aegypti larvae suffer disproportionately from interspecific competition when the superior competitor is aggregated (Fig. 2). The overall improvement of performance by A. aegypti cohorts across the 8-container array when A. albopictus are aggregated results from two effects of aggregation that combine to produce a population-level effect. There is a benefit of aggregation to those larvae escaping interspecific competition (0 A. albopictus containers), and this effect outweighs the detrimental effect of aggregation increasing interspecific competition on larvae where A. albopictus are aggregated (A. albopictus >10 in a container).

Aggregation has received considerable support as a mechanism of coexistence and diversity in several patchy systems that support competing species (Ives 1990, Krijger and Sevenster 2001, Wertheim et al. 2003). We similarly describe intraspecific aggregation of species that experience intense competition in a patchy system with limited evidence of significant resource partitioning. Though vases are highly variable (e.g., in vase material, water volume, amount and type of detritus, canopy cover) colonization by these species does not appear to differ with these characteristics, as their distributions across vases appear to show either independent or positive associations (Table 1). Aggregation of these species has previously been postulated to contribute to observed coexistence (Leisnham and Juliano 2009); however, until now larval abundances at the within-patch level have not been available. A further consideration in our system is the possibility of multiple spatial scales of aggregation influencing interactions between these species. Inouye (2005) showed strong intraspecific aggregation of insects among fruits of the tropical tree Apeiba membranacea at two spatial scales: locally, among fruits under a single tree; and regionally among the trees themselves. Aedes albopictus and A. aegypti do show patterns of large-scale regional spatial variation along an urban -rural landscape gradient and thus regional aggregation may influence coexistence in addition to local aggregation that we observed (Rey et al. 2005).

Our results provide an empirical demonstration that aggregation of a superior competitor can yield significant competitive release for a poorer competitor, and do so for a different type of system than those previously studied (Stoll and Prati 2001, Idjadi and Karlson 2007). Thus, the contribution of aggregation to coexistence of competitors could be a generally important phenomenon for a wide array of organisms. Although our field study did not yield a direct association between aggregation of the superior competitor A. albopictus and abundance of the inferior A. aegypti, we postulate that the consistent aggregation we observed in nature may help to create the conditions necessary for A. aegypti to persist in some locations. We also demonstrate that in this system, competitor performance increases linearly with aggregation, which should focus future modeling efforts on whether the form of such a relationship (linear, asymptotic, etc.) affects the ability of aggregation to foster coexistence of competitors, and focus future empirical studies on determining the shape of relationships of competitive release to quantitative measures of aggregation.