Gamete evolution and sperm numbers: sperm competition versus sperm limitation

Abstract

Both gamete competition and gamete limitation can generate anisogamy from ancestral isogamy, and both sperm competition (SC) and sperm limitation (SL) can increase sperm numbers. Here, we compare the marginal benefits due to these two components at any given population level of sperm production using the risk and intensity models in sperm economics. We show quite generally for the intensity model (where N males compete for each set of eggs) that however severe the degree of SL, if there is at least one competitor for fertilization (N − 1 ≥ 1), the marginal gains through SC exceed those for SL, provided that the relationship between the probability of fertilization (F) and increasing sperm numbers (x) is a concave function. In the risk model, as fertility F increases from 0 to 1.0, the threshold SC risk (the probability q that two males compete for fertilization) for SC to be the dominant force drops from 1.0 to 0. The gamete competition and gamete limitation theories for the evolution of anisogamy rely on very similar considerations: our results imply that gamete limitation could dominate only if ancestral reproduction took place in highly isolated, small spawning groups.

1. Introduction

The evolution of gamete numbers and gamete sizes has immense consequences for subsequent evolution [1], and understanding why males produce many, tiny sperm, whereas females produce relatively smaller numbers of much larger eggs is an important question in evolutionary biology [2–4]. The classical view was that males produce large numbers of sperm through selection to enhance the probability of fertilization. There is much evidence that fertility (the proportion of ova released that are fertilized) increases with both sperm density in the female tract of internal fertilizers (e.g. [5,6]) and sperm density in the surrounding medium of external fertilizers (e.g. [7]). Interest in sperm limitation (SL) has recently increased [8], and under some conditions found in nature, certain broadcast spawning invertebrates experience low levels of fertility owing to SL [9], while others show high fertility [10].

An alternative explanation [11] is that sperm numbers in a species may reflect post-copulatory competition between males for fertilizations, and much evidence now exists that relative testis size increases with increasing sperm competition (SC) levels across species [12]. Thus, both SL and SC appear to play a part in determining the number of sperm ejaculated. Furthermore, both gamete limitation and gamete competition have been claimed to be important drivers of the evolution of anisogamy from ancestral isogamy (i.e. the ancestral divergence of male and female gametes; reviewed by Lessells et al. [2]).

Here, we estimate the relative importance of SL and SC as evolutionary drivers of sperm numbers, by comparing the marginal gains arising from these two fitness components at any given state of sperm numbers in a population.

2. Models

Increasing sperm numbers can increase male fertilization gains, but these benefits (b) must be balanced against costs, such as reduced survival, missed encounters with receptive females, reduced ability to win combats with other males for access to females, or (and most relevant to the evolution of anisogamy from isogamy) if, as the gamete size of one mating type drops, the survival of the resulting zygote diminishes significantly due to decreased resources.

Our analysis resembles the evolutionarily stable strategy (ESS) approach [13]: we calculate the fitness of a mutant male that ejaculates s sperm in a population, where other males ejaculate sperm. At an ESS, marginal benefits balance marginal costs. Here, we are not concerned with the marginal costs, and wish only to consider the marginal benefits. In particular, for any given population state (which could be the ESS), we compare the marginal benefit that arises from SC with that from SL to determine which component is the major force.

When a male increases his sperm numbers, he increases his share of fertilizations relative to his rivals (i.e. the SC component), but also increases the total number of eggs that are fertilized (i.e. the SL component). Here, we isolate and compare the marginal benefits due to these two components using two models widely used in SC to make theoretical analyses tractable [3]. For high SC levels, the ‘intensity’ model assumes that in a population, N males typically compete for fertilization of each set of eggs. For low SC levels, the ‘risk’ model assumes that SC over a given set of eggs occurs between two males with probability q, and that there is no SC with probability (1 − q).

(a). Intensity

We begin with an ‘intensity’ approach, where N males compete for each set of eggs. The simplest interpretation is an external fertilizer with broadcast spawning, in local groups each containing N males, though an equivalent scenario would be an internal fertilizer with sperm storage, where each female receives N different ejaculates that compete equally for fertilization of her clutch.

Suppose that a mutant male releases s sperm in a group of N males, the other (N − 1) males each release sperm. The females in the same local population release E ova in total, and the mutant male gains a proportion 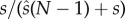of fertilized eggs if SC follows the ‘raffle principle’ [3].

We begin with a general approach. Call F(x) the function relating fertility (proportion of the E eggs that are fertilized) to total sperm density, .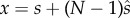

The mutant male's benefits are

2.1

and since

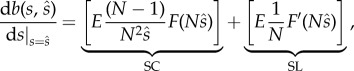2.2

where the term in the first square brackets corresponds to the marginal gain through SC, and the term in the second square brackets the marginal gain through SL.

If there are no sperm, no eggs are fertilized; i.e. F(0) = 0. Excluding the multiplicative constant E, the SC term can therefore be rewritten as

2.3

According to the mean value theorem (e.g. [14]), there exists a point c in the open interval 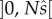, where

2.4

We shall assume that F(x) is a concave function, something that has theoretical as well as empirical support (e.g. [6,7]) and has been assumed in previous analyses (e.g. [11,15]). Then its derivative must be decreasing, and 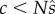implies that 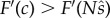. Thus,

2.5

The marginal gains through SC (2.2) are therefore larger than the marginal gains through SL (2.2) if

2.6

Thus provided that there is at least one competitor (N − 1 ≥ 1), SC is the dominant selective force driving increased sperm numbers, whatever the degree of SL.

Having derived a general result for concave functions, we next investigate a specific fertility function, to gain a more concrete, intuitive feel for the result. We use the negative exponential saturation curve

2.7

derived by Vogel et al. [7] as a plausible relationship between fertility and sperm density (see also [6]), where the constant k depends on the properties of the gametes and the fertilization process.

Then we can write the mutant's marginal benefits as

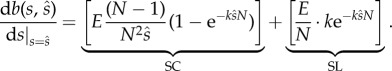2.8

The marginal gain due to SC exceeds that due to SL when

2.9

At any value for intensity, N, (2.9) can be used to assess the relative selective pressure due to SC and SL. As expected, if there is only one male in the group (no SC), (2.9) can never be satisfied, since the l.h.s. → 0 and the r.h.s. is positive, and when N is huge, 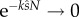(there is no SL) and (2.9) is always satisfied.

Note that 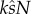forms a single term that defines the proportion of eggs fertilized, i.e. . Then 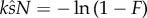, and for (2.9) to be satisfied

2.10

As established by our general result for concave functions, condition (2.10) implies that for SC to be the dominant selective force determining sperm numbers (whatever the fertility level F) requires only that there is at least one competitor. Figure 1 (dashed curve) shows the threshold number of competitors (N − 1) above which marginal gains through SC are greater than those through SL, which falls in the interval ]0, 1[. However, the intensity model is somewhat unrealistic in this range, since strictly speaking (N − 1) should be an integer. To investigate more accurately, we next analyse the risk model.

Figure 1.

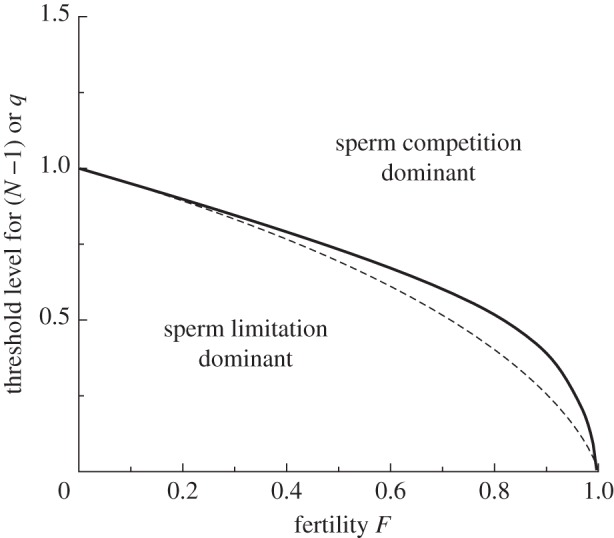
The threshold number (N − 1) of competing males (dashed line), or risk (p) of a competing male (solid line), for SC to give the same marginal gain as SL in relation to the fertility level in a species, F. For species falling above the line, SC is the dominant pressure increasing sperm numbers, and below the line SL is dominant. These results are derived using a negative exponential fertility function [6,7]. More generally, any concave fertility function ensures that the presence of at least one competing male makes SC stronger than SL (see main text).

(b). Risk

In risk models, the population level of SC is usually expressed as q, the proportion of eggs fertilized when two different males ejaculate (i.e. there is SC for those eggs), where (1 − q) is the proportion with only one male present (i.e. no SC). The probability that a given male experiences SC, p, is not equivalent to q, the probability that a given set of eggs faces SC. For each set of E eggs fertilized, one ejaculate is present with probability (1 − q), and two ejaculates with probability q, giving (1 − q) + 2q = (1 + q) ejaculates per set of eggs. Thus, when a male ejaculates, he faces SC on 2q occasions out of a total of (1 + q) occasions, hence the probability that a male faces SC is p = 2q/(1 + q) [16].

Thus now mutant fitness is a little more complex, and it is useful to arbitrarily define two terms for s = s_a = s_b, where s_a relates to how the mutant's sperm numbers affect his gains through SC, and s_b relates to how the mutant's sperm numbers affect his gains through SL. Then

2.11

Evaluating db/ds_a at 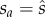, and substituting p = 2q/(1 + q) gives

2.12

and similarly, db/ds_b evaluated at 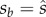gives

2.13

Marginal SC gains exceed those through SL if (2.12) > (2.13), i.e. if

2.14

Note here that 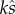remains as a single constant, which again relates to the proportion of eggs fertilized, i.e. 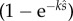when there is no SC and 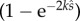when two ejaculates compete. Note also that (2.14) is equivalent to (2.9) at integer values where the two models meet, i.e. when q = 1 and N = 2, and at q = 0 and N = 1.

Let 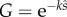be the proportion of unfertilized eggs when one male ejaculates; G² is the proportion when two males ejaculate. Hence 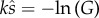, and (2.14) can be written as

2.15

and

2.16

The probability of SC over a specific set of eggs, q, determines the average fertility for the population, i.e.

2.17

Analogous to the intensity model, our aim is to plot the threshold level of SC (previously N − 1, now q) as a function of fertility (F). We were unable to obtain the threshold q as an explicit function of F from (2.16) and (2.17), but q(F) can be obtained by numerical computation, and is shown as the thick solid curve in figure 1. As expected, the threshold level in the risk model is higher than in the intensity model, particularly at intermediate to high fertility levels, though the intensity model can be seen to be a fair approximation at low or very high fertility levels.

3. Discussion

It is important to bear in mind that when gamete competition and gamete limitation do act simultaneously, they act in the same direction: to increase gamete numbers. Cohen [17,18] argued that sperm numbers are too high to account for by SL, and claimed that they reflected ‘redundancy’ due to meiotic errors (the number of sperm that fail to fertilize ova increased with the mean chiasmata frequency across species). Parker [11] attempted to calculate whether SL could account for sperm numbers from empirical data on sperm dilution for artificial insemination in cattle, but his original analysis was flawed [4].

Our intensity model analysis suggests that the presence of one competitor is enough to ensure that SC is the dominant selective pressure determining sperm numbers, even when SL is so severe as to result in fertility approaching zero. This is true as long as the relationship between number of sperm and the proportion of fertilized eggs is a concave (decelerating) function, a very likely biological scenario. However, relatively high values for risk (q) are needed to make SC the dominant pressure, unless fertility is also high. For example, if fertility is 0.9, then q must be over 0.39 for SC to dominate, and even when fertility is 0.99, q must be over 0.09. Many external fertilizers have synchronous group spawning and typically show very high relative testis size, which must be largely attributable to SC [19]. Some internal fertilizing species appear to experience very low SC risk, and here pressure to maintain ejaculate numbers may well relate mainly to SL, though it is worthy of note that they also typically have very low relative testis sizes [19].

In principle, it should be possible from figure 1 to estimate approximately which pressure is foremost in increasing sperm numbers in a given species for which the raffle principle is a fair approximation for the mechanism of SC, provided that its fertility and SC levels are known. However, fertility F(x) must be expressed not in absolute terms but as a proportion of the maximum fertility attainable (e.g. in cattle the maximum conception probability is around 0.75 [5]).

A more accurate, but likely analytically intractable approach would be to allow the number of competing ejaculates to follow a Poisson or geometric distribution, thus avoiding the need for separate intensity and risk approaches (in sperm allocation studies this requires solutions to be obtained numerically [3]). By allowing some ova to be fertilized without SC (in contrast to the intensity model where all eggs are competed over), this approach may allow SL to dominate for N − 1 > 1, though it is unlikely to greatly affect our conclusions in terms of biology.

The relative strength of gamete competition and gamete limitation has important implications for the evolution of anisogamy. The classical theory for the origin of anisogamy by gamete limitation [20,21] was phrased in terms of species selection, although it has recently been resurrected in terms of individual selection for cooperation [22,23]. By contrast, a ‘selfish’ origin via gamete competition has been shown to be entirely plausible [24]. A recent analysis [25] has unified both approaches and demonstrates that although gamete competition is a strong evolutionary force over much of the parameter range, gamete limitation alone can be sufficient if fertility is limited by high gamete mortality, low gamete fusion rates or low resources for making gametes. However, that model did not explicitly estimate the relative strengths of the two evolutionary pressures in scenarios where both are present.

We see a direct analogy between the present models and those for the evolution of anisogamy. In a simple anisogamy model, incorporating both gamete competition and gamete limitation, fitness of a + or − mating type can be calculated as w = gfh. Here 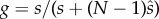in which s and are mutant and population gamete numbers of the focal mating type, f = fertility (number of gametes of opposite mating type that are fertilized) and h = zygote survival. This is analogous to the underlying structure of the model of Lehtonen & Kokko [25], although there this simplicity was hidden in a more complicated and general model framework.

Assuming the fitness function w = gfh, we get

in which the first two terms represent the pressure to increase gamete numbers via competition and limitation, just as in the present model, and the last term represents the pressure to increase gamete size for zygote survival. Gamete sizes diverge if a tiny initial difference in size between the mating types leads to a situation where the last term dominates for one mating type, while the first two terms dominate for the other. Focusing on the terms driving increased gamete numbers, the relative magnitudes of f · dg/ds and g · df/ds could be compared as we have done for SC, but this would now of course apply to both mating types. If f is concave throughout the evolution of anisogamy, our results derived for SC are directly applicable. The value of h will change as gametes evolve, but h multiplies both the limitation and competition terms, and therefore does not affect their relative strengths. Thus, our analysis has relevance to these models and implies that if anisogamy arose in a broadcast spawning ancestor, then, contrary to the claims of Iyer & Roughgarden [22] and Yang [23], gamete limitation could only dominate the evolutionary process if ancestral reproduction took place in highly isolated, small spawning groups. This seems to us a highly restrictive assumption in broadcast spawners, although we may never know the ancestral breeding biology to this level of detail.

Funding statement

J.L. was supported by the Kone Foundation.