Skip to main content
PMC home page
Scientific Reports logoLink to Scientific Reports
. 2014 Aug 22;4:6177. doi: 10.1038/srep06177

Large size three-dimensional video by electronic holography using multiple spatial light modulators

Hisayuki Sasaki 1,a, Kenji Yamamoto 1, Koki Wakunami 1, Yasuyuki Ichihashi 1, Ryutaro Oi 1, Takanori Senoh 1
PMCID: PMC4141271  PMID: 25146685

Abstract

In this paper, we propose a new method of using multiple spatial light modulators (SLMs) to increase the size of three-dimensional (3D) images that are displayed using electronic holography. The scalability of images produced by the previous method had an upper limit that was derived from the path length of the image-readout part. We were able to produce larger colour electronic holographic images with a newly devised space-saving image-readout optical system for multiple reflection-type SLMs. This optical system is designed so that the path length of the image-readout part is half that of the previous method. It consists of polarization beam splitters (PBSs), half-wave plates (HWPs), and polarizers. We used 16 (4 × 4) 4K×2K-pixel SLMs for displaying holograms. The experimental device we constructed was able to perform 20 fps video reproduction in colour of full-parallax holographic 3D images with a diagonal image size of 85 mm and a horizontal viewing-zone angle of 5.6 degrees.

The spatial image reproduction three-dimensional (3D) display methods can display natural 3D images. They can reconstruct spatial images in a similar manner to when objects are being directly observed. These methods can be achieved using integral photography1,2,3 or holography. Integral photography is a method that reproduces discrete light rays from objects. Electronic holography is considered to be the ideal and ultimate 3D video display method since it can reconstruct the wavefronts of the light reflected by objects and propagated through space. There exist advantages and disadvantages of each method. It is desirable for both the image size of a 3D image and the viewing-zone angle to be large. However, there exist many technical problems to overcome. One of the most important problems remaining to be solved for electronic holography is enlargement of the image size.

A wide range of research has been conducted concerning 3D displays using electronic holography for reproducing 3D videos. This includes research directed towards increasing the image size4,5,6 and research directed towards real-time operation using conventional central processing units (CPUs)7 or graphics processing units (GPUs)8,9,10 for hologram calculations.

Various methods for increasing the viewing zone by using space-division multiplexing have been proposed11,12,13. These methods are affected by the problem that part of the viewing-zone angle is missing. To overcome this problem, a method has been proposed that uses a beam splitter and multiple spatial light modulators (SLMs)14. However, the viewing-zone angle is scalable only in the horizontal direction, and each of the gaps between SLMs must equal the width of an SLM.

On the other hand, various methods of increasing the image size by using space-division multiplexing have also been proposed. Some problems with these proposals are that they only provide parallax in the horizontal direction15,16, the image size is only scalable in the horizontal direction17, or high-refresh-rate SLMs are required because the display frame rate is inversely proportional to the space-division multiplexing count18.

In a previous paper, we proposed a method of increasing the image size using space-division multiplexing by locating an optical system containing a lens array and other components in front of multiple SLMs6. Various methods of colourizing reconstructed images by using space-division multiplexing have been proposed19,20,21,22. Although these methods achieve ideal colourization of the reconstructed 3D images without colour breakup, they are too complex to adapt to the multiple-SLM system mentioned above because they will require triple the number of SLMs than in a monochromatic system for static colourization and they will also require a large or complex combination of optical systems. We proposed a colourization technique23,24 based on time-division multiplexing25,26,27,28,29,30,31,32 using three lasers (i.e., red, green, and blue) and a set of three revolving wheel shutters synchronized with 60 Hz driven SLMs.

However, a problem with the method mentioned above is the increased size of the image-readout optical system caused by increasing the number of SLMs to obtain a larger image. The enlargement of this assembly in the direction of the SLM's surface is acceptable, but the enlargement in the direction of its optical axis causes a problem. The proposed method can be scaled to increase the number of SLMs for further enlargement of the image, but there exist three factors limiting the image size. Firstly, the sizes of the lenses, secondly, the physical path length between SLMs and lenses to install the image-readout optical system, and thirdly, vignetting of the first-order diffraction beam by the outer edge of the polarization beam splitter (PBS) that was located at a distance from the SLMs. A solution for the second and third problems is to reduce the number of PBS steps to shorten the path length in the image-readout optical system.

In this paper, we propose a method of producing larger colour electronic holographic images with a newly devised space-saving image-readout optical system for multiple reflection-type SLMs. This optical assembly is designed so that the physical path length of the image-readout part is half that of the previous method and larger number of SLMs can be tiled. We apply this method with 16 (4 × 4) 4K×2K-pixel SLMs to display larger full-parallax colour holographic 3D video than with the previous system23,24.

Results

Limitation of the number of PBS steps in the image-readout optical system

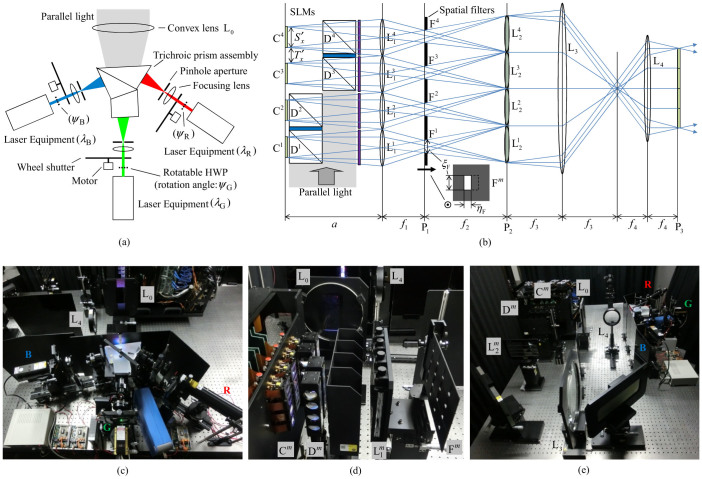
Figure 1 shows the configuration of the developed optical systems and their experimental setup and Table 1 shows a list of parameters. They are examples of the case when the horizontal number of SLMs Kx is four. Time-divided colour parallel light from a coherent light source as shown in Figs. 1(a) and 1(c) is incident perpendicularly on SLMs Cm through PBSs 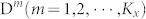Inline graphic in Figs. 1(b) and 1(d). Note that the superscript of the letter represents the location within the array. Figures 1(a) and 1(b) show plane figures of the optical systems in the horizontal direction. Consequently, this is so-called in-line holography. Amplitude modulation (AM) type reflective SLMs Cm are used for displaying hologram data. This kind of SLM creates modulation from the polarization rotation of incident light that corresponds to the input signals. Therefore, the combination of the SLM and each corresponding polarization-selective device (such as a PBS) results in the AM output of the light. The hologram data displayed on Cm are synchronized with the wheel shutters and changed to accommodate the alternating wavelengths of the light sources (i.e., red, green, or blue) every 1/60 second. Since the pixels are arranged in a grid pattern on a thin hologram, the light reflected on Cm contains a primary beam (first-order diffraction beam), conjugate beam (minus first-order diffraction beam), carrier beam (zero-order beam), and high-order diffraction beams of each of them. Since the object beam is generated as a primary beam, the other beams are unnecessary light and must be eliminated.

Figure 1. Configuration of optical systems and their experimental setup.

Figure 1

Open in a new tab

Light source optical system (a) and (c). Image-readout optical system and a part of gap reduction optical system (b) and (d). Overall view of optical systems (e). H.S. took the photographs.

Table 1. System parameters.

Parameter Design value
Wavelength of lasers (λR, λG, λB) (633, 532, 473) [nm]
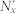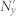Number of pixels for each SLM (horizontal Inline graphic, vertical Inline graphic) (3840, 2160) [pixels]
Pixel pitch for each SLM (horizontal px, vertical py) (4.8, 4.8) [μm]
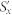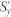Image size for each SLM S′ (horizontal Inline graphic, vertical Inline graphic) Diagonal 21.1 (18.4, 10.4) [mm]
Viewing-zone angle for each SLM (horizontal θx, vertical θy) (5.6, 2.8) [degrees]
Number of SLMs K (horizontal Kx, vertical Ky) 16 (4, 4)
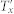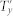Gap between adjacent SLMs T′ (horizontal Inline graphic, vertical Inline graphic) (33.2, 18.7) [mm]
Size of PBS (width and depth ξD, height ηD) (28, 28) [mm]
Relative refractive index of PBS material for air nD 1.52
Lens L0 (focal length f0, diameter ϕ0) (600, 200) [mm]
Focal lengths of lenses 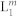Inline graphic (f1, f11, f12) (125, 170, 476) [mm]
Size of lenses 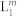Inline graphic (horizontal ξ1, vertical η1) (45, 29) [mm]
Size of apertures in spatial filters Fm (horizontal ξF, vertical ηF) (12.4, 6.2) [mm]
Lenses 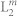Inline graphic (focal length f2, horizontal size ξ2, vertical size η2) (350, 51.6, 29.0) [mm]
Lens L3 (focal length f3, diameter ϕ3) (800, 340) [mm]
Lens L4 (focal length f4, diameter ϕ4) (285, 130) [mm]
Hologram data update rate 60 [fps]
Open in a new tab

In the previous system, an image-readout optical system as shown as Fig. 2(a) was used. PBSs are installed in a step-wise manner. We set the physical path length a and the geometrical path length a′, which was optically compensated by the refractive index of the PBS, between Cm and lenses 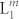Inline graphic as follows:

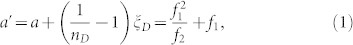graphic file with name srep06177-m1.jpg

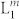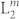where nD is the relative refractive index of the PBS material for air. The sizes (width and depth) of the PBSs are ξD. f1 and f2 are the focal lengths of lenses Inline graphic and Inline graphic, respectively. Equation (1) indicates that a′ equals the sum of a and the optical effect from the difference of the refractive index caused by a PBS in the optical path. The limitation of the number of PBS steps lDa that is limited by a is necessary to satisfy the following relation:

graphic file with name srep06177-m2.jpg

The thickness of the polarizers and lenses and the difference of nD according to wavelength play only small or negligible roles.

Figure 2. Image-readout optical systems.

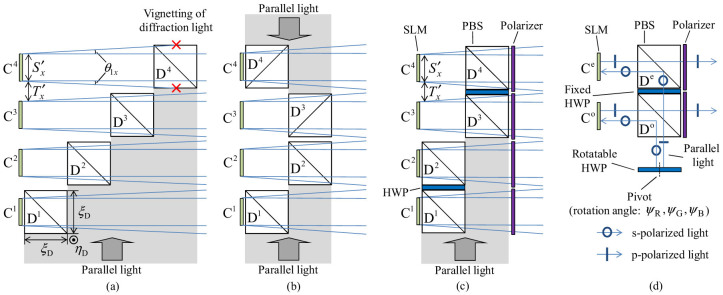
Figure 2

Open in a new tab

AM type reflective SLMs without polarization filters are used for displaying hologram data. Combination of the SLM and each corresponding PBS makes AM output of the light. The PBS in the outer position D4 of conventional image-readout optical system produces the vignetting angled part of the readout light from the corresponding SLM C4 (a). Illumination light enters from multiple directions (e.g., enters from opposite sides) to reduce the number of PBS steps (b). However, this system requires a complex incident optical system. Newly devised image-readout optical system, which consists of pairs of PBSs, SLMs, HWPs, and polarizers (c), and its operating principles (d). The PBS and SLM at odd-numbered positions are Do and Co, and at even-numbered positions are De and Ce. The number of PBS steps can be halved with illumination light entering the PBSs from a single direction.

On the other hand, when the number of SLMs increased, the PBSs in the outer positions produce the vignetting angled part of the readout light from the corresponding SLMs (i.e., light with an angle between the zero- and first-order diffraction beams). To avoid this vignetting by the outermost PBS, limitations of the number of PBS steps lDx and lDy, which depend on the vignetting limits of the horizontal and vertical directions, respectively, are necessary to satisfy the following relations:

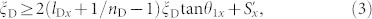graphic file with name srep06177-m3.jpg
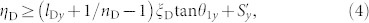graphic file with name srep06177-m4.jpg

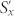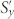where the height of the PBSs is ηD, and the sizes (width and height) of the SLMs are denoted by Inline graphic and Inline graphic. The angles of the first-order diffraction beam θ1x and θ1y are given as follows:

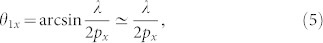graphic file with name srep06177-m5.jpg
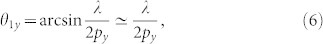graphic file with name srep06177-m6.jpg

where the horizontal and vertical pixel pitches are px and py, and the wavelength of the reproduced light is denoted by λ. Therefore, the overall limitation of the number of PBS steps lD, which depends on either the effect of the physical path length a (derived from f1 and f2: cf. formulas (1) and (2)) or vignetting of the first-order diffraction beam by the outermost PBS (cf. formulas (3) or (4)), is given as follows:

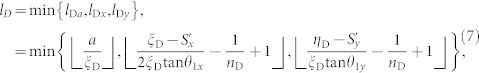graphic file with name srep06177-m7.jpg

where the notation Inline graphic indicates the floor function. lD is equal to the limitation of the horizontal number of PBSs by the optical system in Fig. 2(a).

When ηD equals ξD, the contribution to lD by the effect of horizontal direction lDx is more dominant than the effect of vertical direction lDy in equation (7) because 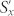Inline graphic is larger than 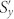Inline graphic and θ1x equals θ1y in most cases. Furthermore, lDa, which depends on a, has enough margin to install PBSs in a step-wise manner if we set the values of f1 and f2 appropriately. It is clear from formulas (1) and (2) that we can easy to enlarge lDa by elongation of f1. Therefore, it can be said that most effective parameter in equation (7) is lDx.

The relations between ξD and the limitation of the horizontal number of PBSs determined by lD are drawn as graphs in Fig. 3, where the above-mentioned parameters are selected as the values shown in Table 1. The dark gray shaded area shows feasible numbers of PBS steps. When PBSs with size ξD = ηD = 28 mm are used, the upper limit of the number of steps is three and it is clear that we cannot arrange four PBSs with four steps regardless of whether we would like to arrange the SLMs 4 × 4 in a plane.

Figure 3. The relations between the size of PBS ξD and the limitation of the horizontal number of PBSs (f1 = 125 mm, f2 = 350 mm, nD = 1.52, ηD = ξD, 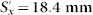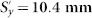Inline graphic, Inline graphic, px = py = 4.8 μm, and λ = λB = 473 nm).

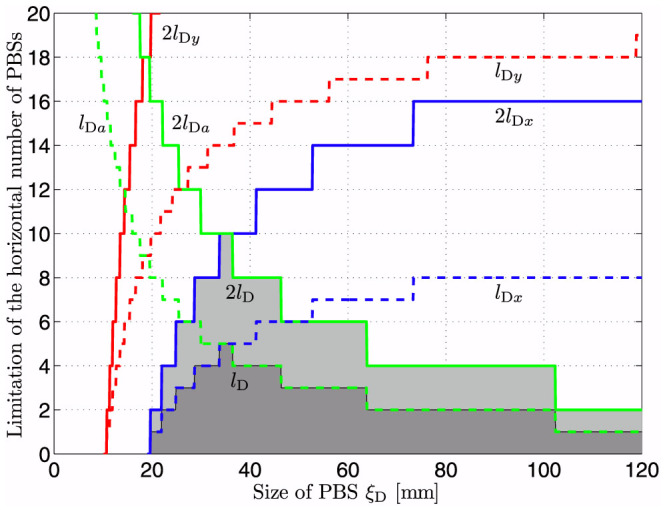
Figure 3

Open in a new tab

The dashed lines show the relations of ξD to lDa (green line), which is the limitation by the path length a, lDx (blue line), which is the limitation by the vignetting limits of the horizontal direction, lDy (red line), which is the limitation by the vignetting limits of the vertical direction, and lD (upper bound of gray shaded area), which is the overall limitation, by the conventional optical system shown in Fig. 2(a). The solid lines show the relations of ξD to 2lDa, 2lDx, 2lDy, and 2lD by the space-saving optical systems shown in Figs. 2(b)–2(d).

To overcome the limitation of image size scalability, it is necessary to increase the gap T′ between SLMs and use a large PBS, or to divide the beam from the light source and make it incident from multiple directions (e.g., enter from opposite sides) to reduce the number of PBS steps as shown in Fig. 2(b). However, the first method mentioned in the previous sentence necessitates huge optical components in the following optical system. For example, if we use PBSs with size ξD = 35 mm so that the upper limit of the number of steps is as high as five, the physical sizes of lenses 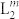Inline graphic and L3 (i.e., ξ2, η2, and ϕ3) would have to be 1.25 times larger than the values shown in Table 1. Furthermore, the second method requires many optical components to create a complex incident light path. These methods are feasible but difficult or complicated.

Therefore, we devised an optical system in which illumination light is enterable from only one direction and the number of PBS steps can be halved. Figures 2(c) and 2(d) show its composition. The limitation of the horizontal number of PBSs by the optical systems in Figs. 2(b)–2(d), which is also drawn as graphs in Fig. 3, is expressed as 2lD because there exist two PBSs in the horizontal direction for every one step of PBSs in the depth direction. The light and dark gray shaded areas in Fig. 3 show feasible regions of the horizontal number of PBSs in this instance.

Experimental setup

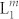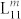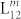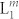We constructed a device with the parameters shown in Table 1. We used a combination lens as Inline graphic to reduce optical distortions. The focal lengths of the individual lenses Inline graphic and Inline graphic in Inline graphic are denoted by f11 and f12, respectively. This device, which uses an optical system and 16 SLMs, corresponds to an SLM with approximately 133 megapixels. We used AM type 4K×2K-pixel reflective liquid crystal on silicon (LCOS) SLMs33,34 that are manufactured by JVC KENWOOD Corporation. Figures 1(c)–1(e) show the experimental setup. We performed the following experiments using hologram data created by computer-generated holography (CGH) technology. 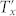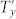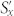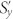Note that in this device, the horizontal and vertical gaps between adjacent SLMs Inline graphic and Inline graphic are 1.8 times the image size of each SLM Inline graphic and Inline graphic due to physical constraints of the SLMs. In other words, for a display area of 1 for the image part, the area of the missing segments is approximately 4.5.

Experiment testing image-readout optical system

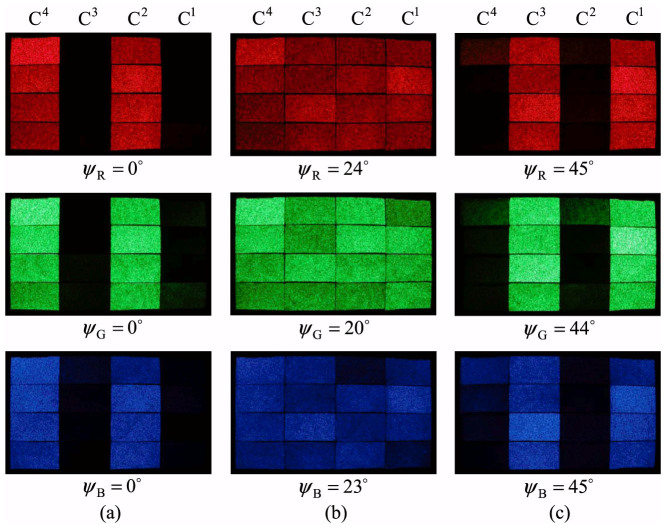
We operated the rotatable half-wave plates (HWPs), which are installed on light paths from every colour laser (cf. Figs. 1(a) and 2(d)), to control the ratio of the s- and p-polarized light that is incident on the PBSs. We defined the rotation angles of the HWPs ψR, ψG, and ψB to be 0 degrees when the illuminated SLMs of Ce are brightest and the illuminated SLMs of Co are darkest. When the HWPs are rotated until Ce and Co have equivalent brightness, ψR, ψG, and ψB become 24, 20, and 23 degrees, respectively. Furthermore, when they are rotated until the Ce are darkest and Co are brightest, ψR, ψG, and ψB become 45, 44, and 45 degrees, respectively. Figure 4 shows the experimental results in each colour channel. From these results, we confirmed that the devised optical system is able to illuminate the SLMs from a single direction, the number of PBS steps can be halved, and the intended function of the image-readout is achieved.

Figure 4. Control of incident light distributions using rotatable HWPs in each colour channel.

Figure 4

Open in a new tab

The illuminated SLMs of Ce (i.e., C2 and C4) are brightest and the illuminated SLMs of Co (i.e., C1 and C3) are darkest and the rotation angles ψR, ψG, and ψB of the HWPs are defined as 0 degrees (a); the HWPs are rotated until Ce and Co have equivalent brightness (b); and they are rotated until the Ce are darkest and Co are brightest (c).

Experiment displaying 3D images

We confirmed that objects with different depths could be reconstructed in colour by using time-division multiplexing. Figures 5 and 6 show colour 3D images that are displayed by this system. Figures 5(a) and 6(a) show the design values of the displayed objects. The displayed hologram data was calculated by using the ray-sampling (RS) plane approach35. In this approach, the RS plane is virtually set near the object and the dense ray information is sampled and converted into the wavefront on that plane as the Ray-Wavefront conversion process36. The light field on the hologram plane is then obtained by a two-dimensional (2D) light propagation calculation from the given RS plane to the hologram plane. The dense ray information was rendered as a set of projection images by general computer-graphics (CG) software.

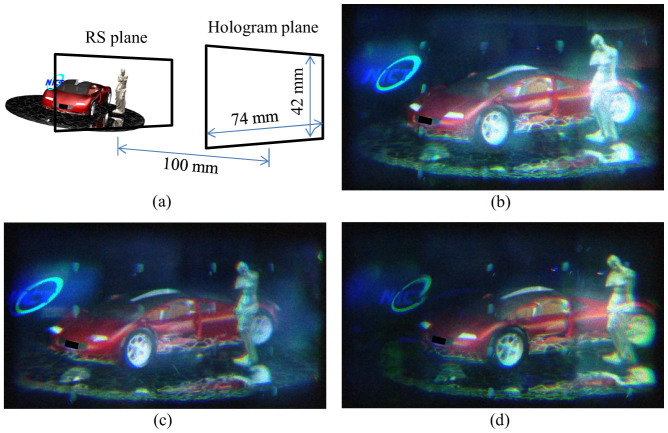
Figure 5. Experimental results.

Figure 5

Open in a new tab

Design values of the displayed objects in the experiment (a). A logotype of NICT is located at 113 mm, the left front wheel of a car is at 118 mm, the left rear wheel is at 143 mm, and a Venus statue is at 120 mm behind the hologram plane, respectively. Photographs that were taken from locations directly in front (b), taken by pointing 2.8 degrees right (c), and 2.8 degrees left (d). K.W. prepared hologram data. H.S. took the photographs.

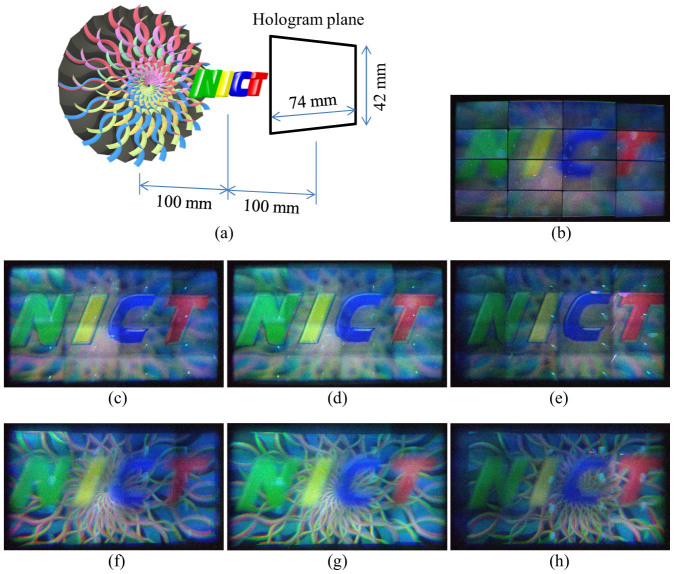
Figure 6. Experimental results.

Figure 6

Open in a new tab

Design values of the displayed objects (a). Photographs that were taken of the reconstructed objects focused on the hologram plane (b), focused on the logotype of NICT (c)–(e), and focused on the background object (f)–(h). Photographs (b), (d), and (g) were taken from locations directly in front; (c) and (f) were taken by pointing 2.8 degrees right; and (e) and (h) were taken by pointing 2.8 degrees left. K.W. prepared hologram data. H.S. took the photographs.

The photographs in Figs. 5(b), 5(c), and 5(d) were taken from a location directly in front, a location determined by pointing 2.8 degrees right, and a location obtained by pointing 2.8 degrees left, respectively. A logotype of NICT is located at 113 mm, the left front wheel of a car is at 118 mm, the left rear wheel is at 143 mm, and a Venus statue is at 120 mm behind the hologram plane, respectively.

The photograph in Fig. 6(b) was taken of the reconstructed objects focused on the hologram plane, the photographs in Figs. 6(c)–6(e) were focused on the logotype of NICT, and the photographs in Figs. 6(f)–6(h) were focused on the background object. Figs. 6(b), 6(d), and 6(g) were taken from locations directly in front; Figs. 6(c) and 6(f) were taken by pointing 2.8 degrees right; and Figs. 6(e) and 6(h) were taken by pointing 2.8 degrees left. The logotype of NICT is located at 100 mm, and the background object is 200 mm behind the hologram plane. When the objects can be reconstructed at different depths, the background object should be blurry in Figs. 6(c)–6(e), and the logotype of NICT should be blurry in Figs. 6(f)–6(h). From the experimental results and the description above, it is apparent that objects with different depths can be reconstructed.

The photographs in Figs. 5 and 6 also show the views of the same displayed objects from other angles. It is apparent that the reconstructed objects can be observed in the designed viewing zone and missing segments that can be seen without the optical system are eliminated.

We also confirmed that a video could be reproduced. Figure 7 shows the experimental results. We used CGH technology to create hologram data for the earth and letters. The letters circle around the earth. The logotype of NICT is located at 104 mm, and the centre of the earth is 127 mm behind the hologram plane. This experiment confirmed that this video sequence could be converted to a frame rate with one set of red, green, and blue per frame in time-division colour and played smoothly at a 20 fps (frames per second).

Figure 7. Experimental results.

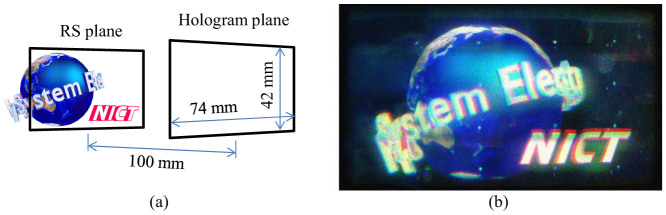
Figure 7

Open in a new tab

Design values of the displayed objects (a) and reconstructed 3D image at video rate (b). The centre of the earth is 27 mm behind the RS plane. K.W. prepared hologram data. H.S. took the photograph.

The displayed size of these 3D images is 74 mm × 42 mm (i.e., the diagonal is 85 mm) and the viewing-zone angles are 5.6 degrees horizontally and 2.8 degrees vertically. From the experiments described above, it is apparent that a colour-moving image of 3D objects having an image size of 16 times that of an SLM can be played with a frame rate of 20 fps using the proposed method.

Figures 5,6,7 show that gaps between the SLMs are eliminated. However, the boundaries of the SLMs are observed. In addition, differences of brightness are observed between each small display area.

Discussion

In this paper, we propose a method of producing larger colour electronic holographic images with a newly devised space-saving image-readout optical system for multiple reflection-type SLMs. We applied the proposed method to 16 SLMs to realize full-parallax video holography at a frame rate of 20 fps with an image size of 16 times that of one SLM and a viewing-zone angle of 5.6 degrees. Although the time-division colourization method had a frame rate of 20 fps because we used 60-fps SLMs, we could also reach 60 fps in colour, for example, if 180-fps SLMs were used.

When observing a 3D image with the focus on a distant location from the hologram plane in the depth direction, the boundaries of the SLMs become diffuse and sparse because these boundaries exist on the hologram plane. Actually, the observed boundaries are sparser in Figs. 6(f)–6(h), which are focused on the background object located far from the hologram plane, than the boundaries in Figs. 6(c)–6(e), which are focused on the logotype of NICT located near the hologram plane.

Differences of brightness exist depending on the difference of contrast between each display area in Figs. 4,5,6,7. Since there is no difference of contrast among SLMs, we think that differences among the optical specifications of the various PBSs that comprise an incident optical system become the dominant influence. Since each PBS provides a different contrast, this produces a detrimental effect for viewing the 3D image. We believe that we can balance the differences of display brightness among the various display areas if we compensate for luminance differences among the hologram data that is displayed on the various SLMs.

Methods

Space-saving image-readout optical system

Figures 2(c) and 2(d) show the space-saving image-readout optical system and its operating principles. Rotatable HWPs are inserted in the light paths from every colour laser because light in which s- and p-polarizations are mixed with the intended ratio is to be incident on the PBSs. We use pairs of PBSs. We denote a PBS and SLM at odd-numbered positions here by Do and Co and at even-numbered positions by De and Ce in a pair of PBSs and their corresponding SLMs, respectively. Do and De are arranged in tandem for the incident light path, and a HWP is fixed between these PBSs. The s-polarized component of the incident light is reflected by the mirror (e.g., dielectric multilayer mirror, wire grid, etc.) in Do and illuminates Co. The p-polarized component passes through Do and is converted to s-polarized light by the HWP. This light is incident on and reflected by De so that it then illuminates Ce.

Readout light from each SLM consists of mixed polarization light because the s-polarized incident light changed to the p-polarized component of the readout light, and this conversion ratio depends on the displayed value of each pixel. Only the p-polarized component of the readout light passes through the PBS and polarizer and is then output from this image-readout optical system. The s-polarized component from the SLMs is reflected by the PBSs and goes back towards the light source. The light that goes back to Do from De is also converted to p-polarized light by the HWP and goes straight through Do ideally. However, there exists a possibility of crosstalk interference by a small quantity of a residual s-polarized component. The polarizer next to Do cuts off this s-polarized component. Essentially, a polarizer does not have to be set next to De, but we use it for luminance equalization of the Do and De optical outputs. Since the modulation type of the SLMs is AM as mentioned previously, polarizers after the PBSs will not affect the modulated wavefront of holograms.

The loss of light that passes through De is greater than the loss of light that only passes through Do because it takes a longer path and also has to pass through the HWP. There exists a possibility of incomplete conversion in the HWP due to wavelength differences, which also leads to a loss of light in De. To compensate for these effects, rotatable HWPs are installed in the light paths from every colour laser as mentioned above. These HWPs can be rotated to control the ratio of the s- and p-components of the incident light and to compensate for luminance differences in the Do and De optical outputs of every colour. Essentially, rotatable HWPs do not have to be set if the corresponding laser equipment is rotatable around each optical axis and fixable at intended attitudes, but we use them to facilitate adjustments of polarizations. The optical systems in Fig. 1 utilize this method.

Image size and viewing-zone angle

If we denote the number of SLM pixels here by Nx and Ny and the pixel pitch in the horizontal and vertical directions by px and py, respectively, then the image sizes Sx and Sy and the horizontal and vertical viewing-zone angles θx and θy are given as follows:

graphic file with name srep06177-m8.jpg
graphic file with name srep06177-m9.jpg
graphic file with name srep06177-m10.jpg
graphic file with name srep06177-m11.jpg

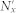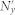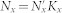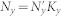where the horizontal and vertical angles of the first-order diffraction beam θ1x and θ1y are given by equations (5) and (6). With the space-division multiplexing method, if we denote the number of pixels in the horizontal and vertical directions of each SLM by Inline graphic and Inline graphic and denote the number of SLMs by Kx and Ky, then Inline graphic and Inline graphic. The only difference between the horizontal and vertical directions is the viewing-zone angles θx and θy.

Light source optical system and colourization

Figure 1(a) shows the light source optical system that implements colourization based on time-division multiplexing23,24. This is also known as the frame sequential method. This system consists of three lasers (i.e., red, green, and blue), rotatable HWPs, wheel shutters, spatial filters, and a trichroic prism assembly. The shutters are rotating wheels driven by motors that are synchronized with the SLMs and controlled so that a beam of one of the three colours is selected to pass through the shutter every 1/60 second. After that, the laser beams pass through a spatial filter (i.e., convex lens and pinhole) so that the shape of the beams gradually becomes wider. Three beams are combined into one by a Phillips type trichroic prism assembly. Finally, the combined white beam is collimated by a large size convex lens L0 to illuminate all of the SLMs.

Gap reduction optical system

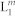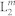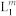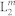Figure 1(b) shows the image-readout and gap reduction optical systems. Emitted light from the image-readout optical system is incident on lenses Inline graphic, spatial filters Fm, and lenses Inline graphic6. Spatial filter Fm is located on the common focal plane P1 of lenses Inline graphic and Inline graphic, and the focal length f1 of 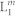Inline graphic and focal length f2 of 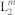Inline graphic are related as follows:

graphic file with name srep06177-m12.jpg

depending on the image size S′ of each SLM and the gap T′ between SLMs. Also, we set the physical path length a and the geometrical path length a′, which was optically compensated by the refractive index of the PBS, between Cm and lenses 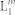Inline graphic as shown in equation (1). This enlarges the image due to Cm by (S′ + T′)/S′ and shifts it to 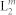Inline graphic (P2).

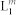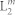The missing image segments due to the gaps between the SLMs will disappear. The group of lenses Inline graphic and Inline graphic forms the lens arrays, respectively. Fm is a spatial filter array for eliminating the conjugate beam, carrier beam, and high-order beams, which are unnecessary light37. Finally, the light is incident on lens L3 and lens L4. By setting the focal length f3 of L3 and focal length f4 of L4 as follows:

graphic file with name srep06177-m13.jpg

the image that had been enlarged at P2 will be returned to its original size at plane P3.

This optical system can exhibit parallax in both the horizontal and vertical directions. However, as shown in equations (10) and (11), the horizontal viewing-zone angle θx is two times the viewingzone angle of the first-order diffraction beam θ1x, but the vertical viewing-zone angle θy equals θ1y. The reason for this is that spatial filters Fm eliminate the first-order diffraction beam in the lower side of the vertical direction to eliminate the conjugate image, i.e., single-sideband holography38 with half-zone-plate processing is performed for the hologram data that is displayed on the SLM39. We used spatial filters Fm as shown in Fig. 1(b). A mask (gray square with white rectangle aperture) shows a plane figure of the configuration of the spatial filter in the vertical direction. The aperture sizes in horizontal and vertical directions of spatial filters ξF and ηF are given as follows:

graphic file with name srep06177-m14.jpg
graphic file with name srep06177-m15.jpg

The conjugate beam and carrier beam are eliminated by the dashed lined rectangle part in the masked area, and the other masked area eliminates the high-order beams. Only the object beam passes through the aperture selectively.

Author Contributions

H.S. designed the optical systems, did experimental work, wrote the main manuscript text, and prepared figures and videos. K.Y. designed the optical systems, did project planning and management. K.W. prepared hologram data and did experimental work. Y.I., R.O. and T.S. did experimental work. All authors reviewed the manuscript.

Supplementary Material

Supplementary Information

Video 1

Download video file (3.8MB, mov)
Supplementary Information

Video 2

Download video file (1.8MB, mov)
Supplementary Information

Video 3

Download video file (4MB, mov)
Supplementary Information

Video 4

Download video file (3.8MB, mov)

Acknowledgments

The authors sincerely thank Ms. Mao Makino at NICT, Kyoto, Japan. She gave us insightful comments and suggestions and furnished 3D CG models that were used in experiment shown in Fig. 6.

Footnotes

A patent application with priority date 23 April 2013 has been filed for the space-saving image-readout optical system.

References

  1. Koike T., Oikawa M., Utsugi K., Kobayashi M. & Yamasaki M. Autostereoscopic display with 60 ray directions using LCD with optimized color filter layout. Proc. SPIE 6490, Stereoscopic Displays and Virtual Reality Systems XIV, 64900T, San Jose, CA, USA. 10.1117/12.704633. (2007, March 5). [Google Scholar]
  2. Sakai H., Yamasaki M., Koike T., Oikawa M. & Kobayashi M. 41.2: Autostereoscopic display based on enhanced integral photography using overlaid multiple projectors. SID Symposium Digest of Technical Papers 40, 611–614. 10.1889/1.3256853. (2009, July 5). [Google Scholar]
  3. Arai J. et al. Integral three-dimensional television using a 33-megapixel imaging system. J. Disp. Technol. 6, 422–430 (2010). [Google Scholar]
  4. Peyghambarian N., Tay S., Blanche P.-A., Norwood R. & Yamamoto M. Rewritable holographic 3D displays. Opt. Photon. News 19, 22–27 (2008). [Google Scholar]
  5. Blanche P.-A. et al. Holographic three-dimensional telepresence using large-area photorefractive polymer. Nature 468, 80–83 (2010). [DOI] [PubMed] [Google Scholar]
  6. Yamamoto K., Ichihashi Y., Senoh T., Oi R. & Kurita T. 3D objects enlargement technique using an optical system and multiple SLMs for electronic holography. Opt. Express 20, 21137–21144 (2012). [DOI] [PubMed] [Google Scholar]
  7. Yamamoto K., Mishina T., Oi R., Senoh T. & Kurita T. Real-time color holography system for live scene using 4K2K video system. Proc. SPIE 7619, Practical Holography XXIV: Materials and Applications, 761906, San Francisco, CA, USA. 10.1117/12.840589. (2010, February 10). [Google Scholar]
  8. Bove, Jr V. M., Plesniak W. J., Quentmeyer T. & Barabas J. Realtime holographic video images with commodity PC hardware. Proc. SPIE 5664, Stereoscopic Displays and Virtual Reality Systems XII, 255–262, San Jose, CA, USA. 10.1117/12.585888. (2005, June 14). [Google Scholar]
  9. Shiraki A. et al. Simplified electroholographic color reconstruction system using graphics processing unit and liquid crystal display projector. Opt. Express 17, 16038–16045 (2009). [DOI] [PubMed] [Google Scholar]
  10. Ichihashi Y., Oi R., Senoh T., Yamamoto K. & Kurita T. Real-time capture and reconstruction system with multiple GPUs for a 3D live scene by a generation from 4K IP images to 8K holograms. Opt. Express 20, 21645–21655 (2012). [DOI] [PubMed] [Google Scholar]
  11. Mishina T., Okui M., Doi K. & Okano F. Holographic display with enlarged viewing-zone using high-resolution LC panel. Proc. SPIE 5005, Practical Holography XVII and Holographic Materials IX, 137–144, Santa Clara, CA, USA. 10.1117/12.473818. (2003, May 30). [Google Scholar]
  12. Hahn J., Kim H., Lim Y., Park G. & Lee B. Wide viewing angle dynamic holographic stereogram with a curved array of spatial light modulators. Opt. Express 16, 12372–12386 (2008). [DOI] [PubMed] [Google Scholar]
  13. Chen R.-Y. & Wilkinson T. Field of view expansion for 3-D holographic display using a single spatial light modulator with scanning reconstruction light. 3DTV Conference: The True Vision - Capture, Transmission and Display of 3D Video (3DTV-CON), 2009, 1–4, Potsdam, Germany. 10.1109/3DTV.2009.5069673. (2009, May 4–6). [Google Scholar]
  14. Yaraş F., Kang H. & Onural L. Circular holographic video display system. Opt. Express 19, 9147–9156 (2011). [DOI] [PubMed] [Google Scholar]
  15. Fukaya N. et al. Expansion of the image size and viewing zone in holographic display using liquid crystal devices. Proc. SPIE 2406, Practical Holography IX, 283–289. San Jose, CA, USA. 10.1117/12.206229. (1995, April 12). [Google Scholar]
  16. Takaki Y. & Nakamura J. Development of a holographic display module using a 4k2k-SLM based on the resolution redistribution technique. OSA Technical Digest: Biomedical Optics and 3D Imaging, DM2C.5, Miami, FL, USA. 10.1364/DH.2012.DM2C.5. (2012, April 28 – May 2). [Google Scholar]
  17. Finke G., Kujawińska M., Zaperty W. & Kozacki T. Spatiotemporal multiplexing method for big images observation in wide angle holographic display. 3DTV-Conference: The True Vision - Capture, Transmission and Dispaly of 3D Video (3DTV-CON), 2013, 1–4, Aberdeen, UK. 10.1109/3DTV.2013.6676642. (2013, October 7–8). [Google Scholar]
  18. Slinger C., Cameron C. & Stanley M. Computer-generated holography as a generic display technology. Computer 38, 46–53 (2005). [Google Scholar]
  19. St-Hilaire P., Benton S. A., Lucente M. E. & Hubel P. M. Color images with the MIT holographic video display. Proc. SPIE 1667, Practical Holography VI, 73–84, San Jose, CA, USA. 10.1117/12.59642. (1992, May 1). [Google Scholar]
  20. Yaraş F., Kang H. & Onural L. Real-time color holographic video display system. 3DTV Conference: The True Vision - Capture, Transmission and Display of 3D Video (3DTV-CON), 2009, 1–4, Potsdam, Germany. 10.1109/3DTV.2009.5069660. (2009, May 4–6). [Google Scholar]
  21. Yaraş F., Kang H. & Onural L. Real-time phase-only color holographic video display system using LED illumination. Appl. Opt. 48, H48–H53 (2009). [DOI] [PubMed] [Google Scholar]
  22. Senoh T. et al. Full-color wide viewing-zone-angle electronic holography system. OSA Techinal Digest: Digital Holography and Three-Dimensional Imaging, DTuA3, Tokyo, Japan. 10.1364/DH.2011.DTuA3. (2011, May 9–11). [Google Scholar]
  23. Sasaki H., Yamamoto K., Ichihashi Y. & Senoh T. Colorization technique for 3D objects enlargement type electronic holography using an optical system and multiple SLMs. OSA Techinal Digest: Digital Holography and Three-Dimensional Imaging, DTh2A.4, Kohala Coast, HI, USA. 10.1364/DH.2013.DTh2A.4. (2013, April 21–25). [Google Scholar]
  24. Sasaki H., Yamamoto K., Ichihashi Y. & Senoh T. Image size scalable full-parallax coloured three-dimensional video by electronic holography. Sci. Rep. 4, 4000 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
  25. Senoh T., Mishina T., Yamamoto K., Oi R. & Kurita T. Wide viewing-zone-angle full-color electronic holography system using very high resolution liquid crystal display panels. Proc. SPIE 7957, Practical Holography XXV: Materials and Applications, 795709, San Francisco, CA, USA. 10.1117/12.876763. (2011, February 07). [Google Scholar]
  26. Shimobaba T. & Ito T. A color holographic reconstruction system by time division multiplexing with reference lights of laser. Optical Review 10, 339–341 (2003). [Google Scholar]
  27. Shimobaba T., Shiraki A., Masuda N. & Ito T. An electroholographic colour reconstruction by time division switching of reference lights. J. Opt. A: Pure Appl. Opt. 9, 757 (2007). [Google Scholar]
  28. Shimobaba T., Shiraki A., Ichihashi Y., Masuda N. & Ito T. Interactive color electroholography using the FPGA technology and time division switching method. IEICE Electronics Express 5, 271–277 (2008). [Google Scholar]
  29. Oikawa M. et al. Time-division color electroholography using one-chip RGB LED and synchronizing controller. Opt. Express 19, 12008–12013 (2011). [DOI] [PubMed] [Google Scholar]
  30. Martínez J. L., Martínez-García A. & Moreno I. Wavelength-compensated color Fourier diffractive optical elements using a ferroelectric liquid crystal on silicon display and a color-filter wheel. Appl. Opt. 48, 911–918 (2009). [DOI] [PubMed] [Google Scholar]
  31. Häussler R. et al. Large real-time holographic displays: from prototypes to a consumer product. Proc. SPIE 7237, Stereoscopic Displays and Applications XX, 72370S, San Jose, CA, USA. 10.1117/12.805873. (2009, February 17). [Google Scholar]
  32. Makowski M. Minimized speckle noise in lens-less holographic projection by pixel separation. Opt. Express 21, 29205–29216 (2013). [DOI] [PubMed] [Google Scholar]
  33. Bleha W. P. & Sterling R. D. D-ILA technology for high-resolution projection displays. Proc. SPIE 5080, Cockpit Displays X, 239–249, Orlando, FL, USA. 10.1117/12.497532. (2003, September 12). [Google Scholar]
  34. Suehiro K. et al. Integral 3D TV using ultrahigh-definition D-ILA device. Proc. SPIE 6803, Stereoscopic Displays and Applications XIX, 680318, San Jose, CA, USA. 10.1117/12.766892. (2008, February 29). [Google Scholar]
  35. Wakunami K. & Yamaguchi M. Calculation for computer generated hologram using ray-sampling plane. Opt. Express 19, 9086–9101 (2011). [DOI] [PubMed] [Google Scholar]
  36. Wakunami K., Yamashita H. & Yamaguchi M. Occlusion culling for computer generated hologram based on ray-wavefront conversion. Opt. Express 21, 21811–21822 (2013). [DOI] [PubMed] [Google Scholar]
  37. Yamamoto K., Oi R., Mishina T. & Okui M. Half-zone-plate processing for objects on both sides of hologram display. Proc. SPIE 6912, Practical Holography XXII: Materials and Applications, 69120Q, San Jose, CA, USA. 10.1117/12.762903. (2008, January 25). [Google Scholar]
  38. Bryngdahl O. & Lohmann A. Single-Sideband Holography. J. Opt. Soc. Am. 58, 620–624 (1968). [Google Scholar]
  39. Mishina T., Okano F. & Yuyama I. Time-alternating method based on single-sideband holography with half-zone-plate processing for the enlargement of viewing zones. Appl. Opt. 38, 3703–3713 (1999). [DOI] [PubMed] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information

Video 1

Download video file (3.8MB, mov)
Supplementary Information

Video 2

Download video file (1.8MB, mov)
Supplementary Information

Video 3

Download video file (4MB, mov)
Supplementary Information

Video 4

Download video file (3.8MB, mov)

Articles from Scientific Reports are provided here courtesy of Nature Publishing Group

RESOURCES