Abstract
In a previous study [W. Whitmer, B. Seeber and M. Akeroyd, J. Acoust. Soc. Am. 132, 369-379 (2012)], it was demonstrated that older hearing-impaired (HI) listeners produced visual sketches of headphone-presented noises that were insensitive to changes in interaural coherence. The current study further explores this insensitivity by comparing (a) binaural temporal fine-stucture (TFS) resolution and (b) sound localization precision to (c) auditory source width judgments. Thirty-five participants aged 26-81 years with normal to moderately impaired hearing (a) discriminated interaurally phase-shifted tones from diotic tones presented over headphones, (b) located 500-ms speech-spectrum filtered click trains presented over loudspeakers between ±30° in quiet, and (c) sketched the perceived width of low-pass, high-pass and speech-spectrum noise stimuli presented over loudspeakers from 0° and simultaneously from ±45° at attenuations of 0-20 dB to generate partially coherent stimuli. The results showed a decreasing sensitivity to width with age and impairment which was related to binaural TFS threshold: the worse one’s threshold – which was correlated with age – the less the perceived width increased with decreasing interaural coherence. These results suggest that senescent changes to the auditory system do not necessarily lead to perceptions of broader, more diffuse sound images based on interaural coherence.
I. INTRODUCTION
A sound in an enclosed space produces a percept of a spatial impression. This spatial impression has numerous attributes, including apparent direction (angle), distance and size – the latter of which, in the horizontal plane, is its apparent auditory source width (ASW). Numerous studies have shown deficits in perceiving sound-source direction for older hearing-impaired individuals (e.g., Noble & Byrne, 1990; Lorenzi et al., 1999b; Dobreva et al., 2011). It would be expected that whatever aspects of aging and impairment lead to these deficits may also lead to changes in the other spatial attributes, such as width.
We have previously examined (a) the ability to discriminate changes in ASW and (b) the visual cross-mapping – sketching – of ASW for broadband noises presented over headphones (Whitmer et al., 2012). For normal-hearing listeners, ASW is known to depend on the similarity or coherence in the sounds received at the two ears and on stimulus level (de villiers Keet, 1968; Ueda et al., 1997). When discriminating changes in ASW based on interaural coherence (IC), we found that older HI listeners had increased thresholds relative to younger NH listeners. This result could be modeled by simply adding interaurally independent noises to the stimuli used by the NH listeners, representing an increased temporal jitter (cf. Pichora-Fuller & Schneider, 1991). When sketching ASW based on IC, the responses of older mild-to-moderate HI listeners did not vary with IC. This insensitivity was not significantly correlated with age or listeners’ pure-tone thresholds. The NH and HI groups in our previous study, though, were dichotomous with regards to both age and hearing loss, and there was no accounting for sensation level in the stimuli. There may also have been difficulties for listeners in describing acoustic phenomena experienced in a room when listening over headphones. To investigate these issues and to better examine how older HI individuals perceive the width of sounds, the current experiments (1) used stimuli presented over loudspeakers, (2) measured the localization and basic binaural resolution abilities of older HI individuals, and (3) compared these abilities to their sketches of ASW.
Perrott and Buell (1982) noted that despite the literature on “tonal volume” dating to the early twentieth century (e.g., Rich, 1916), there had been no evidence of a relation between volume – or width – and localization precision. The term precision refers to the (standard) deviation from the listener’s (mean) judgment of source location, as opposed to accuracy, which refers here to the absolute difference between the mean localization responses and actual source locations.1 Precision is then the second-moment statistic of the direction of a sound source. Unlike accuracy, which directly measures the perception of direction, precision could be related to other spatial aspects of the sound source, such as ASW, as we hypothesized in our previous headphone study (Whitmer et al., 2012). Greene and Paige (2012) recently showed no difference in localization precision for NH listeners when the physical width of a loudspeaker was changed, but did not examine the percept of width. Other studies have shown age- and impairment-related deficits in supra-threshold precision, with precision increasing twofold on average from young normal-hearing to older hearing-impaired individuals (e.g., Noble & Byrne, 1990; Dobreva et al., 2011). It is not clear whether these deficits have an effect on the apparent width and/or changes in width of sound sources.
While there has been no previous evidence of a relationship between localization precision and source-width perception, there has been evidence of a relationship between difficulties in binaural temporal fine structure (TFS) discrimination and decreased speech recognition performance when maskers are spatially separated from the target. Based on a quick lateralization method developed for audiology (Nilsson & Liden, 1976), Hopkins and Moore (2010) developed a task to measure TFS resolution for low-frequency tones using a sequence of 500-Hz tones with alternating interaural phase disparities (described in Section II B). Using this task, Moore et al. (2012) found that the ability to resolve binaural TFS was significantly correlated with age within an older group, aged 60-85 years (r = 0.77; p < 0.01). Neher et al. (2012) used the same task to show a significant correlation between binaural TFS resolution and speech recognition with spatially segregated noises (r = −0.63; p < 0.01). As the ability to resolve interaural phase disparities has links with both aging and the ability to use spatial cues, we used the Hopkins and Moore task here to investigate links between binaural TFS and listeners’ sensitivity to auditory source width.
To measure the percept of ASW with loudpeakers, we used a presentation method similar to Ueda and colleagues (Ueda & Morimoto, 1995; Ueda et al., 1997). In a study of the weighting of frequency bands on ASW judgments in NH listeners, they used three loudspeakers – one on-axis, two symmetrically off-axis – and presented independent third-octave narrowband noises with the off-axis noises attenuated to produce varying interaural coherences. Their listeners indicated ASW by adjusting the extent of two LEDs symmetrically placed in the same arc as the three loudspeakers at 2.5° increments (in the current study, ASW was indicated by sketching the extent with a touch screen). Their results showed little variability in within-subject responses and clear changes in width as a function of IC. Despite findings that three loudspeakers can be heard as separate sources (Santala & Pulkki, 2011), the same three loudspeaker arrangement was used in the current study, partly due to Ueda’s success with it, and also because it is similar to the asymmetric three-generator method (Hartmann & Cho, 2011) used by Blauert and Lindemann (1986) in their headphone study of ASW.
II. METHODS
All participants were tested on (1) the ability to resolve interaural differences in temporal fine structure (i.e., interaural phase discrimination), (2) the ability to localize broadband stimuli and (3) the sketching of the perceived width of low-frequency, high-frequency and speech-spectrum noises. The stimuli varied across the tasks to better relate performance to previous literature for each domain: (1) 500-Hz tones (cf. Hopkins & Moore, 2010), (2) speech-spectrum filtered 100-Hz click trains (cf. Lorenzi et al., 1999b), and (3) low-pass, high-pass and speech-spectrum filtered noise (cf. Blauert & Lindemann, 1986). To reduce the movement between testing booths for participants with limited mobility, all participants were tested on the tasks in this order after initial audiometric data was collected. To ensure audibility, the levels of stimuli across tests were adjusted to approximate at least 20 dB SL for all participants.
A. Participants
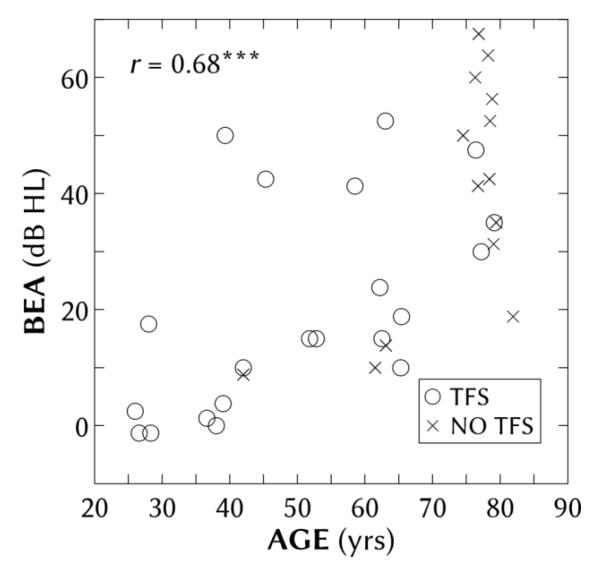
Thirty-five adults (15 female, 20 male), aged 26-81 years (median of 63 years), were recruited from the pool of NH and HI patients available to the MRC Institute of Hearing Research, sourced from attendees at clinics of the local hospitals by a postal survey and employees of the Institute. Pure-tone thresholds were assessed using the modified Hughson–Westlake method (British Society of Audiology, 1981) with a calibrated audiometer (GSI 61). All hearing losses were predominantly sensorineural, with air-bone conduction differences less than 10 dB hearing level (HL). Better-ear four-frequency averages (BEAs), computed from pure-tone thresholds at 500, 1000, 2000 and 4000 Hz, ranged from −1 to 67 dB HL (mean = 28 dB HL; σ = 21 dB HL). Figure 1 shows the individual BEAs as a function of age, which were significantly correlated (r = 0.68; p < 0.001). Their interaural asymmetries ranged from 0 to 21 dB HL, with a median of 3 dB HL. The separation of participants into TFS and no-TFS groups is explained in the next section.
Figure 1.
Pure-tone threshold better-ear four-frequency average (BEA) as a function of age for all participants. The correlation between age and BEA (r = 0.68) was significant (p < 0.001). Symbols refer to participants with (circles) and without (crosses) binaural TFS data.
B. Binaural TFS task
The binaural TFS task was based on the Hopkins and Moore procedure (2010) excepting only that the adaptive procedure was changed from a two-up/one-down to three-up/one-down rule. In the task, participants discriminated a sequence of four 500-Hz tones where the interaural phase difference (IPD) of the second and fourth tone was adaptively adjusted (i.e., an “ABAB” target) from a four-tone sequence where the IPD of all tones was zero (i.e., an “AAAA” probe). The AAAA probe stimulus was composed of a sequence of four diotic 500-Hz sinusoids of 400-ms duration with 50-ms onset and offset (raised-cosine) gates with a gap of 20 ms between each sinusoid. The ABAB target stimulus was composed of a similar sequence, except that an IPD was applied to the second and fourth sinusoids. The total duration of each stimulus was 1660 ms (four 400-ms tones with three 20-ms gaps). The stimuli were presented at a default A-weighted level of 55 dB SPL via a soundcard (RME DIGI-96/8 PAD), audio amplifier (Arcam A80), and circumaural headphones (Sennheiser HD-580). The level was increased in 5 dB increments to ensure presentation at a minimum of 20 dB SL based on the participant’s audiometric thresholds at 500 Hz. The interstimulus interval was 200 ms.
Thresholds were measured using a two-interval forced-choice adaptive procedure. On each trial, participants were presented with two intervals, the probe stimulus and the target stimulus. Participants were asked to determine in which stimulus the tones appeared to move or jump in position (i.e., determine the ABAB stimulus). The IPD of the second and fourth tone of the ABAB stimulus were adjusted using a three-up/one-down rule, which asymptotes to the 79%-correct point of the psychometric function (Levitt, 1971). Participants were seated in a sound-dampened booth (1.5 × 1.3 × 2 m) and instructed on the task. They were then presented an example of the diotic probe stimulus and the target stimulus containing tones with an IPD of 180° (π phase). Next they were given practice with the task using target stimuli with a fixed IPD of 180°, before commencing with the testing. Responses were given via a touch-screen monitor. For experimental trials, the initial IPD was 90° and was adjusted (multiplied/divided) by a factor of 1.253 for the first reversal, 1.252 for the second reversal, and 1.25 for the last six reversals. Thresholds were computed from the average of the last six reversals. The average adaptive track length was 43 trials.
C. Localization task
To examine the precision (and accuracy) in individual localization judgments, we calculated individual standard deviations of an absolute localization task. This task was a reduced form of the task used by Lorenzi et al. (1999b) in which participants located click trains presented in the frontal hemifield in quiet.
Participants were seated in a sound-dampened room (2.5 × 4.4 × 2.5 m) in the middle of a circular 24-loudspeaker array with a radius of 0.9 m and inter-loudspeaker spacing of 15°. The height of the fixed chair was adjusted so that the woofer cones were at 0° elevation relative to the participant’s ear canals. To avoid visually anchoring responses at the loudspeakers in the localization task, the loudspeakers from −45 to +45° were covered with an acoustically transparent black cloth with a single white dot centered just below the cone of the 0° loudspeaker. The stimuli were presented from an outboard signal processor (MOTU 24) through a digital-to-analog convertor (Fostex VC-8), attenuator (Behringer Ultralink) and powered two-way (tweeter vertically above woofer) loudspeakers (Phonic 207). The loudspeakers were all calibrated with pink noise to be within ±1 dB at 500 and 1000 Hz octave bands. A touch-screen monitor was placed as close to the participant as possible just below the loudspeakers to minimize head movements for making responses.
The localization task stimulus was a 100-Hz click train of 500-ms duration that was filtered to mimic long-term average speech spectrum (LTASS) based on Byrne et al. (1994). The stimulus spectrum, measured in third-octave bands, had its peak at 500 Hz and gradually tapered to −21 dB re peak at 16 kHz. Like the binaural TFS task, stimuli were presented at a default A-weighted level of 55 dB SPL. The level was increased in 5 dB increments to ensure presentation at a minimum of 20 dB SL based on the participant’s audiometric thresholds at 500 Hz. The stimuli were presented from −30 to +30° in 5° increments, with locations between the 15°-spaced loudspeakers generated with a sine/cosine amplitude panning of the nearest speaker pair to ensure equal level across all possible locations (Blumlein, 1931).
On each trial, the participant was presented with a stimulus and asked to touch the screen in the response area where they heard the sound. The response area was a 694 × 266 pixel (24.5 × 9.4 cm display size) black rectangle with a white dot in the center. The monitor was repositioned if necessary so that the white dot on the screen was directly below the white dot on the black curtain (over the 0° loudspeaker). Participants were instructed that the white dot on the screen represented the white dot on the curtain, and the extent of the rectangle represented the extent of the black curtain (covering the ±45° loudspeakers); responses were therefore limited to ±45°. The touch screen registered a response with a red crosshair that was displayed for 750 ms, after which the next trial commenced. Participants were given practice trials at ±30 and 0°. During testing, the 13 locations were presented 10 times (a total of 130 trials) in randomized order. Localization precision was calculated as the standard deviation of mean localization responses (i.e., listener’s judgment) averaged across locations. Localization accuracy was also calculated, being the absolute difference between mean localization responses and actual locations. While localization accuracy and precision can decrease away from the midline (e.g., Dobreva et al., 2011), averaging across locations excluding midline (0°) did not increase precision results in the current task by more than 7%.
D. Sketching task
In the sketching task, participants drew a visual representation of the width of the image they perceived on a touch screen (i.e., a visual cross-mapping task). Stimuli of varying ICs were created by attenuating two flanking noises relative to a center “source” noise, a free-field method equivalent to the asymmetric three-generator method (Hartmann & Cho, 2011) used by Blauert and Lindemann (1986) via headphones and Ueda and Morimoto (1995) via loudspeakers.
The apparatus was the same as for the localization task except that there was no curtain cover over the loudspeaker array, giving visual anchors to abet sketching. The stimuli were three types of filtered noise: low-frequency noise (termed LF; 250-1000 Hz), high-frequency noise (termed HF; 2000-8000 Hz) and long-term average speech spectrum noise (LTASS; Byrne et al., 1994). Noises were generated in the Fourier domain using real and imaginary values from a Gaussian distribution at each specified spectral frequency with a sampling rate of 48 kHz. The noises were 1000 ms in duration with 50-ms raised-cosine onset/offset gating. To control IC, independent noises were simultaneously presented from loudspeakers at 0 and ±45°. The 0° noise was presented at an A-weighted level of 55 dB SPL; the level was increased in 5 dB increments to ensure at least 20 dB SL based on each participant’s better-ear 500-Hz threshold for the LF stimuli, 4000-Hz threshold for the HF stimuli and four-frequency (.5, 1, 2 & 4 kHz) threshold average for the LTASS stimuli. The ±45° noises were presented at levels relative to the center noise of −20 to 0 dB in 4 dB increments; the different relative levels produce different ICs (see Figure 2). The overall level of the three noises was equalized across attenuation values; this equalization was confirmed using a sound level meter at the center of the loudspeaker array. To reduce any effect of changing IC on perceived level (Edmonds & Culling, 2009), the overall level on each trial was then roved by a randomly chosen value from a uniform distribution between −2 and +2 dB in 0.1 dB increments.
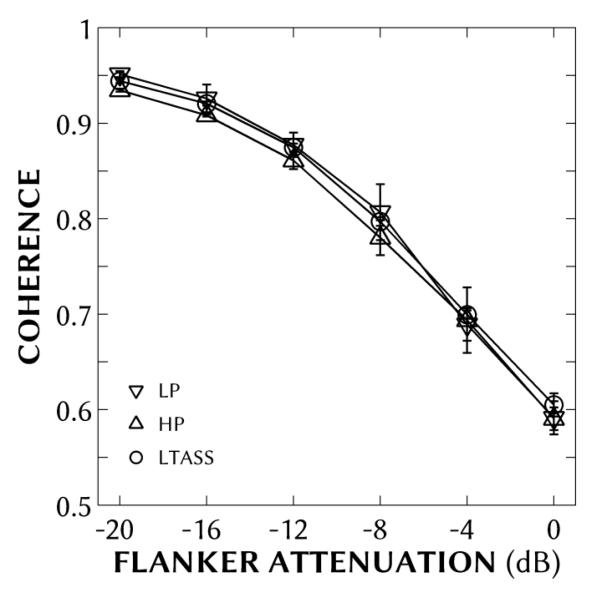
Figure 2.
Interaural coherence as a function of ±45° flanker attenuation as recorded using a mannequin at the listener’s position equipped with artificial pinnae for each stimulus type: low-pass noise (LP; downward triangles), high-pass noise (HP; upward triangles) and long-term average speech-spectrum noise (LTASS; circles). Error bars show ±1 standard deviation of measurement.
The IC produced by these stimuli was measured using a mannequin at the listener’s position equipped with artificial pinnae and ½” microphones (Brüel & Kjær HATS 4100D). Each stimulus type (LF, HF & LTASS) was played and recorded five times at 55 dB (A). The IC was calculated as the average height of the peak in the normalized cross-correlation function from −1 to +1 ms for the central 750 ms of the recording (i.e., ignoring the first and last 125 ms of the recording). The results of this measurement are shown in Figure 2. Using flanker attenuations from 0 to 20 dB re center level produced ICs from 0.95-0.59, respectively, and which were essentially equivalent across stimulus types. As stimulus level was adjusted to accommodate for hearing loss (i.e., to approximate at least 20 dB SL), we confirmed that changing level had only a minimal effect on measured IC: boosting overall level from 60 to 80 dB(A) caused a maximum decrease in IC of 0.02.
In the experiment, participants were asked to sketch the width of the sounds. After the presentation of the stimulus, participants were shown a 754 × 266 pixel (26.6 × 9.4 cm display size) photographic representation of the loudspeakers from ±45° with additional space to either side. The distance between the center of the ±45° loudspeaker cones in the image was 600 pixels. The touch screen was centered in front of them so that the image roughly corresponded to their field of vision. Participants were instructed to draw the extent of the sound from the left to the right using their index finger which, on pressing the screen within the image, displayed a red 8 × 8 pixel square centered at the contact point. Participants were also instructed that sounds could appear to extend to areas between or beyond the loudspeakers. Visual inspection of responses during trials showed no bias towards the visual anchors in sketched widths. Participants were first given practice using all three stimulus types and extreme flanker-attenuation values (−20 and 0 dB). It was verbally confirmed that participants perceived these stimuli as one source, not three. After some practice trials, participants sketched the perceived width for ten presentations of each combination of flanker attenuation and stimulus type for a total of 180 trials. The stimuli were presented in randomized order. If participants did not respond, the same trial was repeated. The width was calculated as the difference (in pixels) between the x-axis minimum and x-axis maximum for each sketch. To account for possible outliers, the analysis excluded the largest and smallest widths sketched for each stimulus type and flanker attenuation, resulting in eight responses per condition per participant.
III. RESULTS
A. Binaural TFS task
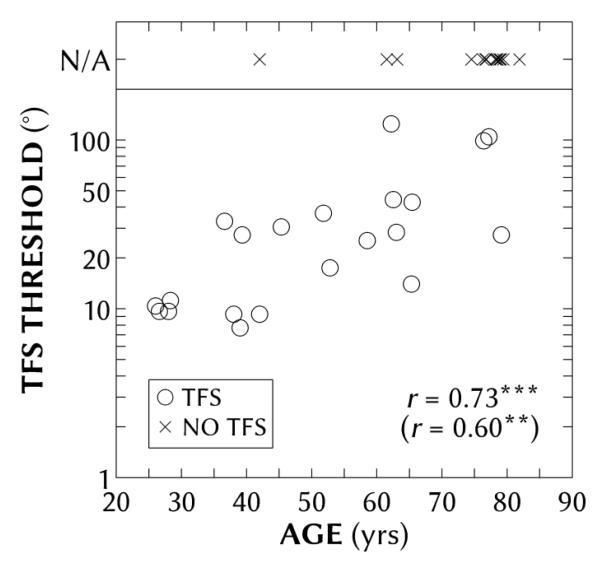
Figure 3 shows the binaural TFS thresholds as a function of age for all participants.2 Fourteen of the participants could not perform the task, regardless of practice; the spread of their ages are shown at the top of the figure, and their inability to perform the task is considered in further detail in the Discussion. The TFS thresholds for the remaining 21 participants ranged from 7.7 to 124.5° (corresponding to ITD thresholds for a 500-Hz tone of 43-692 μs). The median age and BEA of the 21 TFS participants were 51 years and 15 dB HL, respectively. The median age and BEA of the 14 no-TFS participants were 77 years and 42 dB HL, respectively; 11 of these no-TFS participants were aged 74-81 years. For those able to perform the TFS task, there was a significant Pearson product-moment correlation between age and TFS threshold (r = 0.73, p < 0.001), even when controlling for the effects of BEA hearing loss (r = 0.60, p < 0.01) by partialling out the correlations between BEA and age and between BEA and TFS threshold.
Figure 3.
Individual binaural temporal-fine-structure (TFS) thresholds as a function of age for all participants. Symbols refer those with (circles) and those without (crosses at top of panel) binaural TFS data. Binaural TFS threshold and age were significantly correlated (r = 0.73; p(df = 20) < 0.001), even when accounting for better-ear average (r = 0.60; p(df = 20) < 0.01).
B. Localization task
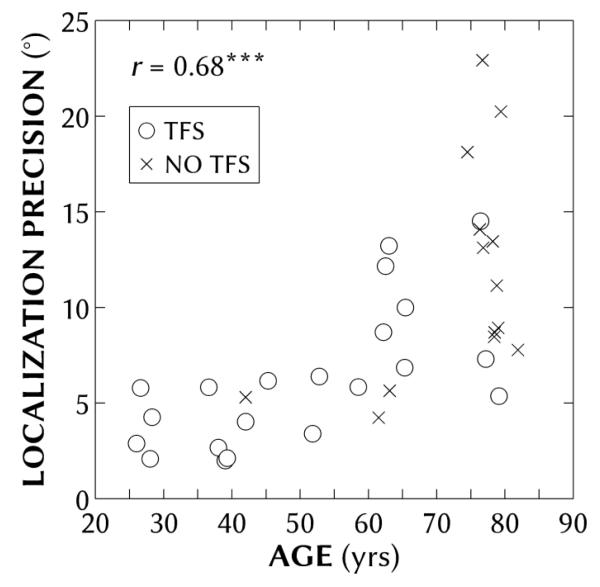
Figure 4 shows the individual localization precision as a function of age for all 35 participants. Precision was significantly correlated with age (r = 0.68; p < 0.001), even when controlling for BEA hearing loss (r = 0.46; p < 0.01). Precision was not significantly correlated with hearing loss when controlling for age (r = 0.21; p > 0.05), nor TFS threshold when controlling for age (r = 0.38; p > 0.05).
Figure 4.
Individual mean localization precision (average standard deviations across locations) as a function of age for all participants. Symbols refer to participants with (circles) and without (crosses) binaural TFS data. Localization precision and age were significantly correlated (r = 0.68; p(df = 34) < 0.001), even when accounting for better-ear average (r = 0.46; p(df = 34) < 0.01).
Localization results were also examined in terms of the age classifications used in previous studies. Using two groups that were either less or greater than 50 years old, comparable to the age division in Lorenzi et al. (1999), the average precision for participants was significantly different between the two groups: 3.9 and 10.1°, respectively [t(30.9) = 5.34; p < 0.001]. Using three groups of younger (26-42), middle (45-65) and older (74-81) aged participants, comparable to the age divisions in Dobreva et al. (2011), also yielded significantly different average precisions: 3.7°, 6.8° and 12.4°, respectively [t(12.7) = 2.70; p < 0.05 for younger vs. middle, and t(22.9) = 3.59; p < 0.01 for middle vs. older].
Individual localization accuracy (i.e., the absolute mean difference between response and actual locations) was significantly correlated with precision (r = 0.50; p < 0.01). Accuracy was also significantly correlated with age (r = 0.39; p < 0.05), though it was significantly less correlated with age than precision was correlated with age [Williams T2(35) = 2.20; p < 0.05]. Accuracy was not correlated with BEA (r = 0.22; p > 0.05) nor TFS threshold (r = 0.17; p > 0.05).
C. Sketching task
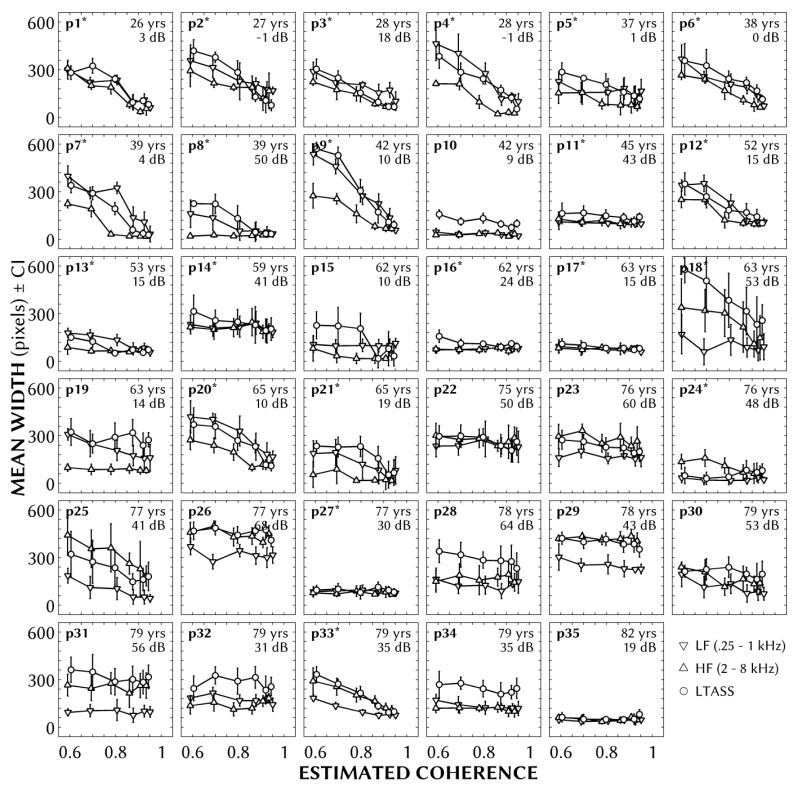
Figure 5 shows the average widths (in pixels) of the sketching responses as a function of IC (estimated from mannequin measurements) and stimulus type, with a separate panel for each participant, sorted by age. The error bars are 95% confidence intervals computed from the standard error within each stimulus condition. Their age and BEA are reported in the upper right of each panel. Asterisks indicate the 21 participants who were able to complete the binaural TFS task. In general, the influence of stimulus and IC on perceived width differed markedly between participants. If participants were sensitive to changes in ASW based on IC, we would expect sketched widths to decrease when increasing IC from 0.6 to 0.95 (e.g., the top left panel, participant 1). In contrast, if participants were insensitive to these changes, we would expect mean widths to remain flat, whether all stimuli were judged narrow (e.g., participant 27) or broad (e.g., participant 29). In lieu of heterogeneous group means, the data was analyzed in three ways: (1) comparisons of the range of widths across sub-groupings of participants, (2) post-hoc comparisons of width differences between most and least coherent stimuli across participants and participant groups, and (3) linear regressions of widths as a function of IC, and correlating the resulting coefficients with age, TFS threshold and localization precision.
Figure 5.
Normalized mean widths as a function of the measured coherence of stimuli for the three stimulus types: low-frequency noise (250-1000 Hz; downward triangles), high-frequency noise (2000-8000 Hz; upward triangles) and long-term average speech-spectrum noise (LTASS; circles). Error bars show ±1 standard error. Each panel represents a different participant, sorted by age from youngest to oldest (p1-p35). An asterisk next to the participant number indicates that participant was able to complete the binaural TFS task. Participant age and better-ear four-frequency average hearing loss is given in the upper right of each panel.
1. Comparing the range of widths across select participants
Examining individual differences, there were several pairs of participants with similar ages and hearing losses that produced different widths as a function of IC. For example, participants 9 and 10 were similar in age (42 years), BEAs (10 and 9 dB HL) and localization precision (4 and 5.3°). The sketching results of participant 9 (second row, third column in Figure 5), who was able to complete the binaural TFS task (threshold = 9.3°), had a relatively large range in mean widths for LF and LTASS stimuli (both 479 pixels), and to a lesser extent, HF stimuli (208 pixels). In contrast, the results of participant 10, who could not complete the TFS task, had a relatively small range in widths for LF, HF and LTASS stimuli (24, 21 and 83 pixels, respectively). Similarly, participants 33 and 34 were similar in age and BEA (both 79 years old, 35 dB HL), but participant 33, who was able to complete the TFS task (threshold = 27°), exhibited a greater range in widths than participant 34, who could not perform the TFS task. These paired differences based on TFS, however, do not completely generalize: participants 11, 14, 16, 17, 24 and 27 all showed sensitivity to binaural TFS, but did not show a range in mean widths across ICs greater than 120 pixels.
2. Differences in widths between age and TFS groups
The widths of the most and least coherent stimuli were next compared across age and the ability to perform the binaural TFS task. This analysis yielded one potentially homogenous group of participants 1-9, being a younger group with TFS data. All nine showed statistically significant decreases in width from the least to most coherent stimuli with the sole exception of HF stimuli for participant 8, which significantly increased by eight pixels.
To examine the effect of age groups comparable with those used in Whitmer et al. (2012), the results were split at 50 years into two groups: 26-45 years (n = 11; participants 1-11 in Figure 5) and 51-81 years (n = 24; participants 12-35). A Student’s t-test, with degrees of freedom adjusted for unequal variances, revealed stimulus-dependent differences. The widths of the most coherent stimuli (flanker attenuation of −20 dB) were sketched significantly wider by the older group for HF stimuli [152 vs. 65 pixels; t(33) = 3.31; p < 0.01] and LTASS stimuli [179 vs. 82 pixels; t(31) = 4.08; p < 0.001]. The widths of the least coherent stimuli were sketched significantly narrower by the older group only for LF stimuli [188 vs. 296 pixels; t(14) = −2.16; p < 0.05]. Participants were also grouped by whether or not they could perform the binaural TFS task. Those without TFS data sketched widths of the most coherent stimuli wider than those with TFS data for HF stimuli [188 vs. 83 pixels; t(15) = 2.84; p < 0.05] and LTASS stimuli [224 vs. 98 pixels; t(17) = 4.21; p < 0.001].
With the exceptions of participants 15 and 25, the participants unable to complete the binaural TFS task (those without asterisks next to their number in Figure 5) did not produce significant changes in width across stimuli, based on paired-sample t-tests of responses to the least and most coherent stimuli (t(df= 7) = −2.22-0.05; all p > 0.05). The widths, averaged across IC, did vary widely across these no-TFS participants: 33-319, 30-445 and 57-459 pixels for LF, HF and LTASS stimuli, respectively. These average widths for LF, HF and LTASS stimuli were not correlated with stimulus sensation level when controlling for age (r = 0.11, 0.06 and 0.43, respectively; all p(df = 13) > 0.05), but were correlated with the level of presentation when controlling for age (r = 0.58, 0.82 and 0.62; all p(df = 13) < 0.05).
Examining the effect of presentation level across all participants, the presentation levels were significantly different between the younger/older groups for LF [57 vs. 60 dB; t(31) = −2.07; p < 0.05], HF [59 vs. 73 dB; t(32) = −3.75; p < 0.001] and LTASS stimuli [58 vs. 67 dB; t(33) = −3.80; p < 0.001], and TFS/no-TFS groups for LF [56 vs. 64 dB; t(14) = −3.79; p < 0.01] and LTASS stimuli [60 vs. 69 dB; t(20) = −2.92; p < 0.01]. The stimulus-dependent significant ASW differences between age and TFS groups noted above could therefore be a by-product of these level effects. Different presentation levels, however, did not affect the acoustic measurement of IC: the IC of mannequin recordings of stimuli presented at 60-80 dB(A) only varied for the least coherent stimuli (flanker attenuation of 0 dB) by 0.02.
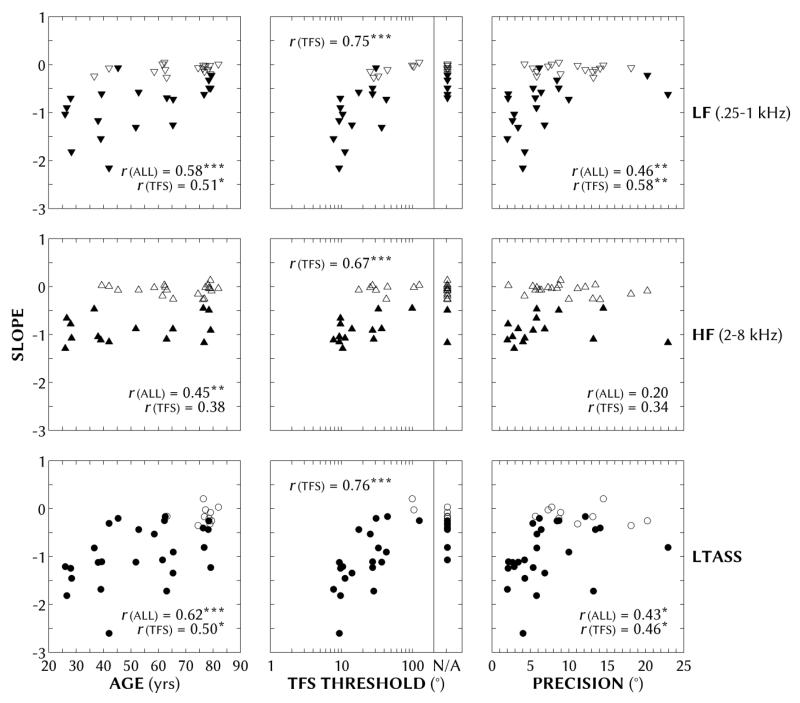
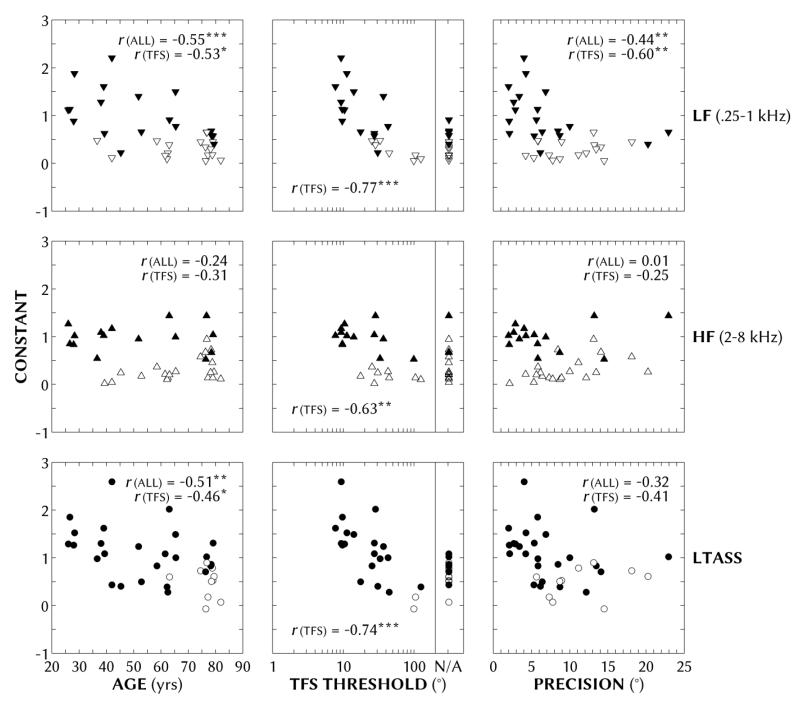
3. Linear regression analysis
To examine our main research question – how the differences in loudspeaker-based ASW sensitivity across individuals were related or not to age, binaural TFS thresholds and localization precision – linear regressions were fit to each individual’s mean normalized widths as a function of IC, using the equation ASW = a × IC + b. The widths were normalized by 600 to accord to the number of pixels between the ±45° loudspeaker cones in the display. The slope a of each regression estimates the degree to which ASW changed with IC. The farther the slope is from zero, the greater the ASW sensitivity to IC. The constant b of each regression estimates the hypothetical (normalized) width of a completely incoherent (IC = 0) source: the greater the constant, the wider the percept of an incoherent source. These slopes and constants are shown in Figures 6 and 7, respectively, for the each of the three stimulus types (rows) as a function of age (left column), binaural TFS threshold (middle column) and localization precision (right column). Regressions that were statistically significant models of the data are shown as filled symbols in Figures 6 and 7; insignificant regressions, due mostly to poorer fits at near-zero slopes, are shown as unfilled symbols.2 A negative slope indicates decreasing width with increasing IC. A summary of the full and partial Pearson product-moment coefficients for correlations of both slopes and constants with age, TFS threshold and precision is given in Table 1. Analyses were also performed as a function of hearing loss (BEA) and localization accuracy. The BEA analyses were very similar to the age analyses shown, and when accounting for age, there were no significant correlations between width results and BEA. Localization accuracy was not significantly correlated with the slopes and constants for any stimulus (r = −0.23 to 0.22; all p > 0.05).
Figure 6.
Linear regression slopes for individual mean normalized (1 normalized unit = 600 pixels) width as a function of interaural coherence. Slopes are shown for low-frequency bandpass (250-1000 Hz) noise (LF; top row), high-frequency bandpass (2-8 kHz) noise (HF; middle row) and long-term-average speech-spectrum noise (LTASS; bottom row) as a function of age (left column), binaural TFS threshold (center column) and localization precision (right column) for all participants. Solid symbols indicate statistically significant regressions (p < 0.05). Pearson product-moment correlation coefficients are given in a corner of each panel for all participants and only participants with TFS data. See Table 1 for correlations controlling for covariates. The number of asterisks (1, 2 or 3) indicates coefficients that were statistically significant at the p < 0.05, 0.01 and 0.001 levels, respectively.
Figure 7.
Same as Figure 6 except for linear regression constants.
Table 1.
Pearson product-moment correlation coefficients of slopes and constants (of the linear regression ASW = a × IC + b) with participants’ age, binaural TFS threshold and localization precision for each of the three stimulus types. Where appropriate, coefficients are shown for all participants (df = 34) and after the forward slash for those with TFS data (df = 20). Below each main coefficient(s) are the coefficients for partial correlations controlling for the specified covariates (e.g., below the slope-age coefficients for all and just TFS participants are the partial slope-age coefficients controlling for precision and TFS threshold). The number of asterisks (1, 2 or 3) indicates coefficients that were statistically significant at the p < 0.05, 0.01 and 0.001 levels, respectively.
| AGE (all / TFS) | TFS | PRECISION (all/TFS) | |||||
|---|---|---|---|---|---|---|---|
| PRECISION | TFS | AGE | PRECISION | AGE | TFS | ||
|
| |||||||
| SLOPE | LF | 0.58*** / 0.51* | 0.75*** | 0.46** / 0.58** | |||
| partial | 0.40* / 0.18 | 0.17 | 0.64** | .059** | 0.11 / 0.36 | 0.16 | |
|
| |||||||
| HF | 0.45** / 0.38 | 0.67*** | 0.20 / 0.34 | ||||
| partial | 0.47** / 0.20 | 0.27 | 0.63** | 0.64** | 0.26 / 0.08 | 0.25 | |
|
| |||||||
| LTASS | 0.62*** / 0.50* | 0.76*** | 0.43* / 0.46* | ||||
| partial | 0.49** / 0.27 | 0.16 | 0.67*** | 0.68*** | 0.01 / 0.18 | 0.10 | |
|
| |||||||
| CONSTANT | LF | −0.55* / −0.53* | −0.77*** | −0.44** / −0.60** | |||
| partial | −0.38* / −0.20 | 0.10 | −0.67*** | −0.63** | −0.09 / −0.37 | −0.17 | |
|
| |||||||
| HF | −0.24 / −0.31 | −0.63** | 0.01 / −0.25 | ||||
| partial | 0.06 / −0.19 | −0.38 | −0.65** | −0.69*** | −0.05 / 0.05 | −0.24 | |
|
| |||||||
| LTASS | −0.51** / −0.46* | −0.74*** | −0.32 / −0.41 | ||||
| partial | −0.42* / −0.26 | −0.20 | −0.66** | −0.68*** | −0.08 / −0.13 | −0.18 | |
With increasing age, binaural TFS threshold and with decreasing localization precision, the slopes (Figure 6) and constants (Figure 7) of the linear regression analysis generally approached zero, indicating decreasing sensitivity to ASW as a function of IC. Since age, binaural TFS threshold and localization precision correlated to one another, we will focus on partial correlations with each of these three factors controlling for the other two factors (see Table 1 for complete coefficient values and significance). The slopes and constants were significantly correlated with age when controlling for variance due to precision, but not when controlling for variance due to the log of binaural TFS thresholds for those participants with TFS data. The slopes and constants were not significantly correlated with localization precision when controlling for either age or the log of TFS thresholds (for those with TFS data). Conversely, the slopes were significantly correlated with the log of binaural TFS thresholds when controlling for variance due to either age or localization precision (center columns in Table 1). That is, the most robust correlation in the current study was between sensitivity to IC-induced ASW and binaural TFS threshold.
IV. DISCUSSION
A. General summary
The current study examined the perception of ASW in a sketching task with noises presented over loudspeakers, and compared results to age, localization precision and binaural TFS resolution. The regression analysis (see Figures 6, 7 and Table 1) indicated that sensitivity to the changes in width expected from varying the level of two flanking noises can be partially predicted from binaural TFS thresholds. We found that the degree to which ASW changed with IC was related to binaural TFS threshold across all stimuli: listeners with lower interaural phase difference thresholds perceived increasing ASW with decreasing stimulus IC (see Figures 6 and 7). The interpretation of this relationship, however, is limited by the large number of participants (14 of 35) showing binaural TFS thresholds beyond the ceiling level of the TFS task. For these participants, ASW did not vary as a function of stimulus IC with the exception of two participants (p15 and p25 in Figure 5).
While the data across all individuals was heterogeneous (comparing panels in Figure 5), there were clear, statistically significant changes in sketched ASW for the nine youngest participants with TFS data (1-9 in Figure 5) as a function of IC, comparable to previous findings (Plenge, 1972; Blauert and Lindemann, 1986; Ueda & Morimoto, 1995; Ueda et al., 1997; Whitmer et al., 2012). These widths were somewhat larger (for comparable ICs) than the two previous studies using a similar method (Ueda & Morimoto, 1995; Ueda et al., 1997). Those studies, though, used third-octave narrowband noises, which would be expected to produce narrower widths than the two-octave and broadband noises used here (cf. Blauert & Lindemann, 1986). In agreement with previous literature (e.g., Blauert and Lindemann, 1986; Mason et al., 2005), there was an effect of frequency on ASW, with low-frequency and broadband stimuli being perceived wider than high-frequency stimuli by younger participants. For some older participants (e.g., participant 31 in Figure 5), there was a partially inverted effect: HF and LTASS stimuli were perceived on average wider than LF stimuli. This might have been a byproduct of increased presentation level compensating for more severe HI.
B. Comparison to headphone results
In our previous study (Whitmer et al., 2012), older HI participants did not produce significantly different widths for changes in IC for broadband noises presented over headphones. To compare that insensitivity to the current results, the previous data was reanalyzed in a similar fashion to the current data: linear regressions of sketched data to IC, although only three ICs were tested (0.6, 0.8 and 1). The slopes and constants of those regressions were significantly correlated with age for all participants (r = 0.74 and −0.71, respectively; p(df = 24) < 0.001), though this may have been confounded by the four younger NH participants, as there was no significant correlation between the slopes and ages of the remaining older participants (47-77 years; median age 65 years; r = 0.19; p(df = 20) > 0.05). Hence, the effect of aging in the ASW insensitivity reported in Whitmer et al. (2012) may have been due to the discrete younger NH and older HI groups.
In the current study, the regression slopes for a similar age group of 51-81 years (n = 24) were also not significantly correlated with their ages for either LF, HF or LTASS stimuli (r = 0.30, 0.04 and 0.31, respectively; all p(df = 24) > 0.05). For the subgroup of older participants with sensitivity to TFS (n = 11), there were significant correlations for LF and LTASS stimuli between TFS thresholds and both slopes (r = 0.61 and 0.68; both p(df = 10) < 0.05) and constants (r = −0.64 and −0.66; both p(df = 10) < 0.05). While binaural TFS thresholds have been shown here and previously (Moore et al., 2012) to be linked with aging, the link between ASW sensitivity with age appears to only operate broadly (i.e., young vs. old in the most general sense) for these experimental conditions.
Whitmer et al. (2012) reported that the mean widths produced by the older HI participant group for broadband noises presented over headphones were significantly broader for diotic stimuli (IC = 1) than those by the younger NH group, but they were significantly narrower for partially coherent stimuli. In the current experiment, the mean widths produced by older participants for broadband noises presented over loudspeakers were significantly broader than those by younger participants for the most coherent (IC ~ 0.94) HF and LTASS noises only, and significantly narrower than younger participants for just the least coherent LF noises. These limited significant differences between older and younger participants – as groups – in the current study were possibly due to presentation level differences between these groups to compensate for more severe impairment. That is, these age-group differences could be a consequence of the effect of level increasing ASW without increasing IC (cf. de villiers Keet, 1968; Ueda et al., 1997). In the previous study, the older participants were matched for hearing loss, hence the presentation level was constant. The statistically significant mean width differences between the two groups in the previous headphone study may then be due to the clearer group differences in both age and HL in that study compared to the current study.
C. Width without coherence
For those with high TFS thresholds (greater than 90°) as well as those participants unable to perform the binaural TFS task, changes in IC did not elicit significant changes in ASW. While these participants were not responding to the intended variable – the IC produced by varying the level of two flanking noises – the perceived widths varied across listeners greatly. For many of these participants, the presentation level was increased from 55 dB(A) to approximate a minimum of 20 dB SL. This adjustment of level for audibility had the unintended consequence of correlating with the mean widths sketched by these participants: participants presented with higher levels tended to perceive broader widths. This level dependence has been previously shown within NH listeners (de villiers Keet, 1968; Ueda & Morimoto, 1995), and likely can be explained across these participants by loudness recruitment (Buus and Florentine, 2002). That is, while a 60-dB sound may be inaudible for a listener with a moderate hearing loss, an 80-dB sound may be perceived as nearly as loud – and as wide – as it would be for an NH participant. While BEA did not correlate with our sketching results when accounting for age, there may still be a role of loudness in the perception of ASW that cannot be explained with the current data.
D. Psychophysical parallels
The binaural TFS thresholds for those older HI able to perform the task were generally similar to previous data reported using the Hopkins and Moore (2010) method. Moore et al. (2012) found binaural TFS thresholds to range from 9-180° within an older population aged 60-85 years, and that thresholds were significantly correlated with age (r = 0.74; p < 0.001). In the current study, the threshold range of participants able to do the task aged 60+ years (61-81 years; n = 8) was comparable (14-124°), but there was no correlation of TFS threshold with age (r = 0.17; p >> 0.05).3 The inability of many of the older participants to detect any phase difference could be further evidence of age-related deficits in binaural processing (Pichora-Fuller & Schneider, 1991). This processing deficit is also apparent in the poorer localization performance of these older no-TFS participants (crosses in Figure 4), but not for the three “younger” no-TFS participants. Floor effects and exaggerated thresholds have been previously found in lateralization tasks with naïve normal-hearing participants (e.g., McFadden et al., 1973; Saberi & Antonio, 2003). It is possible that the no-TFS participants here found a particular aspect of the binaural TFS test unfathomable. While this difficulty with the task may limit its use, it did provide a surrogate for sensitivity to ASW induced by changes in IC in the current scenario. That is, those who could not perform the TFS task often did not sketch substantial changes in width across ICs.
Localization precision – as measured by the standard deviation from the mean response in an absolute localization task – was comparable to previous findings, despite the use of a touch screen for responses and the use of panning between adjacent two-way loudspeakers to create target positions. For example, mean localization RMS error in Lorenzi et al. (1999a; 1999b) increased from 8° for young NH (age 23-33 years) to 20° for older HI (age 54-72 years) participants, which is the same relationship, albeit a different measure, as the current results for similar age groups (3.9 and 10.1° for participants aged 26-45 and 51-81, respectively). In a second example, standard deviations for localization in Dobreva et al. (2011) increased from 2.4° for young (19-41) to 4.1° for middle-aged (45-66) to 5.5° for elderly (70-81 years) participants; in the current study, deviations increased from 3.7 to 6.8 to 12.4° for similar age divisions (26-42, 45-65 and 74-81 years, respectively). Corroborating Dobreva et al., supra-threshold localization precision was more correlated with aging than accuracy.
We hypothesized in our earlier study (Whitmer et al., 2012) that decreases in localization precision with aging could be perceptually manifested in broader, more diffuse images. That is, with increased scattering of perceived locations for the same sound-source location, there may be a broader percept of the sound source. Comparing localization precision to ASW here has yielded only partial evidence for this hypothesis. Precision and the mean sketched width, averaged across stimulus types, was significantly correlated (r = 0.43; p < 0.01) only for the most coherent stimuli, and was not significantly correlated when controlling for the effect of presentation level (r = 0.03; p > 0.05). That is, individuals with lower precision perceived widths that were broader but this was partially due to increased signal levels to account for their hearing loss. Furthermore, with lower precision, ASW was mostly fixed as a function of IC based on the near-zero slopes shown in Figure 6. When statistically controlling for binaural TFS resolution, the correlation between localization precision and change in ASW (the regression slope) was insignificant. This lack of correlation between precision and ASW may be due to the differences in the stimuli used across tasks. It is possible that using the same stimuli across tasks, instead of using the same stimuli as the literature upon which each task was based, could have yielded a significant correlation between the two tasks. It is also possible that absolute localization precision may not be an appropriate measure. Given older HI difficulties with source separation (Noble et al., 1997), measuring relative localization acuity – which can be unrelated to absolute localization precision (Moore et al., 2008) – could be a better psychophysical parallel to ASW sensitivity.
Whitmer et al. (2012) accounted for decreased ASW discriminability for older HI participants in an auditory model by applying attenuated independent noises to the left and right channels. This additive-noise model could not explain, however, decreased ASW sensitivity in that study or the current one. Two recent models do provide some indication of how ASW sensitivity could decrease with binaural TFS acuity. Goupell and Hartmann (2007) showed that sensitivity to narrowband coherence changes for NH listeners was best predicted by the standard deviations across time in interaural time and level differences (ITDs and ILDs, respectively). Yost and Brown (2013) showed that multiple source locations – such as the current three-loudspeaker scenario – could be predicted from the ITDs and ILDs in different spectrotemporal windows (cf. Faller & Merimaa, 2004). Analyzing the mannequin recordings of the current stimuli (see Section II D) with 20-ms time windows showed a common pattern of results with either model: ILDs and their standard deviations did not vary markedly across ICs, whereas ITDs and their deviations did. If the poor-to-no-TFS participants had difficulty perceiving these variations in ITD – which is possible given their poor interaural phase acuity – they could potentially have only focused on the relatively unvarying ILDs (cf. Rakerd & Hartmann, 2010), and therefore produced fixed-width images.4 While this brief analysis does not explain the numerous factors involved in ASW perception, it does demonstrate how the ability to detect 500-Hz interaural phase differences helps to explain ASW insensitivity in the current experiment.
E. Presbycusis and acoustics
This relationship between interaural phase acuity and ASW may have import for the design of rooms for the aged and/or hearing impaired. In a study of the relevance of IC variations in the acoustic measurement of auditoria, de Vries et al. (2001) demonstrated that the variations in IC found through minute changes in measurement location (Okano et al., 1988) would be imperceptible as the scale of variation was below NH IC-discrimination thresholds (Pollack and Trittipoe, 1959). The data here extends these acoustic imperceptions to much broader variations for older HI listeners. In the current study, the IC was controlled by attenuating two independent flanking noises, so the ratio of energy from lateral reflections compared to the energy from all directions – the lateral fraction – was significantly correlated with IC (r = −0.96; p < 0.01). However, lateral reflections are partially correlated with the source signal, whereas here the two flanking noises were uncorrelated with the source. Testing older HI listeners in a more realistic environment – with realistic sounds and proper early and late reflections – may induce variation in ASW along a coarser acoustic measure that can inform our understanding of how presbycusis affects our spatial percept of sound sources.
F. Concluding comments
The primary finding here is that sensitivity to changes in the perception of ASW based on IC can be partly predicted from the listener’s ability to detect interaural phase differences (i.e., binaural TFS thresholds). There were, however, participants with unmeasurable thresholds (i.e., a threshold so large that it does not lead to a perceivable difference with the stimuli used), despite verified audibility and continued practice. To establish better links between width and binaural acuity, better psychophysical measures of binaural TFS are necessary. To establish better links between ASW sensitivity and spatial perception in general, different aspects of localization, such as spatial separation (e.g., Noble et al., 1997) deserve further study. We suggest that the ASW insensitivity in older hearing-impaired individuals exhibited here over loudspeakers and previously over headphones (Whitmer et al., 2012) is because, as the aged auditory system becomes less sensitive to instantaneous variations in TFS, the resultant sound-source images are less dependent on stimulus IC.
ACKNOWLEDGMENTS
We would like to thank Associate Editor Dr. Michael Stone and two anonymous reviewers for their helpful comments. We would also like to thank Kay Foreman, Patrick Howell and Neil Kirk for their assistance in data collection, Dr. W. Owen Brimijoin for his assistance in stimulus presentation, and David McShefferty for his assistance in acoustic analysis. Portions of this research were presented at the International Hearing Aid Research Conference, California, US, August 2012, and the Association for Research in Otolaryngology Midwinter Meeting, Maryland, US, February 2013.
The Scottish Section of IHR is supported by intramural funding from the Medical Research Council (grant number U135097131) and the Chief Scientist Office of the Scottish Government. Seeber received intramural funding from the Medical Research Council (grant number U135097132) and funding by the German Federal Ministry for Education and Research (BMBF 01 GQ 1004B).
Footnotes
These are operational definitions for the current study. The term accuracy can also be used to refer to the RMS error, which conflates both the standard deviations of responses and the differences between responses and actual locations (cf. Hartmann, 1983). Our definition of accuracy is also known as the unsigned bias.
Binaural TFS thresholds are shown on a logarithmic scale in accord with Hopkins and Moore (2010); subsequent correlations with TFS threshold (Section III C 3) are therefore based on the log of these thresholds.
For the LF stimuli, 19 of all 35 regressions were significant fits of the data (R2 = 0.78-0.99; F(1,4) = 14.6-309.6; all p < 0.05); 14 of the 21 regressions for those with TFS data were significant (R2 = 0.82-0.99; F(1,4) = 18.8-309.6; all p < 0.05). For the HF stimuli, only 15 of all 35 regressions were significant (R2 = 0.67-0.98; F(1,4) = 8.1-243.2; all p < 0.05); 13 of the 21 regressions for those with TFS data were significant (R2 = 0.79-0.98; F(1,4) = 15.3-243.2; all p < 0.05). For the LTASS stimuli, 25 of all 35 regressions were significant (R2 = 0.67-0.99; F(1,4) = 8.1-425.8; all p < 0.05); 19 of 21 regressions for those with TFS data were significant (R2 = 0.68-0.99; F(1,4) = 8.5-425.8; all p < 0.05).
The current study used a three-up/one-down adaptive procedure instead of two-up/one-down, which could be expected to elevate thresholds somewhat (i.e., estimating 79% vs. 70% correct; Levitt, 1971). Regardless of this minor procedural change, however, 14 participants could not detect the maximum IPD (180°) used in the current test.
It is important to note that two of the 14 participants without TFS data here did show the expected decreases in ASW with increasing IC (participants 15 and 25 in Figure 5). It is quite possible that despite not being able to perform the binaural TFS task, these two participants still responded to the fluctuations in ITD shown by these models.
PACS numbers: 43.66.Pn, 43.66.Sr
Contributor Information
William M. Whitmer, MRC/CSO Institute of Hearing Research - Scottish Section, Glasgow Royal Infirmary, Glasgow, G31 2ER, United Kingdom
Bernhard U. Seeber, MRC Institute of Hearing Research, University Park, Nottingham, NG7 2RD, United Kingdom.
Michael A. Akeroyd, MRC/CSO Institute of Hearing Research - Scottish Section, Glasgow Royal Infirmary, Glasgow, G31 2ER, United Kingdom
V. REFERENCES
- Blauert J, Lindemann W. Spatial mapping of intracranial auditory events for various degrees of interaural coherence. J. Acoust. Soc. Am. 1986;79:806–813. doi: 10.1121/1.393471. [DOI] [PubMed] [Google Scholar]
- Blumlein A. UK patent 394, 325. 1931 Reprinted in .; J. Audio Eng. Soc. 1958;6:91–98. 130. [Google Scholar]
- British Society of Audiology Recommended procedures for pure tone audiometry using a manually operated instrument. Br. J. Audiol. 1981;15:213–216. doi: 10.3109/03005368109081440. [DOI] [PubMed] [Google Scholar]
- Buus S, Florentine M. Growth of loudness in listeners with cochlear hearing losses: Recruitment reconsidered. J. Assoc. Res. Otolaryngol. 2001;3:120–139. doi: 10.1007/s101620010084. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Byrne D, Dillon H, Tran K, Arlinger S, Wilbraham K, Cox R, Hagerman B, Hetu R, Kei J, Lui C, Kiessling J, Kotby M, Nasser N, El Kholy W, Nakanishi Y, Oyer H, Powell R, Stephens D, Meredith R, Sirimanna T, Tavartkiladze G, Frolenkov G, Westerman S, Ludvigsen C. An international comparison of long-term average speech spectra. J. Acoust. Soc. Am. 1994;96:2108–2120. [Google Scholar]
- de villiers Keet W. The influence of early lateral reflections on the spatial impression. Proceedings of the Sixth International Congress on Acoustics; Tokyo, Japan. 1968. pp. E2–E4. [Google Scholar]
- de Vries D, Hulsebos E, Baan J. Spatial fluctuations in measures for spaciousness. J. Acoust. Soc. Am. 2001;110:947–954. [Google Scholar]
- Dobreva M, O’Neill W, Paige G. The influence of aging on human sound localization. J. Neurophysiol. 2011;105:2471–2486. doi: 10.1152/jn.00951.2010. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Edmonds B, Culling J. Interaural correlation and the binaural summation of loudness. J. Acoust. Soc. Am. 2009;125:3865–3870. doi: 10.1121/1.3120412. [DOI] [PubMed] [Google Scholar]
- Faller C, Merimaa J. Source localization in complex listening situations: Selection of binaural cues based on interaural coherence. J. Acoust. Soc. Am. 2004;116:3075–3089. doi: 10.1121/1.1791872. [DOI] [PubMed] [Google Scholar]
- Goupell M, Hartmann W. Interaural fluctuations and the detection of interaural coherence, III. Narrowband experiments and binaural models. J. Acoust. Soc. Am. 2007;122:1029–1045. doi: 10.1121/1.2734489. [DOI] [PubMed] [Google Scholar]
- Greene N, Paige G. Influence of sound source width on human sound localization. Conf. Proc. IEEE Eng. Med. Biol. Soc.; 2012; 2012. pp. 6455–6458. [DOI] [PubMed] [Google Scholar]
- Hartmann W. Localization of sound in rooms. J. Acoust. Soc. Am. 1983;74:1380–1391. doi: 10.1121/1.390163. [DOI] [PubMed] [Google Scholar]
- Hartmann W, Cho Y. Generating partially correlated noise – A comparison of methods. J. Acoust. Soc. Am. 2011;130:292–301. doi: 10.1121/1.3596475. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hopkins K, Moore B. Development of a fast method for measuring sensitivity to temporal fine structure information at low frequencies. Int. J. Audiol. 2010;49:940–946. doi: 10.3109/14992027.2010.512613. [DOI] [PubMed] [Google Scholar]
- Levitt H. Transformed up-down methods in psychoacoustics. J. Acoust. Soc. Am. 1971;49:467–477. [PubMed] [Google Scholar]
- Lorenzi C, Gatehouse S, Lever C. Sound localization in noise in normal-hearing listeners. J. Acoust. Soc. Am. 1999a;105:1810–1820. doi: 10.1121/1.426719. [DOI] [PubMed] [Google Scholar]
- Lorenzi C, Gatehouse S, Lever C. Sound localization in noise in hearing-impaired listeners. J. Acoust. Soc. Am. 1999b;105:3454–3463. doi: 10.1121/1.424672. [DOI] [PubMed] [Google Scholar]
- Martens W. The impact of decorrelated low-frequency reproduction on auditory spatial imagery: Are two subwoofers better than one?. Proceedings of the Audio Engineering Society 16th International Conference of Spatial Sound Reproduction; Rovaniemi, Finland. 1999. pp. 67–77. [Google Scholar]
- Mason R, Brookes T, Rumsey F. Frequency dependency of the relationship between perceived auditory source width and the interaural cross-correlation coefficient for time-invariant stimuli. J. Acoust. Soc. Am. 2005;117:1337–1350. doi: 10.1121/1.1853113. [DOI] [PubMed] [Google Scholar]
- McFadden D, Jeffress L, Russell W. Individual differences in sensitivity to interaural differences in time and level. Percept. Motor Skills. 1973;37:755–761. doi: 10.2466/pms.1973.37.3.755. [DOI] [PubMed] [Google Scholar]
- Moore B, Glasberg B, Stoev M, Füllgrabe C, Hopkins K. The influence of age and high-frequency hearing loss on sensitivity to temporal fine structure at low frequencies. J. Acoust. Soc. Am. 2012;131:1003–1006. doi: 10.1121/1.3672808. [DOI] [PubMed] [Google Scholar]
- Moore J, Tollin D, Yin T. Can measures of sound localization acuity be related to the precision of absolute location estimates? Hear. Res. 2008;238:94–109. doi: 10.1016/j.heares.2007.11.006. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Neher T, Lunner T, Hopkins K, Moore B. Binaural temporal fine structure sensitivity, cognitive function, and spatial speech recognition of hearing-impaired listeners. J. Acoust. Soc. Am. 2012;131:2561–2564. doi: 10.1121/1.3689850. [DOI] [PubMed] [Google Scholar]
- Nilsson R, Liden G. Sound localization with phase audiometry. Acta Otolaryngol. 1976;81:291–299. doi: 10.3109/00016487609119965. [DOI] [PubMed] [Google Scholar]
- Noble W, Byrne D. A comparison of different binaural hearing aid systems for sound localization in the horizontal and vertical planes. Br. J. Audiol. 1990;24:335–346. doi: 10.3109/03005369009076574. [DOI] [PubMed] [Google Scholar]
- Noble W, Byrne D, Ter-Horst K. Auditory localization, detection of spatial separateness, and speech hearing in noise by hearing impaired listeners. J. Acoust. Soc. Am. 1997;102:2343–2352. doi: 10.1121/1.419618. [DOI] [PubMed] [Google Scholar]
- Okano T, Beranek L, Hidaka T. Relations among interaural cross-correlation coefficient (IACCE), lateral fraction (LFE), and apparent source width (ASW) in concert halls. J. Acoust. Soc. Am. 1988;104:255–265. doi: 10.1121/1.423955. [DOI] [PubMed] [Google Scholar]
- Perrott D, Buell T. Judgments of sound volume: Effects of signal duration, level, and interaural characteristics on the perceived extensity of broadband noise. J. Acoust. Soc. Am. 1982;72:1413–1417. doi: 10.1121/1.388447. [DOI] [PubMed] [Google Scholar]
- Pichora-Fuller M, Schneider B. Masking level differences in the elderly: A comparison of antiphasic and time-delay dichotic conditions. J. Speech Hear. Res. 1991;34:1410–1422. doi: 10.1044/jshr.3406.1410. [DOI] [PubMed] [Google Scholar]
- Pollack I, Trittipoe W. Binaural listening and interaural noise correlation. J. Acoust. Soc. Am. 1959;31:1250–1252. [Google Scholar]
- Rakerd B, Hartmann W. Localization of sound in rooms, V. Binaural coherence and human sensitivity to interaural time differences in noise. J. Acoust. Soc. Am. 2010;128:3052–3063. doi: 10.1121/1.3493447. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Rich G. A preliminary study of tonal volume. J. Exp. Psych. 1916;1:13–22. [Google Scholar]
- Saberi K, Antonio J. Precedence-effect thresholds for a population of untrained listeners as a function of stimulus intensity and interclick interval. J. Acoust. Soc. Am. 2003;114:420–429. doi: 10.1121/1.1578079. [DOI] [PubMed] [Google Scholar]
- Santala O, Pulkki V. Directional perception of distributed sound sources. J. Acoust. Soc. Am. 2011;129:1522–1530. doi: 10.1121/1.3533727. [DOI] [PubMed] [Google Scholar]
- Ueda K, Morimoto M. Estimation of auditory source width (ASW), I: ASW for two adjacent 1/3 octave band noises with equal band level. J. Acoust. Soc. Jpn. 1995;16:77–83. [Google Scholar]
- Ueda K, Tanaka T, Morimoto M. Estimation of auditory source width (ASW), II: ASW for two adjacent 1/3 octave band noises with different band levels. J. Acoust. Soc. Jpn. 1997;18:121–128. [Google Scholar]
- Whitmer W, Seeber B, Akeroyd M. Apparent auditory source width insensitivity in older hearing-impaired individuals. J. Acoust. Soc. Am. 2012;132:369–379. doi: 10.1121/1.4728200. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Yost W, Brown C. Localizing the sources of two independent noises: Role of time varying amplitude differences. J. Acoust. Soc. Am. 2013;133:2301–2313. doi: 10.1121/1.4792155. [DOI] [PMC free article] [PubMed] [Google Scholar]