Skip to main content
PMC home page
BJA: British Journal of Anaesthesia logoLink to BJA: British Journal of Anaesthesia
. 2014 Jul 26;113(4):585–595. doi: 10.1093/bja/aeu086

Theoretical modelling of fibrinogen supplementation with therapeutic plasma, cryoprecipitate, or fibrinogen concentrate

P W Collins 1,*, C Solomon 2,3, K Sutor 4, D Crispin 4, G Hochleitner 5, S Rizoli 6, H Schöchl 7,8, M Schreiber 9, M Ranucci 10
Editor: H C Hemmings
PMCID: PMC4166889  PMID: 25064078

Abstract

Background

We aimed to create a theoretical tool to model the effect of three haemostatic agents containing fibrinogen (therapeutic plasma, cryoprecipitate, and fibrinogen concentrate) on the patient's plasma fibrinogen level.

Methods

A mathematical model was developed step-wise. The relationship between the amount of haemostatic agent and plasma fibrinogen level was plotted for each agent. A fibrinogen concentration simulator (FCSamount) was developed, where the amount of haemostatic agent was calculated from patient characteristics, agent characteristics, and target plasma fibrinogen level. Refinements were introduced so that (i) FCSamount would account for in vivo fibrinogen recovery, (ii) circulatory volume would not increase ad infinitum with increasing amounts, and (iii) red blood cells would be included in the simulation if haematocrit decreased below a certain level. A second FCS (FCSlevel) was created to calculate fibrinogen levels resulting from specified amounts of haemostatic agents.

Results

Fibrinogen concentration in haemostatic agents has a critical impact on their ability to increase patients' fibrinogen levels. If the target plasma fibrinogen level approaches the concentration of the fibrinogen source, the required amounts increase exponentially; it is impossible to achieve a target above the concentration of the fibrinogen source.

Conclusions

We successfully developed two theoretical tools answering the questions: ‘How much therapeutic plasma, cryoprecipitate, or fibrinogen concentrate would be needed to achieve a specified target fibrinogen level?’ and ‘What would be the resultant fibrinogen level for a specified amount of haemostatic agent?’ The current tools are not intended for clinical application, but they are potentially useful for educational purposes.

Keywords: computer simulation, cryoprecipitate, drug dosage calculations, fibrinogen, plasma

Editor's key points.

  • Fibrinogen is an essential clotting factor that becomes critically low in massive haemorrhage.

  • Several fibrinogen-containing preparations are available with different fibrinogen concentrations and volumes.

  • A mathematical model was developed to compare these fibrinogen preparations.

  • Ability to increase patient fibrinogen level is critically dependent on starting fibrinogen level and the fibrinogen concentration of the preparation.

Fibrinogen is essential for blood clotting. Functioning both as a source of fibrin and as a mediator of platelet aggregation, it is the first coagulation factor to reach critically low levels during major blood loss and volume replacement.1 Low plasma fibrinogen levels are a primary issue with haemodilution related to the use of volume expanders, and are predictive of bleeding risk and need for transfusion of allogeneic blood products.2–4 Recent studies have shown favourable outcomes with fibrinogen supplementation in various clinical settings.5–9

There are three options for fibrinogen supplementation: therapeutic plasma, cryoprecipitate, and fibrinogen concentrate. The availability and licensing status of these haemostatic agents vary between countries.5,10,11 Each of them is prepared differently, and each has a different fibrinogen concentration, raising the question of whether they are equivalent in terms of their ability to raise patients' fibrinogen levels. We created two educational tools to explore the effects of these haemostatic agents on plasma fibrinogen level. Specifically, we designed the tools to answer the following questions: ‘How much therapeutic plasma, cryoprecipitate, or fibrinogen concentrate would be needed to achieve a specified target fibrinogen level?’ and ‘What would be the resultant fibrinogen level for a specified amount of haemostatic agent?’ From the outset, clinical application of these tools was not envisaged.

Methods

A mathematical model was constructed to examine the relationship between the amount of haemostatic agent and plasma fibrinogen level.

Assumptions upon which the mathematical model is based

  1. The patient starts with a certain plasma volume and fibrinogen level (it is assumed that all circulating fibrinogen is in the patient's plasma).

  2. Haemostatic agent (therapeutic plasma/cryoprecipitate/fibrinogen concentrate) is added 1 unit at a time until the target fibrinogen level or the specified amount of haemostatic agent is reached. If the number of units required to achieve the target fibrinogen level exceeds 100, the target level is considered as unattainable.

  3. Immediately before adding each unit of haemostatic agent, the same volume of whole blood is removed from the patient's circulation. (This is to avoid modelling an ever-increasing circulatory volume. Removal of volume simulates administration of haemostatic agent during ongoing bleeding; however, the model makes no assumptions regarding bleeding rate.)

  4. Upon adding a volume of haemostatic agent (which is all fluid), the patient's plasma volume is increased by the same amount.

  5. After adding each unit of haemostatic agent, a new fibrinogen level is calculated. This is based on the new plasma volume (changed because of removal of whole blood and subsequent addition of haemostatic agent) and the new total quantity of fibrinogen (changed because of removal of whole blood and addition of haemostatic agent with defined in vivo recovery).

  6. As a result of whole blood removal and subsequent addition of haemostatic agent, haematocrit decreases with every unit of haemostatic agent that is added. If haematocrit decreases below a defined threshold, 1 unit of red blood cells (RBCs) is added to the simulation at the same time as the next unit of haemostatic agent, as follows: (i) whole blood removed (volume removed=volume of one unit RBC+volume of haemostatic agent); (ii) RBC is added (assumption: RBC contains no fibrinogen); (iii) haemostatic agent is added.

  7. Haematocrit is calculated by subtracting plasma volume from the whole blood volume, and dividing the result by the whole blood volume. This approximation, which effectively ignores the volume of white blood cells and platelets, was used to avoid unnecessary complications.

Default values for key parameters

Default values were needed to provide a starting point for the model, although they should not be considered as definitive because of variability, for example, in the concentration of fibrinogen in therapeutic plasma. We searched the literature for data published on each parameter.

Concentration of fibrinogen within therapeutic plasma, cryoprecipitate, and fibrinogen concentrate

Default values: 2.0 g litre−1 (therapeutic plasma); 12 g litre−1 (cryoprecipitate); 20 g litre−1 (fibrinogen concentrate).

Published values for the concentration of fibrinogen in therapeutic plasma range between 1.6 and 5 g litre−1 (Supplementary Table S1); we chose a ‘default’ value of 2.0 g litre−1. A wide range of fibrinogen concentrations have been reported for cryoprecipitate, between 3.5 and 80 g litre−1 (Supplementary Table S1). Our chosen value of 12 g litre−1 is in accordance with these data. There is considerable variation in fibrinogen concentration between units of cryoprecipitate and therapeutic plasma because of differences between donors in plasma fibrinogen level.

The default concentration of fibrinogen in fibrinogen concentrate was chosen as 20 g litre−1 (1 g dissolved in 50 ml).11

Volume per unit of haemostatic agent

Default values: 250 ml (therapeutic plasma); 12.5 ml (cryoprecipitate); 50 ml (fibrinogen concentrate).

The volume of 1 unit of fresh-frozen plasma (FFP) is generally reported as 160–250 ml (Supplementary Table S1). Only one of the publications specified the contribution of citrate to the volume;12 in the other studies, the total volume described presumably includes citrate. The default value of 250 ml agrees with most reported values and ranges. Reported volumes per unit of cryoprecipitate range between 5 and 50 ml (Supplementary Table S1). The default volume of 12.5 ml is in agreement with numerous publications. For fibrinogen concentrate, the volume of 50 ml was chosen.

In vivo recovery

Default values: 100% (therapeutic plasma); 62% (cryoprecipitate); 114% (fibrinogen concentrate).

We are not aware of published data showing the in vivo recovery of fibrinogen after administration of therapeutic plasma. The default assumption is therefore 100%. For cryoprecipitate, in vivo fibrinogen recovery has been calculated as 62%.13 In the absence of any further data, this value is used as the default. In vivo recovery for fibrinogen concentrate has been reported as 114%,14 and this was therefore chosen as the default value.

RBCs: volume, concentration, and administration trigger

Default values: 280 ml (volume); 65% (haematocrit); 21% (patient's haematocrit level triggering RBC administration).

Reported volumes for 1 unit of RBC range between 200 and 350 ml.12,15–22 The default volume of 280 ml is in agreement with values reported by numerous publications.12,15,18–20 The haematocrit of concentrated RBC is reported in the literature to lie between 55% and 80%;12,15–21 a default value of 65% was chosen.

Administration triggers for RBC are usually based on haemoglobin concentration. However, for the mathematical model, a threshold based on haematocrit was required. The chosen default value of 21% corresponds with a proposed haemoglobin threshold of 7 g dl−1.23,24

Development of the mathematical model and the electronic tools

Step 1: initial consideration and graphical illustration

Arbitrary example values were chosen for blood volume and fibrinogen level at baseline (5 litre and 1.5 g litre−1, respectively). This enabled a basic model to be established, comparable with a recent publication,25 whereby haemostatic agents were added to the patient's blood volume. Additional data required for a simple model of ‘amount of haemostatic agent–plasma fibrinogen level’ relationship are the volume and fibrinogen content of the haemostatic agent added (see ‘Default values’ above). Choosing therapeutic plasma as an example, Table 1 shows the initial set of calculations.

Table 1.

Initial consideration of addition of therapeutic plasma to blood volume and the resultant plasma fibrinogen level. Assumptions as follows: the patient has a baseline plasma fibrinogen level of 1.5 g litre−1 and bodyweight of 70 kg (blood volume ∼5000 ml), 1 unit of therapeutic plasma contains 0.5 g fibrinogen in 250 ml, the patient is not actively losing blood, and the blood volume increases by the same volume as the amount of therapeutic plasma added (NB: in the final version of the model, the blood volume is kept static)

Units of therapeutic plasma administered Volume of therapeutic plasma administered (ml) Blood volume (ml) Fibrinogen added (g) Fibrinogen in patient's blood (g) Patient's plasma fibrinogen level (g litre−1)
0 (baseline) 0 5000 0.0 7.5 1.50
1 250 5250 0.5 8.0 1.52
2 500 5500 1.0 8.5 1.55
3 750 5750 1.5 9.0 1.57
4 1000 6000 2.0 9.5 1.58
5 1250 6250 2.5 10.0 1.60
6 1500 6500 3.0 10.5 1.62
7 1750 6750 3.5 11.0 1.63
8 2000 7000 4.0 11.5 1.64
9 2250 7250 4.5 12.0 1.66
10 2500 7500 5.0 12.5 1.67
Open in a new tab

For graphical illustration, the data shown in Table 1 were replicated for the simulations with cryoprecipitate and fibrinogen concentrate, and then plotted on a graph (similar to a graph recently published by Gorlinger and colleagues).25 Again, arbitrary example values were used for the patient's baseline circulation volume (5 litre) and plasma fibrinogen level (1.5 g litre−1); default values described above were incorporated as the volume and fibrinogen concentration of each haemostatic agent.

Step 2: initial development of a fibrinogen concentration simulator

The next step was to enable fibrinogen concentration to be modelled with different parameters (e.g. different bodyweight, baseline, or target fibrinogen level). To this end, the ‘amount of haemostatic agent–plasma fibrinogen level’ relationship was programmed into Microsoft Excel, and a graphical user interface (GUI) was constructed. The GUI was designed for the user to specify key parameters: bodyweight, baseline haematocrit, baseline fibrinogen level, target fibrinogen level for the patient, and concentration of fibrinogen and volume per unit of each haemostatic agent.

Key equations used to develop the fibrinogen concentration simulator for calculation of the amount of haemostatic agent (FCSamount) are detailed in the Supplementary Methods S1. Figure 1 shows a graph created by the Excel tool.

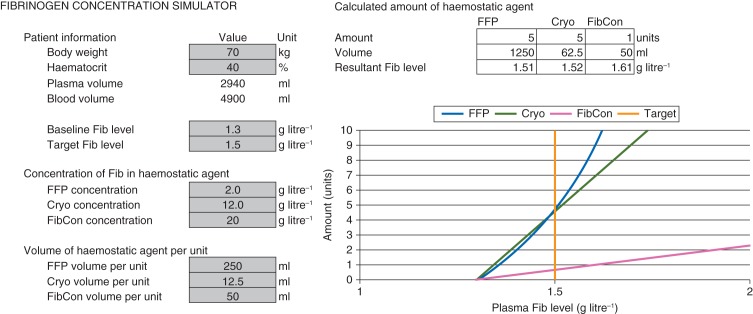
Fig 1.

Fig 1

Open in a new tab

Plot from unrefined version of the Excel-based fibrinogen concentration simulator. Values in cells highlighted grey are specified by the user; the graph is plotted based on these parameters. Cryo, cryoprecipitate; FFP, fresh-frozen plasma; Fib, fibrinogen; FibCon, fibrinogen concentrate.

Step 3: refinements of FCSamount and creation of a second electronic tool

In vivo recovery was added to the model because the increase in plasma fibrinogen level might not correspond exactly with the quantity of fibrinogen administered. In vivo recovery was defined as the actual increase in fibrinogen level divided by the expected increase.14

In the initial version of the model, circulatory volume increased without limitation as haemostatic agents were added. With large amounts of therapeutic plasma, this is unrealistic. The model was modified to keep circulatory volume constant: a volume of whole blood equivalent to the volume of the haemostatic agent is removed from circulation immediately before adding the haemostatic agent. This prompted changing from a continuous to a discrete model, with significant mathematical alterations.

As a result of removing whole blood and subsequently adding therapeutic plasma, cryoprecipitate, or fibrinogen concentrate, haematocrit decreases as each unit of haemostatic agent is added. The model was therefore modified to include addition of RBCs when haematocrit decreases below a defined threshold.

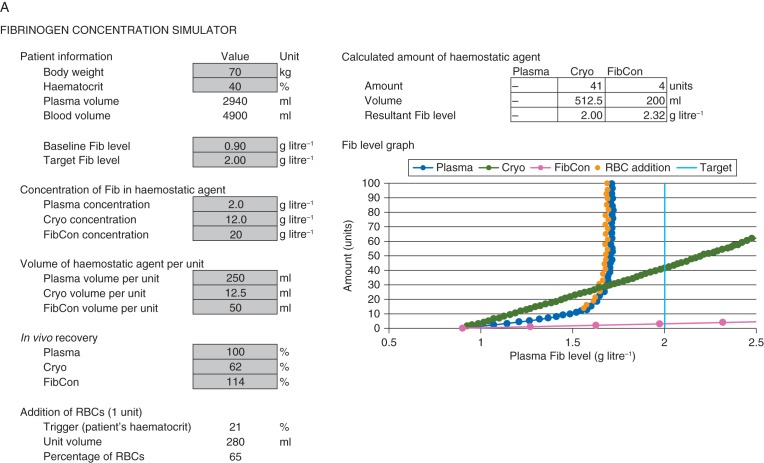
Details of the mathematics associated with these refinements are presented in the Supplementary Methods S2, and the finalized Excel-based FCSamount is shown in Figure 2a.

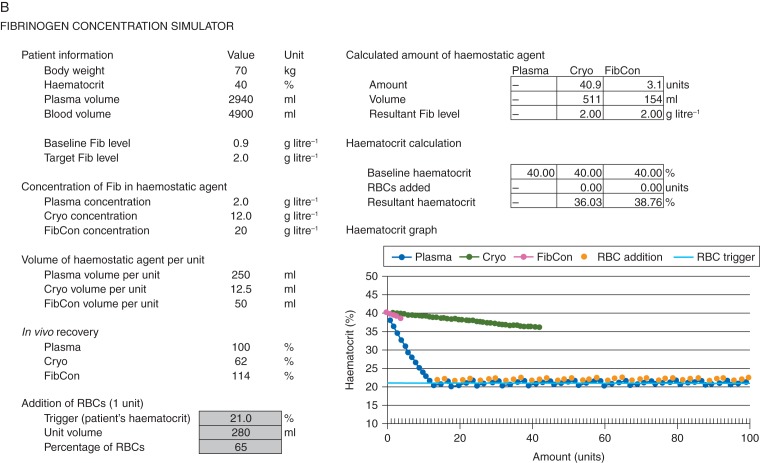
Fig 2.

Fig 2

Fig 2

Fig 2

Open in a new tab

Finalized Excel-based FCSamount and FCSlevel after implementation of refinements. (a) Fibrinogen concentration simulator (FCSamount): calculation of amount of haemostatic agent; (b) haematocrit graph from FCSamount; (c) FCSlevel: calculation of resultant plasma fibrinogen level. Values in cells highlighted light grey are specified by the user; the graphs are plotted based on these parameters. Cryo, cryoprecipitate; Fib, fibrinogen; FibCon, fibrinogen concentrate; RBC, red blood cell.

Since haematocrit was being analysed as part of the model, it was decided to create a second graph to show the relationship between amount of haemostatic agent and haematocrit. For each of the three agents, this graph stops at the number of units needed to reach the target plasma fibrinogen level (or a maximum of 100 units if the target was unattainable). Figure 2b shows the haematocrit graph.

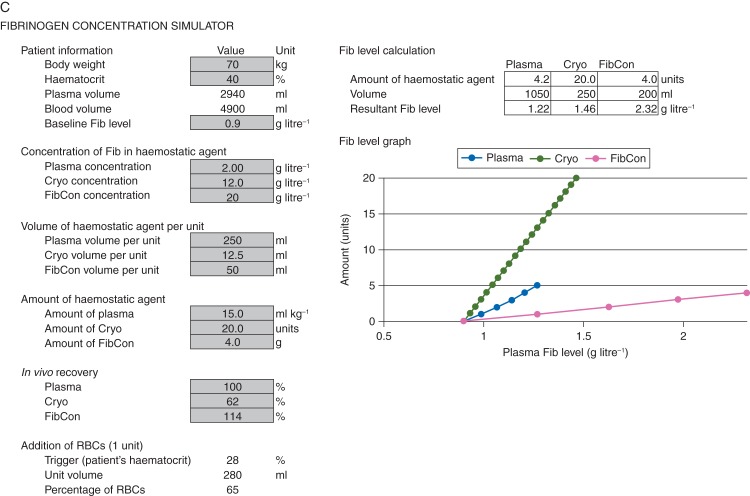
FCSamount was designed to answer the question ‘How much therapeutic plasma, cryoprecipitate, or fibrinogen concentrate would be needed to achieve a specified target fibrinogen level?’ A new tool (FCSlevel) was created using the same mathematical model to address the question ‘What would be the resultant fibrinogen level for a specified amount of haemostatic agent?’ The user is able to specify the amount either by the absolute quantity or by quantity per kilogram of the patient's bodyweight. The resulting Excel-based FCSlevel is shown in Figure 2c; it is intended to complement FCSamount. The following doses appear when FCSlevel is first opened: 15 mL kg−1 for therapeutic plasma, 20 units for cryoprecipitate and 4 g for fibrinogen concentrate.

Results

Initial graphical illustration (before refinements)

From a baseline fibrinogen level of 1.5 g litre−1, it was calculated that 14 units of therapeutic plasma would be needed to surpass a target level of 1.7 g litre−1, compared with 8 units of cryoprecipitate and 2 units of fibrinogen concentrate. Higher target levels, above ∼1.8 g litre−1, would be difficult or impossible to achieve with therapeutic plasma because the number of units increases exponentially as the target value approaches the fibrinogen level of the haemostatic agent itself (2.0 g litre−1).

Initial theoretical fibrinogen concentration simulator

Using lower values for baseline and target fibrinogen level (1.3 and 1.5 g litre−1, respectively) reduced the required amount of therapeutic plasma (Fig. 1; 5 units, compared with 8 units when applying the simulator to baseline and target levels of 1.5 and 1.7 g litre−1). Conversely, the required amounts of cryoprecipitate and fibrinogen concentrate were the same when using the simulator for baseline and target fibrinogen levels of 1.3 and 1.5 or 1.5 and 1.7 g litre−1. The scenario shown in Figure 1 indicates that low target plasma fibrinogen levels would be achievable with modest amounts of all three haemostatic agents. The required amounts of all three haemostatic agents are increased by increasing the patient's bodyweight, decreasing the patient's haematocrit, or increasing the difference between baseline and target plasma fibrinogen level.

For any fibrinogen supplementation option, the required amount would increase exponentially if the target fibrinogen level were to approach that of the haemostatic agent, and this is often very apparent for therapeutic plasma. Recent European guidelines on the management of severe perioperative bleeding or the management of bleeding in trauma recommend fibrinogen supplementation if significant bleeding is accompanied by a plasma fibrinogen level less than 1.5–2 g litre−1.6 The model shows that, from a mathematical point of view, the plasma fibrinogen level cannot be increased above or maintained at 2 g litre−1 if the fibrinogen concentration in the haemostatic agent is 2 g litre−1 or below. For fibrinogen concentrate and cryoprecipitate, in most circumstances, the ‘amount of haemostatic agent–plasma fibrinogen level’ relationship approximates to linear. If the target plasma level were set theoretically high (>10 g litre−1), exponential increases in the required amounts of these haemostatic agents would also become apparent.

Finalized theoretical electronic tools

The inclusion of in vivo recovery added a new level of control to the model, with amount of haemostatic agent increasing as in vivo recovery decreases (greatest effect with cryoprecipitate, whose in vivo recovery has been reported as 62%). On the other hand, the introduction of a constant circulatory volume decreased the amount required to reach a certain target fibrinogen level, particularly with therapeutic plasma (the effect was small with cryoprecipitate and fibrinogen concentrate). The introduction of RBC transfusions into the model for FCSamount had only a small effect on calculated amounts, but it served to highlight the effect of each haemostatic agent on the patient's haematocrit. In many circumstances, the theoretical model indicates that RBC transfusion would not be triggered, but that the addition of therapeutic plasma would result in larger decreases in haematocrit than the other haemostatic agents. FCSlevel indicates the need for relatively high amounts of therapeutic plasma and cryoprecipitate to achieve meaningful increases in plasma fibrinogen levels.

The tools were applied to simulate situations described in post-partum haemorrhage (PPH),26 cardiovascular surgery (aortic replacement),27 and trauma.28 In all three cases, values entered into the FCS were based on published/on-going research, and the results are shown in the Supplementary Appendix. Perhaps most notably, the model suggests that therapeutic plasma may not always increase the patient's plasma fibrinogen level. In the context of PPH, where the patient's fibrinogen plasma level may be above 2 g litre−1 before haemostatic therapy, the mathematics indicate that addition of therapeutic plasma could decrease the plasma fibrinogen level.

Discussion

FCSamount and FCSlevel simulate fibrinogen supplementation with therapeutic plasma, cryoprecipitate, or fibrinogen concentrate, using a theoretical, mathematical model. These tools inform physicians of the theoretical feasibility of achieving a target level of fibrinogen, or the theoretical effect of a specified amount of haemostatic agent on a plasma fibrinogen level. FCSamount and FCSlevel also highlight the lack of effect if the patient's plasma fibrinogen level approaches the concentration of fibrinogen in the haemostatic agent itself.

Despite providing such insight, the theoretical tools we developed do not account for clinical realities such as rate of blood loss, administration of fluid replacement and haemostatic components such as platelets, achievement of haemostasis or vascular constriction (reducing circulatory volume). Also, a significant proportion of bleeding patients receive volume replacement with a colloid or crystalloid. This would have an impact on the plasma fibrinogen level, and potentially impair fibrin polymerization (particularly if hydroxyethyl starch were used).29 The characteristics of coagulopathy are highly variable, and there are differences between countries in haemostatic therapy approaches as well as availability/licensing status of haemostatic agents.5,6,10,11,28,30–32 Another consideration is that the time between ordering a haemostatic agent and its administration to the patient varies between agents and between institutions.11 Clinicians should use their judgement to choose between the available haemostatic agents according to the clinical situation and the patient's haemostatic parameters. The tools we developed should not be used to determine doses in clinical settings. Optimal trigger and target plasma fibrinogen levels are poorly defined but, importantly it has been shown that clot strength increases with fibrinogen level over a wide range of values, from 0 to 9.5 g litre−1.33,34

It is often assumed that plasma fibrinogen level increases linearly with the dose of haemostatic agent containing fibrinogen. In contrast, we observed a non-linear increase in the amount required to achieve a target plasma fibrinogen level as the target approaches the concentration of the fibrinogen source. Moreover, unless in vivo recovery of fibrinogen is above 100%, it is not possible to exceed the concentration of the fibrinogen source. Moreover, unless in vivo recovery of fibrinogen is above 100%, it is not possible to exceed the concentration of the fibrinogen source. Documentation of a specific dose requirement for a given increase in fibrinogen level would be misleading, because the dose would depend on the starting plasma fibrinogen level and other clinical considerations. Another assumption sometimes made is that total fibrinogen dose can be calculated by adding up the quantities of fibrinogen delivered to the patient via different haemostatic agents.35,36 As modelled by FCSamount, the effect of a certain quantity of fibrinogen on plasma fibrinogen level might differ according to the haemostatic agent used. Therefore, calculation of total fibrinogen dose by adding quantities administered via different haemostatic agents may not be meaningful. In some circumstances, the model indicates that the addition of therapeutic plasma may in fact dilute the patient's plasma fibrinogen.

The value of mathematical modelling has been demonstrated previously. Hirshberg and colleagues37 reported that the dilution of clotting factors is a key aspect in severe haemorrhage and that optimal transfusion ratios are 2:3 for FFP:RBC, and 8:10 for platelets:RBC. Similarly, Ho and colleagues38 showed that an FFP:RBC transfusion ratio of 1:1 would be needed to prevent dilution. A 1:1:1 approach for transfusion of allogeneic blood products has been advocated, although the benefit of this approach is still under investigation.39,40

We included in vivo recovery within the modelling for the tools we developed. It must be acknowledged that the evidence base for in vivo recovery of fibrinogen is poor for all three haemostatic agents included in the FCS, and is likely to differ between clinical situations. A further uncertainty is the hypothesis that hypovolaemia might be the cause of in vivo recovery values above 100% for fibrinogen concentrate. The model assumes no change in circulation volume, so, if the hypovolaemia hypothesis were correct, it would be inappropriate to use values above 100% without introducing decreased circulation volume to the model.

Variability of fibrinogen concentration in the haemostatic agents included in our simulations introduces imprecision to any calculations of fibrinogen levels. When using FCSamount or FCSlevel, users can specify different fibrinogen concentrations for these agents, enabling the calculations to be tailored to what is known about the agents used at their centre.

In conclusion, we have successfully developed a mathematical model to show theoretical relationships between amount and plasma fibrinogen level achieved, for therapeutic plasma, cryoprecipitate, and fibrinogen concentrate. The tools we developed are not intended for clinical application and there is scope to develop them further. Nevertheless, we envisage that the current versions might be used for educational purposes.

Authors' contributions

G.H. prepared the initial Excel spreadsheet, represented in Table 1. D.C. developed the mathematical formulae and coding for the electronic tools, working together with K.S., G.H., and C.S. P.W.C. provided clinical input regarding postpartum haemorrhage; S.R., H.S., and M.S. advised regarding trauma; C.S. and M.R. provided expertise relating to cardiovascular surgery. All co-authors contributed to the assumptions on which the modelling was based. K.S. was involved in drafting the manuscript; all co-authors reviewed it critically and approved the final version.

Supplementary material

Supplementary material is available at British Journal of Anaesthesia online.

Declaration of interest

P.W.C. received honoraria for talks and research funding from CSL Behring and research support from Tem International. C.S. is an employee of CSL Behring and, before that, received speaker honoraria, research support, or both from Tem International and CSL Behring, and travel support from Haemoscope Ltd. K.S. and D.C. contributions to the development of the electronic tools and this manuscript was funded by CSL Behring via Meridian HealthComms. G.H. is an employee of CSL Behring. S.R. has received honoraria and is a Scientific Advisory Board member for CSL Behring and KCI; he has also received an investigator award from the Canadian Institutes of Health Research in partnership with Novo Nordisk. H.S. has received speaker fees, travel support, and research support from CSL Behring and Tem International, as well as consultancy fees from CSL Behring. M.R. has received honoraria, research support, or both from CSL Behring, Grifols SA, Medtronic, Novo Nordisk, and Roche.

Funding

Development of the electronic tools and medical writing were supported by CSL Behring.

Supplementary Material

Supplementary Data
supp_113_4_585__index.html (1,013B, html)

Acknowledgements

The authors would like to thank K. Görlinger for the original concept of plotting fibrinogen level against the amount of haemostatic agent added25 and K.A. Tanaka for adding cryoprecipitate to the model.

References

  • 1.Hiippala ST, Myllyla GJ, Vahtera EM. Hemostatic factors and replacement of major blood loss with plasma-poor red cell concentrates. Anesth Analg. 1995;81:360–5. doi: 10.1097/00000539-199508000-00026. [DOI] [PubMed] [Google Scholar]
  • 2.Charbit B, Mandelbrot L, Samain E, et al. The decrease of fibrinogen is an early predictor of the severity of postpartum hemorrhage. J Thromb Haemost. 2007;5:266–73. doi: 10.1111/j.1538-7836.2007.02297.x. [DOI] [PubMed] [Google Scholar]
  • 3.Rourke C, Curry N, Khan S, et al. Fibrinogen levels during trauma hemorrhage, response to replacement therapy, and association with patient outcomes. J Thromb Haemost. 2012;10:1342–51. doi: 10.1111/j.1538-7836.2012.04752.x. [DOI] [PubMed] [Google Scholar]
  • 4.Ucar HI, Oc M, Tok M, et al. Preoperative fibrinogen levels as a predictor of postoperative bleeding after open heart surgery. Heart Surg Forum. 2007;10:E392–6. doi: 10.1532/HSF98.20071065. [DOI] [PubMed] [Google Scholar]
  • 5.Gorlinger K, Dirkmann D, Hanke AA, et al. First-line therapy with coagulation factor concentrates combined with point-of-care coagulation testing is associated with decreased allogeneic blood transfusion in cardiovascular surgery: a retrospective, single-center cohort study. Anesthesiology. 2011;115:1179–91. doi: 10.1097/ALN.0b013e31823497dd. [DOI] [PubMed] [Google Scholar]
  • 6.Spahn DR, Bouillon B, Cerny V, et al. Management of bleeding and coagulopathy following major trauma: an updated European guideline. Crit Care. 2013;17:R76. doi: 10.1186/cc12685. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 7.Kozek-Langenecker S, Sorensen B, Hess JR, Spahn DR. Clinical effectiveness of fresh frozen plasma compared with fibrinogen concentrate: a systematic review. Crit Care. 2011;15:R239. doi: 10.1186/cc10488. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 8.Warmuth M, Mad P, Wild C. Systematic review of the efficacy and safety of fibrinogen concentrate substitution in adults. Acta Anaesthesiol Scand. 2012;56:539–48. doi: 10.1111/j.1399-6576.2011.02586.x. [DOI] [PubMed] [Google Scholar]
  • 9.Yang L, Stanworth S, Baglin T. Cryoprecipitate: an outmoded treatment? Transfus Med. 2012;22:315–20. doi: 10.1111/j.1365-3148.2012.01181.x. [DOI] [PubMed] [Google Scholar]
  • 10.Kozek-Langenecker SA, Afshari A, Albaladejo P, et al. Management of severe perioperative bleeding: guidelines from the European Society of Anaesthesiology. Eur J Anaesthesiol. 2013;30:270–382. doi: 10.1097/EJA.0b013e32835f4d5b. [DOI] [PubMed] [Google Scholar]
  • 11.Levy JH, Welsby I, Goodnough LT. Fibrinogen as a therapeutic target for bleeding: a review of critical levels and replacement therapy. Transfusion. 2014;54:1389–405. doi: 10.1111/trf.12431. [DOI] [PubMed] [Google Scholar]
  • 12.UK Blood Transfusion & Tissue Transplantation Services. Transfusion Handbook. Available from http://www.transfusionguidelines.org.uk/index.aspx?Publication=HTM. accessed January 2013. [Google Scholar]
  • 13.Lee SH, Lee SM, Kim CS, et al. Fibrinogen recovery and changes in fibrin-based clot firmness after cryoprecipitate administration in patients undergoing aortic surgery involving deep hypothermic circulatory arrest. Transfusion. 2014;54:1379–87. doi: 10.1111/trf.12479. [DOI] [PubMed] [Google Scholar]
  • 14.Solomon C, Pichlmaier U, Schoechl H, et al. Recovery of fibrinogen after administration of fibrinogen concentrate to patients with severe bleeding after cardiopulmonary bypass surgery. Br J Anaesth. 2010;104:555–62. doi: 10.1093/bja/aeq058. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 15.American Association of Blood Banks (AABB); American Red Cross; America's Blood Centers; and the Armed Services Blood Program. Circular of information for the use of human blood and blood components. 2009. Available from http://www.aabb.org/resources/bct/Documents/coi0809r.pdf. accessed July 2013.
  • 16.Armand R, Hess JR. Treating coagulopathy in trauma patients. Transfus Med Rev. 2003;17:223–31. doi: 10.1016/s0887-7963(03)00022-1. [DOI] [PubMed] [Google Scholar]
  • 17.Arya RC, Wander G, Gupta P. Blood component therapy: which, when and how much. J Anaesthesiol Clin Pharmacol. 2011;27:278–84. doi: 10.4103/0970-9185.81849. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 18.Canadian Blood Services. Clinical guide to transfusion. Available from http://www.transfusionmedicine.ca/sites/transfusionmedicine/files/CBS-CGT-BM.pdf. accessed January 2013.
  • 19.Elzik ME, Dirschl DR, Dahners LE. Correlation of transfusion volume to change in hematocrit. Am J Hematol. 2006;81:145–6. doi: 10.1002/ajh.20517. [DOI] [PubMed] [Google Scholar]
  • 20.Liumbruno G, Bennardello F, Lattanzio A, Piccoli P, Rossetti G. Recommendations for the transfusion of red blood cells. Blood Transfus. 2009;7:49–64. doi: 10.2450/2008.0020-08. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 21.Vermeulen Windsant IC, de Wit NC, Sertorio JT, et al. Blood transfusions increase circulating plasma free hemoglobin levels and plasma nitric oxide consumption: a prospective observational pilot study. Crit Care. 2012;16:R95. doi: 10.1186/cc11359. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 22.German Medical Association. Cross-sectional Guidelines for Therapy with Blood Components and Plasma Derivatives. 4th revised Edn. 2009. Available from https://www.iakh.de/tl_files/iakh/public/richtlinien/Querschnittsleitlinie_4._Auflage_-_englisch_05.01.2011%20copy.pdf. accessed July 2013. [Google Scholar]
  • 23.Hebert PC, Wells G, Blajchman MA, et al. A multicenter, randomized, controlled clinical trial of transfusion requirements in critical care: Transfusion Requirements in Critical Care Investigators, Canadian Critical Care Trials Group. N Engl J Med. 1999;340:409–17. doi: 10.1056/NEJM199902113400601. [DOI] [PubMed] [Google Scholar]
  • 24.Nijboer JM, van der Horst IC, Hendriks HG, ten Duis HJ, Nijsten MW. Myth or reality: hematocrit and hemoglobin differ in trauma. J Trauma. 2007;62:1310–2. doi: 10.1097/TA.0b013e3180341f54. [DOI] [PubMed] [Google Scholar]
  • 25.Gorlinger K, Shore-Lesserson L, Dirkmann D, Hanke AA, Rahe-Meyer N, Tanaka KA. Management of hemorrhage in cardiothoracic surgery. J Cardiothorac Vasc Anesth. 2013;27:S20–34. doi: 10.1053/j.jvca.2013.05.014. [DOI] [PubMed] [Google Scholar]
  • 26.Cunningham FG. Laboratory values in normal pregnancy. Available from http://onlinelibrary.wiley.com/doi/10.1002/9781444323870.app2/pdf. accessed May 2013.
  • 27.Rahe-Meyer N, Solomon C, Hanke A, et al. Effects of fibrinogen concentrate as first-line therapy during major aortic replacement surgery: a randomized, placebo-controlled trial. Anesthesiology. 2013;118:40–50. doi: 10.1097/ALN.0b013e3182715d4d. [DOI] [PubMed] [Google Scholar]
  • 28.Schochl H, Nienaber U, Hofer G, et al. Goal-directed coagulation management of major trauma patients using thromboelastometry (ROTEM)-guided administration of fibrinogen concentrate and prothrombin complex concentrate. Crit Care. 2010;14:R55. doi: 10.1186/cc8948. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 29.Fries D, Innerhofer P, Reif C, et al. The effect of fibrinogen substitution on reversal of dilutional coagulopathy: an in vitro model. Anesth Analg. 2006;102:347–51. doi: 10.1213/01.ane.0000194359.06286.d4. [DOI] [PubMed] [Google Scholar]
  • 30. American Society of Anesthesiologists Committee on Blood Management. Massive transfusion protocol (MTP) for hemorrhagic shock. Available from: http://www.asahq.org/For-Members/Standards-Guidelines-and-Statements.aspx. (accessed July 2013)
  • 31.Holcomb JB, del Junco DJ, Fox EE, et al. The prospective, observational, multicenter, major trauma transfusion (PROMMTT) study: comparative effectiveness of a time-varying treatment with competing risks. JAMA Surg. 2013;148:127–36. doi: 10.1001/2013.jamasurg.387. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 32.Johansson PI, Stensballe J. Effect of Haemostatic Control Resuscitation on mortality in massively bleeding patients: a before and after study. Vox Sang. 2009;96:111–8. doi: 10.1111/j.1423-0410.2008.01130.x. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 33.Dempfle CE, Kalsch T, Elmas E, et al. Impact of fibrinogen concentration in severely ill patients on mechanical properties of whole blood clots. Blood Coagul Fibrinolysis. 2008;19:765–70. doi: 10.1097/MBC.0b013e32830f1b68. [DOI] [PubMed] [Google Scholar]
  • 34.Bolliger D, Szlam F, Molinaro RJ, Rahe-Meyer N, Levy JH, Tanaka KA. Finding the optimal concentration range for fibrinogen replacement after severe haemodilution: an in vitro model. Br J Anaesth. 2009;102:793–9. doi: 10.1093/bja/aep098. [DOI] [PubMed] [Google Scholar]
  • 35.Weiss G, Lison S, Glaser M, et al. Observational study of fibrinogen concentrate in massive hemorrhage: evaluation of a multicenter register. Blood Coagul Fibrinolysis. 2011;22:727–34. doi: 10.1097/MBC.0b013e32834cb343. [DOI] [PubMed] [Google Scholar]
  • 36.Stinger HK, Spinella PC, Perkins JG, et al. The ratio of fibrinogen to red cells transfused affects survival in casualties receiving massive transfusions at an army combat support hospital. J Trauma. 2008;64:S79–85. doi: 10.1097/TA.0b013e318160a57b. [DOI] [PubMed] [Google Scholar]
  • 37.Hirshberg A, Dugas M, Banez EI, Scott BG, Wall MJ, Jr, Mattox KL. Minimizing dilutional coagulopathy in exsanguinating hemorrhage: a computer simulation. J Trauma. 2003;54:454–63. doi: 10.1097/01.TA.0000053245.08642.1F. [DOI] [PubMed] [Google Scholar]
  • 38.Ho AM, Dion PW, Cheng CA, et al. A mathematical model for fresh frozen plasma transfusion strategies during major trauma resuscitation with ongoing hemorrhage. Can J Surg. 2005;48:470–8. [PMC free article] [PubMed] [Google Scholar]
  • 39.Bhananker SM, Ramaiah R. Trends in trauma transfusion. Int J Crit Illn Inj Sci. 2011;1:51–6. doi: 10.4103/2229-5151.79282. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 40.Dente CJ, Shaz BH, Nicholas JM, et al. Improvements in early mortality and coagulopathy are sustained better in patients with blunt trauma after institution of a massive transfusion protocol in a civilian level I trauma center. J Trauma. 2009;66:1616–24. doi: 10.1097/TA.0b013e3181a59ad5. [DOI] [PubMed] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Data
supp_113_4_585__index.html (1,013B, html)
supp_aeu086_aeu086supp_data.doc (701KB, doc)

Articles from BJA: British Journal of Anaesthesia are provided here courtesy of Elsevier

RESOURCES