The Functional Anatomy of Single-Digit Arithmetic in Children with Developmental Dyslexia

Abstract

Some arithmetic procedures, such as addition of small numbers, rely on fact retrieval mechanisms supported by left hemisphere perisylvian language areas, while others, such as subtraction, rely on procedural-based mechanisms subserved by bilateral parietal cortices. Previous work suggests that developmental dyslexia, a reading disability, is accompanied by subtle deficits in retrieval-based arithmetic, possibly because of compromised left hemisphere function. To test this prediction, we compared brain activity underlying arithmetic problem solving in children with and without dyslexia during addition and subtraction operations using a factorial design. The main effect of arithmetic operation (addition versus subtraction) for both groups combined revealed activity during addition in the left superior temporal gyrus and activity during subtraction in bilateral intraparietal sulcus, right supramarginal gyrus and the anterior cingulate, consistent with prior studies. For the main effect of diagnostic group (dyslexics versus controls), we found less activity in dyslexic children in the left supramarginal gyrus. Finally, the interaction analysis revealed that while the control group showed a strong response in right supramarginal gyrus for subtraction but not for addition, the dyslexic group engaged this region for both operations. This provides physiological evidence in support of the theory that children with dyslexia, because of disruption to left hemisphere language areas, use a less optimal route for retrieval-based arithmetic, engaging right hemisphere parietal regions typically used by good readers for procedural-based arithmetic. Our results highlight the importance of language processing for mathematical processing and illustrate that children with dyslexia have impairments that extend beyond reading.

1. INTRODUCTION

Proficient mathematical cognition is the basis for many routine activities in our daily lives (e.g., keeping track of time or money) and a key factor in children’s academic success. Arithmetic, the branch of mathematics concerned with addition, subtraction, multiplication, and division, is especially important in the early stages of math learning. As such, there has been significant interest in the neural basis of normal mathematical cognition and numerosity (Menon, 2010; Nieder and Dehaene, 2009), as well as its disorders (for review, see Ashkenazi et al., 2013). A model of number processing that integrates brain imaging with behavioral and patient studies has been put forward as the “triple code model” (Dehaene, 1992; Dehaene and Cohen, 1995; Dehaene et al., 2003). This model specifies that distinct brain regions are assigned to specific systems of representations of numerical information (quantitative, verbal, and visual systems), and their respective contributions vary depending on the task. For example, bilateral parietal cortices have been identified as a locus of quantity representation (Nieder and Dehaene, 2009), and have been shown to elicit greater activity when individuals solve arithmetic problems with more procedural demands such as subtraction and division (Rosenberg-Lee et al., 2011; De Smedt et al., 2011). Other aspects of mental arithmetic, such as verbal representation of numbers and fact retrieval, have been associated with language functions (i.e., the verbal representation of numbers in the triple code model) and are thought to be subserved by left hemisphere perisylvian language areas (Dehaene et al., 1999; Dehaene et al., 2003). Examples of brain imaging studies in support of this include the demonstration that addition of small numbers or well-rehearsed arithmetic facts such as multiplication, both of which utilize verbal retrieval, elicit activity in language areas, including left hemisphere angular, supramarginal, and inferior frontal gyri (Stanislas Dehaene et al., 2003; Gruber et al., 2001; Rosenberg-Lee et al., 2011). Grabner et al. (2007) have shown that adults with higher mathematical competence have stronger activation of the left angular gyrus while solving multiplication problems, suggesting their stronger reliance on language-mediated processes for multiplication. Grabner and colleagues (2009) also showed stronger activation of the left angular gyrus while solving arithmetic problems for which participants reported using fact retrieval rather than procedural strategies.

These studies demonstrate an important role for left hemisphere language areas in specific aspects of arithmetic and dovetail with earlier behavioral studies, which have revealed a relationship between phonological awareness (i.e., the ability to isolate and manipulate the sounds of words) and computation of arithmetic problems solved through verbal fact retrieval (i.e., small addition and multiplication, which are more likely to be retrieved), but not subtraction and division problems, which are typically solved through procedural strategies. Studies in typically developing children have identified a relationship between phonological awareness and later mathematical skill development (Simmons and Singleton, 2008). For example, Hecht and colleagues (2001) found that phonological awareness from a range of measures (including phonological memory and the rate of access to phonological codes) was the best long-term predictor of mathematical competency (measured by untimed computation and timed small-digit arithmetic) in the later elementary school years. De Smedt et al. (2010) found a positive correlation in fifth graders between phonological awareness skills (measured by a phoneme elision task) and speed and accuracy on an arithmetic verification task (in which subjects determine whether an arithmetic problem is correct or incorrect). Importantly, this relationship was found to be specific to arithmetic problems likely to be solved through retrieval mechanisms, suggesting an important connection between phonological awareness and arithmetic fact retrieval.

These findings have led to the idea that developmental dyslexia, a reading disability characterized by core weakness in reading and phonological awareness, is concomitant with weaknesses in arithmetic (De Smedt and Boets, 2010; Simmons and Singleton, 2008). Developmental dyslexia is a common learning disability, neurobiological in origin, that is characterized by poor reading that cannot be accounted for by low intelligence (Lyon et al., 2003; Peterson and Pennington, 2012). It is marked by difficulties in recognizing and decoding single words, the latter thought to be due to a deficit in phonological awareness. Many students with dyslexia are also diagnosed with math disability, and incidence rates of deficits in both have been reported to be as high as 50% (Lewis et al., 1994). Further, candidate susceptibility genes for dyslexia (e.g., ROBO1) appear to contribute to not only dyslexia but also its correlated phenotypes like math difficulties (Mascheretti et al., 2014). Interestingly, even dyslexic children with scores in the normal range in mathematics (assessed via standardized tests) show subtle deficits in arithmetic performance (Simmons and Singleton, 2008). For example, they demonstrate decreased accuracy and increased reaction times compared to controls on multiplication problems typically solved via verbal fact retrieval strategy (Boets and De Smedt, 2010). Also, the characteristic ‘operation effect’ seen in typically developing children and adults (Barrouillet et al., 2008; Delazer et al., 2003), in which addition and multiplication problems are solved more quickly than subtraction and division problems, is absent in these struggling readers. Boets and De Smedt (2010) suggest that dyslexic children are unable to implement the same time-saving strategies of verbally retrieving arithmetic facts from memory that are characteristic of typically developing children.

Differences in arithmetic fact retrieval have also been found in adults with developmental dyslexia compared to typical readers (Simmons and Singleton, 2008). For example, dyslexic college students exhibit slower reaction times compared to age-matched controls in solving small addition and multiplication problems, with intact performance on subtraction and measures of basic numerical and spatial processing, including symbolic number comparison and mental rotation (Gobel and Snowling, 2010). Another study of dyslexia in young adults reported slower reaction times compared to controls when solving single-digit arithmetic problems (De Smedt and Boets, 2010). These researchers also found a significant correlation between phonological awareness skills and the frequency of reported use of retrieval strategies during arithmetic and argue that individuals with dyslexia, despite years of experience, have subtle behavioral deficits isolated to simple arithmetic processing attributed to their weaknesses in language-based skills, specifically phonological awareness.

Together, these behavioral findings demonstrate that retrieval-based arithmetic skills rely on mechanisms related to phonological awareness, and that individuals with reading disability (who typically have deficits in phonological awareness) exhibit a weakness in retrieval-based arithmetic even without meeting diagnostic criteria for math disability. These observations lead to the prediction that left hemisphere perisylvian brain regions are compromised in children with dyslexia during the solving of arithmetic problems via retrieval. While the neural basis for reading and phonological awareness has been studied extensively in dyslexia using brain imaging technology (Linkersdörfer et al., 2012; Maisog et al., 2008; Richlan et al., 2013), there have been no such investigations into arithmetic problem solving in dyslexia. Interestingly, Prado and colleagues (2011) demonstrated direct anatomical convergence for brain areas involved in multiplication of small numbers and phonological processing of single words in typical adults, suggesting a shared neural representation for this type of retrieval-based arithmetic and language processing involving the retrieval of phonological codes. These language areas seem to be underutilized by children with weaknesses in arithmetic fluency (De Smedt et al., 2011) but have not been previously studied in children with impaired reading such as in dyslexia. A better understanding of the neural representation of numerical processing in dyslexia is of theoretical importance, as it provides insights into the neural basis for dyslexia’s behavioral manifestations (which includes some weaknesses in specific aspects of arithmetic even in the absence of a formal diagnosis of math disability) and potentially provides an explanation for why a diagnosis of math disability occurs more often in children who are diagnosed with dyslexia than those who are normal readers (Barbaresi et al., 2005; Katusic et al., 2001; Lewis et al., 1994).

Here we examined the neural basis of single-digit addition and subtraction in children with pure developmental dyslexia (i.e., impaired single word reading and phonological awareness skills while maintaining normal performance on a standardized measure of calculation compared to age-matched typical readers). Based on prior work (Grabner et al., 2007, 2009; Prado et al., 2011), we expected that brain regions typically involved in the addition of small numbers, such as left angular gyrus (AG), middle temporal gyrus (MTG), and inferior frontal gyrus (IFG), would be affected in children with dyslexia during primarily retrieval-based arithmetic (addition of small numbers). On the other hand, we predicted that right hemisphere parietal regions underlying nonverbal representation would not be affected in children with dyslexia when performing primarily procedural-based arithmetic (subtraction of small numbers). We used a factorial design to test for the effects of Arithmetic Operation (addition versus subtraction), Diagnostic Group (dyslexic versus non-dyslexic), and their interactions.

2. MATERIAL AND METHODS

2.1 Subjects

The subjects were a subset of a group of children participating in a larger study on reading, reading disability, and reading development (Evans et al., 2013; Krafnick et al., 2014; Krafnick et al., 2011; Olulade et al., 2013a; Olulade et al., 2013b). Subjects with developmental dyslexia had a documented history of their reading disability, and most attended a school that specializes in the teaching of children with learning disabilities. To be included in this study, the dyslexic children had to have a standard score of less than 92 (30^th percentile) on either real word or pseudoword reading accuracy. This cut-off is consistent with prior studies (Bruck, 1992; Krafnick et al., 2014; Olulade, Flowers, et al., 2013) and ensured that we would have a continuum of skills when the dyslexics were combined with the non-dyslexics for later correlation analysis. Control subjects were recruited from within the same geographic region in the Washington DC metropolitan area and came from families with similar socio-economic backgrounds as the dyslexic group (for both groups the majority of parents had college degrees). The Control subjects scored above 92 on both real word and pseudoword reading. All participants were monolingual English speakers and in good physical health. Subjects were included only if both their verbal and performance IQ scores on the Wechsler Abbreviated Scale of Intelligence (Wechsler, 1999) were above a standard score of 80 and their performance on the Woodcock Johnson-III Calculation subtest, that includes simple fact retrieval as well as more complex mathematical functions (Woodcock et al., 2001) was above a standard score of 92. Five subjects (three controls, two dyslexics) were excluded due to excessive head motion in the scanner (see section 2.4 below), leaving 28 children (14 controls, 14 dyslexics) in the final analysis. Their behavioral data are provided in Table 1. The Georgetown University Institutional Review Board approved all experimental procedures, and informed consent was obtained from the legal guardian for all pediatric subjects, who themselves verbally assented. For their participation, the children received toy prizes and pictures of their brains from the MRI.

Table 1.

Behavioral scores for dyslexic and control children. Groups are matched for age, verbal IQ, and Calculation (Woodcock Johnson Test of Achievement III, WJ-III). Dyslexics and controls differ significantly in performance IQ, pseudoword reading (WJ-III Word Attack), real word reading (WJ-III Word ID), and phonemic awareness (LAC-3). All scores are standardized scores.

	Dyslexia n=14(7M)		Controls n=14(9M)
	Mean	SD	Mean	SD	Sig
Age	10.4	1.32	10.2	2.76	ns
Verbal IQ	112	10.8	119	14.5	ns
Performance IQ	98.9	8.70	113	11.4	*
Real Word Reading	81.9	6.81	118	11.0	*
Pseudoword Reading	95.9	5.42	113	10.7	*
Phonemic Awareness	99.4	8.50	114	11.3	*
Calculation	107	13.4	115	7.03	ns

SD = standard deviation, Sig = significance of t-test,

^*p < 0.01, ns = not significant

2.2 Neuropsychological Battery

All subjects were evaluated through standardized measures. We assessed verbal and performance IQ using the Wechsler Abbreviated Scale of Intelligence (WASI, Wechsler, 1999). The Woodcock Johnson Test of Achievement III (WJ-III, Woodcock et al., 2001) was used to assess single real word reading accuracy (Word Identification subtest), pseudoword reading accuracy (Word Attack subtest), and untimed computation (Calculation subtest). Phonemic awareness skills were measured on the Lindamood Auditory Conceptualization Test, Third Edition (Lindamood, 2004).

Controls were matched to the dyslexic children on age, verbal IQ, and the Calculation subtest of the WJ-III. A match on verbal IQ (Vocabulary and Similarities subtests) allowed us to determine that any between-group differences on the retrieval-based arithmetic task were not attributable to general differences in verbal abilities, but rather specific reading-based skills such as phonemic awareness. As expected, the children with developmental dyslexia scored significantly lower (p < 0.05) than controls on measures of single word reading, pseudoword reading, and phonemic awareness. The dyslexic sample on average had a pseudoword reading skill that was more than one standard deviation below their verbal IQ and a real word reading skill that was more than two standard deviations below their verbal IQ score. See Table 1 for details.

2.3 fMRI Task

All children performed single-digit calculation verification for both the addition and subtraction conditions. Both tasks consisted of a two-operand equation and a single-digit resultant (e.g., 2 + 3 = 5 or 7 − 4 = 3) similar to those used by Rivera et al. (2005). Subjects indicated via right or left thumb button press whether the resultant was correct or incorrect. For incorrect experimental stimuli, resultants differed from the correct answer by one (e.g., 2 + 3 = 6). See Supplementary Table A for a full list of arithmetic problems used as experimental stimuli. Addition and subtraction were presented in alternating blocks within the same experimental run, interspersed with blocks of an active control task and rest (fixation). For the active control condition, one of the operands and the resultant in each equation were replaced by pseudofont characters, and subjects indicated whether these characters, which were located on either side of the equal sign were the same (e.g. ) or different (e.g. 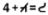). The rest (fixation) condition required subjects to fixate on a central cross hair while “not focusing on any particular train of thought and staying relaxed.” Correct and incorrect arithmetic problems (50% correct, 50% incorrect) and same and different control problems (50% same, 50% different) were randomized within each block.

Accuracy and reaction time were collected while subjects performed the tasks in the scanner using the same software that was utilized in stimulus presentation (Presentation, Neurobehavioral Systems, Albany, CA). A 2 × 2 (Arithmetic Operation x Diagnostic Group) factorial analysis of variance was conducted with Arithmetic Operation (addition and subtraction) as a within-subjects factor and Diagnostic Group (dyslexic and non-dyslexic) as a between-subjects factor. For consistency with the imaging analysis, the difference between the arithmetic task (i.e. addition and subtraction) and the active control task was calculated for accuracy and reaction time.

2.4 fMRI Acquisition

A block design paradigm was utilized, with the run consisting of one block (42 seconds in duration) dedicated to each of the two arithmetic tasks and their respective active control tasks (i.e., addition, addition control, subtraction, and subtraction control). These four blocks alternated with five 18-second blocks of rest (fixation). There were an additional 6 seconds of fixation at the beginning of the run (to allow for saturation effects) and 3 additional seconds at the end that were excluded from analysis. As such, the run was 4 minutes and 27 seconds in duration.

For each trial, stimuli were presented in black font on a white screen for 1200 milliseconds, with a 3000-millisecond interval during which a black fixation cross was presented. For the arithmetic tasks and their control conditions, this resulted in 10 trials per block for each run. With a TR of 3 seconds, this resulted in the acquisition of 14 whole-head EPI volumes for each 42-second block of addition, addition control, subtraction, and subtraction control. Subjects performed two such runs, with each run containing all condition types, and both runs were combined for the analysis, yielding 28 time points per condition. Scanning was performed using a 3.0 Tesla Siemens TIM Trio Magnetom scanner with the following acquisition parameters: TR = 3000 ms, TE = 30 ms, 64 × 64 matrix, 192 mm FOV, 50 axial slices, 3.0 × 3.0 × 2.8 mm voxels. Structural 3-D T1 MPRAGES were acquired and used to co-register the functional data.

2.5 fMRI Analysis

Using SPM8 (SPM8, Wellcome Department of Cognitive Neurology, London), we modeled the hemodynamic response function using the general linear model during performance of the arithmetic operations of (1) addition and (2) subtraction, regressing out the global mean signal and 6 motion parameters (rotational: roll, pitch, yaw; translational: x, y, z) as parameters of no interest. As a conservative measure, reaction time difference (experimental minus control task) was also included as a regressor of no interest. Only subjects with less than 3mm motion across function runs were included in analysis. Contrast maps were generated for each subject for each arithmetic operation contrasted to its active control condition (pseudofonts) and entered into a full factorial 2 (Arithmetic Operation) x 2 (Diagnostic Group) ANOVA with Arithmetic Operation (addition and subtraction) as a within-subjects factor and Diagnostic Group (dyslexic and non-dyslexic) as a between-subjects factor. F-maps were generated to test for main effects and interactions. Thresholds of p < 0.005 (height) and minimum cluster size k > 30 were implemented. For the interaction analysis, the average percent signal change was extracted from the significant cluster for each condition using MarsBar (Brett et al., 2002) to examine the direction of the response. All coordinates are reported using MNI convention. Using the SPM Anatomy Toolbox (Eickhoff et al., 2005, 2007) the location of the peak of the activations were also assigned to the most likely cytoarchitectonic area using the maximum probability map (MPM). The most relevant cytoarchitectonic maps for this study (for parietal cortex) come from Caspers et al. (2006) and Choi et al. (2006).

3. RESULTS

3.1 Behavioral Data

Measures of accuracy and reaction time for each arithmetic operation (addition and subtraction task) and for the difference between the arithmetic task and the active control task can be found in Table 2. Using the difference measures (arithmetic task minus the active control task) for accuracy, there was a significant main effect of Arithmetic Operation (F = 5.798, p < 0.05), with addition problems solved more accurately than subtraction problems. There was no significant main effect of Diagnostic Group (F = 0.248, p = 0.62) or Arithmetic Operation x Diagnostic Group interaction for accuracy. For reaction time difference, there was a significant main effect of Arithmetic Operation (F = 10.938, p < 0.05), where addition problems were solved quicker than subtraction problems. There was no significant main effect of Diagnostic Group (F = 2.478, p = 0.122) or Arithmetic Operation x Diagnostic Group interaction (F = 0.984, p 0.326).

Table 2.

Means and standard deviation for percent accuracy and reaction time in milliseconds are given for the experimental tasks of addition and subtraction, their respective control tasks, and difference scores between the two (arithmetic task minus active control task) for both the dyslexic and the control groups.

	Dyslexics		Controls
	Mean	SD	Mean	SD
Addition
Accuracy	93%	10%	93%	8.2%
Reaction Time	1909 ms	455	1598 ms	500
Control
Accuracy	97%	5%	99%	2.9%
Reaction Time	1214 ms	239	1176ms	188
Addition – Control Acc.	−4%	11%	−6%	8%
Addition – Control RT	695 ms	342	423 ms	363
Subtraction
Accuracy	78%	17%	84%	23%
Reaction Time	2158 ms	412	2103 ms	650
Control
Accuracy	96%	9%	96%	4.5%
Reaction Time	1216 ms	234	1223 ms	205
Subtraction – Control Acc.	−18%	17%	−12%	24%
Subtraction – Control RT	941 ms	319	879 ms	533

SD = standard deviation

3.2 fMRI Data

3.2.1 Main Effect of Arithmetic Operation

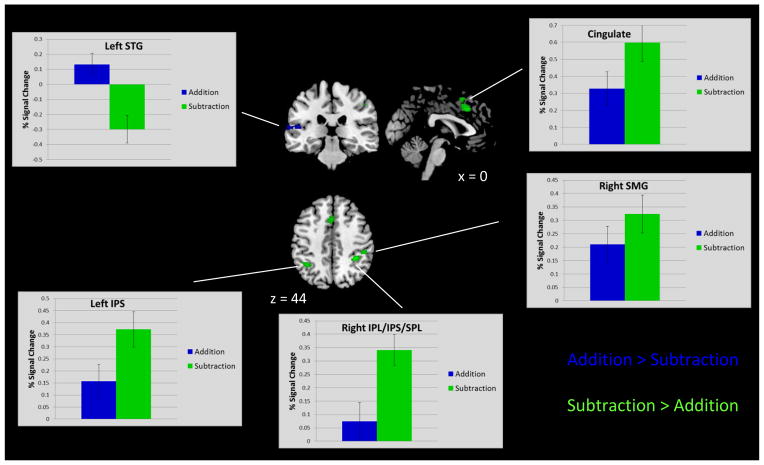
As can be seen in Table 3A and Figure 1, when both groups were combined, a main effect of Arithmetic Operation (addition versus subtraction) was found in five clusters. For one of these regions, greater activation was seen for the addition task, and this was located in the left superior temporal gyrus (OP 1/BA 42). The four other regions elicited significantly greater activity during subtraction: one in the left intraparietal sulcus (hIP1/BA 40), one in the right supramarginal gyrus of the inferior parietal lobe (PFt/BA 40), another in right inferior parietal lobe with extensions into intraparietal sulcus and superior parietal lobe (Area 2/BA 40), and the fourth in the anterior cingulate (BA 32).

Table 3.

Peak coordinates from factorial analysis of Arithmetic Operation, Diagnostic Group and Interactions. All activation peaks were assigned to the most probable brain areas as indicated by the SPM Anatomy Toolbox (Eickhoff et al., 2005, 2007).

Macroanatomical Location	Cytoanatomical Location (Probability)	Brodmann’s Area	Peak MNI Coordinates			Peak F Statistic	Volume (voxels)
			x	y	z
A. Main Effect of Arithmetic Operation
Addition > Subtraction
Left superior temporal gyrus	OP 1(40%)	42	−52	−30	12	24.03	109
Subtraction > Addition
Right inferior/superior parietal lobe, intraparietal sulcus	Area 2 (40%)	40	36	−42	46	18.73	149
Right supramarginal gyrus	PFt (40%)	40	48	−34	48	16.03	82
Anterior cingulate		32	0	22	40	14.71	117
Left intraparietal sulcus	hIP1 (40%)	40	−38	−52	46	13.56	67
B. Main Effect of Diagnostic Group
Controls > Dyslexia
Left supramarginal gyrus	PF (70%)	40	−56	−40	34	15.37	36
Dyslexia > Controls
n.s.
C. Interaction: Diagnostic Group x Arithmetic Operation
Right supramarginal gyrys	PFm (80%)	40	48	−46	52	11.48	69

Fig. 1. Main Effect of Arithmetic Operation.

Displayed is a rendering of the main effect of Arithmetic Operation from the factorial analysis (addition > subtraction in blue, subtraction > addition in green). Bar chart display the percent signal change averaged across children with dyslexia and controls in addition (blue) and subtraction (green) conditions. Detailed results can be found in Table 3A. STG = superior temporal gyrus, IPL = inferior parietal lobe, IPS = intraparietal sulcus, SPL = superior parietal lobe, SMG=supramarginal gyrus

3.2.2 Main Effect of Diagnostic Group

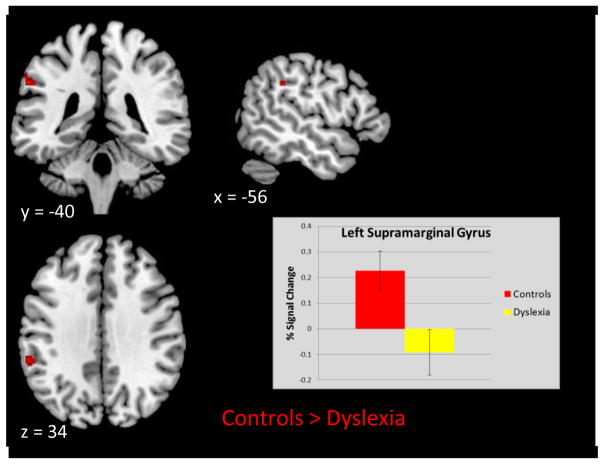
When both arithmetic operations were combined, a main effect of Diagnostic Group (dyslexics versus controls) was found in only one region, namely left supramarginal gyrus of the inferior parietal lobe, (PF/BA 40 – Table 3B). As can be seen in Figure 2, activity in this region was higher in typically reading controls compared to dyslexic children (for addition and subtraction combined).

Fig. 2. Main Effect of Diagnostic Group.

Displayed is a a rendering of the main effect of Diagnostic Group from the factorial analysis. Bar chart displays the percent signal change averaged across addition and subtraction conditions for children with dyslexia (yellow) and controls (red). Detailed results can be found in Table 3B.

3.2.3 Diagnostic Group x Arithmetic Operation Interaction

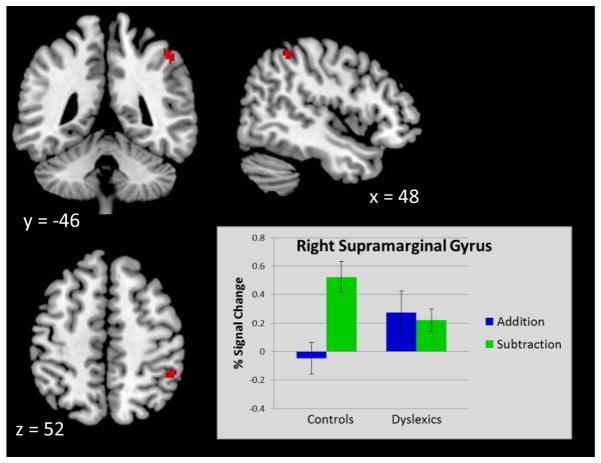
One significant cluster emerged for the interaction of Diagnostic Group by Arithmetic Operation, and it was located in the right supramarginal gyrus of the inferior parietal lobe (PFm/BA 40 – Table 3C). The average percent signal change was extracted from this cluster to determine the nature of this interaction. As can be seen in Figure 3, the controls engaged this region during subtraction but not during addition, resulting in a significant difference in the controls between these two conditions. However, the dyslexics did not show this dissociation, instead having equal activity for addition and subtraction. Therefore, a comparison between the dyslexic and control groups demonstrated less activity in this region for the dyslexics during subtraction and more activity for addition.

Fig. 3. Diagnostic Group x Arithmetic Operation Effect.

Displayed is a rendering of (Diagnostic Group x Arithmetic Operation) interaction from the factorial analysis. Bar chart displays the percent signal change in typically developing controls and in children with dyslexia for addition (blue) and subtraction (green) conditions. Detailed results can be found in Table 3C.

4. DISCUSSION

Performance on some mathematical tasks is impaired in children with dyslexia compared to non-dyslexic controls (Miles et al., 2001). This study investigated the hypothesis that retrieval-based arithmetic skills rely on left hemisphere language areas and are altered in children with dyslexia. First, we combined all children (dyslexics and controls) to examine the main effects for activity underlying two types of single-digit arithmetic: addition of small numbers, which is typically retrieval-based and is thought to rely on left hemisphere perisylvian regions, versus subtraction of small numbers, which is more procedural-based and thought to rely on bilateral parietal cortices. The results revealed, consistent with expectations, that the entire group utilized left superior temporal gyrus (BA 42) for addition and bilateral intraparietal sulcus (BA 40), right supramarginal gyrus (BA 40) and the anterior cingulate (BA 32) for subtraction. Next, for the main effect of Diagnostic Group, we found that children with dyslexia relative to controls exhibited hypoactivity in the left supramarginal gyrus (BA 40) during both arithmetic tasks combined. This result confirms our prediction of less activity in perisylvian brain areas that subserve language processes in children with dyslexia, even during arithmetic tasks. Finally, the interaction of Arithmetic Operation (addition, subtraction) and Diagnostic Group (dyslexics, controls) revealed a significant effect in the right supramarginal gyrus (BA 40). Here, controls showed greater activity for subtraction than addition, as expected. However, this differentiation was absent in the dyslexics (activity for both operations was equal). The dyslexics showed more activity here than controls for addition, and less activity than controls for subtraction, indicating an atypical and perhaps less optimal approach to solving arithmetic problems. These results are consistent with behavioral work in dyslexia which has linked weaknesses in arithmetic to language deficits (De Smedt and Boets, 2010; Simmons and Singleton, 2008). Specifically, our finding of less activity in left supramarginal gyrus in the dyslexic group during arithmetic processing provides support for this hypothesis. Further, our results are also consistent with the hypothesis that children with dyslexia may use less optimal procedural-based strategies when solving what are typically retrieval-based arithmetic tasks such as addition for small numbers (Boets and De Smedt, 2010), as evidenced by the atypical engagement of right inferior parietal cortex (SMG) for addition by the dyslexic group.

4.1 Main Effect of Activity Underlying Arithmetic Operation: Addition versus Subtraction

A main effects analysis of Arithmetic Operation identified greater activation during addition relative to subtraction in the left superior temporal gyrus (STG). Reliance on the left STG for addition is in accordance with previous studies of addition reporting activity in the left STG and neighboring regions. For example, Zhou and colleagues (2007) found that adults activated left STG during addition and multiplication. Other studies examining addition have reported task-related activity in nearby regions such as left angular and supramarginal gyri (Lee, 2000; Rosenberg-Lee et al., 2011). Grabner and colleagues (2009) identified the left angular gyrus as a locus for retrieval-based arithmetic. Our finding in the STG is consistent with these studies in that left temporal-parietal regions are known to subserve verbally mediated retrieval, including phonological processing, and are therefore candidate regions for being involved in retrieval-based arithmetic.

More activity for subtraction relative to addition was observed in bilateral inferior parietal cortices, and bilateral intraparietal sulci extending into right superior parietal lobe, in the dyslexics and controls combined. This observation is highly consistent with prior work on subtraction in typical readers, reflecting greater reliance on quantitative networks for subtraction compared to addition. For example, several studies in adults have shown activity in bilateral parietal cortices during subtraction (Chochon et al., 1999; Fehr et al., 2007; Lee, 2000), as did the only report on children, who activated bilateral parietal cortices during subtraction relative to addition in a forced choice single-digit arithmetic task (De Smedt et al., 2011). Our observation of greater reliance on the cingulate (BA 32) for subtraction is also consistent with earlier studies showing that the anterior cingulate cortex is engaged during mental arithmetic in both adults and children (Chochon et al., 1999; Davis et al., 2009; Fehr et al., 2007), reflecting engagement of executive control processes in mental arithmetic.

4.2 Main Effects of Activity Related to Diagnostic Group: Dyslexics versus Controls

The main effects analysis of Diagnostic Group revealed that the dyslexic group showed less activity during arithmetic performance (addition and subtraction combined) in the left supramarginal gyrus (BA 40). Rivera et al. (2005) identified age-related increases in the left SMG activity during a similar calculation task (addition and subtraction were combined in this study), indicating that our children with dyslexia may be exhibiting a neural profile characteristic of younger typically developing children. Our results also fit well with the recent observation that white matter integrity in left hemisphere fronto-parietal tracts (i.e. the arcuate fasciculus) is associated with children’s arithmetic competence for problems solved via fact retrieval (that is for addition and multiplication, but not subtraction; Van Beek et al., 2013). Further, this correlation disappears when decoding skills are controlled for, suggesting yet again that this relationship is driven by phonological processing abilities.

Importantly, the left SMG has been an area of long-standing interest in dyslexia. Previous work has implicated the left SMG in skilled reading (Jobard et al., 2003), and its role in reading has been attributed to phonological processing (He et al., 2013; Sliwinska et al., 2012; for review see Price, 2012). Studies contrasting dyslexics and non-dyslexic children have shown the left SMG to be hypoactive in dyslexics during reading and reading-related tasks, as illustrated by a recent meta-analysis across a number of investigations (Richlan et al., 2011). This same area also contains relatively less gray matter volume in dyslexics versus non-dyslexics (adults and children combined), as again demonstrated by a meta-analytic approach (Linkersdörfer et al., 2012; Richlan et al., 2013). Our findings demonstrate that underactivity here on the part of the dyslexics is not limited to tasks involving phonological processing but also occurs during verbally-mediated arithmetic processing. Given the key role of this region in reading and phonological processing and the dyslexics’ weakness in these areas, our results of underactivation of the left SMG during arithmetic provides further evidence for verbal representations in the “triple code model” (Dehaene, 1992; Dehaene and Cohen, 1995; Dehaene et al., 2003), and support the theory that language skills (most likely phonological awareness skills, as these are the core deficit in dyslexia) play a key role in arithmetic processing. To test this post hoc, we extracted the fMRI signal from the left SMG cluster using MarsBar (Brett et al., 2002) for both the addition and the subtraction operation and conducted a correlation analysis with these and all of the subjects’ phonemic awareness standardized scores attained using the Lindamood Auditory Conceptualization Test, Third Edition. We found a significant positive correlation for addition (p = 0.00514) but not subtraction (p = 0.3778), in support of the previously described role of phonemic awareness skills in retrieval-based arithmetic (De Smedt et al., 2010; Hecht et al. 2001). Also, the locus of the left SMG can be tied to prior studies, as it is near the angular gyrus, previously reported to be modulated in activity during arithmetic problem solving based on individual differences in ability and interpreted to indicate reliance on language-mediated processes (Grabner et al., 2007).

4.3 Interaction of Arithmetic Operation and Diagnostic Group

We expected an interaction between Diagnostic Group and Arithmetic Operation in left hemisphere language areas, specifically less activity here in the dyslexics during addition. Instead, the interaction analysis revealed an effect in right supramarginal gyrus of the inferior parietal lobe (BA 40), where the control group showed activity for subtraction but not for addition, consistent with prior reports of right parietal cortex involvement in operations such as subtraction that rely to a greater extent on procedural strategy (De Smedt et al., 2011). The dyslexic group, however, demonstrated equal activity underlying addition and subtraction in this region, relying on right IPL for both subtraction and addition thus suggesting utilization of the same quantitative strategy for both operations. This finding is consistent with behavioral work by Boets and De Smedt (Boets and De Smedt, 2010; De Smedt and Boets, 2010), who observed similar reaction times for both operations in dyslexics instead of the faster reaction time observed for addition versus subtraction seen in typical readers (Barrouillet et al., 2008; Delazer et al., 2003). They concluded that the retrieval-based strategies that are more time-efficient were not available to the dyslexics, forcing them to utilize more time-consuming quantitative means, thereby employing similar strategies across both operations. Our imaging results provide direct evidence for this hypothesis by showing equal activity in right IPL for both arithmetic operations in the dyslexics instead of reserving it for the procedural-based calculation task only, as seen in typical readers. This less efficient strategy for addition is likely fostered by limitations in retrieval-based processes in other brain regions, such as the left SMG, which revealed hypoactivity in the dyslexics during arithmetic in the main effects analysis for Diagnostic Group.

Of note is the interesting resemblance between our results and those of De Smedt et al. (2011) in their neuroimaging study of children with typical versus low levels of arithmetical fluency when solving small (e.g., 5 + 2) and large (e.g., 8 + 7) arithmetic problems. Similarly as reported here for the dyslexics, children with poor arithmetic fluency showed equal activity in right parietal cortex for large and small problems, while the children with better arithmetic fluency engage this area only during the larger (more procedurally demanding) problems, much like our controls. Our findings therefore integrate and confirm the predictions made by this earlier behavioral (Boets and De Smedt, 2010) and neuroimaging (De Smedt et al., 2011) work. They also fit well with the observation that greater activity during calculation in the left SMG, associated with arithmetic fact retrieval, is positively correlated with higher Preliminary Scholastic Aptitude Test math subtest scores in high school seniors, whereas higher activity right intraparietal sulcus, an area associated with quantity processing mechanisms, is correlated with lower scores, suggesting that right parietal activation during simple arithmetic is associated with non-optimal math development (Price et al., 2013).

Of note is also the lower activity in the dyslexic children in the right IPL during subtraction relative to controls. This pattern is similar to the profile seen in children with math disability (MD) described by Ashkenazi et al. (2012), who identified reduced activity in MD children solving complex arithmetic problems in right superior and inferior parietal lobes. This result suggests that children with dyslexia also exhibit atypical activity in brain regions outside of the language network which is worthy of further investigation.

4.4 Implications for Dyslexia

This work is consistent with the theory that while showing no measurable deficits on standardized measures of mathematics, children with dyslexia may exhibit subtle impairments in arithmetic skills. Here we show that arithmetic takes on a different neural profile in dyslexia compared to non-dyslexics in left hemisphere language areas, as well as right hemisphere quantity computational areas. This raises the question whether interventions in phonological awareness that have been shown to be effective in improving reading skills in dyslexic children transfer to improvements in retrieval-based arithmetic. It is worth noting that the dyslexic children in our study were not severely impaired on phonological awareness and decoding as is typically reported for dyslexia (even though their performance was significantly lower than that of the controls), raising the possibility that a more impaired population would show even more pronounced differences for the neural bases of retrieval-based arithmetic than those reported here.

4.5 Implications for Math Disability (Dyscalculia)

While the study was conducted in children who would not meet criteria for MD, our results might provide insight into why children with dyslexia are more prone to MD than non-dyslexic children. Children with MD (also referred to as developmental dyscalculia) show a core deficit in comprehending numerosities (for review, see Butterworth et al., 2011) and have impairments in basic number processing tasks (Landerl et al., 2004). Neuroimaging research indicates that children with dyscalculia show less activation than typically developing controls in bilateral intraparietal sulci during tasks of magnitude comparison (Mussolin et al., 2010; Price et al., 2007) and approximate calculation (Kucian et al., 2006). However, no group differences emerge during exact calculation of simple addition problems (Kucian et al., 2006). Anatomical studies have shown reduced gray matter volume in bilateral intraparietal sulcus in dyscalculia (Isaacs et al., 2001; Rotzer et al., 2008; Rykhlevskaia et al., 2009). A topic of much debate has been whether the math disability seen in children with reading disability (MD+RD combined) is the same as that seen in non-dyslexic children or if it is different, possibly driven by the same issues that cause their reading problems. As recently described by Willcutt et al. (2013), there are several theories that could explain combined reading and math disability (MD+RD): (i) a disorder that is completely independent of MD and RD alone; (ii) a disorder that shares the etiology for reading problems with RD alone, but the math difficulties are a consequence of the reading problems and not the same as the number sense deficits seen in MD alone; (iii) a partially shared etiology yet at the same time a separate etiology that accounts for the different manifestations; or (iv) a mostly shared etiology for MD, RD, and MD+RD, but the three can present as alternate manifestations (leading to differential diagnosis) despite generally similar profiles. Willcutt et al. (2013) favors this last model based on behavioral studies of MD+RD, consistent with the position of Branum Martin et al. (2013), who found via modeling that profiles of children in all three categories are similar and that they do not represent distinct groups, even though they can be made to appear as such when using cut-offs. They propose that a dimensional approach must be used when considering these disabilities. Consistent with this position are the findings described in the introduction that children and adults with RD, despite being categorized as “normal” on math using standardized tests, display significant problems in specific aspects of math (Boets and DeSmedt., 2010; DeSmedt and Boets, 2010; Gobel and Snowling, 2010). This is further supported by our results showing that in dyslexia, there are alterations in brain systems that subserve arithmetic even in the absence of a diagnosis of MD. Neurobiological models for MD+RD have been discussed (Ashkenazi et al., 2013), and future studies employing functional brain imaging in children with MD+RD will be able to address this and other hypotheses.

5. Conclusion

In sum, this study provides a neural account for the behavioral deficits observed during arithmetic in children with reading disability, even if they do not manifest in significant and clinically meaningful impairment in calculation as determined by commonly used achievement tests. Children with dyslexia demonstrated underactivation the left supramarginal gyrus during addition and subtraction problem solving, and they showed a lack of differentiation of arithmetic operation in the right inferior parietal lobe compared to their typically reading peers. This work has implications for remediation strategies for mathematics in dyslexic students and potential insights into math disability in children with reading disability.

Highlights.

• First neuroimaging study of arithmetic in individuals with dyslexia.

• Less activity in dyslexics during arithmetic processing in left supramarginal gyrus.

• Lack of modulation by operation in right supramarginal gyrus in children with dyslexia.

• Deficits in retrieval-based arithmetic in addition in dyslexia accompanied atypical brain function.