High-Risk Pools for Uninsurable Individuals: Recent Growth, Future Prospects

Abstract

High-risk pools are State programs that were recently brought under Federal review by the Health Insurance Portability and Accountability Act of 1996 (HIPAA). For a subsidized, yet above-standard premium, they provide coverage to individuals lacking access to private health insurance, typically due to pre-existing conditions. Reducing high-risk pool premiums in all States to the level prevailing in the most generous States (at an annual cost of about $105 million) could lead to a modest but significant increase in enrollment, relative to the uninsurable population. In addition, non-premium changes, for example to benefits and marketing, could also have substantial effects on enrollment.

Introduction

The recent economic downturn has reawakened national concern about the problem of the uninsured. While the proportion of the population without health insurance decreased from 1998 (16.3 percent) to 2000 (14 percent) (U.S. Bureau of the Census, 2000; 2001), the recent economic recession and associated loss of jobs has exacerbated the problem. Between March and November of 2001, nearly one million individuals lost their jobs and their health care coverage; one-half of these losses occurred after the terrorist attacks of September 11, 2001 (Families USA, 2001). The increase in the number of uninsured in 2001 was the largest one-year increase in nearly a decade with 2.2 million losing coverage (Families USA, 2002). An additional 2.4 million people lost coverage in 2002 (Mills and Bhandari, 2003). With higher unemployment and lower consumer spending, State tax revenues are down and budget deficits have reappeared. Consequently, Medicaid budgets are tight and some States have cut optional populations from their programs to reduce expenditures (Simon, 2002; Sloane, 2003).

Among the growing number of uninsured is a class of individuals most in need of insurance: the uninsurable. These are individuals with potentially costly health conditions who pose a high risk to any insurance carrier and who cannot obtain health care coverage due to medical underwriting.¹ In 29 States (as of July 2002), these high-risk, uninsurable individuals are eligible for coverage under special State programs known as comprehensive health insurance plans for high-risk individuals (high-risk pools). All high-risk pools offer coverage for a subsidized premium that is, nevertheless, above standard rates. Though operations vary by State, subsidy financing is generally provided by assessments on private carriers, general State revenue, other public sources (e.g., tobacco-settlement funds), or a combination of these.

High-risk pools have quietly become an important component in the Nation's public/private patchwork of health care coverage. The number of high-risk pools has been gradually growing along with enrollment since the first pools began operation in Connecticut and Minnesota in 1976. Recently, the 1996 HIPAA has encouraged this growth by requiring States to guarantee health insurance portability (i.e., that health insurance is available) to individuals who meet certain requirements. High-risk pools have been designated as one of the State Alternative Mechanisms (SAMs) for compliance with the portability provisions of HIPAA, although the act does not endorse high-risk pools above all other alternatives. Moreover, HIPAA established a Federal regulatory role over States that do not select an acceptable portability mechanism. CMS is responsible for undertaking periodic reviews to determine which States have SAMs that are in compliance with HIPAA. In addition, CMS directly exercises Federal authority in States that are not in compliance. Since this Federal authority overrides what would otherwise be a State prerogative, HIPAA creates an incentive for States to select an alternative mechanism, thereby strengthening the position of high-risk pool advocates.

In this article, we combine high-risk pool operational data with State demographic and health insurance data to investigate the historical growth in high-risk pools and the affordability of high-risk pool premiums. We also study the potential for enrollment growth if the premium subsidies were increased.

Data

We constructed a database consisting of high-risk pool operational data linked to State demographic data. High-risk pool operational data (number of enrollees, actual premiums charged, statutory premium caps, and other financial and benefits data) for the years 1981-2000 were obtained from Communicating for Agriculture (CFA) (1995-2001/2002).

Most State demographic measures (State population, number of uninsured, number of uninsurable, income statistics) were obtained or constructed from the Current Population Survey March Supplement (CPS) (U.S. Bureau of the Census, 1995-2001). We chose the CPS because the data are relatively current, they are comprehensive on demographics and income, and reasonably so on health insurance. Additionally, by using the standard technique of pooling 3 years of data, we were able to obtain adequate sample sizes for annual State-level descriptive analysis from CPS data.² Because high-risk pools serve uninsurable individuals, we needed a measure of the number of uninsurable persons in each State. Unfortunately, we are aware of no broadly accepted statistics on this topic, so we developed an approximation, defining the uninsurable population for each State as individuals who were uninsured and who either could not work, were limited in the type of work they could do, or received any disability or worker's compensation income.³ The remaining data items, namely per capita Medicare expenditures, were obtained from the Statistical Abstract of the United States (U.S. Bureau of the Census, 1995-2001).

The resulting data set consists of 335 observations, each representing a high-risk pool in a single State for a single year during the period from 1981-2000. All of these observations include CFA operational data and the 188 State-year observations corresponding to the years 1995-2000 also include statistical abstract and CPS data.

Descriptive Results

Pool Growth

Three high-risk pools were operating by 1981. The Connecticut and Minnesota pools opened in 1976 and Wisconsin's began operation in 1981. From 1981 to 2000, the number of States with high-risk pools increased nearly every year (Table 1). The only year in which the number of pools decreased is 1995, when Tennessee folded its high-risk pool into TennCare.⁴ Table 1 also shows the number of States that use a high-risk pool as the State alternative mechanism to satisfy the portability requirements of HIPAA. Since the passage of HIPAA in 1996, most States with high-risk pools began offering pool coverage to HIPAA-eligible individuals to satisfy the new portability requirements. Two States created high-risk pools specifically in reaction to the passage of HIPAA (Alabama and Texas) and several new pools have opened more recently (Kentucky, New Hampshire, and Maryland, all too new to be included in this study). Only 4 of the 27 pools operating in 2000 were not HIPAA pools—California, Florida, Missouri, and Washington State.

Table 1. Number of Enrollees and High-Risk Pools: 1981-2000.

Year	Total Number of Enrollees	Number of Pools	Number of HIPAA Pools
1981	6,668	3	0
1982	9,199	5	0
1983	15,448	6	0
1984	19,602	6	0
1985	21,536	6	0
1986	21,833	7	0
1987	24,231	10	0
1988	33,301	12	0
1989	53,458	13	0
1990	68,263	15	0
1991	77,683	17	0
1992	96,245	22	0
1993	101,623	24	0
1994	95,536	24	0
1995	90,405	23	0
1996	86,723	25	0
1997	86,555	25	11
1998	92,101	27	22
1999	104,918	27	22
2000	115,688	27	23

NOTE: HIPAA is Health Insurance Portability and Accountability Act of 1996. SOURCE: Communicating for Agriculture, Inc.: Comprehensive Health Insurance for High-Risk Individuals (9th-15th editions). Fargo Falls, MN. 2001/2002.

Corresponding to the growth in the number of high-risk pools, Table 1 shows nearly steady growth in the number of pool enrollees. The only period of decline was from 1994-1997. During this period, Tennessee folded its pool into TennCare, which accounts for part of the decrease in 1995. The period of declining enrollment also immediately follows or coincides with the passage of small- and non-group insurance reforms in many States (U.S. General Accounting Office, 1995). These reforms may have been associated with reduced financial support for high-risk pools, given the expectation at the time that insurance reform would reduce the need for pool coverage.

The number of individuals with high-risk pool coverage is very small relative to the number of uninsured, as shown in Table 2. However, this number is larger, and in some States substantial, relative to the numbers of medically uninsurable (the target population for the pools).⁵ Presumably due to its low premiums, Minnesota's pool is the largest in absolute terms (with 25,892 covered in 2000) and relative to the State's uninsured and uninsurable populations (covering 6 and 54 percent, respectively). The figures for Minnesota are far above the national averages; nationally, high-risk pool enrollment is 0.5 percent of the total uninsured population and 8 percent of the uninsurable population.

Table 2. High-Risk Pool Enrollees, by State: 2000.

State	High-Risk Pool Enrollees
	Total	Relative to Number Uninsured	Relative to Number Uninsurable
		Percent
Total	115,688	0.45	8
Alabama	2,431	0.37	5
Alaska	395	0.33	4
Arkansas	2,270	0.55	7
California	17,343	0.25	6
Colorado	1,536	0.25	5
Connecticut	1,719	0.51	8
Florida	709	0.03	1
Illinois	10,120	0.58	10
Indiana	6,475	0.89	11
Iowa	271	0.11	2
Kansas	1,283	0.43	6
Louisiana	1,088	0.13	2
Minnesota	25,892	6.14	54
Mississippi	2,231	0.49	7
Missouri	889	0.16	3
Montana	1,687	0.99	12
Nebraska	5,023	3.03	35
New Mexico	1,063	0.25	5
North Dakota	1,307	1.68	18
Oklahoma	1,922	0.32	3
Oregon	5,833	1.22	21
South Carolina	1,451	0.25	3
Texas	8,600	0.18	4
Utah	1,106	0.37	5
Washington	2,333	0.29	4
Wisconsin	10,042	1.90	21
Wyoming	669	0.87	11

SOURCES: Communicating for Agriculture, Inc.: Comprehensive Health Insurance for High-Risk Individuals (9th-15th editions). Fargo Falls, MN. 2001/2002. U.S. Census Bureau, Current Population Survey (1999-2001).

Barriers to Enrollment

Of all the possible barriers to high-risk pool enrollment, two stand out as the most significant: enrollment caps or freezes and high premiums.⁶ California has an enrollment cap and only sells as many policies as it can finance with revenue from a tobacco tax (17,343 in 2000). There is a waiting list of about 4,000 individuals, each expected to wait about a year before being permitted to enroll in California's pool. Florida has an enrollment freeze. In a political settlement with the insurance industry (which protested the size of assessments for pool subsidy funding), Florida's pool has been closed to new enrollment since 1990 and enrollment has declined from a high of 7,500 in that year to 709 in 2000. A pool with an enrollment cap or freeze on HIPAA eligible individuals does not comply with HIPAA regulations, so the pools in Florida and California are not HIPAA pools and could not be unless changes were made to enrollment policy. Note, however, that to comply with HIPAA, a State cannot impose a cap on HIPAA eligibles, but may impose one on enrollees eligible for other reasons (e.g., Louisiana and Illinois).

The most pervasive barrier to enrollment is affordability. In all States, high-risk pool premiums, while subsidized, are above standard rates. Only a small number of States offer additional subsidies for low-income individuals (Wisconsin, Connecticut, New Mexico, Oregon, and Colorado). Consequently, for most people and in most States, high-risk pool premiums are above the already high non-group market rates, rendering high-risk pool coverage unaffordable for many who cannot obtain coverage in any other way.

To provide a sense of the financial burden imposed by pool premiums, Table 3 lists the percents of all individuals, the uninsured, and the uninsurable, for whom the pool premium⁷ is greater than 25 percent and for whom it is less than 10 percent of family income. Although there is no standard of affordability, the 25 and 10 percent thresholds are intended to serve as rough guides. Table 3 shows, for example, that nationally, high-risk pool premiums are above 25 percent of family income (i.e., are unaffordable) for 10 percent of all individuals, 18 percent of the uninsured, and 29 percent of the uninsurable. By these standards, almost one-third of the uninsurable are unable to afford high-risk pool coverage, although there are large variations by State with Minnesota's premiums being the most affordable to its population and Kansas' among the least. Note that only 22 of the 27 high-risk pool States are listed in Table 3 because premium data were not available for five States (Connecticut, Florida, Indiana, Louisiana, and Nebraska).

Table 3. Percent of Population for Whom Premium was Either Less Than 10 Percent or More Than 25 Percent of Family Income, by Selected States: 2000.

State	Premium1	All Individuals		Uninsured		Uninsurable
		>25%	<10%	>25%	<10%	>25%	<10%
Alabama	$192	9	72	18	54	31	36
Alaska	400	14	57	26	35	36	25
Arkansas	153	6	77	11	61	14	39
California	280	12	62	19	42	30	36
Colorado	214	6	79	12	56	22	50
Illinois	292	11	67	20	46	33	28
Iowa	273	9	65	18	35	27	27
Kansas	382	18	50	37	24	51	24
Minnesota	128	2	91	6	82	6	86
Mississippi	215	11	65	18	46	33	46
Missouri	267	10	69	16	49	21	50
Montana	252	14	58	26	36	34	24
New Mexico	202	10	66	16	49	23	48
North Dakota	223	9	65	15	46	26	38
Oklahoma	224	10	67	15	50	25	40
Oregon	232	10	69	20	45	28	26
South Carolina	268	10	63	20	45	35	29
Texas	237	10	67	17	47	24	38
Utah	272	7	73	16	52	25	44
Washington	266	10	69	20	49	28	41
Wisconsin	196	5	79	16	57	22	55
Wyoming	179	6	79	13	63	20	57
All of the Above States	—	10	67	18	46	29	37

¹Premium in 2001 dollars for a 35-year old, non-smoking male at the lowest deductible, and no optional features as reported by the States to Communicating for Agriculture, Inc. Includes 22 States with high-risk pools and available premium data.

SOURCES: Communicating for Agriculture, Inc.: Comprehensive Health Insurance for High-Risk Individuals (9th-15th editions). Fargo Falls, MN. 2001/2002. U.S. Census Bureau, Current Population Survey (1999-2001).

Simulating the Implications of Lower Premiums

Given that affordability is a significant barrier to enrollment, it seems likely that lowering premiums would raise enrollment. To evaluate how much enrollment could grow if premiums were reduced, we conducted a simulation of the impact of lowering all premiums to the level seen in the most generous States.⁸

To conduct the simulation, we first estimated the elasticity of enrollment with respect to premiums using regression methods. The log of enrollment was modeled as a function of the log of high-risk pool premium, the level of benefits, the size and income of the State's uninsured population, and the year. The unit of observation was the State/year. Note that we use measures of the uninsured population as opposed to the uninsurable population because the former is clearly identifiable in the CPS data while the latter is less so due to previously discussed limitations of CPS data.

We began with the specification

$$\text{log}({\text{enrollment}}_{s,t})=\alpha +{\beta }_1\text{log}({\text{premium}}_{s,t})+{\beta }_2{\text{lowest deductible}}_{s,t}+{\beta }_3{\text{multiple deductibles}}_{s,t}+{\beta }_4\text{log}({\text{uninsured population}}_{s,t})+{\beta }_5\text{log}({\text{per capita income of uninsured}}_{s,t})+{\beta }_6{\text{year}}_t+{\varepsilon }_{s,t}$$ (1)

where the subscript s indicates State, the subscript t indicates year and all the variables are as defined in Table 4.⁹ Refer to Technical Note A for the theoretical model that underlies equation 1. Because the actual high-risk pool premium was not available for enough States and years to permit the estimation of equation 1, we used a proxy defined as

Table 4. Definition of Variables.

Variable1	Definition/Comment	Mean	Standard Deviation	Minimum	Maximum
log(enrollments,t)	Log of high-risk pool enrollment.	7.33	1.10	5.16	10.32
log(premiums,t)	Log of high-risk pool premium. Only available for 58 observations.	5.39	0.31	4.75	6.05
log(proxy premiums,t)2	Proxy for high-risk pool premimum.	8.89	0.27	8.42	9.68
pct. of market premiums,t	High-risk pool premium as percent of market premium.	1.52	0.23	1.18	2
per capita Medicare expenditures,t	Used as proxy for market premium.	4,912	924.00	3,139	8,002
lowest deductibles,t	Controls for generosity of benefits.3	543.8	235.10	0	1,000
multiple deductibless,t	Binary variable; controls for generosity of benefits.3	0.85	0.35	0	1
log(uninsured populations,t)	Controls for demand.	12.81	0.78	10.87	14.43
log(per capita family income of uninsureds,t)	Controls for demand.	10.46	0.18	10.08	10.86
yeart	Controls for trends in enrollment and premiums	1997.58	1.70	1995	2,000
lag log(proxy premiums,t) 2	Used as an additional instrumental variable for log(proxy premiums,t).	8.86	0.27	8.2	9.68
lag pct. of market premiums,t2	Used as an additional instrumental variable for log(proxy premiums,t).	1.52	0.22	1.25	2
lag per capita Medicare expenditures,t2	Used as an additional instrumental variable for log(proxy premiums,t).	4,775	992.00	2,425	8,002

¹Subscript s indexes States (Alabama, Alaska, Arkansas, Colorado, Connecticut, Illinois, Indiana, Iowa, Kansas, Louisiana, Minnesota, Mississippi, Missouri, Montana, Nebraska, New Mexico, North Dakota, Oklahoma, Oregon, South Carolina, Utah, Washington, Wisconsin, and Wyoming). Subscript t indexes years (1995-2000). Not every State has an observation in every year. Three States are excluded: California (enrollment is capped), Florida (pool is closed), and Texas (not in equilibrium).

²As described in the text, to remove endogeneity, we instrumented for log(proxy premium_{s, t}). The instruments were lag log(proxy premium_{s, t}), lag pct. of market premium_{s, t}, and lag per capita Medicare expenditure_s,t.

³Benefits vary by State and year and were not consistently provided in our data sources. Deductible levels, however, were consistently provided and serve as a measure of plan generosity.

NOTE: N=137, except where indicated.

SOURCES: Communicating for Agriculture, Inc.: Comprehensive Health Insurance for High-Risk Individuals (9th-15th editions). Fargo Falls, MN. 2001/2002. U.S. Census Bureau, Current Population Survey (1999-2001).

$${\text{proxy premium}}_{s,t}=({\text{pct}.\text{of market premium}}_{s,t})\times ({\text{per capita Medicare expediture},}_{s,t})$$ (2)

where per capita Medicare expenditure serves as a proxy for State-to-State/year-to-year variation in the actual market premium. The percent of market premium variable was established through interviews with State high-risk pool administrators and is often, but not always, set at the statutory maximum. In cases where administrators could not supply the percent of market premium, we used the statutory maximum.

One problem with this specification is that plan administrators might adjust premium levels in reaction to unexpected enrollment changes. In particular, if enrollment is higher than budgeted, plan administrators might raise premiums in an effort to keep enrollment and losses in line with legislative expectations. Thus, to the extent that enrollment changes might cause premium changes, the premium variable in equation 1 is endogenous. To address this endogeneity, we estimate equation 1 by instrumental variables using lagged values of the log of proxy premium, the percent of market premium, and per capita Medicare expenditure as instruments for the log of proxy premium.

Table 5 provides the estimated coefficients for equation 1 using the proxy premium of equation 2 and instrumental variables as described. Our estimate of elasticity of enrollment with respect to premium for this specification is -1.9, which is the value used in the simulation that follows. A variety of other specifications were studied and comparable results were obtained.

Table 5. Estimation Results (Dependent Variable Log [enrollment_s,t])¹.

Variable	Coefficient (Standard Error)
log(proxy premiums,t)	***-1.90 (0.41)
lowest deductibles,t	0.000091 (0.0004)
multiple deductibless,t	-0.23 (0.25)
log(uninsured populations,t)	**0.77 (0.12)
log(per capita family income of uninsureds,t)	*1.03 (0.52)
yeart	0.67 (0.051)
constant	-129 (100)

¹To remove endogeneity, we instrumented for log (proxy premium_{s, t}). The instruments were lag log(proxy premium_{s, t}), lag pct. of market premium_{s, t}, and lag per capita Medicare expenditure_{s, t}. Three States are excluded: California (enrollment is capped), Florida (pool is closed), and Texas (not in equilibrium).

^*Significant at the 5.0 percent level.

^**Significant at the 0.1 percent level.

NOTE: N = 137, R² = 0.25.

SOURCES: Communicating for Agriculture, Inc.: Comprehensive Health Insurance for High-Risk Individuals (9th-15th editions). Fargo Falls, MN. 2001/2002. U.S. Census Bureau, Current Population Survey (1999-2001). U.S. Census Bureau, Statistical Abstract of the United States (1995-2001).

Other researchers have also consistently found that individual insurance purchase responds to price, although magnitudes vary according to the population studied and the source of price variation (Chernew, Frick, and McLaughlin, 1997; Gruber and Poterba, 1994; Ku and Coughlin, 2000; Marquis and Long, 1995; and Stearns and Mroz, 1996). In general, our elasticity is larger in magnitude as compared with those in the literature, which are typically below one in absolute value. However, the market for high-risk pools is unique in that potential enrollees are known to have higher expected health care utilization than the general public as well as being older, having lower incomes, and being less likely to be working. Moreover, high-risk pool enrollees typically pay the entire premium, in contrast to individuals with employer-based group insurance. So, it is reasonable to expect an elasticity larger in magnitude. In a study of disenrollment from eight States' high-risk pools, Stearns and Mroz (1995/1996) observe that severalfold increases in disenrollment rates occurred at the time of selected premium increases, though the degree of response varied across States. Their results are consistent with an elasticity the magnitude of the one we estimate (Technical Note B).

As Table 6 shows, our preferred elasticity estimate of -1.9 implies that if premiums were set to no higher than 125 percent of market rate, enrollment would grow by 33 percent, nationally, reaching 11 percent of the uninsurable (up from 8 percent in 2000—Table 2). Enrollment growth varies by State, depending on how far current pool premiums are from 125 percent of market rate (Technical Note C).

Table 6. Predicted Effect of Reduction in Premiums to 125 Percent of Market Rate, by State: 2000.

State	Premium as Percent of Market	Year 2000 Enrollment	Simulated Enrollment1	Simulated Enrollment Relative to
				Actual Enrollment	Uninsured	Uninsurable
				Percent
Total		115,688	153,666	133	1	11
State
Alabama	175	2,431	4,612	190	1	10
Alaska	200	395	966	245	1	11
Arkansas	150	2,270	3,212	141	1	9
California2,3	125	17,343	17,343	100	0	6
Colorado2	118	1,536	1,536	100	0	5
Connecticut	150	1,719	2,432	141	1	12
Florida3	250	709	709	100	0	1
Illinois	150	10,120	14,318	141	1	14
Indiana	150	6,475	9,161	141	1	16
Iowa	150	271	383	141	0	3
Kansas	150	1,283	1,815	141	1	8
Louisiana	200	1,088	2,662	245	0	5
Minnesota2	125	25,892	25,892	100	6	54
Mississippi	175	2,231	4,233	190	1	13
Missouri	200	889	2,175	245	0	6
Montana	150	1,687	2,387	141	1	16
Nebraska	135	5,023	5,815	116	4	41
New Mexico2	125	1,063	1,063	100	0	5
North Dakota	135	1,307	1,513	116	2	21
Oklahoma	140	1,922	2,385	124	0	4
Oregon2	125	5,833	5,833	100	1	21
South Carolina	200	1,451	3,550	245	1	7
Texas3	165	8,600	8,600	100	0	4
Utah	150	1,106	1,565	141	1	7
Washington	150	2,333	3,301	141	0	6
Wisconsin	200	10,042	24,567	245	5	51
Wyoming	200	669	1,637	245	2	27

¹Enrollment simulated using Δ log(enrollment) = (-1.9) Δ log(premium).

²Premiums in these States are already at or below 125 percent of market rates. Therefore, we did not simulate a change in premium in these States, and thus, there is no change in enrollment.

³Three States are excluded: California (enrollment is capped), Florida (pool is closed), and Texas (not in equilibrium).

SOURCES: Communicating for Agriculture, Inc.: Comprehensive Health Insurance for High-Risk Individuals (9th-15th editions). Fargo Falls, MN. 2001/2002. U.S. Bureau of the Census: Statistical Abstract of the United States, 1995-2001. Internet address: http://www.census.gov/prod/www/statistical-abstract-us.html (Accessed 2004.) U.S. Census Bureau, Current Population Survey (1999-2001).

Using premium, claims, and assessment funding figures from CFA, we can calculate a simple approximation of the cost of subsidizing all premiums to 125 percent of market rates. The increase in cost has two components. The first is the change in enrollment multiplied by the difference between the average claim per person and the new premium. The second is the number of current enrollees multiplied by the change in premium.¹⁰

An order of magnitude estimate of the annual additional cost of reducing premiums to 125 percent of market rate is about $105 million nationally, or about $2,800 per new enrollee per year (Appendix D). This figure assumes no change in the level of assessment funding currently provided in each State and does not include administrative costs. We acknowledge that a major challenge facing any policymaker wishing to finance high-risk pool expansion is how to do it without displacing current funding (a variant of the crowd-out problem¹¹); however, our purpose here is only to develop a first approximation of what might be possible, postponing such implementation issues.

One additional lesson emerges from this simulation. As Table 6 indicates, even when premiums are fixed at approximately the same level relative to the market, States differ dramatically with respect to the proportion of the uninsurable that would be covered. Six States are projected to cover between 20 and 55 percent, eight States are between 10 and 19 percent, and the remaining States are in the low, single digits. These results underscore the fact that considerations other than premium levels have substantial effects on enrollment. Some of these factors could be circumstantial, such as the relative availability of charity care, and some could reflect characteristics of the high-risk pools, such as the extent of marketing and the attractiveness of benefits.

Although this simulation was intended only as a rough approximation, several cautions still apply. First, the proxy premium used in our preferred specification implicitly assumes that per capita Medicare spending is closely correlated with market rates for individual insurance policies. We acknowledge that this assumption is impossible to verify; nevertheless, some support can be drawn from the fact that elasticity estimates were similar across specifications using actual premiums and proxy premiums. Second, we acknowledge that benefits influence enrollment, but we were only able to include a few covariates to control for differences in benefits due to limitations of sample size and inconsistent reporting of benefits across States and years. The fact that specifications including State-fixed effects produced similar elasticity estimates partially mitigates this concern, provided the most important differences in benefits between States were stable through time. Finally, our definition of uninsurable is by necessity somewhat arbitrary. It is likely that a different definition would produce different results, particularly if the chosen definition included substantially more individuals.

Policy Discussion

Other than encouraging the establishment of high-risk pools, the influence of Federal regulation on access to those pools has been modest to date. Of the two chief barriers to access, enrollment caps or freezes and affordability, HIPAA only addresses the first one, establishing that a high-risk pool must not impose restrictions on the number of HIPAA eligible enrollees in order to be an acceptable portability mechanism (25 of the 27 high-risk pools in operation in 2000 satisfy this criterion, though only 23 are HIPAA pools).

Most States do not provide additional premium subsidization for low-income pool applicants. Therefore, for much of the high-risk target population (the medically uninsurable) high-risk pool coverage is unaffordable. Federal regulation regarding the degree of affordability of high-risk pools could encourage additional enrollment and lead to an increase in coverage for the uninsurable population.

Of course, the benefits of lower premiums come at a cost. For about $105 million in additional premium subsidization, high-risk pool enrollment could be expected to grow by about 33 percent and increase coverage of the uninsurable population from 8 to 11 percent. While this increase may be modest, this is a population most in need of coverage and likely to rely on substantial amounts of high-cost emergency care if uninsured.

The fact that substantial projected enrollment variation remains among States after adjusting for premium differences suggests that significant enrollment growth could be encouraged even without additional premium subsidies. In the course of their regular reviews of State alternative mechanisms under HIPAA, it would be reasonable for regulators to focus their attention on the operations of pools with relatively low enrollment, controlling for premium. In addition to improving understanding of the factors that explain enrollment variations, it is possible that such a ranking by itself would serve as an effective incentive for State policymakers and pool administrators to seek to minimize barriers to access.

For the purposes of this discussion, we have sidestepped several challenging issues associated with an increase in the Federal role. Federal regulation involves questions of Federal versus State authority, funding for the Federal activity, information requirements for monitoring, among other things. Moreover, to be effective, regulators must have strategies to prevent unintended consequences such as the use of Federal money to underwrite current costs, rather than expand coverage. Thus, the results of this article should be interpreted as an example of what is possible under ideal circumstances in which these other issues are resolved.

Given the prevailing fiscal climate and the political challenge of simply maintaining the current level of high-risk pool funding, additional funding is unlikely to come from State sources. Federal action, therefore, appears to be the most feasible instrument of expansion in the near future. This study shows that, building on the foundation established by HIPAA, the combination of new Federal funding and Federal affordability and enrollment guidelines could significantly expand access to health insurance for those currently unable to acquire it.

Epilogue

In December 2003, the U.S. Department of Health and Human Services announced the first round of grants to States with high-risk pools (about $30 million to 16 States). The grants, authorized in the Trade Adjustment Assistance Reform Act of 2002, are available only to States with risk-pool premiums no higher than 150 percent of the market rate. States satisfying this and other criteria can apply for a grant totaling up to 50 percent of the losses incurred in risk-pool operation. While it is too soon to tell what effect the grants will have on high-risk pool enrollment, their intent is to encourage the expansion of access to high-risk pool coverage through reduction in premiums (U.S. Health and Human Services 2003).

Technical Note A

Theoretical Model

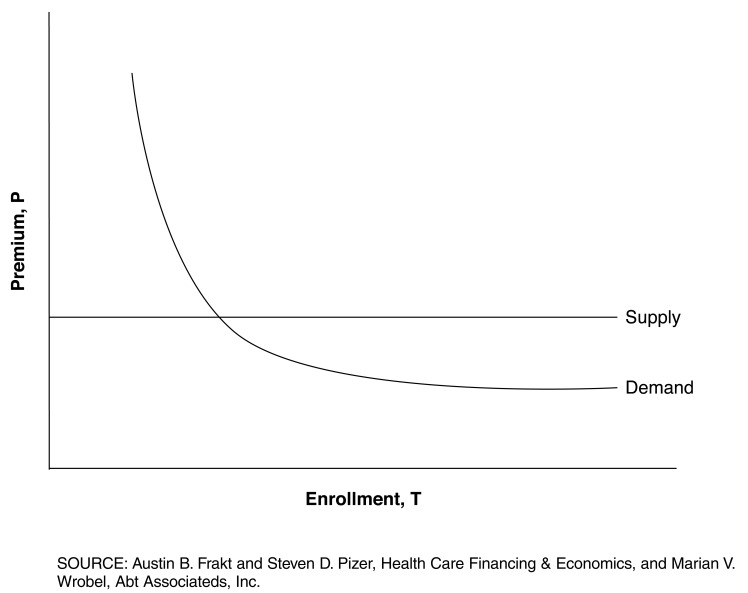
The theoretical model that underlies the empirical work presented in this article begins with the assumption that premiums and benefits are set by high-risk pools prior to enrollment decisions. That is, individuals choose to enroll with full knowledge of the benefits package and cost sharing. We also assume that the elasticity of supply is infinite, i.e., that supply will meet demand, and that elasticity of demand ε_T, is constant. The infinite supply elasticity assumption justifies the exclusion of pools that impose constraints on enrollment (California and Florida) from our estimation of elasticity of demand. Finally, we assume that total enrollment is a complete measure of demand, i.e., that everyone who wishes to enroll does so. This justifies the exclusion of pools that are too new to have signed up everyone who wishes to enroll (Texas).

Figure 1 illustrates some of our assumptions. The horizontal axis is total enrollment, T, and the vertical axis is premium, P. Since the elasticity of supply is infinite, the supply curve is a horizontal line. Thus, the point of equilibrium, where demand meets supply, is uniquely determined by the demand curve and demand is measured as total enrollment, T.

Figure 1. Theoretical Model.

The equation governing the demand curve takes the form

$$\mathrm{T}=\mathrm{T}(\mathrm{P},\text{generosity of benefits},\text{uninsured pop}.,\text{inc}.\text{of uninsured},\text{year})$$ (Eq. A.1)

Demand, T, is a function of premium, P with an elasticity ε_T, which is expected to be negative. All other things being equal, demand should increase with the generosity of benefits. Demand should be higher in State-years with larger uninsured populations since there would be more individuals in need of high-risk pool coverage. Demand should be higher if the population in need of high-risk pool coverage has higher income. Finally, year controls for secular trends in the health insurance market.

Technical Note B

Comparison of Elasticity Estimate to Stearns and Mroz

Stearns and Mroz (1995/1996) compute an elasticity of disenrollment with respect to premium for high-risk pools in eight States. Using some simple calculus, we convert disenrollment elasticity into an upper bound on elasticity of total enrollment.¹² We find that the average upper bound on total enrollment elasticity based on the results of Stearns and Mroz is -1.5, which is consistent with the estimate obtained in this article (because −1.9 is less than the upper-bound of −1.5).

Let T_i, D_i, and E_i represent total enrollment, number of disenrollees, and number of enrollees during period i, then

$${\mathrm{T}}_1={\mathrm{T}}_0+{\mathrm{E}}_1-{\mathrm{D}}_1.$$ (Eq. B.1)

Differentiating Eq. B.1 with respect to premium for period 1, P₁, and multiplying by P₁/T₁ we find an expression for elasticity of total enrollment, ε_T, in terms of changes in E₁ and D₁.

$${\varepsilon }_{\mathrm{T}}=\frac{{\text{dT}}_1}{{\text{dP}}_1}\frac{{\mathrm{P}}_1}{{\mathrm{T}}_1}=\frac{{\text{dE}}_1}{{\text{dP}}_1}\frac{{\mathrm{P}}_1}{{\mathrm{T}}_1}-\frac{{\text{dD}}_1}{{\text{dP}}_1}\frac{{\mathrm{P}}_1}{{\mathrm{T}}_1}$$ (Eq. B.2)

We can express ε_T in terms of elasticity of enrollment, ε_E, elasticity of disenrollment, ε_D, enrollment rate, r_E, and disenrollment rate, r_D:

$${\mathrm{\varepsilon }}_{\mathrm{D}}={\mathrm{\varepsilon }}_{\mathrm{E}}{\mathrm{r}}_{\mathrm{E}}-{\mathrm{\varepsilon }}_{\mathrm{D}}{\mathrm{r}}_{\mathrm{D}}$$ (Eq. B.3)

where

$${\mathrm{\varepsilon }}_{\mathrm{T}}=\frac{{\text{dE}}_1}{{\mathrm{E}}_1}\frac{{\mathrm{P}}_1}{{\text{dP}}_1}\text{and}{\mathrm{\varepsilon }}_{\mathrm{D}}=\frac{{\text{dD}}_1}{{\mathrm{D}}_1}\frac{{\mathrm{P}}_1}{{\text{dP}}_1}$$ (Eq. B.4)

$${\mathrm{r}}_{\mathrm{E}}=\frac{{\mathrm{E}}_1}{{\mathrm{T}}_1}\text{and}{\mathrm{r}}_{\mathrm{D}}=\frac{{\mathrm{D}}_1}{{\mathrm{T}}_1}$$ (Eq. B.5)

All quantities on the right-hand-side of Eq. B.3 are non-negative except ε_E, which is non-positive.¹³ Therefore, the first term on the right-hand-side of Eq. B.3 is negative. Dropping it yields an upper-bound on ε_T:

$${\mathrm{\varepsilon }}_{\mathrm{T}}\le {\mathrm{\varepsilon }}_{\mathrm{D}}{\mathrm{r}}_{\mathrm{D}}$$ (Eq. B.6)

Stearns and Mroz study the effect of an upward premium shock on disenrollment in eight States. For each State, they estimate a disenrollment elasticity, ε_D, and a disenrollment rate, r_D. Using these and Eq. B.6, we can estimate an upper-bound on total enrollment elasticity for each State and an average across States. For example, the annualized disenrollment rate for Iowa is r_D = 0.2 and the disenrollment elasticity is ε_D = 10.2. Thus, ε_T < -2.0. The average upper-bound computed in this way is ε_T < −1.5. This is strong support that the −1.9 value computed in this article is a reasonable estimate of the elasticity of total enrollment for high-risk pools.¹⁴

Technical Note C

Example Simulation Calculation

Below we provide an example calculation of the simulated enrollments provided in Table 6. This example is for the State of Alabama. Let T be total enrollment and P be premium and eT be elasticity of total enrollment with respect to premium. Then, by definition

$${\mathrm{\varepsilon }}_{\mathrm{T}}=\%\mathrm{\Delta }\mathrm{T}/\%\mathrm{\Delta }\mathrm{P}=\text{dlog}(\mathrm{T})/\text{d log}(\mathrm{P}).$$ (Eq. C.1)

To simulate a change in T requires use of an approximation to ε_T. Our approximation is

$${\mathrm{\varepsilon }}_{\mathrm{T}}=\mathrm{\Delta }\text{log}(\mathrm{T})/\mathrm{\Delta }\text{log}(\mathrm{P}).$$ (Eq. C.2)

Using Eq. C.2 and letting T₀, T₁, P₀, and P₁ be original enrollment, new (simulated enrollment), original premium, and new (lower) premium, respectively, the equation for simulated enrollment is

$${\mathrm{T}}_1=\text{exp}[\text{log}({\mathrm{T}}_0)+{\mathrm{\varepsilon }}_{\mathrm{T}}(\text{log}({\mathrm{P}}_1)-\text{log}({\mathrm{P}}_0))].$$ (Eq. C.3)

Using data for Alabama, the calculation is as follows.

Original Premium (P₀)

Per capita Medicare spending (proxy premium) = $5,901.84

Premium as percent of market = 175%

P₀ = 1.75 × $5901.84 = $10,328.22

log(P₀)= 9.25

Original Enrollment (T₀)

T₀ = 2,431

log(T₀) = 7.80

New, Lower Premium (P1)

Premium as percent of market = 125%

P₁ = 1.25 × 5901.84 = $7,377.30

log(P₁) = 8.91

New, Simulated Enrollment Calculation (T₀)

T₁=exp[log(T₀)+ε_T(log(P₁)−log(P₀))] =exp(7.80−1.9×(8.91- 9.25))=exp(8.45)= 4,656

(small difference from Table 6 due to rounding of intermediate results).

Technical Note D

Confidence Interval of Elasticity Estimate and Range of Enrollment Estimate

Our elasticity estimate is −1.9. The 95 percent confidence interval is −2.7 to −1.1. This implies a range of predicted enrollment of 134,485 to 179,249 and a cost range of $55 to $180 million.