Interaural level differences and sound source localization for bilateral cochlear implant patients

Abstract

Objective

The aims of this study were (i) to determine the magnitude of the interaural level differences (ILDs) that remain after cochlear implant (CI) signal processing and (ii) to relate the ILDs to the pattern of errors for sound source localization on the horizontal plane.

Design

The listeners were 16 bilateral CI patients fitted with MED-EL cochlear implants and 34 normal hearing listeners. The stimuli were wideband, high-pass and low-pass noise signals. ILDs were calculated by passing signals, filtered by head-related transfer functions (HRTFs), to a Matlab simulation of MED-EL signal processing.

Results

For the wideband signal and high-pass signals, maximum ILDs of 15–17dB in the input signal were reduced to 3–4dB after CI signal processing. For the low-pass signal, ILDs were reduced to 1–2dB. For wideband and high-pass signals, the largest ILDs for +/− 15 degree speaker locations were between .4 and .7dB; for the +/− 30 degree locations between .9 and 1.3dB; for the 45 degree locations between 2.4 and 2.9dB, for the +/− 60 degree locations, between 3.2 and 4.1dB and for the +/− 75 degree locations between 2.7 and 3.4dB. All of the CI patients in all stimulus conditions showed poorer localization than the normal hearing listeners. Localization accuracy for CI patients was best for the wideband and high-pass signals and was poorest for the low-pass signal.

Conclusions

Localization accuracy was related to the magnitude of the ILD cues available to the normal hearing listeners and CI patients. The pattern of localization errors for the CI patients was related to the magnitude of the ILD differences among loudspeaker locations. The error patterns for the wideband and high-pass signals, suggest that, for the conditions of this experiment, patients, on average, sorted signals on the horizontal plane into four sectors – on each side of the midline, one sector including 0, 15 and possibly 30 degrees, and a sector from 45 degrees to 75 degrees. Resolution within a sector was relatively poor.

The standard view of the use of auditory cues for sound source localization on the horizontal plane suggests that interaural level differences (ILDs) are dominant for signals with frequencies above 1500 Hz and interaural time differences (ITDs) are dominant for frequencies under 1000 Hz (e.g., Stevens and Newman 1936; Blauert 1997). Patients fit with conventional, bilateral cochlear implants using sound processing strategies based on continuous interleaved sampling (CIS) (Wilson et al. 1991) should have little or no access to ITD cues because (i) the envelope signals delivered by the implant’s signal processing are not efficient carriers of ITD information, (ii) independent (non-synchronized) processors drive each implant, and (iii) ITD cues for sound source localization require synchronous input to both ears. It is not surprising to find multiple reports demonstrating that, for this population, the localization of wideband signals is enabled by ILD cues and not ITD cues (e.g., Grantham et al. 2007, 2008; van Hoesel and Tyler 2003; Wilson et al. 2003; Schoen et al. 2005; see, however, Aronoff et al. 2010 for a report on one or possibly two patients who respond to ITD cues).

The aim of this paper was to determine the magnitude of the ILDs that are the basis for sound source localization by CI patients. All commercial CI signal processing includes an automatic gain control (AGC) at the front end of the signal path. In the MED-EL devices this produces a 2:1, 2.5: 1, 3:1, or 3.5:1 compression for signal levels greater than 48 to 67 dB SPL, depending on the processor’s sensitivity setting. Signal levels are further compressed when the acoustic signals, after AGC compression, band-pass filtering, and envelope extraction, are mapped by a logarithmic function into the electric dynamic range. ILDs in the input signal are known to be large for frequencies above 1500 Hz (e.g., approximately 20 dB at 6 kHz for a 60° azimuth) and much smaller for signals under .5 kHz (less than 6 dB) (Shaw 1974). At issue in this research was (i) the magnitude of the ILDs that remain after CI signal processing and (ii) the localization accuracy that those ILDs enable.

Methods

Subjects

Sixteen bilateral CI patients were tested following approval by the IRB at Arizona State University. Eleven used the MED-EL Opus 2 processor with a fine structure processing (FSP) coding strategy. Five used MED-EL processors with a CIS coding strategy similar to that used in Grantham et al. (2007, 2008). The patients ranged in age from 32 to 79 years. Other demographic data are found in Table 1. Twenty-two younger normal hearing listeners (ages 21–40 years) and 12 older (ages 50–70 years) listeners with age appropriate hearing (thresholds at 125 – 2000 Hz were less than or equal to 20 dB HL) were combined into a single group and served as the normative sample.

Table 1.

Demographic characteristics of CI patients.

Subject	Age	Gender	Age HL onset (years)	Years of CI Use RE/LE	Device RE/LE	# active channels RE/LE	Etiology of Deafness
FSP 2	41	M	37	2	Sonata	8/9	Bacterial infection
FSP 3	32	F	14	2/2	Sonata Medium	9/10	Viral infecti
FSP 4	79	M	19	1	Sonata	9/10	Hereditary
FSP 6	53	F	20	8	Combi 40+	9/10	Unknown
FSP 7	59	M	25	1/2	Sonata	11/10	Head Traum
FSP 9	77	F	20	6/2	Combi 40+/Sonata	12/12	Unknown
FSP 10	65	F	30	7/1	Combi 40+/Sonata	9/9	Unknown
FSP 11	43	M	42	.6/.5	Sonata	12/12	Head Traum
FSP 13	50	F	3	5/8	Pulsar/Combi 40+	12/11	Hereditary
FSP 14	66	M	38	.8/.7	Sonata	11/11	Unknown
FSP 15	60	M		2.5/1.9	Sonata	7/10	Unknown
CIS 1	50	F	29	9	Combi 40+	12/12	Unknown
CIS 2	50	F	3	5/9	Pulsar/Combi 40+	10/11	Hereditary
CIS 3	59	M	39	1.5/1	Sonata	7/10	Unknown
CIS 4	39	F	2	8/5	Combi 40+/Pulsar	12/11	Hereditary
CIS 5	39	F	14	1/3	Pulsar/Sonata	12/12	Unknown

Test signals

Three, 200-ms noise-band signals were created. All were shaped with 20-msec rise-decay times. The wideband (WB) signal was band-pass filtered between 125 and 6000 Hz. The low-pass (LP) signal was filtered between 125 and 500 Hz. The high-pass (HP) signal was filtered between 1500 and 6000 Hz. In all cases the filter roll-offs were 48-dB/octave. Broadband overall signal level was 65 dBA.

Test environment

The stimuli were presented from 11 of 13 loudspeakers arrayed within an arc of 180 degrees on the frontal plane. The speakers (Boston Acoustics 110x) were 15 degrees apart. An additional speaker was appended to each end of the 11-loudspeaker array but was not used for signal delivery. The 3.04 m × 3.35 m room was lined with 4 in. acoustic foam (NRC=.9) on all six surfaces along with special sound treatment on the floor and ceiling. The broadband reverberation time (RT₆₀) was 90 ms. Subjects sat in a chair at a distance of 1.67 m from the loudspeakers. Loudspeakers were located at the height of the listeners’ pinna.

Test conditions

Presentation of the three noise stimuli was controlled by Matlab. Each stimulus was presented 4 times from each loudspeaker. The presentation level was 65 dBA with a 2 dB rove in level (the level rove was used to reduce any cues that might be provided by the acoustic characteristics of the loudspeakers). Subjects were instructed to look at the midline (center loudspeaker) until a stimulus was presented. They entered the number of the loudspeaker (1–13) on a keypad.

Simulation conditions

Monophonic wideband, high-pass, and low-pass signals were generated in Matlab, and diffuse head related transfer functions (HRTFs; Gardner and Martin, 1994) with the ear canal and measurement system responses factored out were used to generate stereo signals for each of the three signal types at azimuthal angles from 0 to 75 degrees in 15 degree steps. A new token of noise was generated for each signal prior to shifting its virtual source position via an HRTF. All signals were set to root-mean-square (RMS) levels of −41 dB re. 0.707 (corresponding closely to 65 dB SPL) prior to HRTF processing. Each stereo signal was then passed to a Matlab implementation of symmetrical bilateral MED-EL Opus 2 signal processing that included the Opus 2 microphone response.

Twelve-channel processors were created with lower and upper corner frequencies of 70 and 8500 Hz, respectively. Center frequencies were 120, 225, 384, 579, 836, 1175, 1624, 2222, 3019, 4084, 5507 and 7410 Hz (MED-EL’s Logarithmic FS). The dual-loop, AGC (Stöbich et al., 1999) was implemented with a 3:1 compression ratio and sensitivity set to 75 percent (52.75 dB SPL; −53.75 dB re. 0.707) for both the left and right processors. Output compression was implemented by the “map-law” function y=log(1+cx)/log(1+c), where x is an uncompressed envelope value, c is a constant (equal to 1000 in this simulation), and y is a compressed envelope value representing percent electric dynamic range. Tables 2 and 3 show ILDs computed after the filter bank stage without, and with, the AGC activated, respectively. Table 4 shows ILDs computed after the output compression stage. The ILD values are averages from 100 repetitions for each condition, each with a unique token of noise.

Table 2.

ILDs (dB) for signals subjected to HRTFs, microphone response, pre-emphasis, and frequency decomposition via the Opus filter bank. Signals were not subjected to AGC or output compression. The center frequency of each filter is indicated beneath the channel number.

					WIDEBAND
	ch 1	ch 2	ch 3	ch 4	ch 5	ch 6	ch 7	ch 8	ch 9	ch 10	ch 11	ch 12
	120 Hz	225 Hz	384 Hz	579 Hz	836 Hz	1175 Hz	1624 Hz	2222 Hz	3019 Hz	4084 Hz	5507 Hz	7410 Hz
0°	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0
15°	0.8	1.0	1.7	2.8	3.6	3.2	1.8	3.2	3.0	2.9	2.0	1.4
30°	1.5	1.9	3.0	5.0	6.7	7.1	4.0	5.7	5.9	5.0	3.6	1.9
45°	2.0	2.5	3.7	5.9	8.2	11.4	8.6	7.2	10.0	6.3	5.0	6.2
60°	2.5	3.0	4.1	5.9	7.5	10.8	14.7	14.0	9.9	8.2	11.2	14.6
75°	2.7	3.2	4.3	5.6	6.4	7.7	8.0	11.9	15.3	9.3	11.2	16.6
				HIGH-PASS
0°						0.0	0.0	0.0	0.0	0.0	0.0	0.0
15°						1.7	2.0	3.2	3.0	2.8	2.1	1.7
30°						4.6	3.7	5.8	5.9	4.9	3.6	2.5
45°						10.1	7.8	7.2	10.0	6.2	5.0	4.7
60°							16.5	13.9	9.9	8.3	11.1	12.6
75°						7.8	8.3	12.1	15.3	9.3	11.0	14.9
				LOW-PASS
0°	0.0	0.0	0.0	0.0	0.0
15°	0.6	1.0	1.6	2.1	2.5
30°	1.3	1.8	2.8	3.7	4.5
45°	1.7	2.4	3.6	4.5	5.4
60°	2.1	2.9	3.9	4.7	5.4
75°	2.4	3.2	4.1	4.7	5.3

Table 3.

ILDs for signals subjected to HRTFs, microphone response, pre-emphasis, AGC with 3:1 compression ratio and 75% sensitivity, and the Opus filter bank. Signals were not subjected to output compression.

					WIDEBAND
	ch 1	ch 2	ch 3	ch 4	ch 5	ch 6	ch 7	ch 8	ch 9	ch 10	ch 11	ch 12
	120 Hz	225 Hz	384 Hz	579 Hz	836 Hz	1175 Hz	1624 Hz	2222 Hz	3019 Hz	4084 Hz	5507 Hz	7410 Hz
0°	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0
15°	−0.5	−0.5	0.1	1.1	1.9	1.4	0.2	1.4	1.3	1.1	0.3	−0.1
30°	−1.1	−1.1	−0.1	1.8	3.6	3.7	0.8	2.8	2.6	1.7	0.4	−1.0
45°	−1.9	−1.8	−0.7	1.5	3.7	6.8	3.9	2.8	5.4	1.4	0.5	1.0
60°	−2.2	−2.4	−1.4	0.4	2.0	5.3	9.3	7.8	4.0	2.7	5.6	8.0
75°	−2.4	−2.7	−1.7	−0.4	0.3	1.6	1.9	6.1	8.8	2.9	5.1	9.6
				HIGH-PASS
0°						0.0	0.0	0.0	0.0	0.0	0.0	0.0
15°						0.1	0.4	1.4	1.3	1.0	0.4	0.1
30°						1.4	0.6	2.9	2.6	1.7	0.5	−0.4
45°						5.4	3.2	2.8	5.5	1.4	0.6	0.1
60°							10.8	7.5	3.7	2.5	5.3	6.0
75°						1.8	1.8	5.7	8.2	2.4	4.4	7.6
				LOW-PASS
0°	0.0	0.0	0.0	0.0	0.0
15°	0.1	0.4	0.9	1.5	2.0
30°	0.3	0.7	1.6	2.5	3.6
45°	0.4	0.9	1.9	2.9	4.2
60°	0.6	1.1	2.0	2.9	4.2
75°	0.7	1.2	2.1	2.8	3.9

Table 4.

ILDs (dB) Signals subjected to HRTFs, mic response, pre-emphasis, AGC with 3:1 compression ratio and 75% sensitivity, Opus filter bank, and output compression with parameter = 1000. Blank cells are for signals with RMS levels in the bottom 15 % of the electrical dynamic range.

					WIDEBAND
	ch 1	ch 2	ch 3	ch 4	ch 5	ch 6	ch 7	ch 8	ch 9	ch 10	ch 11	ch 12
	120 Hz	225 Hz	384 Hz	579 Hz	836 Hz	1175 Hz	1624 Hz	2222 Hz	3019 Hz	4084 Hz	5507 Hz	7410 Hz
0°	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0
15°	−0.3	−0.2	0.0	0.4	0.7	0.5	0.1	0.4	0.4	0.3	0.1	0.0
30°	−0.6	−0.4	0.0	0.7	1.3	1.3	0.3	0.9	0.7	0.5	0.1	−0.3
45°	−1.0	−0.7	−0.2	0.6	1.4	2.4	1.3	0.9	1.6	0.4	0.2	0.4
60°	−1.2	−1.0	−0.5	0.2	0.7	1.8	3.2	2.5	1.1	0.8	1.7	3.0
75°	−1.3	−1.1	−0.6	−0.1	0.1	0.6	0.6	1.9	2.7	0.9	1.4	3.4
				HIGH-PASS
0°						0.0	0.0	0.0	0.0	0.0	0.0	0.0
15°						0.1	0.1	0.4	0.4	0.3	0.1	0.0
30°						0.8	0.2	0.9	0.7	0.5	0.1	−0.1
45°						2.9	1.1	0.8	1.5	0.4	0.2	0.1
60°							4.1	2.4	1.0	0.7	1.6	2.2
75°						0.9	0.6	1.8	2.4	0.7	1.2	2.7
				LOW-PASS
0°	0.0	0.0	0.0	0.0	0.0
15°	0.0	0.1	0.3	0.5	1.1
30°	0.1	0.2	0.5	0.8	1.9
45°	0.1	0.3	0.6	0.9	2.1
60°	0.2	0.3	0.6	0.9	2.1
75°	0.2	0.4	0.6	0.9	1.9

Results

ILDs before compression

Table 2 shows the ILDs (in dB), computed without AGC processing, from the output of the left and right filter banks, as a function of channel number for loudspeaker locations at 0 degrees, +/− 15 degrees, +/− 30 degrees, +/− 45 degrees, +/− 60 degrees and +/− 75 degrees. For the wideband and high-pass signals, the largest channel specific ILDs were 14.7 – 16.6dB. For the low-pass signals the largest channel specific ILDs were 5.3 – 5.4dB. A similar analysis of a wideband signal, i.e., ILDs as a function of channel number and speaker location, for an earlier signal processor by MED-EL, appears in Seeber and Fastl (2008). van Hoesel, Ramsden and O’Driscoll (2002) show ILDs before signal processing in octave bands measured at the microphone input to a research processor.

ILDs after compression

As shown in Table 4, after signal processing, the largest channel specific ILDs for the wideband and high-pass signals were between 3 and 4dB. The largest channel specific ILDs for the low-pass signal were between 1 and 2dB.

High-pass signal

The maximum ILD for the high-pass signals at +/− 15° was .4dB in channels 8 and 9. The ILDs for the signals at +/− 30° were only slightly larger – with the largest equal to .9dB in channel 8. However, for the signals at +/− 45°, the largest ILD increased to 2.9dB in channel 6. For signals at 60 degrees, the maximum ILD increased again to 4.1dB in channel 7. At 75 degrees, the maximum ILD was 2.7dB in channel 12.

Wideband signal

The largest ILD for the signal at +/− 15° was .7dB in channel 5. The largest ILD for the signal at +/− 30° was 1.3dB in channels 5 and 6. For the signals at +/− 45°, the largest ILD increased to 2.4dB in channel 6. For signals at 60 degrees, the maximum ILD increased to 3.2dB in channel 7. At 75 degrees, the maximum ILD was 3.4dB in channel 12.

The most marked difference in ILDs for the wideband vs. high-pass signals was the presence of small, negative ILDs in the low-frequency channels (see Tables 3 and 4). As we discuss later, this is a consequence of independent AGCs for the two processors, the head shadow and broadband compression, i.e., the lower level signals in the head shadow can, for some frequencies, receive more amplification than signals on the contralateral side – producing inverted ILDs.

Low-pass signal

As noted above, all ILDs were small – with a maximum of 2.1dB. The maximum ILD for speakers at +/− 15 degrees was 1.1dB. The maximum ILDs for speakers at 30–75 degrees were only slightly larger and were very similar to each other, i.e., 1.9–2.1dB. For each speaker location the largest ILDs were in channel 5.

Localization

Figure 1 shows localization accuracy in terms of RMS error [the D statistic of Rakerd and Hartman (1986)] for normal hearing listeners and for the CI patients. Chance performance was determined in the manner of Grantham et al. (2007) using a Monte Carlo simulation. For one hundred runs of 1000 Monte Carlo trials, the mean chance performance was 73.5 degrees with a standard deviation of 3.2. For the following analyses the CI patients fitted with FSP processors and the patients fitted with CIS processors were combined into a single group because the mean scores for the two groups did not differ significantly in any test condition.

Figure 1.

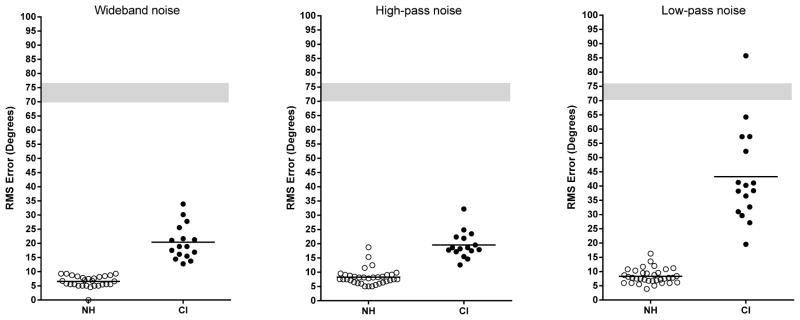
Localization accuracy for normal hearing listeners and CI listeners. The left, middle and right panels show results for wideband, high-pass and low-pass signals, respectively. The horizontal bars indicate the group mean scores. The grey zone indicates +/− 1 standard deviation for chance performance.

For the wideband signal, the mean RMS error scores were 6.6 degrees for the normal hearing group and 20.41 degrees for the CI group. For the high-pass signal, the mean scores were 8.3 degrees and 19.63 degrees, respectively. For the low-pass signal, the mean scores were 8.4 degrees and 43.36 degrees, respectively. The performance of the CI patients as a function of signal type was analyzed by a one-way analysis of variance. There was a significant effect of signal type (F (2, 45) = 24.98, p. < 0.0001). Post tests (Holm-Sidak) indicated that performance in the wideband and high-pass conditions did not differ, and both conditions had smaller RMS errors than the low-pass condition.

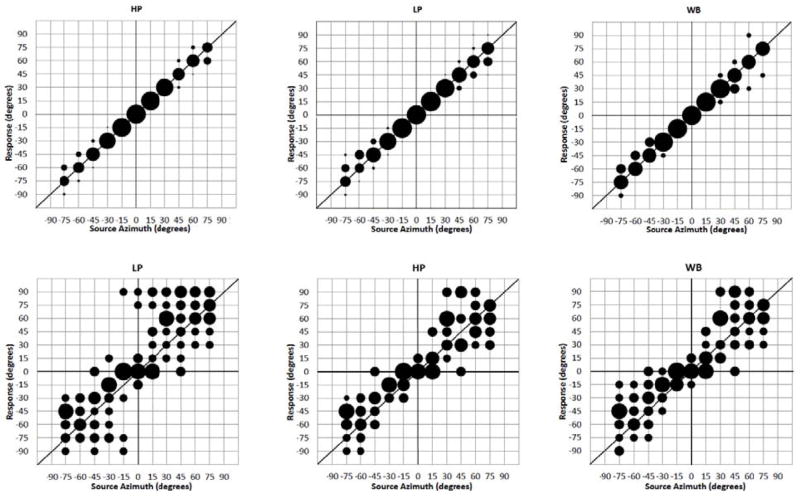
Bubble plots showing group mean responses as a function of speaker azimuth are shown in Figure 2. Figure 3 shows the responses of the best performing patients. We relate the pattern of errors to the magnitude of the ILDs available to the listeners in the paragraphs that follow.

Figure 2.

Group mean localization responses as a function of speaker azimuth for normal hearing (top) and CI patients (bottom) for low-pass, high-pass and wide band signals.. Bubble size is proportional to percent responses. Bubbles ≤ 10% are omitted from the plot. The diagonal line indicates correct responses.

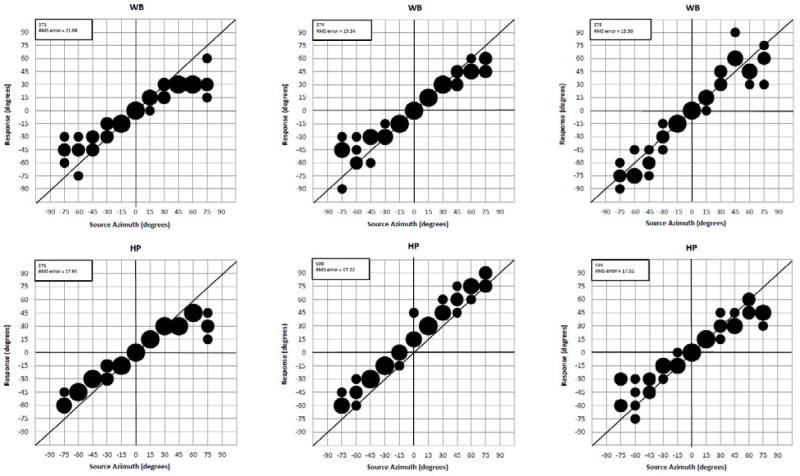
Figure 3.

Localization by best performing CI patients. WB = wide band noise. HP = high-pass noise.

Discussion

The results from our experiments replicate several findings in the literature, i.e., localization accuracy is poorer for bilateral CI patients than for normal-hearing listeners and localization accuracy, for CI patients, is poorer for low-pass signals than for wide-band and high-pass signals. Our results extend previous results (i) by specifying the ILDs, as a function of channel/frequency, that are available to CI patients without the AGC, with the AGC, and with the AGC and the compressive transformation from acoustic to electric stimulation and (ii) by linking the ILDs available to the listeners to the pattern of localization errors.

ILDs and localization accuracy

Localization accuracy was related to the magnitude of the ILDs available to the listeners. In the high-pass noise condition, where, presumably, both the normal hearing listeners and the CI patients were responding to ILD cues, normal hearing listeners averaged 8.3 degrees of error when maximum ILDs were 15–17dB. The CI patients in the same stimulus condition, who were operating on maximum ILDs of 3–4dB, averaged 19.63 degrees of error.

Within the CI group, the magnitude of the ILDs was related to localization accuracy. In the wideband and high-pass conditions, the maximum ILDs were 3–4dB and the patients averaged 20.41 and 19.63 degrees of error, respectively. In the low-pass condition, where ILDs were reduced to 1–2dB, the patients averaged over 43 degrees of error.

ILDs and error patterns

The response patterns, shown in the averaged data, were related to the ILDs at the different speaker locations. Consider, first, the ILDs for the high-pass signals. For speakers at 15 degrees, all ILDs were under .5dB. In contrast, for speakers at 45–75 degrees, the maximum ILDs were 3–4dB. Based on these data, it is reasonable to suppose that most errors to speakers at 15 degrees would be confined to 0, 15, or perhaps, 30 degrees (which had a maximum ILD of .9dB) and very few errors would extend to speakers at 45–75 degrees. Using the same logic, errors for speakers from 45–75 degrees should be within that group of speakers, and few responses should extend to loudspeakers at 0 and 15 degrees. The plot in Figure 2 indicates that these suggestions capture the majority of patient responses. Similar outcomes were obtained for the wideband stimulus (a similar response pattern for a single subject is shown in van Hoesel, 2004).

For the signals presented in the wideband and high-pass stimulus conditions, very few responses crossed the midline even for signals at +/− 15 degrees. Thus, ILDs of less than 1dB appear to be sufficient for hemifield resolution.

For the low-pass signal, the maximum ILD at 15 degrees was 1.1dB. The maximum ILDs for speakers at 30–75 degrees were slightly higher but essentially the same – 1.9–2.1dB. It is not surprising that performance was very poor in this stimulus condition (see also Grantham et al., 2007).

Our averaged results suggest, for wideband and high-pass signals, that the CI patients sorted signals on the horizontal plane into four quadrants. On each side of the midline, one quadrant was from center to 15 or 30 degrees and the second was 45 degrees and greater. As noted above, error responses to signals at +/−15 degrees did not cross the midline..

Individual differences

Average results obscure the poorest and best individual performances. In our study, there were large individual differences in performance. This has been the case in every study of localization by bilateral CI patients in which a large number of patients were tested (e.g., Grantham et al. 2007). There are multiple reasons for poor performance. Consider, first, that Grantham et al. (2008) found the threshold for detection of ILDs in signal processors configured like those in the present experiment, i.e., with compression activated, was, at best, 1 to 2dB and some patients had thresholds of 3 – 10dB. Given that the maximum ILDs for our signals were 3–4 dB, and differences between maximum ILDs at adjacent speaker locations were commonly on the order of 1 dB, then patients with discrimination thresholds of 3–10dB would be expected to show extremely poor localization. Consider also that ILDs in the input signal to normal hearing listeners are frequency and azimuth specific. In an implant processor, input filter frequencies are not matched to the place of stimulation provided by electrical stimulation. Moreover, many patients will have a different number of activated electrodes in their left and right cochlea, and the overall range of frequencies directed to each electrode array can differ. Thus, the ILD-to-frequency map for normal hearing listeners is not reproduced by CIs except by chance, and distortions to ILDs are likely to exist even when an effort is made to match fittings for the two CIs (e.g., Goupell et al. 2013).

The effects of independent AGCs

Another issue is independent AGCs for the two ears. Independent AGCs can influence broadband ILDs as a function of signal levels in three ways: 1) both signal levels are below the compression threshold and ILD cues are preserved; 2) the signal received by the processor closest to the source is above the compression threshold while the signal received by the contralateral ear is below the compression threshold and ILD cues are distorted; 3) both signals are above the compression threshold and ILDs are greatly, but symmetrically, reduced (see discussion by van Hoesel et al. 2002). The detrimental effect of situation 2 (above) is illustrated in the behavior of a subject in van Hoesel et al. (2002). This patient’s RMS error scores increased from 15 to 25 degrees when the signal level was increased from below compression threshold to above the threshold.

Although the AGC is driven by broadband input signals, frequency-specific ILD cues may also be distorted in the presence of two independent AGCs. Signals arriving at the processor contralateral to the position of the source will exhibit a low-pass characteristic relative to the original signal due to a reduction in energy, particularly at higher frequencies, caused by the head-shadow. As the AGC affects the overall spectral level, frequency decomposition may reveal that applying broadband compression has increased low frequency components to levels that exceed the levels of low frequency components on the contralateral side -- producing inverted ILDs. This is evident in the wideband ILDs shown in Tables 3 and 4, where small, negative ILD values can be seen for channels 1 and 2 for all source angles.

Given the issues described above, poor localization by patients fit with commercial signal processors is unremarkable. Good performance, on the other hand, is interesting.

Highest performing patients

To achieve a high level of performance with standard signal processors and input signal levels that invoke the independent AGCs, bilateral CI patients (i) need to adapt to the large change from maximum, frequency specific, ILD values of up to 20dB (when the patients had ‘normal’ hearing) to those available at the output of the CI, i.e., 3–4dB, and (ii) need to adapt to their ‘new’ ILD-by-frequency maps. The patients need to ‘stretch’ the 3–4dB range of ILDs to cover the entire frontal horizontal plane and not just the 15 degrees that 3–4dB ILDs specify for normal hearing listeners. Finally, if they are to locate signals at +/− 15 degrees relative to 0 degrees, they need to resolve ILDs of less than 1dB.

The data shown in Figure 3 indicates that the best performing patients have solved at least some of these problems. Perhaps these are patients with very good ILD resolution. Resolution of less than 1 dB has been reported by Lawson et al. (1998) and by van Hoesel (2004) under tightly controlled conditions. Another possibility is that, for the best performing patients, the settings of the signal processors, and current interactions, resulted in internal representations of ILDs different than those calculated in Table 3. Our data do not speak to the magnitude of the internal representations or the factors, e.g., number of discriminable steps for ILD resolution, that may constrain them. Patient S71 is of interest because of high accuracy for signals at +/− 15 degrees in both the high-pass and wideband stimulus conditions. This was achieved with electrode arrays with 8 and 9 contacts in the left and right ears, respectively.

On the generality of our simulations

The goal of these simulations was to produce generalizable results by quantifying electric ILDs under bilaterally matched conditions. Diffuse transfer functions, with the ear canal and measurement system responses factored out, were applied symmetrically so that original microphone placement and the variation between KEMAR’s left and right ears did not influence the estimated ILDs. The frequency response of the omni-directional microphone used in MED-EL’s Opus processors was incorporated into all simulations. The left and right ears were assumed to be identical in all respects including electrode positions, frequency allocation and electric dynamic ranges.

For a given patient there could be violations of bilateral matching at every stage of CI signal processing. In addition to the effects of head shape and microphone placement, contributions to variability among individual ILDs could arise from: (1) AGC settings; (2) frequency allocation tables; (3) pitch mismatches between electrodes to which common filter outputs are assigned; (4) unequal numbers of electrodes between ears; (5) electrode-specific dynamic ranges; (6) output compression settings; and (7) processor volumes. Deviations, as enumerated above, from the bilaterally matched condition utilized in these simulations could serve to degrade or enhance ILD cues. Comparisons of patient-specific electric ILDs to the generic ILDs reported here may provide additional insight into the effects of various stages of CI processing on localization abilities.

Functional consequences of poorer-than-normal localization

We have found that RMS error, for wideband signals, is, on average, 2 -3 times larger for bilateral CI patients than for normal hearing listeners. For some species even a modest decline in localization accuracy could have a large impact on survival. Barn owls, for example, use very precise sound source localization to aid in finding prey (Knutsen and Konishi 1979). A 2–3 fold loss of acuity could lead, at least initially, to a hungry barn owl. The consequences of a similar decrease in localization accuracy (relative to normal hearing) for bilateral CI patients are likely to be far less dramatic. Indeed, the ability to at localize at all is a significant factor in the improvement in self-reported quality of life for patients fit with bilateral CIs relative to patients fit with a single CI (Bichey et al. 2008).