Heuristics guide the implementation of social preferences in one-shot Prisoner's Dilemma experiments

Abstract

Cooperation in one-shot anonymous interactions is a widely documented aspect of human behaviour. Here we shed light on the motivations behind this behaviour by experimentally exploring cooperation in a one-shot continuous-strategy Prisoner's Dilemma (i.e. one-shot two-player Public Goods Game). We examine the distribution of cooperation amounts, and how that distribution varies based on the benefit-to-cost ratio of cooperation (b/c). Interestingly, we find a trimodal distribution at all b/c values investigated. Increasing b/c decreases the fraction of participants engaging in zero cooperation and increases the fraction engaging in maximal cooperation, suggesting a role for efficiency concerns. However, a substantial fraction of participants consistently engage in 50% cooperation regardless of b/c. The presence of these persistent 50% cooperators is surprising, and not easily explained by standard models of social preferences. We present evidence that this behaviour is a result of social preferences guided by simple decision heuristics, rather than the rational examination of payoffs assumed by most social preference models. We also find a strong correlation between play in the Prisoner's Dilemma and in a subsequent Dictator Game, confirming previous findings suggesting a common prosocial motivation underlying altruism and cooperation.

Cooperation is central to human societies, from personal relationships to workplace collaborations, from environmental conservation to political participation, international relations, and price competition in markets¹,²,³,⁴,⁵,⁶,⁷,⁸,⁹,¹⁰,¹¹,¹²,¹³,¹⁴,¹⁵,¹⁶,¹⁷. A simple model commonly used to study cooperation is the Prisoner's dilemma (PD), in which two agents can either cooperate (C) or defect (D): cooperating means paying a cost c to give a benefit b (b > c) to the other person; defecting means doing nothing. The PD is an attractive model of cooperation because it highlights the tension between individual and collective interests: agents maximize their personal payoff by defecting (and avoiding the cost of cooperation). But if both agents defect, both are worse off than if they had both cooperated.

Since cooperation is individually costly, standard economic models predict that people should not cooperate (unless the game is repeated, in which case theoretical models predict¹⁸,¹⁹,²⁰,²¹,²², and behavioural experiments demonstrate²³,²⁴,²⁵,²⁶,²⁷,²⁸,²⁹, that cooperation can be favoured via ‘reciprocity'; in repeated games, even selfish players may cooperate in order to gain the benefits of reciprocal cooperation in future periods³⁰). Yet cooperation in one-time encounters with strangers is common outside the laboratory, and a substantial amount of cooperative behaviour is observed in one-shot PD experiments in the lab with anonymous players³¹,³²,³³,³⁴,³⁵,³⁶,³⁷,³⁸,³⁹,⁴⁰,⁴¹.

Here we attempt to go beyond the observation that people sometimes cooperate in one-shot anonymous PDs by shedding new light on the motivations underlying this cooperative behaviour. Rather than giving participants a binary choice between C or D as is typically done, we make the decision space continuous (i.e. use a continuous-strategy PD): each participant chooses how much of an endowment to spend on helping the other player, with every c units spent resulting in the other person gaining b units. We also vary the b/c ratio, and ask how the distribution of cooperation levels changes as a result. This allows us to evaluate the predictions of different theories of cooperative behaviour and gain insight into the underpinnings of cooperation in the one-shot PD.

The standard explanation in economics for non-zero cooperation in one-shot games involves social preferences. Social preference theories typically assume that people are rational, but that their utility functions include more than just their own material payoff. Three main types of social preferences have been proposed: efficiency⁴², whereby people get utility from aggregate welfare (i.e. total payoff of all players) and thus may be willing to pay costs to give large benefits to others; inequity aversion⁴³,⁴⁴, whereby people get disutility from unequal payoffs and thus may be willing to pay to reduce the difference between their payoff and the payoffs of others; and reciprocity⁴⁵, whereby people get utility from cooperating with those who are cooperative and not cooperating with (or punishing) those who are uncooperative, and thus may be willing to pay the cost of cooperation if they expect others to do the same.

Efficiency models make a clear prediction regarding the distribution of cooperation levels and b/c dependence of cooperation levels in a continuous-strategy PD: players who primarily care about efficiency should engage in zero cooperation if b/c is below the critical threshold at which it becomes worth it for them to cooperate, and should engage in maximal cooperation if b/c is above this threshold. As threshold values vary across participants, increasing b/c should increase the average level of cooperation by shifting participants from zero cooperation to maximal cooperation.

Theories based on inequity aversion and reciprocity, conversely, do not make clear predictions, either about the distribution of cooperation levels or about their response to changes in b/c. Both inequity aversion and reciprocity favour matching the cooperation level of one's partner; thus any level of cooperation could be supported by these preferences, depending on one's expectations (i.e. ‘beliefs') about the behaviour of the partner. (This includes zero cooperation: if I believe my partner is self-interested, I will not cooperate even though I have a social preference for equality or reciprocity.) Therefore, as people can be expected to differ in their expectations about the cooperation levels of their partners (given variance in past experience inside and outside of the laboratory setting), these models predict a range of different cooperation levels, with no reason to expect specific levels to be more common than others. Furthermore, inequity averse and reciprocal participants will only change their cooperation level in response to changes in b/c in so much as they expect b/c to change the behaviour of their partner (for example, if they assume their partner has some preference for efficiency). Thus an increase in cooperation with b/c is an indication of participants either having efficiency preferences, or expecting others to have such preferences.

We also evaluate predictions generated by another class of models which relax the rationality assumption of standard social preference models. There is considerable evidence that heuristics, rather than rational utility maximization, play an important role in decision-making⁴⁶,⁴⁷,⁴⁸,⁴⁹,⁵⁰. Heuristics are simple rules of thumb prescribing behaviour which is typically desirable, but is not precisely tuned to the details of the current decision. Such heuristics may interact with social preferences in various ways. For example, inequity averse people might seek equal outcomes based on a fairness heuristic which favours equal splits of the endowment (50% cooperation), even in cases where an equal split does not actually lead to equal payoffs (e.g. if money transferred to the other person is multiplied by a constant, b/c > 1, transferring half of the endowment causes the other person to earn more than you). In addition, people with inequity averse or reciprocal preferences who are trying to predict the behaviour of their partner might be influenced by a heuristic that leads them to settle on particularly salient values such as the mid-point of the scale (50% cooperation) rather than carefully reasoning about what partner behaviour is most likely given the b/c ratio. Both of these interactions between heuristic reasoning and social preferences predict relative insensitivity to b/c, as well as a distribution of cooperation levels with substantial weight concentrated at 50%.

Here we evaluate the predictions of these different theories by examining the play of 308 participants in a continuous-strategy PD. Participants were each given ten monetary units and decided how many to transfer to their partner, with any transferred units being multiplied by a constant (the b/c value). We varied the b/c ratio across b/c = [2,3,4,5,10], with each participant making only a single decision with a single b/c. Finally, we sought to replicate recent results regarding the ‘cooperative phenotype'⁵¹, which suggest that a common motivation underlies both cooperation in the PD and altruism. Thus, after they completed the PD, we had participants play a unilateral, zero-sum money transfer (i.e. Dictator Game, DG).

Results

The distribution of cooperation levels for each b/c value is shown in Figure 1. For all values of b/c, we see a strongly tri-modal distribution concentrated on ‘give nothing', ‘give half', and ‘give everything'. Aggregating over all b/c values, we find that 22.4% participants transfer nothing, 19.2% participants transfer half, 52.3% participants transferred all, and only 6.2% participants transfer other amounts.

Figure 1. Distribution of cooperation levels in the PD as a function of benefit-to-cost ratio.

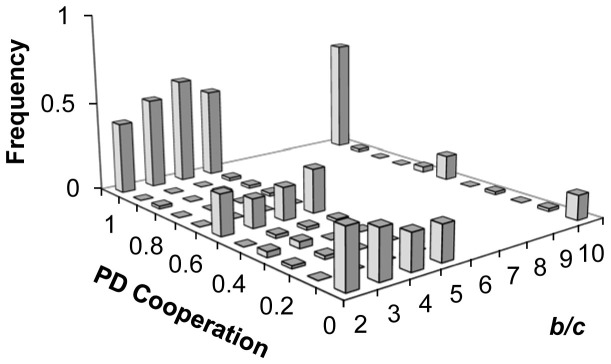
We next ask how the probabilities of giving nothing, half, and everything change with b/c using logistic regression. We find (i) that participants are significantly less likely to give nothing as b/c increases (coeff = −.130, p = .019); (ii) that participants are significantly more likely to give everything as b/c increases (coeff = .106, p = .010); and (iii) that the probability of giving half does not change with b/c (coeff = −.056, p = .302). These results are robust to controlling for age, gender, education, and log-transformed number of previous studies completed (Probability of giving nothing: coeff = −.145, p = .013; giving everything: coeff = .131, p = .003; giving half: coeff = −.085, p = .142); for completeness we report that when including demographics we also find that women (coeff = −0.779, p = 0.003) and participants who have had more experience with economic games (coeff = −0.330, p = 0.030) are significantly less likely to transfer everything. Furthermore, we do not find evidence of diminishing returns on increasing b/c: when redoing all of the above regressions including a (b/c)² term (to capture non-linear effects of b/c), the non-linear term is never significantly different from zero, p > 0.3 for all. Thus it appears that increasing b/c shifts people from transferring nothing to transferring everything, without affecting the percentage who give half the endowment.

We now examine how the mean level of cooperation changes as a function of b/c (Figure 2). Linear regression finds a significant positive relationship between cooperation and b/c (coeff = .239, p = .003; including controls: coeff = .258, p = .002); for completeness we report that when including demographics we find similar results as above, with women (coeff = −1.12, p = 0.022) and participants that have more experience with economic games (coeff = −0.592, p = 0.041) being less cooperative on average (these findings related to experience are consistent with previous work showing that experience with economic games undermines cooperative intuitions⁵⁰,⁵²). We again find no evidence of a non-linear relationship between b/c and cooperation (including (b/c)² term, p = 0.202). We also note that the relationship between mean cooperation and b/c is robust to excluding participants who made transfers other than nothing or everything (coeff = .283, p = .007).

Figure 2. Average amount of cooperation in the PD as a function of benefit-to-cost ratio.

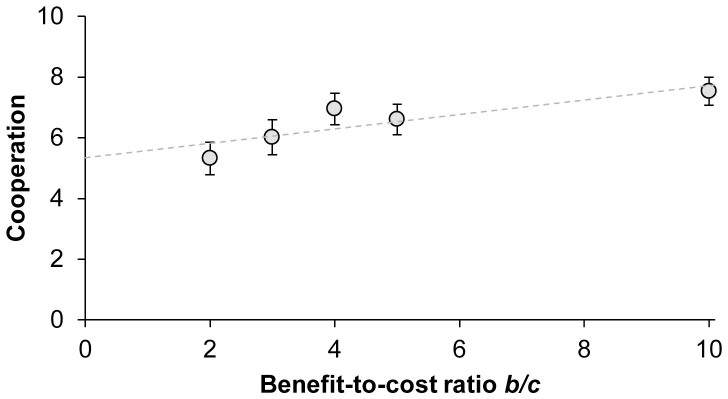
Error bars indicate standard errors of the mean.

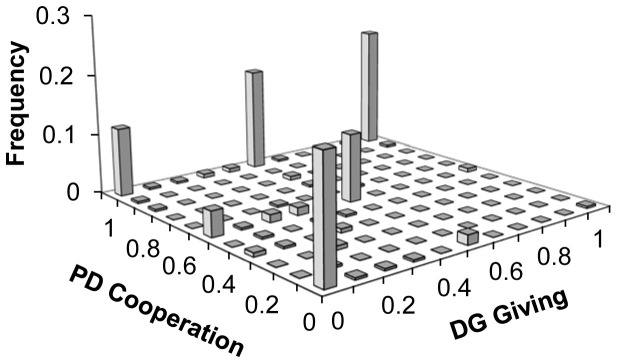
Finally, we analyse the relationship between cooperation in the PD and giving in the subsequent DG (Figure 3). Aggregating across conditions, we find a strong positive association between the PD and the DG (pairwise correlation: r = .561, p < .001; linear regression predicting DG as a function of PD, coeff = .522, p < .001; with controls: coeff = .535, p < .001; no significant correlation between any of the controls and DG giving). Examining Figure 3 shows that this correlation is largely driven by a lack of participants who gave in the DG but did not cooperate in the PD. Put differently, cooperators were not necessarily DG givers, but DG givers were almost certainly PD cooperators.

Figure 3. Joint distribution of cooperation in the PD and giving in the DG.

Discussion

Here we have found that cooperation levels in a one-shot continuous-strategy PD are tri-modally distributed, with peaks at zero, half and full cooperation; and that increasing the b/c ratio reduces zero cooperation and increases full cooperation, but that the influence of the b/c ratio is somewhat limited. Further, we have shown that giving in the PD is strongly correlated with giving in a subsequent DG.

The trimodal distribution we observe is not readily consistent with predictions of standard social preference models in which participants rationally maximize utility functions that depend on the payoffs of others. Efficiency concerns clearly predict a bimodal pattern of zero or full cooperation, with b/c decreasing zero and increasing full cooperation. Seeing as no information was given about the distribution of cooperation levels, inequity averse and reciprocal players would presumably have a range of different beliefs regarding their partner's expected behaviour. As a result, these models would predict a wide range of different cooperation levels, with no reason to expect clear modes at 0%, 50% or 100%. Furthermore, if these players believe that other players may have efficiency preferences, then they should anticipate partner cooperation increasing with b/c and therefore increase their own cooperation levels accordingly. Thus the modes at 0% and 100% cooperation, and the increase in cooperation with b/c, suggest that some participants either have efficiency preferences or anticipate that others will have efficiency preferences.

The observed trimodal distribution with substantial weight at 50%, and the relatively modest increase in cooperation in response to a large increase in b/c, conversely, are surprising in light of traditional social preference models. Both of these features, however, are direct predictions of theories based on heuristic reasoning. A simple fairness heuristic could lead participants to transfer 50% to their partners, mistakenly believing that this would lead to equal payoffs. (Note that in the PD, unlike in the Dictator Game, 50% cooperation is not the naturally equitable choice: this is both because there is multiplier on transfers in the PD, such that if you give 5 out of 10 units, the other person receives 5*b/c units; and because the other person is also making a decision.) Or a heuristic could lead expectations regarding the partner's behaviour to naively anchor on the salient mid-point of 50%.

To gain greater insight into the motivation of participants engaging in 50% cooperation, we examine responses these participants gave at the end of the study to the prompt “please describe why you made your decision in the game” (such free-response texts can give useful insights into participants' decision processes in economic games⁵³,⁵⁴). Consistent with a fairness heuristic, 24% of statements explicitly mentioned a desire to be fair as the main motivator for their choice to transfer half their endowment to the partner (despite the fact that transferring half does not in general create equal outcomes in our design). Consistent with a heuristic focusing beliefs regarding partner behaviour on the salient scale midpoint of 50% cooperation, 15% of statements explicitly said they engaged in 50% cooperation because they expected their partner to engage in 50% cooperation, while an additional 22% of statements said that they were unsure of whether their partner would cooperate and therefore only transferred half of the endowment. (Interestingly, another 16% of statements explained the choice to transfer half of the endowment by saying that it was a compromise between generosity and self-interest, a motivation that to our knowledge has not been previously discussed and which merits further study; the remaining 24% of statements either gave no reason or were not readily categorizable). In sum, participants' post-experimental descriptions of their decision processes provide direct evidence of heuristic use motivating the choice to engage in 50% cooperation.

We note that many of the participants engaging in zero or full cooperation may have also been using heuristics, but for these choices it is difficult to disentangle heuristic reasoning from rational application of social preferences, as they lead to the same outcomes. For example, a heuristic that prescribes contributing everything is indistinguishable in this paradigm from a rationally applied efficiency preference. (This is unlike a fairness heuristic that prescribes giving half of the endowment, because giving half does not in general actually create equal outcomes in our PDs.) Finally, it is important to note that the present study illuminates the role heuristics play in the implementation of social preferences, rather than the role that heuristics formed via internalization of norms may play in the origin of social preferences⁵⁰,⁵²,⁵⁵,⁵⁶,⁵⁷,⁵⁸.

An important limitation of our experiment is that because of our between-subjects design, we cannot observe specific individuals changing their behaviour. Thus we cannot distinguish between two possibilities among our participants that gave either nothing or everything: it could be that each such person has a personal minimum b/c at which the psychological benefits of cooperation begin to outweigh the financial costs. If this was the case, any given person would always give nothing below that critical b/c, and always give everything above it; and the gradual increase in average cooperation with b/c we observe in Figure 2 would be the result of more and more people having passed their personal thresholds. Alternatively, it could be that people behave probabilistically, with their chance of cooperating in any given decision increasing as b/c increases. In this case, the graded response to b/c that we observe at the population level in Figure 2 would also be reproduced within each individual. Distinguishing between these possibilities is likely to be difficult, however, because of consistency and contagion effects, like those we observed between the PD and the DG: if one's choice in a given cooperation decision is heavily influenced by choices in immediately previous decisions, it makes it difficult for experimenters to obtain a clean measure of how the payoff structure influences that person's choices. Nonetheless, this is an important direction for future research.

To our knowledge, only a handful of previous studies have experimentally investigated how the payoff structure affects cooperation in a one-shot PD. All of these studies have used a binary PD, preventing them from drawing conclusions regarding the distribution of cooperation levels as we do here. With respect to the effect of the PD's payoff structure on cooperation, these studies have typically not used the benefit-to-cost ratio decomposition of PD payoffs, but instead directly varied one or more of the payoffs associated with the four possible PD outcomes ([C,C],[C,D],[D,C],[D,D]). An early study found that cooperation increased as the [D,D] payoff was decreased⁵⁹, a finding that was replicated across a wider range of values in a more recent study³⁴. Two other studies found that cooperation decreased as the ratio of payoffs ([D,C]-[D,C])/([C,C]-[D,D]) was increased⁶⁰,⁶¹. We add to these studies by examining the distribution of cooperation amounts, and by using the b/c formulation which is standard in evolutionary game theory⁶² and readily interpretable in terms of predictions based on efficiency preferences.

The substantial correlation we observe between play in the PD and the DG adds weight to previous work from our group showing significant correlations in play across the DG, the Public Goods Game (a 4-person version of our continuous PD), and the Trust Game, which was argued to reflect a ‘cooperative phenotype'⁵¹. This replication is important, given that an earlier study found no correlation between the Public Goods Game and a modified Dictator Game in which participants made 21 decisions between two pairs of options that were more or less fair⁶³. Both our study and ref. 51 had an order of magnitude more participants than ref. 63; thus, it is possible that the latter null result was due to a lack of power. It could also be that the modified DG structure of ref. 63, in which many extremely similar decisions were made in a row, introduced self-consistency effects or other confounds that obscured a true relationship with the Public Goods Game.

Further evidence regarding the relationship between cooperation and fairness comes from the fact that DG givers in our study were almost entirely a strict subset of PD cooperators. This observation suggests that the motives present in the DG (e.g. inequity aversion) are also present in the PD, but that additional motives exist in the PD that do not in the DG (e.g. concerns about efficiency or the choice of the other player).

In sum, our results give insight into the decision-making process in one-shot anonymous Prisoner's Dilemma games. We provide evidence that many people who cooperate deviate from traditional models of rational self-interest not only by being sensitive to the payoffs of others (i.e. being ‘other-regarding'), but also by using simple heuristics. We also provide further evidence for a domain general proclivity to cooperate across games.

Methods

We recruited participants using the online labour market Amazon Mechanical Turk (MTurk)³²,⁶⁴,⁶⁵,⁶⁶. Participants received a $0.35 show-up fee and were told they would be playing a two-stage game in which they could earn additional income.

In the first stage, participants were paired with another MTurk worker, and both were given $0.10. They each then chose how much, if any, to transfer to the other person, with any transfers being multiplied by a constant k. We manipulated the PD payoff structure by varying the value of k across k = [2,3,4,5,10], with a given participant being randomly assigned to a single value of k (i.e. a between-subjects design). In this continuous PD, b/c = k because for each cent participants transferred, the recipient received k cents.

Before making their decision, participants answered comprehension questions to make sure they understood the payoff structure (see Supplementary Figures S1-4 for the exact instructions). Given that our key manipulation involved changing the payoff structure, it was essential that participants understood the payoffs. Therefore, participants who answered any questions incorrectly were not allowed to participate. After answering the comprehension questions, participants made their PD decision, and then moved on to the second stage (without learning their partner's decision in the PD, to prevent contagion effects).

In the second stage, participants were paired with a different MTurk worker. Participants were given $0.10 and had to decide how much, if any, to unilaterally transfer to the other person (transfers were not multiplied, and the other person had no initial endowment and made no transfer decision – i.e. participants played a standard Dictator Game). Finally, participants completed a free-response describing the reasons for their decisions in the games and a demographic survey. After all participants had been recruited, they were matched at random and payoffs were calculated as described. No deception was used in this study, informed consent was obtained from all participants, and the study was approved by the Harvard University Committee on the Use of Human Subjects. Methods were carried out in accordance with the approved guidelines.

A total of 308 US resident participants answered all comprehension questions correctly (mean age = 30.8 years, 62% male) and were thus allowed to participate in the experiment (140 people answered one or more comprehension questions incorrectly and were excluded). IP addresses were screened to prevent the same person from participating repeatedly. 66 participants were assigned to the PD with multiplier k = 2; 56 participants were assigned to the PD with multiplier k = 3; 60 participants were assigned to the PD with multiplier k = 4; 61 participants were assigned to the PD with multiplier k = 5; and 65 participants were assigned to the PD with multiplier k = 10. In addition to the $0.35 show up fee for completing the task, participants earned an average of $0.47 of additional income based on the games.

To assess the free-response statements participants giving half in the PD provided regarding their motivations in the game, two research assistants coded each response from these participants. The coders were not informed about the purpose of the study or the various hypothesis and predictions being tested. For each statement, they were asked which of the following eight categories best described it (fraction of statements assigned to each category indicated in parentheses):

1. The participant explicitly said that they took the action because it was fair (24%)

2. The response indicates that the participant expected the other person to take the same action, so that's why they took it (15%)

3. The response indicates that because the participant felt uncertainty about the other person's action, the participant decided to hedge/reduce variance by taking this action (22%)

4. The response indicates that the participant wanted to compromise between taking a selfish action and taking a generous action (16%)

5. The participant explicitly references intuition/their gut feeling/going with the first thing that came to them (3%)

6. The response indicates that the group payoff would be maximized by taking this action/it would be overall best for everyone to take this action (2%)

7. The response restates what the person did, but does not provide an explanation (13%)

8. The response indicates that the participant didn't want to be greedy/selfish (6%)

For the instructions from our study, see Supplementary Information, Supplementary Figures S1-4.

Author Contributions

V.C., J.J.J. and D.G.R. designed the research, analysed the data and wrote the manuscript.