Abstract
We demonstrate how optical tweezers may provide a sensitive tool to analyze the fluidic vibrations generated by the movement of small aquatic organisms. A single gold nanoparticle held by an optical tweezer is used as a sensor to quantify the rhythmic motion of a Nauplius larva (Artemia salina) in a water sample. This is achieved by monitoring the time dependent displacement of the trapped nanoparticle as a consequence of the Nauplius activity. A Fourier analysis of the nanoparticle's position then yields a frequency spectrum that is characteristic to the motion of the observed species. This experiment demonstrates the capability of this method to measure and characterize the activity of small aquatic larvae without the requirement to observe them directly and to gain information about the position of the larvae with respect to the trapped particle. Overall, this approach could give an insight on the vitality of certain species found in an aquatic ecosystem and could expand the range of conventional methods for analyzing water samples.
Keywords: Biophysics, Issue 89, optical tweezers, particle tracking, plasmonic nanoparticles, Nauplius, bioindicator, water sample analysis
Introduction
Water quality assessment based on chemical and biological indicators is of fundamental importance to gain insight on the state and environmental conditions of an aquatic ecosystem1-3. Classical methods for chemical water analysis are based on organoleptic properties or the determination of physicochemical parameters. Biological indicators, on the other hand, are animal species whose presence and viability provide insight on the environmental conditions and the effect of pollutants for an ecosystem that they occur in. Typical examples for bioindicators are Copepods, a group of small water crustaceans, which can be found in nearly any water habitat4,5. Observing the activity and viability of these species from a water sample can thus be used to obtain information on the overall conditions of an ecosystem5. The larvae of Copepods, which are called Nauplii, use rhythmic strokes of their antennae (each larva has three pairs of appendages at their head region) to swim in water6. The frequency and intensity of these strokes is thereby a direct indicator of the age, fitness, and environmental conditions of the animal7-10. Any investigations on these specimens are usually done with a microscope by observing and counting the antenna strokes of the Nauplii directly. Due to their size (~100-500 µm)11, this often requires to do measurements either one by one or to fix a single Nauplius to a substrate.
Here, we demonstrate a new approach to observe the activity of Copepod larvae in water samples by using an optically trapped gold nanoparticle as an ultra-sensitive detector. Optical tweezers are typically used by many groups as a fine experimental tool to apply or measure forces between molecules down to the piconewton range12-14. More recently, the range of applications for optical tweezers has been expanded to observe acoustic vibrations and solvent fluctuations in liquid media by monitoring the motion of nano- and microparticles that are confined in an optical trap15. Particles that are immersed in a liquid are subjected to Brownian motion. Inside an optical trap, however, this motion is partially damped by a strong, laser induced, gradient force. Therefore, the stiffness of the optical trap and the localization of the particle within the focus of the laser beam can be tuned by the laser power. At the same time, it is possible to reveal characteristics about the trapping potential and to analyze interactions of molecules with the particle by monitoring the time-dependent particle motion in the trap. This approach renders it possible to pick up the frequency, intensity, and the direction of the fluidic motion that is generated by a moving object in its liquid environment. We demonstrate how this general idea can be applied to obtain a frequency spectrum of the motion of an individual Nauplius without the requirement to directly interfere with the specimen. This experimental approach introduces a new general concept for the observation of the motile behavior of aquatic specimens in a very sensitive way. For observations on bioindicator species, this could expand the current methodology for water analysis and could be applied to gain information about the health and the integrity of aquatic ecosystems.
Protocol
1. Experimental Setup
Use an up-right microscope and a dark field oil condenser with a numerical aperture (NA) = 1.2 for dark field illumination. Use a water immersion objective with 100X magnification and a NA = 1.0 for particle observations and trapping. Use an air objective with 10X magnification and a NA = 0.2 to follow the motion of the Nauplius.
Use an optical tweezers setup with a 1,064 nm continuous wave laser coupled into the up-right microscope. Set the laser power of the optical trap to 100 mW (measured with a power meter after the objective).
Use a CMOS high-speed camera or a digital single lens reflex (DSLR) camera to detect and image the gold particle movement in the optical trap and the motion of the Nauplius.
Use a notch filter to prevent the laser from entering the camera.
Use a power meter to measure the laser power after the objective.
2. Sample Preparation
Pipette a water droplet (180 µl) on a microscope glass slide and position the sample on the dark field microscope.
Pipette a Nauplius from a small water tank to the water droplet.
Use a 10X air objective to observe the movement of the Nauplius in the solution and record a video stream.
Use a gold nanoparticle with a diameter of 60 nm as a detector to observe the fluid motion generated by the Nauplius. Therefore, add 5 µl of a highly diluted particle solution into the water droplet, so that approximately one particle can be seen in the field of view with a 100X water immersion objective.
3. Particle Tracking Experiment
Trap one gold nanoparticle with the optical tweezer. Therefore, bring the 1,064 nm trapping laser close to a gold nanoparticle that is diffusing in solution by moving the microscope stage. The attractive optical forces pull the gold nanoparticle towards the focal point of the laser beam. The trapped particle is not diffusing anymore and rather keeps its position. Take a video stream of the trapped nanoparticle with the DSLR camera at a frame rate of 50 Hz for 30 sec.
Turn off the laser of the optical tweezer and release the gold nanoparticle from the trap.
Use a particle tracking program to readout the position of the optically trapped gold particle at each frame of the video stream. A fast Fourier transformation (FFT) of the particle's x-y-position over time reveals a frequency spectrum. NOTE: Here, a self-written ‘IGOR PRO’ computer program code was used to analyze the particle center position in the x-y-plane over time and for FFT analysis.
- As an alternative to a self-written IGOR code use the freely available ‘Video Spot Tracker’ program for tracking the particle in the video. Use the commercial software ‘Origin’ to perform the Fourier transformation of the tracking data:
- Drag the video file to the open program ‘Video Spot Tracker’.
- Mouse click on the particle seen in the first picture of the video stream and a circular region of interest appears.
- Choose “symmetric” and “optimize” in the top command prompt window to optimize the tracking of the particle.
- Mouse click “logging” in the top command prompt window and choose a folder to save the data. The tracking data will be saved as a data spreadsheet.
- Mouse click “play video” on the left command prompt window of the tracking program and wait until all frames of the video are analyzed.
- Close the program and open the saved data spreadsheet with ‘Origin’. Set the column values as “y1” and “y2”.
- Set time steps for each video frame as “x” in the ‘Origin’ data spreadsheet.
- Mark the x-position column and perform a FFT by choosing “Data Analysis” and “FFT” in the top command prompt window. Repeat the step for the y-position column.
- Plot the amplitudes of the calculated FFT signal in x- and y-direction versus the frequency.
4. Numerical Simulation
- Calculate the polarizability α of the 60 nm gold particle by using the computer program ‘Mathematica’.
- Use equation (1) to calculate the polarizability according to Kuwata et al.16:
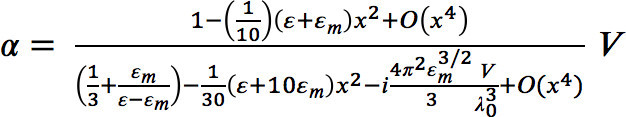 (1)
(1) - Define the following three parameters in the program code: the wavelength-dependent complex dielectric function of the gold particle, the nanoparticle radius, and the refractive index of the surrounding medium.
- Use the description of the electric field distribution of a focused Gaussian beam according to Agayan et al.17 to calculate the optical forces acting on a 60 nm gold particle:
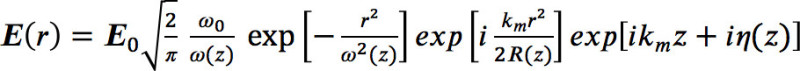 (2)
(2) - Use equations (3)-(6) from Agayan et al.17 to calculate both, the gradient and scattering forces acting on the particle:
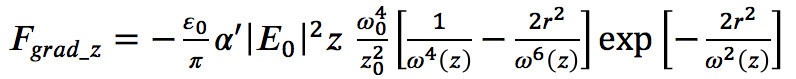 (3)
(3) 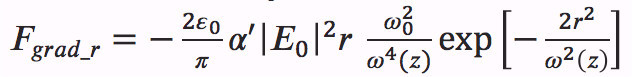 (4)
(4) 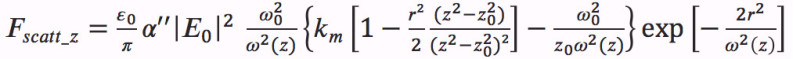 (5)
(5) 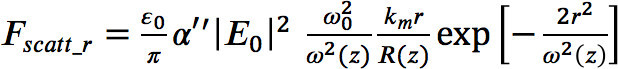 (6)
(6) - In the program code, define the parameters for the laser power, the numerical aperture of the objective, and the complex polarizability of the nanoparticle.
- Sum up the gradient force and the scattering force to calculate the total optical force acting on the gold particle in an optical trap.
Run the simulation by simultaneously pressing “Control” and “Enter”.
Representative Results
A schematic illustration of the experimental setup is shown in Figure 1A. A dark field configuration is necessary to optically detect the displacement of a 60 nm gold particle in an optical trap15. The wavelength of 1,064 nm for the trapping laser is chosen to guarantee a stable confinement of the detector gold particle12,14. A beam splitter in the microscope is used to focus the trapping beam through the objective and a notch filter prevents the trapping laser from entering the detection device of the experiment. The Nauplius was performing movements in the water solution surrounding the optically trapped gold nanoparticle (Figure 1B). The fluidic vibrations that are generated by the animal propagate through the liquid medium and interact with the optically trapped particle.
Figure 2A shows a dark field image of a single 60 nm gold nanoparticle that is trapped by the laser beam. The greenish color under dark field illumination indicates its scattering frequency in that wavelength range. Observing the color of the trapped particle with a DSLR camera ensures that just one plasmonic nanoparticle is trapped by the focused laser since trapping of a second particle would result in a color change due to plasmonic coupling. The calculated distribution of the total optical force that keeps the particle confined in the trap is shown in Figure 2B. Without any external fluidic vibration, the displacement of the trapped plasmonic nanoparticle shows a Gaussian distribution, since its movement is solely subject to Brownian motion (Figure 2C). As soon as one Nauplius is added to the sample, its movement creates a fluidic interaction with the detector particle. The nanoparticle in the optical trap starts to oscillate in the direction of the fluid interaction up to an oscillation amplitude of 100 nm (Figure 2D).
The movements of several Nauplius larvae were independently analyzed by monitoring their swimming behavior with a high speed CMOS camera. An example is shown in Figure 3A. One full oscillation of the periodic motion of the main arm of the large antennae takes 148 msec, which corresponds to a frequency of around 6.75 Hz. We observed the same Nauplius over a time period of several seconds and also different Nauplii from the same sample. From the direct observation we observed frequencies for the antennae strokes in the range between 4.1 and 7.2 Hz.
Figure 3B and Figure 3C show the frequency spectra of a trapped gold nanoparticle without (black curve) and with (red curve) a Nauplius present in the observed water droplet. Almost no signal can be seen in the x-direction of the particle's Fourier spectrum. In contrast, the y-direction of the frequency spectrum shows a strong response. This can be explained by the relative position of the Nauplius with respect to the particle trap. The nanoparticle detects only those vibrations that are generated by the organism. A strong signal in y-direction therefore indicates the direction of the fluidic oscillations and also the position of the animal (cp. Figure 2D). Transforming the time-dependent particle displacement trajectory into Fourier space therefore leads to a direction dependent difference in the signal intensity of the frequency spectra. The broad frequency range present in our measurements is consistent with net organism motility. The movements of the two main antennas of the Nauplius are not the exclusive source of liquid displacement. Movements of smaller antenna pairs and other body protrusions also contribute to the observed signal. For all measurements, we found frequency maxima between 3.0 and 7.2 Hz for the Nauplius movement, which is in a good accordance to the directly observed frequencies of the biological microorganism and also fits well to the expected frequency range for a Nauplius in a larval stage6,8-10.
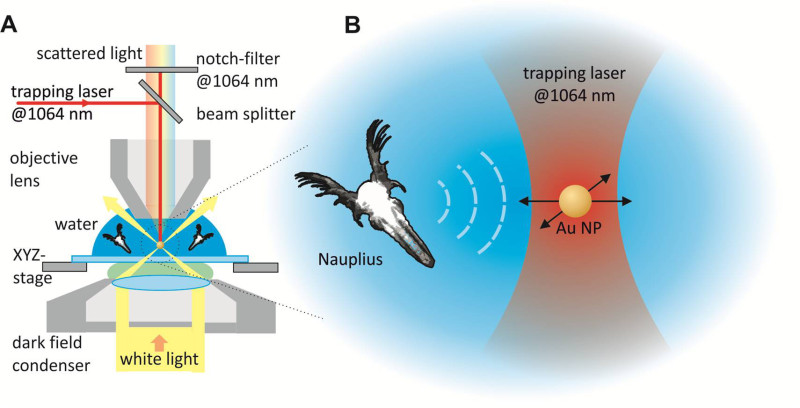 Figure 1. Schematic illustration of the experimental setup. A) Dark field configuration and optical tweezer. A beam splitter in the microscope is used to focus the trapping beam (1,064 nm, continuous wave) to the stage of the dark field microscope. A notch filter prevents the laser from entering the high-speed or DSLR camera. B) One gold nanoparticle is trapped in the optical tweezer to detect the microfluidic vibrations of one Nauplius in the surrounding medium. Please click here to view a larger version of this figure.
Figure 1. Schematic illustration of the experimental setup. A) Dark field configuration and optical tweezer. A beam splitter in the microscope is used to focus the trapping beam (1,064 nm, continuous wave) to the stage of the dark field microscope. A notch filter prevents the laser from entering the high-speed or DSLR camera. B) One gold nanoparticle is trapped in the optical tweezer to detect the microfluidic vibrations of one Nauplius in the surrounding medium. Please click here to view a larger version of this figure.
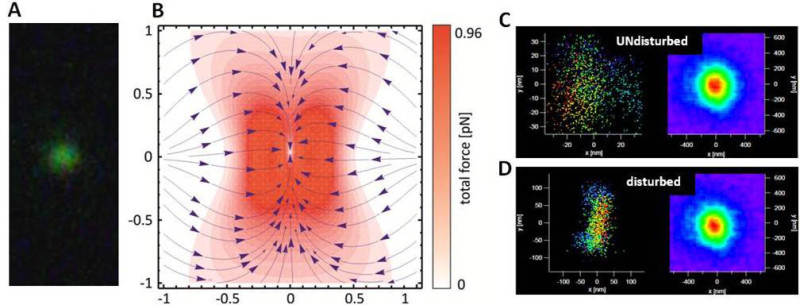 Figure 2: Optical trapping of a gold nanoparticle. A) Dark field image of a single trapped gold particle. B) Calculation of the total force acting on the particle in an optical trap. The laser wavelength is 1,064 nm and the power of 100 mW was measured under the objective. The force is plotted in the region of 2 µm around the focal point. C) x-y-displacement of a gold particle in an optical trap. The particle movement is not disturbed by fluidic vibrations and only caused by Brownian motion. D) x-y-displacement of the gold particle in the trap, after adding a Nauplius to the liquid. The microfluidic flow generated by the animal causes a frequency dependent distortion of the gold nanoparticle displacement in y-direction. Please click here to view a larger version of this figure.
Figure 2: Optical trapping of a gold nanoparticle. A) Dark field image of a single trapped gold particle. B) Calculation of the total force acting on the particle in an optical trap. The laser wavelength is 1,064 nm and the power of 100 mW was measured under the objective. The force is plotted in the region of 2 µm around the focal point. C) x-y-displacement of a gold particle in an optical trap. The particle movement is not disturbed by fluidic vibrations and only caused by Brownian motion. D) x-y-displacement of the gold particle in the trap, after adding a Nauplius to the liquid. The microfluidic flow generated by the animal causes a frequency dependent distortion of the gold nanoparticle displacement in y-direction. Please click here to view a larger version of this figure.
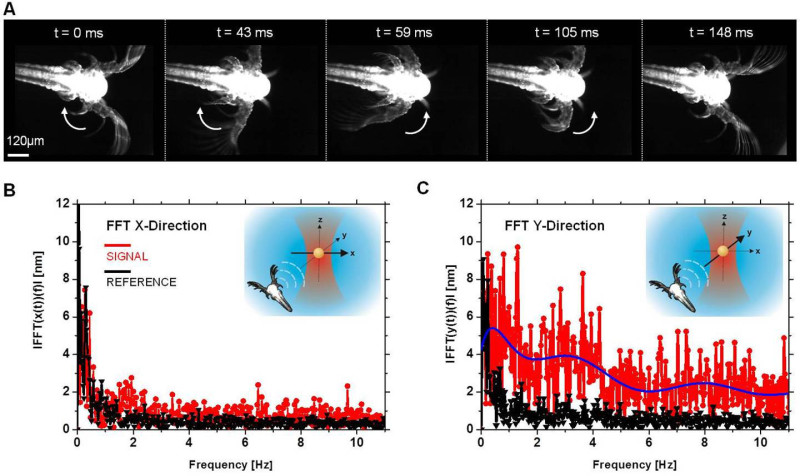 Figure 3: Frequency spectra of a gold nanoparticle trapped next to a swimming Nauplius. A) Antennae strokes of a single Nauplius at different time points. One complete oscillation of the periodic movement of the main antennae takes around 148 msec (6.75 Hz). B) Black curve: Frequency spectrum of the displacement of an undisturbed optically trapped nanoparticle in x-direction that was taken as a reference. Red curve: Frequency spectrum of the gold particle next to a swimming Nauplius in x-direction. The spectrum does not show a strong signal due to the relative position of the Nauplius to the optically trapped particle. Inset: Schematic illustration of the Nauplius and gold nanoparticle position during the experiment. The flow generated by the moving Nauplius is mainly pointing in the y-direction. C) Black curve: Reference frequency spectrum of the undisturbed gold particle in y-direction. Red curve: Frequency spectrum of the gold nanoparticle displacement in presence of a Nauplius. Please click here to view a larger version of this figure.
Figure 3: Frequency spectra of a gold nanoparticle trapped next to a swimming Nauplius. A) Antennae strokes of a single Nauplius at different time points. One complete oscillation of the periodic movement of the main antennae takes around 148 msec (6.75 Hz). B) Black curve: Frequency spectrum of the displacement of an undisturbed optically trapped nanoparticle in x-direction that was taken as a reference. Red curve: Frequency spectrum of the gold particle next to a swimming Nauplius in x-direction. The spectrum does not show a strong signal due to the relative position of the Nauplius to the optically trapped particle. Inset: Schematic illustration of the Nauplius and gold nanoparticle position during the experiment. The flow generated by the moving Nauplius is mainly pointing in the y-direction. C) Black curve: Reference frequency spectrum of the undisturbed gold particle in y-direction. Red curve: Frequency spectrum of the gold nanoparticle displacement in presence of a Nauplius. Please click here to view a larger version of this figure.
Discussion
Dark field microscopy is a powerful tool for visualizing gold nanoparticles with dimensions below the optical diffraction limit, since the scattering cross section of the metal nanoparticles exceeds their geometric cross section (cp. Figure 2A)18. In a tweezer setup, this approach even allows to distinguish if only a single or multiple gold nanoparticles are trapped by the laser beam because plasmonic coupling between the particles causes a red-shift of the plasmon resonance frequency15. Dark field microscopy with an optical tweezer configuration therefore provides numerous new and very useful experimental possibilities, but the combination is not self-evident. For stable optical trapping a strongly focused laser beam is required, since the origin of an optical trap in three dimensions is caused by a gradient of the optical field density. Usually, objectives with high numerical apertures (NA = 1.3-1.4) are used for tweezer setups to achieve a tight focusing of the laser19. The highest NA of commercially available dark-field oil condensers, however, is 1.2. This limits the range of objectives that can be used for trapping the particle to NA < 1.2 because higher NA objectives bear the problem that not only scattered, but also straight light is collected by the objective lens. For our setup, we are able to achieve a stable optical trapping by using a water immersion objective with a NA = 1.0 and a dark field condenser with a NA = 1.2. This is possible, because the laser beam expansion in front of the microscope led to an overfilling of the back aperture of the objective and therefore to a sufficient focusing of the laser (even with a NA of only 1.0).
Stable trapping of a plasmonic gold nanoparticle is also strongly dependent on the wavelength of the trapping laser12-14. In our experiments, a wavelength of 1,064 nm was chosen for the particle trapping because this wavelength is far red-shifted from the particle's plasmon resonance wavelength at ~530 nm. This is important for a stable trapping since optical gradient forces acting on the gold particle are dominant for this wavelength while scattering forces, which originate from a momentum transfer of scattered and absorbed photons, are minimal. Both, gradient and scattering force, cause the particle to move into different directions but only gradient forces lead to a stable optical trapping since they are pointing towards the region of highest intensity which is the focus of the laser beam. Scattering forces, in contrast, are pointing along the axis of the energy flux of the light beam. At a wavelength close to the particle resonance, scattering of light becomes strong and scattering forces dominant. Particles in this case are pushed and not trapped by the laser beam, even beyond the focal plane20,21.
A very stable trapping of the particle is a requirement to detect any small external microfluidic disturbance and to achieve an enhanced signal to noise ratio in the frequency spectrum from the time dependent particle displacement in the optical trap. At the same time, a high laser power can lead to substantial heating of the nanoparticle which could induce unwanted thermal effects including heating of the whole water sample. To achieve a distinct signal in the particle's Fourier space both factors have to be considered and the experiment optimized in such a way that heating effects are minimized but sufficient stable trapping is achieved. It is also important to point out that the conditions of the water sample, such as the temperature and pH, might have an impact on the viability of the larvae during the measurement, and that these factors thus need to be controlled and kept constant. We therefore performed all measurements at room temperature (~20 °C) and at a pH of around 7.5.
Overall, the method to detect the motion of Nauplius larvae by tracking the position of a single gold nanoparticle in an optical trap represents a non-invasive way to analyze the activity of the aquatic specimen without the requirement to disturb or even see the Nauplius during the measurement. Additionally, the direction of the microfluidic oscillations can be determined by analyzing the direction dependent Fourier spectrum of the nanoparticle's displacement. The optical tweezer configuration thus renders it possible to detect even small fluidic vibrations in an aqueous solution with high sensitivity. In the future, this approach could be extended to distinguish between different kinds of organisms in one water sample and at the same time. Furthermore, this approach of using a gold nanoparticle as a sensitive detector is not restricted to the measurement of Nauplius larvae only and can in principle be applied to measure any flow generated by much smaller objects, such as single cells and possibly even bacteria.
Disclosures
The authors declare that they have no competing financial interests.
Acknowledgments
Financial support by the ERC through the Advanced Investigator Grant HYMEM, by the DFG through the Nanosystems Initiative Munich (NIM) and through the Sonderforschungsbereich (SFB1032), project A8 is gratefully acknowledged. We are thankful to Dr. Alexander Ohlinger, Dr. Sol Carretero-Palacios and Spas Nedev for support and fruitful discussions.
References
- Hellawell JM. Biological indicators of freshwater pollution and environmental management. Elsevier Applied Science Publishers; 1986. [Google Scholar]
- Diamond JM, Barbour MT, Stribling JB. Characterizing and comparing bioassessment approaches and their results: A perspective. Journal of the North American Benthological Society. 1996;15:713–727. [Google Scholar]
- Carlisle DM, et al. The quality of our Nation’s waters—Ecological health in the Nation’s streams, 1993–2005. U.S. Geological Survey Circular. 2013;1391 [Google Scholar]
- Boxhall GA, Defaye D. Global diversity of copepods (Crustacea Copepoda) in freshwater. Hydrobiologia. 2008;595(1):195–207. [Google Scholar]
- Ferdous Z, Muktadir AKM. A Review: Potentiality of Zooplankton as Bioindicator. American Journal of Applied Sciences. 2009;6(10):1815–1819. [Google Scholar]
- Andersen Borg CM, Bruno E, Kiørboe T. The Kinematics of Swimming and Relocation Jumps in Copepod Nauplii. PLoS ONE. 2012;7(10) doi: 10.1371/journal.pone.0047486. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gilchrist BM. Growth and Form of the Brine Shrimp Artemia Salina. Journal of Zoology. 1960;134(2):221–235. [Google Scholar]
- Boone E, Baas-Becking LGM. Salt Effects on Egga and Nauplii of Artemia Salina L. Journal of General Physiology. 1931;14(6):453–763. doi: 10.1085/jgp.14.6.753. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Drewes C. Quantitative investigations of hatching in brine shrimp cysts. Association for Biology Laboratory Education. 2006;27:299–312. [Google Scholar]
- Williams TA. A model of rowing propulsion and the ontogeny of locomotion in Artemia larvae. Biological Bulletin. 1994;187:164–173. doi: 10.2307/1542239. [DOI] [PubMed] [Google Scholar]
- Croghan PC. The Mechanism of Osmotic Regulation in the Artemia Salina (L.): The Physiology of the Branchiae. Journal of Experimental Biology. 1958;35:234–242. [Google Scholar]
- Ashkin A, Dziedzic JM, Bjorkholm JE, Chu S. Observation of a single-beam gradient force optical trap for dielectric particles. Optics Letters. 1986;11(5):288–290. doi: 10.1364/ol.11.000288. [DOI] [PubMed] [Google Scholar]
- Svoboda K, Block SM. Optical trapping of metallic Rayleigh particles. Optics Letters. 1994;19(13):930–932. doi: 10.1364/ol.19.000930. [DOI] [PubMed] [Google Scholar]
- Hansen PM, Bhatia VK, Harrit N, Oddershede L. Expanding the Optical Trapping Range of Gold Nanoparticles. Nano Letters. 2005;5(10):1937–1942. doi: 10.1021/nl051289r. [DOI] [PubMed] [Google Scholar]
- Ohlinger A, Deak A, Lutich AA, Feldmann J. Optically Trapped Gold Nanoparticle Enables Listening at the Microscale. Physical Review Letters. 2012;108(1) doi: 10.1103/PhysRevLett.108.018101. [DOI] [PubMed] [Google Scholar]
- Kuwata H, Tamaru H, Esumi K, Miyano K. Resonant light scattering particles: Practical analysis beyond Rayleigh approximation. Applied Physics Letters. 2003;83(22):4625–4628. [Google Scholar]
- Agayan RR, Gittes F, Kopelman R, Schmidt CF. Optical trapping near resonance absorption. Applied Optics. 2002;41(12):2318–2327. doi: 10.1364/ao.41.002318. [DOI] [PubMed] [Google Scholar]
- Klar T, Perner M, Grosse S, von Plessen G, Spirkl W, Feldmann J. Surface-Plasmon Resonances in Single Metallic Nanoparticles. Physical Review Letters. 1998;80:4249–4252. [Google Scholar]
- Ohlinger A, Nedev S, Lutich AA, Feldmann J. Optothermal Escape of Plasmonically Coupled Silver Nanoparticles from a Three-Dimensional Optical Trap. Nano Letters. 2011;11(4):1770–1774. doi: 10.1021/nl2003544. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Urban AS, Lutich AA, Stefani FD, Feldmann J. Laser Printing Single Gold Nanoparticles. Nano Letters. Nano Letters. 2010;10(12):4794–4798. doi: 10.1021/nl1030425. [DOI] [PubMed] [Google Scholar]
- Urban AS, Fedoru KM, Nedev S, Lutich A, Lohmueller T, Feldmann J. Shrink-to-fit Plasmonic Nanostructures. Advanced Optical Materials. 2013;1(2):123–127. [Google Scholar]


