Abstract
Muscle architecture is a determinant for sprinting speed and jumping power, which may be related to anaerobic sports performance. In the present investigation, the relationships between peak (PVJP) and mean (MVJP) vertical jump power, 30m maximal sprinting speed (30M), and muscle architecture were examined in 28 college-aged, recreationally-active men (n = 14; 24.3 ± 2.2y; 89.1 ± 9.3kg; 1.80 ± 0.07 m) and women (n = 14; 21.5 ± 1.7y; 65.2 ± 12.4kg; 1.63 ± 0.08 m). Ultrasound measures of muscle thickness (MT), pennation angle (PNG), cross-sectional area (CSA), and echo intensity (ECHO) were collected from the rectus femoris (RF) and vastus lateralis (VL) of both legs; fascicle length (FL) was estimated from MT and PNG. Men possessed lower ECHO, greater muscle size (MT & CSA), were faster, and were more powerful (PVJP & MVJP) than women. Stepwise regression indicated that muscle size and quality influenced speed and power in men. In women, vastus lateralis asymmetry negatively affected PVJP (MT: r = –0.73; FL: r = –0.60) and MVJP (MT: r = –0.76; FL: r = –0.64), while asymmetrical ECHO (VL) and FL (RF) positively influenced MVJP (r = 0.55) and 30M (r = 0.57), respectively. Thigh muscle architecture appears to influence jumping power and sprinting speed, though the effect may vary by gender in recreationally-active adults. Appropriate assessment of these ultrasound variables in men and women prior to training may provide a more specific exercise prescription.
Key points.
The manner in which thigh muscle architecture affects jumping power and sprinting speed varies by gender.
In men, performance is influenced by the magnitude of muscle size and architecture.
In women, asymmetrical muscle size and architectural asymmetry significantly influence performance.
To develop effective and precise exercise prescription for the improvement of jumping power and/or sprinting speed, muscle architecture assessment prior to the onset of a training program is advised.
Key words: Sports testing, ultrasonography, vertical jump, 30m sprint, muscle symmetry
Introduction
Vertical jump and short distance sprints (< 50m) are convenient field assessment tools used to rate athletic capability in anaerobic activities (Hoffman, 2006). Briefly, vertical jump power and sprinting speed appear to be interrelated predictors of explosive and accelerative capability (Cronin and Hansen, 2005; Habibi et al., 2010; Kale et al., 2009; Maulder et al., 2006; Nesser et al., 1996) and indicative of a variety of game-related performance statistics for several anaerobic sports (Davis et al., 2004; Hoffman et al., 2010; Mangine et al., 2013; McGill et al., 2012). While several factors may contribute, muscular strength and power are essential components in the optimal performance of these tests (Hoffman, 2006). Furthermore, it is largely accepted that muscular strength and power are significantly determined by the structural characteristics of the activated musculature; where greater muscle size, muscle quality, fascicle length, and pennation angles lead to greater force and power production (Cadore et al., 2012; Cormie et al., 2011; Goodpaster et al., 2000; Häkkinen and Keskinen, 1989, Häkkinen et al., 1996; Kumagai et al., 2000; Potteiger et al., 1999). As such, several studies have determined that muscle structure is related to vertical jump and sprinting performance (Abe et al., 2000; 2001; Bell et al., 2014; Ikebukuro et al., 2011; Kubo et al., 2011; Kumagai et al., 2000; Potteiger et al., 1999).
Jumping and sprint performance is dependent upon the synchronized contribution of force and power from each leg. It is known that the combined effort from each leg is not as great as the sum of each leg measured individually (Bobbert et al., 2006; Taniguchi, 1998). Yet, the magnitude and direction of each leg’s force and power is combined to produce a single, linear force/power vector (McGinnis, 2013). Therefore, a vertical jump or linear sprint may suffer from sub-optimal linear propulsion if these forces are unequal or misaligned. Recently, Bell and colleagues (2014) demonstrated relationships between lower limb lean mass asymmetry and asymmetrical jumping force and power (Bell et al., 2014). However, this particular investigation only examined the effect these asymmetries had on maximal jumping height. No study has looked into the effect of asymmetrical muscle architecture (e.g. pennation angle and fascicle length), muscle size, or muscle quality on absolute jumping power or sprinting speed.
To a certain degree, skeletal muscle architecture and its activation are both responsible for the resultant production of force and power (Hoffman, 2006). The manner in which muscle architecture and activation affect force and power may be influenced by physical activity, training status, and gender. During puberty, skeletal structure and circulating testosterone, which affect muscle strength and development, differ significantly between men and women (Komi and Bosco, 1978; Komi and Karlsson, 1978; Saladin, 2009). As a result, absolute differences in strength, rate of force development, explosive capability, muscle recruitment, and muscular symmetry have been previously documented between genders (Hewitt et al., 1999; Komi and Bosco, 1978; Komi and Karlsson, 1978; Lawson et al., 2006; Rodano et al., 1996). It is possible that the manner in which muscle architecture affects vertical jumping and sprinting performance may also vary by gender. Therefore, the purpose of the present investigation was to determine the influence of gender and muscle architecture asymmetry on vertical jump power and short-distance sprinting speed.
Methods
The relationships between skeletal muscle architecture, as measured by ultrasound, and athletic field tests (vertical jump & 30m sprint) were assessed in physically active men and women. Participants reported to the Human Performance Laboratory on three separate occasions. On the first visit (T1), eligible participants were advised of the purpose, risks and benefits associated with the study. After providing their informed consent, body composition was measured in all eligible participants. Within 1 – 2 days of T1, participants returned for the second visit (T2), during which a standardized warm-up preceded the assessment of vertical jump height and power. The final visit (T3) occurred within one week from T2. Following ultrasound measurements of the right and left leg thigh musculature (rectus femoris and vastus lateralis), the participants completed three maximal 30-m sprints. The Institutional Review Board of the University approved this research protocol.
Subjects
Twenty-eight healthy, physically active men (n = 14) and women (n = 14) (Table 1), volunteered to participate in this study. Participants completed a health and physical activity questionnaire, Physical Activity Readiness Questionnaire (PAR-Q), and an informed consent prior to participation. All participants were free of any physical limitations and had been recreationally active (exercised 2 – 3 times per week).
Table 1.
Gender differences in descriptive and performance variables. Data are means (±SD).
| Men | Women | |
|---|---|---|
| Descriptive Measures | ||
| Age (y) | 24.3 (2.2) | 21.5 (1.7)* |
| Body Mass (kg) | 89.1 (9.3) | 65.2 (12.4)* |
| Height (m) | 1.80 (.07) | 1.63 (.08)* |
| Body Fat (%) | 13.7 (5.0) | 25.2 (6.3)* |
| Lean Body Mass (kg) | 76.9 (9.1) | 48.2 (6.0)* |
| Performance Measures | ||
| Vertical Jump (cm) | 66.8 (10.2) | 45.2 (6.5)* |
| Peak Vertical Jump Power (W) | 2663 (547) | 1513 (310)* |
| Mean Vertical Jump Power (W) | 1421 (231) | 868 (167)* |
| 30-m Sprint Rate (m·s–1) | 6.65 (.37) | 5.51 (.49)* |
*Significantly (p < 0.05) different from men.
Descriptive measures
During T1, height (±0.1cm) and body mass (±0.1kg) were measured using a Health-o-meter Professional scale (Patient Weighing Scale, Model 500 KL, Pelstar, Alsip, IL, USA). Skinfold measurements were collected by the same investigator with skinfold calipers (Caliper-Skinfold-Baseline, Model #MDSP121110, Medline, Mundelein, IL, USA) using standardized procedures for measurement of the triceps, suprailiac, abdomen, and thigh (Hoffman, 2006). Previously published formulas were used to calculate body fat percentage (%FAT) (Jackson and Pollock, 1985).
Vertical jump assessment
Following a 5-min warm-up on a cycle ergometer, vertical jump height was measured using a Vertical Jump Testing station (Uesaka Sport, Colorado Springs, CO) during T2. Before testing, standing vertical reach height was determined by colored squares located along the vertical neck of the device. Each square corresponded with similarly colored markings on each horizontal tab, which indicated the vertical distance (in inches) from the associated square. Vertical jump (VJ) height was determined by the indicated distance on the highest tab reached following 3 maximal countermovement jump attempts and in accordance with previously described procedures (Hoffman, 2006). Peak (PVJP) and mean (MVJP) vertical jump power were determined from a Tendo™ Weightlifting Analyzer (Tendo Sports Machines, Trencin, Slovakia) that was attached at the participant’s waist during the vertical jump assessment. The Tendo™ unit consists of a transducer that measured velocity (m·s–1), defined as linear displacement over time. Power (W) was calculated by multiplying vertical jump velocity and the participant’s body mass. PVJP and MVJP were recorded for each jump and used for subsequent analysis. The ICCs for PVJP and MVJP, as measured by the Tendo™ unit in our laboratory, are 0.98 (SEM = 106.2 W) and 0.94 (SEM = 100.3 W), respectively.
Measurements of muscle architecture
Prior testing on T3, participants reported to the laboratory and laid supine for 15 minutes to allow fluid shifts to occur before the collection of ultrasound images (Berg et al., 1993). Subsequently, non-invasive skeletal muscle ultrasound images were collected from the rectus femoris (RF) and vastus lateralis (VL). A 12 MHz linear probe scanning head (General Electric LOGIQ P5, Wauwatosa, WI, USA) was used to optimize spatial resolution and was coated with water soluble transmission gel and positioned on the surface of the skin to provide acoustic contact without depressing the dermal layer to collect the image. All measures were taken in both the RF and VL of both legs and performed by the same technician. The anatomical location for all ultrasound measures was standardized for each muscle in all participants. For measures of RF, the participant was placed supine on an examination table, according to the American Institute of Ultrasound in Medicine, with the legs extended but relaxed and with a rolled towel beneath the popliteal fossa allowing for a 10° bend in the knee as measured by a goniometer (Scanlon et al., 2014). For measures of the VL, the participant was placed on their side with the legs together and relaxed allowing for a 10° bend in the knee as measured by a goniometer. Following scanning, all images were analyzed offline using ImageJ (National Institutes of Health, Bethesda, MD, USA, version 1.45s), an image analysis software available through the National Institute of Health. For these analyses, a known distance of 1cm shown in the image was used to calibrate the software program (Scanlon et al., 2014).
Measures of muscle cross-sectional area (CSA) and echo intensity (ECHO) were obtained using a sweep of the muscle in the extended field of view mode with gain set to 50 dB and image depth to 5cm. For both muscles, landmark measurements were taken in the sagittal plane parallel to the long axis of the femur, while scanning occurred in the axial plane, perpendicular to the tissue interface. For the RF, scanning occurred at 50% of the distance between the anterior, inferior suprailiac crest to the proximal border of the patella. For the VL, scanning occurred at 50% of the distance from the most prominent point of the greater trochanter to the lateral condyle. Three consecutive images were analyzed and averaged using the polygon tracking tool in the ImageJ software to obtain as much lean muscle as possible without any surrounding bone or fascia for CSA (Cadore et al., 2012). The ICCs for rectus femoris CSA and vastus lateralis CSA were 0.97 (SEM = 0.55 cm2) and 0.99 (SEM = 0.49 cm2), respectively. Concurrently, ECHO was determined by grayscale analysis using the standard histogram function in ImageJ (Cadore et al., 2012). ECHO in the measured area was expressed as an arbitrary unit (au) value between 0 – 255 (0: black; 255: white) with an increase in ECHO reflecting an increase in intramuscular connective and adipose tissue relative to lean skeletal muscle (Scanlon et al., 2013, Cadore et al., 2012). ICCs were 0.93 (SEM = 3.80 au) for rectus femoris ECHO and 0.96 (SEM = 2.26 au) for vastus lateralis ECHO.
For both muscles, measures of muscle thickness (MT) and pennation angle (PNG) were obtained from images, taken at the same site described for CSA, but with the probe oriented longitudinal to the muscle tissue interface using Brightness Mode (B-mode) ultrasound (Cadore et al., 2012). Within each muscle, MT was measured perpendicularly from the superficial aponeurosis to the deep aponeurosis. Three consecutive images were analyzed and averaged offline (Thomaes et al., 2012). ICCs for rectus femoris MT and vastus lateralis MT were 0.93 (SEM = 0.08 cm) and 0.98 (SEM = 0.03 cm), respectively. PNG was determined as the intersection of the fascicles with the deep aponeurosis. ICCs for rectus femoris PNG and vastus lateralis PNG were 0.88 (SEM = 1.3°) and 0.87 (SEM = 0.8°), respectively. For both the RF and VL, fascicle length (FL) across the deep and superficial aponeuroses was estimated using MT and PNG. Previously, this methodology for determination of FL has a reported estimated coefficient of variation of 4.7% (Kumagai et al., 2000) and can be found using Equation (1) (Kumagai et al., 2000).
| FL = MT · sin (PNG)–1 | Equation 1 |
For statistical analyses, CSA in the vastus lateralis was used as the determinant of limb dominance, where the limb with the larger CSA was referred to as the dominant (DOM) limb and the opposite limb being non-dominant (ND). The absolute percent difference between limbs (%DIFF: | (DOM – ND) |/ ((DOM + ND) / 2) x 100) was also calculated.
Sprint testing
An electronically-timed 30-m sprint was used to determine maximal sprinting speed on T3. Prior to the sprint, all participants performed a dynamic warm-up that included light jogging for 5 minutes followed by several exercises that included body weight squats, walking lunges, walking knee hugs, and walking quadriceps stretch (one set of 10 repetitions were performed for each exercise). Sprint times were measured using an infrared testing device (Speed Trap II; Brower Timing Systems, Draper, UT, USA) and performed on a hardwood indoor basketball court. Participants were instructed how to begin the sprint from a 3-point stance. Timing began on the participant’s first movement and concluded when he/she sprinted past the infrared sensor located 30-m from the starting point. The best of 3 attempts, separated by 2 – 3 minutes, was recorded as the participant’s fastest time and converted to sprinting rate (30M: 30-m sprint time / 30 meters, m·s–1).
Statistical analysis
A statistical software package JMP Pro (v10, SAS Institute Inc., Cary, NC) was used for all analyses. Prior to relationship analyses, the Shapiro-Wilk test was used to satisfy the assumption of normality for all dependent variables (PVJP, MVJP, & 30M), while a t-test was used to determine whether significant differences existed between men and women for all descriptive and architectural measures (DOM, ND, & %DIFF); within genders, significant differences between DOM and ND were also examined; these data are expressed as mean ± SD. Subsequently, Pearson correlation coefficients were calculated to quantify the relationships between each dependent variable and each measure of muscle architecture (DOM, ND & %DIFF) by gender. Additionally, stepwise regression was used to determine the influence of architectural asymmetry on performance (PVJP, MVJP, & 30M) for each gender. For this procedure, the minimum Bayesian Information Criterion (BIC) technique was used to choose the best model; for each model, the root mean square error (RMSE) is reported. For all analyses, a criterion alpha level of p ≤ 0.05 was used to determine statistical significance.
Results
Significant differences (p < 0.001) between men and women were observed in all performance and descriptive measures (Table 1). Significant differences between men and women were also observed in rectus femoris [MTDOM (p < 0.001), MTND (p < 0.001), FLND (p = 0.004), CSADOM (p < 0.001), CSAND (p < 0.001), ECHOND (p = 0.002)] and vastus lateralis architecture [PNGND (p = 0.044), CSADOM (p < 0.001), CSAND (p < 0.001), ECHODOM (p = 0.033), ECHOND (p = 0.009)]. Additionally, a significant difference in vastus lateralis CSA (p = 0.028) was observed between limbs in women. No other differences were observed (Table 2).
Table 2.
Gender and bilateral differences in dominant and non-dominant limb architecture. Data are means (±SD).
| Rectus Femoris | Vastus Lateralis | ||||||
|---|---|---|---|---|---|---|---|
| DOM | ND | %DIFF | DOM | ND | %DIFF | ||
| Muscle Thickness (cm) | Men | 2.9 (.3 | 2.9 ± 0.3) | 1.8 (1.5) | 1.9 (.4) | 1.9 (.4) | 1.9 (1.5) |
| Women | 2.4 (.2) * | 2.4 (.3) * | 1.1 (1.5) | 1.8 (.3) | 1.8 (.4) | 4.2 (4.1) | |
| Pennation Angle (°) | Men | 16.4 (3.4) | 15.5 (3.0) | 3.9 (2.7) | 13.6 (3.0) | 14.3 (2.3) | 4.8 (4.8) |
| Women | 15.4 (2.3) | 16.4 (2.4) | 4.3 (2.8) | 12.6 (3.4) | 12.2 (2.8) * | 3.8 (3.5) | |
| Fascicle Length (cm) | Men | 10.8 (3.2) | 11.4 (2.5) | 4.1 (3.5) | 8.4 (1.9) | 7.9 (1.7) | 6.0 (4.6) |
| Women | 9.2 (1.4) | 8.7 (1.9) * | 4.7 (3.4) | 8.9 (3.0) | 8.8 (3.1) | 7.6 (5.9) | |
| Cross-Sectional Area (cm2) | Men | 19.9 (5.0) | 20.0 (3.3) | 2.9 (2.5) | 39.8 (7.3) † | 37.0 (8.1) | 2.0 (2.3) |
| Women | 14.0 (2.7) * | 13.7 (3.0) * | 2.7 (2.1) | 27.1 (3.7) * | 23.8 (3.8) * | 3.3 (3.0) | |
| Echo Intensity (au) | Men | 58.0 (8.7) | 55.9 (8.3) | 2.4 (1.5) | 60.4 (12.6) | 62.4 (10.9) | 2.2 (1.6) |
| Women | 65.8 (11.9) | 66.0 (7.3) * | 1.8 (2.4) | 71.2 (12.8) * | 72.9 (8.5) * | 2.5 (1.9) | |
*Significantly (p < 0.05) different from men.
† Significantly (p < 0.05) different from the non-dominant leg.
‡ DOM = Dominant Limb; ND = Non-Dominant Limb; %DIFF = Absolute percent difference between limbs
Vertical jump performance
The relationships between muscle architecture and vertical jump power were different between men and women (Table 3). In men, PVJP and MVJP were similarly related to rectus femoris architecture (MTND, PNGDOM, FLDOM, & FLND). However, in relation to vastus lateralis architecture, the only significant relationship observed was seen between the dominant limb (MTDOM, CSADOM, & ECHODOM) and PVJP; no relationships were observed with MVJP and VL architecture. In women, PVJP was significantly related to rectus femoris PNGDOM and vastus lateralis (MTND, MT%DIFF, FLND, & FL%DIFF), while MVJP was related to rectus femoris (CSAND & ECHO%DIFF) and vastus lateralis (MTND, MT%DIFF, FLND, FL%DIFF, & ECHO%DIFF).
Table 3.
Relationships between vertical jump performance and muscle architecture in the dominant and non-dominant limbs for men (n=14) and women (n=14) [r (p-value)].
| Peak Vertical Jump Power | Mean Vertical Jump Power | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Men | Women | Men | Women | ||||||
| RF | VL | RF | VL | RF | VL | RF | VL | ||
| Muscle Thickness (cm) | Dominant | .44 (.16) | .35 (.20) | .30 (.38) | .15 (.57) | .31 (.32) | .11 (.72) | .47 (.14) | .24 (.38) |
| Non-Dominant | .66 (.01) | .55 (.04) | .36 (.27) | .81 (.00) | .48 (.04) | .37 (.17) | .51 (.10) | .78 (.00) | |
| %DIFF | .04 (.92) | .14 (.65) | .14 (.65) | -.73 (.00) | .11 (.73) | .31 (.29) | .40 (.16) | -.76 (.00) | |
| Pennation Angle (°) | Dominant | -.74 (.00) | .29 (.32) | .54 (.05) | .09 (.77) | -.71 (.01) | .03 (.95) | .42 (.13) | -.02 (.95) |
| Non-Dominant | -.24 (.40) | -.05 (.85) | .16 (.60) | -.33 (.26) | -.34 (.24) | -.08 (.80) | -.01 (.95) | -.44 (0.12) | |
| %DIFF | -.26 (.37) | -.08 (.80) | -.42 (.13) | -.25 (.39) | -.41 (.15) | -.23 (.42) | -.10 (0.74) | -.27 (.35) | |
| Fascicle Length (cm) | Dominant | .83 (.00) | .05 (.86) | -.37 (.19) | -.1 (.73) | .67 (.01) | .06 (.85) | -.15 (.62) | .08 (.79) |
| Non-Dominant | .62 (.02) | .53 (.05) | .12 (.68) | .65 (.01) | .54 (.05) | .36 (.21) | .34 (.25) | .72 (.00) | |
| %DIFF | -.37 (.19) | -.13 (.65) | -.33 (.25) | -.6 (.02) | -.48 (.08) | -.23 (.43) | -.07 (0.81) | -.64 (.01) | |
| Cross-Sectional Area (cm2) | Dominant | .39 (.16) | .64 (.01) | .34 (.23) | .39 (.16) | .40 (.16) | .53 (0.05) | .37 (.20) | .25 (.39) |
| Non-Dominant | .52 (.05) | .5 (.07) | .51 (.06) | .35 (.22) | .40 (.15) | .47 (0.09) | .59 (0.03) | .25 (.38) | |
| %DIFF | -.26 (.36) | .11 (.73) | .1 (.74) | -.03 (.91) | -.23 (.42) | -.08 (.79) | .07 (.81) | -.08 (.79) | |
| Echo Intensity (au) | Dominant | -.36 (.21) | -.56 (.04) | -.42 (.14) | -.29 (.32) | -.07 (.82) | -.30 (.30) | -.44 (.11) | -.32 (.27) |
| Non-Dominant | -.07 (.81) | -.53 (.05) | -.32 (.26) | -.13 (.65) | .24 (.40) | -.29 (.31) | -.35 (.22) | -.14 (.64) | |
| %DIFF | -.48 (.09) | .38 (.18) | .36 (.21) | .30 (.31) | -.48 (.09) | .38 (.19) | .54 (.05) | .55 (.04) | |
%DIFF = Absolute percent difference between dominant and non-dominant limbs.
Stepwise regression revealed that PVJP was most influenced by rectus femoris ECHO%DIFF (R2 = 0.23, BIC = 219.6, RMSE = 501W, p = 0.087) in men (Figure 1A) and vastus lateralis MT%DIFF (R2 = 0.53, BIC = 196.7, RMSE = 222W, p = 0.003) in women (Figure 1B). For MVJP in men, rectus femoris FL%DIFF (R2 = 0.23, BIC = 195.3, RMSE = 211W, p = 0.082) was the most influential variable (Figure 2A). In women, MVJP was most influenced by vastus lateralis MT%DIFF (R2 = 0.58, BIC = 177.8, RMSE = 113W, p = 0.002) (Figure 2B).
Figure 1.
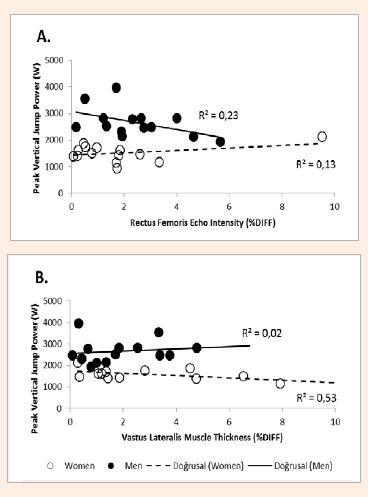
Relationships between peak vertical jump power and influential bilateral asymmetry by gender (A. Percent Difference in Rectus Femoris Echo Intensity; B. Percent Difference in Vastus Lateralis Muscle Thickness). Note: Solid Black Circles = Men; Open Circles = Women; Solid Black Line = Line of Best Fit for Men; Dashed Line = Line of Best Fit for Women
Figure 2.
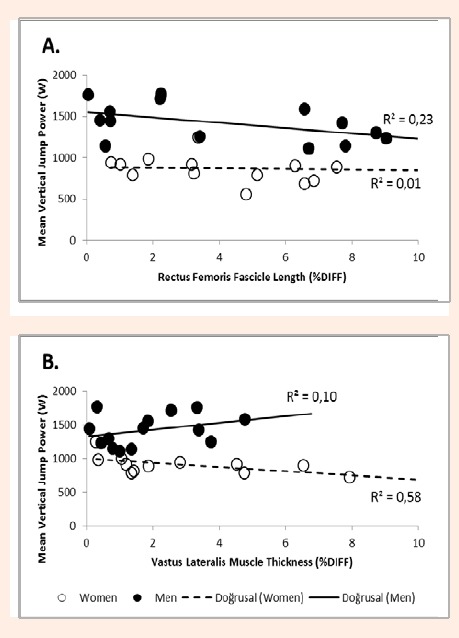
Bivariate relationships between mean vertical jump power and influential bilateral asymmetry by gender (A. Percent Difference in Rectus Femoris Fascicle Length; B. Percent Difference in Vastus Lateralis Muscle Thickness). Note: Solid Black Circles = Men; Open Circles = Women; Solid Black Line = Line of Best Fit for Men; Dashed Line = Line of Best Fit for Women
30m sprint speed
The data revealed 30M to be significantly (p < 0.05) related to rectus femoris architecture in men (PNGDOM, PNGND, FLDOM, & FLND) and women (FLND & FL%DIFF), but not to vastus lateralis architecture (Table 4).
Table 4.
Relationships between 30m sprint speed and muscle architecture in the dominant and non-dominant limbs in men (n=14) and women (n=14) [r (p-value)].
| Rectus Femoris | Vastus Lateralis | ||||
|---|---|---|---|---|---|
| Men | Women | Men | Women | ||
| Muscle Thickness (cm) | Dominant | .16 (.63) | .14 (.65) | .01 (.98) | -.34 (.24) |
| Non-Dominant | .29 (.27) | .32 (.27) | .22 (.37) | -.24 (.34) | |
| %DIFF | -.20 (.49) | .34 (.22) | .21 (.47) | .03 (.93) | |
| Pennation Angle (°) | Dominant | -.69 (.01) | .07 (.82) | -.04 (.89) | .50 (.07) |
| Non-Dominant | -.56 (.04) | -.50 (.07) | -.16 (.58) | .32 (.28) | |
| %DIFF | .10 (.74) | .49 (.08) | -.05 (.87) | .35 (.22) | |
| Fascicle Length (cm) | Dominant | .54 (.05) | .02 (.94) | .06 (.85) | -.50 (.07) |
| Non-Dominant | .65 (.01) | .59 (.03) | .29 (.31) | -.32 (.26) | |
| %DIFF | -.10 (.74) | .57 (.03) | -.11 (.72) | .17 (.56) | |
| Cross-Sectional Area (cm2) | Dominant | -.01 (.98) | .07 (.80) | .44 (.12) | .16 (.58) |
| Non-Dominant | -.20 (.51) | -.07 (.82) | .44 (.12) | .40 (.16) | |
| %DIFF | -.48 (.08) | .42 (.13) | -.17 (.55) | -.38 (.18) | |
| Echo Intensity (au) | Dominant | -.02 (.94) | .39 (.17) | -.38 (.19) | .20 (.50) |
| Non-Dominant | -.02 (.95) | .44 (.12) | -.48 (.08) | .09 (.75) | |
| %DIFF | .02 (.92) | -.29 (.32) | -.25 (.38) | -.01 (.99) | |
‡ %DIFF = Absolute percent difference between dominant and non-dominant limbs.
Stepwise regression also indicated, 30M is most influenced by rectus femoris CSA%DIFF (R2 = 0.23, BIC = 15.09, RMSE = 0.34s, p = 0.081) in men (Figure 3A) and rectus femoris FL%DIFF (R2 = 0.32, BIC = 21.3, RMSE = 0.42s, p = 0.034) in women (Figure 3B).
Figure 3.
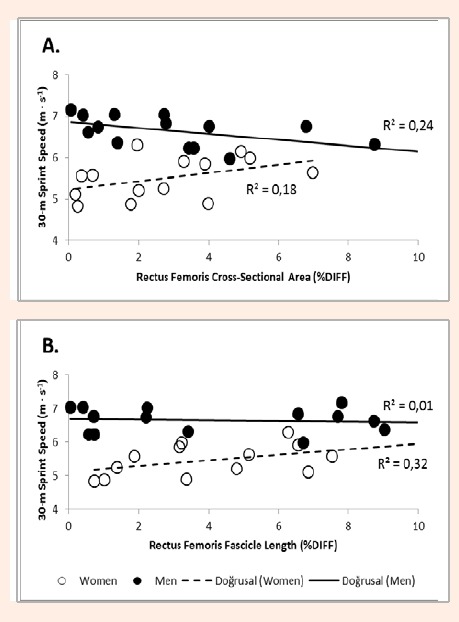
Bivariate relationships between 30-m sprint speed and influential bilateral asymmetry by gender (A. Percent Difference in Vastus Lateralis Muscle Thickness; B. Percent Difference in Rectus Femoris Echo Intensity). Note: Solid Black Circles = Men; Open Circles = Women; Solid Black Line = Line of Best Fit for Men; Dashed Line = Line of Best Fit for Women
Discussion
The present investigation provides evidence that the relationships between skeletal muscle structure and anaerobic performance measures (jumping power and sprinting speed) may vary by gender in a recreationally active population. Following puberty, substantial differences in absolute muscular mass, strength, and quality have been reported to favor men over women (Arts et al., 2010; Komi and Bosco, 1978; Komi and Karlsson, 1978; Saladin, 2009), while relative measures of muscle function (e.g. quality) remain equal. Our data supports these ideas as men in our study possessed greater muscle mass (as reflected by greater MT & CSA than women) and lower ECHO (RFND, VLDOM, & VLND), which are known to influence force-generating capability (Cadore et al., 2012; Cormie et al., 2011; Goodpaster et al., 2001; Häkkinen et al., 1996; Häkkinen and Keskinen, 1989; Potteiger et al., 1999). Although strength was not measured in our study, greater muscle mass has also been linked to jumping power (Potteiger et al., 1999) and sprinting performance (Ikebukuro et al., 2011, Kubo et al., 2011). Our results appear to support these studies as men produced greater jumping power and completed the 30M in less time than women.
As for fascicle arrangement (PNG & FL), no differences were observed between genders. However, the manner in which these measures influenced performance between the genders was different. For men, performance (PVJP, MVJP, & 30M) was inversely related to PNG and positively related to FL. In contrast, only positive relationships between fascicle arrangement (PNG & FL) and performance (PVJP & 30M) were observed in women. It appears that the contribution of force production outweighs contraction speed in women, while men possess a more balanced contribution (Cormie et al., 2011). Regardless, the observed gender similarities and differences appear to corroborate previous reports on gender-specific muscle characteristics in relation to sprinting and jumping (Abe et al., 2001; Hewitt et al., 1999; Kumagai et al., 2000; Lawson et al., 2006; Rodano et al., 1996).
Jumping and sprinting performance are both associated with power, which is the product of force and velocity (Cronin and Hansen, 2005; Habibi et al., 2010; Kale et al., 2009; Maulder et al., 2006; McGinnis, 2013 Nesser et al., 1996). The magnitude of the force and velocity, produced by the activated musculature, are dependent upon the sum and direction of the force-generating vectors of each leg (McGinnis, 2013). Consequently, asymmetrical force production will result in a force magnitude or direction that is biomechanically sub-optimal, which may affect jumping power and sprinting speed. Recently, Bell and colleagues (2014) demonstrated how lean mass asymmetry was in part related to jumping force and power asymmetry (Bell et al., 2014). Our data expands upon those findings by providing evidence that architectural asymmetry affects vertical jumping power and sprinting speed in women, while men were influenced by the magnitude, and not the asymmetry, of muscle architecture.
In women, jumping power was negatively affected by asymmetrical architecture (MT & FL) in the vastus lateralis, while asymmetrical ECHO (VL) and FL (RF) positively influenced MVJP and 30M, respectively. While the former observed relationships are consistent with the literature (Cormie et al., 2011; Häkkinen and Keskinen, 1989; Häkkinen et al., 1996), the latter observations appear to be contradictory. However, they may also be the result of physical activity and/or limb preference (Sipila and Suominen, 1991; Kearns et al., 2001). Both contractile (i.e. actin and myosin) and non-contractile components (e.g. glycogen, water, enzymes, etc.) contribute to muscle size (Tesch, 1988, Saladin, 2009). When muscle size is measured by ultrasound (MT & CSA), these components are not distinguished, leaving force capability only partially explained by muscle size (Cormie et al., 2011; Goodpaster et al., 2001; Häkkinen and Keskinen, 1989; Häkkinen et al., 1996; Potteiger et al., 1999). In contrast, ECHO is reflective of both contractile and non-contractile components, which respond specifically to training programs (Scanlon et al., 2014; Tesch, 1988). Therefore, differences in fascicle length may develop in response to the manner in which each leg is regularly utilized. The results of this study appear to indicate that recreationally-active women tend to rely on a single limb for jumping and sprinting, which may result in potential asymmetries in muscle quality and fascicle length. Nevertheless, the influence of architecture only appears to partially (23 – 58%) explain performance, which supports previous work (Bell et al., 2014). Consequently, future investigations should consider the influence of bilateral activation, in addition to architecture, in order to explain the individual influence of each limb on jumping and sprinting in both men and women.
Conclusion
Muscle architecture of the thigh appears to be related to jumping power and sprinting speed. However, the manner in which it affects performance appears to vary between men and women. In men, jump and sprint performance is influenced by the magnitude of muscle architecture. While this is true for women, asymmetry of these measures between limbs also influences performance. In women, asymmetrical muscle size negatively influences performance, while asymmetrical muscle quality and fascicle length positively influences jumping power and sprinting speed, respectively. Muscle architecture assessment prior to the onset of a training program in individuals looking to improve jumping power or sprinting speed may enable coaches and trainers to provide a more effective and precise exercise prescription.
Acknowledgement
The authors of this manuscript certify that they have NO affiliations with or involvement in any organization or entity with any financial interest (such as honoraria; educational grants; participation in speakers’ bureaus; membership, employment, consultancies, stock ownership, or other equity interest; and expert testimony or patent-licensing arrangements), or non-financial interest (such as personal or professional relationships, affiliations, knowledge or beliefs) in the subject matter or materials discussed in this manuscript.
Biographies

Gerald T. MANGINE
Employment
The University of Central Florida
Degree
BSc, MEd
Research interest
Sports Science
E-mail: Gerald.mangine@ucf.edu

David H. FUKUDA
Employment
The University of Central Florida
Degree
PhD
Research interest
Sports Science
E-mail: david.fukuda@ucf.edu

Michael B. LaMONICA
Employment
The University of Central Florida
Degree
BSc
Research interest
Sports Science
E-mail: lamonica@knights.ucf.edu

Adam M. GONZALEZ
Employment
The University of Central Florida
Degree
BSc, MEd
Research interest
Sports Science
E-mail: adam.gonzalez@ucf.edu

Adam J. WELLS
Employment
The University of Central Florida
Degree
BSc, MS
Research interest
Sports Science
E-mail: adam.wells@ucf.edu

Jeremy R. TOWNSEND
Employment
The University of Central Florida
Degree
BSc, MS
Research interest
Sports Science
E-mail: Jeremy.townsend@ucf.edu

Adam R. JAJTNER
Employment
The University of Central Florida
Degree
BSc, MS
Research interest
Sports Science
E-mail: adam.jajtner@knights.ucf.edu

Maren S. FRAGALA
Employment
The University of Central Florida
Degree
PhD
Research interest
Sports Science
E-mail: maren.fragala@ucf.edu

Jeffrey R. STOUT
Employment
The University of Central Florida
Degree
PhD
Research interest
Sports Science
E-mail: Jeffrey.stout@ucf.edu

Jay R. HOFFMAN
Employment
The University of Central Florida
Degree
PhD
Research interest
Sports Science
E-mail: jay.hoffman@ucf.edu
References
- Abe T., Fukashiro S., Harada Y., Kawamoto K. (2001) Relationship between sprint performance and muscle fascicle length in female sprinters. Journal of Physiological Anthropology and Applied Human Science 20, 141-147. [DOI] [PubMed] [Google Scholar]
- Abe T., Kumagai K., Brechue W.F. (2000) Fascicle length of leg muscles is greater in sprinters than distance runners. Medicine and Science in Sports and Exercise 32, 1125-1129. [DOI] [PubMed] [Google Scholar]
- Arts I., Pillen S., Schelhaas H.J., Overeem S., Zwarts M.J. (2010) Normal values for quantitative muscle ultrasonography in adults. Muscle & Nerve 41, 32-41. [DOI] [PubMed] [Google Scholar]
- Bell D.R., Sanfilippo J., Binkley N., Heiderscheit B.C. (2014) Lean Mass Asymmetry Influences Force and Power Asymmetry During Jumping in Collegiate Athletes. Journal of Strength and Conditioning Research 28 (4), 884-891. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Berg H., Tedner B., Tesch P. (1993) Changes in lower limb muscle cross-sectional area and tissue fluid volume after transition from standing to supine. Acta Physiologica Scandinavica 148, 379-385. [DOI] [PubMed] [Google Scholar]
- Bobbert M.F., De Graaf W.W., Jonk J.N., Casius L.R. (2006) Explanation of the bilateral deficit in human vertical squat jumping. Journal of Applied Physiology 100, 493-499. [DOI] [PubMed] [Google Scholar]
- Cadore E.L., Izquierdo M., Conceiçä M., Radaelli R., Pinto R.S., Baroni B.M., Vaz M. A., Alberton C.L., Pinto S.S., Cunha G. (2012) Echo intensity is associated with skeletal muscle power and cardiovascular performance in elderly men. Experimental Gerontolog, 47, 473-478. [DOI] [PubMed] [Google Scholar]
- Cormie P., McGuigan M.R., Newton R.U. (2011) Developing maximal neuromuscular power. Sports Medicine 41, 17-38. [DOI] [PubMed] [Google Scholar]
- Cronin J.B., Hansen K.T. (2005) Strength and power predictors of sports speed. Journal of Strength and Conditioning Research 19, 349-357. [DOI] [PubMed] [Google Scholar]
- Davis D.S., Barnette B.J., Kiger J.T., Mirasola J.J., Young S.M. (2004) Physical characteristics that predict functional performance in Division I college football players. Journal of Strength and Conditioning Research 18, 115-120. [DOI] [PubMed] [Google Scholar]
- Goodpaster B.H., Carlson C.L., Visser M., Kelley D.E., Scherzinger A., Harris T.B., Stamm E., Newman A.B. (2001) Attenuation of skeletal muscle and strength in the elderly: The Health ABC Study. Journal of Applied Physiology 90, 2157-2165. [DOI] [PubMed] [Google Scholar]
- Goodpaster B.H., Kelley D.E., Thaete F.L., He J., Ross R. (2000) Skeletal muscle attenuation determined by computed tomography is associated with skeletal muscle lipid content. Journal of Applied Physiology 89, 104-110. [DOI] [PubMed] [Google Scholar]
- Habibi A., Shabani M., Rahimi E., Fatemi R., Najafi A., Analoei H., Hosseini M. (2010) Relationship between Jump Test Results and Acceleration Phase of Sprint Performance in National and Regional 100m Sprinters. Journal of Human Kinetics 23, 29-35. [Google Scholar]
- Häkkinen K., Keskinen K. (1989) Muscle cross-sectional area and voluntary force production characteristics in elite strength-and endurance-trained athletes and sprinters. European Journal Of Applied Physiology and Occupational Physiology 59, 215-220. [DOI] [PubMed] [Google Scholar]
- Häkkinen K., Kraemer W.J., Kallinen M., Linnamo V., Pastinen U. M., Newton R.U. (1996) Bilateral and unilateral neuromuscular function and muscle cross-sectional area in middle-aged and elderly men and women. The Journals of Gerontology Series A: Biological Sciences and Medical Sciences 51, B21-B29. [DOI] [PubMed] [Google Scholar]
- Hewitt T., Lindenfeild T., Riccobene J., Noyes F. (1999) The effects of neuromuscular training on the incidence of knee injury in female athletes. American Journal of Sports Medicine 27, 699-704. [DOI] [PubMed] [Google Scholar]
- Hoffman J.R. (2006) Norms for Fitness, Performance, And Health, Human Kinetics. [Google Scholar]
- Hoffman J.R., Vasquez J., Pichardo N. (2010) Anthropometric And Performance Comparisons In Professional Baseball Players. Journal of Strength and Conditioning Research 23(8), 2173-2178. [DOI] [PubMed] [Google Scholar]
- Ikebukuro T., Kubo K., Okada J., Yata H., Tsunoda N. (2011) The Relationship Between Muscle Thickness in the Lower Limbs and Competition Performance in Weightlifters and Sprinters. Japanese Journal of Physical Fitness and Sports Medicine 60, 401-411. [Google Scholar]
- Jackson A.S., Pollock M.L. (1985) Practical assessment of body composition. Physician and Sports Medicine 13, 76-90. [DOI] [PubMed] [Google Scholar]
- Kale M., Asçi A., Bayrak C., Açikada C. (2009) Relationships among jumping performances and sprint parameters during maximum speed phase in sprinters. Journal of Strength and Conditioning Research, 23, 2272-2279. [DOI] [PubMed] [Google Scholar]
- Kearns C.F., Isokawa M., Abe T. (2001) Architectural characteristics of dominant leg muscles in junior soccer players. European Journal of Applied Physiology 85, 240-243. [DOI] [PubMed] [Google Scholar]
- Komi P., Bosco C. (1978) Utilization of stored elastic energy in leg extensor muscles by men and women. Medicine & Science in Sports 10, 261-265. [PubMed] [Google Scholar]
- Komi P., Karlsson J. (1978) Skeletal muscle fiber types, enzyme activities and physical performance in young males and females. Acta Physiological Scandinavica, 103, 210-218. [DOI] [PubMed] [Google Scholar]
- Kubo K., Ikebukuro T., Yata H., Tomita M., Okada M. (2011) Morphological and mechanical properties of muscle and tendon in highly trained sprinters. Journal of Applied Biomechanics, 27, 336. [DOI] [PubMed] [Google Scholar]
- Kumagai K., Abe T., Brechue W.F., Ryushi T., Takano S., Mizuno M. (2000) Sprint performance is related to muscle fascicle length in male 100-m sprinters. Journal of Applied Physiology 88, 811-816. [DOI] [PubMed] [Google Scholar]
- Lawson B.R., Stephens II T.M., Devoe D.E., Reiser II R.F. (2006) Lower-extremity bilateral differences during step-close and no-step countermovement jumps with concern for gender. Journal of Strength and Conditioning Research 20, 608-619. [DOI] [PubMed] [Google Scholar]
- Mangine G. T., Hoffman J. R., Vazquez J., Pichardo N., Fragala M. S., Stout J. R. (2013) Predictors of Fielding Performance in Professional Baseball Players. International Journal of Sports Physiology and Performance 8 (5), 510-516. [DOI] [PubMed] [Google Scholar]
- Maulder P.S., Bradshaw E.J., Keogh J. (2006) Jump kinetic determinants of sprint acceleration performance from starting blocks in male sprinters. Journal of Sports Science and Medicine 5, 359-366. [PMC free article] [PubMed] [Google Scholar]
- McGill S.M., Andersen J.T., Horne A.D. (2012) Predicting performance and injury resilience from movement quality and fitness scores in a basketball team over 2 years. Journal of Strength and Conditioning Research 26, 1731-1739. [DOI] [PubMed] [Google Scholar]
- McGinnis P. (2013) Biomechanics of Sport and Exercise. Human Kinetics. [Google Scholar]
- Nesser T.W., Latin R.W., Berg K., Prentice E. (1996) Physiological determinants of 40-meter sprint performance in young male athletes. Journal of Strength and Conditioning Research 10, 263-267. [Google Scholar]
- Potteiger J.A., Lockwood R.H., Haub M.D., Dolezal B.A., Almuzaini K.S., Schroeder J.M., Zebas C.J. (1999) Muscle power and fiber characteristics following 8 weeks of plyometric training. Journal of Strength and Conditioning Research 13, 275-279. [Google Scholar]
- Rodano R., Squadrone R., Mingrino A. (1996) Gender differences in joint moment and power measurements during vertical jump exercises. Proceeding of XIV Symposium on Biomechanics in Sports, Madera, Portugal: 308-310. [Google Scholar]
- Saladin K. S. (2009). Anatomy and Physiology, McGraw-Hill College. [Google Scholar]
- Scanlon T.C., Fragala M.S., Stout J.R., Emerson N.S., Beyer K.S., Oliveira L.P., Hoffman J.R. (2014) Muscle architecture and strength: Adaptations to short-term resistance training in older adults. Muscle & Nerve 49(4), 584-592. [DOI] [PubMed] [Google Scholar]
- Sipila S., Suominen H. (1991) Ultrasound imaging of the quadriceps muscle in elderly athletes and untrained men. Muscle & Nerve 14, 527-533. [DOI] [PubMed] [Google Scholar]
- Taniguchi Y. (1998) Relationship between the modifications of bilateral deficit in upper and lower limbs by resistance training in humans. European Journal of Applied Physiology and Occupational Physiology 78, 226-230. [DOI] [PubMed] [Google Scholar]
- Tesch P. (1988) Skeletal muscle adaptations consequent to long-term heavy resistance exercise. Medicine and Science in Sports and Exercise 20, S132-134. [DOI] [PubMed] [Google Scholar]
- Thomaes T., Thomis M., Onkelinx S., Coudyzer W., Cornelissen V., Vanhees L. (2012) Reliability and validity of the ultrasound technique to measure the rectus femoris muscle diameter in older CAD-patients. BMC Medical Imaging 12, 7. [DOI] [PMC free article] [PubMed] [Google Scholar]


