The Large Genome Constraint Hypothesis: Evolution, Ecology and Phenotype

Abstract

• Background and Aims If large genomes are truly saturated with unnecessary ‘junk’ DNA, it would seem natural that there would be costs associated with accumulation and replication of this excess DNA. Here we examine the available evidence to support this hypothesis, which we term the ‘large genome constraint’. We examine the large genome constraint at three scales: evolution, ecology, and the plant phenotype.

• Scope In evolution, we tested the hypothesis that plant lineages with large genomes are diversifying more slowly. We found that genera with large genomes are less likely to be highly specious – suggesting a large genome constraint on speciation. In ecology, we found that species with large genomes are under-represented in extreme environments – again suggesting a large genome constraint for the distribution and abundance of species. Ultimately, if these ecological and evolutionary constraints are real, the genome size effect must be expressed in the phenotype and confer selective disadvantages. Therefore, in phenotype, we review data on the physiological correlates of genome size, and present new analyses involving maximum photosynthetic rate and specific leaf area. Most notably, we found that species with large genomes have reduced maximum photosynthetic rates – again suggesting a large genome constraint on plant performance. Finally, we discuss whether these phenotypic correlations may help explain why species with large genomes are trimmed from the evolutionary tree and have restricted ecological distributions.

• Conclusion Our review tentatively supports the large genome constraint hypothesis.

INTRODUCTION

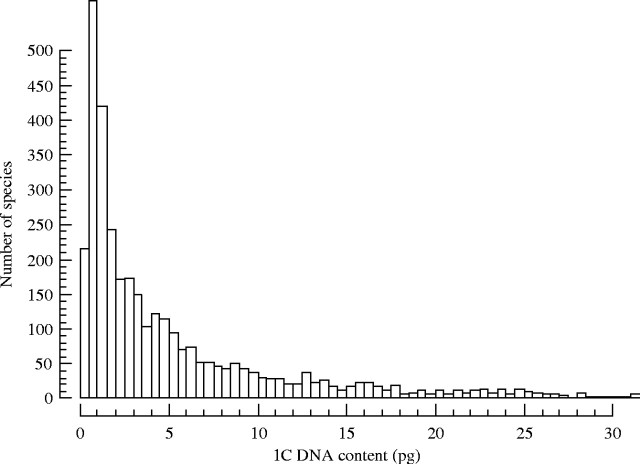
It is well known that there is significant variation in nuclear DNA content, or genome size (GS), within plants (greater than 1000-fold variation, Fig. 1), and eukaryotes in general (greater than 200 000-fold variation). Genome size varies considerably even in very closely related species. However, the evolutionary or ecological significance of this extreme variation is still largely unknown (but see Grime and Mowforth, 1982; Bennett, 1987). Much of this extreme genome size variation in plants is due to non-genic, repetitive DNA, much of which is generated by transposable elements. Given that the number of genes varies much less than GS, it appears that large genomes do not need to be large for any informational reasons. If large genomes are truly saturated with unnecessary ‘junk’ DNA, it would seem natural that there would be costs associated with accumulation and replication of this excess DNA. Here we examine the available evidence to support this hypothesis, which we term the ‘large genome constraint’.

Fig. 1.

Histogram of 3493 angiosperm genome sizes from the Plant DNA C-values database (www.rbgkew.org.uk/cval). Note that this histogram is cut off at 1C DNA content of 30 pg but the full histogram continues out to 127·4 pg.

One extreme of thinking on this issue is to reject the existence of true ‘junk’ DNA (Bennett, 1971; Cavalier-Smith, 1985, 2005). Indeed, a large number of correlations between DNA amount and cellular and physiological characters of clear functional importance is reason to believe that GS variation carries with it functional consequences (see the section on phenotype below). ‘Junk’ DNA may in fact be playing an important role, albeit non-coding in nature, but nevertheless just as important at the phenotypic level.

The other extreme is to suggest that the cost of carrying ‘junk’ DNA is so minimal, even in extreme cases, that there is no noticeable selective consequence. In this case the organism may compensate for GS effects on phenotype until the effects become deleterious. The evidence for this way of thinking comes from the ‘selfish’ nature of most of the ‘junk’ DNA (Doolittle and Sapienza, 1980; Orgel and Crick, 1980), making it more likely that its accumulation has little to do with the organism's fitness. Also, it appears that many organisms can undergo sharp increases in genome size without consequence (polyploidy formation in plants, for example). Here we present evidence to the contrary.

Within plants the distribution of genome sizes is significantly skewed, with decreasing numbers of species for every doubling of genome size (Fig. 1). One way to explain this skew is to suggest that increases in genome size are rare and that only a few lineages have experienced them. But we know that transposable elements are ubiquitous in plants and polyploidy is exceedingly common (Wendel, 2000). Both of these processes operate rapidly on evolutionary time scales. Certainly it appears that there has been enough time and ample means for all plant genomes to become large. But they are not, which in itself may provide the best argument that unchecked genomic enlargement carries maladaptive consequences.

It is also possible that genome shrinkage is a powerful and common process and can counteract the many mechanisms for genome growth (Petrov, 2002). There is evidence for genome size shrinkage and we know of mechanisms capable of causing such reduction (Petrov et al., 1996; Kirik et al., 2000; Orel et al., 2003; Bennetzen et al., 2005). But, unless one invokes selection favouring genome size reduction, it is not clear why, over time, more species would end up having small genomes. So, maybe there is selection acting against organisms with large genomes after all? However, in the case of plant species with large genomes, perhaps such selection was weak and could not stem sharp genome size increases perpetuated by fast and powerful forces of DNA addition.

In this paper we discuss the hypothesis that lineages with large genomes do pay costs. First, we may be able to detect that cost by examining the evolution of species with large genomes. Vinogradov (2003) found that species with large genomes are less likely to generate progenitor species, either through decreased speciation rates or increased rates of extinction. We re-examine this result using quantile regression analyses to show that the negative trend between the number of species in a genus and the average genome size of a genus is driven by a more significant negative relationship for genera with the largest genome sizes. We also test the relationship at higher taxonomic levels using Magallon and Sanderson's (2001) molecular clock and fossil record corrected estimates of diversification rates for several angiosperm lineages. Combined with Vinogradov's (2003) observations, these results may at least partly explain the skewed distribution of plant genome sizes.

We further explore possible ecological constraints on the distribution and abundance of species with large genomes. We find that species with large genomes are restricted to less stressful environments with longer growing seasons. Once again, careful statistical analyses are necessary to pick up trends at the edges or boundaries of these complex bi-variate distributions. These ecological constraints, combined with strong positive correlations with seed mass, may lead to species with large genomes having smaller effective population sizes, which in turn may lead to the higher probabilities of extinction in general and ‘mutational meltdown’ scenarios (Lynch et al., 1993) in particular.

Ultimately, if these ecological and evolutionary constraints are real, the genome size effect must be expressed in the phenotype and confer selective disadvantages. Therefore, we review the available data on the physiological correlates with genome size variation, present new analyses involving maximum photosynthetic rate and specific leaf area, and discuss whether these relationships help explain why species with large genomes are trimmed from the evolutionary tree and have restricted ecological distributions.

The paper is divided into three sections discussing genome size effects on (1) evolution, (2) the distribution and abundance of species (ecology), and (3) phenotype. While we present some new analyses, this paper is intended primarily to define concepts and to review the large body of research in these areas. Fitting with this purpose we combine our methods, results and discussion into one section for each of the three topics.

EVOLUTION

Recently Vinogradov (2003) reported a negative correlation between the number of species in a genus and the average GS of that genus, suggesting a genome size constraint on evolvability. Vinogradov (2003) also consulted a conservation database and found that species listed as rare or endangered tended to have larger genomes (rarity status was determined both locally and world-wide by the United Nations Environment Program World Conservation and Monitoring Center, UNEP-WCMC). Both of these lines of evidence suggest that large genomes are maladaptive at the species level, and reduce the abundance of species with large genomes.

To further probe this interesting relationship, we re-tabulated the data on the genus-level diversity and the genus-average genome size. We used the Plant DNA C-values database compiled at the Royal Botanical Gardens at Kew by Bennett et al. (2001; www.rbgkew.org.uk/cval) to acquire the average GS for 761 genera. We related these values to the number of species in each genus using the database compiled by Mabberley (1999). While genus is a somewhat arbitrary grouping, it is currently the best system available for this type of anaylsis. Some genera have been studied more than others, perhaps leading to specious genera as a result of investigative effort. In addition, taxonomic ‘splitters’ and ‘lumpers’ abound. However, by studying 761 genera we expect that errors introduced by these effects are random.

Similar to Vinogradov (2003), we found a very weak negative Spearman's rank correlation (r = −0·065, one-tailed P = 0·036). However, for our data, the relationship is only significant with a one-tailed test. A randomization test also showed the presence of a weak negative correlation of similarly marginal statistical significance (3·2 % of randomized samples generated as strong, or stronger negative correlation as the data). It is noteworthy that the correlation coefficient in Vinogradov (2003), although also negative and weak (r = −0·11; P < 0·001), was nevertheless stronger than ours. Perhaps the source for this discrepancy involves our use of Mabberly (1999) to tabulate the number of species in a genus while Vinogradov used the International Plant Names Index. These differences notwithstanding, either analysis shows at best a weak relationship between genus-level diversity and GS (Fig. 2A).

Fig. 2.

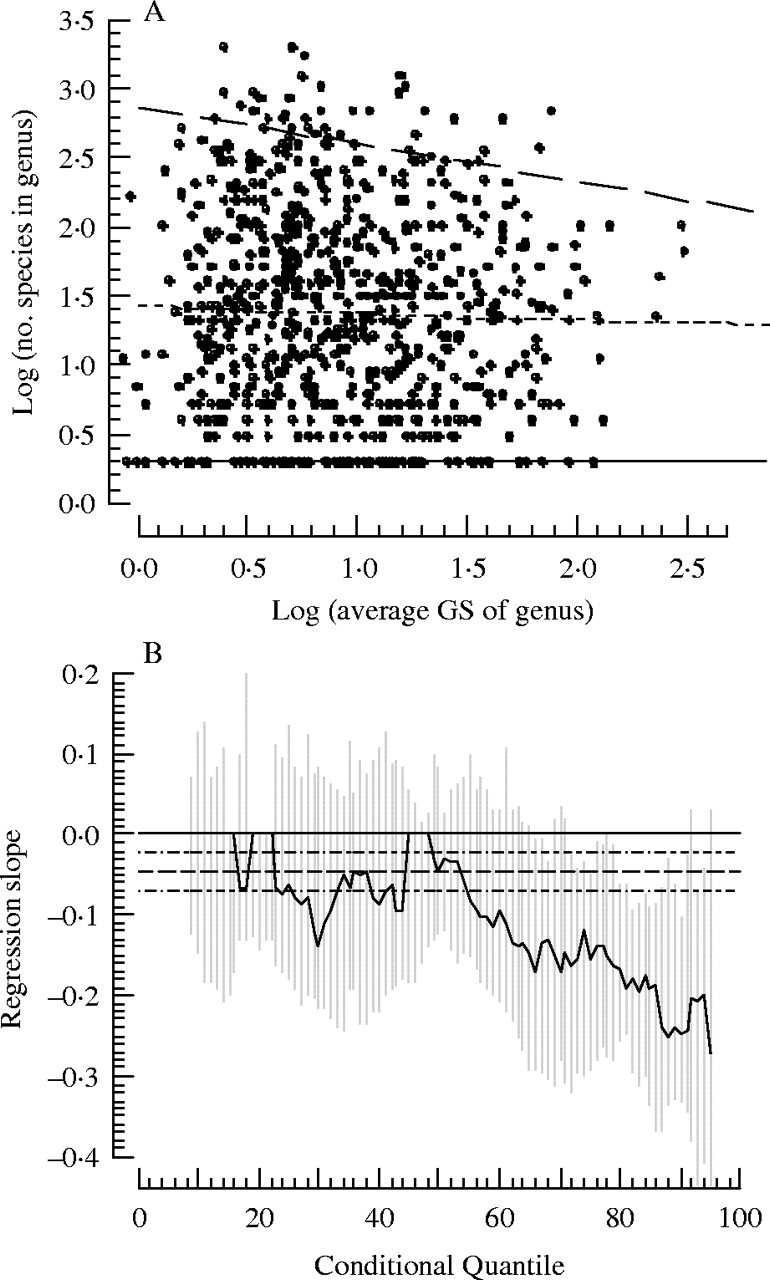
(A) Scatter plot of the log of the average genome size (1C gigabase pairs) of a genus versus the log of the number of species in that genus. (B) Quantile regression analysis of (A) showing a decreasing regression slope with increasing quantiles. The lines in (A) correspond to the 5th quantile (thin solid line), the 50th quantile (thin dashed line) and the 95th quantile (thick dashed line). The lines in (B) correspond to the least squares estimate for the linear relationship between x and y in (A) (dashed line) and the confidence interval of that estimate (double-dashed line). The grey bar surrounding the quantile dependent regression slope estimate is the standard error of the estimate.

We wanted to further explore these data to address the model of a large genome constraint on evolvability more specifically. The correlation statistics employed above test for the existence of a relationship through the means (or centre) of the data distribution. We have a more specific model. Our hypothesis suggests that there should be little signal for species with small GS. In those cases, many other determinants of diversification rates may come into play. More specifically, we would like to test whether species with large or very large genomes are less likely to attain high diversification rates and whether they are more likely to exhibit higher extinction rates.

To test this more specific model, we employed quantile regression analyses. Each quantile regression estimate involves every point in a bi-variate data distribution, but the points above the regression line are weighted by the quantile (for instance 0·65 for the 65th quantile) while the points falling below the regression line are weighted by one minus the quantile (corresponding to 0·35 for the same 65th quantile). The 65th quantile regression also implies that 65 % of the observations fall below the regression line while 35 % of the observations fall above the line. The 50th quantile regression estimate is the same as the traditional least-squares regression estimate with the condition that equal numbers of points fall above and below the line (here both groups are equally weighted by 0·5). Because of the quantile-dependent partitioning of data points above and below the regression line, quantile regression is a non-parametric technique. Results for log-transformed or untransformed data give identical results. Koenker and Bassett (1978), Cade and Richards (1996), Cade et al. (1999), Cade and Guo (2000), Koenker and Hallock (2001), Knight and Ackerly (2002) and Cade and Noon (2003) provide detailed discussion of quantile regression methods.

Our quantile regression results (Fig. 2) show that the weak negative trend for standard correlation coefficients must be due to the increasingly negative trend in the larger quantiles. Quantile regression estimates between the 5th and 70th quantile are not significantly different from zero. However, quantile regression estimates between the 70th and 95th quantile are negative and significantly different from zero. The negative relationships in these quantiles (corresponding to the species with larger GS) must drive the statistical significance of Spearman rank correlations. These results provide support for the model of large genome constraint. Not only does it show that much of the signal is in the low diversity of large GS genera, but also that large genomes can reduce diversity quite substantially.

Despite the apparent success in the quantile regression statistics for pin-pointing the cause of the negative relationship, there are several potential sources of error. What we are really after is the genome size of the ancestral species that either did or did not diversify. Whether or not the average genome size of extant species captures this ancestral value is not clear. The average genome size of a genus often does not take into consideration every species in the genus and some genera are under-represented compared to other genera, both for sampling reasons (i.e. in some cases, such as Atriplex, only 10 out of 300 species have been measured) or for taxonomic reasons. For example, three of the four Milium species have been estimated, but with a maximum possible sample size of four there is little confidence in the ancestral genome state. But removing these lineages from the analyses would bias results towards high-diversity clades. In addition, different genera may have been diversifying for very different amounts of time. Ideally one could perform an independent contrast test with a complete molecular-clock-corrected genus-level phylogeny. This type of phylogeny is one of the last gaps in the Angiosperm Phylogeny Working Group's (APG) endeavour to produce a comprehensive phylogeny for angiosperms (The Angiosperm Phylogeny Group, 2003). Historically, individual investigators have focused on molecular phylogenies within genera. Recently, family-level phylogenies have received considerable interest (The Angiosperm Phylogeny Group, 2003). The placement of genera is still uncertain. In the future, independent contrast tests for the relationship between genome size and the number of species in a genus may ascertain whether the relationship is truly robust.

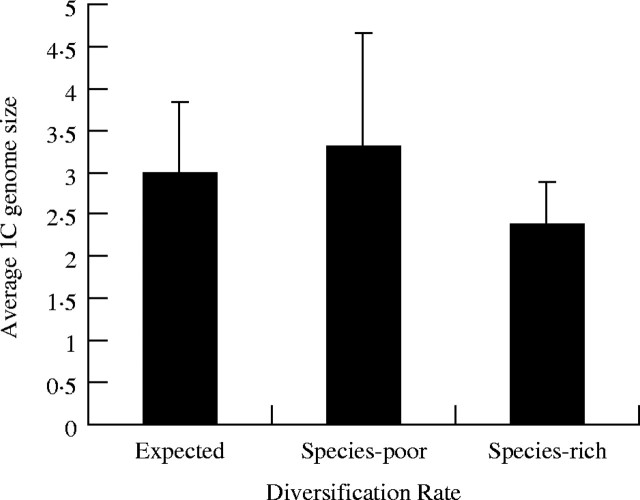
We can, however, use a family-level phylogeny where the diversification rates have been estimated using a molecular clock and the fossil record (Magallon and Sanderson, 2001). The Magallon and Sanderson (2001) study provided estimates of diversification rates for several major angiosperm groups. They identified ten lineages that were diversifying significantly faster, 13 lineages that were diversifying significantly slower, and 17 lineages classified as having the expected diversity based on their age. We tabulated the GS for these groups and found no significant GS effect for diversification rate (Spearman's rank correlation, r = 0·10, P > 0·05, Fig. 3). The lineages that were identified as species-rich did have lower GS compared to both the species-poor clades and the clades diversifying at the expected or average rate. However, these results were also not statistically significant. It is possible that the GS effect is too weak to be picked up in such a small dataset (40 lineages). In addition, family-level means may saturate any signal for potential causative effect on diversification rates. Molecular-clock and fossil-record-corrected phylogenies at the genus level should help disentangle some of these ambiguities.

Fig. 3.

Average 1C genome size (gigabase pairs) for angiosperm clades identified by Magallon and Sanderson (2001) as being species-rich or species-poor compared with the expected diversity based on molecular clock and fossil record corrected estimates of the age of clades (compared to the rest of the angiosperms).

Self-incompatibility may be one of the factors favouring persistence and diversification of lineages because of fitness advantages to out-crossing (Richards, 1997). In most cases polyploidy breaks down self-incompatibility due to the genetic interaction of diploid pollen grains with the haploid egg (Richards, 1997). Polyploids can buffer the effects of inbreeding better than diploid species because of their increased heterozygosity (Husband and Schemske, 1997; Lande and Schemske, 1985). However, in changing environments self-compatible species may be at a disadvantage. It is estimated that between 47 and 70 % of flowering plants are the descendants of polyploid ancestors (Masterson, 1994). Therefore, if genome size increases are brought about by polyploidy (with associated re-diploidization), perhaps one of the large genome constraints involves the breakdown of self-incompatibility.

Given the analyses presented above, at best we can suggest that there is a tentative GS effect for the generation of plant species diversity that is worthy of further investigation. Lineages with small or average GS may diversify at both fast and slow rates. However, when the analysis is restricted to lineages with the largest GS (the quantile regression analysis) the constraint on diversification rate becomes more pronounced.

ECOLOGY

In an attempt to provide an ecological significance to the pronounced variation in plant genome size (GS), early investigators used altitude and latitude as proxies for abiotic selection pressures putatively acting on GS. A summary of GS/altitude studies reveals that nine found positive correlations, eight found negative correlations, and six were inconclusive or not statistically significant (see Table 1 for references). On first impression it would seem that there is no general relationship between altitude of origin and GS. However, it may be that the trend is not linear, and mean regression statistics across the whole range of environments may not fully capture the relationship. Rayburn (1990) found both positive and negative correlations for 23 populations of Zea mays when comparing their altitude of origin in Mexico. Rayburn's observations suggest that species with large genomes occur at intermediate elevations and species with small genomes tend to occur at both low (sea-level) and high (2440 m) elevations. Perhaps results for altitude have been inconclusive because the real trend is not linear but rather hump-shaped or unimodal.

Table 1.

Previous studies on the relationship between genome size with altitude (Alt.) and latitude (Lat.)

Variable	Correlation	Level	Description	Authors
Alt.	+	Pop.	15 Dasypyrum villosum in Italy	Caceres et al., 1998
Alt.	+	Sp.	4 Crambe and 3 Sonchus in Macronesia	Suda et al., 2003
Alt.	+	Pop.	12 Zea mays southwestern USA	Rayburn and Auger, 1990
Alt.	+	Sp.	Crepis praemorsa	Godelle et al., 1993
Alt.	+	Sp.	Hypocheris	Cerbah et al., 1999
Alt.	+	Sp.	Secale species	Bennett, 1976a; Smith et al., 1976
Alt.	+	Pop.	Teosinte	Laurie and Bennett, 1985
Alt.	+	Sp.	11 Salix (mountain species with greatest DNA content)	Thibault, 1998
Alt.	−	Sp.	8 Argyranthemum in Macronesia	Suda et al., 2003
Alt.	−	Pop.	20 Arachis duranensis in Argentina/Bolivia	Temsch and Greilhuber, 2001
Alt.	−	Pop.	8 Dactylis glomerata in Spain	Creber et al., 1994
Alt.	−	Pop.	17 Dactylis glomerata in France and Italy	Reeves et al., 1998
Alt.	−	Pop.	12 Zea mays in New Mexico	Rayburn, 1990
Alt.	−	Pop.	Corn (knob number)	Mangelsdorf and Cameron, 1942; Longley and Kato, 1965; Wellhausen et al., 1952; Bennett, 1976b
Alt.	−	Pop.	24 Berberis in Patagonia	Bottini et al., 2000
Alt.	−	Pop.	15 Zea mays	Poggio et al., 1998
Alt.	−	Pop.	11 Arachis duranensis	Singh et al., 1996
Alt.	∧	Pop.	23 Zea mays Southwestern USA.	Rayburn, 1990
Alt.	ns	Sp	4 Silene and 5 Micromeria in Macronesia	Suda et al., 2003
Alt.	ns	Pop.	10 Sesleria albicans European	Lysak et al., 2000
Alt.	ns	Sp.	21 Artemisia	Torrel and Valles, 2001
Alt.	ns	Sp.	51 neotropical Lonchocarpus trees	Palomino and Sousa, 2000
Alt.	ns	Pop.	5 Dactylis glomerata in Slovenian Alps	Vilhar et al., 2002
Alt.	ns	Sp.	7 Echeandia	Palomino, 1993
Lat.	+	Pop.	Several Picea sitchensis	Miksche, 1967, 1971
Lat.	+	Sp.	Tropical vs. temperate grasses	Avdulov, 1931
Lat.	+	Sp.	329 tropical vs. 527 temperate plants	Levin and Funderburg, 1979
Lat.	+	Sp.	17 Poaceae and 15 Fabaceae crops	Bennett, 1976a
Lat.	+	Pop.	24 Berberis in Patagonia	Bottini et al., 2000
Lat.	−	Sp.	20 Arachis duranensis in Argentina/Bolivia	Temsch and Greilhuber, 2001
Lat.	−	Pop.	Several Festuca arundinacea	Ceccarelli et al., 1992
Lat.	−	Pop.	North American cultivars of Zea mays	Rayburn et al., 1985
Lat.	−	Sp.	162 British plants	Grime and Mowforth, 1982
Lat.	−	Sp.	23 Arctic plants	Bennett et al., 1982
Lat.	−	Pop.	22 North American Zea mays	Rayburn et al., 1985
Lat.	−	Pop.	11 North American Zea mays	Laurie and Bennett, 1985
Lat.	ns	Sp.	18 pines	Joyner et al., 2001
Lat.	ns	Pop.	6 Allium cepa cultivars	Bennett et al., 2000
Lat.	ns	Pop.	Several Picea glauca	Teoh and Rees, 1976
Lat.	ns	Pop.	10 Dactylis glomerata	Creber et al., 1994
Lat.	ns	Sp.	11 Tropical vs. temperate Pines	Hall et al., 2000
Lat.	ns	Sp.	19 Helianthus	Sims and Price, 1985

Correlations are either +, −, not significant (ns), or non-linear (∧). Studies were classified into different levels: those dealing with different populations of the same species (Pop.) or multiple species (Sp.).

Results for GS and latitude of origin mirror those for altitude. Five studies found positive, seven found negative, and five found non-significant correlations (see Table 1 for references). Levin and Funderburg (1979) did a discrete test between tropical and temperate species and found that temperate species had nearly double the GS of tropical plants, while Bennett et al. (1982) and Bennett (1987) suggest that angiosperm species with large GS are progressively excluded from northern latitudes—again suggesting a unimodal or hump-shaped distribution for GS across latitudinal gradients, similar to Rayburn's observations for altitude.

Confusion about the relationship between GS and altitude or latitude may arise because factors such as temperature and precipitation, which may more accurately represent selection pressures acting on GS, do not vary linearly with altitude or latitude. In addition, most studies did not consider a full range of elevations or latitudes—from sea-level to mountain tops and from the tropics to the arctic. Finally, even using the correct underlying variables over the whole range of values, we may still find that the relationship is truly non-linear. Results from Rayburn (1990), Levin and Funderburg (1979) and Bennett et al. (1982; Bennett, 1987) suggest that the true relationship between GS and altitude or latitude may more accurately be represented by a unimodal distribution where species with low DNA content may exist at any elevation or latitude but species with the largest DNA contents may be excluded from the extremes.

Knight and Ackerly (2002) confirmed this prediction using GS trends across environmental gradients of temperature and precipitation in the California flora. They used a geographic information system to calculate mean July maximum temperature and annual precipitation inside the geographic range of 401 species in the California flora and compared these values to tabulated measurements of GS for these species (taken from the Plant DNA C-values database). Their findings show that species with large genomes tend to be excluded from extreme environments with shorter growing seasons (high or low July maximum temperatures, or reduced annual precipitation). Similar to the results presented in the evolution section, these results were not obvious with mean regression statistics. The trends only became apparent when quantile regression analyses were applied. In this case, a quadratic quantile regression was used to model the predicted unimodal trend. With increasing quantiles, the quadratic coefficient became more negative, implying increased concavity of the inverted parabolic function. We re-analysed the relationships presented by Knight and Ackerly (2002) with 20 additional species (421 in total). These analyses were not significantly different from the original interpretation (Fig. 4), and again support the hypothesis that species with large genomes are progressively excluded from habitats with extreme July maximum temperatures and decreased annual precipitation. Qualitatively, results were similar when performed with basic genome size (2C values divided by ploidy level) and 1C DNA contents.

Fig. 4.

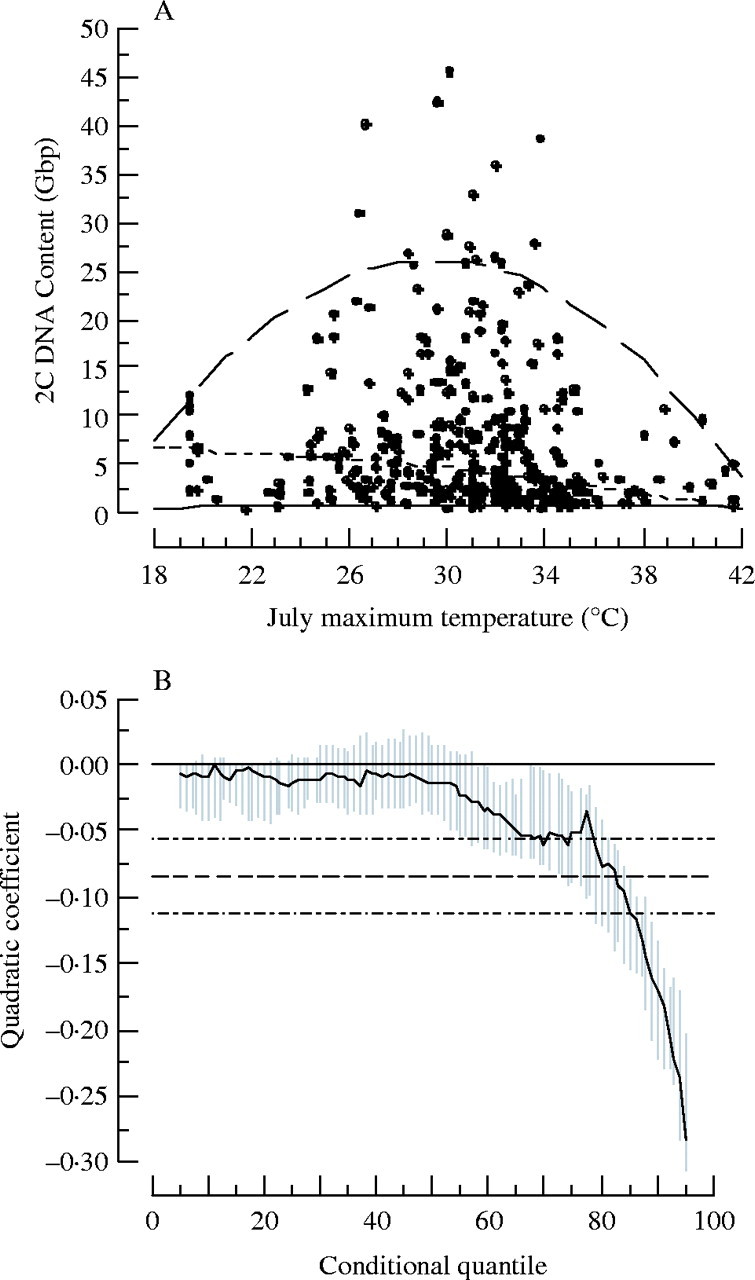
(A) Scatter plot of the mean July maximum temperature inside the range of 421 species in the California flora versus the mean 2C DNA content of those species in gigabase pairs (Gbp). (B) Quantile regression analysis of (A), showing a decreasing quadratic coefficient for increasing quantiles. The lines in A correspond to the 5th quantile (thin solid line), the 50th quantile (thin dashed line) and the 95th quantile (thick dashed line). The lines in (B) correspond to the least-squares estimate for the normal mean quadratic function for the relationship depicted in (A) (dashed line) and the confidence interval of that estimate (double-dashed line). The grey area depicts the quantile dependent confidence interval for the quadratic coefficient.

Other investigators have used mean temperatures inside species' geographic ranges as a correlate with GS (see Table 2 for complete references). Suda et al. (2003) found both positive and negative relationships in the Macronesian flora with mean annual temperature. Turpeinen et al. (1999) found a positive correlation between GS and mean January temperatures in populations of wild barley in Israel, and Wakamiya et al. (1993) found a negative correlation in pines for the highest spring mean monthly air temperature (Table 2). Combining geographic information system (GIS) analyses with species-specific plant functional traits, such as genome size, should continue to be a fruitful endeavour for the analysis of putative abiotic selection pressures operating on GS.

Table 2.

Previous studies on the relationship between genome size and temperature

Correlation	Level	Description	Authors
+a	Sp.	8 Argyranthemum, 4 Silene and 5 Micromeria in Micronesia	Suda et al., 2003
+b	Pop.	10 Hordeum spontaneum in Israel	Turpeinen et al., 1999
+a	Pop.	Several Festuca arundinacea	Ceccarelli et al., 1992
+d	Pop.	45 Dactylis glomerata	Bretagnolle and Thompson, 1996
−c	Pop.	Herbaceous plants in UK	MacGillivray and Grime, 1995
−a	Sp.	4 Crambe and 3 Sonchus in Micronesia	Suda et al., 2003
−e	Sp.	18 North American pines	Wakamiya et al., 1993
−f	Pop.	7 white clover	Campbell et al., 1999
−j	Pop.	8 maize populations	McMurphy and Rayburn, 1991
−g	Sp.	131 British angiosperms	Thompson, 1990
−f	Sp.	30 Grasses and forbs in UK	Grime et al., 1985
−f	Sp.	24 UK geophytes and grasses	Grime and Mowforth, 1982
−g	Sp.	43 UK angiosperms	Grime et al., 1997
∧h	Sp.	401 California Angiosperms	Knight and Ackerly, 2002
nsi	Pop.	6 Hordeum spontaneum in Israel	Kalendar et al., 2000

Correlations are either +, −, not significant (ns), or non-linear (∧). Studies were classified into different levels: those dealing with different populations of the same species (Pop.) or multiple species (Sp.). Footnotes a–j describe the temperature variable used.

^aMean annual temperatures.

^bmean January temperature

^clowest temperature survived (frost tolerance) – higher values equals less frost tolerance

^dability for winter growth

^ehighest mean monthly spring air temperature

^ftiming of spring growth

^gminimum germination temperature

^hJuly maximum temperature

ⁱnorth-to-south facing transects (individuals on south-facing slope had slightly larger genome)

^jcold tolerance and frost tolerance.

Other temperature variables have been used as correlates with GS, including (1) the timing of spring growth, (2) germination temperatures, and (3) frost tolerance. Grime and Mowforth (1982) and Grime et al. (1985) measured the timing of spring growth for several species in the UK flora. Species that delayed growth until the warmer spring and summer months tended to have smaller GS. Those that grew early in the spring had larger GS (here recorded as a negative correlation with temperature, Table 2.). Campbell et al. (1999) found the same correlation for populations of white clover. However, Bretagnolle and Thompson (1996) found the opposite relationship for sympatric Dactylis glomerata populations. MacGillivray and Grime (1995) measured frost tolerance and found that species that survived colder temperatures tended to have larger GS (also recorded as a negative correlation with temperature in Table 2). Thompson (1990) and Grime et al. (1997) measured optimal germination temperatures for several species in the UK flora and found that species that germinated at lower temperatures tended to have larger GS (also recorded as a negative correlation with temperature in Table 2).

Grime and Mowforth (1982) suggested that early spring growth is facilitated by large GS and the associated larger cell sizes (see section on phenotype). Their model suggests that growth at low temperatures is facilitated by cell expansion driven by turgor pressure and not cell division. The theory is supported by observations that cell size is positively correlated with GS (Rees et al., 1966; Bennett et al., 1983; Anderson et al., 1985) and the fact that cell division is inhibited at lower temperatures (Haber and Luippold, 1960).

Considering the proposed importance of cell size, turgor pressure and cell expansion for predicting responses of large GS species to low temperatures, it is not surprising that several studies have also found positive correlations between GS and estimates of water availability or mean annual precipitation within species ranges (see Table 3). Knight and Ackerly (2002) reported positive correlations between GS and annual precipitation for 401 species in the California flora. The relationship was steeper and more significant as GS increased (see quantile regression analyses presented above). Price et al. (1981), and Castro-Jimenez et al. (1989) also found positive relationships. Bottini et al. (2000) report a negative correlation, but water availability differed between sites independently of the annual average rainfall. Populations that occurred at sites with high water availability tended to have larger GS (no statistics were presented to support this claim). Other investigators have found negative or inconclusive results. For example, Wakamiya et al. (1993) found a negative correlation between annual precipitation and GS for 18 North American pines. Suda et al. (2003) also found a negative correlation for several species in the Micronesian flora. Sims and Price (1985) found no significant relationship between GS and estimates of mean annual precipitation within the ranges of 16 Helianthus species. Taken together, one might conclude that there is no interaction between annual precipitation or water availability with genome size. However, the sample sizes were small for many of these analyses. In addition, the combined effects of water stress and high temperature stress may amplify putative environmental effects on nuclear DNA content (annual precipitation and July maximum temperature are strongly correlated at the high temperature extreme).

Table 3.

Previous studies on the relationship between genome size and precipitation

Correlation	Level	Description	Authors
+	Sp.	5 Microseris bigelovii and 5 M. laciniata	Castro-Jimenez et al., 1989
+	Sp.	4 Crambe and 3 Sonchus in Micronesia	Suda et al., 2003
+a	Pop.	24 Microseris douglasii	Price et al., 1981
+	Sp.	401 California angiosperms	Knight and Ackerly, 2002
−	Sp.	8 Argyranthemum, 4 Silene and 5 Micromeria in Micronesia	Suda et al., 2003
−b	Sp.	18 North American Pinus	Wakamiya et al., 1993
−c	Pop.	24 Berberis in Patagonia	Bottini et al., 2000
ns	Sp.	19 Helianthus	Sims and Price, 1985

Correlations are either +, −, not significant (ns). Studies were classified into different levels: those dealing with different populations of the same species (Pop.) or multiple species (Sp.).

^aTemporal variation within a single population

^blowest mean monthly precipitation

^cannual rainfall, but there was a positive correlation with water availability (no statistical analyses shown).

Studying six pine species, Wakamiya et al. (1996) found that species with higher turgor-loss points (i.e. greater water stress sensitivity) had larger GS. These species would be less able to survive drought and therefore excluded from areas with decreased water availability. Further studies on turgor-loss point, cell size and DNA content, with measures of water availability, should be performed to further characterize the effects of DNA content on the distribution and abundance of species with large genomes across gradients of precipitation.

PHENOTYPE

Although the causal nature of the large genome constraint outlined in the previous sections is uncertain, it may arise from correlated effects beginning at the cellular level. Here we discuss several well-established cellular correlations with GS: the strong positive correlation with seed size, GS correlations with life history parameters (annual vs. perennial growth habit, relative growth rate and generation time), and new analyses dealing with GS effects on maximum photosynthetic rate and specific leaf area.

Cellular correlations

Early investigators found several fascinating cellular and developmental correlations with GS (see Bennett, 1973, 1987, and Cavalier-Smith, 1985, for reviews). First, nuclear DNA content is positively and quite directly correlated with chromosome size, nuclear size and cell size (Rees et al., 1966; Baetecke, 1967; Edwards and Endrizzi, 1975; Bennett et al., 1983; Lawrence, 1985). Second, nuclear DNA content is positively and strongly correlated with the duration of cell division (Bennett, 1971, 1977; Evans et al., 1972; Van't Hof, 1975). The causal nature of these correlations may arise from simple first principles. More DNA may require a larger container and require more time for replication. Gregory (2002) suggests that these phenomena may be manifest by GS interaction with cell cycle regulation via an unknown mechanism. It seems plausible that a species with a small genome might have a larger cell size or longer cell cycle, but that is rarely the case. These simple predictions may manifest at higher phenotypic levels, first and perhaps most directly, with seed mass.

Seed mass

Seed mass (also called seed size, the oven dry mass of the average seed) is thought to be a significant factor affecting seedling survival (Willson, 1983; Westoby et al., 1992). The positive correlation between seed size and GS has been shown repeatedly, both between populations of the same species (Caceres et al., 1998; Chung et al., 1998) and across large numbers of species (see Table 4, and Fig. 5). The consistency of these results is remarkable in comparison to the results for altitude, latitude and temperature (see Tables 1 and 2). Mowforth (1985) showed that the relationship between seed size and GS was a filled triangle rather than simply a linear positive trend. As seed mass increases there is a larger range of observed GS, but species with the largest GS always have large seeds.

Table 4.

Previous studies on the relationship between genome size and seed mass

Correlation	Level	Description	Authors
+	Pop.	15 Dasypyrum villosum	Caceres et al., 1998
+	Pop.	12 Soybean strains	Chung et al., 1998
+	Sp.	131 British angiosperms	Thompson, 1990
+	Sp.	43 British plants	Grime et al., 1997
+	Sp.	22 Crepis	Jones and Brown, 1976
+	Sp.	12 Allium and 6 Vicia	Bennett, 1972
+	Sp.	18 North American pines	Wakamiya et al., 1993
+	Sp.	19 Mediterranean annuals	Maranon and Grubb, 1993
+	Sp.	148 species in California flora	Knight and Ackerly, 2002
+	Sp.	Several Poaceae and Fabaceae	Mowforth, 1985
Ns	Sp.	16 grassland species, UK	Leishman, 1999

Correlations are either positive (+) or not significant (ns). Studies were classified into different levels: those dealing with different populations of the same species (Pop.) or multiple species (Sp.).

Fig. 5.

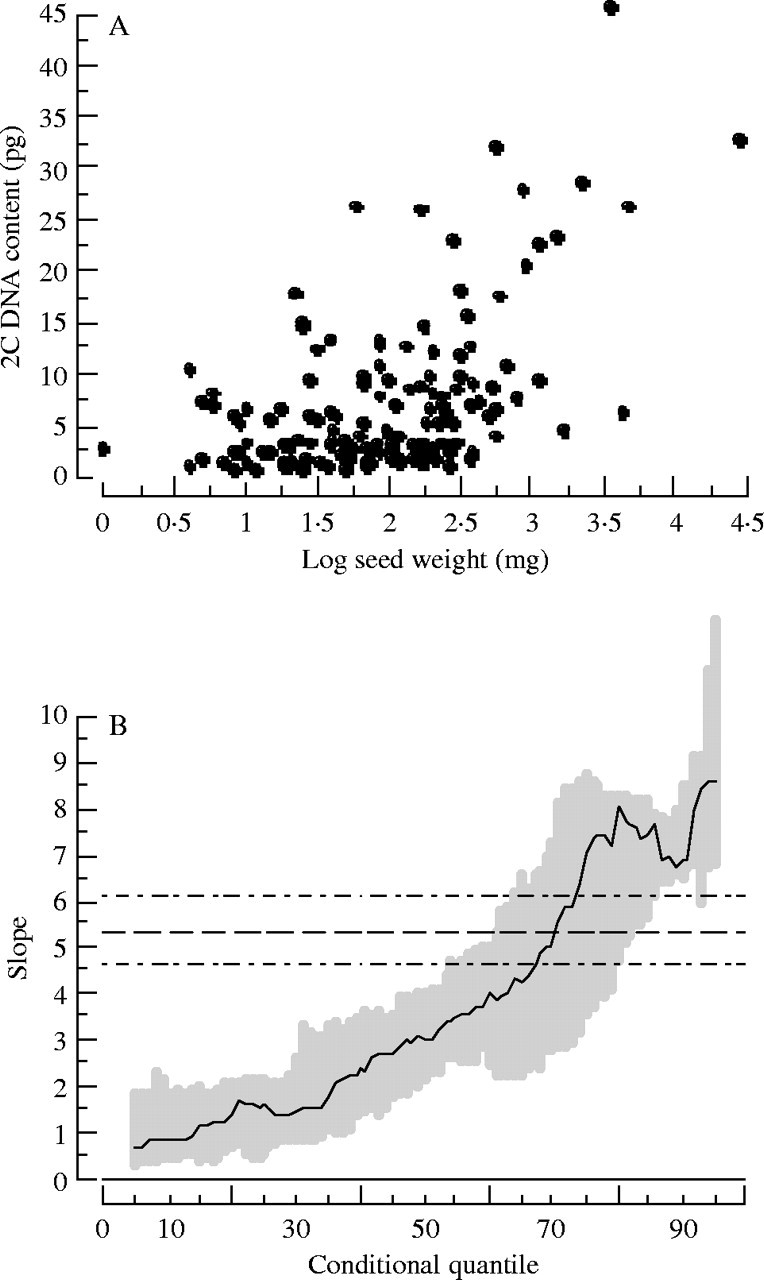
(A) 2C DNA content variation with seed size for 148 angiosperms in the California flora. Notice that there is an absence of species with large 2C DNA contents and small seeds. Species with small genome sizes can have a range of seed sizes while species with larger genome sizes are restricted to having larger seed sizes. (B) A quantile regression plot of (A) showing how the linear slope between DNA content and the log of seed weight changes with increasing quantiles. The dashed line is the least-squares regression with confidence intervals (double-dashed lines).

We reanalysed Knight and Ackerly's (2002) seed mass/GS data using a semi-log plot. Displayed this way, their data take on the triangular appearance shown by Mowforth (1985, Fig. 5). We also performed a quantile regression analysis of these data. This demonstrated a threshold or limit for the relationship between GS and seed size. With increasing quantiles the slope of the linear regression became steeper. The quantile regression analysis supports the idea that there is a GS-dependent constraint on attainable seed masses. There was a shallow positive slope for the lowest quantiles, with significantly steeper slope estimates for the largest quantiles. As GS increases, cell sizes increase, which may naturally force seed sizes to become larger. There may be some mechanisms for counteracting this tendency, but these mechanisms may evolve more slowly than the accumulation of DNA.

Species that produce small seeds can increase reproductive output because small seeds are produced in greater numbers (Cornelissen et al., 2003), which may also lead to greater dispersal ability. However, the positive fitness consequences of having large seeds have also been examined. It is thought that large-seeded species tend to produce larger seedlings (Leishman et al., 2000) with greater reserves for growth, enabling seedlings to survive under shaded canopies (Grime and Jeffery, 1965; Leishman and Westoby, 1994a; Saverimuttu and Westoby, 1996), in dry soil (Leishman and Westoby, 1994b) and in low-nutrient environments (Milberg et al., 1998). Large-seeded species may also compete better for resources (Black, 1958; Gross and Werner, 1982; Reader, 1993) and better withstand herbivory and pathogen attack (Armstrong and Westoby, 1993; Harms and Dalling, 1997). Large-seeded species, and thus large genome species, may increase their probability of regeneration in their current environment at the expense of dispersal into new environments.

If the small-genome plants can evolve larger seeds but rarely do, it would suggest that having a larger number of smaller seeds is the superior strategy. The large-genome plants may often be unable to counteract the increase of seed size due to the increase in GS and have to evolve a suite of traits that ameliorate such negative consequences. In any case, the greater dispersal ability brought about by the increased seed number may contribute to the reduced rates of extinction of small-genome species (see the evolution section) and may also contribute to the increased dispersal into extreme habitats and thus increase the probability of allopatric speciation events.

Leaf anatomical traits

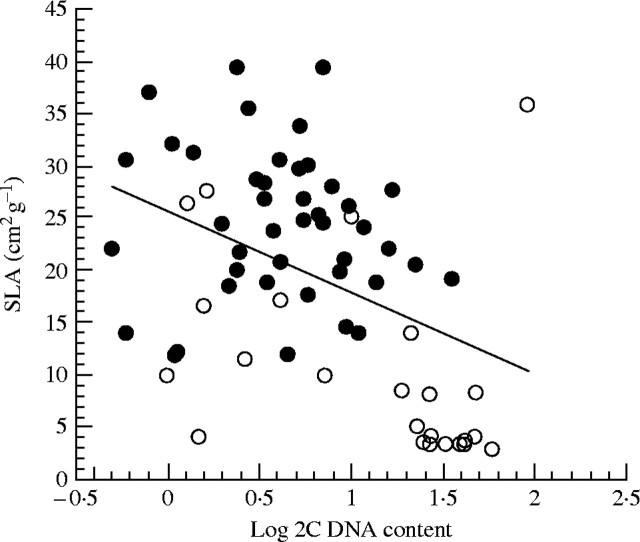
The rate of cell division and cell size could have significant effects on leaf morphology. Other investigators have found both positive and negative correlations between GS and leaf area, length or width (see Table 5). The relationship of leaf area to leaf mass (specific leaf area, SLA) is a trait at the nexus of a suite of co-varying traits related to the efficiency of carbon gain and leaf longevity (Reich et al., 1997, 1998). Given the cellular correlations presented above and the potential functional associations to be gleaned from a relationship between GS and SLA, we examined this relationship using two functional trait databases (that of Grime et al., 1997 and Reich et al., 1998). We obtained SLA estimates for 67 species with known 2C DNA content. We found a significant negative correlation between GS and SLA (r = −0·42, P < 0·0001; Fig. 6). Species with low SLA (typically smaller, thicker leaves) tended to have larger GS.

Table 5.

Previous studies on the relationship between genome size and leaf anatomical traits

Correlation	Level	Description	Authors
+	Sp.	5 Microseris bigelovii and 5 M. laciniata (leaf area)	Castro-Jimenez et al., 1989
+	Sp.	12 Soybean strains (leaf length and width)	Chung et al., 1998
+	Sp.	18 North American Pinus (needle fresh and dry mass)	Wakamiya et al., 1993
−	Pop.	15 Dasypyrum villosum in Italy (leaf length)	Caceres et al., 1998
−	Sp.	43 British plants (leaf width)	Grime et al., 1997
−	Pop.	30 Festuca arundinacea (leaf length)	Ceccarelli et al., 1993
Ns	Sp.	43 British plants (leaf area)	Grime et al., 1997

Correlations are either +, −, or not significant (ns). Studies were classified into different levels: those dealing with different populations of the same species (Pop.) or multiple species (Sp.).

Fig. 6.

The relationship between specific leaf area (SLA) versus log of the 2C DNA content (gigabase pairs) The solid line depicts the normal least squares regression line. Data from Grime et al. (1997, solid circles) and Reich et al. (1998, open circles).

The association between GS and SLA suggests that several other plant traits may also be associated with GS given their strong interdependence with SLA. These include maximum photosynthetic rate, dark respiration rate, leaf nitrogen content and leaf lifespan (Reich et al., 1997, 1998). Below we test for relationships with maximum photosynthetic rate. The endeavour of joining results from plant functional trait databases with estimates of GS has considerable promise to shed new insight into the phenotypic and physiological consequences of GS variation in plants.

Photosynthetic rate

Previous investigators have examined the effect of within-species ploidy variation on photosynthetic rate. Both positive (Randall et al., 1977; Joseph et al., 1981) and negative (Garrett, 1978; Setter et al., 1978; Austin et al., 1982; Wullschleger et al., 1996) correlations have been reported. To our knowledge, no cross-species comparisons have been made. Here we test for GS-dependent variation in maximum photosynthetic rate using data from a published plant functional trait database (Reich et al., 1998). We compiled estimates of mass-based maximum photosynthetic rate for 24 species with known GS. These analyses revealed a significant negative correlation. 6 Species with large genomes tend to have lower maximum photosynthetic rates.

These results parallel observations for mammals and birds where metabolic rate is negatively correlated with GS (Vinogradov, 1995, 1997; Gregory, 2002). These complementary results suggest similar scaling mechanisms for metabolic efficiency that are somehow associated with GS. While the causative nature of this relationship is still uncertain in both cases, the implications are far-reaching. The result may help to explain the relationship between GS and minimum generation time. Species with small seeds also tend to have greater mass-based photosynthetic rates because they must acquire resources rapidly on emergence rather than relying on seed stores. Species with small genomes are thus able to complete their life cycle faster. The negative correlation between SLA and GS may be a consequence of overlapping adaptive strategies selecting for a well-described suite of physiological traits fine-tuned to achieve rapid growth.

Growth rate and generation time

The relative growth rate and growth interval before reproduction are both significant factors predicting the life history and regeneration niche of a species. Species that grow fast and reproduce in short intervals are more likely to be weedy or invasive and are opportunists that occupy disturbed sites. Several studies have found negative correlations for GS and relative growth rate (RGR) and positive correlations between GS and generation time (days to flowering, seed-bearing age, flowering date; Table 6). These correlations are equivalent since species that grow faster also tend to reproduce earlier. However, some studies have found the opposite relationships for RGR and generation time (Table 6), making generalizations difficult. Bennett et al. (1998) found that invasive species tend to have smaller GS. Annual species also typically have smaller GS (see Table 6 for references). Nonetheless, decreased growth rate and increased generation time may, in some cases, form the physiological link for the observed constraints on the distribution of large genome species across environmental gradients, as well as the evolutionary constraints outlined above.

Table 6.

Previous studies on the relationship between genome size (GS) and generation time (Gen.) or relative growth rate (RGR)

Variable	Correlation	Description	Authors
Gen.	+	GS annuals <GS perennialsin 45 spieces of Vicia	Chooi, 1971
Gen.	+	GS annuals <GS obligate perennials in 271 angiosperms	Bennett, 1972
Gen.	+	GS annuals <GS perennials in 22 species of Crepis	Jones and Brown, 1976
Gen.	+	GS annuals <GS perennials in 18 species of Helianthus	Sims and Price, 1985
Gen.	+	GS annuals <GS perennials in 10 Arachis sp	Singh et al., 1996
Gen.	+	GS annuals <GS perennials in 565 angiosperms (genus level)	Vinogradov, 2001
Gen.	+	GS annuals <GS perennials in 24 species of Lathyrus	Nandini and Murray, 1997
Gen.	ns	Annuals vs. perennials in 401 CA angiosperms	Knight and Ackerly, 2002
Gen.	+	Length of vegetative period in maize strains	Tito et al., 1991
Gen.	+	Helianthus annuus	Natali et al., 1993
Gen.	+	Days to flowering in 30 populations of Festuca arundinacea	Ceccarelli et al., 1993
Gen.	+	Minimum seed-bearing age in 18 North American pines	Wakamiya et al., 1993
Gen.	+	Maize	Rayburn, 1994
Gen.	−	Initial month flowering in 42 Allium species	Labani and Elkington, 1987
Gen.	−	Tetraploids flowered earlier than diploids, populations of D. glomerata	Bretagnolle and Thompson, 1996
Gen.	−	First month flowering in 28 Allium species	Baranyi and Greilhuber, 1999
Gen.	ns	162 British annuals and perennials	Grime and Mowforth, 1982
Gen.	ns	Pisum sativum	Cavallini et al., 1993
Gen.	ns	Seed germination to anthesis, 15 populations of Dasypyrum villosum	Caceres et al., 1998
RGR	+	Rate of leaf extension at low temperatures for 24 UK species	Grime et al., 1985
RGR	+	Pisum sativum	Cavallini et al., 1993
RGR	+	Epicotyl length 2–3 d after germination in 10 populations of Vicia faba	Minelli et al., 1996
RGR	+	Growth at low temperatures for 7 population of Trifolium repens	Campbell et al., 1999
RGR	+	16 grassland species in UK	Leishman, 1999
RGR	−	6 weeks growth for 44 tillers of Poa annua	Mowforth and Grime, 1989
RGR	−	10 d after germ. in 30 populations of Festuca arundinaceae	Ceccarelli et al., 1993
RGR	−	12 Southwestern Zea mays	Biradar et al., 1994
RGR	−	Relative growth rate for 30 pines	Grotkopp et al., 1998
RGR	ns	Helianthus annuus	Natali et al., 1993
RGR	ns	Growth 7–21 d following germination for 43 species in the UK	Grime et al., 1997

Correlations are either +, −, or not significant (ns).

CONCLUSION AND FUTURE DIRECTIONS

Having reviewed the current data on the macroevolutionary, conservation, ecological and functional significance of GS variation, what can we say about the viability of the large genome constraint hypothesis? We believe that we can endorse it, but rather cautiously at present. A recurrent theme is that strict correlation analyses often do not tell the whole story. Sophisticated statistical techniques that highlight larger genome sizes are needed to understand more clearly constraints on evolution, species distribution and phenotype. In this paper we have demonstrated the utility of quantile regression methods for addressing these complexities.

Macroevolutionary and conservation data show that lineages with the largest genomes have slower than average rates of diversification and disproportionately higher extinction risk (Vinogradov, 2003). However, these relationships are non-linear. For example, lineages with small genomes are not uniformly specious and are often as depurate as lineages with very large average genome sizes. However, lineages with large genomes are rarely highly specious. It appears that diversity is constrained in some way in these lineages.

A very similar relationship is evident when examining trends across environmental gradients. Species with small genomes tend to be found in widely varying habitats. However, lineages with very large genomes appear to be excluded from the most extreme habitats. Again, it does not appear that genome size is generating a consistent causative effect across the whole genome size range; rather it appears that lineages with the largest genome sizes are constrained from finding a way to survive in extreme environments. We encourage further examination of genome size distribution across abiotic gradients, perhaps even including analyses across gradients of elevation and latitude. However, these analyses should include descriptions of mean environmental conditions and habitat types spanning the range of elevations or latitudes considered. A greater range of habitat types is more likely to reveal significant trends—perhaps only after employing quantile regression to examine the effects at the boundaries of these bivariate distributions.

Ultimately the large genome constraints for evolution and ecology must be due to phenotypic variation manifest either directly or indirectly by changes in DNA content. Associations between GS and maximum photosynthetic rate, SLA, seed mass, relative growth rate or generation time are just a few examples of ecologically relevant phenotypic traits that may form a causative link for the large genome constraints outlined above. Results for photosynthetic rate and GS are particularly intriguing. The possibility of arriving at universal scaling laws originating from nucleotypic effects that predict the metabolic efficiency of organisms is exciting. If confirmed, these results may have far-reaching implications for the ecology and evolution of species.

Phenotypic associations with GS also often exhibit non-linear distributions. In general, large-genome species tend to display restricted trait variation, while small-genome species can attain a much wider array of trait states. For example, large-genome species never have small seeds while small-genome species display a much wider range of seed sizes. Similarly, large-genome species have lower photosynthetic rates while small-genome species have a wider range of photosynthetic performance. Also, large-genome species have reduced variation in SLA and tend to have lower SLA in general, compared to small-genome species which have a wider range of SLA.

It is likely that there is strong interdependence of the large genome constraints found at the evolutionary, ecological and functional levels. We can speculate that restricted ecological tolerances may increase probabilities of extinction by reducing population sizes. It may also increase the potential of ‘mutational meltdown’ scenarios to drive populations into extinction (Lynch et al., 1993). In addition, the inability to colonize extreme environments may decrease the chances of long-term isolation and allopatric speciation of large-genome lineages. The latter effect is also amplified by the tendency of large-genome lineages to have large seeds and thus to have lower dispersal abilities.

We emphasize that the endeavour of joining results from plant functional trait databases with estimates of GS has considerable promise to provide new insight into the phenotypic and physiological consequences of variation in plant GS. Combining GIS analyses with these results will also add clues in the search for putative abiotic selection pressures operating on GS. The development of these holistic databases will perhaps allow us to progress beyond pairwise correlations to partial correlation and path analyses. Such analyses should help us finally arrive closer to direct rather than correlated statistical effects. This is a goal worth striving for.