Skip to main content
PMC home page
Annals of Botany logoLink to Annals of Botany
. 2005 Mar 24;95(7):1211–1220. doi: 10.1093/aob/mci133

Applicability and Limitations of Optimal Biomass Allocation Models: A Test of Two Species from Fertile and Infertile Habitats

YOKO OSONE 1,*, MASAKI TATENO 1
PMCID: PMC4246905  PMID: 15790584

Abstract

• Background and Aims The practical applicability of optimal biomass allocation models is not clear. Plants may have constraints in the plasticity of their root : leaf ratio that prevent them from regulating their root : leaf ratio in the optimal manner predicted by the models. The aim of this study was to examine the applicability and limitations of optimal biomass allocation models and to test the assumption that regulation of the root : leaf ratio enables maximization of the relative growth rate (RGR).

• Methods Polygonum cuspidatum from an infertile habitat and Chenopodium album from a fertile habitat were grown under a range of nitrogen availabilities. The biomass allocation, leaf nitrogen concentration (LNC), RGR, net assimilation rate (NAR), and leaf area ratio (LAR) of each species were compared with optimal values determined using an optimal biomass allocation model.

• Key Results The root : leaf ratio of C. album was smaller than the optimal ratio in the low-nitrogen treatment, while it was almost optimal in the high-nitrogen treatment. In contrast, the root : leaf ratio of P. cuspidatum was close to the optimum under both high- and low-nitrogen conditions. Owing to the optimal regulation of the root : leaf ratio, C. album in the high-nitrogen treatment and P. cuspidatum in both treatments had LNC and RGR (with its two components, NAR and LAR) close to their optima. However, in the low-nitrogen treatment, the suboptimal root : leaf ratio of C. album led to a smaller LNC than the optimum, which in turn resulted in a smaller NAR than the optimum and RGR than the theoretical maximum RGR.

• Conclusions The applicability of optimal biomass allocation models is fairly high, although constraints in the plasticity of biomass allocation could prevent optimal regulation of the root : leaf ratio in some species. The assumption that regulation of the root : leaf ratio enables maximization of RGR was supported.

Keywords: Chenopodium album, Polygonum cuspidatum, model, nitrogen, root : leaf ratio, biomass allocation, relative growth rate

INTRODUCTION

A number of experimental studies have demonstrated that under conditions of limited nitrogen availability, plants increase biomass allocation to roots at the expense of leaves (e.g. Brouwer, 1962; Boot and Den Dubbelden, 1990; Olff et al., 1990; Olff, 1992). These observations have led to the formulation of mathematical models that investigate the optimal root : leaf (shoot) ratio, i.e. the root : leaf ratio at which the relative growth rate (RGR) is at its maximum for a given level of soil nitrogen (e.g. Johnson and Thornley, 1987; Hirose, 1988; Kachi and Rorison, 1989; Hilbert, 1990; Gleeson, 1993; Van der Werf et al., 1993; Kastner-Maresch and Mooney, 1994; Thornley, 1995; Ågren and Franklin, 2003; Ishizaki et al., 2003; Osone and Tateno, 2003; Yin and Schapendonk, 2004). The qualitative responses in optimal root : leaf ratios, predicted by these models, are similar to the observation. This has long been considered a theoretical basis for the contention that plasticity in the root : leaf ratio allows plants to maximize their RGR and confers an adaptive advantage.

Real plants, however, may not regulate their root : leaf ratio exactly in the manner predicted by optimal biomass allocation models owing to constraints in the phenotypic plasticity of the root : leaf ratio. Plant morphological traits are under genetic control and are subject to natural selection (Bradshaw, 1965; Schilichting, 1986). Therefore, it has been assumed that plasticity in the root : leaf ratio is selected for by the environmental conditions of the habitat (Bradshaw, 1965; Grime et al., 1986; Schilichting, 1986). For example, Chapin (1980) suggested that plants from infertile soils should have high, inflexible root : leaf ratios in comparison with those of plants from fertile soils. If such constraints exist, the root : leaf ratio of species from infertile soils cannot be decreased to the optimal level in response to increased soil nitrogen availability, whereas it may be optimal or greater than optimal under conditions of low nitrogen availability. Van der Werf et al. (1993) hypothesized that plants partition their biomass close to the optimum when grown under nitrogen conditions similar to those of their native habitats, but not when grown under conditions that differ from those of their native habitats. In contrast to their expectation, Van der Werf et al. (1993) found that species from both fertile and infertile sites regulated their biomass close to their optimum under a range of nitrogen availability. However, more evidence is needed to determine the applicability of optimality models and to draw conclusions regarding which species optimize their root : leaf ratio under which conditions.

Because the optimal root : leaf ratio is by definition the root : leaf ratio at which RGR is maximized (i.e. maximum RGR), plants that fail to regulate their root : leaf ratio in an optimal manner are not expected to achieve maximum RGR. A larger than optimal root : leaf ratio causes a smaller than maximum RGR because of a smaller than optimal leaf area ratio (LAR, total leaf area per plant biomass). On the other hand, a smaller than optimal root : leaf ratio leads to a smaller than optimal leaf nitrogen concentration (LNC), which in turn results in a smaller net assimilation rate (NAR) and a smaller RGR. If plants cannot regulate their root : leaf ratio optimally as a consequence of constraints in plasticity, their RGR should be reduced following either of the two scenarios. Although this relationship between root : leaf ratio and RGR is an important basis for optimal biomass allocation models, few studies have tested these scenarios.

In this study, two herbaceous species, Polygonum cuspidatum from an infertile habitat and Chenopodium album from a fertile habitat, were grown under different nitrogen availabilities. The following questions were addressed: Do species in nitrogen-poor and nitrogen-rich sites both partition their biomass as predicted by optimal biomass allocation models? If not, do these plants have a smaller RGR than the predicted maximum RGR? To assess these questions, the root : leaf ratio, nitrogen concentration, and growth components of the plants were compared with those predicted by an optimal biomass allocation model (Osone and Tateno, 2003). Five harvests were conducted during the 64-day experimental period to evaluate whether plants achieve optimal growth at multiple points during a growth period.

MATERIALS AND METHODS

Plant materials

Two species from contrasting nutrient habitats were used in the experiment. Polygonum cuspidatum Sieb. et Zucc. is a perennial herb that is a typical pioneer species in Japan and colonizes extremely nitrogen-poor, bare ground following volcanic eruptions (Tateno and Hirose, 1987). Chenopodium album L. is a tall annual nitrophilic herb that grows vigorously in nitrogen-rich soils. Seeds of P. cuspidatum were collected from the slopes of Mt Fuji, where primary succession is in progress, and seeds of C. album were collected from the experimental gardens of Tohoku University in Sendai, Japan.

Plant growth

Plants were grown under five different nitrogen conditions that were controlled using nutrient solutions with different nitrogen concentrations. The seeds were sown in plastic pots (11 cm in diameter, 12 cm deep) filled with river sand, and the plants were grown in a greenhouse at the Nikko Botanical Garden in Nikko (37 °N, 139 °E), Japan. The experimental conditions in the greenhouse during the study period were as follows: relative photosynthetic photonflux density, 65–75 %; average temperature, 20·1 °C; average relative humidity, 75 %. Following Hirose and Kitajima (1986), the composition of the nutrients other than N in these solutions was 3 mm K2HPO4, 1 mm MgSO4 · 7H2O, 3 mm CaCl2, 25 μm H3BO3, 2 μm MnSO4 · 5H2O, 2 μm ZnSO4ċ7H2O, 0·5 μm CuSO4ċ5H2O, 0·5 μm Na2MoO4 · 2H2O and 20 μm Fe-EDTA. The addition of NH4NO3 to the basal solution yielded five solutions with final N concentrations of 0, 0·2, 2, 5 and 20 mm. The pH of the solutions was adjusted to 6·0 with 1 n HCl. Application of nutrient solutions was started 5 d after the plants germinated, and 300 mL of a solution was applied to each pot every 2 d during the early stages of growth. In the later stages of growth, nutrient solutions were applied once or twice a day, depending on plant size, to avoid depletion of nutrients in the pots and to maintain a constant supply of nitrogen in proportion to plant size (Poorter et al., 1995). Every 2 d, the pots were flushed with tap water to minimize salt accumulation.

Plants were harvested at 5, 20, 35, 50 and 64 d after germination. No reproductive growth occurred during the experiment. At each harvest, plants were washed out of the pots and divided into leaves, stems and roots. Leaf area was determined immediately after sampling. Dry masses of plant parts were measured after oven drying at 80 °C for 3 d. The nitrogen contents of the plant parts were measured using an automatic N/C analyser (NC-80, Shimadzu, Kyoto, Japan). At the first two harvests, when the plants were still small, leaf area, dry mass and nitrogen content were determined as totals of 10–15 plants, and the averages of a plant were calculated. At the last three harvests, four to eight plants were harvested for each treatment and species, and the leaf area, dry mass and nitrogen content of individual plants were determined.

Data analysis and simulations

Biomass characteristics (leaf mass ratio, stem mass ratio, root mass ratio and root : leaf ratio), components of growth analysis (RGR, LAR and NAR), and some of the parameters of the model [fraction of stem biomass in shoot biomass, specific absorption rate (SAR), specific leaf area (SLA)] were presented by data obtained in the lowest (0 mm N) and highest (20 mm N) nitrogen treatments. RGR, NAR and SAR were calculated from data for two successive harvests assuming that changes in leaf area and biomass were exponential between harvests (denoted as days 12, 27, 42 and 57). Other variables were calculated as the arithmetic means for the period between two successive harvests.

Optimal plant properties were determined by an optimal biomass allocation model developed by Osone and Tateno (2003). Equations of the model are presented in the Appendix. All parameters were determined in the present experiment. Following previous studies (e.g. Hirose, 1986; Van der Werf et al., 1993), the relationships between NAR and area-based LNC (LNCa) were obtained by regression using a rectangular hyperbolic function (see the Appendix, eqn 10). As the NAR–LNCa relationship differed depending on the date of harvest, regression curves were obtained individually for a harvest, pooling the data for the five nitrogen treatments. The data of P. cuspidatum on days 27 and 57 could not be regressed without being assigned a minimum N content (cmin in eqn 10). Thus, a cmin of 0·41 (derived from the regression for P. cuspidatum on day 42) was used for days 27 and 57. Data at 0·2 mm N were not available for P. cuspidatum on day 12; therefore, a regression curve obtained on day 27 was used. To determine the relationships between mass-based LNC (LNCm) and stem nitrogen concentration, and between LNCm and root nitrogen concentration, linear regressions were performed for the pooled data of all harvests of the five nitrogen treatments.

RESULTS

Parameters of the model

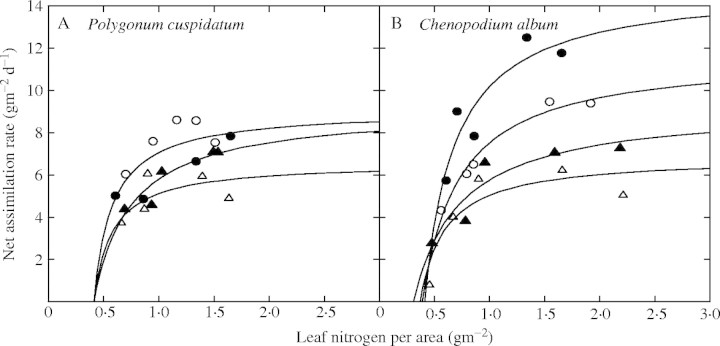
The parameters of the model differed among nitrogen treatments, harvest dates and species. Stem fraction (p2 in eqns 4 and 5, the proportion of stem biomass to shoot biomass) increased with plant growth except in P. cuspidatum in the high-nitrogen treatment (Table 1). The increase was the most remarkable in C. album in the high nitrogen treatment, where stem biomass reached about one-half of the shoot biomass at the end of experiment. SAR (nitrogen absorbed per unit root biomass per day) was more than ten times higher in high-nitrogen treatments than in low-nitrogen treatments for both species, reflecting the differences in the nitrogen concentrations of the nutrient solutions, and decreased over time for both species. In the high-nitrogen treatment, C. album showed a higher SAR than P. cuspidatum during early stages of growth (days 12 and 27), which suggests that the physiological ability to absorb nitrogen was greater in C. album than in P. cuspidatum. SLA also decreased with time for both species, and this decrease was more distinct in the high-nitrogen treatments. Differences in SLA between species were small, except during the initial stages of growth in the high-nitrogen treatment, where C. album had a larger SLA than P. cuspidatum. NAR increased with increasing LNCa and showed diminishing returns at larger LNCa for both species (Fig. 1). While this pattern was maintained at all harvests, the maximum NAR, the NAR value reached at larger LNCa (Amax in eqn 10), generally decreased with time. Again, maximum NAR was larger in C. album than in P. cuspidatum during the initial stages of growth. Stem and root nitrogen concentrations increased with increases in LNCm in both species (Fig. 2).

Table 1.

Means of stem fraction, specific absorption rate and specific leaf area of Polygonum cuspidatum and Chenopodium album

P. cuspidatum
 C. album
Days
 Low N
 High N
 Low N
 High N
Stem fraction 12 0·274 0·277 0·286 0·261
27 0·298 ± 0·089 0·200 ± 0·132 0·328 ± 0·014 0·271 ± 0·015
42 0·352 ± 0·136 0·207 ± 0·004 0·384 ± 0·011 0·382 ± 0·009
55 0·371 ± 0·154 0·259 ± 0·07 0·413 ± 0·017 0·512 ± 0·002
Specific absorption rate (gN g−1 d−1) 12 0·0060 0·0306 0·0069 0·1167
27 0·0037 ± 0·0007 0·0300 ± 0·0052 0·0040 ± 0·0006 0·0549 ± 0·0018
42 0·0016 ± 0·0008 0·0270 ± 0·0026 0·0020 ± 0·0007 0·0260 ± 0·0017
55 0·0012 ± 0·0005 0·0269 ± 0·0055 0·0006 ± 0·0007 0·0204 ± 0·0004
SLA (m2 g−1) 12 0·0343 0·0397 0·0314 0·0414
27 0·0312 ± 0·0009 0·0387 ± 0·0011 0·0316 ± 0·0021 0·0378 ± 0·0045
42 0·0287 ± 0·0018 0·0348 ± 0·0009 0·0336 ± 0·0021 0·0302 ± 0·0008
55 0·0294 ± 0·0012 0·0304 ± 0·0008 0·0329 ± 0·0022 0·0264 ± 0·0008
Open in a new tab

Fig. 1.

Fig. 1.

Open in a new tab

Relationships between net assimilation rate and area-based leaf nitrogen concentration for Polygonum cuspidatum (A) and Chenopodium album (B). Each point is the mean for each of the five nitrogen treatments. Data were fitted to a rectangular hyperbolic function individually for a harvest. Because the data of P. cuspidatum at 0·2 mm N was missed and reliable regression could not be done for day 12, the equation of day 27 was used for day 20. Filled circles, day 12; open circles, day 27; filled triangles, day 42, open triangles, day 57. Polygonum cuspidatum: y = 9·09(x − 0·41)/(0·17 + x − 0·41) (r = 0·75), day 12, 27; y = 9·14(x − 0·41)/(0·34 + x − 0·41) (r = 0·92), day 42; y = 6·59(x − 0·41)/(0·17 + x − 0·41) (r = 0·62), day 55. C. album: y = 15·0(x − 0·41)/(0·28 + x − 0·41) (r = 0·92), day 12; y = 11·7(x − 0·39) (0·34 + x − 0·39) (r = 0·99), day 27; y = 9·72 (x − 0·44)/(0·40 + x − 0·44) (r = 0·81), day 42; y = 6·87(x − 0·37)/(0·23 + x − 0·37) (r = 0·91).

Fig. 2.

Fig. 2.

Open in a new tab

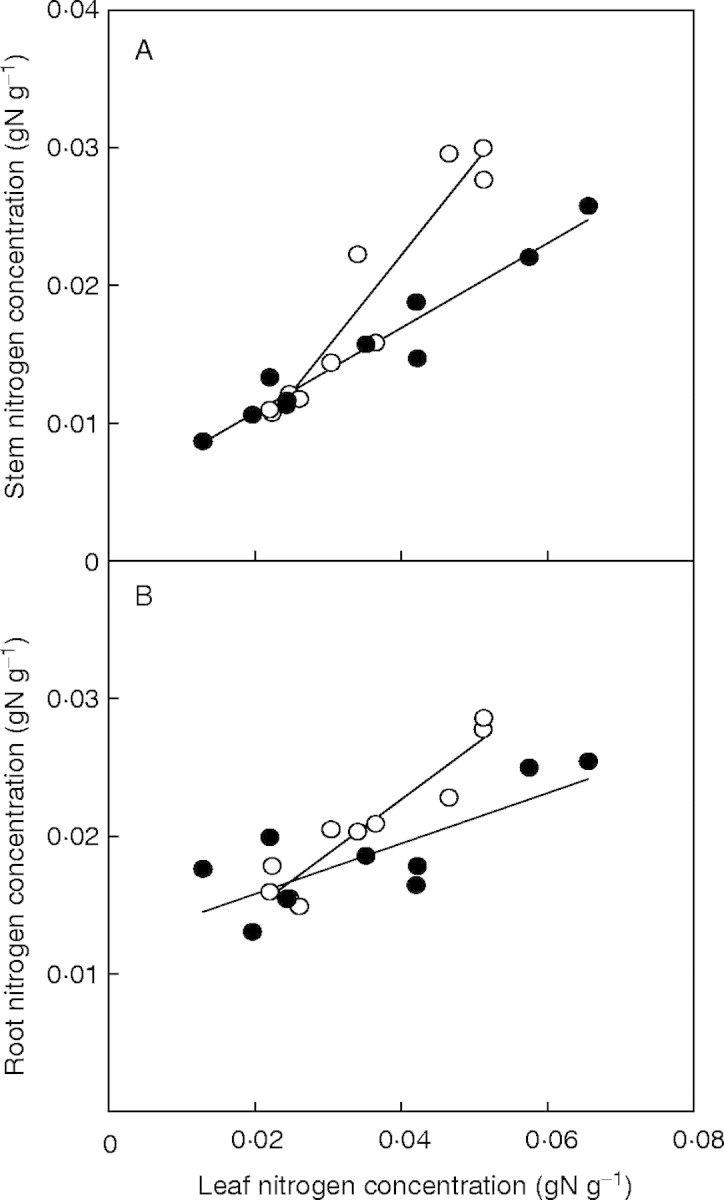
Stem nitrogen concentration (A) and root nitrogen concentration (B) as functions of leaf nitrogen concentration. Open circles, Polygonum cuspidatum; filled circles, Chenopodium album. Regressions were done for pooled data of all of the harvests and nitrogen treatments: y = 0·66x − 0·0043 (r = 0·94), stem nitrogen concentration of P. cuspidatum; y = 0·31x + 0·0047 (r = 0·97), stem nitrogen concentration of C. album; y = 0·39x + 0·0070 (r = 0·94), root nitrogen concentration of P. cuspidatum; y = 0·18x + 0·0122 (r = 0·78), root nitrogen concentration of C. album (modified from Osone and Tateno, 2003).

Biomass allocation and LNC

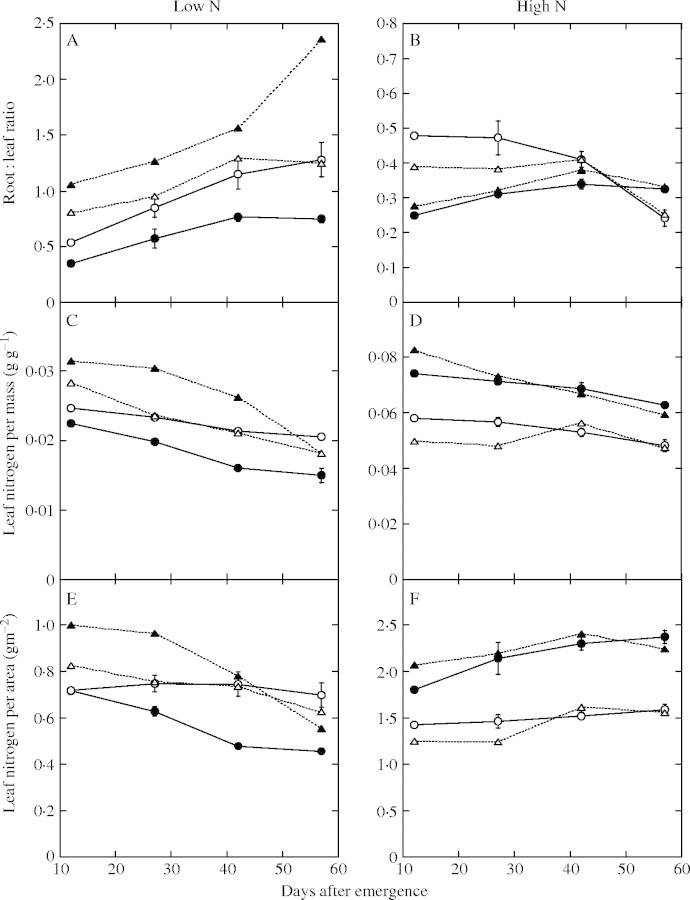
In the low-nitrogen treatment, the root : leaf ratio was larger in P. cuspidatum than in C. album (Fig. 3A, data points denoted by circles). The root : leaf ratio of P. cuspidatum was mostly consistent with its optimum (data points denoted by triangles) throughout the experimental period. Reflecting the accordance between the measured and optimal root : leaf ratios, LNCm and LNCa of P. cuspidatum were also very close to their optima (Fig. 3C and E). On the other hand, the root : leaf ratio of C. album was considerably smaller than its optimum (Fig. 3A), resulting in lower LNCm and LNCa than the optima (Fig. 3C and E).

Fig. 3.

Fig. 3.

Open in a new tab

Measured and optimal root : leaf ratio (A and B), optimal mass-based (C and D) and area-based LNC (E and F) of Polygonum cuapidatum and Chenopodium album. (A, C and E) Plants grown in the low nitrogen treatment (0 mm N); (B, D and F) plants grown in the high nitrogen treatment (20 mm N). Open circles, measured data of P. cuspidatum; open triangles, optimal data of P. cuspidatum; filled circles, measured data of C. album; filled triangles, optimal data of C.album. Bars indicate standard error.

In the high-nitrogen treatment, the root : leaf ratios of both species were generally smaller than in the low-nitrogen treatment (Fig. 3A and B). Polygonum cuspidatum had a large root : leaf ratio comparable to that in the low-nitrogen treatment at the start of experiment, but this ratio decreased over time; in contrast, C. album had a consistently low root : leaf ratio during the experiment (Fig. 3B). As a result, the root : leaf ratio was larger in P. cuspidatum than in C. album initially, but this relationship was reversed by the end of the experiment. The root : leaf ratio of P. cuspidatum was larger than its optimum in the early stages, but was almost optimal in the later stages (Fig. 3B). In concert with this, LNCm and LNCa of P. cuspidatum were also initially slightly larger than the optimum, but reached optimum in the later stages (Fig. 3D and F). The root : leaf ratio, LNCm and LNCa of C. album were close to their optima throughout.

Effects of optimizing the root : leaf ratio on RGR

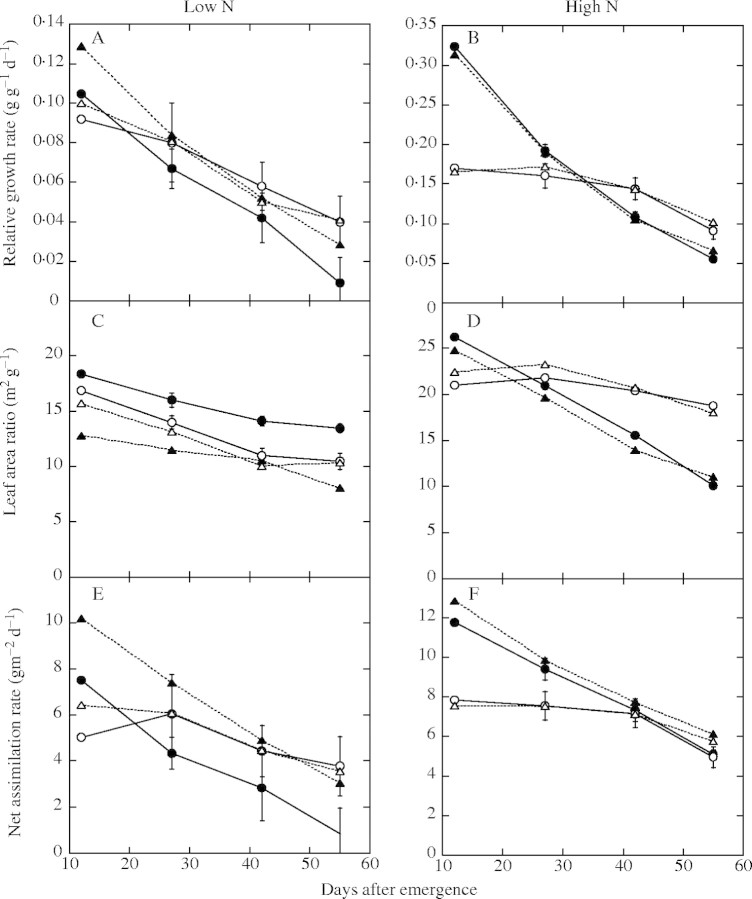
Figure 4 shows RGR and its two components, LAR and NAR. In the low-nitrogen treatment, P. cuspidatum, which optimally regulated the root : leaf ratio and LNCa (Fig. 3A and E), had LAR and NAR values close to their optima (Fig. 4C and E). Consequently, the measured RGR of P. cuspidatum was mostly consistent with the maximum RGR (Fig. 4A). Meanwhile, C. album, whose root : leaf ratio was smaller than the optimum (Fig. 3A), had a smaller than optimal LNCa (Fig. 3E) and thereby a considerably smaller than optimal NAR (Fig. 4E). This resulted in a smaller than maximum RGR in C. album (Fig. 4A), despite the larger than optimal LAR (Fig. 4C).

Fig. 4.

Fig. 4.

Open in a new tab

Measured and optimal relative growth rate (A and B), leaf area ratio (C and D) and net assimilation rate (E and F) of Polygonum cuapidatum and Chenopodium album. (A, C and E) Plants grown in the low nitrogen treatment (0 mm N); (B, D and F) plants grown in the high nitrogen treatment (20 mm N). Symbols are as shown in Fig. 3. Bars indicate standard error.

In the high-nitrogen treatment, because the root : leaf ratio of both species was regulated mostly in an optimal manner except in P. cuspidatum during the early stages of growth (Fig. 3B), plants also achieved optimal LAR, optimal NAR and maximum RGR (Fig. 4B, D and F). The initial differences in the measured and optimal root : leaf ratios of P. cuspidatum had a smaller effect on RGR and its two components.

DISCUSSION

Applicability of biomass allocation models

The present study indicated some constraints in phenotypic plasticity of the root : leaf ratio. Although C. album increased its root : leaf ratio in response to decreased nitrogen availability, it could not achieve the optimal root : leaf ratio in the low-nitrogen treatment (Fig. 3A and B). Chenopodium album, which usually inhabits nitrogen-rich environments, may not have the ability to increase its root : leaf ratio to the optimal level under nitrogen-limited conditions. Additionally, the root : leaf ratio of P. cuspidatum was initially larger than the optimum in the high-nitrogen treatment, while it reached its optimum later in the experiment (Fig. 3B). This suggests that P. cuspidatum has a high plasticity in the root : leaf ratio but the root : leaf ratio of its seedlings is adapted to low nutrient availability. Kachi and Rorison (1989) also demonstrated an initial larger than optimal root : leaf ratio in a species adapted to low soil fertility. Such evidence suggests that each species has intrinsic constraints in the plasticity of root : leaf ratio, which limit the practical use of optimal biomass allocation models under conditions seldom experienced by a species in its native habitat. Furthermore, whether P. cuspidatum attained an optimal root : leaf ratio depended on the harvest date (Fig. 3B), which highlights the importance of conducting multiple harvests when applying optimality models in practice.

Plants other than those discussed above regulated their root : leaf ratios close to their optima (Fig. 3A, B), suggesting a fairly wide applicability for optimal biomass allocation models. Limited studies that quantitatively compared measured and optimal root : leaf ratios also reported high accordance between the two. Van der Werf et al. (1993) showed that species from fertile and infertile habitats both achieved optimal root : leaf ratios under a wide range of nitrogen availabilities. Other studies have shown rather high agreement, as well as partial disagreement under extremely high- or low-nitrogen conditions (Hirose, 1988; Ågren and Franklin, 2003).

Effects of parameters on optimal properties

Optimal properties differed between the species in identical nitrogen treatments (Figs 3 and 4). This was due to the differences in parameters between the species (Table 1 and Fig. 1). Theoretically, a larger nitrogen absorption ability (represented as a larger SAR), which causes a larger nitrogen gain relative to carbon gain, decreases the optimal root : leaf ratio, and increases optimal LNCm and LNCa. On the other hand, factors that cause a large carbon gain relative to nitrogen gain, such as a larger SLA and larger NAR per unit LNCa (Amax in eqn 10), result in a larger optimal root : leaf ratio and smaller optimal LNCa. Additionally, a larger stem fraction causes a larger root : leaf ratio, LNCm and LNCa (Osone and Tateno, 2003). Chenopodium album had a larger SAR, SLA and NAR per unit LNC than P. cuspidatum, especially in the initial stages of growth in the high-nitrogen treatment (Table 1 and Fig. 1), which agrees with the general observation that fast-growing species from fertile habitats have a larger SLA, larger carbon assimilation rates per unit LNC, and larger nitrogen absorption ability of roots than do slow-growing species (e.g. Poorter and Remkes, 1990; Poorter et al., 1991; Reich et al., 1991, 1992, 1998; Wright and Westoby, 2000; Craine et al., 2001). In the high-nitrogen treatment, the nearly three-fold larger SAR of C. album largely caused the smaller optimal root : leaf ratio and larger optimal LNC in C. album than in P. cuspidatum during the initial stages of growth (Fig. 3). Later, the larger stem fraction of C. album caused the larger optimal root : leaf ratio and LNC of C. album. In the low nitrogen treatment, the larger SLA and NAR of C. album than P. cuspidatum caused the larger optimal root : leaf ratio and smaller LNC of C. album. Studies of biomass allocation models have focused mainly on the effects of external environmental conditions (e.g. Johnson and Thornley, 1987; Hirose, 1988; Hilbert, 1990; Gleeson, 1993; Kastner-Maresch and Mooney, 1994, for soil nitrogen availability; Hilbert et al., 1991; Ishizaki et al., 2003, for CO2; Hilbert et al., 1991, for photon flux density). The present results indicate that not only environmental factors but also intrinsic differences in parameters cause a considerable difference in optimal properties between species.

Growing evidence indicates that leaf and root properties largely differ between species of different potential growth rates, habitats and functional groups (e.g. Poorter and Remkes, 1990; Poorter et al., 1991; Reich et al., 1991, 1992, 1998; Wright and Westoby, 2000; Craine et al., 2001). According to the discussion above, these species could have different root : leaf ratios and LNC even when they grow under identical environmental conditions. From this point of view, Osone and Tateno (2005) demonstrated that the larger mass- and area-based LNC and light-saturated photosynthetic rates of herbs as compared with those of coexisting deciduous trees could be ascribed to the larger nitrogen absorption capacity of the herbs. These effects of parameters may also explain the lack of consistent patterns between species type (fast-growing or slow-growing) and root : leaf ratios or LMR (see Reynolds and D'Antonio, 1996; Aerts and Chapin, 2000). Theoretically, the combination of a larger SLA, larger carbon assimilation rates per unit LNC, and larger SAR, which is generally observed for fast-growing species (e.g. Poorter and Remkes, 1990; Poorter et al., 1991; Reich et al., 1991, 1992, 1998; Wright and Westoby, 2000; Craine et al., 2001), has contradictory effects on the root : leaf ratio: a larger SLA and NAR per unit LNCa increase the root : leaf ratio, and a larger SAR decreases the root : leaf ratio. Therefore, the optimal root : leaf ratio of a fast-growing species could be larger or smaller than that of a slow-growing species depending on which parameters differ more between the two species.

Parameter values also differed among harvests (Table 1). For both species, SLA, SAR, and carbon assimilation rates per unit LNC decreased and stem fraction increased as the plants grew, which agreed with general observations (e.g. Poorter and Pothmann, 1992, for SLA; Kitajima et al., 1997; Escudero and Mediavilla, 2003, for carbon assimilation rates per unit LNC; Robinson, 1986; Shipley and Meziane, 2002, for SAR; Niklas, 1992, for stem fraction). Decreases in SAR can be caused by root ageing or by more rapid depletion of nutrients with increasing plant size (Robinson, 1986; Shipley and Meziane, 2002). The frequency of fertilization was increased with increasing plant size to minimize nitrogen depletion. However, nitrogen depletion may be involved in the decreases in SAR since the decrease in SAR was most rapid in C. album in the high-nitrogen treatment, where absolute plant size was largest.

Owing to the changes in parameters (Table 1), the optimal properties of the species studied showed certain trajectories with time during the growth period (Figs 3 and 4). The measurements showed good agreement with the trajectories except in C. album under low-nitrogen levels, suggesting that plants have the ability to regulate biomass allocation so as to track the changes in the optimal root : leaf ratio during plant development and growth. However, the trajectories of optimal root : leaf ratios should be interpreted with caution. To determine the optimal root : leaf ratio, optimality models assume balanced exponential growth, in which plants grow exponentially with constant parameter values (e.g. Thornley, 1976). Therefore, the trajectories are not the optimal time courses of the root : leaf ratio that maximizes the biomass of a plant whose parameters change over time at the end of the growth period (‘global optimization’; Kastner-Maresch and Mooney, 1994). Rather, it should be interpreted as a series of optimal root : leaf ratios, each of which maximizes RGR in a plant presumed to undergo exponential growth with a set of parameters at a given harvest (‘local optimization’; Kastner-Maresch and Mooney, 1994). Since RGR decreased over the experimental period (Fig. 4), the species studied obviously did not undergo exponential growth at every instance during this period and, therefore, did not satisfy these assumptions. Nevertheless, the measured values showed good agreement with the optimal values. There is no good theoretical basis to explain this result. However, it is possible that, in certain cases, this type of optimality model can be used for plants that do not experience exponential growth. Kastner-Maresch and Mooney (1994) suggested that the optimal root : leaf ratios predicted by local optimization (similar to the present study) show a trajectory qualitatively similar to that of the optimal time course of the root : leaf ratio that maximizes the RGR over the whole growth period (global optimization). Wikström and Ågren (1995) also suggested that plants under non-steady growth rapidly achieved a root : leaf ratio assumed at steady-state growth.

Adaptive significance of plasticity in root : leaf ratios

Chenopodium album failed to optimize the root : leaf ratio and did not achieve maximum RGR in the low-nitrogen treatment (Figs 3A and 4A). The smaller than optimal root : leaf ratio caused a smaller than optimal LNCa, which in turn resulted in a smaller than optimal NAR. This is consistent with the scenario expected by optimal biomass allocation models. In contrast, P. cuspidatum in both nitrogen treatments and C. album in the high-nitrogen treatment achieved the optimal root : leaf ratio and also achieved optimal LNC, NAR, and LAR, thereby achieving the maximum RGR (Figs 3 and 4). These results suggest that regulating the root : leaf ratio in response to changes in external nitrogen availability or plant properties is adaptive in the sense that it allows plants to maximize RGR.

Why, then, did C. album show limited plasticity in the root : leaf ratio, which was only efficient at higher nitrogen availability, even though greater plasticity could have allowed it to be more successful under low nitrogen availability? This species may benefit from specializing in fertile environments. Maintaining phenotypic plasticity in a trait requires extra costs compared with having a fixed trait (Schlichting, 1986; DeWitt, 1998). A fixed trait requires only the production machinery (e.g. structural genes, ribosomes) that leads to an expected phenotype (DeWitt et al., 1998), whereas plasticity may involve additional mechanisms for sensing the environment, processing that information, and invoking regulation. Given that competition is more severe at productive sites (Grime, 1979), giving up these energetic costs and shifting the energy to growth may provide a large competitive advantage to plants in productive habitats. Moreover, in these habitats, competition is generally for light, and a taller plant acquires a disproportionate share of the resources (Weiner, 1990). Therefore, plants that decrease biomass allocation to above-ground parts sensitively in response to temporal nitrogen limitations may be overtopped by plants with a fixed low root : leaf ratio during a temporal decrease in nitrogen availability.

CONCLUSIONS

Chenopodium album from a fertile site failed to optimize its root : leaf ratio in the low-nitrogen treatment because of its limited ability to increase the root : leaf ratio in response to the nitrogen deficiency. In contrast, P. cuspidatum from an infertile site showed high plasticity in its root : leaf ratio, optimizing the root : leaf ratio under both low and high nitrogen levels. From these results, it is concluded that optimal biomass allocation models have fairly wide applicability, although they may not be applicable to plants grown under conditions that are very different from their original habitats. The sub-optimal root : leaf ratio of C. album in the low-nitrogen treatment resulted in a smaller than maximum RGR, whereas C. album in the high-nitrogen treatment and P. cuspidatum achieved their maximum RGRs. These results support the assumption of optimal biomass allocation models that regulation of the root : leaf ratio enables the maximization of RGR.

APPENDIX

Equations used in the model simulations are shown. For detailed explanation of the model, see Osone and Tateno (2003). A list of symbols is given in Table A1.

Table A1.

List of symbols and units used in the model

Symbols
 Descriptions
 Units
M Whole plant biomass g
ML Leaf biomass g
MS Stem biomass g
MR Root biomass g
AL Total leaf area m2
s Specific leaf area m2 g−1
N Total nitrogen content g
nL Mass based leaf nitrogen concentration gN g−1
nS Stem nitrogen concentration gN g−1
nR Root nitrogen concentration gN g−1
nLA Area based leaf nitrogen concentration gN m−2
P1 Allocation of biomass to shoots –
p2 Fraction of stem in the shoot biomass –
UL Net assimilation rate g m−2 day−1
UR Specific absorption rate gN g−1 day−1
c1 Constant for stem nitrogen concentration –
c2 Constant for stem nitrogen concentration g g−1
c3 Constant for root nitrogen concentration –
c4 Constant for root nitrogen concentration g g−1
Amax Maximum net assimilation rate g m−2 day−1
km Constant that determines the initial slope of net assimilation rate g m−2
cmin Minimum N content for assimilation g m−2
Open in a new tab

Whole plant biomass (M) is:

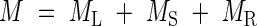graphic file with name M1.gif

where ML, MS and MR are the leaf, stem and root biomass, respectively.

Total leaf area (AL) is:

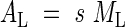graphic file with name M2.gif

where s is the specific leaf area.

Absolute growth rate is a product of the net assimilation rate (UL) and leaf area:

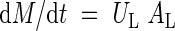graphic file with name M3.gif

Newly produced assimilates are allocated to roots and shoots according to an allocation coefficient, P1 (relative allocation of total assimilates to shoots). The assimilates allocated to shoots are further partitioned between leaves and stems according to a coefficient, p2 (stem biomass per total shoot biomass). Therefore, absolute growth rates of the leaves (dML/dt), stems (dMS/dt) and roots (dMR/dt) are:

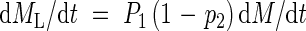graphic file with name M4.gif
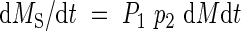graphic file with name M5.gif

and

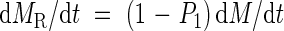graphic file with name M6.gif

where 0 < P1 < 1 and 0 ≤ p2 < 1.

Rate of nitrogen uptake is proportional to root biomass:

graphic file with name M7.gif

where N is plant nitrogen content and UR is specific absorption rate of roots (nitrogen uptake rate per unit of root biomass). Specific absorption rate represents both soil nitrogen availability and nitrogen absorption ability of the roots.

Stem (nS) and root (nR) nitrogen concentrations are defined as functions of leaf nitrogen concentration (nL):

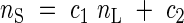graphic file with name M8.gif

and

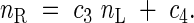graphic file with name M9.gif

Plant nitrogen (N) is partitioned among the three organs so that these relationships are satisfied.

Net assimilation rate is a rectangular hyperbolic function of the area-based LNC (nLA):

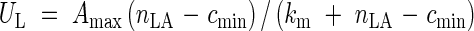graphic file with name M10.gif

where Amax is the maximum net assimilation rate, cmin is the minimum nitrogen concentration for assimilation, and km is a constant that determines the initial slope of the curve. nLA is given by:

graphic file with name M11.gif

To find optimal root : leaf ratio, LNC and maximum RGR of a plant at a given harvests, s, p2, UR, c1–c4, Amax, km, cmin are determined experimentally and P1 was varied for a given set of parameters. If the amount of biomass and total nitrogen at time t is given, the equations above calculate biomass and nitrogen increment at time t, and then amount of leaf area biomass and total nitrogen at time t + 1 is determined. If these calculations are repeated under given parameters and P1, the whole process approaches a steady-state exponential growth (Thornley, 1976), which defines RGR at steady-state. Under steady-state exponential growth, varying P1 yields a series of RGRs which plots a specific curve with a peak (= maximum RGR) against P1 for a given set of parameters. Optimal root : leaf ratio can be calculated as MR/ML at this maximum RGR.

Acknowledgments

We thank H. Nagashima, N. Osada, H. Taneda, A. Ishida, G. I. Ågren, an anonymous reviewer and the editor for valuable comments on an earlier version of this manuscript. We also thank S. Nemoto, H. Takahashi and the staff of Nikko Botanical Garden for assistance in the experiments. This research was supported by a fellowship from the Japan Society for the Promotion of Science (JSPS) for Japanese Junior Scientists.

Footnotes

†

Present address: Department of Plant Ecology, Forestry and Forest Products Research Institute, Matsunosato 1, Tsukuba, Ibaraki 305-8687, Japan.

LITERATURE CITED

  1. Aerts R, Chapin III FS. 2000. The mineral nutrition of wild plants revisited: a re-evaluation of processes and patterns. Advances in Ecological Research 30: 1–67. [Google Scholar]
  2. Ågren GI, Franklin O. 2003. Root : shoot ratios, optimization and nitrogen productivity. Annals of Botany 92: 795–800. [DOI] [PMC free article] [PubMed] [Google Scholar]
  3. Boot RGA, Den Dubbelden KC. 1990. Effects of nitrogen supply on growth, allocation and gas exchange characteristics of two perennial grasses from inland dunes. Oecologia 85: 15–121. [DOI] [PubMed] [Google Scholar]
  4. Bradshaw AD. 1965. Evolutionary significance of phenotypic plasticity in plants. Advances in Genetics 13: 115–155. [Google Scholar]
  5. Brouwer R. 1962. Distribution of dry matter in the plant. Netherlands Journal of Agricultural Science 10: 361–376. [Google Scholar]
  6. Chapin III FS. 1980. The mineral nutrition of wild plants. Annual Review of Ecology and Systematics 11: 233–260. [Google Scholar]
  7. Craine JM, Froehle J, Tilman DG, Wedin DA, Chapin III FS. 2001. The relationships among root and leaf traits of 76 grassland species and relative abundance along fertility and disturbance gradients. Oikos 93: 274–285. [Google Scholar]
  8. DeWitt TJ, Sih A, Wilson DS. 1998. Costs and limits of phenotypic plasticity. Trends in Ecology and Evolution 13: 77–81. [DOI] [PubMed] [Google Scholar]
  9. Escudero A, Mediavilla S. 2003. Decline in photosynthetic nitrogen use efficiency with leaf age and nitrogen resorption as determinants of leaf life span. Journal of Ecology 91: 880–889. [Google Scholar]
  10. Gleeson SK. 1993. Optimization of tissue nitrogen and root-shoot allocation. Annals of Botany 71: 23–31. [Google Scholar]
  11. Grime JP. 1979.Plant strategies and vegetation processes. Chichester: John Wiley. [Google Scholar]
  12. Grime JP, Crick JC, Rincon JE. 1986. The ecological significance of plasticity. In: Jennings, DH, Trewavas, AJ, eds. Plasticity in plants. Cambridge: The Company of Biologists, 5–30. [Google Scholar]
  13. Hilbert DW. 1990. Optimization of plant root : shoot ratios and internal nitrogen concentration. Annals of Botany 66: 91–99. [Google Scholar]
  14. Hilbert DW, Larigauderie A Reynolds JF. 1991. The influence of carbon dioxide and daily photon-flux density on optimal leaf nitrogen concentration and root:shoot ratio. Annals of Botany 68: 365–376. [Google Scholar]
  15. Hirose T. 1986. Nitrogen uptake and plant growth. II. An empirical model of vegetative growth and partitioning. Annals of Botany 58: 487–496. [Google Scholar]
  16. Hirose T. 1988. Nitrogen availability, optimal shoot/root ratios and plant growth. In: Werger MJA, van der Aart PJM, During HJ, Verhoeven, JTA, eds. Plant form and vegetation structure. The Hague: SPB Academic Publishing, 135–145. [Google Scholar]
  17. Hirose T, Kitajima K. 1986. Nitrogen uptake and plant growth I. Effect of nitrogen removal on growth of Polygonum cuspidatum Annals of Botany 58: 479–486. [Google Scholar]
  18. Ishizaki S, Hikosaka K, Hirose T. 2003. Increase in leaf mass per area benefits plant growth at elevated CO2 concentration. Annals of Botany 91: 905–914. [DOI] [PMC free article] [PubMed] [Google Scholar]
  19. Johnson IR, Thornley JHM. 1987. A model of shoot : root partitioning with optimal growth. Annals of Botany 60: 133–142. [Google Scholar]
  20. Kachi N, Rorison IH. 1989. Optimal partitioning between root and shoot in plants with contrasted growth rates in response to nitrogen availability and temperature. Functional Ecology 3: 549–559. [Google Scholar]
  21. Kastner-Maresch AE, Mooney HA. 1994. Modelling optimal plant biomass partitioning. Ecological Modelling 75/76: 309–320. [Google Scholar]
  22. Kitajima K, Mulkey SS, Wright SJ. 1997. Decline of photosynthetic capacity with leaf age in relation to leaf longevities for five tropical canopy tree species. American Journal of Botany 84: 702–708. [PubMed] [Google Scholar]
  23. Niklas KJ. 1992.Plant biomechanics. Chicago: University of Chicago Press. [Google Scholar]
  24. Olff H. 1992. Effects of light and nutrient availability on dry matter and N allocation in six successional grass species. Testing for resource ratio effects. Oecologia 89: 412–421. [DOI] [PubMed] [Google Scholar]
  25. Olff H, Van Andel J, Bakker JP. 1990. Biomass and shoot/root allocation of five species from a grassland succession series at different combinations of light and nutrient supply. Functional Ecology 4: 193–200. [Google Scholar]
  26. Osone Y, Tateno M. 2003. Effects of stem fraction on the optimization of biomass allocation and maximum photosynthetic capacity. Functional Ecology 17: 627–636. [Google Scholar]
  27. Osone Y, Tateno M. 2005. Nitrogen absorption by roots as a cause of interspecific variations in leaf nitrogen concentration and photosynthetic capacity. Functional Ecology (in press). [Google Scholar]
  28. Poorter H, Pothmann P. 1992. Growth and carbon economy of a fast-growing and a slow growing grass species as dependent on ontogeny. New Phytologist 120: 159–166. [Google Scholar]
  29. Poorter H, Remkes C. 1990. Leaf area ratio and net assimilation rate of 24 wild species differing in relative growth rate. Oecologia 83: 533–559. [DOI] [PubMed] [Google Scholar]
  30. Poorter H, Claudiusm ADM, Van de Vijver CADM, Boot RGA, Lambers H. 1995. Growth and carbon economy of a fast-growing and slow-growing grass species as dependent on nitrate supply. Plant and Soil 171: 217–227. [Google Scholar]
  31. Poorter H, Van der Werf A, Atkin OK, Lambers H. 1991. Respiratory energy requirements of roots vary with the potential growth rate of a plant species. Physiologia Plantarum 83: 469–475. [Google Scholar]
  32. Reich PB, Uhl C, Walters MB, Ellsworth DS. 1991. Leaf life span as a determinant of leaf structure and function among 23 Amazonian tree species. Oecologia 86: 16–24. [DOI] [PubMed] [Google Scholar]
  33. Reich PB, Walters MB, Ellsworth DS. 1992. Leaf life span in relation to leaf, plant, and stand characteristics among diverse ecosystems. Ecological Monographs 62: 365–392. [Google Scholar]
  34. Reich PB, Walters MB, Tjoelker MG, Vanderklein DW, Buschena C. 1998. Photosynthesis and respiration rates depend on leaf and root morphology and nitrogen concentration in nine boreal tree species differing in relative growth rate. Functional Ecology 12: 395–405. [Google Scholar]
  35. Reynolds HL, D'Antonio C. 1996. Ecological significance of plasticity in root weight ratio in response to nitrogen: opinion. Plant and Soil 185: 75–97. [Google Scholar]
  36. Robinson D. 1986. Limits to nutrient inflow rates in roots and root systems. Physiologia Plantarum 68: 551–559. [Google Scholar]
  37. Shilichting CD. 1986. The evolution of phenotypic plasticity in plants. Annual Review of Ecology and Systematics 17: 667–693. [Google Scholar]
  38. Shipley B, Meziane D. 2002. The balanced-growth hypothesis and the allometry of leaf and root biomass allocation. Functional Ecology 16: 326–331. [Google Scholar]
  39. Tateno M, Hirose T. 1987. Nitrification and nitrogen accumulation in the early stages of primary succession on Mt. Fuji. Ecological Research 2: 113–120. [Google Scholar]
  40. Thornley JHM. 1976.Mathematical models in plant physiology. London: Academic Press. [Google Scholar]
  41. Thornley JHM. 1995. Shoot : root allocation with respect to C, N and P: an investigation and comparison of resistance and teleonomic models. Annals of Botany 75: 391–405. [Google Scholar]
  42. Van der Werf A, Visser AJ, Schieving F, Lambers H. 1993. Evidence for optimal partitioning of biomass and nitrogen at a range of nitrogen availabilities for a fast- and slow-growing species. Functional Ecology 7: 63–74. [Google Scholar]
  43. Weiner J. 1990. Asymmetric competition in plant populations. Trends in Ecology and Evolution 5: 360–364. [DOI] [PubMed] [Google Scholar]
  44. Wikström F, Ågren GI. 1995. The relationship between the growth rate of young plants and their total-N concentration is unique and simple: a comment. Annals of Botany 75: 541–544. [Google Scholar]
  45. Wright IJ, Westoby M. 2000. Cross-species relationship between seedling relative growth rate, nitrogen productivity and root vs leaf function in 28 Australian woody species. Functional Ecology 14: 97–107. [Google Scholar]
  46. Yin X, Schapendonk AHCM. 2004. Simulating the partitioning of biomass and nitrogen between roots and shoot in crop and grass species. NJAS-Wageningen Journal of Life Sciences 51: 407–426. [Google Scholar]

Articles from Annals of Botany are provided here courtesy of Oxford University Press

RESOURCES