Trapping the ATP binding state leads to a detailed understanding of the F₁-ATPase mechanism

Significance

F₁-ATPase is a motor protein that converts the free energy of binding of ATP and its hydrolysis products ADP and P_i into a mechanical force for γ-subunit rotation. It is the catalytic moiety of F_oF₁-ATPase, which synthesizes ATP. There are two metastable states along each 120° rotation of the γ-subunit, one associated with ATP hydrolysis (the “catalytic dwell”) and the other with ATP binding (the “ATP waiting dwell”). We use molecular simulations to determine the ATP waiting dwell structure. With this structure and the catalytic dwell X-ray structure, we develop an atomic-level model of the coupling between ATP hydrolysis and γ-subunit rotation. The molecular-level understanding of this motor will aid in its use in nanomachines and cancer therapy.

Abstract

The rotary motor enzyme F_oF₁-ATP synthase uses the proton-motive force across a membrane to synthesize ATP from ADP and P_i (H₂PO₄⁻) under cellular conditions that favor the hydrolysis reaction by a factor of 2 × 10⁵. This remarkable ability to drive a reaction away from equilibrium by harnessing an external force differentiates it from an ordinary enzyme, which increases the rate of reaction without shifting the equilibrium. Hydrolysis takes place in the neighborhood of one conformation of the catalytic moiety F₁-ATPase, whose structure is known from crystallography. By use of molecular dynamics simulations we trap a second structure, which is rotated by 40° from the catalytic dwell conformation and represents the state associated with ATP binding, in accord with single-molecule experiments. Using the two structures, we show why P_i is not released immediately after ATP hydrolysis, but only after a subsequent 120° rotation, in agreement with experiment. A concerted conformational change of the α₃β₃ crown is shown to induce the 40° rotation of the γ-subunit only when the β_E subunit is empty, whereas with P_i bound, β_E serves as a latch to prevent the rotation of γ. The present results provide a rationalization of how F₁-ATPase achieves the coupling between the small changes in the active site of β_DP and the 40° rotation of γ.

The molecular motor F_oF₁-ATP synthase is composed of two domains: a transmembrane portion (F_o), the rotation of which is induced by a proton gradient, and a globular catalytic moiety (F₁) that synthesizes and hydrolyzes ATP. The primary function of the proton-motive force acting on F_oF₁-ATP synthase is to provide the torque required to rotate the γ-subunit in the direction for ATP synthesis (1, 2). The catalytic moiety, F₁-ATPase, has an α₃β₃ “crown” composed of three α- and three β-subunits arranged in alternation around the γ-subunit, which has a globular base and an extended coiled-coil portion (3) (Fig. 1A). F₁-ATPase by itself binds ATP and hydrolyzes it to induce rotation of the γ-subunit (in the opposite direction from that for synthesis) on the millisecond time scale under optimum conditions (4, 5). All of the α- and β-subunits bind nucleotides, but only the three β-subunits are catalytically active. The original crystal structure (3) of F₁-ATPase from bovine heart mitochondria (MF₁) led to the identification of three conformations of the β-subunit: β_E (empty), β_TP (ATP analog bound), and β_DP (ADP bound); Fig. 1A. In the known structures of F₁-ATPase, which apparently are near the “catalytic dwell” state, the state in which catalysis occurs (6, 7), the β_E subunit conformation is partly to fully open and is very different from those of the β_TP and β_DP subunits, which are closed and very similar to each other (SI Appendix, SI1).

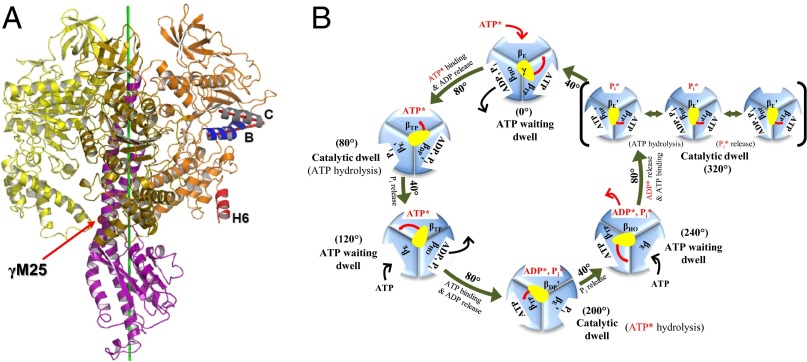
Fig. 1.

(A) F₁-ATPase. The three β-subunits and the γ-subunit are shown (α-subunits are not shown for clarity): β_E (yellow), β_DP (orange), β_TP (gold), and γ (purple). To define the β_DP subunit conformation we use the angle between helix B (βT163-A176) and helix C (βT190-G204). The two helices are highlighted: helix B (blue) and helix C (gray); the B^C angle is depicted as a red angle. The β_DPH6 helix, whose orientation was reported to undergo a 20° change during the 40° substep γ-rotation, is highlighted as red. During the forced rotation simulations with an external torque, the force acts on the C_α atom of MF₁:γM25 (shown as a red sphere). The direction of the force is determined as the cross-product of the radial vector of γM25:C_α and the rotational axis (green). (B) Proposed 360° rotation cycle of F₁-ATPase showing the subunit conformations, as well as the binding–release of ligands and the hydrolysis of ATP. Starting from the binding of an ATP* to the β_E subunit in the ATP waiting state (0°), rotation of the γ-stalk by 200° (80°, 40°, 80°) leads to the transition of β_E (γ = 0°) via β_TP (γ = 80°) to β_DP (γ = 200°), the catalytic dwell state where hydrolysis of ATP* takes place. The hydrolysis product P_i* in the β_DP subunit is not released at this catalytic dwell (200°). Instead, the other hydrolysis product ADP* is released first after a 40° rotation [β_DP (200°) → β_HO (240°)]. Then, β_HO is transformed to β_E and P_i* is released after an additional 80° rotation to another catalytic dwell state (320°); the latter is shown in brackets outside the main cycle (see below). Finally, the release of P_i* from β_E leads to a 40° rotation that completes the 360° cycle (21, 41). The other subunits are going through corresponding cycles offset by 120° (β_DP) and 240° (β_TP), respectively. Here, the prime symbol when it appears on the β_DP and β_E conformations indicates that the conformation of corresponding subunits change slightly in or near the specified reaction steps. The γ-subunit is shown as a yellow oval, and its rotation during the hydrolysis cycle is indicated by a red arrow. The reaction steps occurring in or near the catalytic dwell and corresponding changes of ligands in each β-subunit are also shown in the 320° catalytic dwell: The first state (Left in the 320° catalytic dwell) has a bound ATP in β_DP′, and is thus referred to as a prehydrolysis state (the state before the hydrolysis of ATP during the catalytic dwell). The second state (Middle) represents the state after ATP hydrolysis (posthydrolysis state), and the third state (Right) presents the state after the release of P_i bound in β_E′ (postrelease state).

Searching for the ATP Waiting State

Because no X-ray structure is available for the ATP waiting state, we searched for it by molecular dynamics (MD) simulations with an external torque applied to the γ-subunit in the hydrolysis direction while introducing different conformations of the β_DP subunit in the α₃β₃ crown, in accord with suggestions from single-molecule experiments (8). The results are shown in Fig. 2 (see Methods and SI Appendix, SI2 for details of the simulations). In Fig. 2, we refer 200° for the γ-rotation angle of the catalytic dwell state and 240° for the ATP waiting dwell state, respectively, to stress that the hydrolysis of an ATP, denoted as ATP*, bound after the ATP waiting dwell at 0°, takes place at the 200° catalytic dwell state (see Fig. 1B for the rotation angle of γ relative to the α₃β₃ complex). The initial simulation used the “Walker” crown structure [Protein Data Bank (PDB) ID code 1BMF] (3), in which the β_DP subunit is closed with the angle (BˆC) formed by helices B and C equal to 21.6°, and the γ-subunit structure of Gibbons et al. (PDB ID code 1E79) (9) (Fig. 1; see Methods for system preparation). It was represented by an all-atom model based on the CHARMM program (10), combined with a coarse-grained plastic network model (PNM) (11, 12). Even for an applied torque of 2,500 pN·nm, much higher than is generated in the normal function (13), the γ-subunit, which has an initial rotation angle of 200°, stalled at an angle of about 220°. In the present work, we define the rotation angle of γ as the angle formed between an instantaneous vector and a reference vector, each defining the orientation of γ relative to the three β-subunits for the instantaneous configuration from MD or the reference Walker structure, respectively (see Methods and SI Appendix, SI2 for angle definition). Major clashes between residues γS12-I16 and β_DPL384-I388, near the DELSEED motif, prevented further rotation (SI Appendix, SI3 and Fig. S1). When the external torque was removed, the γ-subunit returned to within 2.5° of the crystal orientation in 200 ps, indicating that for the Walker crown structure, the γ-subunit orientation (200°) is a minimum.

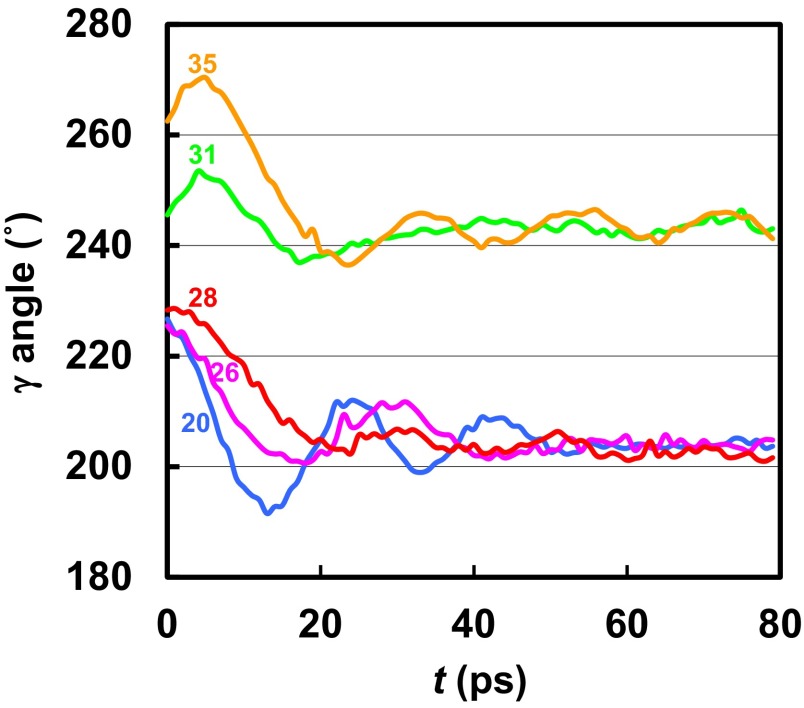
Fig. 2.

Rotational angle of the γ-subunit as a function of time during the relaxation simulations (see text) for different B^C angle values of the β_DP subunit after the simulations with the applied torque. The values of the B^C angle in the β_DP subunit are shown for each trajectory to indicate the stage of binding cleft opening during the transition from the β_DP (B^C = 21.6°) to the β_E (B^C = 48.6°) conformation. The B^C angles were maintained at their initial values by the PNM restraining potentials during the simulations.

To find the ATP waiting state, we restarted the external torque simulations with more open conformations for the β_DP subunit, keeping the rest of the crown near its original structure with the PNM. Fig. 2 shows the γ-rotation angle and the (BˆC) angle of β_DP along the simulated conformational transition of β_DP to the more open conformation in the presence of the external torque. For a slightly more open β_DP subunit (BˆC = 28°), similar to that of the half-open β_HO conformation (BˆC = 23°; PDB ID code 2HLD_I, where “I” denotes the first α₃β₃γ-complex among the three complexes in the crystallographic asymmetric unit of 2HLD) (14), the γ-subunit returned to fluctuate around 202°. With the β_DP subunit having BˆC equal to 31° and 35°, similar to that of the half-closed β_HC conformation (BˆC = 32°) (see also below) in the Menz et al. structure (PDB ID code 1H8E) (15), the γ-subunit rotated to 255° and 271°, respectively, before it stalled again. When the torque was removed, the γ-subunit relaxed rapidly in both cases to near 240° and remained there during the rest of the simulation. The results show that there exists a locally stable state with the γ-rotation angle near 240° and the α₃β₃ crown with the catalytic subunits having conformations corresponding to β_E-like, β_HC-like (based on the BˆC angle), and β_TP-like. We note that the BˆC angles are β_E = 49°, β_HC = 32°, β_HO = 23°, β_DP = 22°, and β_TP = 19°.

To check the trapping simulation, we used an alternative protocol (SI Appendix, SI2) and experimental data from Masaike et al. (8), who estimated that the helix-6 angle of β_DP is rotated by 20° in the ATP waiting state. The β_DP subunit and the γ-subunit were subjected to a biased simulation (16) and it was found that for the partly open structure of β_DP (helix-6 angle equal 20°; BˆC = 23°) the γ-subunit had rotated by 40° to reach the 240° state. A number of interactions stabilize the 240° state (see Fig. 3 and SI Appendix, SI4 for details and a comparison with the interactions in the trapped structure). The structure was then subjected to all-atom explicit water MD simulations with no PNM (see SI Appendix, SI5 for details). Throughout the simulation (20 ns), the γ-stalk stayed near 240°, supporting the fact that it is a (locally) stable state. In SI Appendix, Fig. S2 A and B, we show the structure from the simulation, and compare it with the structure at the catalytic dwell. The conformations of β_DP for the two states differ as expected. Comparisons of β_DP with various β-conformations in crystal structures in terms of the rmsd values and the BˆC angles (SI Appendix, Fig. S2 C and D) indicate that the conformation of the partly open β_DP structure is between β_HO and β_HC. Interestingly, the partly open β_DP subunit shares many structural features with β_DP in the recent F₁-ATPase structure of Rees et al. (PDB ID code 4ASU) (17); its BˆC angle equals 24° and the helix-6 angle is close to 20°. In addition to the fact that the γ-subunit of the Rees et al. structure is rotated only by 32° relative to the reference Walker structure, the most significant difference between the present model structure and the structure by Rees et al. is that the C-terminal domain of β_DP of the model ATP waiting dwell structure clashes sterically with the γ-subunit of the Rees et al. structure (SI Appendix, Fig. S2B). One possible reason for the observed difference is that the structure trapped by Rees et al. contains nucleotide in all three active sites; therefore, it may represent another intermediate, rather than the ATP waiting dwell state modeled here. Although both the present work and Rees et al. (17) show a partial opening of β_DP, it is not observed in the model structure of the intermediate state proposed by Okazaki and Hummer (18). In addition, we observe that the C-terminal helix–turn–helix (hth) motif of β_E has closed slightly in the MD simulated structure (SI Appendix, Fig. S2A). Although Masaike et al. (8) concluded in their single-molecule experiments that β_E does not change its C-terminal helix orientation during the 40° substep rotation, the large fluctuations of the orientation angle in the measurements suggest that the small change of β_E observed in the MD simulation is below their resolution. We use this experimentally derived structure for the analysis that follows. Because the structure of β_DP is closest in rmsd to β_HO (SI Appendix, Fig. S2C), we denote the conformation of the partly open β_DP as β_HO-like (or simply β_HO for brevity when no confusion arises).

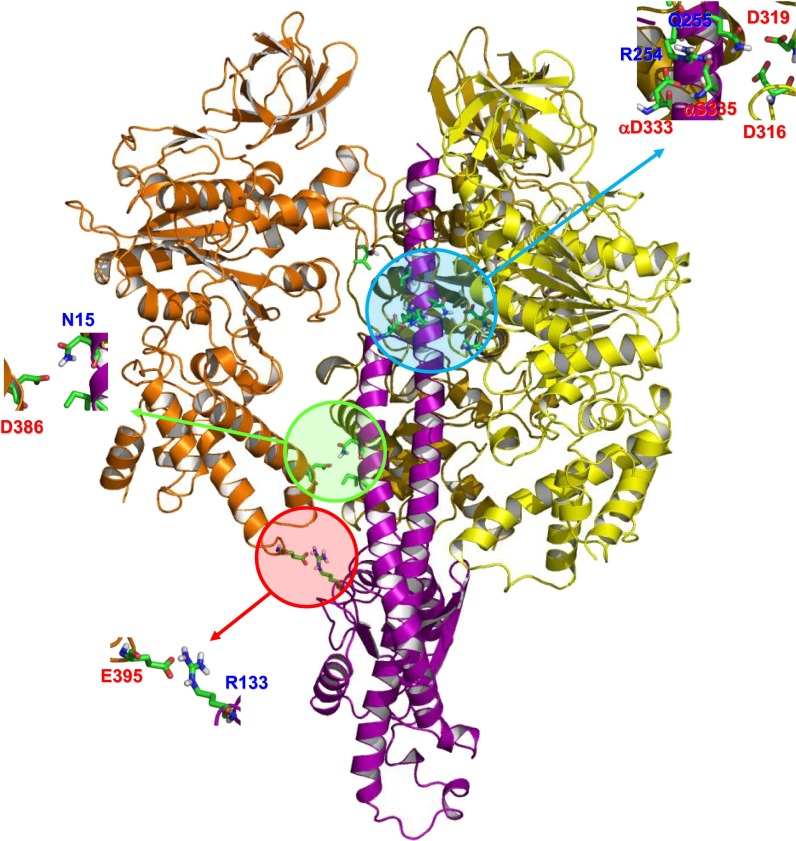
Fig. 3.

Interactions between the γ-subunit and the surrounding subunits for a representative structure of the simulated ATP waiting state. The color scheme is the same as Fig. 1. Residues from the γ-subunit are labeled in blue, and residues from other subunits are labeled in red. Each residue involved in the interaction is shown in stick representation.

Timing of P_i Release

The results from free-energy simulations and multiple MD simulations (SI Appendix, SI6 and Fig. S3) indicate that any of ATP hydrolysis, ADP and/or P_i release, can lead to the partly open β_HO-like conformation required to reach the ATP waiting state at 240° from the catalytic dwell (200°). All of these, as well as ADP and P_i repulsion, have been suggested as triggers for the γ-rotation (19–21). To investigate possibilities for the actual mechanism, we calculated the probability of ligand release, particularly of P_i, from different conformations of the β-subunits with different ligand occupancies. Given the results shown in Fig. 4 and additional experimental data [particularly Watanabe et al. (21)], we summarize in Fig. 1B the rotation cycle of the subunit conformations and their occupations. For an ATP, denoted as ATP*, bound after the ATP waiting dwell at 0°, the release of P_i* generated from the ATP* is shown to occur after an additional 120° rotation of the γ-subunit to 320° from the catalytic dwell at 200° where the ATP* hydrolysis takes place. This contrasts with an earlier conclusion, also based on single-molecule experiments, that P_i is released immediately after ATP* hydrolysis at 200° (20, 22, 23).

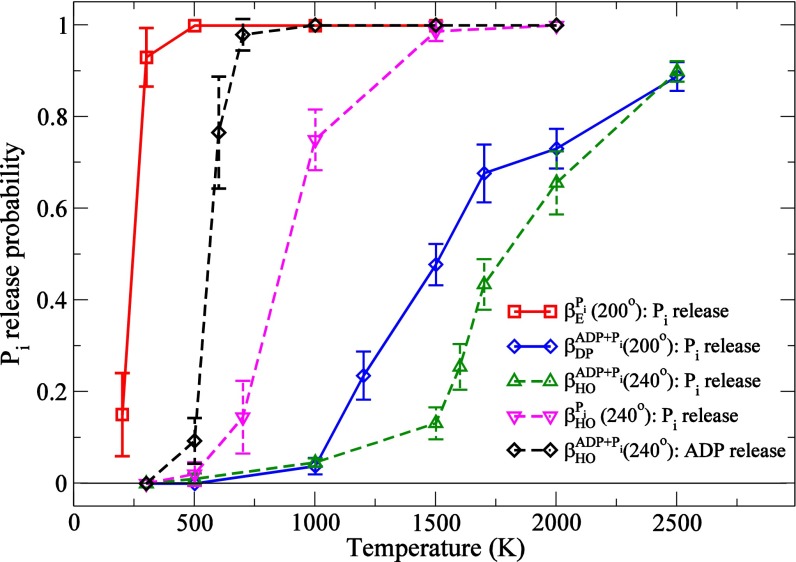
Fig. 4.

Probability of P_i release as a function of the temperature applied to P_i in the MCES simulations (see Methods): P_i release from β_E in the catalytic dwell (200°; red), and the release from ADP and P_i bound β_DP at 200° (blue). The angle definition is provided in Fig. 1B. The results test the two competing models for P_i release: one from β_DP immediately after its cleavage from bound ATP and the other from β_E after the rotation of γ by 120° to reach the next catalytic dwell, during which the P_i release from β_DP is prevented. The latter model corresponds to the reaction cycle proposed in the present paper and Watanabe et al. (21). We also show the release probabilities of P_i and ADP from the β_HO conformation at the ATP waiting dwell with different ligand contents: P_i release in the presence of ADP (green), P_i release in the absence of ADP (pink), and ADP release in the presence of P_i (black).

Because the P_i and ADP release takes place on the millisecond time scale, too long to be sampled in accessible simulation times at 300 K (nanoseconds), we use the high-temperature multicopy enhanced sampling (MCES) method (see Methods) to accelerate the events. This approach has been used previously to provide meaningful results on ligand release from proteins and its dependence on their conformation [e.g., CO from myogobin by Elber and Karplus (24); P_i from myosin by Cecchini et al. (25)]. MCES was used here to explore the ligand release probabilities of P_i and ADP from subunits with the conformations β_E, β_DP, and β_HO-like as part of the α₃β₃γ-complex. For β_E and β_DP, we considered a structure at or near the catalytic dwell (200°), based on the X-ray structure of Braig et al. (PDB ID code 1E1R) (26); for β_OH, we considered the ATP waiting dwell (240°), based on the model structure reported here. Fig. 4 shows the probabilities of P_i release from β-subunits in different conformational states as a function of the temperature of the multiple copies of P_i. In the Braig et al. structure used for the catalytic dwell state (200°), β_E is occupied by P_i; β_DP by ADP, AlF₃ (which was replaced with P_i), and Mg²⁺; and β_TP by ATP analog and Mg²⁺. The P_i present in β_E was produced during the catalytic dwell at 80° from an ATP bound at −120°. Because P_i has a high release probability from β_E at a temperature as low as 250 K, it is very weakly bound. Interestingly, in one of the all-atom explicit water MD simulations (see below), we observed the spontaneous release and rebinding of P_i from β_E (Movie S1). The release and rebinding accompany a large fluctuation of the P-loop structure. This finding is consistent with the present P_i release data. By contrast, a much higher temperature (1,500 K) is required for a significant release probability of P_i from the closed β_DP subunit in the catalytic dwell structure (200°). This result indicates that in the 200° structure, release of P_i from β_E is the dominant process and that release of P_i* from β_DP immediately after its cleavage from ATP* does not occur to a significant extent, in accord with Fig. 1B and the proposal of Watanabe et al. (21).

In the ATP waiting state (240°), where β_E is empty (Fig. 1B), β_DP has opened more to become β_HO, but P_i* release is even more hindered than in the 200° structure as long as ADP* (and Mg²⁺) is present (Fig. 1B); i.e., release of P_i* at 240° would be possible only after ADP* has been released (also see SI Appendix, SI7, SI8, and Fig. S4). To confirm this result, we performed a set of MCES simulations for β_HO in the 240° structure, in which ADP and P_i were both represented by multiple copies and thus competed for release. As expected, ADP* is released at a significantly lower temperature than P_i* (Fig. 4). Once ADP* is no longer present, P_i* is released easily (SI Appendix, Fig. S5). However, as shown by Adachi et al. (20) and Martin et al. (27), ADP* is released only during (or after) the rotation of the γ-subunit by another 80° to the catalytic dwell at 320° when β_HO has opened further to β_E (see Fig. 1B legend). Very recently, Czub and Grubmüller have shown by MD simulations that β_E closes spontaneously to β_HO during the 80° rotation in the synthesis direction in the absence of ligand (ADP or P_i) in β_E (28). However, because changes of the ligand occupation of each β-subunit during the rotation were not taken into account in the simulations, an understanding of the entire sequence of events that occurs during the 80° rotation is not possible based on their results. Nevertheless, the results are consistent with the mechanism that the β_HO → β_E conformational transition and the rotation of γ to the catalytic dwell occur during or after the release of ADP*. Taken together, the P_i release simulations show that P_i* is released after the β_HO → β_E transition is completed as part of the rotation from 320° to 360°.

Coupling Between ATP Hydrolysis, P_i Release, and γ-Rotation

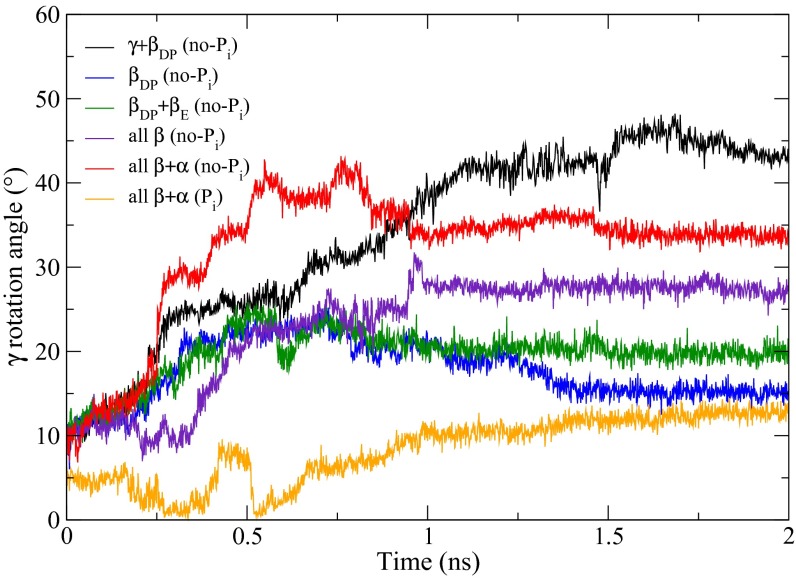
An essential element for understanding F₁-ATPase function is knowledge of the mechanism by which the torque for γ-rotation is generated. The dominant factor in the 80° rotation from the waiting dwell is known to be ATP binding to a β_E subunit and its closure to form β_TP (12, 29), in which repulsive van der Waals interactions contribute dominantly in the generation of torque. On the other hand, our understanding of the 40° rotation is much more limited (20, 30). To explore the mechanism, we carried out targeted molecular dynamics (TMD) (31) simulations with the model generated in this paper for the ATP waiting structure as the target (see Methods). As a reference, a TMD simulation was performed, starting with the catalytic dwell structure, and a perturbation was applied to the γ- and β_DP subunits of the entire α₃β₃γ-complex to induce the 40° rotation of γ and the transition of β_DP to β_HO required to reach the model ATP waiting structure. The simulation produces a structure with the γ-subunit rotated by 40° and β_DP partially open; the structure differs slightly from the model ATP waiting structure in the orientations of the C-terminal hth motifs of β_E and all of the α-subunits (SI Appendix, Fig. S6). The change of the β_E structure during or after the 40° rotation is in accord with the results of Watanabe et al. (30) that in the neighborhood of the ATP waiting state, the affinity for ATP changes with γ-rotation, implying a change in the β_E structure, and the all-atom explicit water MD simulation (SI Appendix, Fig. S2A). Because the TMD perturbation was applied only to γ and β_DP, the structural changes of the other parts of the α₃β₃γ-complex reflect their spontaneous response to the rotation of γ and the partial opening of β_DP. The resulting structure was used as the target structure in subsequent TMD simulations, where the perturbation was applied only to various parts of the α₃β₃ crown, with or without P_i in the β_E subunit (Fig. 5; see legend for details). With β_E empty, we obtained the striking result that transformations of all α- and β-subunits are required to induce the 40° rotation (Fig. 5). As shown in the figure, when fewer elements of the α₃β₃ crown are transformed (e.g., all β-subunits), only intermediate rotation of the γ-subunits is produced during the simulation. Moreover, with P_i present in β_E, the P_i stays bound in the active site throughout the entire TMD simulation and only a 10° rotation of γ was achieved even with the full α₃β₃ transformation in the simulation (Fig. 5, orange; and see Movies S2 and S3). These results show that the presence of P_i in the β_E subunit blocks the γ-rotation.

Fig. 5.

γ-Rotation angles from the TMD simulations (see text). The no-P_i systems are systems with empty β_E: γ + β_DP simulation (black), β_DP simulation (blue), β_DP + β_E simulation (green), all β simulation (purple), all β + α simulation (red). For the P_i system, which refers to the system with P_i bound in β_E: all β + α simulation (yellow). The simulation time is shown in nanoseconds, and the γ-rotation angle is defined as in Pu and Karplus (12). Except for the γ + β_DP simulation, the TMD simulations continued for 1 ns and were followed by 1-ns unperturbed simulations to relax the system; during the latter all these systems reached a plateau for the rotation angle of γ. In the γ + β_DP TMD simulation, it took 2 ns before the rmsd distance to the 240° rotated structure fell below 0.75 Å; this was followed by a 1-ns relaxation simulation as in the other cases.

Dynamic Lock by P_i

To determine the mechanism of the β_E (P_i) lock, the structure and dynamics of the α₃β₃γ-complex in the catalytic dwell state with different occupations of β_DP and β_E were studied by all-atom explicit water MD simulations (see SI Appendix, SI5 for details). In the simulations, the binding pockets of β_TP and all α-subunit are occupied by ATP, whereas β_DP and β_E have different occupations: In the prehydrolysis state simulation, ATP occupies β_DP and P_i occupies β_E; in the posthydrolysis state simulation, ADP and P_i occupy β_DP and P_i occupies β_E; and in the postrelease state simulation, β_DP is occupied by ADP and P_i and β_E is empty. (See Fig. 1B and its legend for identification of the three states.) The structural comparisons reveal that during or after the hydrolysis of ATP in β_DP and the release of P_i from β_E, small changes occur in the C-terminal hth motif and at the intersubunit interfaces of the subunits (SI Appendix, Figs. S2E and S7, and Movie S4). In addition, there are significant differences in the dynamics, as evidenced in the cross-correlation maps of the α₃β₃γ-complex; they are shown in SI Appendix, Fig. S8. Details of the structural and dynamic changes are given below.

SI Appendix, Fig. S8 shows the cross-correlation maps of the entire α₃β₃γ complex for the prehydrolysis state (SI Appendix, Fig. S8A), posthydrolysis state (SI Appendix, Fig. S8B), and the postrelease state (SI Appendix, Fig. S8C). In comparing the simulation of the posthydrolysis state to that of the prehydrolysis state, there is a rigid-body rotation of the C-terminal hth motif of α_DP toward β_DP in the former, relative to the latter. This rotation is caused by the cleavage of ATP into ADP and P_i in β_DP (SI Appendix, Fig. S7A). The rotation increases the contact between the two subunits and leads to a more closed α_DP–β_DP interface, as is evident from the increased buried surface area (SI Appendix, Fig. S9). It also leads to enhanced positive cross-correlation between the two subunits without a significant change of the intrasubunit cross-correlation of β_DP (compare SI Appendix, Fig. S8 A and B). A similar closure of the α_DP–β_DP interface is observed experimentally. In SI Appendix, Fig. S7B, the X-ray structure with the transition-state analog (26) is superimposed on the structure with the ATP analog (14). The superposition shows that the C-terminal domain of α_DP is rotated toward β_DP for the transition-mimic state to make the interface tighter (SI Appendix, Fig. S7B, Left), in agreement with the simulations. The origin of this structural change appears to involve the displacement of α_DPR373, which moves to interact with the P_i after it is cleaved from the ATP. SI Appendix, Fig. S7A shows the displacement of α_DPR373 upon the cleavage of ATP in the simulations and a similar displacement in the transition-state mimic structure (SI Appendix, Fig. S7B; also see SI Appendix, Fig. S7C for the changes of interactions at the interface between the two subunits). In this interpretation, α_DPR373 functions as a sensor that probes the progress of the hydrolysis reaction in β_DP and dynamically links the two subunits (β_DP and α_DP). This is consistent with mutation experiments, which suggested that α_DPR373 is involved in the rearrangement of the α_DP–β_DP interface upon ATP hydrolysis and the catalytic cooperativity of the enzyme (32).

We also find a noticeable difference between the cross-correlation maps of the posthydrolysis and postrelease states, i.e., there is an increase of intrasubunit correlation of both α_E and β_E in the postrelease state (SI Appendix, Fig. S8C). The cross-correlation maps of α_E and β_E in the postrelease state show cross-correlations that extend over the C-terminal and nucleotide binding domains (SI Appendix, Fig. S8 C and D), suggesting that the two domains behave like a rigid body. This difference in the dynamics of β_E is of interest because the differences between the β_E structures with or without P_i or a P_i analog are found to be negligible (SI Appendix, SI9 and Fig. S10). The anticorrelation between α_E and the C-terminal domain of β_E has also increased significantly (compare SI Appendix, Fig. S8 B and D). This result indicates that the two subunits move concertedly but in opposite directions. In this case, α_ER373 could play an important role in controlling the dynamics of α_E and β_E, similar to the role of α_DPR373 in ATP hydrolysis. In this mechanism, the interaction between P_i in β_E and α_ER373 keeps α_E close to β_E and away from β_DP, preventing α_E from responding to the change of β_DP, thus blocking the rotation of γ. Once P_i leaves the binding pocket, the interaction is lost, so that α_E and in particular its C-terminal domain are able to respond to the change occurring in β_DP and the rotation of γ.

To test the proposed mechanism, we have performed an additional TMD simulation. The simulation was carried out with the TMD perturbation applied to all α- and β-subunits but without the interaction between P_i in β_E and α_ER373. If these interactions were important in blocking the γ-rotation, it would be expected that γ would rotate further in their absence than when the interactions between P_i in β_E and α_ER373 were present. The simulation produced a γ-rotation that is larger (close to 20°) than the simulation with the P_i−α_ER373 interaction present, but then it falls back to the lower rotation angle during the subsequent relaxation simulation (SI Appendix, Fig. S11). The result confirms the proposed role of the interaction between P_i and α_ER373 in blocking γ-rotation. The result also suggests that interactions (within or between β_E and α_E), other than the interactions between P_i in β_E and α_ER373, are important in preventing the rotation—for example, reducing the increase of the intrasubunit cross-correlation in the β_E and α_E subunits and the increase of the anticorrelation between them, which occurs upon the release of P_i. In this regard, we note that α_E is the subunit forming the most extensive surface contacts with γ among the α-subunits and has an extensive surface contact with β_DP (SI Appendix, Fig. S9). The surface contacts of α_E with γ are as extensive as the contact between β_DP and γ, which is the largest surface contact among all βs.

Taken together, the present analysis shows how the interactions between β_E and α_E, including the interaction between P_i and α_ER373, act as a “dynamic lock” to keep the protein in the prerotated catalytic dwell state. Only after P_i in β_E is released is α_E freed from β_E and able to fully engage with β_DP to complete the concerted conformational transition of the α₃β₃ complex by which the γ-subunit rotates to reach the ATP waiting dwell state. Such dynamic locks have been proposed for different systems by Laity et al. for zinc finger proteins (33) and by Young et al. for c-Src (34).

Concluding Remark

The present study provides a structural model for the ATP waiting state of F₁-ATPase, in agreement with single-molecule experiments which have suggested that it does not coincide with any of the known crystal structures. Knowledge of this structure, combined with that of the state in which catalysis takes place, makes possible the development of a detailed atomic-level description of the coupling between the binding and hydrolysis of ATP and the γ-subunit rotation induced by the conformational changes of the α- and β-subunits. The suggested tests of the proposal structure and a possible method for trapping it in a crystallographically accessible conformation should stimulate experimental studies (see SI Appendix, SI10 and SI11 for details).

Methods

Forced Rotation Simulation for Finding the ATP Waiting State.

The structure of the minimal rotary complex α₃β₃γ was prepared based on the α₃β₃ subcomplex of the 1BMF structure (3) and the γ-subunit of the 1E79 structure (9) by a procedure similar to that of Ma et al. (35). The CHARMM19 all-atom force field (36) and the EEF1 implicit solvation model (37) were used to describe the protein system and water solvation, respectively. In addition, the coarse-grained PNM (11, 12), in which each PNM node was assigned to the corresponding C_α atom position of the protein, was used to stabilize the protein conformation in the presence of the high forces used in the simulation. The system was first heated from 0 K to 300 K in 60 ps and then equilibrated at 300 K for 300 ps (see details in SI Appendix, SI2). The MD simulations were carried out with a 2-fs integration time step and SHAKE (38) applied to the bonds involving hydrogen atoms. The temperature was controlled using the Langevin thermostat.

After equilibration at 300 K for 300 ps, a large external torque was applied to drive the rotation of the γ-subunit in the hydrolysis direction (counterclockwise as seen from the membrane). Using the PULL command of the CHARMM program (10), an external force of 2,500 pN was applied to the C_α atom of residue γM25. Residue γM25 was identified by Pu and Karplus to provide a key contact point for the torque generation (12). In the forced rotation simulation, the external torque was applied only when backward rotation is detected. In that way, the simulation was biased toward the hydrolysis direction only when γ rotates backward but not when the forward rotation occurs spontaneously; the γ-rotation angle was checked at each update step (at every 1 ps). The γ-rotation angle is defined as in Pu and Karplus (12) using the α₃β₃(1BMF)–γ(1E79) Walker structure as the reference structure for the catalytic dwell state, and a similar definition was used in the work by Koga and Takada (39). The direction of the force was determined as the instantaneous cross-product between the radial vector of the residue γM25 (perpendicular to the rotational axis) and the rotational axis itself (Fig. 1A). See SI Appendix, SI2 for details of forced rotation simulations and definition of the γ-rotation angle.

P_i Release Simulations.

The 200° rotated system was prepared using the α₃β₃ subcomplex of the 1E1R structure (26) and the γ-subunit from the 1E79 structure (9). For the 240° state, the starting structure was the present ATP waiting state model structure. For the P_i release MCES simulation (25), the P_i molecule was replicated 30 times by using the BLOCK module of the CHARMM program. In all simulations, P_i was treated as doubly protonated (H₂PO₄⁻), which was found to be favored in the active site of β-subunit (40). The interaction between the multiply copied P_i and both the protein and the solvent was scaled by a factor that is inversely proportional to the number of P_i copies, whereas each P_i has no interaction with other P_i molecules. The remaining interactions were not scaled. The temperature of P_i was controlled by attaching each P_i to a separate Langevin thermostat, while the remainder of the system was maintained at 300 K. At each P_i temperature, the MCES simulation was repeated 40 times (SI Appendix, SI7). Each simulation was started with different initial velocities and ran for 2 ns with a 1-fs integration time step. SHAKE was applied to constrain bonds involving hydrogen atoms.

Targeted MD Simulations of the Coordinated Conformational Transition of the α₃β₃γ-Complex.

The 40° substep rotation was simulated by applying the TMD simulation method (31). The TMD simulation was first carried out with the 200° rotated catalytic dwell structure, which was prepared for the MCES P_i release simulations. The TMD perturbation was applied to the nonhydrogen atoms of the γ- and β_DP subunits of the entire α₃β₃γ-complex for the β_DP → β_HO transition and the 40° γ rotation; the ATP waiting model structure was the target structure. Subsequently, using this TMD-produced structure as the target structure for the α₃β₃ complex, a set of TMD simulations was carried out with the TMD perturbation applied to various parts of the α₃β₃ crown with or without P_i in β_E and without any perturbation to γ (see Fig. 5 legend for the notation of each TMD simulation). In the simulations the rmsd distance to the target structure was decreased by 0.2 × 10⁻⁶ Å at each MD step until the rmsd distance reached a value lower than 0.75 Å.