Variation potential influence on photosynthetic cyclic electron flow in pea

Abstract

Cyclic electron flow is an important component of the total photosynthetic electron flow and participates in adaptation to the action of stressors. Local leaf stimulation induces electrical signals, including variation potential (VP), which inactivate photosynthesis; however, their influence on cyclic electron flow has not been investigated. The aim of this study was to investigate VP's influence on cyclic electron flow in pea (Pisum sativum L.). VP was induced in pea seedling leaves by local heating and measured in an adjacent, undamaged leaf by extracellular electrodes. CO₂ assimilation was measured using a portable gas exchange measuring system. Photosystem I and II parameters were investigated using a measuring system for simultaneous assessment of P700 oxidation and chlorophyll fluorescence. Heating-induced VP reduced CO₂ assimilation and electron flow through photosystem II. In response, cyclic electron flow rapidly decreased and subsequently slowly increased. Slow increases in cyclic flow were caused by decreased electron flow through photosystem II, which was mainly connected with VP-induced photosynthetic dark stage inactivation. However, direct influence by VP on photosystem I also participated in activation of cyclic electron flow. Thus, VP, induced by local leaf-heating, activated cyclic electron flow in undamaged leaves. This response was similar to photosynthetic changes observed under the direct action of stressors. Possible mechanisms of VP's influence on cyclic flow were discussed.

Introduction

Photosynthesis in plants is based on three light-driven electron flows, namely noncyclic, pseudocyclic, and cyclic flows (Allen, 2003). Cyclic electron flow is connected with photosystem I (PSI) and cytochrome b₆f (Joliot and Joliot, 2006; Joliot and Johnson, 2011; Roach and Krieger-Liszkay, 2014), whereas other flows are also connected with photosystem II (PSII) (Allen, 2003; Roach and Krieger-Liszkay, 2014). In contrast to noncyclic and pseudocyclic electron flows, cyclic flow only yields ATP synthesis and does not generate NADPH for the Calvin cycle or reactive oxygen species (Allen, 2003).

Cyclic electron flow can be activated by different stressors and might serve as an adaptive mechanism (Bukhov et al., 1999; Rumeau et al., 2007; Zhang and Sharkey, 2009; Zivcak et al., 2013). In particular, under stress conditions, cyclic flow might regulate photosynthetic generation of reactive oxygen species (Zhang and Sharkey, 2009; Roach and Krieger-Liszkay, 2014), contribute to oxidation of the PSI acceptor side, thereby protecting it from damage (Rumeau et al., 2007; Roach and Krieger-Liszkay, 2014), and support the transthylakoid proton gradient (Bukhov et al., 1999; Joliot and Joliot, 2006; Zhang and Sharkey, 2009). In turn, support of the pH gradient contributes to ATP synthesis, fluorescence non-photochemical quenching (NPQ), and thylakoid membrane stability (Zhang and Sharkey, 2009; Joliot and Johnson, 2011). Thus, it can be concluded (Roach and Krieger-Liszkay, 2014) that activation of cyclic electron flow by the direct action of stressors plays an important role in adaptive responses in plants.

Local action by stressors induces electrical signals, namely action potential (AP) induced by non-damaging stimuli and variation potential (VP) caused by damaging stimuli, which propagate through the unstimulated parts of higher plants (Volkov, 2000; Dziubinska, 2003; Brenner et al., 2006; Mancuso and Mugnai, 2006; Stahlberg et al., 2006; Trebacz et al., 2006). Self-propagating AP is mainly caused by fluxes of Ca²⁺, K⁺, and Cl⁻ ions (Felle and Zimmermann, 2007), while transient H⁺-ATPase inactivation and proton influx participate in its generation to a lesser degree (Sukhov and Vodeneev, 2009). VP generation is connected with transient plasmalemma H⁺-ATPase inactivation (Stahlberg et al., 2006); however, fluxes of Ca²⁺, K⁺, and Cl⁻ ions might also participate in VP development (Vodeneev et al., 2011; Sukhov et al., 2013b; Katicheva et al., 2014). VP propagation is probably connected with transmission of hydraulic and/or chemical signals (Stahlberg et al., 2006; Trebacz et al., 2006; Vodeneev et al., 2012), which induce an electrical reaction.

Electrical signals can induce numerous functional responses (Dziubinska, 2003; Davies and Stankovic, 2006; Stahlberg et al., 2006; Volkov et al., 2008; Fromm and Lautner, 2012). In particular, they inactivate photosynthesis in unstimulated leaves (Koziolek et al., 2004; Krupenina and Bulychev, 2007; Grams et al., 2009; Pavlovič et al., 2011; Sukhov et al., 2012, 2013a, 2014a,b; Bulychev and Komarova, 2014). The first stage of photosynthetic response development is possibly ion influx. Investigations of Bulychev and coworkers (Krupenina and Bulychev, 2007) have shown that Ca²⁺ influx is a potential mechanism for AP influence on photosynthesis in Chara alga. In regard to VP, according to studies by Grams et al. (2009) and our previous investigations (Sukhov et al., 2013a, 2014a), plasmalemma H⁺-ATPase inactivation and subsequent proton influx are main mechanisms for VP influence on photosynthesis in higher plants.

Independent of mechanisms for initial photosynthetic-response induction, subsequent development of this response is mainly connected with inactivation of the photosynthetic dark stage (Krupenina and Bulychev, 2007; Pavlovič et al., 2011; Pavlovič, 2012; Sukhov et al., 2012, 2014a,b), which decreases quantum yields of photosystem I and II and increases non-photochemical fluorescence quenching. Influence of Ca²⁺ influx on photosynthetic dark stage can be connected (Krupenina and Bulychev, 2007) with the dependence of Calvin cycle enzymes on calcium concentrations in chloroplast stroma (Wolosiuk et al., 1993). Proton influx into cytoplasm and stroma can influence CO₂ transport, changing carbonic anhydrase (Grams et al., 2009) and/or aquaporin (Gallé et al., 2013) activities and modifying the CO₂/HCO⁻₃ ratio (Bulychev et al., 2001). This influx might also reduce Calvin cycle activity (Wolosiuk et al., 1993). However, the direct influence of AP and VP on the light stage is also possible (Pavlovič et al., 2011; Sukhov et al., 2012, 2014a,b). The influence can be related to the rise of fluorescence non-photochemical quenching (Sukhov et al., 2014a) and reduced electron flow through the acceptor side of PSI (Sukhov et al., 2012), which might be caused by acidification of the stroma and lumen (Müller et al., 2001; Alte et al., 2010; Benz et al., 2010).

According to Retivin et al. (1997), rapid and transient increases in plant resistance to stressors (10–25 min after stimulation) are the final result of electrical signal-induced functional responses. The resistance increase in unstimulated parts of plant contributes to plant survival under systemic action of stressor which may follow after electrical signal induction (Retivin et al., 1997). Decrease of photosynthetic machinery damage can be a mechanism contributing to the influence of electrical signals on plant resistance to stressors (Sukhov et al., 2014b). AP (Retivin et al., 1999) and VP (Sukhov et al., 2014b) increase the resistance of photosynthetic machinery to the effects of temperature changes. Our previous results (Sukhov et al., 2014b) have shown that increased resistance of photosynthetic machinery to heat is caused by VP-induced inactivation of the photosynthetic dark stage. Changes in cyclic electron flow can link the VP-induced dark stage inactivation and photosynthetic machinery resistance increase (Sukhov et al., 2014b). However, experimental investigations of electrical signal influence on cyclic electron flow are lacking. Thus, the aim of the present study was to investigate VP influence on cyclic electron flow in pea (Pisum sativum L.).

Materials and methods

Plant material

Seedlings of pea (Pisum sativum L.) were cultivated hydroponically in a Binder KBW 240-plant growth chamber (Binder GmbH, Tuttlingen, Germany) at 24°C under a 16/8 h (light/dark) photoperiod. Seedlings used in experiments were 14–21 days old.

Stimulation and electrical measurements

VP was induced by heating ~1 cm² of a leaf tip (a stimulated leaf) over a flame for 3–4 s, representing a standard damaging stimulus (Koziolek et al., 2004; Vodeneev et al., 2012; Sukhov et al., 2014a,b).

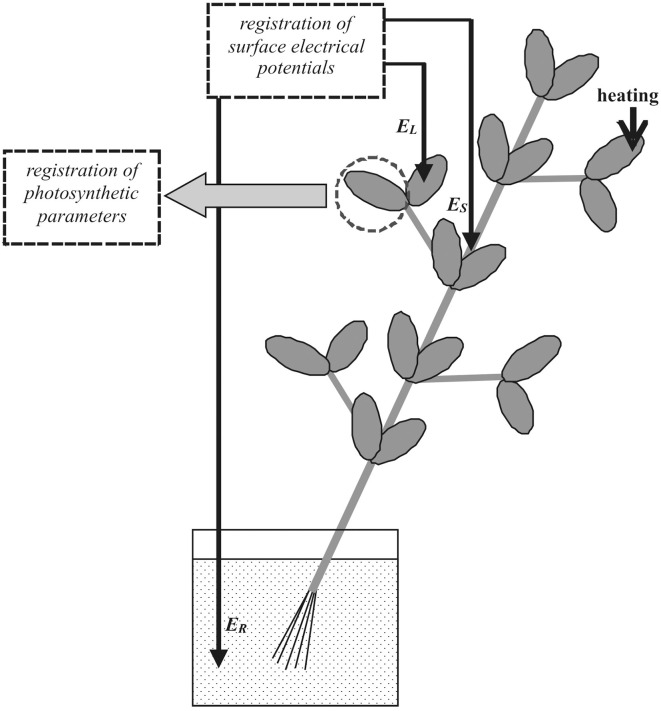
The surface electrical potential was measured using Ag⁺/AgCl electrodes (Gomel Plant of Measuring Equipment, Gomel, Belarus), a high-impedance amplifier IPL-113 (Semico, Novosibirsk, Russia) and a PC. Measurement electrodes contacted an unstimulated leaf via “Uniagel” conductive gel (Geltek-Medica, Moscow, Russia), according to our previous studies (Sukhov et al., 2014a,b). Electrical activity was monitored by two electrodes (Figure 1), with the first (E_S) placed on a stem and the second (E_L) connected with a leaflet center of an unstimulated leaf. The distance between the E_S site and the damaged area was 6–7 cm and the distance between E_S and E_L 3–5 cm. It should be noted that, as electrical responses in conjugate leaflets of a leaf were very similar in pea (Sukhov et al., 2014a,b), the electrical reaction, registered by E_L, was used for investigation of VP parameters in conjugate leaflets in which photosynthesis was measured. The E_R was placed in a standard solution surrounding the root.

Figure 1.

Positions of stimulation, electrical potential, and photosynthetic parameter measurements in pea plants. E_L, electrode connected to leaf; E_S, electrode connected to stem; and E_R, reference electrode; distance between E_L and E_S, 3–5 cm; and heating of leaf tip (arrow) used as external stimulus.

Measurements of photosynthetic parameters

Photosynthetic parameters in intact pea leaves were measured by a system composed of a GFS-3000 portable gas exchange measuring system, a Dual-PAM-100 measuring system for simultaneous assessment of P700 oxidation and chlorophyll fluorescence, and a measuring head Dual-PAM gas exchange Cuvette 3010-Dual (Heinz Walz GmbH, Effeltrich, Germany). The system was employed for simultaneous recording of photosynthetic dark and light stage parameters in unstimulated leaf lamina (measured area, 1.3 cm²).

The initial parameters of PSII fluorescence, the dark and maximal fluorescence yields (F₀ and F_m, respectively), were measured after dark adaptation for 20 min. The maximal change in the P700 signal (P_m) of PSI, reflecting maximal P700 oxidation, was measured after preliminary illumination by far red light for 10 s. The steady-state fluorescence yields in light (F and F′_m, respectively), and steady-state and maximal signals in light (P and P′_m, respectively) were measured using saturation pulses generated every 10 s. Quantum yields of PSI (ϕ_PSI), non-photochemical energy dissipation in PSI because of donor side limitation (ϕ_ND), and non-photochemical energy dissipation in PSI connected with acceptor-side limitation (ϕ_NA) were calculated using the equations ϕ_PSI = (P′_m − P)/P_m, ϕ_ND = P/P_m, and ϕ_NA = (P_m − P′_m)/P_m (Klughammer and Schreiber, 2008). The effective quantum yield of PSII (ϕ_PSII) and fluorescence non-photochemical quenching (NPQ) were calculated using the equations ϕ_PSII = (F′_m − F) / F′_m and NPQ = (F_m − F′_m) / F′_m (Maxwell and Johnson, 2000). The CO₂ assimilation rate (A, μmol CO₂·m⁻²·s⁻¹) was measured using the GFS-3000 system and its software, and the parameter programmatically calculated according to Von Caemmerer and Farquhar (1981).

The external CO₂ concentration ([CO₂]) was 360 ppm in the control and ~10–15 ppm under low [CO₂] conditions. In some series of experiments, CO₂ concentration was decreased from 360 ppm to ~150 or ~10–15 ppm. Relative air humidity and leaf temperature were ~60% and ~23°C, respectively. Blue actinic light (460 nm) intensity in the control was 239 μmol·m⁻²·s⁻¹. In a separate experimental series, far red light (240 μmol·m⁻²·s⁻¹, 730 nm) was used as actinic light.

VP was induced in plants ~1 h after initiation of actinic light, and photosynthetic responses monitored for 30 min.

Calculations of electron flows

Electron flows through PSI [EF(PSI)] and PSII [EF(PSII)] were calculated using Equations (1) and (2) (Miyake et al., 2004, 2005; Huang et al., 2012; Zivcak et al., 2013):

$$EF(PSI)={\alpha }_I\times {\varphi }_{PSI}\times PFD,$$ (1)

$$EF(PSII)={\alpha }_{II}\times {\varphi }_{PSII}\times PFD,$$ (2)

where PFD was the photosynthetically-active photon flux density of light illuminating a leaf, α_I = p × (1 − dII) and α_II = p × dII the fractions of photon flux distributed to PSI and PSII, dII the fraction of absorbed light distributed to PSII, and p the fraction of PFD absorbed by leaves.

The electron flow through PSI included noncyclic, pseudocyclic, and cyclic flows, whereas the electron flow through PSII included only noncyclic and pseudocyclic flows (Allen, 2003). Thus, cyclic electron flow [EF(C)] is described as Equation (3) (Miyake et al., 2004, 2005; Huang et al., 2012; Zivcak et al., 2013):

$$EF(C)=EF(PSI)-EF(PSII).$$ (3)

Calculation of EF(C) required values for p and dII [Equations (1)–(3)]. The value of p was measured according to Berger et al. (2004), using a standard procedure in IMAGING-PAM M-Series MINI Version (Heinz Walz GmbH) and found to be 0.88 ± 0.01 (n = 10).

According to a number of studies (Miyake and Yokota, 2000; Makino et al., 2002; Miyake et al., 2004, 2005), the fraction of absorbed light distributed to PSII was calculated on the basis of the Farquhar, Von Caemmerer and Berry photosynthetic model of Von Caemmerer et al. (2009). According to this model, CO₂ assimilation (A) under electron transport limited conditions is described by the Equation (4):

$$A=\frac{\left(C_c-{\Gamma }^{\ast }\right)}{4\left(C_c+2{\Gamma }^{\ast }\right)}\times EF(PSII)-R_d,$$ (4)

where Γ^* is the photosynthetic CO₂ compensation point in the absence of mitochondrial respiration (36.9–39.6 ppm), R_d the respiration rate in darkness, and C_c the mole fraction of CO₂ in chloroplasts. Under high [CO₂] (C_c → ∞) Equation (4) transforms to Equation (5):

$$EF(PSII)=4\left(A+R_d\right),$$ (5)

Combining Equations (1) and (3) yields:

$$dII=\frac{4\left(A+R_d\right)}{p\times {\varphi }_{PSII}\times PFD},$$ (6)

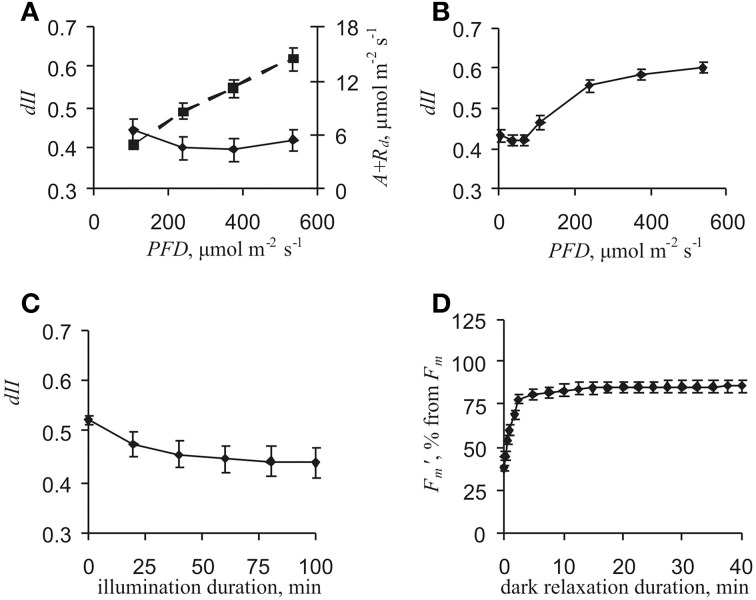
Equation (6) was in good accordance with the works of Miyake et al. (2004); Miyake et al. (2005). Figure 2A shows dII calculated under different PFDs and an external [CO₂] of 2000 ppm. This condition was electron transport limited because A + R_d depended on PFD in a linear manner. The value of dII varied from 0.40 to 0.44 and was not significantly dependent on light intensity (p > 0.05).

Figure 2.

Dependencies of photosynthetic parameters on light intensity and duration of illumination by actinic light and darkness. (A) dependencies of dII and A + R_d on light intensity after 1 h control actinic light. Values for dII calculated using Equation (4) under external CO₂ concentration of 2000 ppm (n = 7). (B) dependence of dII on light intensity after 1 h control actinic light. Value of dII calculated using equation (7) (n = 6). (C) dependence of dII on duration of control actinic light illumination. Value of dII calculated using Equation (7); actinic light intensity, 47 μmol·m⁻²·s⁻¹ (n = 5). (D) dependence of F′_m on duration of darkness after actinic light illumination for 1 h.

For the purpose of additional dII control, an alternative method for measuring dII was used (Huang et al., 2012) that was simpler than the previous method. It is known that plants have slight cyclic electron flow under low light intensity and that flow magnitude increases with increasing PFD (Miyake et al., 2005; Joliot and Joliot, 2006; Huang et al., 2011; Zivcak et al., 2013). Therefore, EF(PSI) approximately equals EF(PSII) under low light condition (Huang et al., 2012). Taking into account that EF(PSI) = EF(PSII) and using Equations (1) and (2), Equation (7) was deduced:

$$dII=\frac{1}{{\varphi }_{PSII}/{\varphi }_{PSI}+1}.$$ (7)

Figure 2B shows that dII equaled ~0.42 under low light conditions (PFD ≤ 65 μmol·m⁻²·s⁻¹). Increases in dII were observed under light intensity equaling 108 μmol·m⁻²·s⁻¹ and greater. This increase probably reflected increased cyclic electron flow, i.e., EF(PSI) ≠ EF(PSII) under moderate and high light conditions. Thus, Equation (7) could also be used for dII calculation under low actinic light (≤65 μmol·m⁻²·s⁻¹).

Values for dII calculated after a 1 h illumination by control actinic light (239 μmol·m⁻²·s⁻¹) were in accordance with the time of VP induction. However, dII might have depended on the duration of actinic light illumination, which could have influenced results. Values of dII decreased from about 0.51–0.53 to ~0.41–0.46 with increased light duration (t_1/2 = 20 min, Figure 2C), but it was essentially unchanged from the 60th to 100th min; i.e., dII was constant in the range of photosynthetic response investigated here.

Initial dII changes could have been connected with a state-transition and/or PSII damage. State-transition relaxation duration is from minutes to tens of minutes and damage relaxation time in hours (Maxwell and Johnson, 2000; Müller et al., 2001). As a result, state-transition might have been altered under VP rather than with PSII damage. Analysis of F′_m relaxation kinetics in darkness after 1 h of actinic light illumination showed that there were insignificant changes in this parameter from the 5th to 40th min (Figure 2D); i.e., there was no essential state-transition under these experimental conditions. In addition, this result supported the observed dII stability in the time range of VP-induced photosynthetic responses. Thus, taking into account these results, a dII value of 0.42 was used in the present work.

Far red light, which is absorbed predominantly by PSI, was used as actinic light in an individual series of experiments. In this case, EF(PSII) was also described by Equation (1); however, far red light absorption by PSII, which is low (Joliot and Johnson, 2011), was assumed equal to zero and PFD to be small (5 μmol·m⁻²·s⁻¹, measuring light). Here, EF(PSI) was described by Equation (8):

$$EF(PSI)=p\times \left(1-dII\right)\times {\varphi }_{PSI}\times \left(PFD+FRFD\times \delta \right),$$ (8)

where FRFD was the far red light flux density, δ the ratio of far red light to actinic light absorption by leaf (730 and 460 nm, respectively), PFD = 5 μmol·m⁻²·s⁻¹, and δ has been calculated from green leaf absorption spectra (Hogewoning et al., 2012) and equaled ~0.12.

Relative EF(C) was also used in analyses, as the percentage of cyclic electron flow in the total flow. Relative EF(C) was calculated using Equation (9):

$$relativeEF(C)=\frac{EF(C)}{EF(C)+EF(PSII)}\times 100\%.$$ (9)

Statistics

Each series of experiments comprised 5–17 measurements, with each measurement performed on a separate plant. Representative records, obtained for individual measurements, mean values, standard errors, and correlation coefficients, are presented in the accompanying figures and tables. Significant differences in experiments were indicated according to the paired Student's t-test.

Results

Photosynthetic responses induced by variation potential

Local heating of a leaf induced VP propagation through the stem (Figure 3A). The signal amplitude was 40–90 mV, the duration wide-ranging (from 5 to 60 min), and the profile varied. In the most experiments (~80%), VP propagated into leaf lamina with an amplitude of 20–75 mV, with propagation velocities between steam and leaf at 0.02–0.20 cm·s⁻¹. In some experiments (~20%), only electrical reactions with small amplitude (<15 mV) were observed in lamina (Figure 3B).

Figure 3.

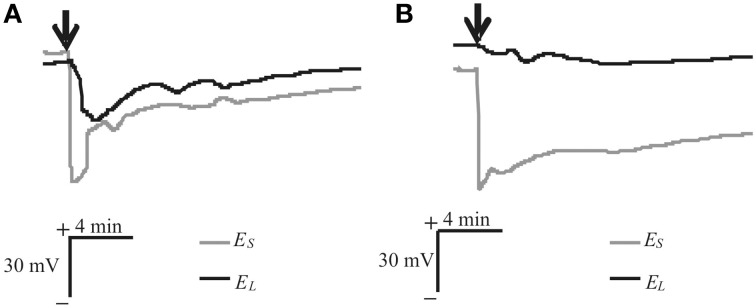
Heat-induced changes in surface electrical potentials of steam and leaf. (A) VP propagated into leaf (n = 17). (B) Only electrical reactions of small amplitude (<15 mV) propagated into leaf (n = 4). VP induced by heating tip of another leaf (arrow), and E_S and E_L, changes in electrical potential measured by electrodes on stem and lamina, respectively.

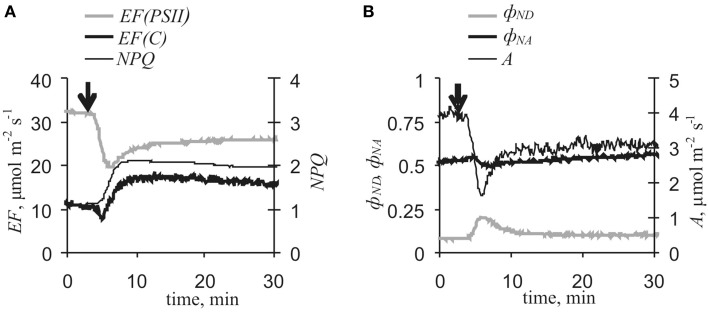
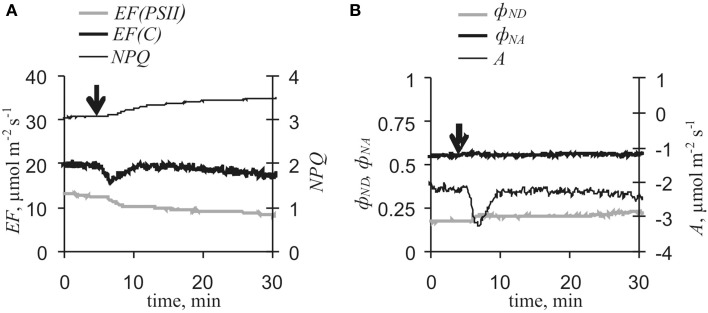
Figure 4 and Table 1 show that VP reduced CO₂ assimilation rates and electron flows through PSII, increased NPQ and ϕ_ND, and weakly decreased ϕ_NA. The response of EF(C) comprised two stages: fast inactivation of cyclic flow (EF(C)_min – EF(C)_initial) and a following slow activation (EF(C)_max - EF(C)_min). Extremes of EF(PSII) decrease, EF(C) fast inactivation, and EF(C) slow activation were observed at 2.8 ± 0.3, 1.0 ± 0.1 and 5.9 ± 0.3 min, respectively, after the start of photosynthetic responses. Fast inactivation and slow activation were probably independent of each other under control conditions because the correlation coefficient (r) between EF(C)_min - EF(C)_initial and EF(C)_max - EF(C)_min was −0.07 (p > 0.05). If only electrical reactions with small amplitudes (<15 mV) were observed in leaf lamina, a photosynthetic response was not developed.

Figure 4.

Variation potential-induced changes in photosynthetic light stage parameters and CO₂ assimilation under control conditions (n = 17). (A) EF(PSII), EF(C) and NPQ. (B) ϕ_ND, ϕ_NA, and A. Arrow, VP induction by local heating.

Table 1.

Photosynthetic parameters and changes induced by VP and [CO₂]-lowering.

	VP under [CO2] = 360 ppm	[CO2]- lowering to 10–15 ppm	[CO2]- lowering to 150 ppm	VP under [CO2] = 10–15 ppm
n	17	9	10	11
A decrease, μmol·m−2·s−1	−1.95 ± 0.19*	−4.08 ± 0.3*	−1.91 ± 0.20*	−1.03 ± 0.15*
EF(PSII)initial, μmol·m−2·s−1	30.1 ± 1.2	33.1 ± 2.2	34.3 ± 1.3	13.7 ± 1.4
EF(PSII)min, μmol·m−2·s−1	23.6 ± 1.1*	13.5 ± 1.6*	25.9 ± 1.1*	8.1 ± 1.0*
EF(PSII)min- EF(PSII)initial, μmol·m−2·s−1	−6.5 ± 0.7*	−19.7 ± 1.3*	−8.3 ± 0.9*	−5.5 ± 0.8*
EF(C)initial, μmol·m−2·s−1	11.1 ± 1.4	11.7 ± 2.3	11.3 ± 1.9	18.1 ± 1.2
EF(C)min, μmol·m−2·s−1	8.6 ± 1.2*	11.7 ± 2.3	11.1 ± 1.8	15.0 ± 1.2*
EF(C)max, μmol·m−2·s−1	14.4 ± 1.1*	20.0 ± 1.6*	15.3 ± 2.1*	18.6 ± 1.3
EF(C)min- EF(C)initial, μmol·m−2·s−1	−2.6 ± 0.3*	0	−0.2 ± 0.1	−3.1 ± 0.4*
EF(C)max- EF(C)min, μmol·m−2·s−1	5.8 ± 0.4*	8.3 ± 1.1*	4.2 ± 0.5*	3.6 ± 0.5*
NPQinitial	1.16 ± 0.05	1.25 ± 0.14	1.29 ± 0.09	2.76 ± 0.14
NPQmax	1.97 ± 0.08*	2.69 ± 0.17*	1.86 ± 0.14*	3.44 ± 0.16*
NPQmax- NPQinitial	0.81 ± 0.06*	1.34 ± 0.11*	0.57 ± 0.08*	0.68 ± 0.13*
ϕND initial	0.081 ± 0.009	0.071 ± 0.016	0.048 ± 0.006	0.202 ± 0.025
ϕND max	0.142 ± 0.011*	0.182 ± 0.014*	0.100 ± 0.012*	0.253 ± 0.021*
ϕND max- ϕND initial	0.061 ± 0.008*	0.111 ± 0.010*	0.035 ± 0.006*	0.051 ± 0.009*
ϕNA initial	0.584 ± 0.015	0.559 ± 0.027	0.563 ± 0.027	0.536 ± 0.028
ϕNA min	0.572 ± 0.014*	0.531 ± 0.025*	0.551 ± 0.028*	0.549 ± 0.026
ϕNA min- ϕNA initial	−0.012 ± 0.002*	−0.029 ± 0.007*	−0.012 ± 0.003*	0.013 ± 0.006

^*p < 0.05 compared with control, paired Student t-test.

Responses in EF(C) might have been connected with VP-induced changes in dII. The method for dII calculation by Huang et al. (2012) could not be used for plants that were stressed after local heating. However, dII changes must have modified F′_m under dark conditions. VP's influence on F′_m without actinic light was investigated here. It was shown that VP induced only small decrease of F′_m (3 ± 1%, n = 5), i.e., VP weakly influence dII.

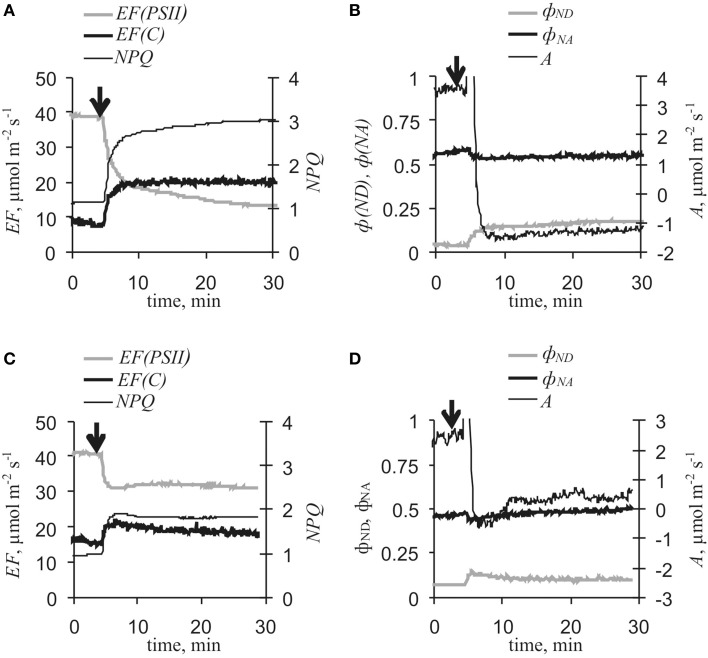
Inactivation of the photosynthetic dark stage is an initial process of VP-induced photosynthetic responses in pea (Sukhov et al., 2014a,b) and geranium (Sukhov et al., 2012). The present results showed that VP-induced A and EF(PSII) decreases were strongly correlated (r = 0.78, p < 0.001). Artificial reduction of dark stage activity through lowering of external [CO₂] decreased EF(PSII) and increased EF(C), ϕ_ND and NPQ (Figure 5), but fast inactivation of cyclic flow was absent. Moreover, VP-induced decreases in A and EF(PSII), increases in ϕ_ND and NPQ, and slow activation of EF(C) were collectively smaller under low [CO₂] conditions (10–15 ppm) than under control conditions (Figure 6, Table 1). In particular, maximum cyclic flow after VP was not distinguishable from the flow before electrical signal propagation under these conditions. However, fast inactivation of cyclic flow induced by VP under low [CO₂] was not significantly different from the controls. It should be noted that the correlation coefficient between EF(C)_min - EF(C)_initial and EF(C)_max - EF(C)_min was −0.81 (p < 0.01) under low [CO₂].

Figure 5.

[CO₂]-lowering-induced changes in photosynthetic light stage parameters and CO₂ assimilation (n = 9–10). (A) EF(PSII), EF(C) and NPQ under [CO₂]-lowering to 10–15 ppm. (B) ϕ_ND, ϕ_NA, and A under [CO₂]-lowering to 10–15 ppm. (C) EF(PSII), EF(C) and NPQ under [CO₂]-lowering to 150 ppm. (D) ϕ_ND, ϕ_NA, and A under [CO₂]-lowering to 150 ppm. Initial [CO₂] was 360 ppm. Arrow, start of [CO₂]-lowering.

Figure 6.

Variation potential-induced changes in photosynthetic light stage parameters and CO₂ assimilation under low [CO₂] conditions (n = 11). (A) EF(PSII), EF(C) and NPQ. (B) ϕ_ND, ϕ_NA, and A. [CO₂] was 10–15 ppm. Arrow, VP induction by local heating.

Correlation analysis of the mechanism of VP-induced cyclic electron flow changes

Changes in EF(PSII) and EF(C) might be different stages of united VP-induced photosynthetic response. This hypothesis was tested by analysis that revealed correlations between photosynthetic parameter changes (Table 2). There were strong connections between VP or [CO₂]-lowering-induced reduction of electron flow through PSII and slow activation of cyclic electron flow under control initial conditions. Correlation between decreases in EF(PSII) and fast inactivation of EF(C) was insignificant. Under low [CO₂], VP-induced responses of EF(PSII) and EF(C) were weakly connected with each other.

Table 2.

Correlation coefficients between changes in photosynthetic parameters induced by VP and [CO₂]-lowering.

Parameters	VP under [CO2] = 360 ppm	[CO2]- lowering to 10–15 or 150 ppm	VP under [CO2] = 10–15 ppm
n	17	19	11
EF(PSII)min- EF(PSII)initial and EF(C)min - EF(C)initial	0.11	−	−0.15
EF(PSII)min- EF(PSII)initial and EF(C)max- EF(C)min	−0.89*	−0.85*	−0.04
EF(PSII)min- EF(PSII)initial and ϕND max - ϕND initial	−0.90*	−0.90*	−0.73
ϕND max - ϕND initial and EF(C)max- EF(C)min	0.80*	0.77*	−0.20

^*Correlation coefficient is significant (p < 0.05), Student t-test.

The connection between EF(PSII) decrease and slow EF(C) activation might have been caused by changes in ϕ_ND. Really, correlation analysis showed that electron flow reductions through PSII induced by VP or [CO₂]-lowering was strongly correlated with increases in ϕ_ND (Table 2). Conversely, ϕ_ND increases were correlated with slow cyclic electron flow activation under control initial conditions, but the correlation coefficient was insignificant for VP-induced responses under initial low [CO₂].

VP-induced cyclic electron flow changes under far red light

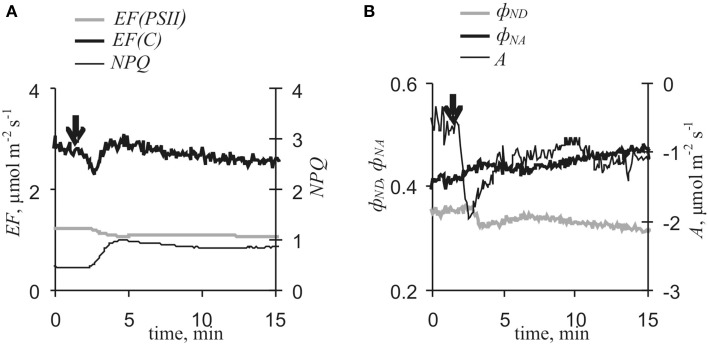
Far red light selectively activates PSI and is widely used for cyclic electron flow investigations (Joliot and Johnson, 2011). Here, far red light conditions were used for more detailed investigation of VP's influence on cyclic electron flow, and Figure 7 and Table 3 show VP-induced photosynthetic responses under far red light. VP decreased A, EF(PSII), and ϕ_ND and increased ϕ_NA and NPQ. VP-induced changes in EF(C) included two stages as described above, inactivation and subsequent activation. Both activation and inactivation were weakly connected with decreased electron flow through PSII. Correlation between EF(PSII) and ϕ_ND decreases was also insignificant. Conversely, slow EF(C) activation was strongly correlated with decreased ϕ_ND. Connections of changes in ϕ_NA with decreases in EF(PSII) and increases in EF(C) were insignificant (data not shown). It should be noted that there was a tenuous connection between EF(C)_min - EF(C)_initial and EF(C)_max - EF(C)_min under far red light conditions (r = −0.65, p = 0.06).

Figure 7.

Variation potential-induced changes in photosynthetic light stage parameters and CO₂ assimilation under far red light conditions (n = 9). (A) EF(PSII), EF(C) and NPQ. (B) ϕ_ND, ϕ_NA, and A. Arrow, VP induction by local heating.

Table 3.

Photosynthetic parameters and changes induced by VP and correlation coefficients under far red light conditions.

PARAMETERS AND THEIR CHANGES
A decrease, μmol·m−2·s−1	−1.08 ± 0.10*	ϕND initial		0.337 ± 0.013
EF(PSII)initial, μmol·m−2·s−1	1.24 ± 0.02	ϕND min		0.295 ± 0.014*
EF(PSII)min, μmol·m−2·s−1	1.11 ± 0.03*	ϕND min - ϕND initial		−0.042 ± 0.005*
EF(PSII)min- EF(PSII)initial, μmol·m−2·s−1	−0.13 ± 0.02*	ϕNA initial		0.420 ± 0.012
EF(C)initial, μmol·m−2·s−1	2.98 ± 0.06	ϕNA max		0.447 ± 0.013*
EF(C)min, μmol·m−2·s−1	2.60 ± 0.07*	ϕNA max - ϕNA initial		0.027 ± 0.002*
EF(C)max, μmol·m−2·s−1	3.27 ± 0.10*	NPQinitial		0.52 ± 0.03
EF(C)min- EF(C)initial, μmol·m−2·s−1	−0.38 ± 0.04*	NPQmax		0.99 ± 0.11*
EF(C)max- EF(C)min, μmol·m−2·s−1	0.66 ± 0.08*	NPQmax- NPQinitial		0.47 ± 0.08*
CORRELATION COEFFICIENTS
EF(PSII)min- EF(PSII)initial	0.35	EF(PSII)min- EF(PSII)initial		0.46
and		and
EF(C)min- EF(C)initial		ϕND max - ϕND initial
EF(PSII)min- EF(PSII)initial	−0.53	ϕND max - ϕND initial		−0.86#
and		and
EF(C)max- EF(C)min		EF(C)max- EF(C)min

^*p < 0.05 compared with control, paired Student t-test (n = 9).

^#Correlation coefficient is significant (p < 0.05), Student t-test.

VP- and [CO₂]-lowering-induced increases in relative cyclic electron flow

Examination of changes in relative cyclic electron flow induced by VP or [CO₂]-lowering showed that, with VP under control, low [CO₂], and far red light conditions as well as decreased [CO₂] alone induced increases in relative electron flow (Table 4). This effect was observed even if the absolute EF(C) showed no changes (photosynthetic response induced by VP under low [CO₂]).

Table 4.

Relative cyclic electron flow and changes induced by VP and [CO₂]-lowering.

	VP	[CO2]- lowering to 10–15 ppm	[CO2]- lowering to 150 ppm	VP under [CO2] = 10-15 ppm	VP under far red light
Relative EF(C)initial, %	26.6 ± 3.2	25.5 ± 4.9	23.5 ± 3.3	57.2 ± 3.8	70.7 ± 0.5
Relative EF(C)max, %	37.9 ± 2.7*	59.9 ± 3.5*	35.8 ± 3.7*	69.5 ± 3.0*	74.5 ± 0.9*
Change in relative EF(C), %	11.3 ± 1.3*	34.5 ± 3.0*	12.3 ± 1.0*	12.3 ± 1.6*	3.9 ± 0.6*

^*p < 0.05 compared with control, paired Student t-test (n = 9–17).

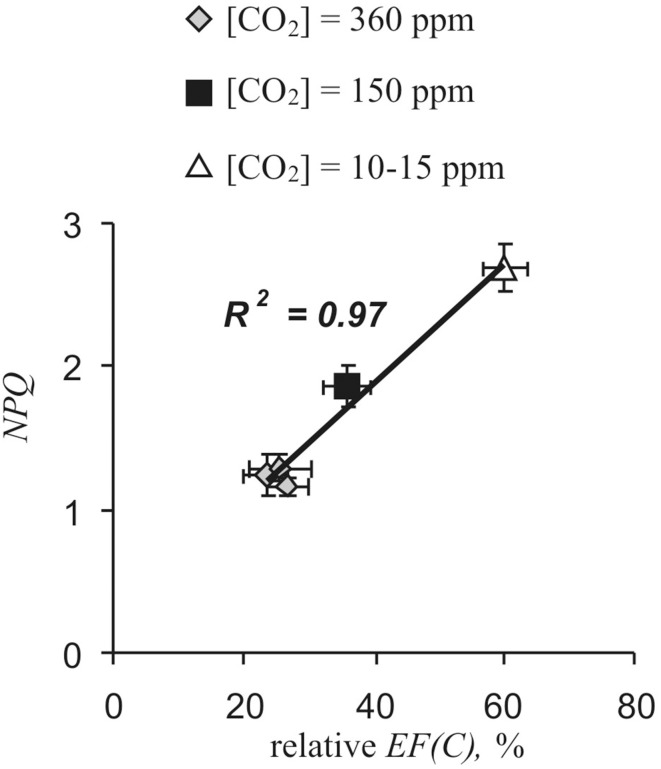
Stimulation of NPQ is mechanism of cyclic electron flow influences on plant resistance to stressors (Zhang and Sharkey, 2009; Joliot and Johnson, 2011). NPQ has been plotted against relative EF(C) under different CO₂ concentrations (Figure 8). EF(C) and NPQ were taken from the Tables 1, 4 (unheated plants). Figure 8 shows that EF(C) and NPQ were linearly connected.

Figure 8.

Fluorescence non-photochemical quenching (NPQ) was plotted against relative cyclic electron flow [relative EF(C)] under different CO₂ concentrations. Relative EF(C) and NPQ were taken from the Tables 1, 4 (unheated plants).

Connection between relative cyclic electron flow and fluorescence non-photochemical quenching were observed under control conditions too. Correlation coefficient between EF(C) and NPQ was 0.64 (n = 36. p < 0.001).

Discussion

Electrical signals can inactivate photosynthesis in plants (Koziolek et al., 2004; Krupenina and Bulychev, 2007; Grams et al., 2009; Pavlovič et al., 2011; Sukhov et al., 2012, 2013a, 2014a,b). In particular, VP reduces ϕ_PSI, ϕ_PSII, and A, and increases NPQ in pea (Sukhov et al., 2014a,b). Inactivation of the photosynthetic dark stage appears to be the initiator of photosynthetic responses induced by AP (Pavlovič et al., 2011) and VP (Sukhov et al., 2012, 2014a,b). However, direct influence of electrical signals on PSI (Sukhov et al., 2012) and PSII (Pavlovič et al., 2011; Sukhov et al., 2014a,b) is also observed.

Our results showed that VP, propagating into a leaf (Figure 3), induced changes in photosynthetic electron flows (Figure 4). VP-induced EF(PSII) decreases were in good accordance with data regarding ϕ_PSII decreases caused by electrical signals (see above). However, changes in EF(C), which included fast cyclic electron flow inactivation and its subsequent slow activation, were not previously shown and required detailed analysis.

Here, dII was shown to be stable in the range of photosynthetic response investigated (Figure 2C), and a state-transition was insignificant under these experimental conditions (Figure 2D). Also, VP weakly influenced F_m without actinic light and, therefore, slightly changed dII. These results revealed that the EF(C) response was not connected with dII changes.

Slow cyclic electron transport activation induced by VP and [CO₂]-lowering was observed to be connected with decreased EF(PSII) and that this connection was mediated by increased ϕ_ND (Table 2). Low activity of the photosynthetic dark stage and noncyclic electron flow are known to be accompanied by high EF(C) (Joliot and Joliot, 2006) as well as ϕ_ND value is positively correlated with cyclic flow magnitude (Munekage et al., 2002, 2004; Zivcak et al., 2013); however, the mechanisms of these connections remain unclear.

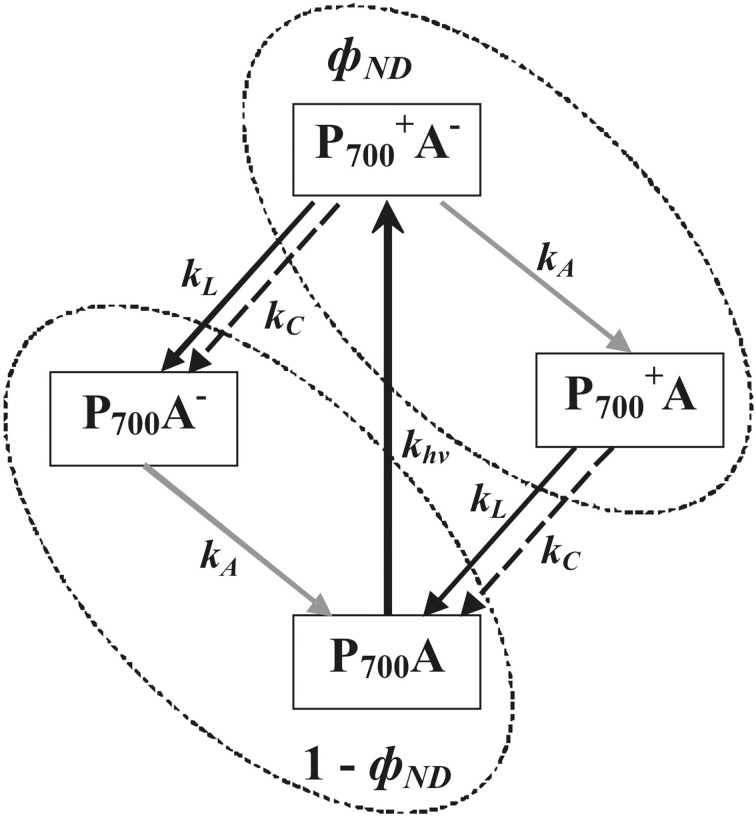
The simplest schema of PSI, based on Klughammer and Schreiber (1994) and Vredenberg and Bulychev (2010) and including P₇₀₀ oxidation and P⁺₇₀₀ reduction, was used here for analysis of photosynthetic response mechanisms (Figure 9). Using this schema, EF(PSII) and EF(C) were described by Equations (10) and (11):

$$EF(PSII)=k_L\times {\varphi }_{ND}$$ (10)

$$EF(C)=k_C\times {\varphi }_{ND}$$ (11)

Figure 9.

A simple schema of PSI states, based on Klughammer and Schreiber (1994) and Vredenberg and Bulychev (2010). P⁺₇₀₀ and P₇₀₀, oxidized and reduced forms of primary electron donor in PSI, respectively; A and A⁻, oxidized and reduced forms PSI acceptor, respectively; parameter k_hv = γ × PFD× p × (1 − dII), velocity constant of light-dependant P₇₀₀ oxidation, with γ, proportionality coefficient; parameters k_L = k_PC × [PC⁻ ]_L and k_C = k_PC × [PC⁻]_C, velocity constants of electron flow through PS II (mainly noncyclic) and cyclic electron flow, respectively; k_PC, velocity constant of plastocyanin oxidation by P⁺₇₀₀; [PC⁻]_L, concentration of reduced plastocyanin, participating in flow through PS II; [PC⁻]_C, concentration of reduced plastocyanin, participating in cyclic flow; k_A, velocity constant of PSI acceptor side oxidation being connected with cyclic, noncyclic and pseudocyclic flows; ϕ_ND, portion of P⁺₇₀₀A⁻ and P⁺₇₀₀A (Klughammer and Schreiber, 2008); and 1 - ϕ_ND, portion of P₇₀₀A⁻ and P₇₀₀A.

There are two possible mechanisms for the observed connection between increases in ϕ_ND and EF(C) (Table 2). (i) Increased k_C is the main mechanism for increasing cyclic electron flow induced by VP or [CO₂]-lowering under control conditions. This can be caused by activation of any stage of cyclic electron flow, with the exception of P⁺₇₀₀ reduction, and increased concentrations of reduced plastocyanin. In this case, k_C increases contribute to transformation of P⁺₇₀₀ into P₇₀₀ and thereby lowers ϕ_ND (Figure 9). Thus, decreased ϕ_ND, increased photosynthetic cyclic electron flow, and negative correlation between changes in EF(C) and ϕ_ND must appear that are contrary to the present experimental results (Figures 4, 5, Tables 1, 2). (ii) Decreases in k_L suppress transformation of P⁺₇₀₀ into P₇₀₀ and/or increases in k_hv activate conversion of P₇₀₀ into P⁺₇₀₀ that then increases ϕ_ND (Figure 9). According to Equation (11), increased ϕ_ND must activate cyclic electron flow. As a result, ϕ_ND and EF(C) increase and, in this case, a positive correlation between changes in EF(C) and ϕ_ND should be observed. The second variant was in perfect accordance with the present experimental results. Thus, the following chain of events is supposed: VP → … → ϕ_ND increase → EF(C) growth.

Similar analysis can be employed for examining connections between increased ϕ_ND and reduced EF(PSII) (Figures 4, 5, Tables 1, 2). The positive influence of k_C changes was not probable because increased k_C decreases ϕ_ND(see above) and decreased k_C decreases EF(C) (Equation 11). Increased k_hv appeared to increase ϕ_ND(Figure 9); however, if ϕ_ND increased and k_L was not changed, then EF(PSII) must increase (Equation 10), which is contrary to experimental results. Moreover, the main reason for k_hv changes was modification of dII, but dII was probably not affected by VP. Alternatively, decreased k_L suppressed transformation of P⁺₇₀₀ into P₇₀₀ and increased ϕ_ND (Figure 9), while also lowering EF(PSII) (Equation 10). The k_L decrease could have been caused by inactivation of any stage of noncyclic electron transport, which preceded PSI, and which induced decreased concentrations of reduced plastocyanin. This last variant was in a good accordance with the experimental results obtained here (decreased EF(PSII), increased ϕ_ND, and negative correlation between changes in EF(PSII) and ϕ_ND). Decreased k_L reflected decreased electron flow from PSII. Thus, the chain of events was extended to yield: VP→ … → EF(PSII) decrease → ϕ_ND increase → EF(C) growth.

The present results indicate that decreased EF(PSII) reflects VP-induced lowering of ϕ_PSII (Figure 4, Table 1). According to published data (Pavlovič et al., 2011; Sukhov et al., 2012, 2014a,b) and the present results, this decrease in EF(PSII) was mainly induced by photosynthetic dark stage inactivation. In support of this conclusion, changes in A and EF(PSII) were strongly correlated and reduced CO₂ assimilation rate induced by [CO₂]-lowering decreased electron flow through PSII was similar to VP's effect. In addition, VP-induced EF(PSII) changes under low [CO₂] conditions were smaller than changes under control conditions. However, VP-induced EF(PSII) decreases were not absent under low [CO₂]. Considering that decreased A in these experiments (−1.08 ± 0.21 μmol·m⁻²·s⁻¹) was indistinguishable from a VP-induced respiration response (−1.10 ± 0.20 μmol·m⁻²·s⁻¹, Sukhov et al., 2014a), it was concluded that VP could also have suppressed electron flow through PSII without photosynthetic dark stage inactivation. Increased NPQ was a potential mechanism for VP's influence on PSII because its response is not dependent on electrical signal-induced decrease of CO₂ assimilation (Sukhov et al., 2014a). As a result, the following chain of events was proposed here: VP → … → inactivation of photosynthetic dark stage and NPQ increase → EF(PSII) decrease → ϕ_ND increase → EF(C) increase.

VP-connected proton flux from apoplast to cytoplasm, stroma, and lumen is a possible mechanism for initial induction of a photosynthetic response, including decreased ϕ_PSII (Grams et al., 2009; Sukhov et al., 2014a). It is known that VP generation is connected with transient H⁺-ATPase inactivation and proton influx (Stahlberg et al., 2006; Sukhov et al., 2013b), which changes intra- and extracellular pH (Grams et al., 2009; Sukhov et al., 2014a). However, decreased intracellular pH can suppress PSII photosynthetic activity and induces NPQ (Grams et al., 2009; Bulychev et al., 2013a,b; Sukhov et al., 2013a, 2014a). Taking into account these facts, it can be proposed that VP → H⁺ influx → inactivation of photosynthetic dark stage and NPQ growth → EF(PSII) decrease → ϕ_ND increase → EF(C) growth. Figure 10 shows this possible mechanism of VP influence on cyclic electron flow in more detail.

Figure 10.

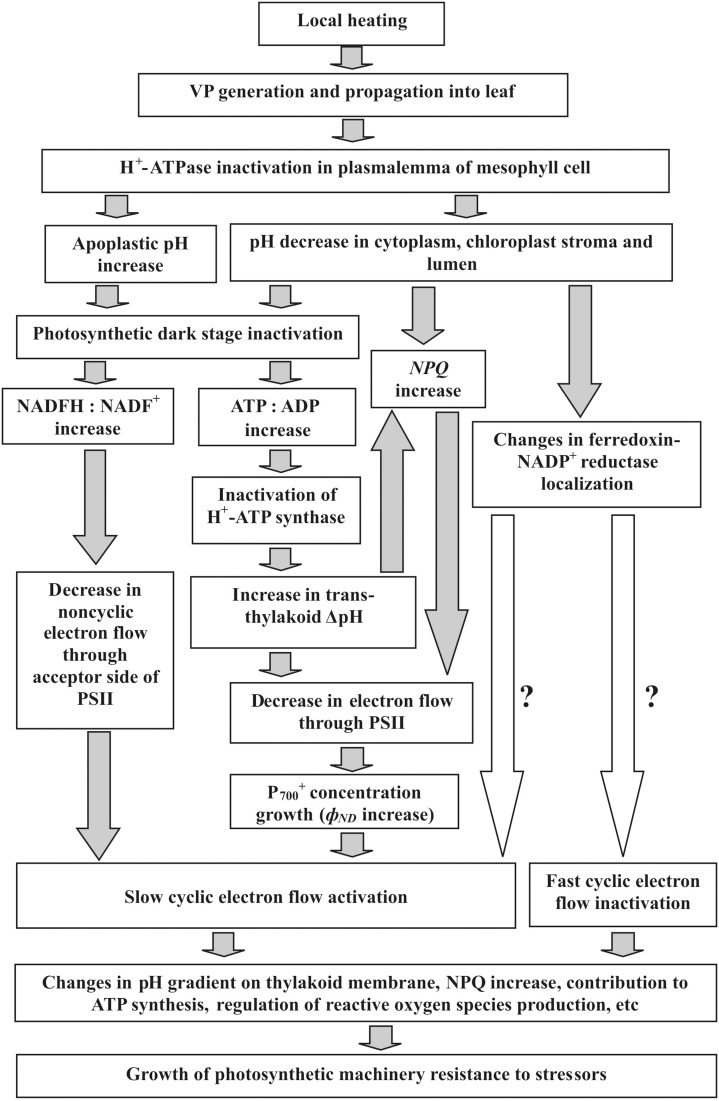
Potential mechanisms of VP-induced cyclic electron flow responses and their functional role in higher plants. Local heating induces VP, which propagates into leaves (our results, Sukhov et al., 2012, 2014a,b). VP generation connected with transient inactivation of H⁺-ATPase in plasmalemma (Stahlberg et al., 2006; Sukhov et al., 2013b). Alkalization of apoplast and acidification of cytoplasm (Grams et al., 2009; Sukhov et al., 2014a) are results of this inactivation. As chloroplast pH strongly depends on external medium in chloroplast suspensions (Werdan et al., 1975), cytoplasmic acidification can reduce pH in chloroplasts. Alkalization of apoplast and acidification of cytoplasm can suppress CO₂ influx from apoplast to cytoplasm and stroma, and reduce Calvin cycle activity (Bulychev et al., 2001; Grams et al., 2009; Sukhov et al., 2014a). Acidification of stroma essentially suppresses photosynthesis (Werdan et al., 1975) that might be connected with pH-dependent Calvin cycle enzymes (Wolosiuk et al., 1993) and nonphotochemical energy dissipation in PSII (Müller et al., 2001). VP-induced photosynthetic dark stage suppression (our results, Sukhov et al., 2012, 2014a,b) inactivates H⁺-ATP synthase and increases trans-thylakoid ΔpH (Pavlovič et al., 2011) that reduces electron flow through PSII (Schönknecht et al., 1995) and additionally stimulates NPQ (Müller et al., 2001). Increase of nonphotochemical energy dissipation in PSII (our results, Sukhov et al., 2014a) also decreases electron flow through PSII (our results). In turn, this increases P⁺₇₀₀ concentration and, thereby, stimulates cyclic electron flow (our results). Alternatively, photosynthetic dark stage inactivation may increase NADFH:NADF⁺ (Pavlovič et al., 2011) that can also intensify cyclic electron flow. There is an additional VP-induced slow cyclic electron flow activation, which is connected with fast cyclic flow inactivation, and is not affected by electron flow through PSII (our results). pH-Dependent changes in ferredoxin-NADP⁺ reductase localization (Alte et al., 2010; Benz et al., 2010) might participate in both additional activation and fast inactivation, because this enzyme possibly plays a role in cyclic electron flow (Joliot and Johnson, 2011). Finally, changes in cyclic electron flow might participate in electrical signal induced resistance of photosynthetic machinery to stressors (Retivin et al., 1999; Sukhov et al., 2014b). This might be because cyclic flow contributes to ATP synthesis, regulates oxygen species production, additionally increases NPQ, and keeps the PSI acceptor side oxidized, protecting it from damage (Joliot and Joliot, 2006; Rumeau et al., 2007; Zhang and Sharkey, 2009; Roach and Krieger-Liszkay, 2014).

It should be noted that photosynthetic dark stage inactivation can increase NADFH:NADF⁺ (Pavlovič et al., 2011) that decreases noncyclic electron flow through acceptor side of PSI and may stimulate cyclic electron flow. However, this process (decrease in k_A in Figure 9) induces increase in P₇₀₀A⁻ (ϕ_NA) that was not observed in experiments with varied CO₂ concentrations (Table 1). Thus, change in NADFH:NADF⁺ in unlikely to be main mechanism of EF(C) growth, but it can play minor role in the process.

VP-induced responses under far red light indicated that another mechanism of cyclic electron flow activation, not connected with noncyclic flow changes, also participated in the photosynthetic response. In this case, EF(C) activation and decreased ϕ_ND were observed and correlation between these parameters was negative (Table 3). Considering Figure 9 and Equation (11), it was concluded that such effects could have been caused by increased k_C. The mechanism of EF(C) activation was not clarified here, but the magnitude of the activation correlated with the magnitude of fast inactivation of cyclic electron flow; i.e., similar mechanisms for both processes were probable. It is known that pH decreases can change ferredoxin-NADP⁺ reductase localization (Alte et al., 2010; Benz et al., 2010); in addition, reductase possibly participates in cyclic electron flow (Joliot and Johnson, 2011). Considering this information, it was speculated that stromal pH changes influenced ferredoxin-NADP⁺ reductase localization and induced a two-stage EF(C) response, including inactivation and subsequent activation of cyclic flow (Figure 7). VP-induced inactivation of the acceptor side of PSI, which was not connected with decreased photosynthetic dark stage activity (Sukhov et al., 2012) and can caused by changes in ferredoxin-NADP⁺ reductase localization (Sukhov et al., 2014a), supported this hypothesis.

Thus, VP increased cyclic electron flow in an absolute (Tables 1, 3) and relative (Table 3) manner. A physiological role for this response could have been connected with increased photosynthetic machinery resistance to environmental stressors. It is known that cyclic electron flow can maintain a high proton gradient on thylakoid membrane and, thereby, contributes to ATP synthesis and NPQ increases (Zhang and Sharkey, 2009; Joliot and Johnson, 2011). Also, cyclic electron flow protects PSI and can regulate reactive oxygen species production by photosynthetic electron transfer chain (Rumeau et al., 2007; Roach and Krieger-Liszkay, 2014). It is known that electrical signals exert influence on the resistance of photosynthetic machinery to stressors in higher plants (Retivin et al., 1999; Sukhov et al., 2014b). In particular, VP increases PSI resistance to heating in pea (Sukhov et al., 2014b), and it was supposed here that VP-induced activation of cyclic electron flow participated in this increased resistance.

Stimulation of NPQ is important mechanism of cyclic electron flow influence on photosynthetic machinery resistance to stressors (Munekage et al., 2002, 2004; Zhang and Sharkey, 2009; Joliot and Johnson, 2011). This stimulation was observed under moderate actinic light (about 200 μmol·m⁻²·s⁻¹) (Munekage et al., 2002, 2004). Miyake et al. (2004, 2005) showed that NPQ was strongly depended on cyclic electron flow when EF(PSI) / EF(PSII) > 1.2–1.3. Table 4 shows that VP activated relative EF(C) from 27 to 38% in pea (EF(PSI) / EF(PSII) increased from 1.37 to 1.61), i.e., activation of cyclic electron flow can influence NPQ. Figure 8 shows that NPQ and relative EF(C) were linearly connected that can be interpreted according to Miyake et al. (2004, 2005) as stimulation of NPQ by cyclic electron flow. Positive correlation between NPQ and relative EF(C) under control conditions also supports this hypothesis. Thus, it may be supposed that VP-induced cyclic electron flow activation stimulates NPQ and, thereby, increases of photosynthetic machinery resistance.

Conflict of interest statement

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.