Abstract
Theoretical and empirical literature asserts that the sex ratio (i.e. M/F) at birth gauges the strength of selection in utero and cohort quality of males that survive to birth. We report the first individual-level test in humans, using detailed life-history data, of the ‘culled cohort’ hypothesis that males born to low annual sex ratio cohorts show lower than expected infant mortality and greater than expected lifetime reproductive success. We applied time-series and structural equation methods to a unique multigenerational dataset of a natural fertility population in nineteenth century Finland. We find that, consistent with culled cohorts, a 1 s.d. decline in the annual cohort sex ratio precedes an 8% decrease in the risk of male infant mortality. Males born to lower cohort sex ratios also successfully raised 4% more offspring to reproductive age than did males born to higher cohort sex ratios. The offspring result, however, falls just outside conventional levels of statistical significance. In historical Finland, the cohort sex ratio gauges selection against males in utero and predicts male infant mortality. The reproductive success findings, however, provide weak support for an evolutionarily adaptive explanation of male culling in utero.
Keywords: culled cohorts, male frailty, sex ratio, lifetime reproductive success, infant mortality
1. Introduction
Mean male lifespan falls below female lifespan in all human societies [1]. Historical mortality series also show greater risk of male relative to female mortality across virtually all age groups. This persistent and widespread male disadvantage has generated much research to identify mechanisms and test underlying theories regarding its causes [2]. Less research, however, examines male frailty in utero despite its potential to shape variation in human life-history traits including mortality and reproduction.
In humans, between 30% and 70% of conceptions do not survive to birth [3]. Male fetal losses outnumber female losses by 9–20% [4]. Recent work indicates that the secondary sex ratio (i.e. the ratio of male to female live births; hereafter, referred to as the sex ratio) may gauge important variation over time in male frailty in utero. The sex ratio falls in populations encountering ambient shocks, including cold, earthquakes and natural disasters [5–7]. In addition, male fetal deaths rose, and the sex ratio fell, following the terrorist attacks of 11 September 2001 [8]. These results indicate that ambient stressors raise the risk of fetal death more among male than female gestations and result in a lower birth sex ratio.
A separate but related literature builds on this evidence and assumes that a component of temporal variation in the sex ratio does not involve sampling error. Aggregate-level analyses of males born to low-sex-ratio cohorts report lower mortality in infancy than that of males born to high-sex-ratio cohorts ([9,10]; but also see [6]). Researchers infer from these results that low birth sex ratios may reflect greater than expected ‘culling’ of frail males in utero. This culling would thereby result in a relatively robust cohort of males that survive to birth.
One popular but controversial argument for selective male loss following perturbations involves the conservation of adaptive maternal mechanisms that spontaneously abort less fit fetuses [11,12]. Maternal mechanisms presumably assess the fitness of a fetus and environmental challenges to its survival if carried to birth [12,13]. These mechanisms would terminate gestations that, if continued to birth, would have yielded an infant with relatively low likelihood of surviving to reproductive age and producing offspring [14]. According to theory, if ambient stressors raise the risk of death more among male than female infants and children, then aborting these males in utero would increase the woman's overall yield of offspring because frail sons produce fewer offspring than do frail daughters. Natural selection would favour any mechanism that enabled a woman to abort a frail male fetus if it allowed the mother either to invest more in existing children or to conceive future offspring with greater lifetime reproductive success (LRS) than the frail son [14].
The argument that male fetal loss and sex ratio variation reflect an adaptive response to stressors would enjoy empirical support if males born to the most ‘culled’ (i.e. low sex ratio) cohorts showed greater offspring survival to adulthood than did males born to the least culled cohorts. The literature reports no such test of LRS. This circumstance likely arises from the rarity of the requisite historical, multigenerational data in humans. We address this gap in the literature and examine high-quality life-history data from a pre-industrial population in Finland.
We test two hypotheses implied by culled cohorts. First, we test whether male mortality rises with the cohort sex ratio at birth, implying that males from culled (i.e. low sex ratio) cohorts appear relatively more robust. Second, we test whether LRS rises above expected values among males born to the most culled (i.e. low-sex-ratio) cohorts, implying that such males would also enjoy relatively improved LRS due to their higher quality. To suggest potential mechanisms that may produce higher LRS in men from culled cohorts, we also examined the influence of cohort sex ratio on probability of marriage (i.e. sexual selection) and total fecundity. We focus our test on males given that the culled cohort hypothesis makes no prediction about females [10].
In addition to providing the first test, to our knowledge, of sex ratio variation and LRS, we improve upon earlier work on age-specific mortality by examining a historical, natural fertility population that more closely approximates the evolution of human life histories than do post-industrial societies [14]. Our stepwise time-series and structural equation methods also advance earlier work by using rich individual-level information contained in the Finland dataset to rule out a competing hypothesis that spurious findings arise owing to shared temporal patterns in the sex ratio, cohort mortality and LRS.
2. Material and methods
(a). Variables and data
We retrieved birth, reproductive success and death data from historical church records in Finland. Since the eighteenth century in Finland, the Lutheran Church has required the submission of accurate registers of all births, inter-parish movements, marriages and deaths in the country. From these records, we compiled life histories for individuals from rural parishes previously used in analyses of life-history traits (see the electronic supplementary material for more details) [15].
To derive our sex ratio variable, we used data from five parishes that contained a sufficiently large number of births per year to permit stable annual estimates. These data included two archipelagic (Kustavi and Hiittinen), two mainland (Ikaalinen and Tyrvää) and one Northern (Pulkkila) parish. Consistent with the literature, we used the sex ratio for each annual birth cohort as our independent variable [10]. Electronic supplementary material, figure S1, plots the annual sex ratio of the study population. The mean sex ratio was 1.06 (range: 0.80–1.37).
Given our interest in a cohort whose fertility and mortality schedules did not experience the industrial transition, we restricted our analytic sample to males born from 1790 to 1870. This restriction yielded 7824 Finnish males. This population depended on small-scale farming and supplemented their food supply by fishing. This pre-industrial period in Finland shows high birth and death rates. For example, mean male lifespan was 29.1 years for this population, and over 17% of males died in infancy (electronic supplementary material, table S2). Significant improvements in the standard of living occurred primarily during the twentieth century in Finland [16]. Almost all women finished reproduction by age 45 and few gave birth out of wedlock [17].
Previous literature on sex ratios and male lifespan indicates distinct age-specific mortality responses [9]. We therefore analysed the relation between birth cohort sex ratio and male mortality by distinct age groups. We analysed male mortality in infancy (before 1 year of age), childhood (ages 1–4), youth (ages 5–14) and over peak reproductive ages (15–50 years). Consistent with Hamilton's logic [18] in which the force of natural selection declines monotonically with age, as well as recent empirical findings in historical Finland [15], we hypothesized that any relation between the sex ratio and male mortality would appear strongest in infancy and decline thereafter.
We used as the LRS outcome the father's total number of children who were successfully raised to 15 years of age. We chose this measure over other indicators of fecundity (e.g. total number of live births) since high background infant and child mortality indicates that total number of children may not approximate LRS [19]. This dependent variable also correlates strongly with the long-term contribution to the future population gene pool [20].
(b). Approach
(i). Mortality
Our regression approach models the odds of male death within a specific age interval (died before end of interval = 1; survived to end of interval = 0) and uses a logit link function for the binary outcome. Only individuals alive at the beginning of the age interval qualify for inclusion in that age-specific test. We controlled for many individual-level variables that could confound the relation between the annual cohort sex ratio and male mortality, given their known relationship with survival and/or sex ratio in the study population. These factors included the following: month of birth [21], interbirth interval, firstborn status [22] and family socioeconomic status at birth (i.e. ‘wealthy’ farm owners and merchants; ‘middle class’ craftsmen and tenant farmers; and ‘poor’ crofters and laborers) [23]. We also controlled for region of birth (archipelago, northern region or mainland region) given the different background levels of mortality across the rural parishes. None of the independent variables correlated above 0.35, which minimizes the threat of multicollinearity.
Although the demographic transition arrived in Finland in the twentieth century, initial gains in cohort lifespan may have begun in Finland before 1870. To the extent that annual sex ratios and male mortality share secular patterns (including trend), this pattern could lead to spurious associations. To control for these secular patterns, we applied autoregressive, integrated, moving average (ARIMA) time-series routines to annual aggregate values of the risk of age-specific male mortality over our test period [24]. This purely empirical approach, employed frequently in biodemography [5,25], identifies autocorrelation in the time series. We used this identification strategy to arrive at annual, best-fitted values of male mortality rates for each specific birth cohort. This time propensity gauges the predicted value, conditional on time, of age-specific mortality based solely on secular patterns from 1790 to 1870. After yielding a time propensity of male mortality for each birth cohort year, we assigned these fitted values back to each individual and used it as a covariate in the logistic regression. The ARIMA routines detected unique autocorrelation ‘signatures’ for each mortality series (results available upon request), which supports the need to control such patterns with time-propensity values.
(ii). Lifetime reproductive success
We did not have access to all reproductive histories after 1900, which led to substantial missing data on offspring yield among cohorts born after 1850. We therefore restricted the LRS analysis to cohorts born from 1790 to 1850. This process yielded a sample of 3503 males.
We included all covariates as in the mortality analyses, with the addition of total annual cohort size. According to Easterlin's hypothesis, relatively small annual birth cohorts may have less within-cohort competition for employment and goods at adult ages and therefore yield greater than average marital success and fertility [26]. As with the mortality analyses, we used ARIMA time-series routines to arrive at fitted, time-propensity values for offspring that survived to 15 years. We then included this time-propensity value as a control variable in the individual-level analyses to ensure that secular trends in LRS do not confound our tests.
We used structural equation modelling (SEM) to test the relation between cohort birth sex ratio and offspring surviving to 15 years. The Finnish data also include information on marital status and number of children born. These variables may serve as ‘bottlenecks’ for our LRS measure in that, in the Lutheran Church culture in Finland, marriage correlates positively with the likelihood of bearing children, which in turn correlates positively with the number of children surviving to 15 years. The SEM permits testing these three dependent variables simultaneously within the same analytic framework and an exploration of whether the cohort sex ratio also predicted marital status and number of live offspring. We reasoned that the coefficients of the sex ratio for marital status, and number of live offspring, could suggest potential pathways by which the sex ratio ultimately affects LRS.
The numbers of live offspring, and offspring surviving to 15 years, had a large excess of zeroes (58% and 62%, respectively). We compared models with different count distributions (Poisson versus negative binomial) and with and without zero-inflation. We ultimately fitted these dependent variables in SEM using a zero-inflated Poisson distribution given that this model yielded the smallest Akaike Information Criterion (AIC) and Bayesian Information Criterion (BIC) value. In addition, we included in the model the residual covariances of marital status, live offspring and offspring surviving to 15 years. This inclusion accounts for the within-individual correlation among dependent variables and improves efficiency of standard error estimates (see additional details of SEM in the electronic supplementary material). We used robust maximum-likelihood estimation with Monte Carlo integration (5000 integration points) that allows for non-normal data and clustering of observations within mother (e.g. siblings). We handled missing data using full-information maximum likelihood. We performed the LRS analysis using Mplus v. 7.11 [27].
We employed two sensitivity analyses to assess the robustness of mortality and LRS results. First, for results that rejected the null, we included twin births. Second, we included cohort size in the mortality analyses to control for the possibility that sampling variation drove results.
3. Results
Males born during years with a relatively low annual sex ratio show higher quality in terms of early survival and later reproductive output. To aid interpretation, we standardized the sex ratio coefficient such that the mean is zero and a one unit change reflects a movement of 1 s.d. First, we analysed survival and found that, consistent with the culled cohort hypothesis, the risk of male infant mortality is reduced with a lower cohort sex ratio (likelihood ratio χ2 = 6.25, p = 0.01; table 1). This coefficient implies that a 1 s.d. decrease in the sex ratio predicts an 8% reduction in the odds of male infant death. This relation appears confined to infancy. We cannot reject the null for other age spans.
Table 1.
Coefficients (s.e.) predicting the log-odds of death at specific age intervals for males as a function of the annual cohort sex ratio at birth and control variables. Sex ratio scaled as a z-score (i.e. one unit change is 1 s.d.). All analyses control for clustering of sibling observations within mother.
| variable | death before 1 year |
death 1–4 years |
death 5–14 years |
death 15–49 years |
||||
|---|---|---|---|---|---|---|---|---|
| coef. | s.e. | coef. | s.e. | coef. | s.e. | coef. | s.e. | |
| cohort sex ratio at birth | 0.08 | (0.03)** | 0.04 | (0.04) | −0.05 | (0.05) | −0.03 | (0.04) |
| firstborn son (ref: all others) | −0.06 | (0.10) | −0.07 | (0.12) | −0.33 | (0.15)** | 0.01 | (0.13) |
| interbirth interval (in years) | −0.06 | (0.03)** | 0.01 | (0.03) | 0.01 | (0.04) | −0.01 | (0.03) |
| socioeconomic status at birth† | 0.09 | (0.05)* | −0.10 | (0.05)* | −0.31 | (0.07) | −0.11 | (0.07) |
| region of birth (ref: mainland) | ||||||||
| archipelago | −0.10 | (0.07) | −0.11 | (0.08) | −0.01 | (0.10) | 0.58 | (0.10) |
| northern region | 0.12 | (0.10) | 0.55 | (0.11) | 0.63 | (0.14) | 0.67 | (0.15) |
| time propensity of death¶ | 7.07 | (2.62)*** | 8.14 | (0.82) | 15.82 | (2.45) | 4.57 | (1.09) |
| indicators for birth month | included; not shown | included; not shown | included; not shown | included; not shown | ||||
| number of observations used | 7824 | 6500 | 5446 | 2292 | ||||
*p < 0.10; **p < 0.05; ***p < 0.01; two-tailed tests. †Continuous: 1 = poor; 2 = middle class; 3 = wealthy. ¶Please refer to §2b(i) for a description.
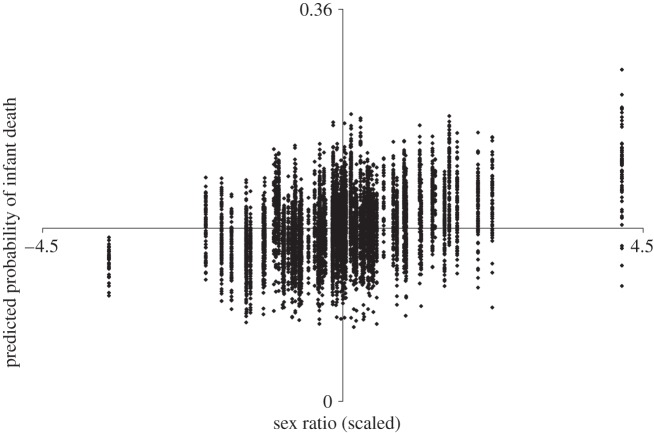
Figure 1 shows this discovered relation by plotting the predicted values of the probability of infant death, based on our regression model, as a function of the scaled sex ratio. The best-fitting line yields the positively signed sex ratio coefficient in table 1. At the lowest sex ratios (i.e. far left), the probability of infant death falls below the mean (i.e. 0.16). By contrast, males born in the highest sex ratio years show a probability of infant death that disproportionately lies above the mean.
Figure 1.
Scatter diagram of predicted probability of male infant death as a function of the cohort sex ratio at birth. Sex ratio is mean-centred at 0 and scaled in standard deviation units.
Males born to lower-sex-ratio cohorts raise slightly more offspring to 15 years than do males born to higher-sex-ratio cohorts (likelihood ratio χ2 = 3.02, p = 0.08; table 2). Although the result falls outside conventional levels of statistical significance, the sign of the coefficient lies in the hypothesized direction. The magnitude of this finding is modest in that a 1 s.d. decrease in the cohort sex ratio predicts a 4.1% increase in offspring that fathers raised to 15 years (95% confidence interval: −0.3 to 8.7%). This finding offers some support to the hypothesis that birth during a ‘low-sex-ratio’ year precedes increased LRS.
Table 2.
Coefficients (SEs) from the structural equation model predicting number of fathered offspring surviving to 15 years as a function of the annual cohort's sex ratio at birth and control variables. Father's marital status and number of liveborn offspring were used to estimate standard errors. Sex ratio scaled as a z-score. We fitted a zero-inflated Poisson distribution given its smallest AIC and BIC value relative to that of Poisson and negative binomial distributions. Results control for clustering of sibling observations within mother.
| father's total number of children surviving to 15 years |
|||
|---|---|---|---|
| variable | coef. | s.e. | p-value |
| cohort's sex ratio at birth | –0.042 | (0.023) | 0.08 |
| firstborn son (ref: all others) | –0.22 | (0.07) | 0.003 |
| interbirth interval (in years) | –0.04 | (0.02) | 0.04 |
| socioeconomic status at birth† | 0.09 | (0.04) | 0.02 |
| annual cohort size | –0.06 | (0.02) | 0.003 |
| region of birth (ref: mainland) | |||
| archipelago | –0.27 | (0.05) | <0.001 |
| northern region | –0.63 | (0.08) | <0.001 |
| time propensity of outcome | 0.10 | (0.05) | 0.03 |
| indicator variables for month of birth | included; not shown | ||
| number of observations analyzed | 3503 | ||
†1, poor; 2, middle class; 3, wealthy.
Exploration of the relation between the sex ratio and marital success, and number of live offspring, could not reject the null. The sex ratio was neither related to the likelihood of marriage (coef.: 0.017, s.e. = 0.046, p = 0.72) nor to the number of offspring (coef.: −0.022, s.e. = 0.020, p = .29). The slight LRS gain for males born to low-sex-ratio cohorts does not likely accrue via higher marriage rates nor number of born offspring.
Figure 2 displays the scatter plot of the fathers' predicted number of children surviving to 15 years (from the SEM analysis) and the scaled cohort sex ratio. Several LRS values are zero given that many Finnish males did not marry and yielded no offspring. Predicted LRS at extremely high and low sex ratios shows the hypothesized inverse association in that LRS is above the mean (i.e. 2.9 children) at low sex ratios and is below the mean at high sex ratios. The pattern of results at more modest deviations of the sex ratio, however, suggests a weak, non-significant inverse association.
Figure 2.

Scatter diagram of father's predicted number of children surviving to age 15 years as a function of the cohort sex ratio at birth (mean-centred at 0 and scaled in standard deviation units).
Sensitivity analyses for both the mortality and LRS outcome yielded essentially the same inference as in the initial tests (see the electronic supplementary material).
4. Discussion
The culled cohort hypothesis contends that the sex ratio at birth gauges male cohort quality. According to this argument, lower than expected cohort sex ratios reflect an excess of male fetal loss that occurs disproportionately among frail gestations [10]. Using life-history data from eighteenth and nineteenth century Finland, we examined whether males born to low-sex-ratio cohorts show greater than expected survival. We also tested whether these males show relatively greater LRS. Findings support the culled cohort hypothesis in that birth in a low-sex-ratio year predicts lower than expected male infant mortality. Men from low-sex-ratio cohorts also show a slightly greater than expected number of offspring reared to reproductive age. This finding, however, falls outside of conventional levels of statistical significance. Taken together, results indicate that the sex ratio may sensitively gauge the quality of male cohorts that survive to birth. The relatively small LRS finding among men, however, offers only modest support to the proposition that population-level sex ratio variation predicts fathers' yield of robust offspring.
Strengths of our analyses include the high quality of the Finnish dataset. The pre-industrial time period we investigate better approximates human mortality and reproductive schedules over much of human history than do contemporary datasets [28]. This circumstance allows for more refined tests of evolutionary hypotheses that assume high background rates of mortality. We also employ advanced methods using both time-series and individual-level control variables, which minimizes the likelihood of spurious findings.
Limitations include that we did not have complete life-history information on all cohorts. Missing data led to smaller sample sizes when examining mortality and reproductive outcomes later in life. We used the total number of offspring reared to age 15 years as a proxy of fitness, which includes both the quantity as well as the quality of the produced offspring. Other rate-sensitive measures of reproduction (i.e. individual lambda) may more sensitively capture fitness in the Finnish population that experienced expansion over the nineteenth century [29].
We lacked information on fetal deaths for each mother and therefore inferred excess culling of frail male fetuses among low-sex-ratio cohorts. Low sex ratios may arise due to a variety of other mechanisms (e.g. those that affect sex at conception) [30]. We, however, know of no other hypothesized mechanism for sex ratio variation that would predict its inverse relation with male infant mortality and LRS.
The magnitude of our infant mortality result appears larger than those of an ecological test in historical Sweden [9], but diverges from a null result in a Finnish study from 1865 to 2003 [6]. We attribute this divergence to the difference in time period used. We also caution against making direct comparisons given that we, unlike earlier work, use individual-level life-history data and its attendant methodology.
We view the male infant mortality finding as important for two reasons. First, results converge with previous research, which finds that individual variation in relative fitness arose largely from variation in male mortality rates before reproductive age [15]. Although we use a cohort measure of variation in male mortality, a more refined individual-level indicator of male frailty, such as low birthweight or prematurity, could hold implications for the conservation of a maternal selection mechanism in utero. Second, findings extend the ‘heterogeneity of frailty’ argument to the developmental stage in which mortality selection appears the strongest—in utero. Vaupel et al. [31] theorize, and others find empirical support for [32], the notion that the compositional change of cohorts, through the selective early mortality of its more frail members, leaves behind a smaller but more robust cohort with decelerating mortality rates. If this argument pertains to pregnancy, the sex ratio may sensitively gauge male cohort morbidity well beyond infancy [33].
The LRS findings indicate that culled males may not reflect a facultative adjustment conserved by natural selection. We note, however, that support for culled males in utero as an individual maternal adaptation [13] depends on sons yielding marginal gains in potential LRS relative to that of daughters [29]. This condition typically holds in populations with high reproductive skew (e.g. polygynous societies). In Finland, the Lutheran church precluded these conditions, which yielded a conservative test for LRS. We further note that rigorous tests of sex ratio variation as an adaptive maternal mechanism would want to account for any marginal fitness benefit of having a son and the trade-off cost to the mother's future reproduction of having a son [34,35].
Our male infant mortality finding coheres with Hamilton's logic that natural selection exerts its greatest pressure on mortality at earlier ages, when the replacement cost to the parents is relatively low [18]. As this argument relates to our findings, the failure of a male to survive past infancy imposes fitness costs since it precludes him from engaging in competition for female mates. By contrast, mortality at an older age (i.e. in mid-adulthood) would allow that male an opportunity to reproduce.
We hesitate to speculate post hoc on why the data support the infant mortality more than the LRS aspect of our hypothesis. However, one review posits that the early-life environment could exert independent effects on infant survival and later-life reproductive success (see fig. 2 of [36]). In addition, after birth, parental investment or local resource levels may exert a relatively strong influence on LRS that acts separately from effects on infant morbidity.
Scant work examines the role of mortality selection in utero on cohort quality over the life course. We report that, in a pre-industrial, natural fertility population, the sex ratio predicts male infant mortality and, to a lesser extent, number of fathered offspring that survive to reproductive age. These findings strengthen the evidence base that sex ratio variation sensitively gauges culling in utero among male gestations. We encourage more refined tests, especially in historical populations, of the extent to which variation in selection during pregnancy precedes cohort mortality and reproductive success.
Supplementary Material
Acknowledgements
We thank Lasse Iso-Iivari, Kimmo Pokkinen and Aino Siitonen for their assistance with data collection.
Data accessibility
We will make data available via the Dryad Data repository.
Funding statement
We thank the European Research Council for funding the data collection. S.H. was supported by The Turku Collegium for Science and Medicine.
References
- 1.Human Mortality Database. University of California, Berkeley (USA), and Max Planck Institute for Demographic Research (Germany) See www.mortality.org (Accessed on 11 May 13).
- 2.Maklakov AA, Lummaa V. 2014. Evolution of sex differences in lifespan and aging: causes and constraints. Bioessays 35, 717–724. ( 10.1002/bies.201300021) [DOI] [PubMed] [Google Scholar]
- 3.Boklage CE. 1990. The survival probability of human conceptions from fertilization to term. Int. J. Fertil. 35, 75–94. [PubMed] [Google Scholar]
- 4.Byrne J, Warburton D. 1987. Male excess among anatomically normal fetuses in spontaneous abortions. Am. J. Med. Genet. 26, 605–611. ( 10.1002/ajmg.1320260315) [DOI] [PubMed] [Google Scholar]
- 5.Catalano R, Bruckner T, Smith KR. 2008. Ambient temperature predicts sex ratios and male longevity. Proc. Natl Acad. Sci. USA 105, 2244–2247. ( 10.1073/pnas.0710711104) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 6.Helle S, Helama S, Lertola K. 2009. Evolutionary ecology of human birth sex ratio under the compound influence of climate change, famine, economic crises and wars. J. Anim. Ecol. 78, 1226–1233. ( 10.1111/j.1365-2656.2009.01598.x) [DOI] [PubMed] [Google Scholar]
- 7.Fukuda M, Fukuda K, Shimizu T, Moller H. 1998. Decline in sex ratio at birth after Kobe earthquake. Hum. Reprod. 13, 2321–2322. ( 10.1093/humrep/13.8.2321) [DOI] [PubMed] [Google Scholar]
- 8.Bruckner TA, Catalano R, Ahern J. 2010. Male fetal loss in the U.S. following the terrorist attacks of September 11, 2001. BMC Public Health 10, 273 ( 10.1186/1471-2458-10-273) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 9.Bruckner T, Catalano R. 2007. The sex ratio and age-specific male mortality: evidence for culling in utero. Am. J. Hum. Biol. 19, 763–773. ( 10.1002/ajhb.20636) [DOI] [PubMed] [Google Scholar]
- 10.Catalano R, Bruckner T. 2006. Secondary sex ratios and male lifespan: damaged or culled cohorts. Proc. Natl Acad. Sci. USA 103, 1639–1643. ( 10.1073/pnas.0510567103) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 11.Forbes LS. 1997. The evolutionary biology of spontaneous abortion in humans. Trends Ecol. Evol. 12, 446–450. ( 10.1016/S0169-5347(97)01179-8) [DOI] [PubMed] [Google Scholar]
- 12.Wells J. 2000. Natural selection and sex differences in morbidity and mortality in early life. J. Theor. Biol. 202, 65–76. ( 10.1006/jtbi.1999.1044) [DOI] [PubMed] [Google Scholar]
- 13.Kruuk LE, Clutton-Brock TH, Albon SD, Pemberton JM, Guinness FE. 1999. Population density affects sex ratio variation in red deer. Nature 399, 459–461. ( 10.1038/20917) [DOI] [PubMed] [Google Scholar]
- 14.Lummaa V. 2001. Reproductive investment in pre-industrial humans: the consequences of offspring number, gender and survival. Proc. R. Soc. Lond. B 268, 1977–1983. ( 10.1098/rspb.2001.1786) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 15.Courtiol A, Pettay JE, Jokela M, Rotkirch A, Lummaa V. 2012. Natural and sexual selection in a monogamous historical human population. Proc. Natl Acad. Sci. USA 109, 8044–8049. ( 10.1073/pnas.1118174109) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 16.Liu J, Rotkirch A, Lummaa V. 2012. Maternal risk of breeding failure and life-history shifts during demographic transitions in Finland. PLoS ONE 7, e34898 ( 10.1371/journal.pone.0034898) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 17.Lahdenperä M, Russell AF, Tremblay M, Lummaa V. 2011. Selection on menopause in two premodern human populations: no evidence for the mother hypothesis. Evolution 65, 476–489. ( 10.1111/j.1558-5646.2010.01142.x) [DOI] [PubMed] [Google Scholar]
- 18.Hamilton WD. 1966. The moulding of senescence by natural selection. J. Theor. Biol. 12, 12–45. ( 10.1016/0022-5193(66)90184-6) [DOI] [PubMed] [Google Scholar]
- 19.Gillespie DO, Russell AF, Lummaa V. 2008. When fecundity does not equal fitness: evidence of an offspring quantity versus quality trade-off in pre-industrial humans. Proc. R. Soc. B 275, 713–722. ( 10.1098/rspb.2007.1000) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 20.Brommer JE, Gustafsson L, Pietiainen H, Merilä J. 2004. Single-generation estimates of individual fitness as proxies for long-term genetic contribution. Am. Nat. 163, 505–517. ( 10.1086/382547) [DOI] [PubMed] [Google Scholar]
- 21.Lummaa V, Lemmetyinen R, Haukioja E, Pikkola M. 1998. Seasonality of births in Homo sapiens in pre-industrial Finland: maximisation of offspring survivorship? J. Evol. Biol. 11, 147–157. ( 10.1007/s000360050072) [DOI] [Google Scholar]
- 22.Faurie C, Russell AF, Lummaa V. 2009. Middleborns disadvantaged? Testing birth-order effects on fitness in pre-industrial Finns. PLoS ONE 4, e5680 ( 10.1371/journal.pone.0005680) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 23.Pettay JE, Helle S, Jokela J, Lummaa V. 2007. Natural selection on female life-history traits in relation to socio-economic class in pre-industrial human populations. PLoS ONE 2, e606 ( 10.1371/journal.pone.0000606) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 24.Box G, Jenkins G, Reinsel G. 1994. Time series analysis: forecasting and control, 3rd edn London, UK: Prentice Hall. [Google Scholar]
- 25.Catalano R, Ahern J, Bruckner T. 2007. Estimating the health effects of macrosocial shocks: a collaborative approach. In Macrosocial determinants of population health (ed. Galea S.), pp. 375–397. New York, NY: Springer. [Google Scholar]
- 26.Easterlin RA. 1976. The conflict between aspirations and resources. Popul. Dev. Rev. 2, 417–425. ( 10.2307/1971619) [DOI] [Google Scholar]
- 27.Muthén LK, Muthén BO. 1998–2013. Mplus user's guide, 7th edn Los Angeles, CA: Muthén & Muthén. [Google Scholar]
- 28.Partridge L. 1997. Evolutionary biology and age-related mortality. In Between Zeus and the Salmon: the biodemography of longevity (eds Wachter KW, Finch CE.), pp. 78–95. Washington, DC: National Academy Press. [PubMed] [Google Scholar]
- 29.Roff DA. 2002. Life history evolution. Sunderland, MA: Sinauer Associates. [Google Scholar]
- 30.James WH. 2012. Hypotheses on the stability and variation of human sex ratios at birth. J. Theor. Biol. 310, 183–186. ( 10.1016/j.jtbi.2012.06.038) [DOI] [PubMed] [Google Scholar]
- 31.Vaupel JW, Manton KG, Stallard E. 1979. The impact of heterogeneity in individual frailty on the dynamics of mortality. Demography 16, 439–454. ( 10.2307/2061224) [DOI] [PubMed] [Google Scholar]
- 32.Zajacova A, Burgard SA. 2013. Healthier, wealthier, and wiser: a demonstration of compositional changes in aging cohorts due to selective mortality. Popul. Res. Policy Rev. 32, 311–324. ( 10.1007/s11113-013-9273-x) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 33.Bruckner TA, Nobles J. 2013. Intrauterine stress and male cohort quality: the case of September 11, 2001. Soc. Sci. Med. 76, 107–114. ( 10.1016/j.socscimed.2012.10.012) [DOI] [PubMed] [Google Scholar]
- 34.Penn DJ, Smith KR. 2007. Differential fitness costs of reproduction between the sexes. Proc. Natl Acad. Sci. USA 104, 553–558. ( 10.1073/pnas.0609301103) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 35.Trivers RL, Willard DE. 1973. Natural selection of parental ability to vary the sex ratio of offspring. Science 179, 90–92. ( 10.1126/science.179.4068.90) [DOI] [PubMed] [Google Scholar]
- 36.Lummaa V, Clutton-Brock T. 2002. Early development, survival and reproduction in humans. Trends Ecol. Evol. 17, 141–147. ( 10.1016/S0169-5347(01)02414-4) [DOI] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Data Availability Statement
We will make data available via the Dryad Data repository.