Novel Quantitative Assessment of Metamorphopsia in Maculopathy

Abstract

Purpose.

Patients with macular disease often report experiencing metamorphopsia (visual distortion). Although typically measured with Amsler charts, more quantitative assessments of perceived distortion are desirable to effectively monitor the presence, progression, and remediation of visual impairment.

Methods.

Participants with binocular (n = 33) and monocular (n = 50) maculopathy across seven disease groups, and control participants (n = 10) with no identifiable retinal disease completed a modified Amsler grid assessment (presented on a computer screen with eye tracking to ensure fixation compliance) and two novel assessments to measure metamorphopsia in the central 5° of visual field. A total of 81% (67/83) of participants completed a hyperacuity task where they aligned eight dots in the shape of a square, and 64% (32/50) of participants with monocular distortion completed a spatial alignment task using dichoptic stimuli. Ten controls completed all tasks.

Results.

Horizontal and vertical distortion magnitudes were calculated for each of the three assessments. Distortion magnitudes were significantly higher in patients than controls in all assessments. There was no significant difference in magnitude of distortion across different macular diseases. There were no significant correlations between overall magnitude of distortion among any of the three measures and no significant correlations in localized measures of distortion.

Conclusions.

Three alternative quantifications of monocular spatial distortion in the central visual field generated uncorrelated estimates of visual distortion. It is therefore unlikely that metamorphopsia is caused solely by retinal displacement, but instead involves additional top-down information, knowledge about the scene, and perhaps, cortical reorganization.

We describe three novel quantitative measures of metamorphopsia in participants with retinal disease and age-matched controls. Metamorphopsia measures were significantly higher in the retinal disease group; however, the magnitude of displacement was not correlated among the three measures.

Introduction

Age-related macular degeneration, epiretinal membrane (ERM), macular edema, macular hole, and central serous retinopathy (CSR) have significant impairments of central visual functioning, through reductions in acuity,^1–3 the development of visual scotomas,^4,5 and the experience of distortions of visual space (metamorphopsia).^6–10 It is thought that metamorphopsia is a consequence of structural changes in the retina that result in displacement of retinal layers, and in turn, spatial distortion.^6,11 Metamorphopsia may therefore serve as a sensitive biomarker for detecting the presence, progression, and remediation of macular disease. However, while acuity and scotomas are routinely used as quantitative outcome measures, metamorphopsia is only assessed qualitatively, if at all. We recently reported a nonlinear interaction between letter identification and the size and shape of visual distortion, which suggests that it may be difficult to infer changes in distortion from changes in acuity.¹² Despite advances in vitrectomy, anti-VEGF, and laser treatments, metamorphopsia continues to be a common complaint of maculopathy patients.^8,13–15

Since metamorphopsia may go undetected during normal activity,¹⁶ Amsler grids are widely used to monitor metamorphopsia in the clinic but have limited value. Lack of fixation monitoring and perceptual “filling in” (e.g., in areas of the grid missing due to visual field loss) make it extremely difficult to identify the location or extent of distortion.^17,18 Other techniques have been developed to quantify distortion, including M-CHARTS,^7,19,20 preferential hyperacuity perimeters,^21–23 and shape discrimination hyperacuity.²⁴ However, none of these tests track eye movements and must assume that participants retain fixation so that measurements are relative to a constant retinal position, ideally the fovea. Although such assessments intend to monitor disease progression, it has proven difficult to localize distortion and quantify distortion across participants. More information available on the location, size, and nature of a perceived distortion would allow it to be mapped to an anatomical correlate. Moreover, understanding the retinal basis of metamorphopsia across different pathologies promises to provide information about disease progression derived from noninvasive perceptual measurements.

We examined three methods for quantifying metamorphopsia that focus on characterizing the location and spatial extent of monocular visual distortion. First, we determine if these methods can quantify metamorphopsia by comparing measurements in participants with maculopathy and age-matched controls. Second, we hypothesize if metamorphopsia arises directly from disruptions of retinal layers, measurements of distortion obtained with different methods should be correlated if those methods are indeed measuring pathological changes in the retina. Alternatively, if methods are uncorrelated, we can assume that other perceptual factors play a role in the final percept of metamorphopsia and the symptom is not only dependent on photoreceptor displacement in the retina. This would also imply the quantification of metamorphopsia is entirely dependent on the measurement tool, which is important in light of the many tools currently used in research and clinic.

Methods

Participants

Eighty-three participants were recruited from Advanced Eye Centers in Dartmouth, Massachusetts, based on the presence of macular pathology based on clinical examination and testing including slit-lamp biomicroscopy and SD-OCT retinal imaging, identified by an ophthalmologist (KL). Fifty had monocular maculopathy, 33 had binocular maculopathy, and all participants had intact foveal vision in the tested eye (no geographic atrophy at the fovea). The Table lists the age and number of participants who completed each task, but the majority of diagnoses were AMD, epiretinal membrane, macular edema, macular hole, and central serous retinopathy. This group took part in a parallel study in which we measured qualitative experiences of metamorphopsia with a questionnaire.¹⁶ Ten age-matched control participants were also tested who had best-corrected vision of 20/30 or better in both eyes and no known ocular pathology. The study was approved by the institutional review board committees of Schepens Eye Research Institute and adhered to the tenets of the Declaration of Helsinki.

Table.

Diagnosis and Age of Participants

	Computerized Amsler Grid		Square Completion Task		Dichoptic Pointing Task
	Participants, n	Mean Age	Participants, n	Mean Age	Participants, n	Mean Age
Wet AMD	24	78	13	74	2	62
Dry AMD	10	77	7	76	1	80
ERM	18	66	14	67	11	65
Macular edema	14	69	9	70	5	67
Macular hole	6	71	5	72	4	71
CSR	7	53	7	53	7	53
Retinal detachment	2	55	2	55	1	57
Macular scar	1	61	0	NA	1	61
Best disease	1	76	1	76	0	NA
Control	10	62	10	62	10	62

Synthesis of Distortions on a Computer-Generated Amsler Grid With Eye Tracking

All 83 participants and 10 controls performed a computer-based Amsler grid task (custom software developed in MATLAB; MathWorks, Inc., Natick, MA, USA). Participants viewed a grid monocularly on a LG 23-inch LCD monitor with 1920 × 1080 resolution at viewing distance of 90 cm. Gaze was monitored by a remote eye tracker operating at 500 Hz (EyeLink 1000; SR Research Ltd., Ontario, Canada). Eye positions were initially calibrated using a 16-point calibration in the eye tracker software (SR Research Ltd.). To bring awareness to eye movements and gaze direction, a green ring pattern was presented on the screen at the participant's point of regard. Participants were instructed to try to keep fixation centered on the fixation point in the center of the display, guided by the green ring.

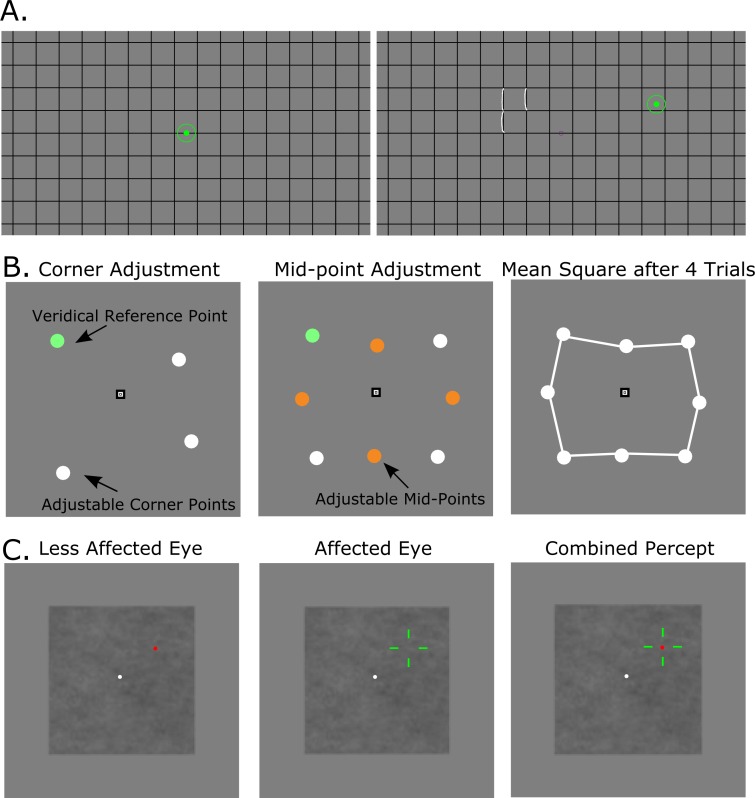
The Amsler grid was presented with two different line spacings, with lines spaced either 2 or 6° apart. The line thickness was adjusted from 0.04 to 0.18° (2–10 pixels) to make the grid easily visible for each participant, and the mean line thickness was 0.1° (±0.03°). Participants were required to maintain fixation on a central point, while their eye position was displayed (updated in real time) as a 1°, green circle overlaid on the grid. Each participant was first asked to determine the location of any distorted areas (while maintaining central fixation). The participant was then was asked to report the extent and shape of the perceived distortion on the grid, and then to guide the experimenter (EW) as she used the mouse to draw the described visual distortion as a series of overlaid white lines. Note that the white distorted line drawn by the experimenter was only visible to the participant when the participant was not fixating centrally. This allowed the participant to move his/her eyes back and forth between the fixation marker (when they could experience the distorted view of the unmodified grid without any overlaid drawing) and the distorted region (when they could experience the overlaid drawing of the distortion). This facilitated comparison of the participant's perceived distortion with the experimenter's on-screen rendition. This procedure was then repeated using the grid with 2° line spacing. The entire task took anywhere from 1 to 5 minutes per eye depending on the amount of distortion experienced by the patient. A demonstration of this task is shown in Figure 1A.

Figure 1.

Stimuli examples. (A) Example stimuli for the computerized Amsler grid task. The left panel shows the blank grid (i.e., how it appeared when the participant was fixating centrally), with the green circular disk representing the location of fixation as measured by the eye tracker. The right panel shows the gaze shifted away from central fixation, displaying the series of white drawn lines that depict the described distortion by the participant. To avoid confusion, the rendition of distortion was only visible when the participant was not fixating centrally. (B) Stimuli for the square completion task. The left panel depicts the first set of dots, a fixed green dot and three adjustable white dots, which the observer was required to move to create the four corners of a notional square. The center panel shows the set of four orange-colored bisecting dots that the observer was required to move to midpoints of the sides of the square. The right panel shows an example of a square created by one participant who completed four trials of both the corner and midpoint portion of the task. (C) Stimuli for the dichoptic pointing task. The left panel shows the moveable green crosshairs that were displayed only to the affected eye or a randomly chosen eye of the controls. The central panel shows a red target disk that displayed only to the less affected eye, or the other eye of controls. The right panel shows the combined cyclopean percept when the participant was wearing shutter glasses. A 1/F noise background was used to mask crosstalk.

Square Completion Task

Our second measure of metamorphopsia was a hyperacuity task used to assess the local magnitude and direction of visual distortion; 81% (67/83) of participants completed this task monocularly with an eye patch occluding the less affected eye. All 10 controls completed the task monocularly and a randomly chosen eye was patched. Apparatus and gaze position feedback were as in the Amsler grid task described above. Four circular disks, each 0.5° in diameter, were presented as four corners of a notional polygon on a gray background, as illustrated in Figure 1B. On the first trial, the disk in one (randomly selected) corner of the square was colored green, and it served as an immovable veridical anchor point. The positions of the three remaining white disks were adjustable and were initially positioned with a random displacement (±0.30°) from the corners of the notional veridical square. The participants' task was to maintain fixation on a central point and use the computer mouse to adjust the positions (click and drag) of the three white corner disks until they were perceived as aligned with the corners of the notional square. No time limit was enforced. Once the participant was satisfied that the four disks defined the corners of a square, the disk locations were fixed and four new orange-colored bisection disks were presented between the locations of the white corner disks with a random initial displacement of ±0.30° (Fig. 1B, center panel). The participant was asked to adjust the bisection points so they were evenly spaced between and inline with the corners of the square. Once the participant indicated (s)he was satisfied, the task was repeated with the immovable green anchor disk at a different corner of the square. This task was completed with the fixed corners of the notional square positioned at two eccentricities, 1.125 and 2.25° from fixation. Each participant completed the task a total of eight times with the immovable veridical anchor disk in a different location each trial so that all four corners at each eccentricity served as the anchor once. On completion of the task at each eccentricity, the participant viewed an average square composed of the eight geometric mean locations (from four trials) of the corners and bisection points (Fig. 1B, right panel). The participant assessed his/her individually created “average” square while fixating centrally to report to the experimenter if any part of the square now appeared distorted, and the experimenter recorded any locations that still appeared distorted. A total of 13% (9/67) of participants, but no controls, noted the square did not look straight or could not complete the task due to advanced dry AMD (geographic atrophy), and their data for this task were eliminated from further analysis. If the participant successfully compensated for any spatial distortion when aligning disks in the previous trials, the veridical image of the square may have appeared distorted center to a normally sighted viewer, but straight to the participant. The entire task took approximately 10 minutes per eye.

Dichoptic Pointing Metamorphopsia Assessment

The third task was performed by 50 participants who reported distortion in only one eye, or not in either eye (this was confirmed with the computer-based Amsler grid) and 10 control observers. The task was an adapted version of binocular correspondence perimetry²⁵ except with shutter glasses (nVidia, Santa Clara, CA, USA) instead of anaglyph glasses (to reduce crosstalk and color rivalry) and eye movement monitoring (to ensure compliant fixation). The same apparatus was as in the previous experiments. Although it was possible to track eye position through the shutter glasses, the reduction in signal strength made the eye tracker more vulnerable to noise so that the gaze was lost more frequently. Consequently, we also ensured compliance by having the experimenter observe the participant's eye position via the infrared image recorded by the eye tracker. Sixteen red circular points, each 0.35° in diameter falling on a 4 × 4 grid subtending 4.5 × 4.5° were presented only to the unaffected eye, one at a time in random sequence (Fig. 1C, left panel). A green crosshair (whose line width was adjusted to be easily visible to the participant) was presented only to the more impaired eye, and the participant controlled its location with the computer's mouse (Fig. 1C, central panel). The participant was required to fixate a central stationary white point and use the mouse to move the green crosshair so it was aligned with the red circular point (Fig. 1C, right panel). Again, points were presented one at a time at all 16 locations. It was desirable to have the dynamic/moveable crosshair presented to the impaired eye and the static alignment point presented to the better eye because it helped overcome any intraocular suppression so that participants were able to see both on screen at the same time.²⁶ A background of 1/F noise (noise whose amplitude spectrum was filtered to resemble that of natural images) was used to minimize the impact of any crosstalk between the two panels of the shutter-glasses, which could otherwise allow the reference dots and crosshair to be visible to either eye, and potentially cause an underestimation of the magnitude of distortion.²⁵ An example of this stimulus is shown in Figure 1C. Each participant completed two runs of the task (where each of the 16 points were presented once per run) to estimate variability around each reference location. The entire task took approximately ten minutes.

Surprisingly, even with movement of the cross hairs, 36% (18/50) of maculopathy participants with monocular impairment could not see the cross hairs and so were unable to successfully complete this task. We occluded their less-affected eye to confirm that the crosshairs were potentially visible to their impaired eye under these conditions. However, as soon as the better eye was not occluded, they became unable to see the crosshairs even after prolonged periods of moving the crosshairs with the mouse. We conclude that the better eye chronically suppresses the output of the impaired eye.¹⁶ This suppression was not observed in any of the control participants.

Results

Synthesis of Distortions on an Amsler Grid

A total of 95% (79/83) of participants identified distortions in at least one eye and 35% (29/83) in both eyes when presented with the monocular Amsler task. Additionally, 60% (20/33) of binocular participants reported central distortion in both eyes (central 3°). Central distortion was defined as any drawn distortion occurring in the central 4° of visual field. None of the control participants reported any distortion on the Amsler grid in at either eye when tested monocularly.

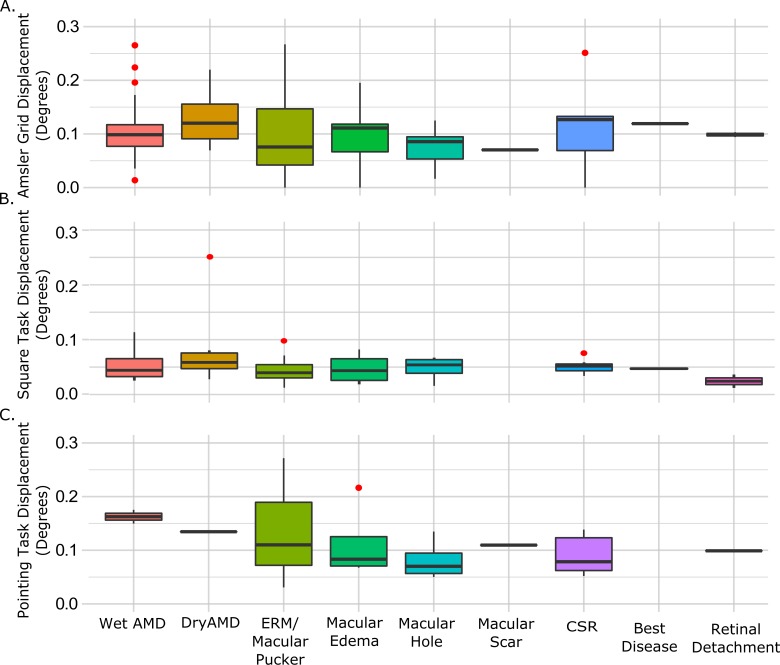
We computed a score for overall magnitude of distortion based on each participant's Amsler grid report. For regions of the grid where distortions were present, the absolute horizontal and vertical difference between the physical grid lines and those points drawn on the grid (or grid space) by the experimenter were averaged across the entire field space to compute the overall magnitude of distortion in both directions. Each individual (x, y) distortion point was classified as a horizontal or vertical displacement by assuming it belonged to the plane that it was minimally distant from (i.e., vertical displacement required the point to be closer to a vertical line than the nearest horizontal line and vice versa for horizontal displacement). We did not find any systematic differences between the two different grid spacings in either location or magnitude of distortion; therefore, data were combined across the two grid spacings to sample coarse and fine scales across the visual field. The averaged global distortion magnitudes ranged from 0.025 to 0.44° (μ = 0.106 ± 0.083°) in the horizontal and 0.002 to 0.397° (μ = 0.113 ± 0.081°) in the vertical direction. Global magnitude of distortion was calculated by averaging the vertical and horizontal displacements. An analysis of variance showed no significant difference in global magnitude across different maculopathy diagnoses (P = 0.75, F = 0.63; Fig. 2A). Furthermore, a two-sample t-test showed there was no significant difference in the magnitude of distortion in participants who experienced central distortion in both eyes when viewing the Amsler grid and those who did not experience central distortion in both eyes (P > 0.2).

Figure 2.

Magnitude of distortion across diagnostic categories. Absolute magnitude of distortion in the Amsler grid (A), Square completion task (B) and dichoptic pointing task (C). Bars show median values, the span of the boxes show the interquartile range, the lines (whiskers) show 95% confidence intervals, and the red dots show outliers.

Square Completion Task

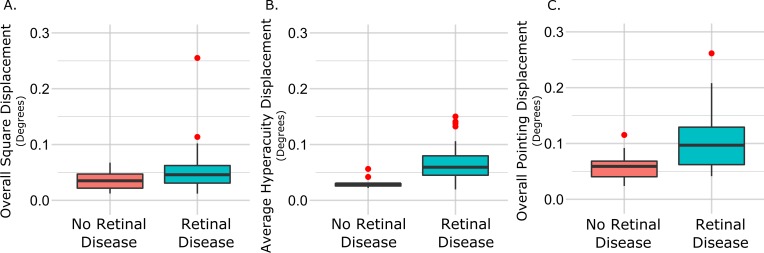
For each of the eight points that defined the corners and sides of the square for each eccentricity, we calculated the local horizontal and vertical differences between the veridical and adjusted points. The absolute (i.e., unsigned) local displacements at all 16 locations were then averaged (in both directions) to compute a global distortion magnitude. The distortion magnitudes ranged from 0.002 to 0.32° horizontally (μ = 0.06 ± 0.05°) and from 0.006 to 0.19° vertically (μ = 0.04 ± 0.03°). There was no significant difference in global distortion magnitude across maculopathy diagnosis groups along either axis (Fig. 2B). Ten control participants completed the task with a mean error of 0.03 horizontally and 0.04 vertically. A t-test confirmed overall magnitude of distortion was significantly lower in the 10 controls than in participants with maculopathy, but a nonparametric Mann-Whitney test did not reach significance (P = 0.04, P = 0.11, respectively). A comparison is shown in Figure 3A.

Figure 3.

Magnitude of distortion across maculopathy participants and controls. Comparison of distortion magnitude between maculopathy participants and controls in overall distortion magnitude (A) and hyperacuity errors (B) in the square completion task, and overall distortion magnitude in the dichoptic pointing task (C).

The square completion task requires both shape discrimination hyperacuity,²⁴ as well as Vernier hyperacuity,²⁷ thus we additionally quantified distortion in the square completion task by considering each side of the notional square as an individual hyperacuity task. At both eccentricities and on each side of the square, we took the difference in location of each adjusted point of the square to a line drawn through the midpoint. We averaged the absolute differences for each side at both eccentricities to obtain a mean Vernier hyperacuity error for horizontal and vertical planes (top and bottom, left and right sides of the square, respectively) for each individual. Mean Vernier hyperacuity errors were not significantly different across disease groups (P > 0.5). However, Vernier alignment error was significantly higher in participants with macular disease both horizontally (0.064°) and vertically (0.091°) than control participants (0.027 and 0.032°, respectively) as confirmed by both a t-test and Mann-Whitney test (P < 0.001). This comparison is shown in Figure 3B.

Dichoptic Pointing Task

A total of 32 participants completed the dichoptic pointing assessment. For each of the 16 alignment points, we calculated the local horizontal and vertical differences between physical and perceived location. As a conservative correction for any errors due that might be due to ocular deviation from esotropia or exotropia rather than metamorphopsia, we normalized every point by the mean displacement of all points. Without this correction, vergence errors would elevate any local differences between physical and perceived locations arising from perceptual spatial distortion. The absolute displacements at all 16 points were then averaged across the entire field to yield a single horizontal and vertical distortion magnitude score for the pointing task. Horizontal distortion magnitudes ranged from 0.031 to 0.272° (0.11 ± 0.06°) and vertical distortion magnitudes from 0.019 to 0.378° (0.10 ± 0.07°). There were no significant differences across maculopathy, as shown in Figure 2C. Ten control participants showed an average displacement of 0.05° in the horizontal direction and 0.038° in the vertical direction after correction for any ocular deviation. Both a t-test and Mann-Whitney test confirmed overall magnitude of distortion was significantly lower in the 10 controls than the participants with maculopathy (P < 0.001, P = 0.004, respectively). Error distributions for macular disease participants and controls are shown in Figure 3B.

Comparison Between Measurements

When all maculopathy participants and controls were included, there was only a significant correlation between the estimates of distortion magnitude assessed by Amsler grid and dichoptic pointing (n = 36, r = 0.45, P = 0.002), but not for overall distortion magnitude for the square completion and dichoptic pointing (n = 38, r = 0.04, P = 0.79), and Amsler grid and square completion (n = 61, r = 0.14, P = 0.24). Only points that fell within comparable visual field areas (i.e., central 5°) between the three different quantitative measures were used in this analysis. Within the maculopathy group alone, there was no significant correlation between the estimates of distortion magnitude assessed by square completion and dichoptic pointing (n = 28, r = 0.02, P = 0.94); Amsler grid and dichoptic pointing (n = 26, r = 0.21, P = 0.25); or Amsler grid and square completion (n = 51, r = 0.038, P = 0.77). All correlations between maculopathy participant scores across tests are shown in Figure 4. We also considered correlations between all three measures for only ERM patients, the diagnostic group most represented across all three measures, and found no significant correlations between any measures (P > 0.1).

Figure 4.

Correlation of distortion magnitude across measurements. Correlations between overall distortion magnitude estimated by three methods for participants with retinal disease. The line of best fit was calculated using linear regression and the shaded region represents the 95% confidence intervals. The top panel shows the comparison between Amsler grid displacement and dichoptic pointing, the middle panel compares the Amsler grid with the square completion task, and the bottom panel compares the dichoptic pointing with the square completion task.

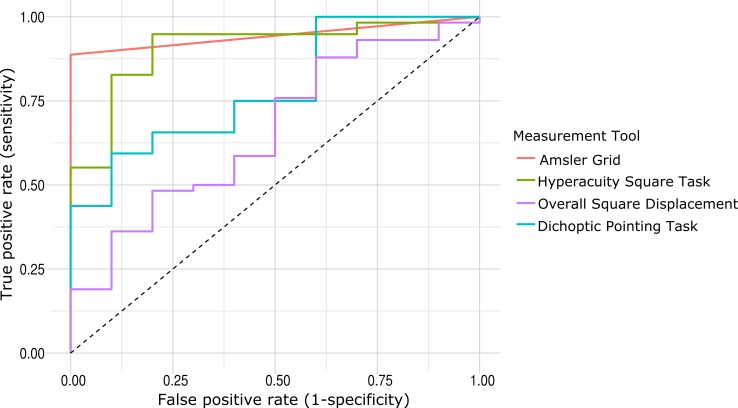
We plotted receiver operating characteristic curves for each of the measures to consider both the specificity and sensitivity of the measurements. We found that the Amsler grid task had the highest area under the curve (AUC) value of 0.944, meaning it was the most accurate test at distinguishing between participants with retinal disease and control participants. This high AUC value is due to the low false positive rate of the task (i.e., no control participants reported experiencing any distortion on the grid). The overall displacement value from the square completion task had the lowest AUC at 0.660. The dichoptic pointing and hyperacuity measure for the square Completion task had AUCs of 0.784 and 0.907, respectively. The ROC curves are plotted in Figure 5.

Figure 5.

Receiver operating characteristics (ROC) curves for all measurement tools. Each colored line represents the ROC curve for each measurement tool. The square completion task had two outcome measures, both the global displacement as well as the hyperacuity measures along each side, as described above.

Localized Measures of Distortion

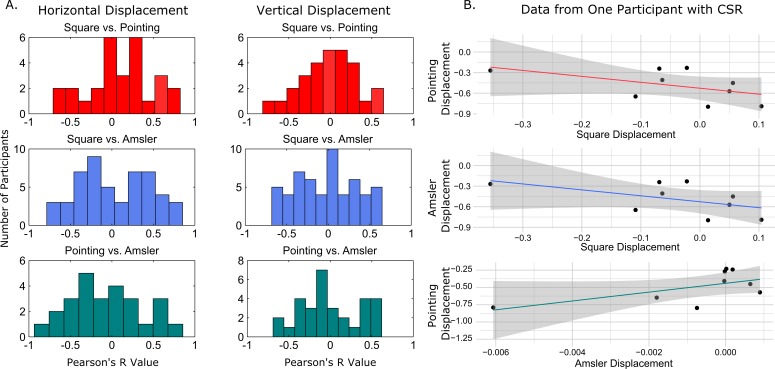
We also used a localized measure of displacement where all displacement values (from each of the 16 points in the square and pointing tasks) were interpolated to create a map of local displacement spanning 4.5 × 4.5° for both the vertical and horizontal dimensions. Negative values were assigned to points falling to the left of the veridical point in the horizontal dimension and below the veridical in the vertical direction. Nine corresponding points were selected across the interpolated map for each dimension, in each measure, to compare displacement based on location in the visual field. These points were selected at evenly spaced intervals at 1.5° eccentricities from both the horizontal and vertical median. Figure 6 depicts the steps in this process and locations of the points. Displacement values at these nine point locations were compared across distortion assessment methods within an individual. Figure 7A shows a distribution of Pearson's r values for each individual pairwise comparison for all three metamorphopsia measurements, and Figure 7B shows comparisons for one example participant with maculopathy. After Bonferroni adjustment for multiple comparisons, only one participant had a significant correlation in local distortion magnitude between the Amsler grid score and dichoptic pointing score in the horizontal direction.

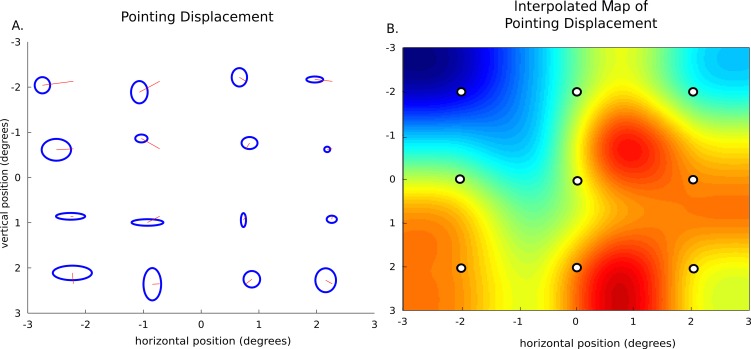
Figure 6.

Method of localized distortion measurements. Description of interpolation and localized measure selection. (A) Shows the displacement calculated from one participant's dichoptic pointing. Black lines represent the mean alignment endpoints at each of the 16-point locations, blue ellipses show the horizontal and vertical standard deviations. (B) Shows the interpolated map of the displacement around the 4.5 × 4.5° visual field. Blue color indicates displacement to the left and red indicates displacement to the right. The nine white points represent the sampled points used in the pairwise, location comparison across measurements.

Figure 7.

Localized pairwise comparisons across measurements. (A) Histograms of correlation coefficients for pairwise comparison between the three measures of metamorphopsia. The left panel shows localized comparisons for displacement values in the horizontal direction and the right panel shows comparison of vertical displacements. (B) Shows example comparisons for the point-by-point directional displacement values for one participant with CSR.

Discussion

The Amsler grid is currently the most widely used method for clinical and at-home assessment of metamorphopsia. In this paper, we addressed several problems with the conventional Amsler grid by implementing a computer-based Amsler test along with introducing two alternative methods for quantifying metamorphopsia.^17,18 Eye tracking ensured central fixation, monocular presentation avoided interocular suppression, and we were able to compute a quantitative displacement measure from the grid rather than merely classifying participants' responses as consistent with the presence of metamorphopsia. We measured perceived distortion around the central 5° in a square completion task and used a dichoptic setup to measure intraocular displacement in participants with monocular metamorphopsia. Our age-matched control group provided a baseline measure, and we found that distortion magnitudes were significantly lower in controls than maculopathy participants in all three tasks. This finding is an initial step in validating that all three metrics are able to detect metamorphopsia in retinal disease patients, as they were able to distinguish between participants with and without retinal disease. However, further research is necessary to determine the repeatability of these novel measures in a clinical population.

The fixation-compliant Amsler grid measured the direct perception of distortion as a spatial shift of grid lines as experienced by participants when maintaining central fixation. The dichoptic-pointing task also measured the direct perception of distortion: the placement of the crosshairs presented to the affected eye corresponded to the vertical and horizontal directional spatial shift to measure the distorted percept. In contrast, the square completion task measured spatial offsets that were required to correct distortion. The participant was asked to adjust the corners of a notional square until it appeared straight, therefore compensating for any perceived directional distortion. For example, if the participant's inherent distortion caused a square to appear to bulge outwards to the right, in order to perceive a “straight” square, the participant was required to compensate for the directional shift and draw a square bulging inward to the left. Thus we expected a positive correlation between local measures from Amsler and dichoptic pointing, but negative correlations between the square completion and the other tasks. However, there were no significant correlations between the localized directional displacements between any of the three measures.

All three tasks were similarly based on the perceived alignment of simple, retinotopically localized targets composed of lines or dots. However, the spatial structure of the stimulus varied across the three methods, and predictability (relative spatial information) systematically increased from the Amsler grid to the dichoptic pointing task; the Amsler grid provided geometric contours across the tested visual field, the square completion task involved the relative alignment of a group of points across the central visual field, whereas the dichoptic-pointing task tested single locations across the visual field independently with no relative structure ever being present (each dot was presented one at a time). Furthermore, the spatial extent of the stimuli across three measures may have contributed to differences across measures. The Amsler grid measured beyond the central 5° of visual angle, and although any distortions noted on the Amsler grid outside the 5° were not included in the correlation analysis, the global spatial extent of metamorphopsia may have influenced the local appearance of distortion measured in the other tasks.

The physiological origin of visual distortion remains unknown, yet there is evidence that metamorphopsia may in some cases be retinotopic and caused by displacement of retinal layers resulting in a mislocalization of light on the retina.^11,28 If perceptual distortions originated entirely in the retina, the relative spatial structure of the test stimulus used to measure that distortion should be irrelevant and all three measurements should equivalently map any mislocalization across the visual field. Our results clearly challenge this predication and therefore implicate other visual processes in the manifestation of metamorphopsia.

There is evidence that visual distortions may involve the combination of both retinal and cortical processing and may also be influenced by information about the scene/stimulus. It has recently been shown that our visual systems are especially tolerant to spatial distortions in natural scenes due to the visual systems ability to infer information about the scene.^29,30 Additionally, the phenomenon of “filling in” could contribute to distortions in locations where information is missing due to physiological,^31,32 pathological,³³ or transiently induced blind spots³⁴ (for reviews see Refs. 35 and 36). Filling in is dependent on the structure of the surrounding stimulus and involves spatial integration,³⁴ and has been shown to result in distorted shape perception due to cortical reorganization.³⁷ Charles Bonnet Syndrome is phenomenon where in patients with visual field loss, which may be based in the retina, report complex visual hallucinations.³⁸ This serves as additional evidence that retinal deficits in combination with cortical effects can produce an unexpected percept.

We did not compare results found in the computerized Amsler grid with a standard Amsler, and we are unable to conclude if the computerized version more accurately measured metamorphopsia without conducting a longitudinal study of disease progression. Additionally, the point of gaze is not recorded with a standard grid, so it would be difficult to align distortion collected with different methods. The computerized version of the Amsler grid allowed us to quantify distortion while ensuring the accuracy of central fixation with eye tracking.

One of the common subjective descriptions of distortions experienced by observers is of “wave-like” structure. Although our participants often reported no perceived distortion in natural settings,¹⁶ when measured under controlled laboratory conditions, the distortions were unambiguously locked to the orientation of the lines of an Amsler grid. Interestingly, we did not find any systematic differences in distortion magnitude between the two different grid spacings used in the Amsler grid task. However, anecdotally, a majority of participants' descriptions were “wave-like” patterns linked to the size of the grid spacing, similar to pattern repetition effects observed in descriptions of filling in.³⁴

The structural information in both the Amsler grid task and the square completion task have opportunities to be impacted by cortical “filling in” as well as top-down inferences about the shape and/or orientation of the stimulus. In contrast, the dichoptic pointing stimulus involved the sequential presentation of single points without any relative spatial structure. This key difference in the presence of relative structure of the stimulus may help to explain the differences in measured distortion across all three methods. Only the dichoptic pointing task eliminated any additional sources of spatial information and is therefore most likely to reflect pathological displacements of the retina. We therefore speculate that the dichoptic pointing assessment would more closely correspond to pathological displacement of retinal layers and we are currently studying this question in ongoing research.

Our most significant observation, that even though observers experienced some distortion in at least one eye there was no correlation between the magnitude of distortions measured with different methods, has fundamental implications. First, it suggests that efforts to measure and correct visual distortions through image remapping methods^39–41 are not likely to be successful. This is because the estimated inverse-distortion function will strongly depend on the method used to measure it and the effectiveness of correction may depend on the nature of the image to be viewed. Second, this finding challenges our initial assumption that visual distortions would be directly related to pathological changes in the retina. Instead, the results imply that significant perceptual reorganization may have occurred following retinal insult. This reorganization must be considered when evaluating existing methods to measure metamorphopsia in the home and clinic.

This is consistent with recent evidence that remapping can occur in patients with macular degeneration. For example, Casco et al.⁴² reported that the performance of a patient with Stargardt disease was better than that of age-matched controls at the same eccentricity across a variety of tasks, including contrast sensitivity, crowding and visual search. Similarly, Chung⁴³ showed changes in the shape of crowding zones in patients with AMD that were consistent with reorganization relative to a preferred retinal locus instead of a former fovea. These psychophysical data are in good agreement with functional magnetic resonance imaging data indicating sensory reorganization. Several groups have observed cortical response in foveal retinotopic locations whose input has been lost due to retinal lesions,^44–48 although others have not observed such effects.^49,50

It is also pertinent to consider the applications of our methods to at-home testing. If metamorphopsia is used to monitor disease progression, measurement tools must be user friendly, quick, and mobile. Although this study did not intentionally develop at-home monitoring methods, there may be future opportunities for such applications. All of our tests took under 20 minutes to complete binocularly. Additionally, the increasing availability of low-cost mobile eye-tracking systems can contribute to more accurate measurements with fixation monitoring. Further work is needed to see if the methods reported in this study can be translated for use in the clinic and at home.

A primary goal of the present study was to test if measurements of distortion varied based on the method used for testing. Quantification of the location and extent of metamorphopsia with accurate eye tracking leads to the possibility of correlating these measures with anatomical structural information from OCT data. A longer term goal of this research is to inform the structure function relationship between visual distortion and progressing macular pathology.

Conclusions

Absolute levels of metamorphopsia can be measured with a variety of qualitative and quantitative methods. There is little or no correlation between estimates of local distortion estimated with different methods, so it is unlikely that metamorphopsia is caused exclusively by displacement of retinal layers. The results suggest instead that metamorphopsia involves top-down information, knowledge about the scene, and perhaps, cortical reorganization.