Abstract
Water scarcity constrains the livelihoods of millions of people in tropical drylands. Tree planting in these environments is generally discouraged due to the large water consumption by trees, but this view may neglect their potential positive impacts on water availability. The effect of trees on soil hydraulic properties linked to groundwater recharge is poorly understood. In this study, we performed 18 rainfall simulations and tracer experiments in an agroforestry parkland in Burkina Faso to investigate the effect of trees and associated termite mounds on soil infiltrability and preferential flow. The sampling points were distributed in transects each consisting of three positions: (i) under a single tree, (ii) in the middle of an open area, and (iii) under a tree associated with a termite mound. The degree of preferential flow was quantified through parameters based on the dye infiltration patterns, which were analyzed using image analysis of photographs. Our results show that the degree of preferential flow was highest under trees associated with termite mounds, intermediate under single trees, and minimal in the open areas. Tree density also had an influence on the degree of preferential flow, with small open areas having more preferential flow than large ones. Soil infiltrability was higher under single trees than in the open areas or under trees associated with a termite mound. The findings from this study demonstrate that trees have a positive impact on soil hydraulic properties influencing groundwater recharge, and thus such effects must be considered when evaluating the impact of trees on water resources in drylands.
Key Points
Trees in dryland landscapes increase soil infiltrability and preferential flow
Termite mounds in association with trees further enhance preferential flow
Keywords: semiarid tropics, dry lands, agroforestry parklands, open woodlands, trees, termites, infiltration, preferential flow, rainfall simulations, Brilliant Blue FCF
1. Introduction
Drylands, defined here as arid, semiarid, and dry subhumid regions, cover around 35% of the global land area and are home to nearly 34% of the world population [Safriel et al., 2005]. People living in dryland environments, especially those in tropical areas, are among the poorest in the world [Safriel et al., 2005; SEI, 2005] and their livelihoods are strongly hindered by water scarcity [Falkenmark et al., 1989]. Access to water is officially recognized as a key limiting factor to socioeconomic development by the United Nations [1997]. Therefore, tree planting activities in these regions have been strongly discouraged based on the prevalent paradigm in forest hydrology that more trees lead to fewer water resources [Farley et al., 2005; Jackson et al., 2005]. This view, however, has been disputed as it focuses exclusively on the negative implications of increased tree cover (i.e., losses through transpiration) but neglects any beneficial impacts of trees on water availability, such as those derived from improved soil hydraulic properties or reduced soil evaporation [Bruijnzeel, 2004; Malmer et al., 2010]. Clarifying how the prevalent changes in tree cover in drylands affect water resources is an essential step to better decision and policy making concerning land use.
Dryland degradation encompasses both vegetation cover and soil degradation. It is estimated that some 10–20% of the world's drylands are degraded [Safriel et al., 2005]. In dry Africa, annual net changes of tree cover and other wooded land have been estimated at −0.91 Mha (0.34% annual rate of loss) and −0.89 Mha (0.20%), respectively, between 1990 and 2000, while the annual net change from dense to open tree cover was −0.39 Mha (0.32%) [Bodart et al., 2013]. Conversion of natural ecosystems to agricultural land not only entails changes in vegetation cover but leads to various forms of soil degradation such as decreased content of soil organic matter and nutrients, reduced soil infiltrability and water holding capacity, and soil structure deterioration [Islam and Weil, 2000; Nyberg et al., 2012; Oldeman, 1994]. These processes are even more prevalent in tropical dryland soils, since they are highly susceptible to degradation [El-Swaify et al., 1984].
Tree planting has been extensively promoted and adopted as a tool to restore or enhance the provision of ecosystem goods and services [Chidumayo and Gumbo, 2010; Jindal et al., 2008]. In Africa alone, over 715 Mha of deforested and degraded lands have been identified as potentially suitable for tree-based restoration [Minnemeyer et al., 2011]. However, tree planting in these regions has been increasingly questioned due to the large water use by trees [Hayward, 2005; Jackson et al., 2005]. Such claims are based on empirical evidence from numerous paired-watershed experiments showing that forest cover reduces both annual and low streamflows [Andreassian, 2004; Farley et al., 2005]. For applications in most drylands, however, it must be noted that these studies have some severe limitations. First, they compare extremes; they focus on open land versus dense forest plantations or closed forests and thereby neglect areas with partial tree cover. Second, they look mainly at fast-growing species, which have high water consumption rates. Third, they are largely biased toward temperate and subtropical regions. Fourth, none of them investigate the effects of trees on dry season flows on degraded land. The omission of areas with partial tree cover is of special concern given that in tropical Africa alone the area of open and fragmented forests and other wooded lands (including savannas and agroforestry systems with a tree cover below 10%) is 3 times larger than that of closed forests [Shvidenko et al., 2005]. These limitations make it difficult to draw any sound conclusions on the impact of trees on water resources in tropical drylands.
Trees could positively affect groundwater recharge through improvement of two soil properties: soil infiltrability and preferential flow. Soil infiltrability [Hillel, 2004] partitions rain water at the soil surface into Hortonian overland flow and infiltration into the soil, and therefore, controls the potential groundwater recharge and the generation of overland flow. A systematic review and meta-analysis on the effect of afforestation on water infiltrability in the tropics showed that soil infiltrability increased after tree planting across a wide range of rainfall conditions [Ilstedt et al., 2007]. Preferential flow refers to the mechanism by which water moves along certain preferred pathways bypassing a large fraction of the matrix porous network, which can result in a large portion of the infiltrating water moving through these pathways at significantly faster rates than the rest of the infiltrating water [Hendrickx and Flury, 2001]. The presence of preferential flow improves groundwater recharge since water flows faster through the vadose zone and is therefore less available for plant roots uptake and evaporation [Allison and Hughes, 1983; Johnston, ,; Mathieu and Bariac, 1996]. Trees can be responsible for enhancing preferential flow through macropores formed by their roots and associated soil fauna [Beven and Germann, 2013]. Root pathways are deeper and wider under woody perennials compared to annual crops and pastures, and preferential flow is consequently enhanced [Yunusa et al., 2002]. It is likely that the effect of trees on macroporosity extends beyond the canopy edge of single trees as the influence from roots is still present. For trees and shrubs, the ratio between the radius of the root system and that of the canopy has been estimated to be up to values of 10 [Lejeune et al., 2004]. Additionally, soil fauna exert important changes in soil fabric through mounding, mixing, and void formation [Hole, 1981]. In open woodlands, soil fauna is more abundant and diverse near isolated trees than in the adjacent open areas due to the improved microclimate near trees [Dunn, 2000]. In tropical dry Africa, the most characteristic soil macrofauna are termites. In the study site, termite mounds are usually associated with trees, while they are rarely found in the open areas. Among the impacts of termite activity on soil physical properties, there are changes in texture, structure, bulk density, soil water storage, and water infiltration and drainage [Lobry de Bruyn and Conacher, 1990]. In general, termite activity has been shown to increase soil infiltrability and soil water storage and drainage [Elkins et al., 1986; Leonard et al., 2004; Mando et al., 1996]. Other important influences of termites on ecosystem processes are those they exert on atmospheric nitrogen fixation [Fox-Dobbs et al., 2010] or on soil structure and dynamics [Darlington, 2005].
Little is known about the potential impacts on water resources from different levels of sparse tree cover in tropical drylands. The aim of this study was therefore to assess the effect of tree cover on two soil properties closely linked to groundwater recharge, namely, infiltrability and preferential flow, in an agroforestry parkland in semiarid Burkina Faso (West Africa). We hypothesized that: (a) preferential flow and soil infiltrability are highest next to trees; (b) in open areas among tree canopies, preferential flow and soil infiltrability decrease with increasing size of the open area, that is, with increasing distance to the nearest tree stem; (c) termite mounds associated with trees further increase preferential flow and soil infiltrability near the trees.
2. Material and Methods
2.1. Study Site Description
We conducted our study in the agroforestry parklands of Saponé, a municipality located 30 km south of Ouagadougou, in central Burkina Faso, West Africa (12°04′48″N, 1°34′00″W). Agroforestry parklands are land use systems in which scattered multipurpose trees are deliberately preserved on land also used for crops and/or grazing [Boffa, 1999; Bonkoungou et al., 1994]. These cultivated open woodland landscapes occur extensively around the world and constitute the predominant agricultural system in semiarid West Africa [Boffa, 1999]. The dominant tree species in our study area is Vitellaria paradoxa C.F. Gaertn. Sapotaceae (Karité in French and Shea in English) [Bayala, 2002], which typifies the West African parkland ecosystem in general [Breman and Kessler, 1995]. Overall mean tree density is about 20 trees ha−1, which is within the range of reported tree densities for sub–Saharan agroforestry parklands [Boffa, 1999]. Mean diameter at breast height for Shea trees within the study area is 45 cm. Annual crops such as millet, sorghum, cowpea, and beans are cultivated among scattered trees, and fields are regularly fallowed for 3–5 years. Grazing occurs in the fallows and in the cultivated plots once they have been harvested, but not during the cropping season.
The study area covers approximately 90 ha and has a rather flat topography (310–325 m a.s.l). Mean annual temperature (1952–2011) at Ouagadougou is about 28°C, with mean annual precipitation of 785 mm yr−1, ranging between 570 and 1180 mm yr−1 (Direction de la Météorologie du Burkina Faso). There is a single rainy season from April to October, with around 70% of the rainfall falling between July and September. About 50%, 25%, and 10% of the total rainfall in Ouagadougou falls with intensities above 30, 55, and 100 mm h−1, respectively, based on data from 1979 to 1994 [Hoogmoed and Stroosnijder, 1996]. The soils have sandy clay textures and low nutrient content [Jonsson et al., 1999] and have been classified as sols ferrugineux tropicaux lessivés [CPCS, 1967], corresponding to Ferric Lixisols [FAO-ISRIC-ISSS, 1998] or Alfisols [Soil Survey Staff, 2006].
Termite mounds are abundant within the study area, and they are most often found under trees. In the open areas, termite mounds are rarely present, especially large ones. Under trees, termite mounds are usually between 0.5 and 1.5 m height, and they cover around 20–50% of the projected canopy area of the trees.
2.2. Experimental Setup
We carried out 18 rainfall simulations and dye infiltration experiments within the study area (Figure 1) during July and August 2011. We selected six open areas among tree canopies which had at least one termite mound associated with one of the delimiting Shea trees. Each of these open areas constituted a transect consisting of three sampling positions (Figure 1b): (i) Shea tree associated with a termite mound, (ii) single Shea tree opposite to (i), and (iii) center of the open area. Under trees, the measurements were always performed under the canopy edge. Two size classes of open areas were selected to span a range of tree densities: large (between 77 and 127 m from tree to tree) and small (between 20 and 30 m), with three transects for each class (see Figure 1a for an overview of the location of the six transects).
Figure 1.

Study area: (a) location of the 18 sampling positions. Groups of three points correspond to a transect, with triangle symbols indicating points located in large open areas (lower tree densities) and circles in small open areas (higher tree densities). (b) Detail of a transect located in a small open area with its three sampling positions (tree: under a Shea tree; open: center of the open area; termite: under a Shea tree associated with a termite mound).
2.3. Rain Simulations and Blue Dye Tracing Experiments
We conducted one rainfall simulation per sampling position. A drip-type Amsterdam simulator [Bowyer-Bower and Burt, 1989] was used to simulate rainfall over a 106 × 55 cm runoff collection plot. A metal frame was inserted 5 cm into the soil and drained the collected runoff into a bucket through a pipe placed at the center of one of its long sides. Simulations were performed at an intensity of 45 mm h−1 for at least 1 h or until steady state infiltrability [Hillel, 2004] was reached. Hortonian overland flow (surface runoff onward) volume was recorded manually every 2 min, and the infiltration rate was subsequently obtained by subtracting the runoff rate from the rainfall intensity. Steady state infiltrability was afterward estimated by means of curve fitting to Philip's equation [Philip, 1957] using Excel's solver tool (Microsoft 2007). When no runoff was generated during the simulation period, steady state infiltrability was assumed to be equal to the rainfall intensity (45 mm h−1), which is the most conservative assumption possible.
Following each rainfall simulation, we carried out a dye infiltration experiment to visualize the flow pattern of the infiltrated water. This was done immediately after the rainfall simulation to ensure that soil saturation conditions were maintained. Fifty liters of Brilliant Blue FCF (C.I.42090, supplied by Astek CR) dye solution at a concentration of 4 g L−1 [Flury and Fluhler, 1995] were added into the runoff collection plot, which now had the outlet pipe closed, and allowed to infiltrate. This resulted in an initial water level of approximately 86 mm. Brilliant Blue FCF was used because it offers a good compromise between visibility, mobility, and toxicity [German-Heins and Flury, 2000]. The experiment was conducted under saturation conditions to enhance preferential flow initiation [Jarvis, 2007] and to ensure that differences in stained patterns were not related to initial soil moisture content [Bouma, 1991].
One hour after the ponding solution had infiltrated, soil profiles were prepared for photographing. A Nikon D-3100 digital camera with a focal length of 35 mm was used, and the resulting pictures had a resolution of 4608 × 3072 pixels. First, a pit was dug adjacent to one of the short sides of the dye infiltration experiment plot. The soil pit was 70 cm wide and 2 m long in order to accommodate a custom-made camera supporting device. This device consisted of a graded frame with inner dimensions 50 × 50 cm connected to a camera holding platform by way of three beams (see Figure S1 in the supporting information). The device was built in such a way that the focal point of the camera was at a distance of 1.5 m from the frame and centered into it. The camera holding platform, on the other side, was perpendicular to the frame to produce orthogonal pictures.
Once the soil pit was dug, six parallel vertical soil sections were prepared and photographed at intervals of 10 cm from each other (Figure 2). The sections were properly leveled avoiding smearing. The graded frame was placed with its top border adjacent to the soil surface and centered within the vertical dyed profile, leaving 2.5 cm to each side to reduce edge effects. Pictures were taken under day light conditions beneath an iron sheet to provide diffuse light and minimize direct radiation [Forrer et al., 2000].
Figure 2.
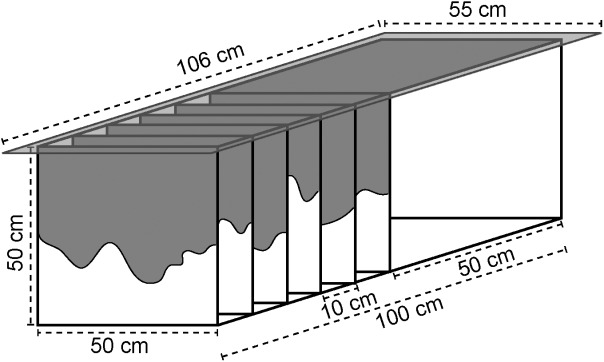
Schematic layout of the six vertical soil sections at one sampling position. The area of application of the dye solution is also shown (106 × 55 cm).
2.4. Image Processing and Classification
The pictures from the soil profiles were corrected for geometric distortion and then classified into dye-stained and nonstained classes using a supervised classification [Lillesand et al., 2008] (see supporting information for details). Moreover, two more classes were manually digitized: roots and black areas. Black areas corresponded to areas larger than 2 cm in any direction whose classification into dye-stained or nonstained classes could not be determined with certainty due to their dark (i.e., lack of) color. These areas, which ranged from 0 to 0.8% of the total area over all images (mean of 0.4% area), were excluded from further analyses. Black areas generally corresponded to macropores or macropores' parts which appeared black in the image. All image processing and classification steps were performed with ERDAS Imagine v9.3 image processing software (Erdas Inc., Atlanta, Georgia, U. S.).
2.5. Image Analysis and Preferential Flow Indices
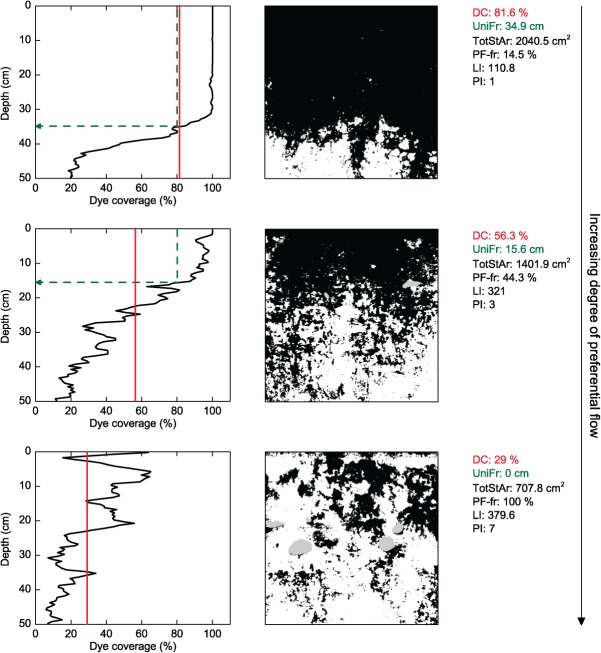
We quantified preferential flow from the classified images of the soil profiles. The analysis of the images was performed in ArcMap 10 (ESRI Inc., Redlands, California, U.S.) using the Tabulated area tool. This tool calculates the area covered by each class within different zones in the image which are defined by a polygon shapefile. Our polygon shapefile divided the 500 × 500 mm image into a grid with 100 rectangular polygons of 250 mm2 (500 mm wide by 5 mm high). We used the output data generated with this tool to calculate the following parameters as indices of preferential flow for each individual image (see Figure 3 for visual examples):
Figure 3.
Examples of classified stained profiles (black: dye stained; white: nonstained; gray: roots and black areas) and their corresponding profiles of dye coverage representing a gradient with increasing degree of preferential flow. Calculated parameters used to quantify the degree of preferential flow are shown next to each picture (DC: dye coverage (%), shown in red solid line; UniFr: uniform infiltration depth (cm), shown in green dashed line; TotStAr: total stained area (cm2); PF-fr: preferential flow fraction (%); LI: length index; PI: peak index).
Dye coverage, DC [Flury et al., 1994]: percentage ratio of the dye-stained area to the sum of dye-stained and nonstained area (equation 1). In preferential flow, in contrast to uniform flow, water flows through preferential pathways, which represent a lower volume of the soil matrix. Thus, total dye coverage will be lower when there is a higher degree of preferential flow. Dye coverage was also calculated for each of the 100 zones defined within each image to produce profiles of dye coverage (dye coverage versus depth).
| 1 |
where DC (%) is the dye coverage, D is the dye-stained area (cm2), and ND is the nonstained area (cm2).
Uniform infiltration depth, UniFr [van Schaik, 2009]: defined as the depth at which the dye coverage decreases below 80%, which represents the depth of the uniform infiltration front where the infiltration process is dominated by uniform flow. Soils with a high degree of preferential flow will therefore have low values of this parameter.
Preferential flow fraction, PF-fr [van Schaik, 2009]: fraction of the total infiltration that flows through preferential flow paths. It is calculated as (equation (2)):
| 2 |
where PF-fr is the preferential flow fraction (%), UniFr is the uniform infiltration depth (cm), which is multiplied by the width of the profile (50 cm), and TotStAr is the total stained area (cm2).
Length index, LI: Sum of the absolute differences between consequent dye coverage values with depth in a profile (equation 3). This parameter is related to the degree of heterogeneity of the dye infiltration pattern. Stained patterns from soils where infiltration is dominated by preferential flow are more heterogeneous than those where uniform flow is predominant. Therefore, soils presenting a high degree of preferential flow will have high values of this parameter.
| 3 |
where LI is the length index, and i represents a given depth interval (or zone) of the 100 in which dye coverage (DC (%)) was calculated.
Peak index, PI: Number of times that the vertical line defined by the total dye coverage intersects the dye coverage profile. This parameter is also related to the heterogeneity of the stained patterns, with high values of the parameter indicating a high degree of preferential flow.
These five parameters were used because they are fairly simple to calculate once the images have been classified into stained and nonstained areas. The first three parameters have been used in similar studies before, while the two last are new and tested here since we argue they are simple to calculate but may provide more detailed information about the dye patterns. Other more detailed indices and analysis techniques have been proposed in the literature [e.g., Hatano and Booltink, 1992; Hatano et al., 1992; Weiler and Fluhler, 2004]; however, these have not been used widely due to their complexity.
2.6. Statistical Analysis
The mean value for each of the calculated parameters of preferential flow was computed from the six vertical soil sections in each sampling position. We then used nonparametric statistical methods to test our hypotheses due to small sample sizes and the inability to adequately test for normality. The statistical analysis was performed with the package stats in R, version 3.0.1 [R Core Team, 2013]. Wilcoxon signed rank test (wilcox.test function in R) was used to test the hypotheses that soil steady state infiltrability and degree of preferential flow were higher under single trees (n = 6) compared to the open areas (n = 6) and also to test whether these parameters were higher in areas under trees associated with termite mounds (n = 6) compared to areas under single trees and open areas. To test the hypothesis that in open areas among trees soil steady state infiltrability and preferential flow decreased with increasing distance to the nearest tree stem, we used Spearman's correlation test (cor.test function in R). Spearman's rho statistic (rs) ranges between −1 and 1 and estimates a rank-based measure of association. The significance level was set to 0.05.
3. Results
Under single Shea trees, both the degree of preferential flow and soil steady state infiltrability were generally higher than in open areas, while under trees with an associated termite mound preferential flow was further enhanced and steady state infiltrability was reduced (Figure 4).
Figure 4.
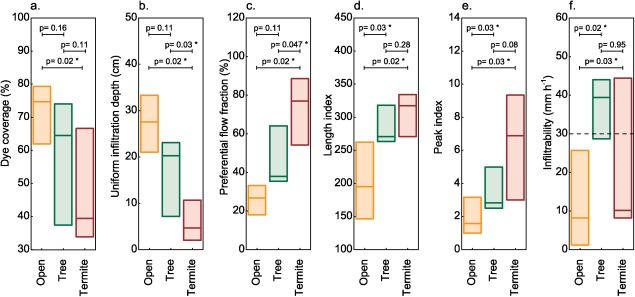
Boxplots (median, first, and third quartile; n = 6) of the different measured variables ((a) dye coverage, (b) uniform infiltration depth, (c) preferential flow fraction, (d) length index, (e) peak index, and (f) steady state infiltrability) in the different positions (open: center of open areas; tree: under Shea trees; termite: under Shea trees associated with termite mounds). P values for the Wilcoxon sign ranked test are also shown. The dashed line in Figure 4(f) indicates the rainfall intensity above which 50% of the total rainfall falls in Ouagadougou [Hoogmoed and Stroosnijder, 1996].
All five parameters used in this study to quantify the degree of preferential flow show the same overall trend: preferential flow was highest under trees associated with termite mounds and lowest in open areas, while single trees represented an intermediate stage (Figures 4a–4e). The length index, which quantifies the degree of irregular patterns in the stained profile and hence the degree of preferential flow, was significantly lower in open areas than under trees, irrespective of the presence or absence of termite mounds (Figure 4d). In open areas far from tree influence, the median length index was 195, while under single trees and trees associated with termite mounds it was 271 and 317, respectively (Figure 4d). This indicates that infiltration pathways were more irregular under trees and more uniform in the open areas. Similarly, the results from the peak index show that in positions under trees the stained profiles were significantly more irregular than in open areas, with median values of 2.8, 6.9, and 1.6 for single trees, trees with termite mounds and open areas, respectively (Figure 4e). Differences in the degree of preferential flow between open areas and single trees were not statistically different for dye coverage, uniform infiltration depth, and preferential flow fraction, but followed the same overall trend. Moreover, differences in these variables were always significant between open areas and trees associated with termite mounds. Median dye coverage from stained patterns in open areas was 75%, while under single trees and under trees associated with termite mounds it was 65% and 39%, respectively (Figure 4a). This difference indicates that under trees with termite mounds, a larger fraction of the infiltrating water was channelized through preferential pathways. The median depth of the uniform infiltration front, below which preferential flow is considered to initiate, was 5 cm under trees associated with termite mounds, a value significantly lower than that for positions under single trees and open areas which were 28 and 20 cm, respectively (Figure 4b). Therefore, in open areas and under single trees the water flowed uniformly through the soil matrix down to deeper depths in contrast to positions under trees associated with termite mounds, where the preferential flow was initiated earlier as shown by the shallower uniform infiltration front. Accordingly, median values of preferential flow fraction indicate that in open areas only 27% of the stained area corresponded to preferential flow pathways, whereas under single trees this value increased up to 38%, and was significantly higher when the trees had an associated termite mound, with a median value of 77% (Figure 4c).
Median steady state infiltrability under single trees was 39 mm h−1 and was significantly higher than in open areas (8 mm h−1; Figure 4f). Contrarily to our hypothesis, however, median steady state infiltrability under trees associated with termite mounds was lower (10 mm h−1) than under single trees. Steady state infiltrability under trees was in most cases high enough to avoid the generation of surface runoff during rainfall events with intensities up to 30 mm h−1 (Figure 4f), which is the median rainfall intensity at Ouagadougou [Hoogmoed and Stroosnijder, 1996]. On the other hand, in the open areas, even the less intense rainfalls would result in surface runoff. Under trees associated with termite mounds, steady state infiltrability was highly variable; low-intensity rainfalls would usually generate surface runoff, but in some cases even more intense rainfalls could infiltrate. This can also be observed when looking at the plots of runoff versus time for the 18 performed rainfall simulations (see Figure S2 in the supporting information).
The size of the open areas, and thus tree density, also influenced the degree of preferential flow, but not steady state infiltrability. Overall, preferential flow in open areas decreased with increasing distance to the nearest tree stem (Figure 5). Both dye coverage and peak index were significantly correlated with distance to the nearest tree, while in the case of preferential flow fraction and length index the correlations were close-to-significant. Steady state infiltrability in the open areas, on the other hand, was not affected by distance to the nearest tree.
Figure 5.
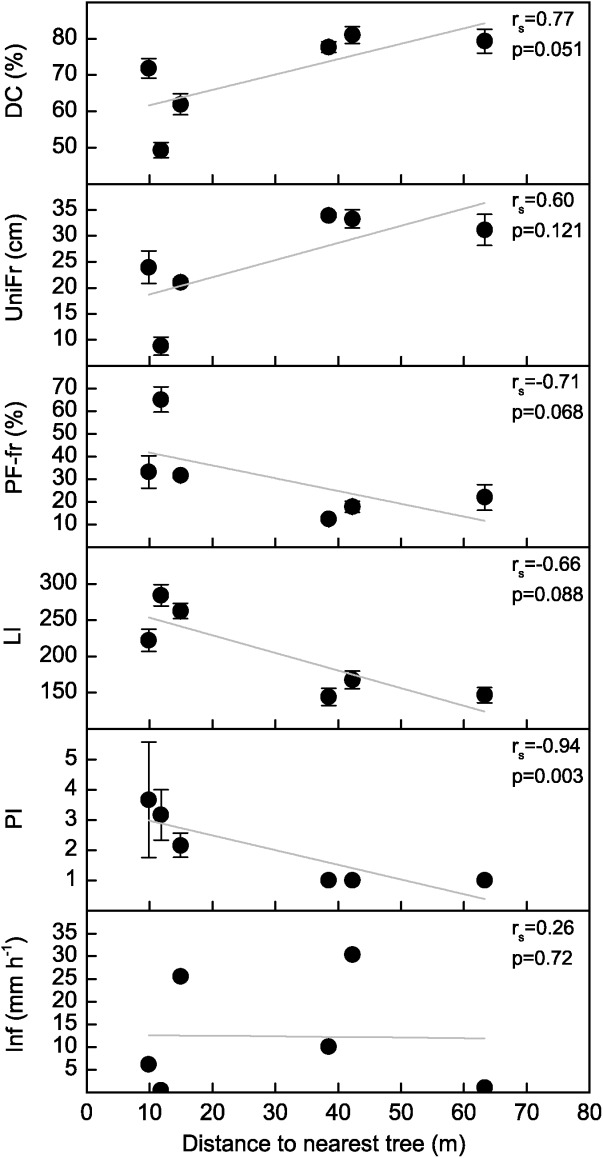
Scatterplot of the different preferential flow indicators (DC: dye coverage (%); UniFr: uniform infiltration depth (cm); PF-fr: preferential flow fraction (%); LI: length index; PI: peak index) and steady state infiltrability (Inf) versus distance from the sampling point to the nearest tree stem for the open areas (n = 6). Error bars indicate standard error of the mean (n = 6, only for preferential flow indicators). Spearman's rho statistic (rs) and P values for the Spearman's correlation test are shown. A linear regression is also shown for illustrative purposes only.
4. Discussion
In our study site, a clear positive effect of Shea trees on soil hydraulic properties was observed, with soil infiltrability and preferential flow being higher under the canopy edge of trees than in the surrounding open areas. These results may be explained by the improved soil structure and porosity beneath trees, mainly linked to the higher content of soil organic matter and increased faunal and root activity. In drylands, the content of organic matter is enriched in the soils beneath the canopies of scattered trees because of the larger root and leaf litter inputs they receive [Bayala et al., 2006; Belsky et al., 1993; Breman and Kessler, 1995]. As a result of this increase in organic matter and the improved microclimate beneath trees [Belsky et al., 1993], the activity of soil fauna is enhanced [Dunn, 2000]. The enhanced faunal and root activity under the canopy of woody plants, coupled with larger contents of organic matter, contributes to the development of macropores and more stable soil aggregates, which in turn improves soil structure and soil hydraulic properties [Belsky et al., 1993; Greene, 1992; Mordelet et al., 1993]. Moreover, the soil surface beneath trees is generally protected from raindrop impact, which combined with the improved soil stability, reduces the formation of soil crusts which strongly limit water infiltration [Hoogmoed and Stroosnijder, 1984].
Median steady state infiltrability under single trees was about 5 times larger than in the open areas. This finding is in agreement with previous research from other dryland ecosystems showing that soil infiltrability beneath the canopies of woody plants is generally higher than in the intercanopy patches [Belsky et al., 1993; Dunkerley, 2000; Eldridge and Freudenberger, 2005; Elkins et al., 1986; Lyford and Qashu, 1969; Wilcox et al., 2003]. Such variability in infiltration rates among different areas in the landscape can lead to the spatial redistribution of surface water from low-infiltrability patches functioning as sources of surface runoff to high-infiltrability patches functioning as sinks. Patterns of surface water redistribution from bare to vegetated areas have been observed in various dryland systems [e.g., Bromley et al., 1997; Reid et al., 1999] and are believed to promote the development of the so-called “islands of fertility” under plant canopies [Schlesinger et al., 1990]. In the present study site, the low infiltrability rates in the open areas, coupled with the prevailing high-intensity rainfalls [Hoogmoed and Stroosnijder, 1996], will ensure the production of surface runoff, which is frequently observed in the study area during rainfall events. On the other hand, the higher infiltrability values under trees will attenuate the generation of runoff. Our results suggest that open areas in dryland woodlands act as sources of surface runoff, while trees capture part of this water and thus may act as water collectors or “ecosystem wicks” [Eldridge and Freudenberger, 2005]. The occurrence of this phenomenon is important for the water balance since sink areas can end up receiving more water than the actual rainfall [e.g., Bromley et al., 1997; Gaze et al., 1997], thereby locally changing the balance between evapotranspiration and soil water infiltration and potentially leading to improved soil moisture and groundwater recharge. Moreover, less surface runoff generation under trees translates into lower erosion potential compared to open areas [Reid et al., 1999], which highlights the importance of trees in preventing soil erosion in dryland landscapes. Indeed, vegetation cover patterns strongly affect the leakiness of a landscape, or its potential to lose soil sediments, an indicator that is used to monitor the health of arid and semiarid landscapes [Ludwig et al., 2007].
Under trees, the degree of preferential flow was higher than in the open areas. This result is consistent with the observed increase in preferential flow along decayed root channels under shrubs in a desert ecosystem in the United States [Devitt and Smith, 2002], or along root channels and termite tunnels under vegetated patches in tiger bush vegetation in Niger [Bromley et al., 1997].
As expected, termite mounds associated with Shea trees further increased the degree of preferential flow. Termite mounds are signs of increased termite activity and the construction of galleries and tunnels further enhances macroporosity. However, contrary to our hypothesis and despite the observed increase in the degree of preferential flow, soil infiltrability decreased under trees associated with a termite mound compared to single trees. A possible explanation for this might be the differences in soil surface characteristics between these two positions; in the field it was observed that the soil surface around termite mounds was covered by a surface crust, despite having some large macropores that connected to the surface. The intensity of the simulated rainfall was probably too low to generate a water pressure close to saturation that allowed these large macropores connected to the surface to fill with water and therefore initiate preferential flow [Jarvis, 2007]. In contrast, when dye solution was applied under ponding conditions, preferential flow was rapidly initiated and infiltration rates were higher [Joelsson, 2012]. Reduced soil infiltrabilty, measured using rainfall simulations, around Trinervitermes sp. mounds has also been observed in a study conducted in Ivory Coast [Janeau and Valentin, 1987]. This reduction was ascribed to low-permeability sealed rings surrounding the termite mounds which result from depositions of fine materials in the topsoil as a result of termite activity. Other studies, however, have reported an increase in soil infiltrability with termite activity [Elkins et al., 1986; Leonard et al., 2004; Mando et al., 1996], though they do not specify whether the measurements were done around mounds. In a semiarid woodland in Australia, it was shown that steady state infiltration differed among locations around the termite nests, with significantly lower values on the heavily compacted pavement zone than in the surrounding annular and interpavement zones [Eldridge, 1994]. In our study site, the results suggest that under intense rainfall events where large amounts of runoff are generated in the open areas or around termite mounds, enough surface water can be accumulated around termite mounds to activate the transport of water through macropores. We also observed a high variability in steady state infiltrability for positions under trees associated with termite mounds compared to single trees or open areas; the specific location of the simulations around the termite mound, related to the presence or absence of compacted soil or macropores connecting to the sealed surface, could explain such high variability. The high variability in steady state infiltrability around termite mounds could also be related to the fact that steady state infiltrability does not capture well enough the actual process of infiltration occurring in these highly macroporous soils. When the degree of macroporosity in the soil is high, the steady state assumption might not hold. In these cases, alternative, more complex models of water flow should be used, such as dual porosity models [Gerke and Vangenuchten, 1993], models based on macropores characteristics [Leonard et al., 2004], or other alternative models [Franz et al., 2011].
In the open areas, the degree of preferential flow decreased with increasing size of the open area (i.e., with increasing distance to the nearest tree stem), while infiltrability remained unaffected. Moreover, preferential flow was more variable among small open areas than among large ones. It is reasonable that in small open areas, where tree influence is higher, the soil's physical properties are more spatially variable, with more macropores occurring at points where tree roots can reach and fewer in locations where there are no roots. On the other hand, in large open areas, tree influence is likely minimal. Soil properties are therefore more spatially uniform in these areas and uniform flow dominates the infiltration process because of fewer available preferential pathways. The influence of trees on soil hydraulic properties in drylands is usually studied by assessing differences between two distinct zones: beneath tree canopies and in the open areas. However, our results show that in the open areas preferential flow changes with distance to the nearest tree stem, indicating that a simple comparison between canopy and intercanopy zones is not adequate to study the spatial influence of trees on soil hydraulic properties. Similar to our results, Dunkerley [2000] showed that infiltration rates decreased gradually with increasing distance from the stem of shrubs in dryland Australia and defined a shrub influence zone which was 3.3 times larger than that of the projected canopy area. Lyford and Qashu [1969] also reported changes in soil infiltrability with distance from shrubs in Arizona. In our case, we did not see changes in soil infiltrability with distance from the nearest tree stem within the open areas. This might be due to the fact that in our study area there is a sharp decrease of soil infiltrability with distance from the tree stem, which would mean that we would not have been able to capture any changes using the present experimental design. In further studies, radial transects from the tree stem, with more sampling points per transect, may be more suitable to analyze the influence of trees on soil infiltrability in our study site. Changes in both infiltrability and preferential flow with distance from trees and shrubs in drylands need to be further studied. Further knowledge about the spatial variation in these two properties can contribute to improve the self-organization models of vegetation pattern formation in drylands based on the symmetry-breaking instability concept [Barbier et al., 2008; Borgogno et al., 2009; Franz et al., 2012]. These models explain the emergence of vegetation patterns as a result of coexisting short-range facilitation interactions and long-range competitive interactions between plants. Facilitative effects, such as higher infiltration, are usually believed to occur within the range of the crown area, while competitive effects extend further away, over the root zone [Borgogno et al., 2009]. Our results, however, show that the effect of trees on preferential flow extends beyond the canopy edge, indicating thus that the range of facilitative effects might be larger than usually thought.
The combined increase in preferential flow and infiltrability observed in the soil beneath trees can have important implications for groundwater recharge. In general, trees can have negative and positive effects on groundwater recharge, the negative being increased interception of rainfall and transpiration and the positive being increased stemflow, infiltration, and preferential flow, and reduced soil evaporation [Le Maitre et al., 1999]. Another effect of trees on groundwater recharge might be through enhanced or decreased soil moisture. If soil moisture is enhanced, water flux through the vadose zone would increase. Hence, the net effect of tree cover on groundwater recharge will be the result of a balance between each of these effects. While it is well known that trees can transpire large quantities of water and that in dry environments they can have deep roots that allow them to tap water from deep layers [Stone and Kalisz, 1991], thus negatively impacting groundwater recharge, our results indicate that such losses could be counteracted by the increased soil infiltrability and preferential flow derived from trees. Larger infiltrability rates under trees compared to open areas can result in more water being accumulated and subsequently infiltrated around trees. Moreover, the existence of preferential flow under trees means that a large fraction of this infiltrated water will bypass the surface soil matrix and penetrate the soil rapidly, therefore reducing the time for water to percolate to deep layers and also reducing evaporation losses from the topsoil layers, which together will lead to enhanced deep drainage and potentially groundwater recharge. High recharge rates in other dryland systems have been linked to occurrence of preferential flow [Allison and Hughes, 1983; Johnston, ,; Mathieu and Bariac, 1996].
Our study suggests that scattered trees in dryland landscapes can improve soil infiltration and preferential flow. Therefore trees may function as water harvesters contributing to deeper drainage and recharge. In contrast, open areas function as sources of surface runoff and infiltrating water flows down in the soil more slowly, which should result in higher evaporation losses. This has important implications for the role of trees in tropical dryland landscapes: (a) trees may be less detrimental to groundwater resources, or may even have a positive effect, than has been previously suggested; (b) the degree of tree cover and the spatial distribution of trees in the landscape affect soil hydraulic properties. These two variables have been neglected when assessing the effects of changes in tree cover on water resources in drylands. Therefore, the predominant bias toward studies where only closed forest and open land are considered is potentially misleading for policy and management decisions. The overall effect of tree planting on water resources in drylands will be the result of a balance between their negative and positive effects, the contributions of which are strongly dependant on tree density. Tree density is thus a key variable when addressing the role of trees on water in drylands.
Acknowledgments
The project is funded by the Swedish International Development Cooperation Agency (Sida). The authors are grateful to Julien Belem, Nazaire Belem, Somaila Kabore, and Léonard Ouedraogo for fieldwork assistance. We would like to thank villagers and landowners in the village of Saponé for their permission to carry out the fieldwork. We are also thankful to Hugues Roméo Bazié and Josias Sanou from INERA for logistic assistance during fieldwork. Thanks to Kristi Kuljus and Bo Ranneby from the Centre of Biostochastics at SLU for their help with the statistical analysis. The data for this paper can be made available by request to the corresponding author.
Supporting Information
Supporting Information:
Read Me
Text file 1
Figures S1
Figures S2
References
- Allison GB. Hughes MW. The use of natural tracers as indicators of soil-water movement in a temperate semi-arid region. J. Hydrol. 1983;60(1–4):157–173. doi:10.1016/0022-1694(83)90019-7. [Google Scholar]
- Andreassian V. Waters and forests: From historical controversy to scientific debate. J. Hydrol. 2004;291(1–2):1–27. doi:10.1016/j.jhydrol.2003.12.015. [Google Scholar]
- Barbier N, Couteron P, Lefever R, Deblauwe V. Lejeune O. Spatial decoupling of facilitation and competition at the origin of gapped vegetation patterns. Ecology. 2008;89(6):1521–1531. doi: 10.1890/07-0365.1. doi:10.1890/07-0365.1. [DOI] [PubMed] [Google Scholar]
- Bayala J. 2002. Tree crown pruning as a management tool to enhance the productivity of parklands in West Africa, PhD thesis, Sch. of Agric. and For. Sci., Univ. of Wales, Bangor, U. K.
- Bayala J, Balesdent J, Marol C, Zapata F, Teklehaimanot Z. Ouedraogo SJ. Relative contribution of trees and crops to soil carbon content in a parkland system in Burkina Faso using variations in natural C-13 abundance. Nutr. Cycling Agroecosyst. 2006;76(2–3):193–201. doi:10.1007/s10705-005-1547-1. [Google Scholar]
- Belsky AJ, Mwonga SM, Amundson RG, Duxbury JM. Ali AR. Comparative effects of isolated trees on their undercanopy environments in high- and low-rainfall savannas. J. Appl. Ecol. 1993;30(1):143–155. doi:10.2307/2404278. [Google Scholar]
- Beven K. Germann P. Macropores and water flow in soils revisited. Water Resour. Res. 2013;49:3071–3092. doi:10.1002/wrcr.20156. [Google Scholar]
- Bodart C, Brink AB, Donnay F, Lupi A, Mayaux P. Achard F. Continental estimates of forest cover and forest cover changes in the dry ecosystems of Africa between 1990 and 2000. J. Biogeogr. 2013;40(6):1036–1047. doi: 10.1111/jbi.12084. doi:10.1111/jbi.12084. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Boffa JM. Agroforestry Parklands in Sub-Saharan Africa. Food and Agric. Organ. 1999 Rome. [Google Scholar]
- Bonkoungou E, Alexandre D, Ayuk E, Depommier D, Morant P. Ouadba J. Agroforestry parklands of the West African semi-arid lands, conclusions and recommendations of an international symposium. Ouagadougou. 1994:25–27. October, 1993, report, 18 pp., ICRAF/SALWA, Nairobi. [Google Scholar]
- Borgogno F, D'Odorico P, Laio F. Ridolfi L. Mathematical models of vegetation pattern formation in ecohydrology. Rev. Geophys. 2009;47:RG1005. doi:10.1029/2007RG000256. [Google Scholar]
- Bouma J. Influence of soil macroporosity on environmental quality. Adv. Agron. 1991;46:1–37. doi:10.1016/s0065-2113(08)60577-5. [Google Scholar]
- Bowyer-Bower TAS. Burt TP. Rainfall simulators for investigating soil response to rainfall. Soil Technol. 1989;2(1):1–16. doi:10.1016/s0933-3630(89)80002-9. [Google Scholar]
- Breman H. Kessler JJ. Woody Plants in Agro-Ecosystems of Semi-arid Regions: With an Emphasis on the Sahelian Countries. Berlin: Springer; 1995. [Google Scholar]
- Bromley J, Brouwer J, Barker AP, Gaze SR. Valentin C. The role of surface water redistribution in an area of patterned vegetation in a semi-arid environment, south-west Niger. J. Hydrol. 1997;198(1–4):1–29. doi:10.1016/s0022-1694(96)03322-7. [Google Scholar]
- Bruijnzeel LA. Hydrological functions of tropical forests: Not seeing the soil for the trees? Agric. Ecosyst. Environ. 2004;104(1):185–228. doi:10.1016/j.agee.2004.01.015. [Google Scholar]
- Chidumayo EN. Gumbo DJ. The Dry Forests and Woodlands of Africa: Managing for Products and Services. London: Earthscan; 2010. p. 288. [Google Scholar]
- CPCS. Classification des sols. France: Grignon; 1967. p. 96. [Google Scholar]
- Darlington J. Termite nest structure and impact on the soil at the radar site, Embakasi, Kenya (Isoptera: Termitidae) Sociobiology. 2005;45(3):521–542. [Google Scholar]
- Devitt DA. Smith SD. Root channel macropores enhance downward movement of water in a Mojave Desert ecosystem. J. Arid Environ. 2002;50(1):99–108. doi:10.1006/jare.2001.0853. [Google Scholar]
- Dunkerley D. Hydrologic effects of dryland shrubs: Defining the spatial extent of modified soil water uptake rates at an Australian desert site. J. Arid Environ. 2000;45(2):159–172. doi:10.1006/jare.2000.0636. [Google Scholar]
- Dunn RR. Isolated trees as foci of diversity in active and fallow fields. Biol. Conserv. 2000;95(3):317–321. doi:10.1016/S0006-3207(00)00025-2. [Google Scholar]
- El-Swaify SA, Walker TS. Virmani SM. 1984. and Dryland management alternatives and research needs for Alfisols in the semi-arid tropics: An interpretative summary of the Consultant's Workshop on the State of the Art and Management Alternatives for Optimizing the Productivity of SAT Alfisols and Related Soils, ICRISAT Center, 1-3 December 1983, report, 38 pp., ICRISAT, India.
- Eldridge DJ. Nests of ants and termites influence infiltration in a semiarid woodland. Pedobiologia. 1994;38(6):481–492. [Google Scholar]
- Eldridge DJ. Freudenberger D. Ecosystem wicks: Woodland trees enhance water infiltration in a fragmented agricultural landscape in eastern Australia. Austral Ecol. 2005;30(3):336–347. doi:10.1111/j.1442-9993.2005.01478.x. [Google Scholar]
- Elkins NZ, Sabol GV, Ward TJ. Whitford WG. The influence of subterranean termites on the hydrlogcal characteristics of a Chihuauan desert ecosystem. Oecologia. 1986;68(4):521–528. doi: 10.1007/BF00378766. doi:10.1007/bf00378766. [DOI] [PubMed] [Google Scholar]
- Falkenmark M, Lundqvist J. Widstrand C. Macro-scale water scarcity requires micro-scale approaches-aspects of vulnerability in semi-arid development. Nat. Resour. Forum. 1989;13(4):258–267. doi: 10.1111/j.1477-8947.1989.tb00348.x. [DOI] [PubMed] [Google Scholar]
- FAO-ISRIC-ISSS. 1998. World Reference Base for Soil Resources, vii + 88pp., Rome.
- Farley KA, Jobbagy EG. Jackson RB. Effects of afforestation on water yield: A global synthesis with implications for policy. Global Change Biol. 2005;11(10):1565–1576. doi:10.1111/j.1365-2486.2005.01011.x. [Google Scholar]
- Flury M. Fluhler H. Tracer characteristics of Brilliant Blue FCF. Soil Sci. Soc. Am. J. 1995;59(1):22–27. [Google Scholar]
- Flury M, Fluhler H, Jury WA. Leuenberger J. Susceptibility of soils to preferential flow of water—A field study. Water Resour. Res. 1994;30(7):1945–1954. doi:10.1029/94WR00871. [Google Scholar]
- Forrer I, Papritz A, Kasteel R, Fluhler H. Luca D. Quantifying dye tracers in soil profiles by image processing. Eur. J. Soil Sci. 2000;51(2):313–322. doi:10.1046/j.1365-2389.2000.00315.x. [Google Scholar]
- Fox-Dobbs K, Doak DF, Brody AK. Palmer TM. Termites create spatial structure and govern ecosystem function by affecting N-2 fixation in an East African savanna. Ecology. 2010;91(5):1296–1307. doi: 10.1890/09-0653.1. doi:10.1890/09-0653.1. [DOI] [PubMed] [Google Scholar]
- Franz TE, Nolan J, Nordbotten JM, Caylor KK. Slater LD. Quantifying transient soil moisture dynamics using multipoint direct-current resistivity in homogeneous sand. Vadose Zone J. 2011;10(1):284–290. doi:10.2136/vzj2010.0031. [Google Scholar]
- Franz TE, Caylor KK, King EG, Nordbotten JM, Celia MA. Rodriguez-Iturbe I. An ecohydrological approach to predicting hillslope-scale vegetation patterns in dryland ecosystems. Water Resour. Res. 2012;48:W01515. doi:10.1029/2011WR010524. [Google Scholar]
- Gaze SR, Simmonds LP, Brouwer J. Bouma J. Measurement of surface redistribution of rainfall and modelling its effect on water balance calculations for a millet field on sandy soil in Niger. J. Hydrol. 1997;188–189:267–284. doi:10.1016/S0022-1694(96)03162-9. [Google Scholar]
- Gerke HH. Vangenuchten MT. A dual-porosity model for simulating the preferential movement of water and solutes in structured porous-media. Water Resour. Res. 1993;29(2):305–319. doi:10.1029/92WR02339. [Google Scholar]
- German-Heins J. Flury M. Sorption of Brilliant Blue FCF in soils as affected by pH and ionic strength. Geoderma. 2000;97(1–2):87–101. doi:10.1016/s0016-7061(00)00027-6. [Google Scholar]
- Greene RSB. Soil physical properties of three geomorphic zones in a semiarid mulga woodland. Aust. J. Soil Res. 1992;30(1):55–69. doi:10.1071/SR9920055. [Google Scholar]
- Hatano R. Booltink HWG. Using fractal dimensions of stained flow patterns in a clay soil to predict bypass flow. J. Hydrol. 1992;135(1–4):121–131. doi:10.1016/0022-1694(92)90084-9. [Google Scholar]
- Hatano R, Kawamura N, Ikeda J. Sakuma T. Evaluation of the effect of morphological features of flow paths on solute transport by using fractal dimensions of methylene-blue staining pattern. Geoderma. 1992;53(1–2):31–44. doi:10.1016/0016-7061(92)90019-4. [Google Scholar]
- Hayward B. From the Mountain to the Tap: How Land Use and Water Management Can Work for the Rural Poor. Hayle, U. K: FRP-DFID; 2005. [Google Scholar]
- Hendrickx JMH. Flury M. Conceptual Models of Flow and Transport in the Fractured Vadose Zone. Washington, D. C: Natl. Acad. Press; 2001. Uniform and preferential flow mechanisms in the vadose zone; pp. 149–187. [Google Scholar]
- Hillel D. Introduction to Environmental Soil Physics. Calif: Academic, San Diego; 2004. p. 494. xvi + . [Google Scholar]
- Hole FD. Effects of animals on soil. Geoderma. 1981;25(1–2):75–112. doi:10.1016/0016-7061(81)90008-2. [Google Scholar]
- Hoogmoed WB. Stroosnijder L. Crust formation on sandy soils in the Sahel. 1. Rainfall and infiltration. Soil Tillage Res. 1984;4(1):5–23. doi:10.1016/0167-1987(84)90013-8. [Google Scholar]
- Hoogmoed WB. Stroosnijder L. Evaluation des risques d'érosion sur un bassin versant dégradé utilisant le SIG et la simulation: Le cas de Silmiougou (région de Kaya du Burkina Faso) In: Roose E, editor; De Noni G, Lamachère JM, editors. Etats de Surface du Sol et Risques de Ruissellement et D'érosion. Vol. 12. Paris: Journées du Réseau Erosion; 1996. pp. 391–401. [Google Scholar]
- Ilstedt U, Malmer A, Elke V. Murdiyarso D. The effect of afforestation on water infiltration in the tropics: A systematic review and meta-analysis. For. Ecol. Manage. 2007;251(1–2):45–51. doi:10.1016/j.foreco.2007.06.014. [Google Scholar]
- Islam K. Weil R. Land use effects on soil quality in a tropical forest ecosystem of Bangladesh. Agric. Ecosyst. Environ. 2000;79(1):9–16. doi:10.1016/S0167-8809(99)00145-0. [Google Scholar]
- Jackson RB, Jobbagy EG, Avissar R, Roy SB, Barrett DJ, Cook CW, Farley KA, le Maitre DC, McCarl BA. Murray BC. Trading water for carbon with biological carbon sequestration. Science. 2005;310(5756):1944–1947. doi: 10.1126/science.1119282. doi:10.1126/science.1119282. [DOI] [PubMed] [Google Scholar]
- Janeau JL. Valentin C. Relations entre les termitières Trinervitermes sp. et la surface du sol: Réorganisations, ruissellement et érosion. Rev. Ecol. Soil Biol. 1987;24(4):637–647. [Google Scholar]
- Jarvis NJ. A review of non-equilibrium water flow and solute transport in soil macropores: Principles, controlling factors and consequences for water quality. Eur. J. Soil Sci. 2007;58(3):523–546. doi:10.1111/j.1365-2389.2007.00915.x. [Google Scholar]
- Jindal R, Swallow B. Kerr J. Forestry-based carbon sequestration projects in Africa: Potential benefits and challenges. Nat. Resour. Forum. 2008;32(2):116–130. doi:10.1111/j.1477-8947.2008.00176.x. [Google Scholar]
- Joelsson KH. 2012. ), Soil organic carbon and infiltrability in relation to distance from trees (Vitellaria paradoxa) with and without termite mounds in a parkland of central Burkina Faso, MS thesis, SLU, Umeå, Sweden.
- Johnston CD. Preferred water-flow and localized recharge in a variable regolith. J. Hydrol. 1987a;94(1–2):67–88. doi:10.1016/0022-1694(87)90036-9. [Google Scholar]
- Johnston CD. Distribution of environmental chloride in relation to subsurface hydrology. J. Hydrol. 1987b;94(1–2):67–88. doi:10.1016/0022-1694(87)90033-3. [Google Scholar]
- Jonsson K, Ong CK. Odongo JCW. Influence of scattered nere and karite trees on microclimate, soil fertility and millet yield in Burkina Faso. Exp. Agric. 1999;35(1):39–53. doi:10.1017/s0014479799001039. [Google Scholar]
- Le Maitre DC, Scott DF. Colvin C. A review of information on interactions between vegetation and groundwater. Water SA. 1999;25(2):137–152. [Google Scholar]
- Lejeune O, Tlidi M. Lefever R. Vegetation spots and stripes: Dissipative structures in arid landscapes. Int. J. Quantum Chem. 2004;98(2):261–271. doi:10.1002/qua.10878. [Google Scholar]
- Leonard J, Perrier E. Rajot JL. Biological macropores effect on runoff and infiltration: A combined experimental and modelling approach. Agric. Ecosyst. Environ. 2004;104(2):277–285. doi:10.1016/j.agee.2003.11.015. [Google Scholar]
- Lillesand TM, Kiefer RW. Chipman JW. Remote Sensing and Image Interpretation. xii. Chichester, U. K: John Wiley; 2008. p. 756. + . [Google Scholar]
- Lobry de Bruyn LA. Conacher AJ. The role of termites and ants in soil modification: A review. Aust. J. Soil Res. 1990;28(1):55–93. [Google Scholar]
- Ludwig JA, Bastin GN, Chewings VH, Eager RW. Liedloff AC. Leakiness: A new index for monitoring the health of arid and semiarid landscapes using remotely sensed vegetation cover and elevation data. Ecol. Indicators. 2007;7(2):442–454. doi:10.1016/j.ecolind.2006.05.001. [Google Scholar]
- Lyford FP. Qashu HK. Infiltration rates as affected by desert vegetation. Water Resour. Res. 1969;5(6):1373–1376. doi:10.1029/WR005i006p01373. [Google Scholar]
- Malmer A, Murdiyarso D, Bruijnzeel LA. Ilstedt U. Carbon sequestration in tropical forests and water: A critical look at the basis for commonly used generalizations. Global Change Biol. 2010;16(2):599–604. doi:10.1111/j.1365-2486.2009.01984.x. [Google Scholar]
- Mando A, Stroosnijder L. Brussaard L. Effects of termites on infiltration into crusted soil. Geoderma. 1996;74(1–2):107–113. doi:10.1016/S0016-7061(96)00058-4. [Google Scholar]
- Mathieu R. Bariac T. An isotopic study (H-2 and O-18) of water movements in clayey soils under a semiarid climate. Water Resour. Res. 1996;32(4):779–789. doi:10.1029/96WR00074. [Google Scholar]
- Minnemeyer S, Laestadius L, Sizler N, Saint-Laurent C. Potapov P. A World of Opportunity, Washington, D. C: World Res. Inst., IUCN; 2011. p. 5. [Google Scholar]
- Mordelet P, Abbadie L. Menaut JC. Effects of tree clumps on soil characteristics in a humid savanna of West-Africa (Lamoto, Cote-d'Ivoire) Plant Soil. 1993;153(1):103–111. doi:10.1007/BF00010549. [Google Scholar]
- Nyberg G, Tobella AB, Kinyangi J. Ilstedt U. Soil property changes over a 120-yr chronosequence from forest to agriculture in western Kenya. Hydrol. Earth Syst. Sci. 2012;16(7):2085–2094. doi:10.5194/hess-16-2085-2012. [Google Scholar]
- Oldeman L. Symposium on Soil Resilience and Sustainable Land Use, the 2nd Workshop on the Ecological Foundations of Sustainable Agriculture (WEFSA II) CAB Int., Budapest; 1994. The global extent of soil degradation. [Google Scholar]
- Philip JR. The theory of infiltration: 4. Sorptivity and algebraic infiltration equations. Soil Sci. 1957;84(3):257–264. [Google Scholar]
- R Core Team. R: A Language and Environment for Statistical Computing. Vienna: R Found. for Stat. Comput; 2013. [Google Scholar]
- Reid KD, Wilcox BP, Breshears DD. MacDonald L. Runoff and erosion in a pinon-juniper woodland: Influence of vegetation patches. Soil Sci. Soc. Am. J. 1999;63(6):1869–1879. [Google Scholar]
- Safriel U, Adeel Z, Niemeijer D, Puigdefabregas J, White R, Lal R, Winslow M, Ziedler J, Prince S. Archer E. Dryland systems. In: Ash N, editor; Hassan R, Scholes R, editors. Ecosystems and Human Well-being: Current State and Trends: Findings of the Condition and Trends Working Group. Washington, D. C: Island Press; 2005. pp. 623–662. [Google Scholar]
- Schlesinger WH, Reynolds JF, Cunningham GL, Huenneke LF, Jarrell WM, Virginia RA. Whitford WG. Biological feedbacks in global desertification. Science. 1990;247(4946):1043–1048. doi: 10.1126/science.247.4946.1043. doi:10.1126/science.247.4946.1043. [DOI] [PubMed] [Google Scholar]
- SEI. 2005. Sustainable pathways to attain the millennium development goals. Assessing the key role of water, energy and sanitation, report, Stockholm Environ. Inst., Stockholm.
- Shvidenko A. Forest and woodland systems. In: Ash N, editor; Hassan R, Scholes R, editors. Ecosystems and Human Well-being: Current State and Trends: Findings of the Condition and Trends Working Group. Washington, D. C: Island Press; 2005. p. 917. [Google Scholar]
- Soil Survey Staff. Keys to Soil Taxonomy. Washington, D. C: USDA-Nat. Resour. Conserv. Serv; 2006. [Google Scholar]
- Stone EL. Kalisz PJ. On the maximum extent of tree roots. For. Ecol. Manage. 1991;46(1):59–102. [Google Scholar]
- United Nations. Comprehensive Assessment of the Freshwater Resources of the World. Switzerland: World Meteorol. Org., Geneva; 1997. p. 33. [Google Scholar]
- van Schaik N. Spatial variability of infiltration patterns related to site characteristics in a semi-arid watershed. Catena. 2009;78(1):36–47. doi:10.1016/j.catena.2009.02.017. [Google Scholar]
- Weiler M. Fluhler H. Inferring flow types from dye patterns in macroporous soils. Geoderma. 2004;120(1–2):137–153. doi:10.1016/j.geoderma.2003.08.014. [Google Scholar]
- Wilcox BP, Breshears DD. Turin HJ. Hydraulic conductivity in a Piñon-Juniper Woodland. Soil Sci. Soc. Am. J. 2003;67(4):1243–1249. doi:10.2136/sssaj2003.1243. [Google Scholar]
- Yunusa IAM, Mele PM, Rab MA, Schefe CR. Beverly CR. Priming of soil structural and hydrological properties by native woody species, annual crops, and a permanent pasture. Aust. J. Soil Res. 2002;40(2):207–219. doi:10.1071/sr01038. [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Read Me
Text file 1
Figures S1
Figures S2