Abstract
Aims
Class III and IV drugs affect cardiac human ether-a-go-go related gene (IKr) and L-type calcium (ICaL) channels, resulting in complex alterations in repolarization with both anti- and pro-arrhythmic consequences. Interpretation of their effects on cellular and electrocardiogram (ECG)-based biomarkers for risk stratification is challenging. As pharmaceutical compounds often exhibit multiple ion channel effects, our goal is to investigate the simultaneous effect of ICaL and IKr block on human ventricular electrophysiology from ionic to ECG level.
Methods and results
Simulations are conducted using a human body torso bidomain model, which includes realistic representation of human membrane kinetics, anatomy, and fibre orientation. A simple block pore model is incorporated to simulate drug-induced ICaL and IKr blocks, for drug dose = 0, IC50, 2× IC50, 10× IC50, and 30× IC50. Drug effects on human ventricular activity are quantified for different degrees and combinations of ICaL and IKr blocks from the ionic to the body surface ECG level. Electrocardiogram simulations show that ICaL block results in shortening of the QT interval, ST elevation, and reduced T-wave amplitude, caused by reduction in action potential duration and action potential amplitude during the plateau phase, and in repolarization times. In contrast, IKr block results in QT prolongation and reduced T-wave amplitude. When ICaL and IKr blocks are combined, the degree of ICaL block strongly determines QT interval whereas the effect of IKr block is more pronounced on the T-wave amplitude.
Conclusion
Our simulation study provides new insights into the combined effect of ICaL and IKr blocks on human ventricular activity using a multiscale computational human torso model.
Keywords: hERG block, L-type calcium block, QT interval, ECG modelling, Computer simulation, Dispersion of repolarization
What's new?
A mathematical model of the human cardiac ventricles embedded in a torso is used to simulate the effect of multiple ion channel block on the electrical activity of the heart from ion channel to electrocardiogram.
L-type calcium block results in QT interval shortening, ST elevation, and T-wave amplitude reduction, caused by decrease in action potential duration and action potential amplitude and in dispersion of repolarization.
When L-type calcium current (ICaL) and rapidly activating potassium current (IKr) blocks are simultaneously applied, QT interval is more sensitive to the degree of ICaL block whereas T-wave amplitude is mostly determined by IKr block.
Introduction
Calcium (Ca2+) is the ionic link in cardiac excitation–contraction coupling, which, in cardiac cells, starts following the upstroke of the action potential (AP) and causes the opening of the L-type voltage-dependent Ca2+ channels. These ion channels are expressed by the CACNA1C gene. L-type Ca2+ channels constitute one of the most important Ca2+ entry pathways into the cell and have been classified by their sensitivity to dihydropyridine-based compounds (e.g. nifedipine). Different compounds have been developed to target calcium channels and in particular L-type calcium channels. The compounds aim to modulate the cardiac ventricular L-type Ca2+ current (ICaL). These agents have been classified to three main classes: phenylalkylamines (e.g. verapamil), benzothiazepines (e.g. diltiazem), and dihydropyridines (e.g. nifedipine).1 Calcium channel blockers have wide clinical applicability and they are used to decrease blood pressure in patients with hypertension. Calcium channel blockers are also frequently used to alter heart rate, to prevent cerebral vasospasm, and to reduce chest pain caused by angina pectoris. However, it has been shown that they could increase the mortality rate in elderly patients, and have been known to have multiple side effects.2,3 They have also been associated with a risk of cancer.4 Abnormalities in ICaL, in particular, have also been linked to ventricular arrhythmias, impaired excitation–contraction coupling leading to heart failure, as well as atrial fibrillation.5 These abnormalities could be related to cardiac diseases or to drug-induced side effects. In both cases, they result in ionic currents abnormalities, which cause alterations in both ventricular depolarization and repolarization properties.
Of particular concern are changes in ventricular repolarization caused by combined ICaL and rapidly activating potassium current (IKr) alterations, which can manifest themselves as changes in the QT interval and T-wave of the electrocardiogram (ECG), and have been linked to increased risk of arrhythmic death. QT prolongation is considered an indicator of increased risk of torsades de pointes and can lead to the abandonment of the compound development. However, some QT prolonging drugs present no arrhythmic episodes and some others are known to be useful antiarrhythmic drugs for most patients. Interpretation of drug-induced effects on the ECG is however challenging due to the variability of cardiac substrates and also to the frequent multichannel action of most compounds.6–9 Therefore, a better understanding of drug-induced changes in the ECG and how they relate to ionic mechanisms and arrhythmic risk is needed.
Recent reviews have highlighted how computational modelling and simulation can help in the investigation of arrhythmias and antiarrhythmic therapy.10–13 Recent studies have specifically shown the usefulness of whole-ventricular computer simulations to investigate drug effects on the electrical activity of the heart.9,14–16 In this study, we aim at using a multiscale computational human torso model to investigate and quantify the combined effect of ICaL and IKr block on human ventricular activity from the ionic to the ECG level.
Methods
Human multiscale torso–heart model
A human model of the cardiac ventricles embedded in a torso is used and illustrated in Figure 1A and B. The human ventricular model includes realistic representation of human ventricular kinetics, anatomy, and fibre orientation as previously described in Zemzemi et al.15 Briefly, the anatomical model is based on data presented in Chapelle et al.17 and the computational mesh contains 2 401 151 vertices and 14 336 528 tetrahedral elements.15 The Ten Tusscher and Panfilov human AP model18 is used to represent human membrane kinetics throughout the myocardium, including epicardial, endocardial, and mid-myocardial ionic properties based on transmural location. Therefore, transmural heterogeneity in the human AP was introduced in our human ventricular model, in agreement with previous studies such as Okada et al.19
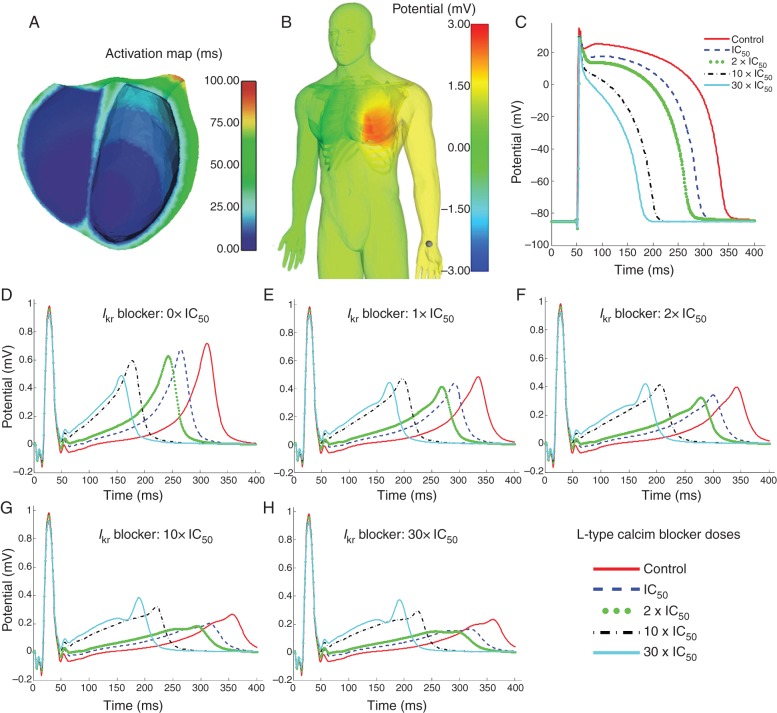
Figure 1.
(A) A cross-section of the human ventricles showing the activation times map transmurally and on the endocardium. (B) Distribution of the body surface potential (right) for the IC50 ICaL block dose in a snapshot taken during the repolarization phase. (C) Simulated effect of different doses (control, 1× IC50, 2× IC50, 10× IC50, 30× IC50) of ICaL blocker on a representative ventricular AP at a point located at the base of the ventricles. X-axis time (ms). Y-axis potential (mV). (D–H) Simulated effect of combined ICaL and IKr blockers on the second lead of the ECG. Drug dose is 0× IC50, 1× IC50, 2× IC50, 10× IC50, and 30× IC50 for IKr blocker in Panels D–H, respectively, and in each panel a different dose of ICaL block is shown with a different colour as shown in the legend. X-axis shows time in ms, Y-axis potential in mV
The human ventricular model is coupled to the diffusion equation in the torso to take into account the propagation of the electrical wave from heart to torso to compute the ECGs on the body surface.15,20
To simulate sinus rhythm activation, a time-dependent stimulus of 0.5 ms duration and 100 mA cm−3 strength is applied to the endocardium. To mimic Purkinje network activation, the endocardium surface is then progressively activated from apex to base to produce a simulated activation sequence illustrated in Figure 1A, in line with the experimental results by Durrer et al.21
Drug/ion channel interaction model
The human AP model18 was modified to enable simulation of drug action on ICaL and IKr using the simple pore block model7,15,22. The degree of channel block is a function of drug concentration and the drug half maximal inhibitory concentration (IC50) value for the targeted ionic channel. Thus, for a given drug dose [D] and IC50 value with respect to the channel j the formulation of drug action on the ionic current conductance gj is given by:
with Hill coefficient n = 1.
Computational methods and data analysis
All the simulations in this paper have been conducted using the software CHASTE (Cancer Heart and Soft Tissue Environment).23,24 Details of models and numerical methods are as described in the supplementary material in Zemzemi et al.15 Simulations were run in the Bordeaux federative platform for research in computer sciences and mathematics called PLAFRIM (https://plafrim.bordeaux.inria.fr/).
We compute the standard 12-lead ECG by extracting the electrical potential values at the standard 9 electrode locations on the body surface of the human torso model.15 A snapshot of the distribution of the electrical potential on the body surface from which the ECG is obtained is represented in Figure 1B. In the results of this study, all the ECG-based biomarkers are quantified for the second Einthoven lead of the ECG. The QT interval is computed as the time interval between the Q- and the T-wave peak of the ECG. At each ventricular node, the the action potential duration at 90% (APD90) is computed as the time interval between the depolarization time and the time at which the transmembrane potential is 90% repolarized. Electrophysiological alterations caused by ionic current block are also described by analysing APD90 and AP morphology in one selected node located at the base of the left ventricle. Previous studies have linked changes in T-wave amplitude with the Wilson ventricular gradient, which is the gradient of the transmembrane potential in the ventricles.25,26 As in references,27–29 we therefore quantified the Wilson ventricular gradient by computing the spatial dispersion of ventricular repolarization. To do so, the spatial dispersion of transmembrane potential throughout the human ventricles is calculated at each time step during the simulations, as the voltage difference between the maximal and minimal values of the transmembrane potential throughout the ventricles at each time step. Its maximum value over time during the repolarization phase is quantified and referred to in the paper as the maximum (spatial) dispersion of transmembrane potential, for each simulation.
Results
The human whole-ventricular model embedded in the torso (Figure 1A and B) was used to simulate the effect of ICaL and IKr blocks on ventricular electrophysiology and the ECG. Figure 1C shows the simulated effect of ICaL block on the AP for blocker dose [D] increasing from zero (control) to [D] = IC50, 2× IC50, 10× IC50, and 30× IC50 (corresponding to 50, 66, 90, and 96% of ICaL block, respectively). Increasing ICaL blocker dose results in decrease in AP amplitude and shortening of APD90. Indeed APD90 shortens from 290 ms in control to 242, 219, 150, and 126 ms for ICaL block dose of [D] = IC50, 2× IC50, 10× IC50, 30× IC50, respectively. The effect of IKr block was shown in our previous work,15 and as expected, it leads to a progressive prolongation of the APD90 from 290 ms in control conditions to 312 ms for IC50 IKr block, and to 320, 334, and 337 ms) for 2× IC50, 10× IC50, and 30× IC50 IKr block dose, respectively. This corresponds to APD90 prolongation by 7.5, 10, 15, and 16% for IC50, 2× IC50, 10× IC50, and 30× IC50 IKr block dose, respectively.
Drugs often exhibit multichannel action and therefore their effects on the heart are difficult to interpret. This is especially the case, when the ion channels affected by the drug have counteracting effects on cardiac behaviour such as ICaL and IKr, inward and outward currents, respectively, and both important during the plateau and repolarization phases of the human ventricular AP. Therefore, simulations using the human torso model were conducted to investigate the effect of combined ICaL and IKr blocks on the ECG for 25 different dose combinations. Figure 1D–H displays ECG traces obtained for five ICaL block doses, for no IKr block (e.g. control, Figure 1D), and IC50, 2× IC50, 10× IC50, 30× IC50 doses of IKr blocker (Figure 1E–H, respectively).
For all IKr blocker doses, the most notable effects of increasing ICaL block in the ECG are a progressive shortening of the QT interval and simultaneous elevation of the ST segment with increasing dose. Indeed, the QT interval decreases from 315 ms in control to 265 ms (by 15%) for ICaL block dose of IC50, to 240 ms (by 23%) for 2× IC50, to 176 ms (by 44%) for 10× IC50 dose, and to 157 ms (by 57%) for ICaL block dose of 30× IC50. The ECG changes caused by ICaL block in Figure 1D–H, and specifically the QT shortening, are explained by the AP changes shown in Figure 1C, and in particular the decrease in both APD and plateau levels caused by the reduced ICaL as blocker dose is increased. Our simulation results also show that when IKr block is added to ICaL block, QT shortening still occurs but becomes milder due to the APD90 prolongation effects of IKr block. In addition, increasing IKr block results in significant decrease in T-wave peak for all ICaL blocker doses, as shown when comparing Figure 1D–H.
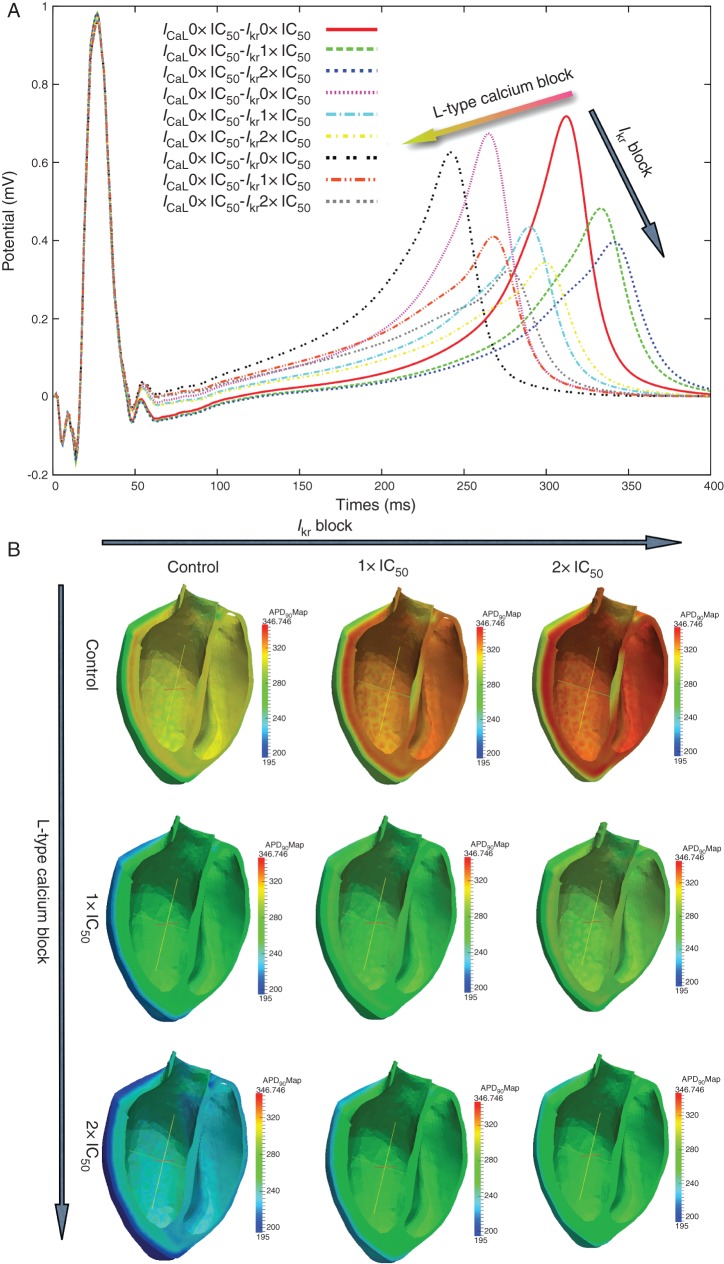
The simulated effects of combined ICaL and IKr blocks on the human ECG are further illustrated and examined in Figure 2. The second lead of the ECG is shown in Figure 2A superimposed for different combinations of IC50 and 2× IC50 block. As previously shown in Figure 1, selective IKr block prolongs the QT whereas selective ICaL block shortens it, in line with their respective effects on the APD of human ventricular myocytes. In both cases, the T-wave amplitude decreases, in a larger amount for IKr than for ICaL block (as shown in Figure 1D–H). Combined IKr and ICaL blocks result in more moderate changes in QT than single channel block due to the counteracting effects of the two current blocks on the APD. Furthermore, Figure 2A shows that IKr block does not affect the QRS interval, whereas ICaL block slightly widens it, due to a very small slowing of the conduction velocity.
Figure 2.
(A) Effect of different combinations of IKr and ICaL blocks doses (0× IC50, 1× IC50, 2× IC50) on the second lead of the ECG. X-axis time (ms). Y-axis potential (mV). (B) Effect of different combinations of IKr (0× IC50, 1× IC50, 2× IC50) from left to right and ICaL (0× IC50, 1× IC50, 2× IC50) from top to bottom on the APD90 distribution. Colour bar in millisecond.
Figure 2B illustrates the simulated effect of simultaneous ICaL and IKr block on the distribution of APD90 values in a transmural cross-section of the human ventricular model used in the simulations. The panels in Figure 2B correspond to increasing ICaL block from top to bottom and to increasing IKr block from left to right. As expected, the simulations show that overall APD90 shortens with increasing ICaL blocker dose, whereas it is prolonged when increasing IKr block dose. Combined block results in moderate APD90 shortening throughout the ventricles, which explains the moderate QT shortening seen in Figure 2A. In all cases, the epicardial surface shows shorter APD values than mid-myocardial and endocardial tissue resulting in transmural dispersion in APD90. The APD maps also show how dispersion in APD90 is modulated by different degrees of ICaL and IKr blocks (Figure 2B).
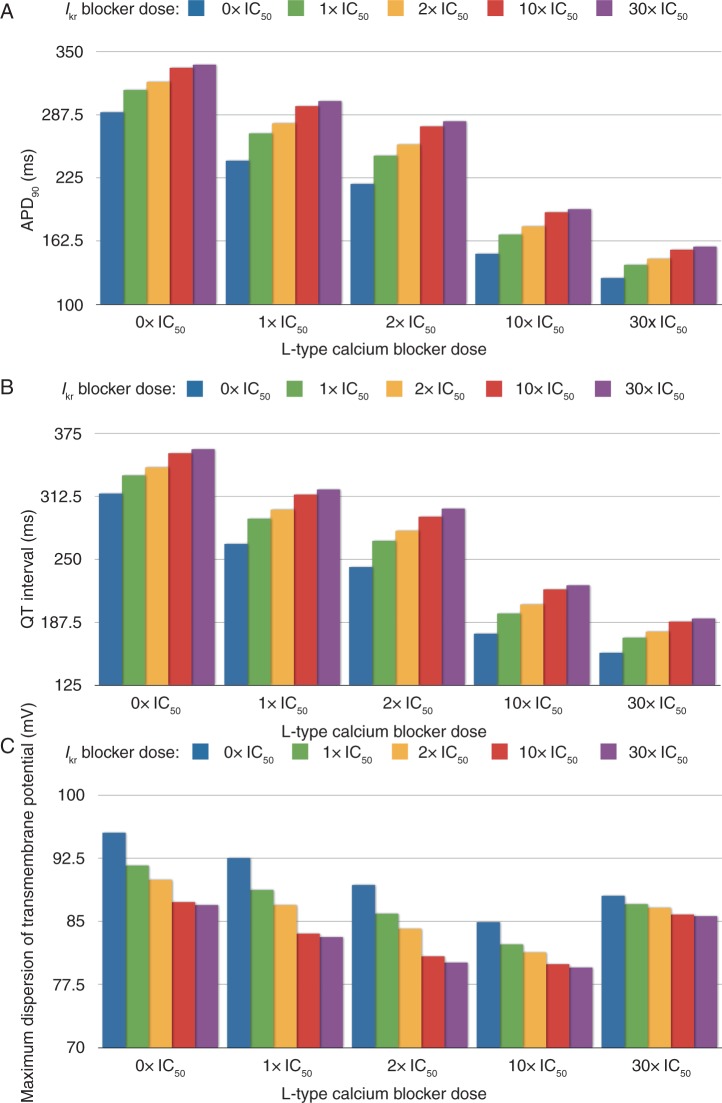
Figure 3 shows a quantification of the APD90 in a selected node at the base of the ventricles (Figure 3A), QT interval (Figure 3B), and maximum spatial dispersion of transmembrane potential (Figure 3C) for the 25 combined doses of ICaL and IKr blocks considered in the simulations. Figure 3A provides further quantification of the fact that combined ICaL and IKr blocks at similar doses (diagonal of Figure 2B) results in APD90 shortening by 23 and 40 ms for IC50 and 2× IC50 doses for both currents, respectively. Simulation results therefore reflect the fact that APD90 using our human model is more sensitive to ICaL block than to IKr block for a similar drug dose with respect to the IC50. This is also reflected in the QT interval, quantified for the 25 simulations in Figure 3B. Therefore, as for APD90, the effect of ICaL block on reducing the QT interval is more pronounced than the prolongation caused by IKr block for the same degree of block.
Figure 3.
Effect of combining doses of L-type calcium and hERG blockers (control, 1× IC50, 2× IC50, 10× IC50, 30× IC50) on the QT interval (units are mV, ms, and ms, respectively) (A), on the APD90 (B), and on the maximum dispersion of the transmembrane potential during the repolarization phase (C). The APD90 is computed from a point located at the base of the left ventricle.
As shown in Figures 1 and 2, the decrease in the T-wave amplitude is more pronounced for IKr than for ICaL block. It decreases from 0.72 mV in control to 0.68 and 0.64 mV for IC50 and 2× IC50 dose of ICaL blocker, whereas it decreases from 0.72 mV to 0.48 and 0.39 mV) for IC50 and 2× IC50 dose of IKr blocker. Alterations in the T-wave amplitude in our simulations are complex and affected by heterogeneous changes in AP morphology and duration. Our results show that they could be explained, at least in part, by alterations in the maximum dispersion of transmembrane potential, which result from the changes in heterogeneity in APD90 illustrated in Figure 2B. As shown in the histograms in Figure 3C, both ICaL and IKr blockers result in maximum spatial dispersion of transmembrane potential below its value in control. The decrease in maximum dispersion caused by IKr block is more pronounced than the decrease caused by ICaL block for similar doses. This is in line with the decrease in T-wave peak shown in the ECG in Figure 1D–H.
Figure 3C also shows that the maximum spatial dispersion of transmembrane potential decreases with increasing ICaL block dose up to 10× IC50. Further increase in ICaL block dose results in a trend inversion: the maximum dispersion of transmembrane potential is higher for 30× IC50 than for 10× IC50 (but still smaller than in control). This could be explained by the fact that for 30× IC50 ICaL block dose, the AP repolarization phase becomes very sharp, as illustrated in Figure 1C. Sharp repolarization phases of the AP result in large spatial gradients in transmembrane potential throughout the human ventricles. As shown in Figure 1E–H, the increased transmembrane potential gradient also results in higher values of the T-wave peak in the ECG, for 30× IC50 than for 10× IC50 ICaL block dose.
Discussion
In this work, we have shown the ability of computer simulations to provide insights into the effect of multiaction ion channel blockers on the electrical activity of the human heart and its reflection on the body surface ECG. This is possible through the use of a multiscale human torso–heart anatomical model with biophysically detailed representation of human ventricular membrane dynamics, fibre orientation, and electrophysiological heterogeneity. Our study extends our previous work showing the effect of potassium and sodium channel block on the ECG.15,16 Here, we focus on investigating the manifestation of the simultaneous block of the inward ICaL and the outward IKr on human whole-ventricular activity, which has opposite effects on cardiac repolarization and therefore presents a challenge in the interpretation of the ECGs.
Our results show that ICaL block results in shortening of the QT interval, reduction of T-wave amplitude, elevation of the ST segment, and slight widening of the QRS complex. The changes in the ECG are explained by the shortening of APD, reduction of AP amplitude during the plateau phase, decrease of maximum dispersion of the transmembrane potential, and a slight reduction in conduction velocity.
Clinical data on the effect of calcium blockers on the ECG have mostly focused on their effect on the atria and the atrioventricular node,30,31 rather than in the human ventricles and the ECG, and often in combination with other drugs. Our simulation results are however in agreement with experimental studies reporting APD and QT shortening caused by verapamil in feline wedge preparations.32 Furthermore, the QT interval shortening and the ST segment elevation in human have also been linked to L-type calcium channel function. Thus, Antzelevitch et al.33 reported that gene mutations mainly in CACNA1C and CACNB2 result in loss of ICaL function and cause a QT shortening and ST elevation, as reported in our simulations. It has also been reported in Proano et al.34 that overdoses of calcium blockers come with abnormalities in T-wave and ST segment. These abnormalities could be seen in our simulation in Figure 1D and E for high doses of L-type calcium block (e.g. 10× IC50 and 30× IC50).
Our theoretical investigation aims at providing new insights, which could be valuable for ECG interpretation. The combined action of ICaL and IKr blocks investigated in our study is particularly interesting as the two currents have opposite effects on cardiac repolarization and therefore lead to complex and counteracting changes in ECG biomarkers with difficult interpretation. Our results show that the main effect of ICaL block on the ECG is QT shortening and slight ST elevation, whereas IKr block results in QT prolongation and T-wave peak reduction. The simultaneous application of IKr and ICaL blocks in doses from IC50 to 30× IC50 block results in overall decrease in APD and QT interval, which means that for a similar IC50/dose relationship, the effect of ICaL block on the APD and QT interval is more pronounced than IKr block in our human model.
Importantly, our simulations show that IKr block effects are more important in determining the T-wave amplitude than ICaL block. Moreover for high doses of IKr block, our study shows that there is a critical dose of ICaL block, in this case 10× IC50, in the regulation of T-wave peak: lower ICaL blocker doses reduce the T-wave amplitude and higher doses, increases the T-wave amplitude. Our results suggest that the maximum spatial dispersion in transmembrane potential during the repolarization phase plays an important role in this modulation, as suggested by results shown in (Figure 3C).
A strong correlation is seen between changes in APD and QT interval caused by both IKr and ICaL blocks and also by their combination. This is mainly due to the fact that both of IKr and ICaL blockers affect the repolarization phase, and have negligible effects during the depolarization of the AP, as shown in our simulations. Therefore, IKr and ICaL blockers also have negligible effects on conduction velocity, activation patterns under sinus rhythm and therefore the QRS complex is not affected (Figures 1 and 2). The QT interval is therefore mostly affected by changes in APD. In the presence of sodium blockers however, as considered in our previous study,15 increasing drug dose results in changes in activation sequence and a prolongation of the QRS and QT interval durations, without any significant effects on the APD. Therefore, the QT interval prolongation caused in the presence of sodium block is not solely correlated with APD.
The human torso–heart model presented in our study could be used to simulate additional drug effects, and our results could be of value to guide the evaluation and interpretation of ECG data obtained following application of Class III and Class IV blockers exhibiting multichannel action.35,36 Future work could also extend our work to include inter-subject variability in anatomy and electrophysiology37,38 and by extending the simple pore block drug model to more comprehensive models of drug/ion channel interactions, especially when arrhythmic mechanisms are investigated.11,22,39 Furthermore, the human whole-ventricular model includes transmural heterogeneities in ionic currents but neglects the potential contribution of apex–base and left-to-right heterogeneities.40,41 The simulation study by Okada et al.19 shows however that the presence of transmural heterogeneities is sufficient to produce ECGs with the right polarity of the T-wave, whereas apex-to-base heterogeneities need to be very large to produce an effect on the ECG. We therefore believe that apex-to-base and left-to-right heterogeneities as measured in the in vivo human ventricles are likely to produce a small modulation of the results presented in this study and could be the focus of future investigations.
Conclusion
In this study, we present a simulation study of the effect of ion channel block on the electrical activity of the heart from drug/ion channel interactions to the ECG. The human bidomain model of the heart embedded in a torso allows for ECG simulations to be conducted with realistic representation of human anatomy and electrophysiological function, and could be used for further investigations including specific drug effects or disease conditions. We show how ICaL block reduces the APD and the AP amplitude during the plateau phase, which, in the ECG, results in ST elevation, QT interval shortening, and slight T-wave amplitude reduction. As drugs often exhibit multiple channel effects, we evaluated the simultaneous effect of ICaL and IKr on human cardiac activity. As expected, ICaL and IKr blocks have opposite effects on the QT interval of the ECG: ICaL reduces the QT interval whereas IKr prolongs it. However, QT interval is more sensitivity to ICaL block, and therefore combined block results in QT shortening. On the contrary, T-wave amplitude is mostly determined by IKr block dose, even though both ICaL and IKr decrease T-wave peak. Our simulations show that modulation of the T-wave peak in most cases reflects changes in the maximum spatial dispersion of transmembrane potential. Finally, our study illustrates how the multiscale nature of our human torso/heart model allows for the assessment of the consequences of drug-induced ionic changes on the AP at the cell level, on the APD distribution throughout the ventricles, activation and repolarization maps at the organ level, and on the ECG at the body surface level. Both the human model and the insights provided by the simulation study could contribute to a better characterization of drug-induced effects on the heart for pre-clinical drug testing and also to unravel the ionic basis of new biomarkers of predicting drugs cardiotoxicity.
Funding
This study was supported financially by the European Commission preDiCT grant (DG-INFSO—224381), a UK Medical Research Council Career Development Award (to B.R.) and a UK Wellcome Trust Senior Fellowship in Basic Biomedical Sciences (to B.R.). Funding to pay the Open Access publication charges for this article was provided by the UK Wellcome Trust Senior Fellowship in Basic Biomedical sciences.
Acknowledgements
The authors thank Drs Philippe Moireau, Miguel Fernandez, and Elsie Phe from INRIA Paris-Rocquencourt for their work on the anatomical models and meshes. We are also grateful to Professors Dominique Chapelle and Jean-Frederic Gerbeau heads of MACS and REO teams, respectively, in INRIA Paris-Rocquencourt for providing us with the meshes.
Conflict of interest: none declared.
References
- 1.Hockerman G, Peterson B, Johnson B, Catterall W. Molecular determinants of drug binding and action on l-type calcium channels. Annu Rev Pharmacol Toxicol. 1997;37:361–96. doi: 10.1146/annurev.pharmtox.37.1.361. [DOI] [PubMed] [Google Scholar]
- 2.Furberg CD, Psaty BM, Meyer JV. Nifedipine: dose-related increase in mortality in patients with coronary heart disease. Circulation. 1995;92:1326–31. doi: 10.1161/01.cir.92.5.1326. [DOI] [PubMed] [Google Scholar]
- 3.Pahor M, Guralnik JM, Corti MC, Foley DJ, Carbonin P, Havlik RJ. Long-term survival and use of antihypertensive medications in older persons. J Am Geriatr Soc. 1995;43:1191–7. doi: 10.1111/j.1532-5415.1995.tb07393.x. [DOI] [PubMed] [Google Scholar]
- 4.Pahor M, Guralnik JM, Ferruci L, Corti MC, Salive ME, Cerhan JR, et al. Calcium-channel blockade and incidence of cancer in aged populations. Lancet. 1996;348:493–9. doi: 10.1016/S0140-6736(96)04277-8. [DOI] [PubMed] [Google Scholar]
- 5.Bodi I, Mikala G, Koch S, Akhter S, Schwartz A. The L-type calcium channel in the heart: the beat goes on. J Clin Invest. 2005;115:3306–17. doi: 10.1172/JCI27167. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 6.Davies MR, Mistry HB, Hussein L, Pollard CE, Valentin JP, Swinton J, et al. An in silico canine cardiac midmyocardial action potential duration model as a tool for early drug safety assessment. Am J Physiol Heart Circ Physiol. 2012;302:1466–80. doi: 10.1152/ajpheart.00808.2011. [DOI] [PubMed] [Google Scholar]
- 7.Mirams GR, Cui Y, Sher A, Fink M, Cooper J, Heath BM, et al. Simulation of multiple ion channel block provides improved early prediction of compounds clinical torsadogenic risk. Cardiovasc Res. 2011;91:53–61. doi: 10.1093/cvr/cvr044. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 8.Rodriguez B, Burrage K, Gavaghan D, Grau V, Kohl P, Noble D. The systems biology approach to drug development: application to toxicity assessment of drug toxicity. Nat Clin Pharmacol Ther. 2010;88:130–4. doi: 10.1038/clpt.2010.95. [DOI] [PubMed] [Google Scholar]
- 9.Wilhelms M, Rombach C, Scholz EP, Doessel O, Seemann G. Impact of amiodarone and cisapride on simulated human ventricular electrophysiology and electrocardiograms. Europace. 2012;14:90–6. doi: 10.1093/europace/eus281. [DOI] [PubMed] [Google Scholar]
- 10.Carusi A, Burrage K, Rodriguez B. Bridging experiments, models and simulations: an integrative approach to validation in computational cardiac electrophysiology. Am J Physiol Heart Circ Physiol. 2012;303:144–55. doi: 10.1152/ajpheart.01151.2011. [DOI] [PubMed] [Google Scholar]
- 11.Moreno JD, Clancy CE. Using computational modelling to predict arrhythmogenesis and antiarrhythmic therapy. Drug Discov Today. 2009;6:71–84. doi: 10.1016/j.ddmod.2010.03.001. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 12.Quinn TA, Kohl P. Combining wet and dry research: experience with model development for cardiac mechano-electric structure-function studies. Cardiovasc Res. 2013;97:601–11. doi: 10.1093/cvr/cvt003. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 13.Trayanova NA. Whole-heart modeling: applications to cardiac electro-physiology and electromechanics. Circ Res. 2011;108:113–28. doi: 10.1161/CIRCRESAHA.110.223610. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 14.Corrias A, Jie X, Romero L, Bishop MJ, Bernabeu M, Pueyo E, et al. Arrhythmic risk biomarkers for the assessment of drug cardiotoxicity: from experiments to computer simulations. Philos Trans R Soc A, Math Phys Eng Sci. 2010;368:3001–25. doi: 10.1098/rsta.2010.0083. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 15.Zemzemi N, Bernabeu M, Saiz J, Cooper J, Pathmanathan P, Mirams GR, et al. Computational assessment of drug-induced effects on the electrocardiogram: from ion channel to body surface potentials. Br J Pharmacol. 2013;168:718–33. doi: 10.1111/j.1476-5381.2012.02200.x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 16.Zemzemi N, Bernabeu MO, Saiz J, Rodriguez B. Simulating drug-induced effects on the heart: from ion channel to body surface electrocardiogram. Functional Imaging and Modeling of the Heart; 2011. pp. 259–66. [Google Scholar]
- 17.Chapelle D, Fernndez MA, Gerbeau JF, Moireau P, Sainte-Marie J, Zemzemi N. Numerical simulation of the electromechanical activity of the heart. Functional Imaging and Modeling of the Heart. 2009:357–65. volume 5528 of Lecture Notes in Computer Science. [Google Scholar]
- 18.Ten Tusscher K, Panfilov AV. Cell model for efficient simulation of wave propagation in human ventricular tissue under normal and pathological conditions. Phys. Med Biol. 2006;51:6141–56. doi: 10.1088/0031-9155/51/23/014. [DOI] [PubMed] [Google Scholar]
- 19.Okada JI, Washio T, Maehara A, Momomura SI, Sugiura S, Hisada T. Transmural and apicobasal gradients in repolarization contribute to T-wave genesis in human surface ECG. Am J Physiol Heart Circ Physiol. 2011;301:200–8. doi: 10.1152/ajpheart.01241.2010. [DOI] [PubMed] [Google Scholar]
- 20.Zemzemi N. 2009. Etude theorique et numerique de l'activite electrique du Coeur: Application aux electrocardiogrammes. DPhil Thesishttp://tel.archives-ouvertes.fr/tel-00470375/en/ [Google Scholar]
- 21.Durrer D, van Dam RT, Freud GE, Janse MJ, Meijler FL, Arzbaecher RC. Total excitation of the isolated human heart. Circulation. 1970;41:899–912. doi: 10.1161/01.cir.41.6.899. [DOI] [PubMed] [Google Scholar]
- 22.Brennan T, Fink M, Rodriguez B. Multiscale modelling of drug-induced effects on cardiac electrophysiological activity. Eur J Pharm Sci. 2009;36:62–77. doi: 10.1016/j.ejps.2008.09.013. [DOI] [PubMed] [Google Scholar]
- 23.Mirams GR, Arthurs CJ, Bernabeu MO, Bordas R, Cooper J, Corrias A, et al. Chaste: an open source C++ library for computational physiology and biology. PLoS Comput Biol. 2013;9:970–1002. doi: 10.1371/journal.pcbi.1002970. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 24.Pitt-Francis J, Pathmanathan P, Bernabeu MO, Bordas R, Cooper J, Fletcher AG, et al. Chaste: a test-driven approach to software development for biological modelling. Comput Phys Commun. 2009;180:2452–71. [Google Scholar]
- 25.Draisma HH, Schalij MJ, van der Wall EE, Swenne CA. Elucidation of the spatial ventricular gradient and its link with dispersion of repolarisation. Heart Rhythm. 2006;3:1092–9. doi: 10.1016/j.hrthm.2006.05.025. [DOI] [PubMed] [Google Scholar]
- 26.Plonsey R. A Contemporary view of the ventricular gradient of Wilson. J Electrocardiol. 1979;12:337–41. doi: 10.1016/s0022-0736(79)80001-1. [DOI] [PubMed] [Google Scholar]
- 27.Antzelevitch C. Transmural dispersion of repolarisation and the T wave. Cardiovasc Res. 2001;50:426–31. doi: 10.1016/s0008-6363(01)00285-1. [DOI] [PubMed] [Google Scholar]
- 28.Behrens S, Li C, Knollmann BC, Michael RF. Dispersion of ventricular repolarisation in the voltage domain. Pacing Clin Electrophysiol. 1998;21:100–7. doi: 10.1111/j.1540-8159.1998.tb01067.x. [DOI] [PubMed] [Google Scholar]
- 29.Yan GX, Antzelevitch C. Long-QT syndrome cellular basis for the normal T wave and the electrocardiographic manifestations of the long-QT syndrome. Circulation. 1998;98:1928–36. doi: 10.1161/01.cir.98.18.1928. [DOI] [PubMed] [Google Scholar]
- 30.Somogyi A, Albrecht M, Kliems G, Schafer K, Eichelbaum M. Pharmacokinetics, bioavailability and ECG response of verapamil in patients with liver cirrhosis. Br J Clin Pharmacol. 1981;12:51–60. doi: 10.1111/j.1365-2125.1981.tb01854.x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 31.Wood MA, Gilligan DM, Brown-Mahoney C, Nematzadeh F, Stambler BS, Ellenbogen KA. Clinical and electrophysiologic effects of calcium channel blockers in patients receiving ibutilide. Am Heart J. 2002;143:176–80. doi: 10.1067/mhj.2002.120156. [DOI] [PubMed] [Google Scholar]
- 32.Aiba T, Shimizu W, Inagaki M, Noda T, Miyoshi S, Wei-Guang D, et al. Cellular and ionic mechanism for drug-induced long QT syndrome and effectiveness of verapamil. J. Am Coll Cardiol. 2005;45:300–7. doi: 10.1016/j.jacc.2004.09.069. [DOI] [PubMed] [Google Scholar]
- 33.Antzelevitch C, Pollevick GD, Cordeiro JM, Casis O, Sanguinetti MC, Aizawa Y, et al. Loss-of-function mutations in the cardiac calcium channel underlie a new clinical entity characterized by ST-segment elevation, short QT intervals, and sudden cardiac death. Circulation. 2007;115:442–9. doi: 10.1161/CIRCULATIONAHA.106.668392. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 34.Proano L, Chiang WK, Wang RY. Calcium channel blocker overdose. Am J Emerg Med. 1995;13:444–50. doi: 10.1016/0735-6757(95)90137-X. [DOI] [PubMed] [Google Scholar]
- 35.Huang ZJ, Dai DZ, Li N, Na T, Ji M, Dai Y. Calcium antagonist property of CPU228, a dofetilide derivative, contributes to its low incidence of torsades de pointes in rabbits. Clin Exp Pharmacol Physiol. 2007;34:310–7. doi: 10.1111/j.1440-1681.2007.04555.x. [DOI] [PubMed] [Google Scholar]
- 36.Prystowsky EN. Effects of bepridil on cardiac electrophysiologic properties. Am J Cardiol. 1992;69:63D–7D. doi: 10.1016/0002-9149(92)90961-w. [DOI] [PubMed] [Google Scholar]
- 37.Britton O, Bueno-Orovio A, Van Ammel K, Lu HR, Towart R, Gallacher D, et al. Experimentally-calibrated population of models predicts and explains inter-subject variability in cardiac cellular electrophysiology. Proc. Natl Acad Sci USA. 2013;110:2098–105. doi: 10.1073/pnas.1304382110. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 38.Walmsley J, Rodriguez JF, Mirams GR, Burrage K, Efimov IR, Rodriguez B. mRNA expression levels in failing human hearts predict cellular electrophysiological remodelling: a population-based simulation study. PLoS ONE. 2013;8:e56359. doi: 10.1371/journal.pone.0056359. doi:10.1371/journal.pone.0056359. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 39.Moreno JD, Clancy CE. Pathophysiology of the cardiac late Na current and its potential as a drug target. J Mol Cell Cardiol. 2012;52:608–19. doi: 10.1016/j.yjmcc.2011.12.003. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 40.Bueno-Orovio A, Hanson B, Gill J, Taggart P, Rodriguez B. In vivo human left-to-right ventricular differences in rate adaptation transiently increase pro-arrhythmic risk following rate acceleration. PLoS ONE. 2012;7:e52234. doi: 10.1371/journal.pone.0052234. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 41.Ramanathan C, Jia P, Ganem R, Ryu K, Rudy Y. Activation and repolarization of the normal heart under complete physiological conditions. Proc Natl Acad Sci USA. 2006;103:6309–14. doi: 10.1073/pnas.0601533103. doi:10.1073/pnas.0601533103. [DOI] [PMC free article] [PubMed] [Google Scholar]