Mechanotransduction Mechanisms for Intraventricular Diastolic Vortex Forces and Myocardial Deformations: Part 1

Abstract

Epigenetic mechanisms are fundamental in cardiac adaptations, remodeling, reverse remodeling, and disease. This 2-article series proposes that variable forces associated with diastolic RV/LV rotatory intraventricular flows can exert physiologically and clinically important, albeit still unappreciated, epigenetic actions influencing functional and morphological cardiac adaptations and/or maladaptations. Taken in-toto, the 2-part survey formulates a new paradigm in which intraventricular diastolic filling vortex-associated forces play a fundamental epigenetic role, and examines how heart cells react to these forces. The objective is to provide a perspective on vortical epigenetic effects, to introduce emerging ideas and suggest directions of multidisciplinary translational research. The main goal is to make pertinent biophysics and cytomechanical dynamic systems concepts accessible to interested translational and clinical cardiologists. I recognize that the diversity of the epigenetic problems can give rise to a diversity of approaches and multifaceted specialized research undertakings. Specificity may dominate the picture. However, I take a contrasting approach. Are there concepts that are central enough that they should be developed in some detail? Broadness competes with specificity. Would however this viewpoint allow for a more encompassing view that may otherwise be lost by generation of fragmented results? Part 1 serves as a general introduction, focusing on background concepts, on intracardiac vortex imaging methods, and on diastolic filling vortex-associated forces acting epigenetically on RV/LV endocardium and myocardium. Part 2 will describe pertinent available pluridisciplinary knowledge/research relating to mechanotransduction mechanisms for intraventricular diastolic vortex forces and myocardial deformations and to their epigenetic actions on myocardial and ventricular function and adaptations.

1. Introduction

Modern imaging modalities allow visualization of intraventricular blood flow structures, including diastolic large-scale vortical flow patterns [1]. The shear and normal forces exerted by these flows over the endocardial surfaces are important components of the internal environment of the myocardium. Despite this and numerous multifaceted intracardiac flow studies, imaging of diastolic vortex variability still finds no use in elucidating myocardial dynamics and adaptations in health, disease and heart failure. And this, in the face of burgeoning appreciation of cellular, molecular, genetic and epigenetic aspects of endocardial and myocardial function [2,3]. This neglect of mechanisms arising out of intracardiac flow considerations applies also to research sponsored by the Human Epigenome Project (HEP) and the International Human Epigenome Consortium (IHEC), which share the ultimate goal of cataloging the human epigenome and uncovering its relation to health and disease [4]. Nonetheless, critical aspects of RV/LV function and adaptations can be recognized only through drawing together intracardiac fluid mechanics with myocardial histomorphology and function, and with molecular genomic and epigenetic studies [1,5].

The objective of this 2-part article is to provide a perspective on these issues and to introduce emerging ideas and directions of multidisciplinary translational research. My goal here is to make pertinent biophysics and cytomechanical dynamic systems concepts accessible to interested translational and clinical cardiologists. I recognize that the diversity of the epigenetic problems can give rise to a diversity of approaches and multifaceted specialized research endeavors. Specificity may dominate the picture. However, I take the opposite approach. Are there concepts that are central enough that they should be developed in some detail? Broadness competes with specificity. Would however this viewpoint allow for a more encompassing view that may otherwise be lost by generation of fragmented results? Part 1 serves as a general introduction, focusing on background concepts, on intracardiac vortex imaging methods, and on diastolic filling vortex-associated forces acting epigenetically on RV/LV endocardium and myocardium. Part 2 will describe pertinent available pluridisciplinary knowledge/research relating to mechanotransduction mechanisms for intraventricular diastolic vortex forces and myocardial deformations and to their epigenetic actions on myocardial and ventricular function and adaptations.

2. Epigenetic forces serve as a bridge between genotype and phenotype

All bodily cells and tissues including the myocardium live in an internal environment, the milieu intérieur, a concept expounded in his posthumously published work “Leçons sur les phénomènes de la vie communs aux animaux et aux végétaux” [6], by the prodigious French physiologist Claude Bernard (1813–78), who also invented cardiac catheterization [1]. Conrad Waddington [7], the professor of animal genetics at the University of Edinburgh, defined epigenetics as “the interactions of genes with their environment which bring the phenotype (from Greek phainein, ‘to show’ + typos, ‘type,’ the composite of an organism’s characteristics, such as its morphology, biochemical, and physiological properties) into being.”

All cells, tissues and organs respond in some way to their environment, in health and disease, and this distinguishes them from inanimate matter. Consider, e.g., why monozygotic twins frequently vary in their susceptibility to diseases although they are basically identical genetically; their epigenomes most likely are not alike. The epigenome comprises all the chemical compounds that have been added to the entirety of one’s DNA (genome) to regulate the activity (expression) of all the genes within the genome. The chemical compounds of the epigenome (see below) are not part of the DNA sequence, but are on or attached to DNA (epi- means ‘above’ or ‘onto’ in Greek). Evidently, even small variations in environmental exposures during pregnancy and beyond can have profound effects on the epigenome and subsequent phenotype, including susceptibility to various abnormalities and to disease.

It is self-evident that the interfaces between bodily organ cells and tissues and their environment must play a crucial role in epigenetics. It is here that special sensory and transduction mechanisms develop that can respond to changing dynamic environmental conditions and forces exerting pervasive influences on the behavior of individual cells, tissues and organs. The stress across a given surface is the force that the material on one side exerts on the material on the other side, divided by the area of the surface Thus, in the case of the ventricular myocardium, it is at the endocardial and myocardial cell membranes and their attachments to cytoskeletal and extracellular matrix (ECM) components that the necessary mechanotransductive “sensory” systems originate. Being sensitive to changes in its environment, involving excessively weakened or intensified dynamic shear (acting parallel to the surface considered) and normal (compressive or tensile) stresses exerted by the flowing intraventricular blood (cf. Table 2), the myocardium of each ventricle undergoes corresponding strains (variably intense deformations) and can react by adapting its phenotype accordingly. In this process lie dangers of transition to maladaptive remodeling results, as occurs in eccentric hypertrophy and dilatation with heart failure [8,9].

Among his numerous anatomical studies in medieval times, Leonardo da Vinci’s sketches identify unmistakably cardiac vortical flow within the sinuses of Valsalva behind the aortic valve cusps [1,10]. Unaware of the circulation, he believed that ejection of blood from the heart, with its attendant eddying motion, “makes in itself great friction which heats and subtilizes the blood, and augments and vivifies the vital spirits which always maintain themselves in warmth and humidity” [11]. Nonetheless, Leonardo observed that, in addition to generating heat, the eddying motion in the sinuses promoted valve closure. In addition, our own recent work [1,8,12–15] has identified the effectiveness of the intraventricular diastolic filling vortices in impounding inflow kinetic energy and preventing an inflow-impeding Bernoulli pressure-rise between the RV/LV inflow orifice and the expanding endocardial surface. Regrettably, these two important actions, namely, promoting competent atrioventricular and semilunar cardiac valve closure and facilitating and boosting diastolic filling, have continued to be the only generally accepted [5] physiologic functions of intracardiac large-scale vortical motions.

3. Bringing together intracardiac fluid mechanics, myocardial mechanotransduction pathways and epigenetics

Cardiac function and adaptations are intrinsically multiscale—small-scale events at the cellular and subcellular level modify macroscopic organ functioning, structure and properties. Indeed, diverse phenomena at the ventricular organ level can be linked to analogous occurrences in cardiomyocytes or isolated papillary muscle [16]. Myocardial cells are continually adapting through activities encompassing gene transcription, protein translation and posttranslational conformation adjusting, as well as the assembly of assorted organelles – see Table 1. Cell–ECM interactions contribute to adaptive closely regulated responses, linking cellular, tissue, and organ phenotype adjustments to alterations in ECM components that serve as monitors of the Bernardian environment.

Table 1.

Epigenetic modes of gene regulation modifying gene expression in response to signals from the internal and external environment.

I. Epigenetic regulation controlling protein synthesis.
1. Pre transcriptional regulation
Chromatin modification
2. Transcriptional regulation: controlling the production of mRNA, rather than its use once produced (e.g., RNA Interference – see below), is a more efficient use of cellular resources
Methylation – methyl group (CH3) added to DNA Histone modification – chemical adjustment of histone proteins in the nucleosome (controls the “density” of DNA packaging in the chromosomes) Transcription factors: proteins or protein complexes that promote or inhibit transcription (gene silencers and enhancers)
3. Editing process in gene regulation: possibly explains why number of human genes is relatively small ≈ 25,000
Alternative RNA splicing: different exons are spliced together to provide diverse polypeptide blueprints
4. Pre translational/post transcription regulation: sensitively controlling the “gain” of the regulated gene expression once mRNA is produced, by reducing translation
RNA Interference: mechanism of decreasing or silencing gene expression after the gene has been transcribed to mRNA (mRNA transport, stability & degradation; control of translation at ribosomes)
5. Post translational regulation: protein activation/deactivation, after protein synthesis
Ubiquitination: adding the protein ubiquitin to an existing protein to instruct the cellular machinery to degrade/destroy the protein Correct protein folding Interconversion between inactive and active states Binding by ligands in allosteric binding sites Availability of accessory molecules (Cofactors or coenzymes)

From morphological adjustments in ventricular remodeling or its reversal [17], to the initiating underlying processes, many cellular myocardial adaptations rely upon coordinated feedbacks between mechanical forces sensed by the cardiac tissues and ensuing biochemical activities. Thus, in deciphering the functions and adaptations of the heart that blend biochemical processes and fluid mechanics of the diastolic RV/LV vortices in health and disease, a comprehensive biochemical description of proteins, enzymatic activities and genes involved, although essential, can furnish only a partial picture. To assume otherwise, would divest myocardial cells of their vital interrelations with their Bernardian environment, which provide a rapid and reversible modulation of the repertoire of expressed genes.

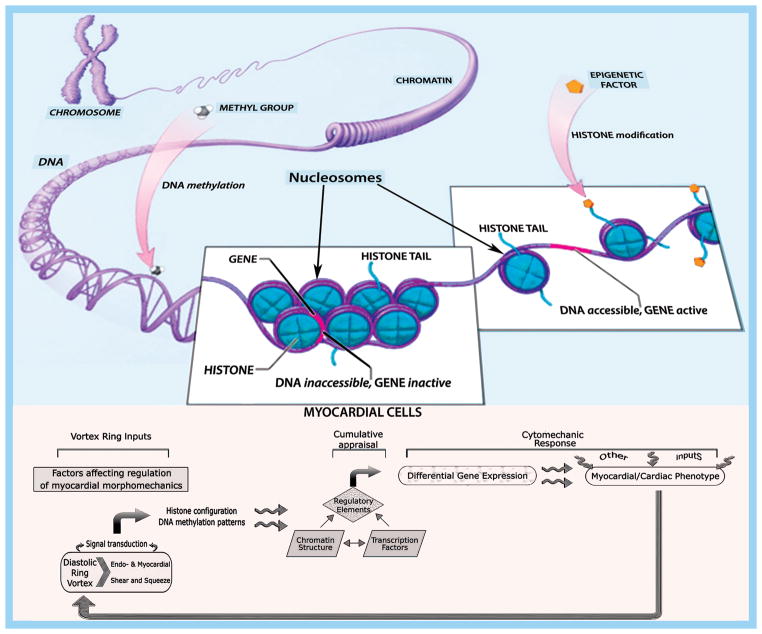
Epigenetics delineates mechanisms of regulation of gene expression generating variable cellular responses to the dynamic environment without involving changes in the underlying DNA nucleotide sequence. That gene mutations can profoundly alter cardiac structure and function, is unequivocal [18,19]. Nonetheless, even knowing the entire sequence of one’s DNA does not suffice to predict cardiac phenotype at all times. To be more precise, it is generally the interacting genes and cell environment together that construct the characteristics of the myocardium and of the pumping heart. Epigenetic functions are mediated by either chemical adjustments of the nuclear chromosomal DNA itself or by alterations in proteins closely associated with the DNA that regulate chromatin packaging (Table 1 and Figure 1). Chromatin is negatively-charged DNA adhering to positively-charged proteins named histones, which package compactly and stably the DNA double helix within the nucleus [20], and to regulatory RNAs involved in the control of genome organization and gene expression [21]; the fundamental subunit of chromatin is the nucleosome (Figure 1, top). Epigenetic modifications involve DNA methylation changes and modifications of chromatin-associated proteins – histones and transcription factor proteins [22]. Such alterations control which genes are turned on or off by binding to DNA and other proteins to promote or block transcription, thus rendering genes more or less active (Table 1). Predictably, the epigenetic state of the genome is modulated by age, gender, nutrition, and disease [23].

Figure 1.

Top: A nucleosome is a section of DNA that is wrapped around a core of positively charged proteins called histones that bind the negatively charged DNA and aid in its packaging. Double-stranded DNA loops around 8 histones twice, forming the nucleosome, which is the building block of chromatin packaging. Chromatin forms chromosomes within the nucleus and exists in two forms: euchromatin, is less condensed and can be transcribed; heterochromatin, is highly condensed and is typically not transcribed. There are many ways that gene expression is controlled. Adding or removing chemical groups to or from histones can alter gene expression; acetylation and phosphorylation make the histones less positively charged because acetyl and phosphoryl groups are negative and their tight hold on DNA becomes looser. Conversely, extensive methylation of cytosine in DNA is correlated with reduced DNA transcription.

Bottom: Endo- and myocardial shear and “squeeze” can impact myocardial and RV/LV morphomechanics by affecting chromatin structure and differential gene expression. See discussion in text.

It is through the action of transcription factors, that the myocardial cells can morph and function differently, e.g., bringing forth eccentric hypertrophy/dilatation or concentric hypertrophy, under a range of normal and abnormal dynamic conditions in life. Hemodynamic forces associated with intracardiac rotatory flows exert important, albeit still unappreciated, epigenetic actions [5]. The time has come to espouse a new paradigm in which diastolic vortex-associated forces play a vital role and to figure out how heart cells react to combined vortical shear and “squeeze” [1,5]. To this end, we must consider findings from various disciplines – imaging modalities, computational fluid dynamics (CFD), and molecular cell biology – whose practitioners are commonly unacquainted with each other’s fields, despite newly emerging multitudinous and profound pluridisciplinary interconnections. We can then place whirling flow patterns of RV and LV filling within a working context that has cytomechanics bearings, to help integrate, focus and steer pertinent pluridisciplinary research. In the process, we should be cognizant that the scientific fields concerned have become so internally heterogeneous and externally interdependent (a move from autonomous specialization toward imbrication), that they do not have uniformly distinctive and distinguishable boundaries. A return to the Aristotelian (or, Leonardian) mode of scientific inquiry has been taking place [1, 1 0]!

4. Digital noninvasive imaging of intraventricular diastolic filling vortex flows

All noninvasive imaging modalities for intracardiac flow are versatile but ineffectual for quantitative detailed study under conditions of pronounced beat-to-beat unsteady vortical pattern variability. Currently, echocardiography and cardiac magnetic resonance (CMR) are applied clinically to non-invasive visualization and analysis of intraventricular vortical blood flow measurements. Real-time three-dimensional (3D) echocardiography [1] color flow imaging is currently under intense development, and may in the future become a valuable, relatively inexpensive tool for the assessment of intracardiac vortical blood flow dynamics.

Intracardiac flow image reconstruction comprises methods that pool information from assorted images in an attempt to obtain features that are not encompassed in any single image. When periodic or quasi-periodic phenomena occur in a flow, as in the formation of diastolic large intraventricular vortices at a given steady physiologic state, and phase information is recoverable from each image, we may phase average a number of images to improve the signal-to-noise ratio (SNR) and promote the emergence of shared patterns, which in distinct frames may be considerably distorted by random fluctuation effects [1]. We can also superimpose overlapping images of different flow field zones to generate images of larger regions. If synchronized images of the same flow pattern are taken concurrently from different orientations, they could be statistically correlated so that 3D representations may be reconstructed, which subsequently may well be viewed from any desired orientation. Similarly, 3D images of vortical spatio-temporal flow patterns can be assembled tomographically, by the correlation of simultaneous images on multiple parallel planes.

4.1 2D Color Doppler echocardiography and vector flow mapping

Color Doppler echocardiography employs arrays of pulsed-wave, multigated Doppler elements, to image intracardiac flows in clinical practice [1,8]. The multigated 2D processor system assigns different colors to local velocities, depending on direction and magnitude. Flow field velocities are synchronously superimposed on the 2D echocardiographic images [24,25], to correlate spatial and temporal evolution. Large intraventricular vortical patterns cannot be imaged easily because of two technical complications [1]: First, high-pass filtering or other rejection algorithms remove the “clutter signal” from adjacent moving heart walls [26]; inadvertently, this also removes the lowest blood velocities. Secondly, at insonation angles close to ± 90°, the Doppler shift frequencies are low and are similarly removed by the clutter filter. These limitations impact Doppler determinations of intraventricular vortex flows, characterized by velocities in many directions and near moving walls.

For more informative images of intraventricular flow structures, vector velocity fields can be generated by postprocessing routine color Doppler velocities [25]. Conventionally, only data received in the direction of the beam are extracted from the Doppler information. Vector Flow Mapping (VFM) is an innovative approach that uses color Doppler velocity data, sound fluid dynamic assumptions, and mathematical techniques to derive intracardiac blood flow distributions in an observation plane, and generates 2D images of the corresponding evolving velocity fields [27–29]. VFM is often able to sketch out the principal structures of intraventricular flows. It estimates mathematically the radial (perpendicular) component of the velocity vector and displays the flow distribution without angle dependency, thus allowing investigators to visualize, measure and analyze various instantaneous quantities of interest from the time-dependent blood flow distribution, such as velocity vectors, flow streamlines and intraventricular vortices [30].

4.2 2D and tomographic 3D particle image velocimetry (PIV)

Echocardiographic PIV is a relatively new method [1], using intravenous contrast agents with strong ultrasound signal backscatter, such as perfluoropropane microbubbles (1–3 μm in diameter), to track intracardiac vortical kinematics [31–33]. It provides sequential-slice 2D images of the velocity vectors on successive scan planes within a region of interest [34–36]. Conceptually, it is based on an optical technique for tracking flow particles and measuring their serial displacement patterns at successive time frames, and thence deriving regional flow velocities. In particle-tracking, we extract the trajectories of the moving point-like particles from a stack of images. Given the locations of the particles in successive images, we must determine which particles in one frame correspond to which particles in a frame taken a small time-interval later. We basically need to match particles at given time t with particles at a time t + d t later, and do this for successive image frames. Cross-correlation software searches for corresponding particle patterns in consecutive image pairs; it then computes implied particle velocities and displays the result. Thus, given a set of particles in a stack of successive flow field images and a correspondence between particles in successive images, it is possible to obtain a set of trajectories that describe the motion of the individual particles and so the flow field.

Tomographic PIV uses tomographic techniques to reconstruct a 3D particle field for volumetric correlation to provide the complete implied particle velocity field within a given measurement volume [37]. This innovative approach can take advantage of a high particle seeding density and can provide relatively high spatiotemporal resolution, contrary to real-time 3D echocardiography which currently has limited spatiotemporal resolution for PIV processing of vortical flow details. Echocardiographic PIV has limitations because relatively long-wavelength ultrasound is used: fast flow regions can cause blurred images; and, ultrasound cannot be focused into a thin enough sheet, so that only particles in that “plane” are in fact imaged.

4.3 Cardiac MRI and phase contrast (PC-MRI) flow velocity imaging

Cardiac MRI is based on imaging of hydrogen nuclei (protons) in a strong magnetic field. Radiofrequency pulses are used to localize their distribution in intracardiac blood and cardiac walls, and signal intensity depends on proton relaxivities [1]. Movement related signal changes are exploited in phase contrast (PC) imaging to measure velocities. A symmetric bipolar magnetic field gradient is applied to tissue slices; in static tissue, the two equal but opposite gradient poles cancel out; however, if a tissue, or blood, is moving between the two, the phase of the tissue/blood gets shifted proportionately to its velocity. By comparing images with and without the applied bipolar gradient, velocity in one direction is computed at each image pixel [1].

The bipolar gradient can be implement along any axis, or combination of axes (x, y, z), in 3D space depending on the direction along which flow velocity is to be determined. During each frame, protons moving at a constant velocity within each voxel of an MRI slice and exposed to a flow-encoding bipolar gradient will accumulate a phase shift proportional to their velocity. PC-MRI can be intuitively extended to higher dimension imaging for intracardiac intricate vortical flow patterns. Specifically, 3D flow data can be easily obtained via PC-MRI by repeating the imaging pulse sequence with a flow encoding gradient applied in each coordinate direction. Since a velocity map in one direction is obtained by subtraction, there are at least 3 measurements required for a 2D dataset, and 4 measurements for a 3D dataset [38].

Despite the slowness of this procedure, the strength of the method is that besides imaging blood flow patterns, quantitative measurements of flow velocities evolving in time in 3D space can be obtained [1]. The phase shift vs. velocity relationship depends on the strength and duration of the flow-encoding gradients. Encoding velocity (V_enc) is the velocity associated with a phase shift of +180° [39]. The range of measurable velocities is ±V_enc; if it exceeds the maximum velocity, ±V_enc, the flow velocity is aliased to a mistakenly low value. It bears noting too that, because of heart motion, an MRI slice position may image different heart sections during the acquisition.

MR pulse sequences can measure in-plane flow (2D velocity encoding, x- and y-directions), or through-plane flow (1-D velocity encoding, z-component) [40,41]. As PC velocimetry requires data acquisition over multiple beats, imaging sequences are ECG-gated, prospectively or retrospectively [1,42], to produce sequential images throughout the R-R interval, yielding cine-MRI. Supplementary respiratory gating or breath-holding effectively suppress respiratory motion artifacts. Direct 3D intracardiac velocity determinations using 3D spatial encoding – as opposed to 3D reconstructions from successive tomographic measurements – are currently making progress [43–47]. Using ECG and respiratory gating, the complete time-resolved, 3-dimensional (3D), and 3-directional blood velocity field can be measured over a volume that encompasses the complete heart and large vessels [40,47]. Such “4D flow MRI” data can expedite considerably scan times and the evaluation of more advanced metrics of intracardiac fluid dynamics that are associated with complex vortical blood flow patterns, including the quantification of vortical velocities, of flow-induced centrifugal forces “squeezing” the myocardium, and of viscous shear stresses exerting traction on the endocardium [1,5].

4.4 Functional Imaging (FI), or numerical flow visualization

The FI method for the investigation of intracardiac blood flows by CFD was developed by our group at the Cardiac Surgical Research Laboratory at Duke University and the Duke/NSF Center for Emerging Cardiovascular Technologies [1,48,49]. It evolved from our method of Predetermined Boundary Motion (PBM), which allows the movement of the inner (endocardial) ventricular surface to drive the flow, and renders itself well to patient-specific simulations and evaluations of intracardiac flow fields [1,50–52].

The PBM and FI methods combine geometric modeling of the cardiac chambers, throughout the cardiac cycle, with CFD. In both methods, the movement of the endocardial chamber boundary is determined independently of the flow, which is generated by and depends on it. Only the resulting intraventricular flow field must be computed, by incorporating the uncoupled wall motion into the CFD. This strategy allows computation of the experimental animal- or patient-specific fluid dynamics, and provides detailed insights into the examined intracardiac flow field. In investigations of intracardiac blood flow phenomena, in particular of the intraventricular diastolic vortices, it is unachievable to extract high spatiotemporal resolution results from various catheter-mounted sensor measurements, or even from the currently available imaging modalities, including live 3D echocardiography and Doppler velocimetry, MRI, and multidetector CT [1]. Accordingly, Functional Imaging – or, numerical flow visualization – represents an invaluable alternative.

A fusion of imaging modalities, e.g., real-time 3D echocardiography with multiaxial sonomicrometry, provides dynamic 3D cardiac chamber contours with high spatiotemporal resolution. Operative sequential geometric chamber shape is derived directly from the endocardial 3D contours and is combined with the effectively instantaneous sonomicrometric measurements, allowing detailed individualized intraventricular flow simulations [1,8,12–15,49]. FI can reveal detailed quantitative flow patterns in the hearts of individual experimental animals and human subjects. It gives values of the flow variables at literally thousands of discrete points in space and time [1,49]. From this high-density information, using modern visualization software for building and deploying data displays, we can extract informative graphic visual representations, or snapshots, of spatiotemporal vortical patterns, instantaneous velocities, pressures, and shear [1,5,8,12–15,48,49]. These can improve cognition by exploiting images to enhance the human visual system’s ability to see patterns and trends.

5. Variable diastolic filling vortex-associated forces on RV/LV endocardium and myocardium

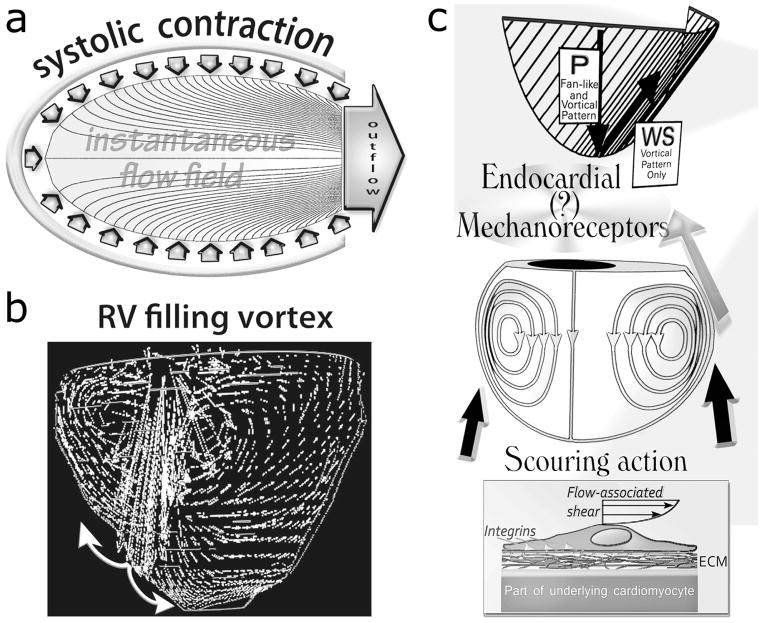
Given the “no-slip” condition for viscous flow at a solid-fluid interface, the intraventricular ejection flow field streamlines take perpendicular origin from the contracting endocardial surface [1,18,19,50,51]. Accordingly, there is no shear (tangential) endocardial stress during ejection, as is shown in Figure 2, a. Endocardial shear comes about upon transition, early during diastolic filling, from unstable fan-like flow to vortical rotatory intraventricular flow (Figure 2, b). Diastolic vortical flow patterns within the cardiac ventricles were first studied [1,5] by Bellhouse [53] and Taylor and Wade [54].

Figure 2.

a. Satisfying the viscous flow “no-slip” condition at a solid-fluid interface, the intraventricular ejection flow field streamlines are perpendicular to the contracting endocardial surface; consequently, there is no shear (tangential) endocardial stress during ejection.

b. RV/LV diastolic flow patterns. Fortuitously, flow-governing fluid dynamic principles do not allow inflow to mirror outflow streamlines throughout the ensuing filling phase. During filling, there ensues flow separation and formation of a toroidal vortex that surrounds a central jet. Thus, laminar vortical shear and “squeeze” forces can come into existence. The incoming jet strikes the vicinity of the ventricular apex and is surrounded by the toroidal vortex whose main strength is within the outflow tract of each chamber.

c. Rotation in the intraventricular blood stream naturally encourages a more or less vigorous scouring (tractive shear and torque) of the endocardium lining the RV/LV chamber and causes centrifugal “squeeze” forces to come into existence; see discussion in text and Figure 3. P, pressure: acts perpendicularly; WS, wall shear: acts tangentially on the endocardial surface. (Adapted, in part, from Pasipoularides et al. [12], by kind permission of the American Physiological Society.)

Normal diastolic intraventricular vortical patterns and their alterations in heart disease or following surgical procedures, including prosthetic atrioventricular valve implantation and ventricular reconstruction, have been established in numerous studies [1,5,8,12–15,24,33,55–61]. Blood circulates in the toroidal (ring-shaped) vortex such that there results a jet through the torus center and blood is contra-rotating on cross-sections astride this central jet – cf. Figure 2, b and c. The blood is rotating poloidally [15]; this poloidal rotation pattern is akin to that of the magnetic field in a magnet, which flows from the north pole to the south pole; hence, the name. The ensuing vortical hydrodynamic effects (see Table 2) and some of their cytomechanical consequences have been recently surveyed [1,5].

Table 2.

Mechanical effects of the intraventricular RV-LV filling vortex.

1. Shear forces appear because the velocity of the vortical flow is different on neighboring streamlines. A velocity gradient exists perpendicular to the streamlines and the effect of viscosity manifests itself in a shear stress, force per unit area, proportional to the dynamic viscosity and to the velocity gradient, the velocity difference over a unit distance perpendicular to the streamlines.

A shear stress will also appear if turbulent, unsteady flow fluctuations are present that transfer energy between the streamlines. The turbulent shear stress will also be proportional to the velocity gradient, but the constant of proportionality will not be a material constant but will depend on the local intensity of turbulent fluctuations. Shear forces become important only when high-velocity gradients exist perpendicular to the streamlines near the endocardial surfaces – the fluid at the endocardial boundaries does not move, the “no slip” condition; the shear stress is highest at the endocardial surface.

2. A centrifugal force always acts perpendicular to the axis of rotation of the whirling vortical blood and increases linearly with the distance from the axis and quadratically with the angular velocity. Against this centrifugal force an equally large and opposite centripetal force must act to maintain equilibrium. The centripetal force is provided by the myocardial wall elements.

The pressure in the vortex increases with the distance from the center of rotation. The vortical centrifugal force pushes onto the curved endocardial surface, giving rise not only to compression but also to stretching (tension) forces because, by the Poisson effect, when a material is compressed in one direction, it usually tends to expand in the other two directions perpendicular to the direction of compression. Under the action of the coupled centripetal and centrifugal forces the endocardium and other RV/LV wall components get “squeezed.” There is a (quasi) periodic, rotating wave action of compression-decompression: a gyratory “milking” action.

3. Mixing processes are strongly enhanced by advection, the movement of any material dissolved or suspended in the rotating vortical blood.

Effective diffusional processes are greatly accelerated; material that is near the endocardial surface goes into the bulk blood phase and vice versa, thereby eliminating or reducing any slowdown due to diminishing concentration gradients.

5.1 Endocardial vortex-associated shear stress

Any real fluid moving along a solid boundary will incur a tangential shear stress on that boundary. For all Newtonian fluids (blood included at high shear rates) in laminar flow, shear stress is proportional to the strain rate in the fluid, and the viscosity is the constant of proportionality [1]. Considering the diastolic intraventricular filling vortex, the so-called no-slip condition dictates that the speed of the fluid at the boundary (relative to the boundary) is zero, but at some height from the endocardial boundary the flow speed must equal the curved-path (tangential to the RV/LV endocardial boundary surfaces) velocity of the rotating blood, denoted by C (for curved). The region between these two points is aptly named the boundary layer [1,51]. The tractive shear stress is imparted onto the boundary as a result of this loss of velocity. The shear stress exerted on the endocardial surfaces by the rotating blood, τ_e, is given by: ${\tau }_e=\mu \frac{dC}{dr}|$, where μ is blood’s viscosity, r is the radial distance (height) above the boundary, and $\frac{dC}{dr}|$ is the strain rate of the fluid at the endocardial surface.

As is shown in Figure 2, b, where the incoming jet strikes the RV/LV apex, flow is brought to rest (stagnation region), and $\frac{dC}{dr}|$ and the shear stress, τ_e, between blood and endocardium is low. With distance from the stagnation region, velocities near the wall and wall shear rise rapidly. As indicated in Figure 2, b, FI simulations show that the stagnation “point” swerves around from beat to beat, thus not keeping fixed the non-sheared area [1,5,12–15], a behavior typical of nonlinear systems that, generally, are exquisitely sensitive to even slight random disturbances [1]. Since the asymmetric vortex typically grows stronger in the outflow tract, this area is scoured most vigorously in both ventricles (Figure 2, b and c). FI computer simulations [1,5,12–15] indicate that the vortex-associated endocardial shear stresses can attain ~ 4 0 dynes/cm², or 4 Pa; as we shall see in Part 2 of this article series, such shear levels are by no means inconsequential. Normal and abnormal vortex-generated diastolic endocardial shear levels can thus be expected to play important, albeit generally unrecognized, roles in myocardial function and adaptations (Figure 3, top), and should not continue to be disregarded as likely epigenetic factors influencing cardiac adaptations, maladaptations and disease [5].

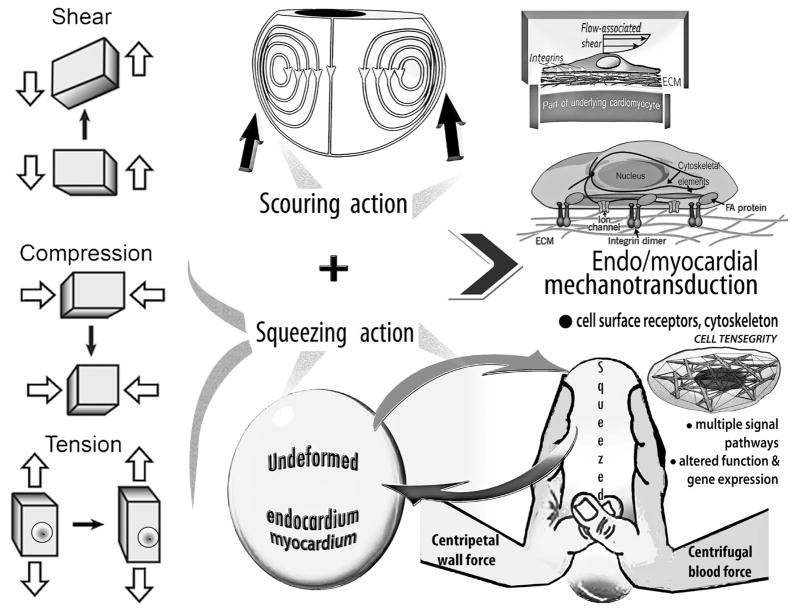
Figure 3.

Top: Rotational large-scale vortical motion in the intraventricular flow field can generate a more or less vigorous scouring (tractive shear and torque) of the endocardium lining the chamber.

Bottom: Under the action of centripetal and centrifugal forces associated with RV/LV rotatory diastolic flow, the endocardium and other wall components get deformed, akin to a ball squeezed between one’s palms. This dynamic interplay and its disturbances are likely to have intriguing epigenetic actions affecting cardiac function, adaptations, and abnormalities acting concurrently with the vortex-induced shear (Top), as summarized in the Middle.

Middle: Myocardial cells (endocardium, myocytes, and fibroblasts) can move, change shape, and switch genes on and off in response to changes in hydrodynamic shear and “squeeze” (see discussion in text). They sense their physical 3D Bernardian “environment,” including variable diastolic vortical shear and “squeeze” forces, by transducing mechanical deformations and forces into differentiated transcription and translation signals, which can adjust cellular and extracellular tissue and organ structure. Mechanosensitive controls modulate myocyte shape and intracellular architecture, and processes as diverse as concentric and eccentric hypertrophy, remodeling and apoptosis, involved in cardiac homeostasis and adaptations, and also in maladaptive responses and disease.

5.2 Endocardiomyocardial vortex-associated centrifugal-centripetal “squeeze”

In absence of accelerating forces, objects do not naturally follow circular trajectories. By Newton’s 2^nd Law, there is a centripetal (from Latin centrum, meaning “center” and petere, meaning “to seek”) force exerted on the rotating blood in the diastolic vortex by the RV/LV walls [1,5]. It imparts the centripetal acceleration. By his 3^rd Law, there is also an equal and opposite centrifugal (from Latin centrum “center”, and fugere “to flee”) force exerted by the rotating blood on the walls; its inertia yields this centrifugal effect, since continued motion in a straight line in absence of the walls would tend to carry it away from the instantaneous axis of its rotation. You can feel the centrifugal acceleration toward the outside of the curved path of your car when you go around a corner. The faster you go, the greater is this outward acceleration; moreover, the sharper the curve (smaller radius), the greater is this outward acceleration and the corresponding centrifugal force. Centripetal and centrifugal acceleration are instantaneous accelerations considering that their direction is constantly changing.

A flow on a curved path is always coupled to pressure gradients perpendicular to the flow direction, such that the pressure decreases from the outside to the inside toward the momentary center of the streamline curvature. This pressure differential provides the centripetal force which makes flow on a curved path possible in the first place, by keeping the body force (centrifugal force) acting on the rotating blood at equilibrium. Letting C represent the curved-path (tangential) velocity and r t he radius of curvature of the curved path, the centrifugal acceleration per unit mass of rotating blood is C²/r. The corresponding centripetal force per unit volume of blood is none other than the radial pressure gradient: $\frac{dp}{dr}=\frac{\rho C^2}{r}$, where ρ denotes the mass density of blood. The range of applicability of this equation excludes the center of the streamline curvature (r = 0) and its immediate vicinity.

The preceding is a simple but extremely important equation, for our present purposes: it shows the quadratic dependence of the centripetal and the centrifugal force on the applying curved-path (tangential) velocity, and its proportionality to the reciprocal of r, the radius of curvature of the curved path. We see that reducing vortex strength and the curved-path velocity by, say, a factor of 3 in a dilated (increased effective r) RV/LV chamber can diminish the centrifugal force and the “squeeze” exerted by the rotating blood on the endocardium and myocardium (Figure 3, middle and bottom) by roughly a factor of 12–14 ! FI computer simulations [1,5,12–15] indicate that the vortex-associated centrifugal force squeeze on the endocardial surface normally amounts to a radial compression of a few mm Hg. The situation is somewhat analogous to the case exemplified by the pill rolling around the circular housing of a roulette “wheel.” The rotatable pill exerts a centrifugal force on the housing, balancing the centripetal force exerted on it by the housing.

The vortical centrifugal force pushes onto the curved endocardial surface, giving rise not only to compression but also to stretching (tension) forces because, by the Poisson effect, when a material is compressed in one direction, it usually tends to expand in the other two directions perpendicular to the direction of compression, as is shown in Figure 3, left middle & bottom. Interestingly, recent investigations on rabbit hearts [62] have shown that stretch is a primary trigger for the induction of connective tissue growth factor (CTGF/CCN2) mRNA expression in cardiomyocytes, indicating that stretch-induced deformations of the cardiomyocytes contribute importantly to the sustained upregulation of CTGF in myocardial eccentric hypertrophy.

Under the action of the coupled centripetal and centrifugal forces the endocardium and other RV/LV wall components get deformed [1,5,15], akin to a ball squeezed between the palms of one’s hands (Figure 3, right bottom). This dynamic interplay and its disturbances are more than likely to have intriguing epigenetic actions affecting cardiac function and adaptations [1,5], acting concurrently with the vortex-induced shear stresses. Furthermore, adverse repercussions could also accrue from diminished or absent “milking-action” of cyclic diastolic vortical squeeze on intramural coronary blood and lymphatic vascular components [1,5].

6. Variable vortex forces’ intensities impact myocardial cytomechanics and adaptations

Individual myocardial cells both respond to externally applied mechanical forces and also generate internal forces that are transmitted to adjacent cells and the ECM. The endomysial connective tissue matrix surrounding the sarcolemma of the cardiomyocytes is essential for the transmission of forces both in amount and in direction [63]. This obviously applies to the transmission of vortical shear (traction and torque) and squeeze (compression and elongation) forces to individual cardiomyocytes within their fibrous bed, across the myocardial walls. Mechanotransduction in ventricular myocardium not only regulates cardiac beat-to-beat performance but also affects strongly sarcomere growth patterns, hypertrophy, adhesiveness and survival of the cardiomyocytes, and myocardial histoarchitectonics. Myocardial cells (endocardium, cardiomyocytes, and fibroblasts) must implement subtle or major morphomechanical adaptations to tune RV/LV pump function to varying operating conditions [1,64–66]. Relying on mechanosensing and mechanotransduction [67], heart cells sense not only biochemical but also physical regulatory stimuli, such as transmural pressure and diverse mural stresses, to modulate ventricular function, DNA transcription and translation, and adaptive remodeling [2,5]. Nonetheless, intracardiac hydrodynamic regulatory epigenetic mechanisms, such as shear and centrifugal effects, are not appreciated as factors in ventricular adaptations and remodeling in health and disease, although Murray’s law of optimal blood vessel sizing to maintain constant endothelial shear stress levels is generally known [1].

Mechanisms analogous to Murray’s law are likely to couple endocardial vortical shear and “squeeze” to RV/LV morphomechanical adaptations and abnormalities. To advantageously exploit diastolic vortex forces as signals leading to adjustments of tissue morphomechanics, myocardial cells must sense different types of stress and associated strains, namely, shear, compression, and tension. In fact, myocardial cells can move, change shape, and switch genes on-or-off in response to changes in hydrodynamic shear and squeeze [1,5,15]. Thus, abnormally elevated filling vortex strength in a new-onset volume overload could switch on genes and proteomic processes, culminating in RV/LV enlargement by myofibril sarcomere replication in-series, tending to reduce the vortex strength [1,5,15]. If this negative feedback process overshoots to excessive chamber enlargement, the ensuing subnormal diastolic vortex strength levels [8, 1 5] might contribute to intramural “milking-action” disturbances (cf. Figure 3, right bottom) and transition to disease.

Cytomechanics encompasses, among others, structural dynamics of the cytoskeleton and the ECM, mechanotransduction and signaling, and mechanical epigenetic influences on genetic expression. An example of the latter is hydrodynamic shear on the endocardial lining of the developing heart, shown to underlie splendid adaptations of form to function during cardiogenesis [1,68,69]. The endocardium regulates mural myocardial cell disposition in the developing heart; it provokes critical transitions in cardiomyocyte movements and dictates the angular direction of cardiomyocytes within the growing walls [70]. During cardiogenesis, therefore, endocardial shear links the sinuous flow within the developing heart and the spiraling, intertwining myocardial fibers and surfaces in its forming walls [1,5].

Compared to the endothelial, the endocardial [2,3] cytomechanics literature is quite underdeveloped. On vascular cytomechanics there exists a vast literature and, although it refers to vascular cells, there are significant structural homologies (as endothelial and endocardial cells share a common origin in their embryological development) and activity–function analogies [71, 72] with myocardial structures and interactions. Modulation of cardiomyocyte contraction and relaxation by the endocardium resembles modulation of myocardial contraction and relaxation by the coronary endothelial lining, and the effects are additive [73]! We can consequently draw upon ontogenetically parallel aspects of vascular cytomechanics adaptations to hydrodynamic forces, to aid consideration of myocardial cytomechanics as they pertain to vortical shear and squeeze effects.

Part 2 of this article series will consider, among others, structural dynamics of myocardial cells (endocardium, myocytes, and fibroblasts), cytoskeleton, nucleoskeleton, and extracellular matrix, mechanotransduction and signaling, and mechanical epigenetic influences of the diastolic vortex on genetic expression. This new germinal frontier in contemporary cardiac research should yield versatile mechanistic insights linking filling vortex patterns and attendant forces to variable expressions of gene regulation in RV/LV myocardium. In due course, it should characterize subtle intrinsic homeostatic arrangements that support ventricular myocardial function and adaptability and, in addition, unappreciated aspects of maladaptive morphomechanical myocardial changes.